Sequences of Groups, Hypergroups and Automata of Linear Ordinary Differential Operators
Abstract
1. Introduction
2. Sequences of Groups and Hypergroups: Definitions and Theorems
2.1. Notation and Preliminaries
2.2. Results
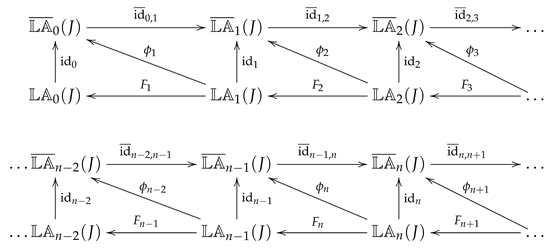
3. Automata and Related Concepts
3.1. Notation and Preliminaries
3.2. Results
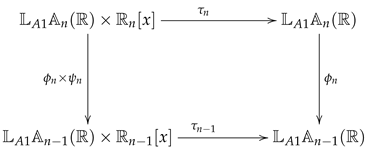
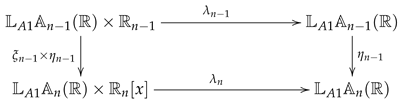
4. Practical Applications of the Sequences
4.1. Cascades of Neurons Determined by Right Translations
- for any neuron .
- for any integers and any artificial neuron . Notice that the just obtained structure is called a cascade within the framework of the dynamical system theory.
4.2. An Additive Group of Differential Neurons
4.3. A Cyclic Subgroup of the Group Generated by Neuron
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Neuman, F. Global Properties of Linear Ordinary Differential Equations; Academia Praha-Kluwer Academic Publishers: Dordrecht, The Netherlands; Boston, MA, USA; London, UK, 1991. [Google Scholar]
- Chvalina, J.; Chvalinová, L. Modelling of join spaces by n-th order linear ordinary differential operators. In Proceedings of the Fourth International Conference APLIMAT 2005, Bratislava, Slovakia, 1–4 February 2005; Slovak University of Technology: Bratislava, Slovakia, 2005; pp. 279–284. [Google Scholar]
- Chvalina, J.; Moučka, J. Actions of join spaces of continuous functions on hypergroups of second-order linear differential operators. In Proc. 6th Math. Workshop with International Attendance, FCI Univ. of Tech. Brno 2007. [CD-ROM]. Available online: https://math.fce.vutbr.cz/pribyl/workshop_2007/prispevky/ChvalinaMoucka.pdf (accessed on 4 February 2021).
- Chvalina, J.; Novák, M. Laplace-type transformation of a centralizer semihypergroup of Volterra integral operators with translation kernel. In XXIV International Colloquium on the Acquisition Process Management; University of Defense: Brno, Czech Republic, 2006. [Google Scholar]
- Chvalina, J.; Křehlík, Š.; Novák, M. Cartesian composition and the problem of generalising the MAC condition to quasi-multiautomata. Analele Universitatii “Ovidius" Constanta-Seria Matematica 2016, 24, 79–100. [Google Scholar] [CrossRef]
- Křehlík, Š.; Vyroubalová, J. The Symmetry of Lower and Upper Approximations, Determined by a Cyclic Hypergroup, Applicable in Control Theory. Symmetry 2020, 12, 54. [Google Scholar] [CrossRef]
- Novák, M.; Křehlík, Š. EL–hyperstructures revisited. Soft Comput. 2018, 22, 7269–7280. [Google Scholar] [CrossRef]
- Novák, M.; Křehlík, Š.; Staněk, D. n-ary Cartesian composition of automata. Soft Comput. 2020, 24, 1837–1849. [Google Scholar]
- Cesarano, C. A Note on Bi-orthogonal Polynomials and Functions. Fluids 2020, 5, 105. [Google Scholar] [CrossRef]
- Cesarano, C. Multi-dimensional Chebyshev polynomials: A non-conventional approach. Commun. Appl. Ind. Math. 2019, 10, 1–19. [Google Scholar] [CrossRef]
- Chvalina, J.; Smetana, B. Series of Semihypergroups of Time-Varying Artificial Neurons and Related Hyperstructures. Symmetry 2019, 11, 927. [Google Scholar] [CrossRef]
- Jan, J. Digital Signal Filtering, Analysis and Restoration; IEEE Publishing: London, UK, 2000. [Google Scholar]
- Koudelka, V.; Raida, Z.; Tobola, P. Simple electromagnetic modeling of small airplanes: Neural network approach. Radioengineering 2009, 18, 38–41. [Google Scholar]
- Krenker, A.; Bešter, J.; Kos, A. Introduction to the artificial neural networks. In Artificial Neural Networks: Methodological Advances and Biomedical Applications; Suzuki, K., Ed.; InTech: Rijeka, Croatia, 2011; pp. 3–18. [Google Scholar]
- Raida, Z.; Lukeš, Z.; Otevřel, V. Modeling broadband microwave structures by artificial neural networks. Radioengineering 2004, 13, 3–11. [Google Scholar]
- Srivastava, N.; Hinton, G.; Krizhevsky, A.; Sutskever, I.; Salakhutdinov, R. Dropout: A simple way to prevent neural networks from overfitting. J. Mach. Learn. Res. 2014, 15, 1929–1958. [Google Scholar]
- Neuman, F. Global theory of ordinary linear homogeneous differential equations in the ral domain. Aequationes Math. 1987, 34, 1–22. [Google Scholar] [CrossRef]
- Křehlík, Š.; Novák, M. From lattices to Hv–matrices. An. Şt. Univ. Ovidius Constanţa 2016, 24, 209–222. [Google Scholar] [CrossRef]
- Novák, M. On EL-semihypergroups. Eur. J. Comb. 2015, 44, 274–286. [Google Scholar] [CrossRef]
- Novák, M. Some basic properties of EL-hyperstructures. Eur. J. Comb. 2013, 34, 446–459. [Google Scholar] [CrossRef]
- Bavel, Z. The source as a tool in automata. Inf. Control 1971, 18, 140–155. [Google Scholar] [CrossRef][Green Version]
- Dörfler, W. The cartesian composition of automata. Math. Syst. Theory 1978, 11, 239–257. [Google Scholar] [CrossRef]
- Gécseg, F.; Peák, I. Algebraic Theory of Automata; Akadémia Kiadó: Budapest, Hungary, 1972. [Google Scholar]
- Massouros, G.G. Hypercompositional structures in the theory of languages and automata. An. Şt. Univ. A.I. Çuza Iaşi, Sect. Inform. 1994, III, 65–73. [Google Scholar]
- Massouros, G.G.; Mittas, J.D. Languages, Automata and Hypercompositional Structures. In Proceedings of the 4th International Congress on Algebraic Hyperstructures and Applications, Xanthi, Greece, 27–30 June 1990. [Google Scholar]
- Massouros, C.; Massouros, G. Hypercompositional Algebra, Computer Science and Geometry. Mathematics 2020, 8, 138. [Google Scholar] [CrossRef]
- Křehlík, Š. n-Ary Cartesian Composition of Multiautomata with Internal Link for Autonomous Control of Lane Shifting. Mathematics 2020, 8, 835. [Google Scholar] [CrossRef]
- Borůvka, O. Lineare Differentialtransformationen 2. Ordnung; VEB Deutscher Verlager der Wissenschaften: Berlin, Germany, 1967. [Google Scholar]
- Borůvka, O. Linear Differential Transformations of the Second Order; The English University Press: London, UK, 1971. [Google Scholar]
- Borůvka, O. Foundations of the Theory of Groupoids and Groups; VEB Deutscher Verlager der Wissenschaften: Berlin, Germany, 1974. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chvalina, J.; Novák, M.; Smetana, B.; Staněk, D. Sequences of Groups, Hypergroups and Automata of Linear Ordinary Differential Operators. Mathematics 2021, 9, 319. https://doi.org/10.3390/math9040319
Chvalina J, Novák M, Smetana B, Staněk D. Sequences of Groups, Hypergroups and Automata of Linear Ordinary Differential Operators. Mathematics. 2021; 9(4):319. https://doi.org/10.3390/math9040319
Chicago/Turabian StyleChvalina, Jan, Michal Novák, Bedřich Smetana, and David Staněk. 2021. "Sequences of Groups, Hypergroups and Automata of Linear Ordinary Differential Operators" Mathematics 9, no. 4: 319. https://doi.org/10.3390/math9040319
APA StyleChvalina, J., Novák, M., Smetana, B., & Staněk, D. (2021). Sequences of Groups, Hypergroups and Automata of Linear Ordinary Differential Operators. Mathematics, 9(4), 319. https://doi.org/10.3390/math9040319





