Reachable Set and Robust Mixed Performance of Uncertain Discrete Systems with Interval Time-Varying Delay and Linear Fractional Perturbations
Abstract
:1. Introduction
- (1)
- Reachable set estimation and mixed / performance for an uncertain discrete system with interval time-varying delay and linear fractional perturbations are considered in this paper.
- (2)
- A new improved analytic result is proposed based on the approach developed in [4]. Less conservative results for an uncertain discrete system with slow variation interval time-varying delay are provided for more accurate estimation of the reachable set. The / performance can also be guaranteed from the design scheme.
- (3)
- The LMI optimization approach is used to guarantee the minimization of the reachable set and achievement of mixed performance of the system under consideration. The proposed conditions can be solved easily by the Matlab LMI toolbox.
2. Problem Formulation and Mixed Performance Analysis
- (i)
- With , the system (1) with (2) and (7) is asymptotically stable, and we can find an to satsify the inequality
- (ii)
- With zero initial conditions (i.e., ,the signals and are bounded by
- (I)
- The following inequality is satisfied:
- (II)
- There exists a scalar such that
3. Main Results
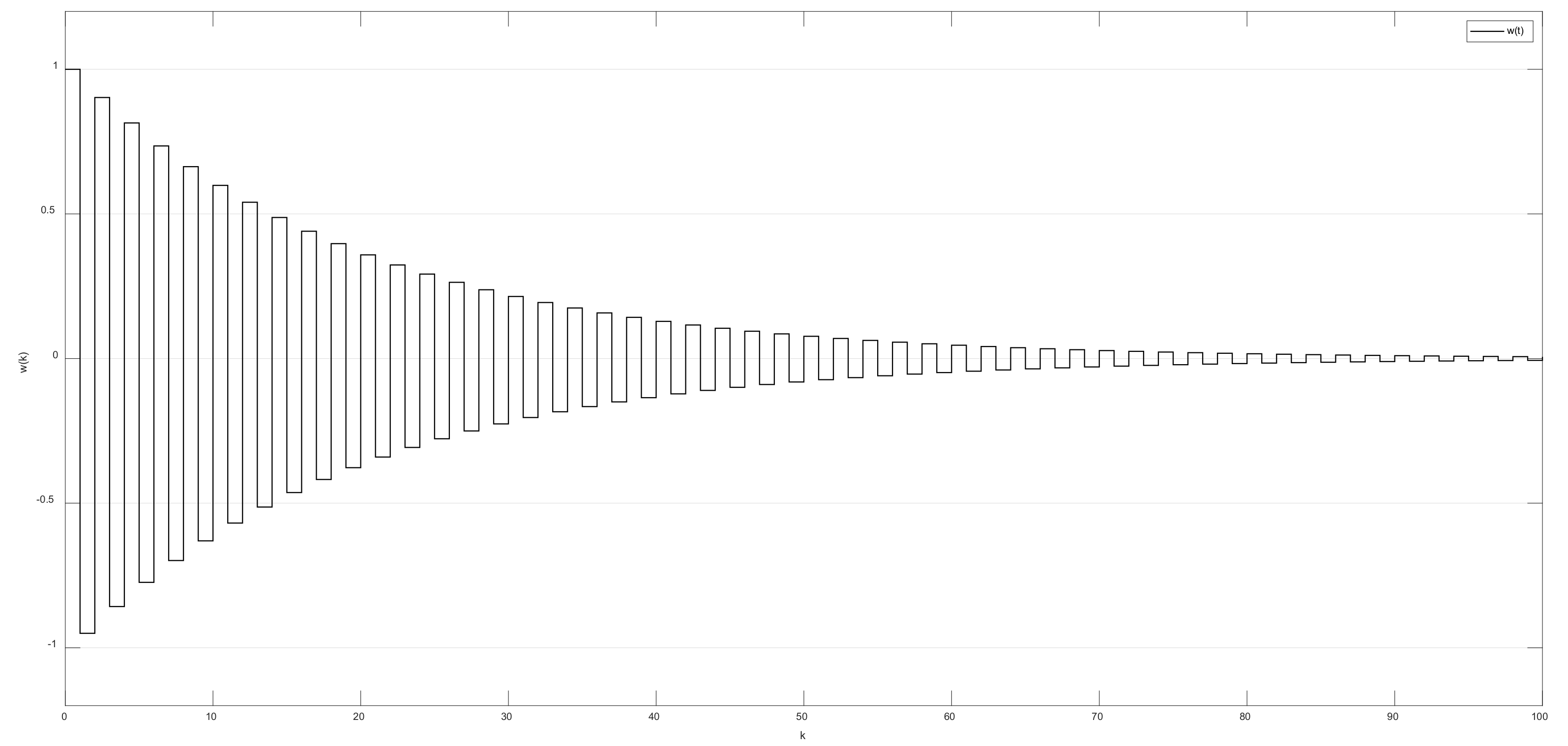
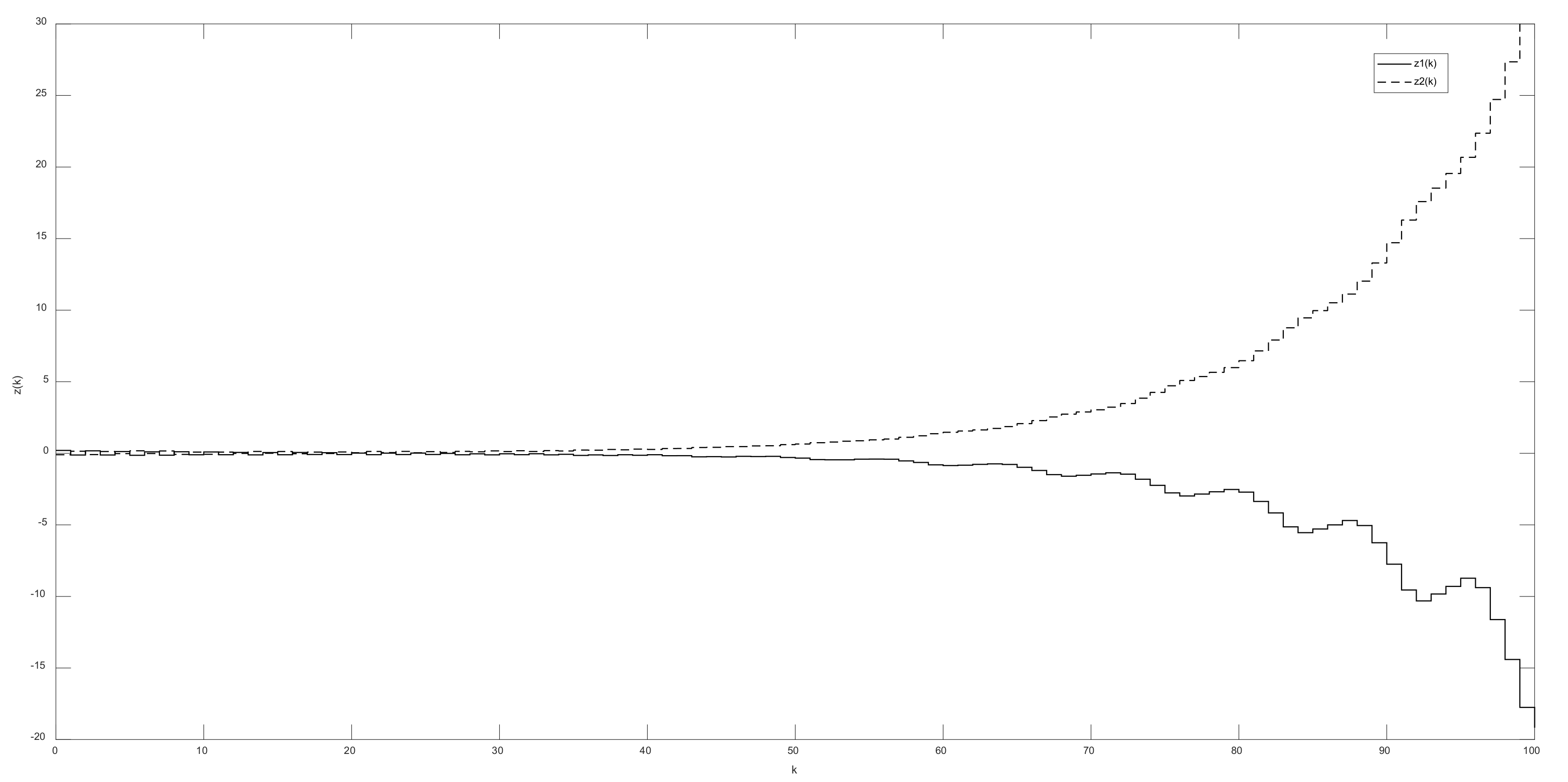
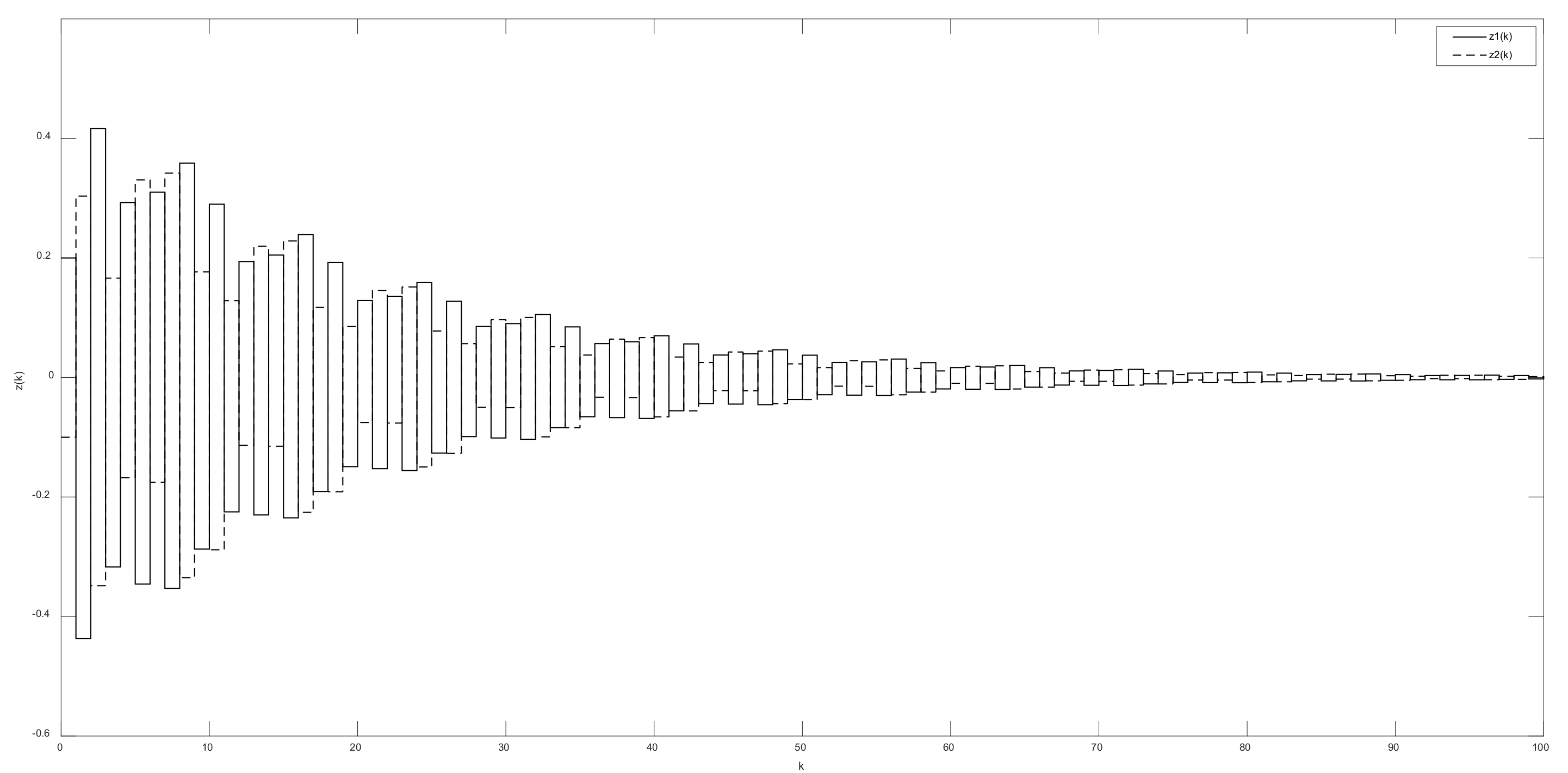
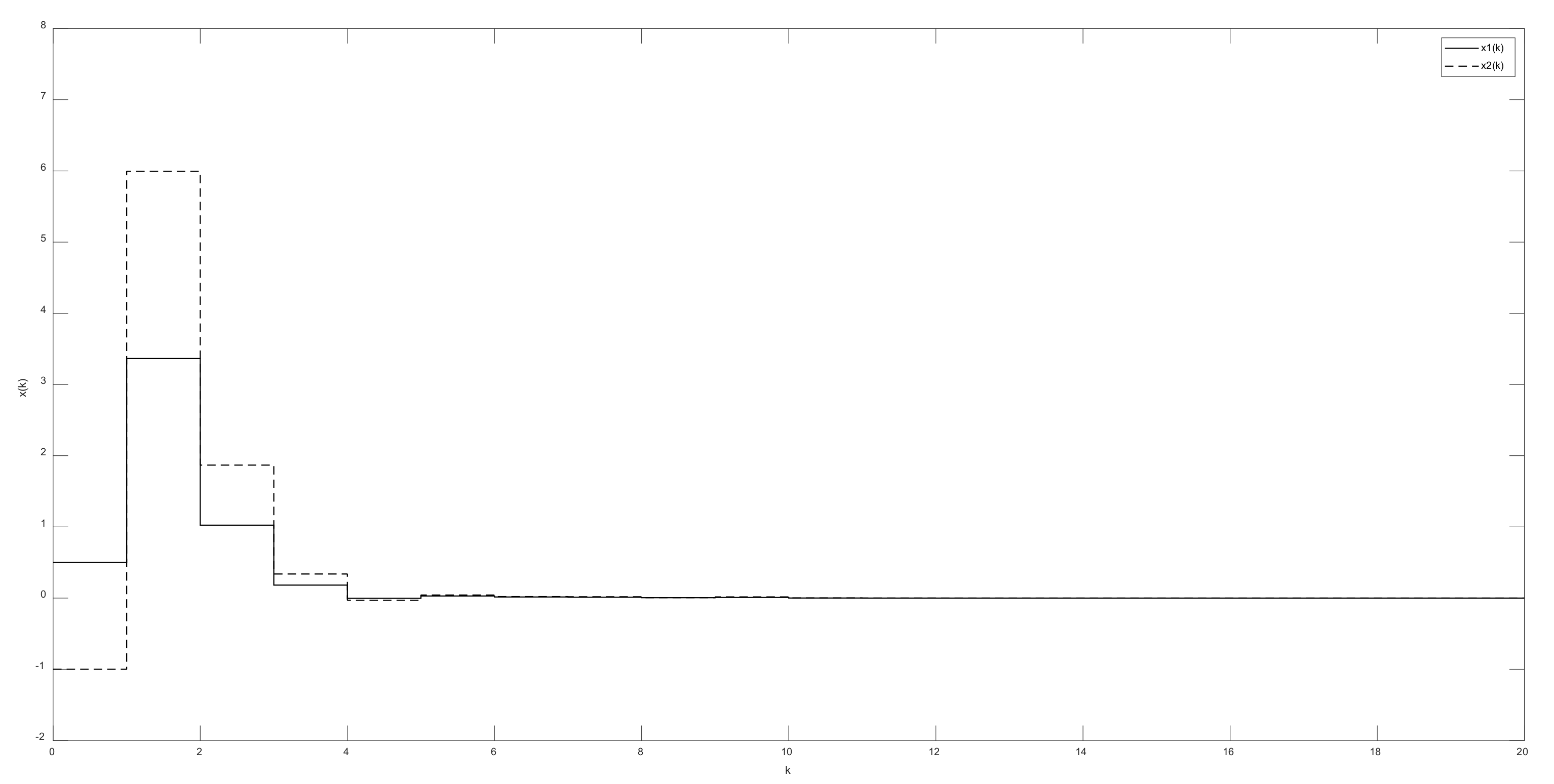
4. Illustrative Examples
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gu, K.; Kharitonov, V.L.; Chen, J. Stability of Time-Delay Systems; Birkhauser: Boston, MA, USA, 2003. [Google Scholar]
- Hale, J.K.; Verduyn Lunel, S.M. Introduction to Functional Differential Equations; Springer: New York, NY, USA, 1993. [Google Scholar]
- Kolmanovskii, V.B.; Myshkis, A. Introduction to the Theory and Applications of Functional Differential Equations; Kluwer Academic Publishs: Dordrecht, The Netherlands, 1999. [Google Scholar]
- Chen, Y.; Lam, J.; Cui, Y.; Kwok, K.W. Switched systems approach to state bounding for time delay systems. Inf. Sci. 2018, 465, 191–201. [Google Scholar] [CrossRef]
- Lien, C.H.; Yu, K.W.; Lin, Y.F.; Chung, Y.J.; Chung, L.Y.; Chen, J.D. Exponential stability analysis for uncertain switched neutral systems with interval time-varying state delay. Nonlinear Anal. Hybrid Syst. 2009, 3, 334–342. [Google Scholar] [CrossRef]
- That, N.D.; Nam, P.T.; Ha, Q.P. Reachable set bounding for linear discrete-time systems with delays and bounded disturbances. J. Optim. Theory Appl. 2013, 157, 96–107. [Google Scholar] [CrossRef]
- Tunç, C.; Tunç, O.; Wang, Y.; Yao, J.C. Qualitative analyses of differential systems with time-varying delays via Lyapunov–Krasovski approach. Mathematics 2021, 9, 1196. [Google Scholar] [CrossRef]
- Mahmoud, M.S. Switched Time-Delay Systems; Springer: Boston, MA, USA, 2010. [Google Scholar]
- Sun, Z.; Ge, S.S. Stability Theory of Switched Dynamical Systems; Springer: London, UK, 2011. [Google Scholar]
- Baldi, S.; Xiang, W. Reachable set estimation for switched linear systems with dwell-time switching. Nonlinear Anal. Hybrid Syst. 2018, 29, 20–33. [Google Scholar] [CrossRef] [Green Version]
- Chen, G.; Xiang, G.; Karimi, H.R. Observer-based robust H∞ control for switched stochastic systems with time-varying delay. Abstr. Appl. Anal. 2013, 2013, 320703. [Google Scholar] [CrossRef]
- Chen, Y.; Lam, J.; Zhang, B. Estimation and synthesis of reachable set for switched linear systems. Automatica 2016, 63, 122–132. [Google Scholar] [CrossRef]
- Lien, C.H.; Yu, K.W.; Hsieh, J.G.; Chung, L.Y.; Chen, J.D. Simple switching signal design for H∞ performance and control of switched time-delay systems. Nonlinear Anal. Hybrid Syst. 2018, 29, 203–220. [Google Scholar] [CrossRef]
- Sun, X.M.; Wang, W.; Liu, G.P.; Zhao, J. Stability analysis for linear switched systems with time-varying delay. IEEE Trans. Syst. Man Cybern. B 2008, 38, 528–533. [Google Scholar]
- Yu, K.W.; Lien, C.H.; Chang, H.C. H∞ analysis and switching control for uncertain discrete switched time-delay systems by discrete Wirtinger inequality. Adv. Differ. Equ. 2017, 2017, 349. [Google Scholar] [CrossRef] [Green Version]
- Chen, H.; Cheng, J.; Zhong, S.; Yang, J.; Kang, W. Improved results on reachable set bounding for linear systems with discrete and distributed delays. Adv. Differ. Equ. 2015, 2015, 145. [Google Scholar] [CrossRef] [Green Version]
- Fridman, E.; Shaked, U. On reachable sets for linear systems with delay and bounded peak inputs. Automatica 2003, 39, 2005–2010. [Google Scholar] [CrossRef]
- Xiao, J.; Xu, F. State bounding estimation for a linear continuous-time singular system with time-varying delay. Adv. Differ. Equ. 2019, 2019, 120. [Google Scholar] [CrossRef]
- Chang, X.H. Robust nonfragile filtering of fuzzy systems with linear fractional parametric uncertainties. IEEE Trans. Fuzzy Syst. 2012, 20, 1001–1011. [Google Scholar] [CrossRef]
- Jerbi, H.; Kchaou, M.; Boudjemline, A.; Regaieg, M.A.; Aoun, S.B.; Kouzou, A.L. H∞ and Passive Fuzzy Control for Non-Linear Descriptor Systems with Time-Varying Delay and Sensor Faults. Mathematics 2021, 9, 2203. [Google Scholar] [CrossRef]
- Lien, C.H.; Hou, Y.Y.; Yu, K.W.; Chang, H.C. Aperiodic sampled-data robust H∞ control of uncertain continuous switched time-delay systems. Int. J. Syst. Sci. 2020, 51, 2005–2024. [Google Scholar] [CrossRef]
- Du, D. Generalized output feedback controller design for uncertain discrete-time switched systems via switched Lyapunov functions. Nonlinear Analy. Theory Methods Appl. 2006, 65, 2135–2146. [Google Scholar] [CrossRef]
- Karimi, H.R.; Gao, H. Mixed H2/H∞ output-feedback control of second-order neutral systems with time-varying state and input delays. ISA Trans. 2008, 47, 311–324. [Google Scholar] [CrossRef]
- Kim, J.H. Robust mixed H2/H∞ control of time-varying delay systems. Int. J. Syst. Sci. 2001, 32, 1345–1351. [Google Scholar] [CrossRef]
- Lien, C.H.; Yu, K.W.; Chang, H.C. Robust mixed performance switching control for uncertain discrete switched systems with time delay. Int. J. Syst. Sci. 2018, 49, 2144–2154. [Google Scholar] [CrossRef]
- Li, T.; Guo, L.; Sun, C. Robust stability for neural networks with time-varying delays and linear fractional uncertainties. Neurocomputing 2007, 71, 421–427. [Google Scholar] [CrossRef]
- Yang, J.; Luo, W.; Li, G.; Zhong, S. Reliable guaranteed cost control for uncertain fuzzy neutral systems. Nonlinear Anal. Hybrid Syst. 2010, 4, 644–658. [Google Scholar] [CrossRef]
- Boyd, S.P.; El Ghaoui, L.; Feron, E.; Balakrishnan, V. Linear Matrix Inequalities in System and Control Theory; SIAM: Philadelphia, PA, USA, 1994. [Google Scholar]



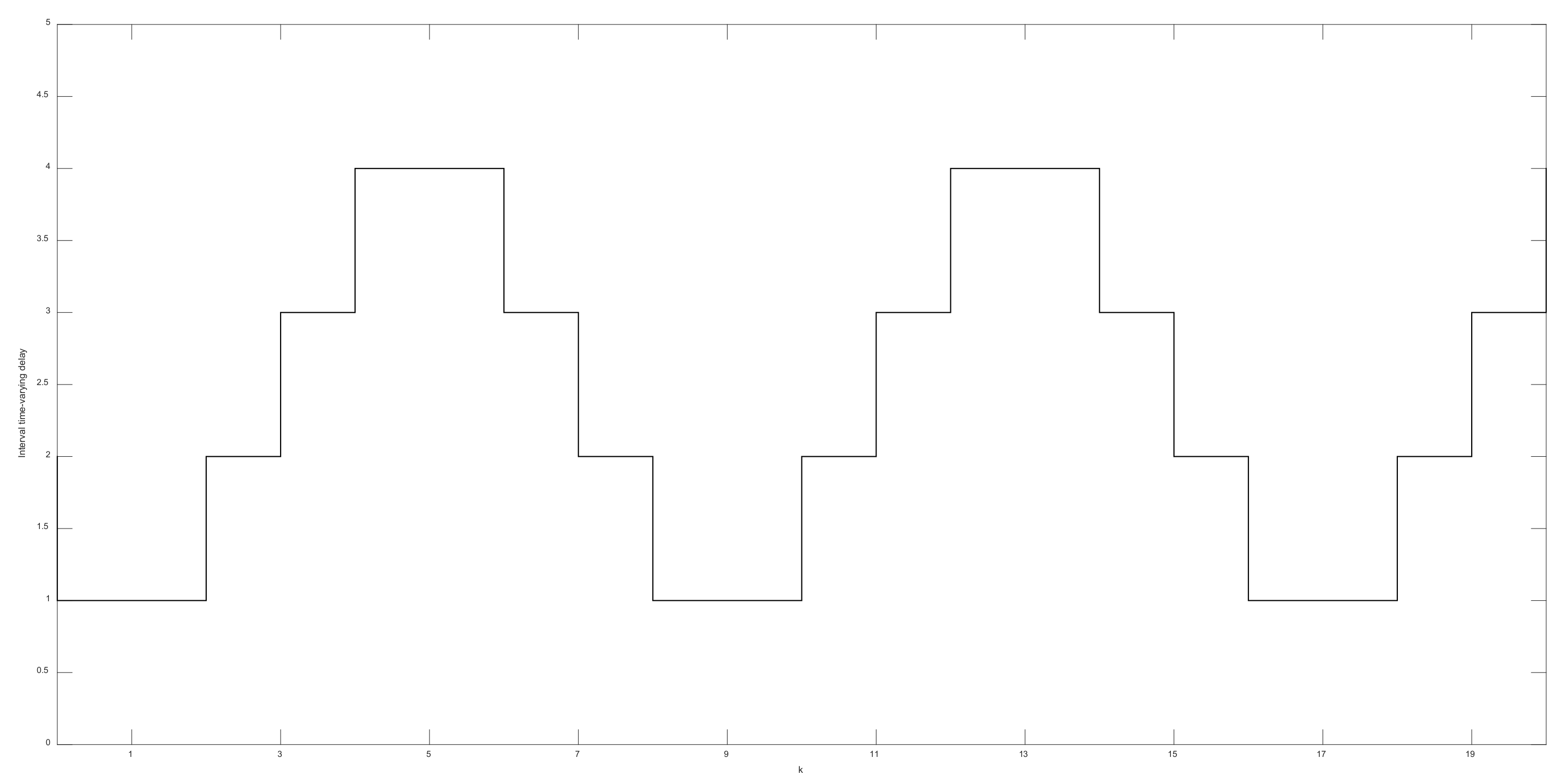
| Some Comparisons Regarding System (1) or (18) with (7) and (28) | |||
|---|---|---|---|
| Results | Interval Time-Varying Delay | Conditions | Reachable Set and Performance |
| [6] | No perturbations () and no control | ||
| [4] | |||
| The proposed results in this paper | measure performance | ||
and no control | measure performance | ||
| No perturbations () Switched control in (20d) with (27a) | measure performance | ||
Switched control in (20d) with (27b) | measure performance | ||
| Some Comparisons Regarding System (1) or (18) with (7) and (29) | |||
|---|---|---|---|
| Results | Interval Time-Varying Delay | Conditions | Reachable Set and Performance |
| [6] | No perturbations () and no control | Fail | |
| [4] | Fail | ||
| The proposed results in this paper | No perturbations () Switched control in (20d) with (30) | measure performance | |
Switched control in (20d) with (31) | measure performance | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lien, C.-H.; Chang, H.-C.; Yu, K.-W.; Li, H.-C.; Hou, Y.-Y. Reachable Set and Robust Mixed Performance of Uncertain Discrete Systems with Interval Time-Varying Delay and Linear Fractional Perturbations. Mathematics 2021, 9, 2763. https://doi.org/10.3390/math9212763
Lien C-H, Chang H-C, Yu K-W, Li H-C, Hou Y-Y. Reachable Set and Robust Mixed Performance of Uncertain Discrete Systems with Interval Time-Varying Delay and Linear Fractional Perturbations. Mathematics. 2021; 9(21):2763. https://doi.org/10.3390/math9212763
Chicago/Turabian StyleLien, Chang-Hua, Hao-Chin Chang, Ker-Wei Yu, Hung-Chi Li, and Yi-You Hou. 2021. "Reachable Set and Robust Mixed Performance of Uncertain Discrete Systems with Interval Time-Varying Delay and Linear Fractional Perturbations" Mathematics 9, no. 21: 2763. https://doi.org/10.3390/math9212763
APA StyleLien, C.-H., Chang, H.-C., Yu, K.-W., Li, H.-C., & Hou, Y.-Y. (2021). Reachable Set and Robust Mixed Performance of Uncertain Discrete Systems with Interval Time-Varying Delay and Linear Fractional Perturbations. Mathematics, 9(21), 2763. https://doi.org/10.3390/math9212763







