Stability Switches and Double Hopf Bifurcation Analysis on Two-Degree-of-Freedom Coupled Delay van der Pol Oscillator
Abstract
:1. Introduction
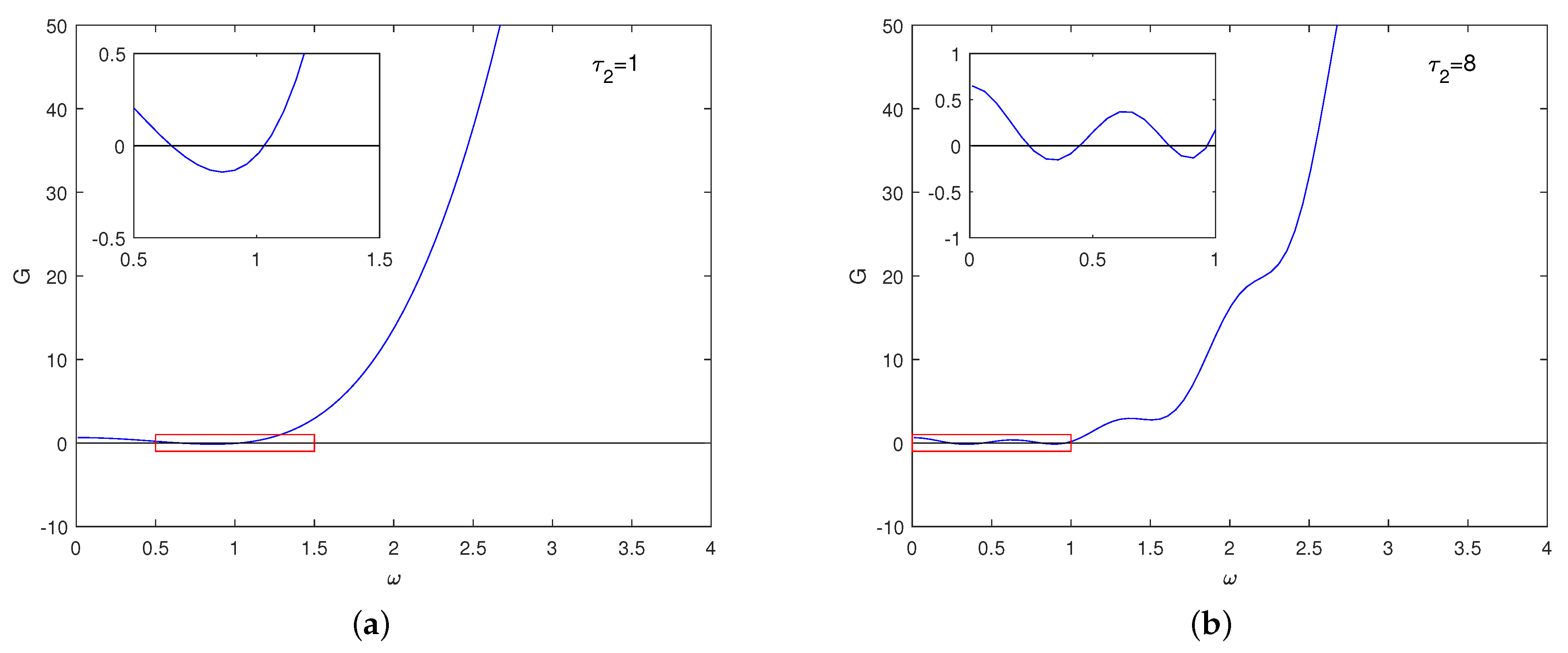
2. Stability Analysis and Hopf Bifurcation
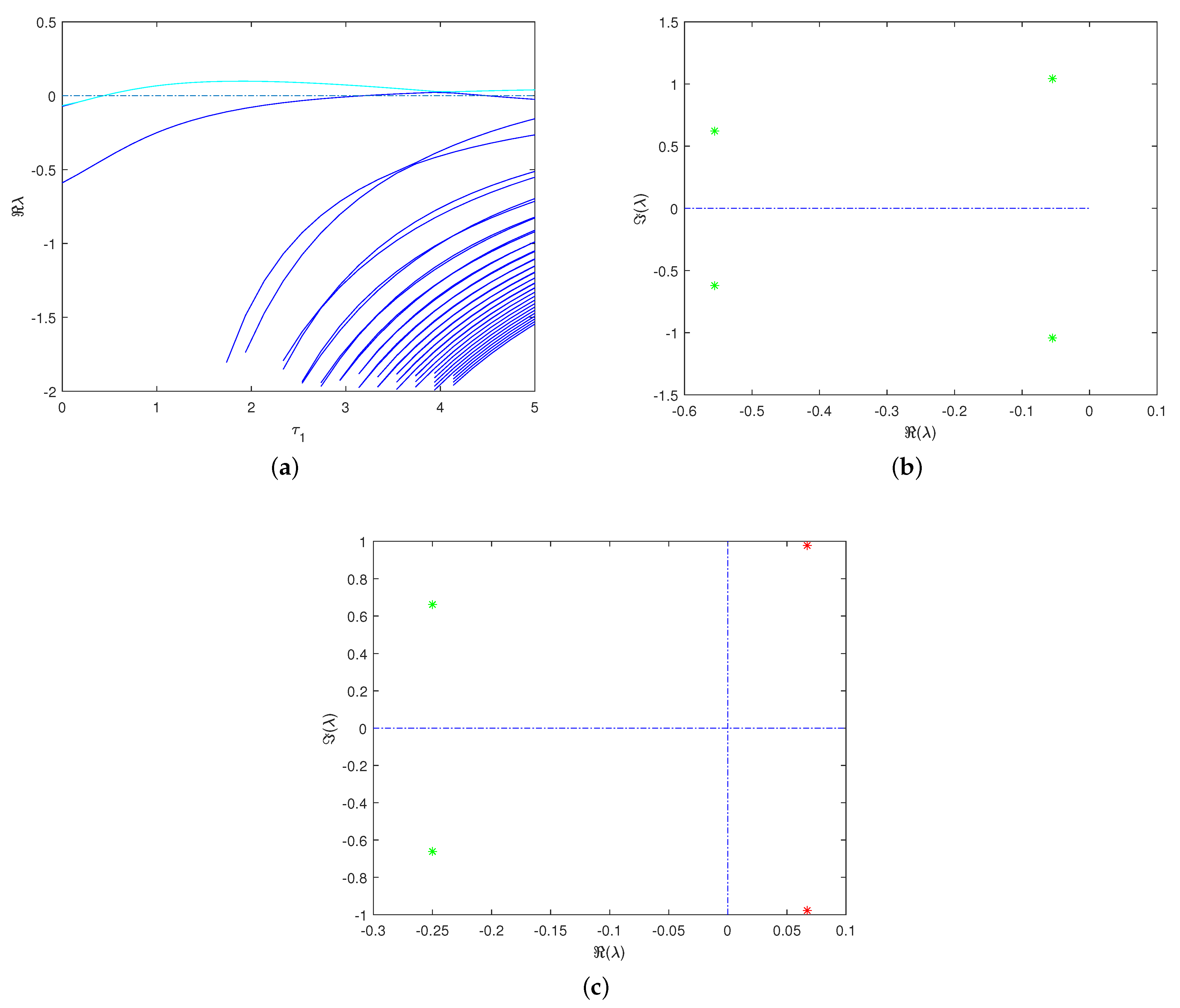
3. Analysis of Double Hopf Bifurcation
3.1. Computation of Normal Form and Center-Manifold Reduction
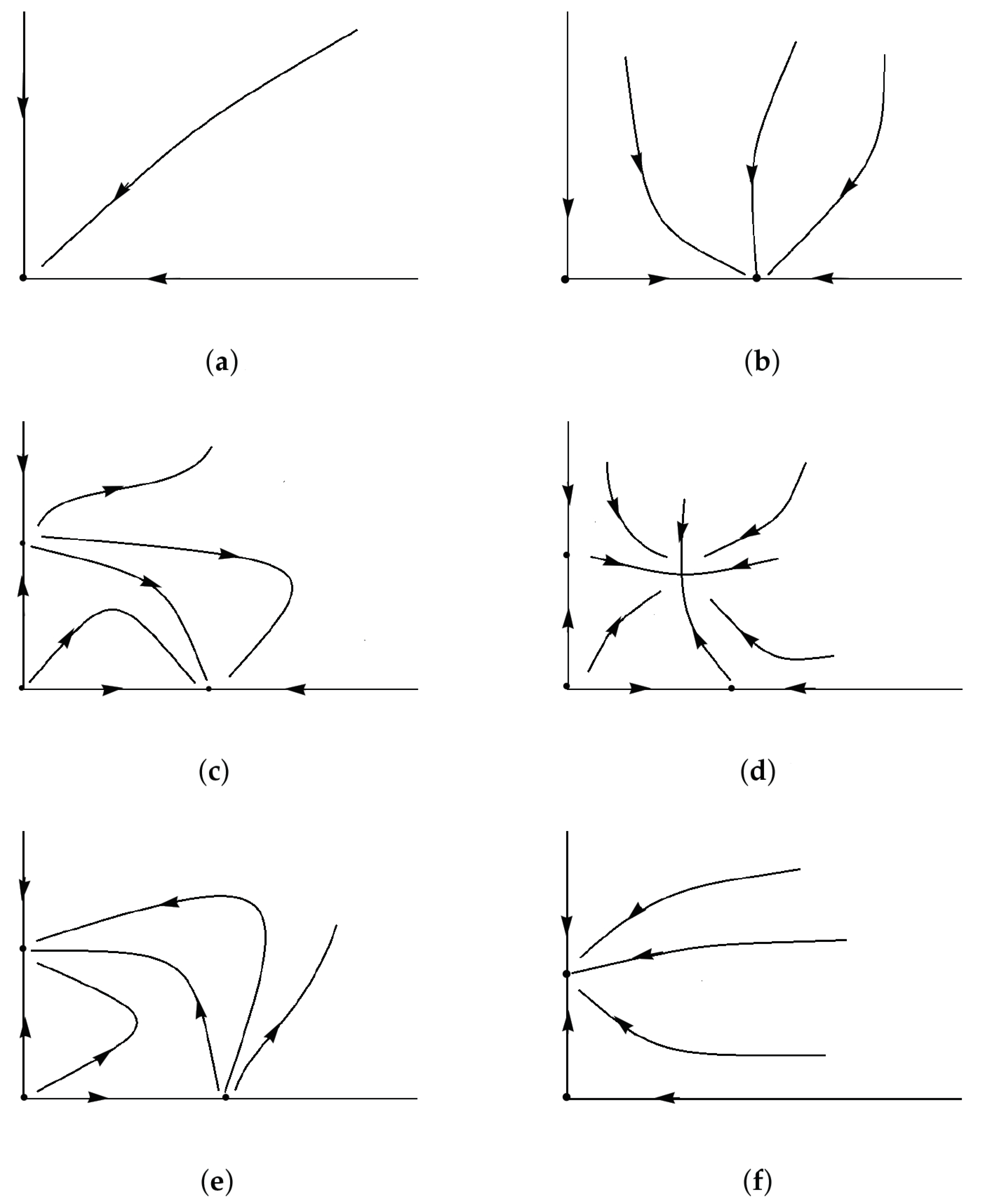
3.2. Classification of Dynamical Behaviours
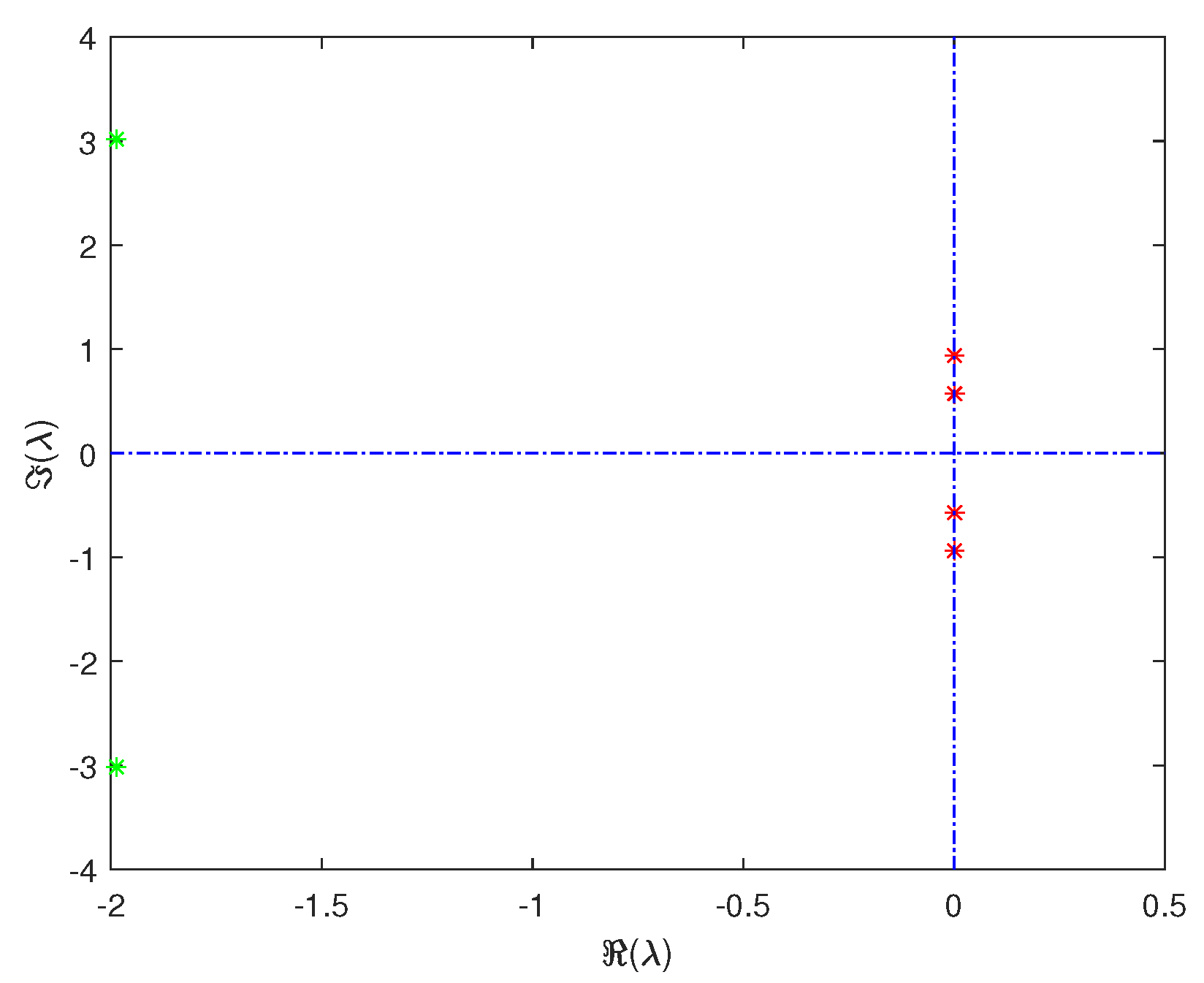
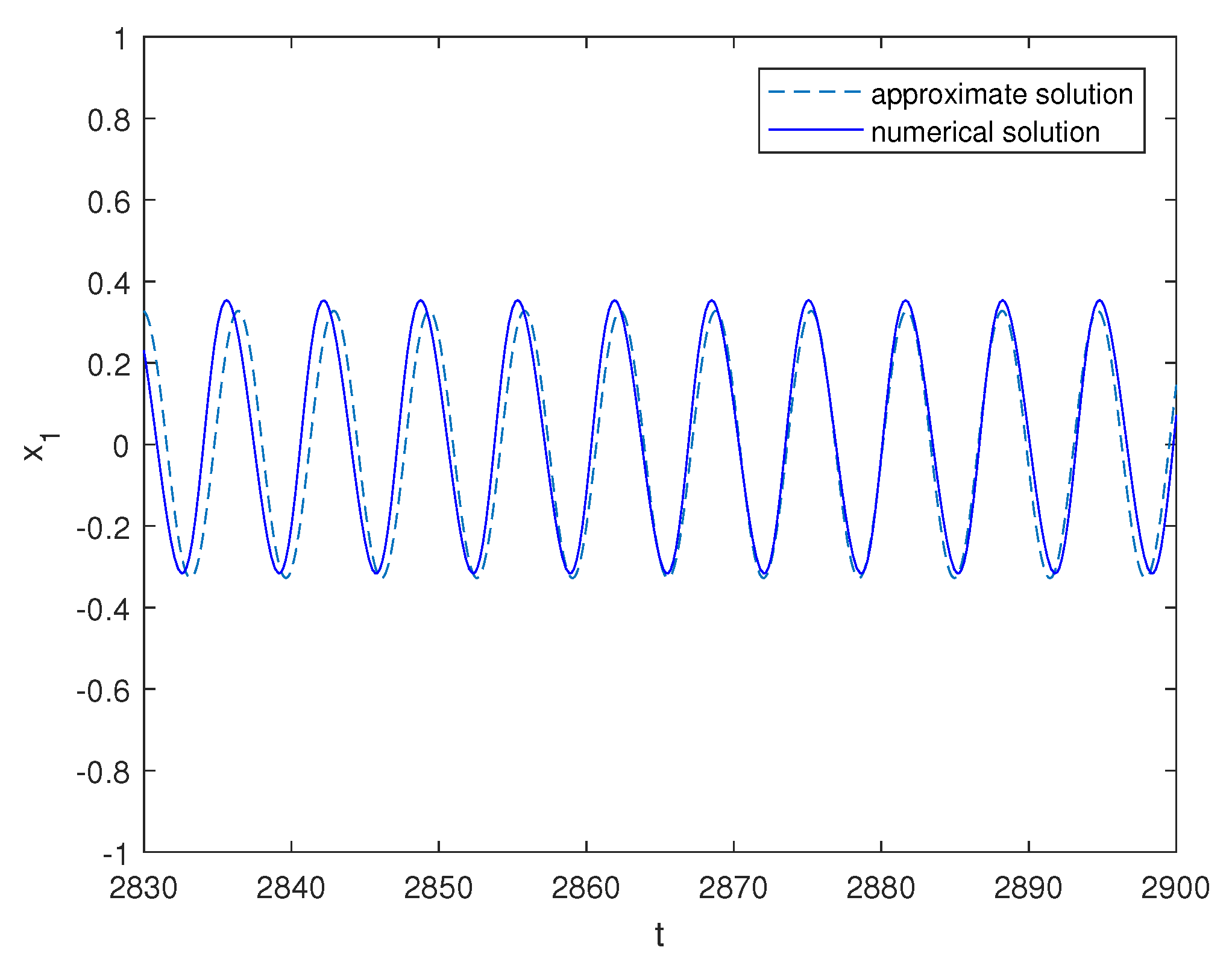
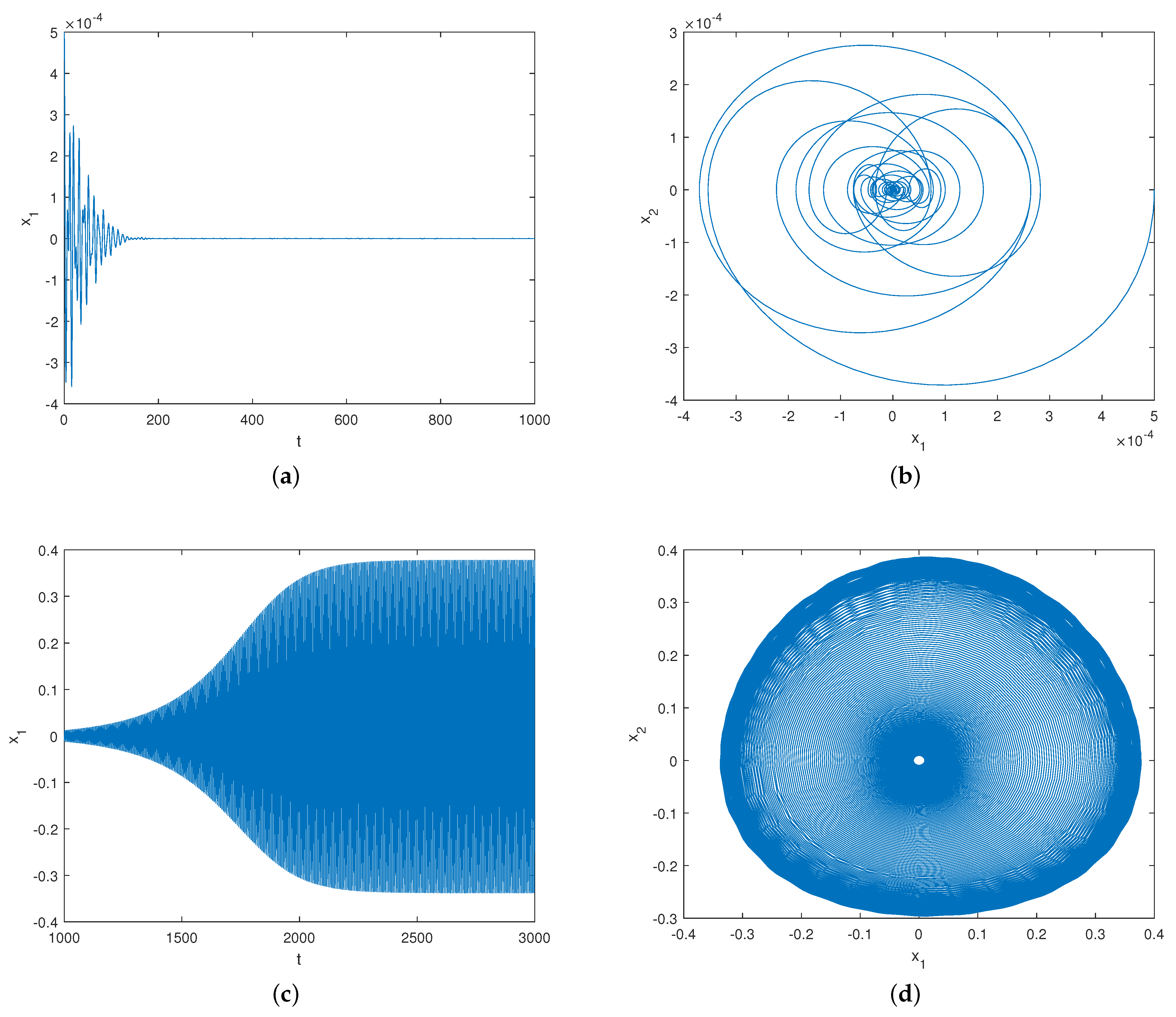
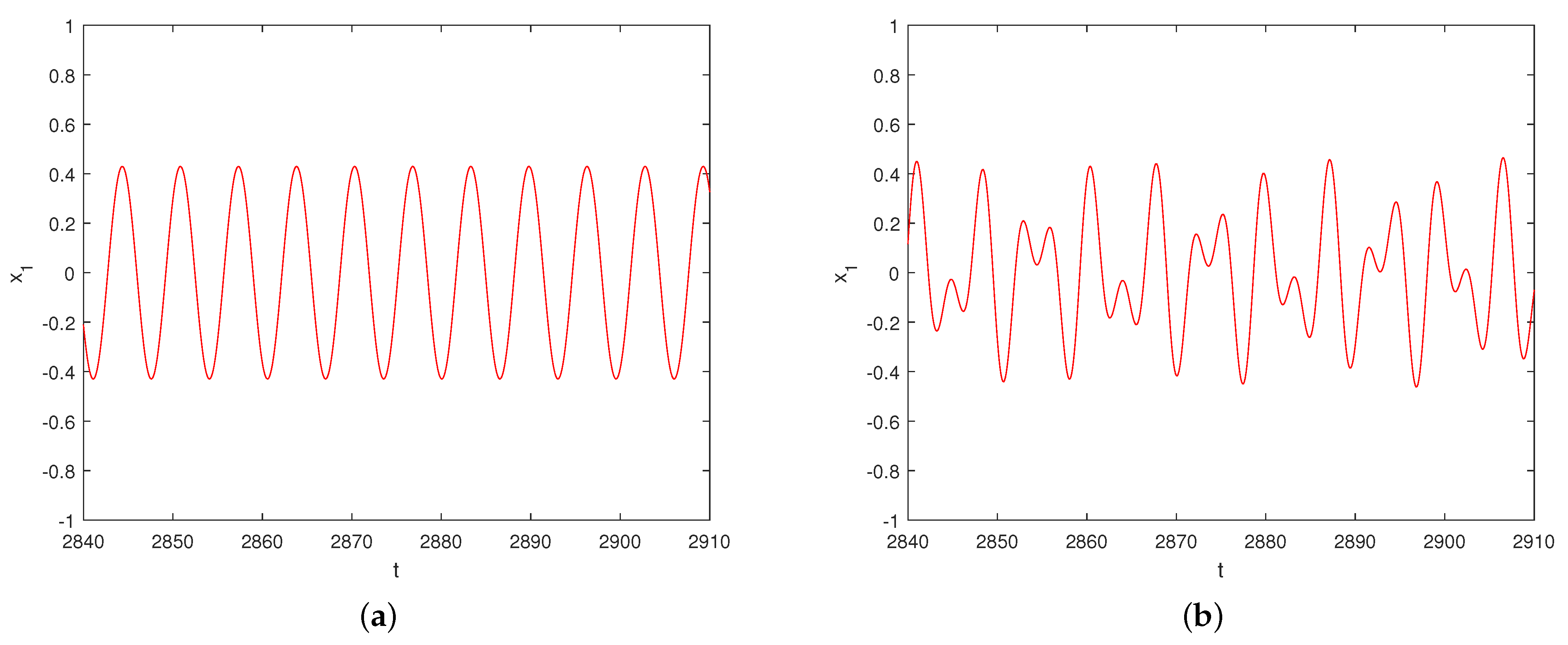
3.3. Numerical Simulation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Ouyang, D.Q.; Shao, J.; Jiang, H.J.; Nguang, S.K.; Shen, H.T. Impulsive synchronization of coupled delayed neural networks with actuator saturation and its application to image encryption. Neural Netw. 2020, 128, 158–171. [Google Scholar] [CrossRef] [PubMed]
- Wang, K.; Yan, X.P.; Yang, Q.; Hao, X.H.; Wang, J.T. Weak Signal Detection Based on Strongly Coupled Duffing-Van der Pol Oscillator and Long Short-term Memory. J. Phys. Soc. Jpn. 2020, 1, 014003. [Google Scholar] [CrossRef]
- Dal, F. The Method of Multiple Time Scales and Finite Differences Method for the van der Pol Oscillator with Small Fractional Damping. Asian Res. J. Math. 2017, 2, 1–11. [Google Scholar] [CrossRef]
- Dong, Y.K.; Wang, D.; Cui, L. Correction to: Assessment of depth-averaged method in analysing runout of submarine landslide. Landslides 2020, 17, 557–565. [Google Scholar] [CrossRef] [Green Version]
- Belhaq, M.; Ghouli, Z.; Hamdi, M. Energy harvesting in a Mathieu–Cvan der Pol–CDuffing MEMS device using time delay. Nonlinear Dyn. 2018, 94, 2537–2546. [Google Scholar] [CrossRef]
- Yang, R.Z.; Zhang, C.R. Dynamics in a diffusive modified Leslie–CGower predator–Cprey model with time delay and prey harvesting. Nonlinear Dyn. 2017, 87, 863–878. [Google Scholar] [CrossRef]
- Shi, J.F.; Gou, X.F.; Zhu, L.Y. Bifurcation of multi-stable behaviors in a two-parameter plane for a non-smooth nonlinear system with time-varying parameters. Nonlinear Dyn. 2020, 100, 3347–3365. [Google Scholar] [CrossRef]
- Stankevich, N.V.; Kuznetsov, A.P.; Seleznev, E.P. Quasi-periodic bifurcations of four-frequency tori in the ring of five coupled van der Pol oscillators with different types of dissipative coupling. Tech. Phys. 2017, 62, 971–974. [Google Scholar] [CrossRef]
- Bukh, A.V.; Anishchenko, V.S. Spiral, Target, and Chimera Wave Structures in a Two-Dimensional Ensemble of Nonlocally Coupled van der Pol Oscillators. Tech. Phys. Lett. 2019, 45, 675–678. [Google Scholar] [CrossRef]
- Singh, A.K.; Yadava, R.D.S. Transient motion and chaotic dynamics in a pair of van der Pol oscillators. Eur. Phys. J. Plus 2019, 134, 1–10. [Google Scholar]
- Algaba, A.; Chung, K.W.; Qin, B.W.; Rodriguez-Luis, A.J. Analytical approximation of the canard explosion in a van der Pol system with the nonlinear time transformation method. Phys. D Nonlinear Phenom. 2020, 406, 132384. [Google Scholar] [CrossRef]
- Qian, Y.H.; Fu, H.X. Research for Coupled van der Pol Systems with Parametric Excitation and Its Application. Z. für Naturforschung 2017, 72, 1009–1020. [Google Scholar] [CrossRef]
- Kumar, M.; Varshney, P. Numerical Simulation of Van der Pol Equation Using Multiple Scales Modified Lindstedt–Poincare Method. Proc. Natl. Acad. Sci. India Sect. A Phys. Sci. 2020, 67, 1–11. [Google Scholar] [CrossRef]
- Guckenheimer, J.; Holmes, P. Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields. Appl. Math. Sci. 1983, 42, 353–411. [Google Scholar]
- Peng, M.; Zhang, Z.D.; Qu, Z.F.; Bi, Q.S. Qualitative analysis in a delayed Van der Pol oscillator. Phisica A Stat. Mech. Its Appl. 2020, 554, 123482. [Google Scholar] [CrossRef]
- Sidorov, N.A. Explicit and implicit parametrizations in the construction of branching solutions by iterative methods. Sb. Math. 1995, 186, 297–310. [Google Scholar] [CrossRef]
- Sidorov, N.A.; Leont’ev, R.Y.; Dreglya, A.I. On small solutions of nonlinear equations with vector parameter in sectorial neighborhoods. Math. Notes 2012, 91, 90–104. [Google Scholar] [CrossRef]
- Chen, Z.; Yu, P. Double-Hopf bifurcation in an oscillation with external forcing and time-delayed feedback control. Int. J. Bifurc. Chaos Appl. Sci. Eng. 2006, 16, 3523–3537. [Google Scholar] [CrossRef] [Green Version]
- Du, Y.F.; Niu, B.; Guo, Y.X.; Wei, J.J. Double Hopf Bifurcation in Delayed reaction-diffusion Systems. J. Dyn. Differ. Equ. 2020, 32, 313–358. [Google Scholar] [CrossRef] [Green Version]
- Song, Z.G.; Xu, J. Bifurcation and chaos analysis for a delayed two-neural network with a variation slope ratio in the activation function. Int. J. Bifurc. Chaos Appl. Sci. Eng. 2012, 22, 1250105. [Google Scholar] [CrossRef]
- Ge, J.H.; Xu, J. Fold-Hopf bifurcation in a simplified four-neuron BAM (bidirectional associative memory) neural network with two delays. Sci. China Technol. Sci. 2010, 53, 633–644. [Google Scholar] [CrossRef]
- Qian, Y.H.; Fu, H.X.; Guo, J.M. Weakly resonant double Hopf bifurcation in coupled nonlinear systems with delayed freedback and application of homotopy analysis method. J. Low Freq. Noise Vib. Act. Control 2019, 38, 1651–1675. [Google Scholar] [CrossRef]
- Song, Z.G.; Xu, J. Stability switches and double Hopf bifurcation in a two-neural network system with multiple delays. Cogn. Neurodynamics 2013, 7, 505–521. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Song, Z.G.; Xu, J. Stability switches and multistability coexistence in a delay-coupled neural oscillators system. J. Theor. Biol. 2012, 313, 98–114. [Google Scholar] [CrossRef]
- Song, Z.G.; Xu, J. Codimension-two bursting analysis in the delayed neural system with external stimulations. Nonlinear Dyn. 2012, 67, 309–328. [Google Scholar] [CrossRef]
- Song, Z.G.; Wang, C.H.; Zhen, B. Codimension-two bifurcation and multistability coexistence in an inertial two-neuron system with multiple delays. Nonlinear Dyn. 2016, 85, 2099–2113. [Google Scholar] [CrossRef]
- Engelborghs, K.; Luzyanina, T.; Roose, D. Numerical bifurcation analysis of delay differential equations using DDE_BIFTOOL. ACM Trans. Math. Softw. 2002, 28, 1–21. [Google Scholar] [CrossRef]


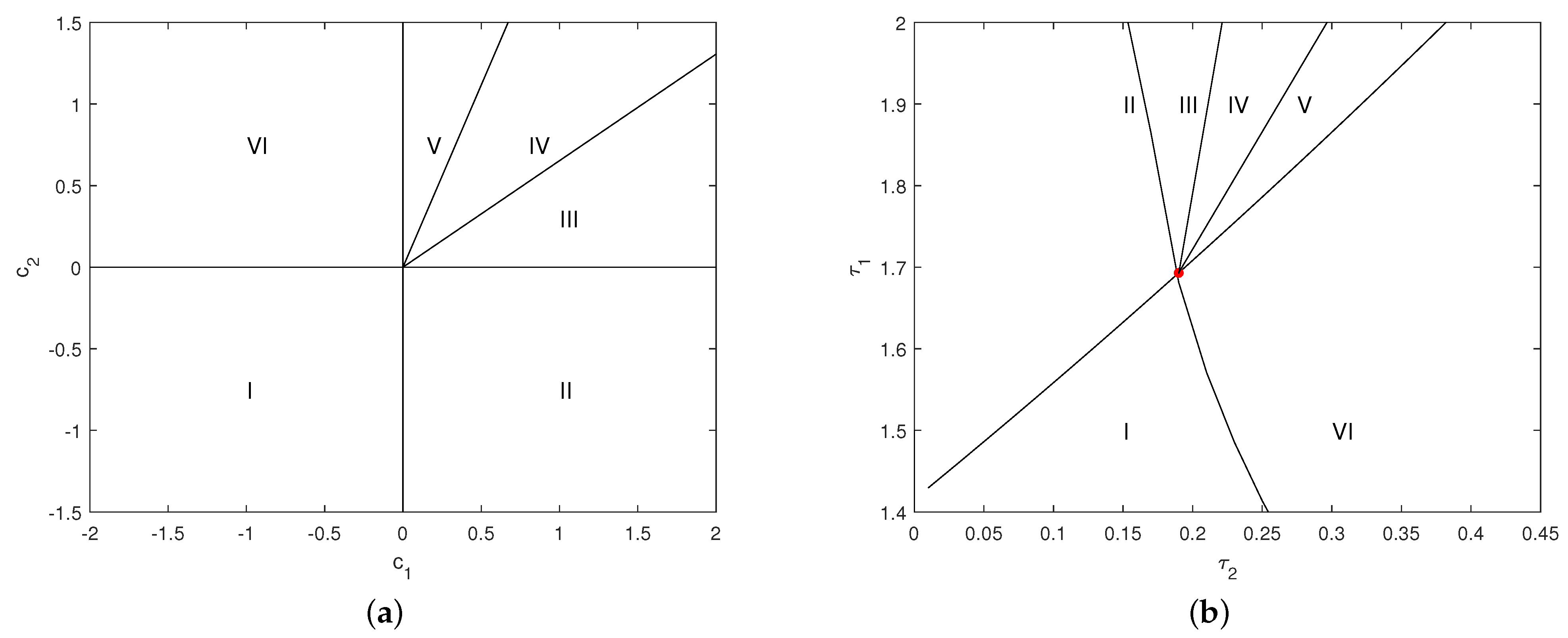
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.; Qian, Y. Stability Switches and Double Hopf Bifurcation Analysis on Two-Degree-of-Freedom Coupled Delay van der Pol Oscillator. Mathematics 2021, 9, 2444. https://doi.org/10.3390/math9192444
Chen Y, Qian Y. Stability Switches and Double Hopf Bifurcation Analysis on Two-Degree-of-Freedom Coupled Delay van der Pol Oscillator. Mathematics. 2021; 9(19):2444. https://doi.org/10.3390/math9192444
Chicago/Turabian StyleChen, Yani, and Youhua Qian. 2021. "Stability Switches and Double Hopf Bifurcation Analysis on Two-Degree-of-Freedom Coupled Delay van der Pol Oscillator" Mathematics 9, no. 19: 2444. https://doi.org/10.3390/math9192444
APA StyleChen, Y., & Qian, Y. (2021). Stability Switches and Double Hopf Bifurcation Analysis on Two-Degree-of-Freedom Coupled Delay van der Pol Oscillator. Mathematics, 9(19), 2444. https://doi.org/10.3390/math9192444





