Assessing the Non-Linear Dynamics of a Hopf–Langford Type System
Abstract
1. Introduction
2. Analytical Results
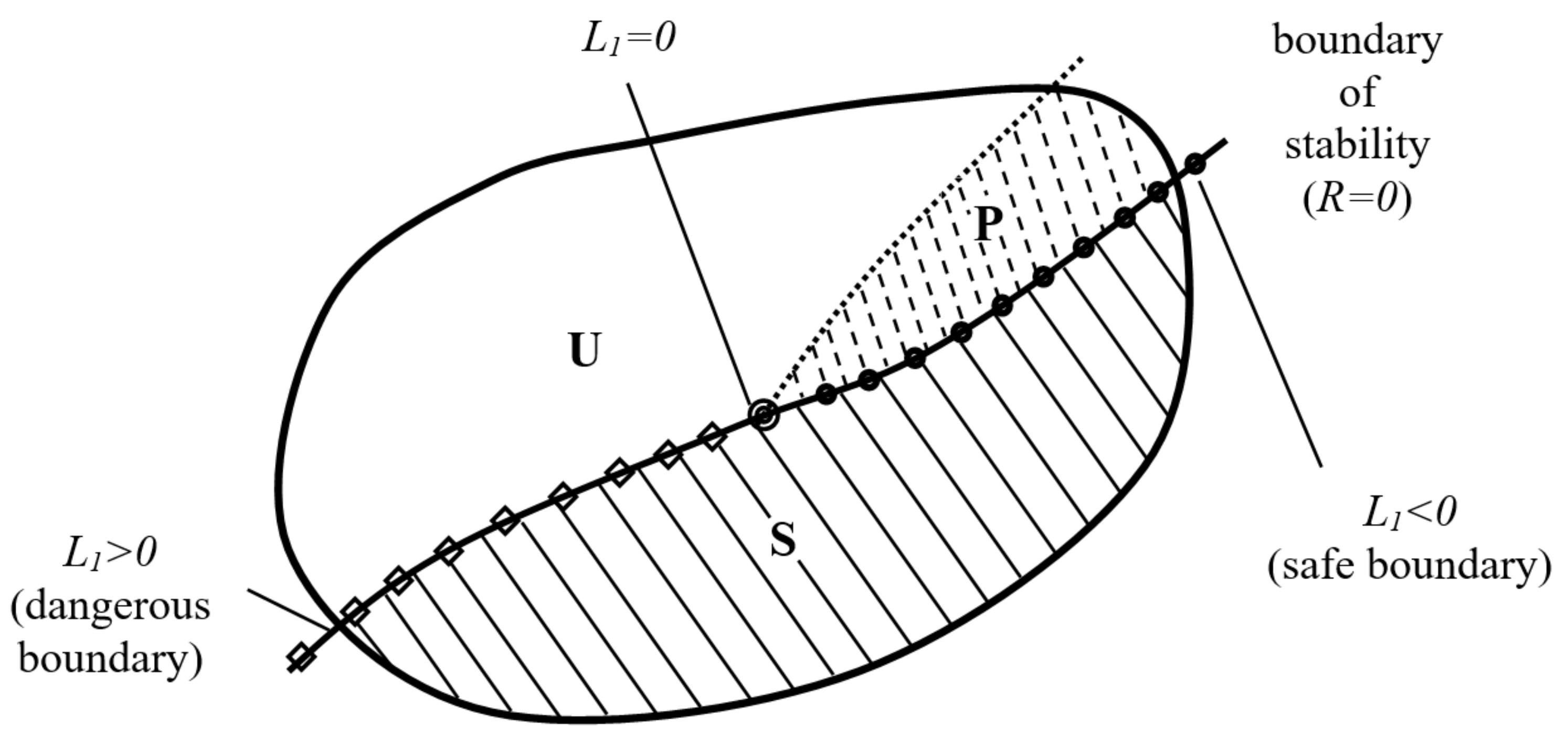
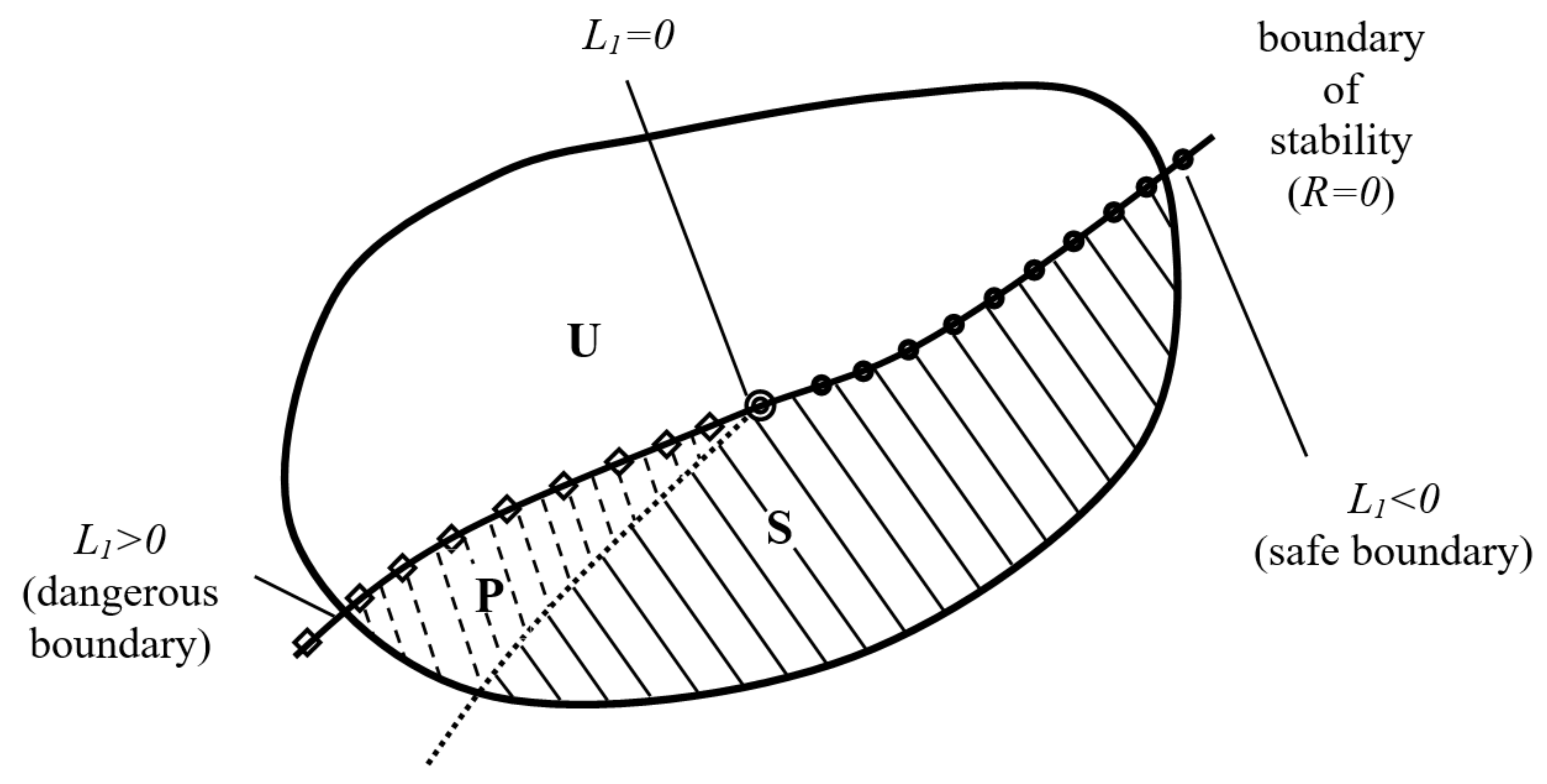
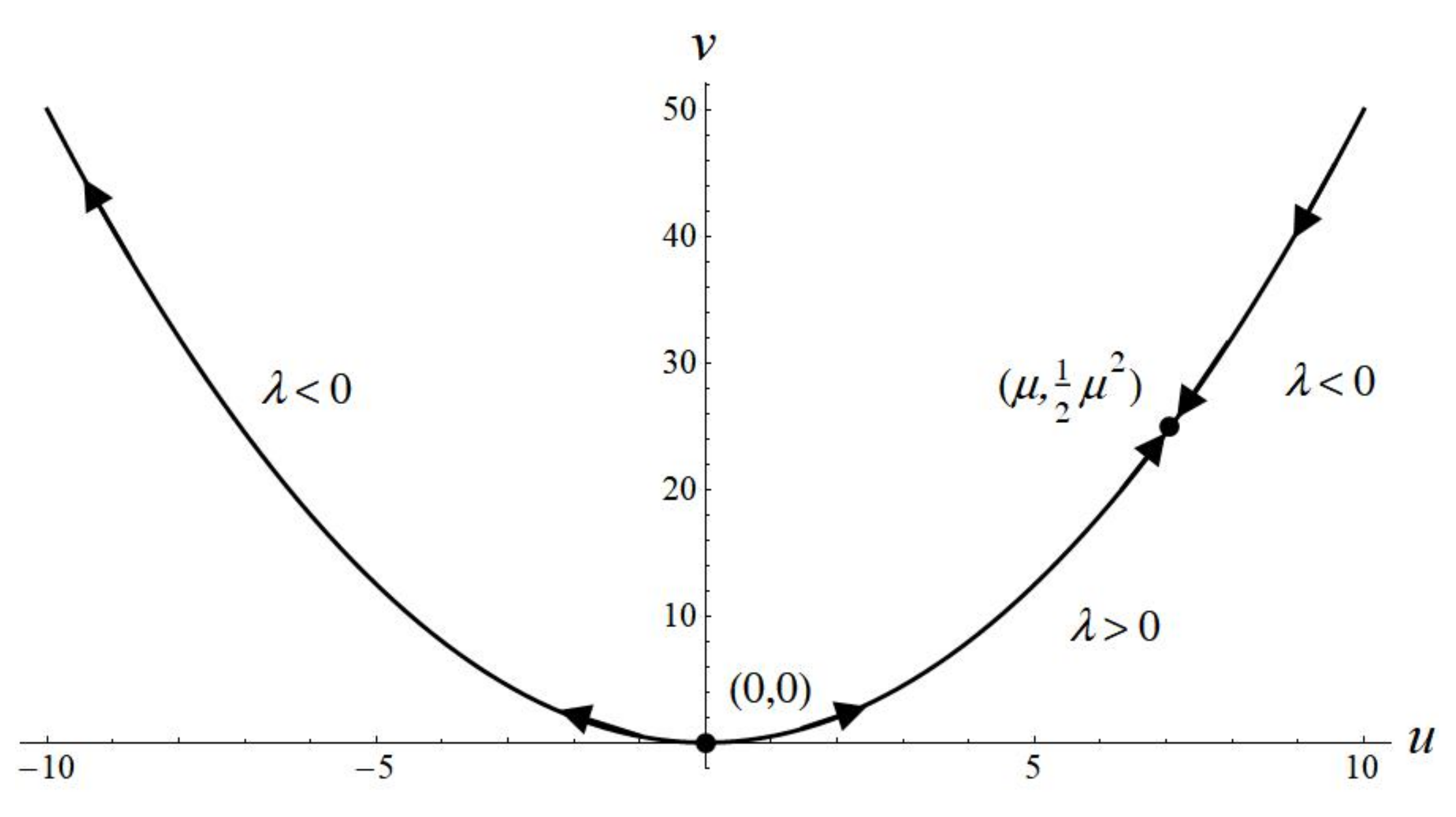
2.1. Qualitative Analysis
2.2. A Family of Exact Solutions
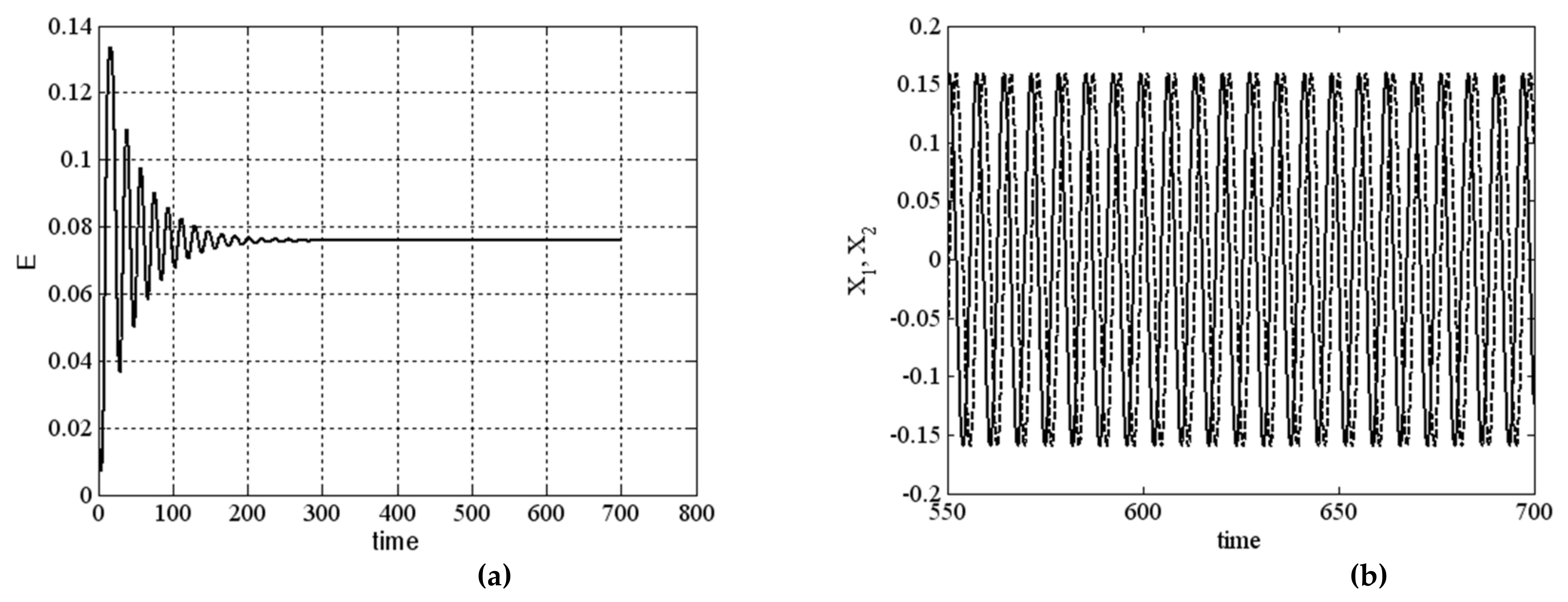
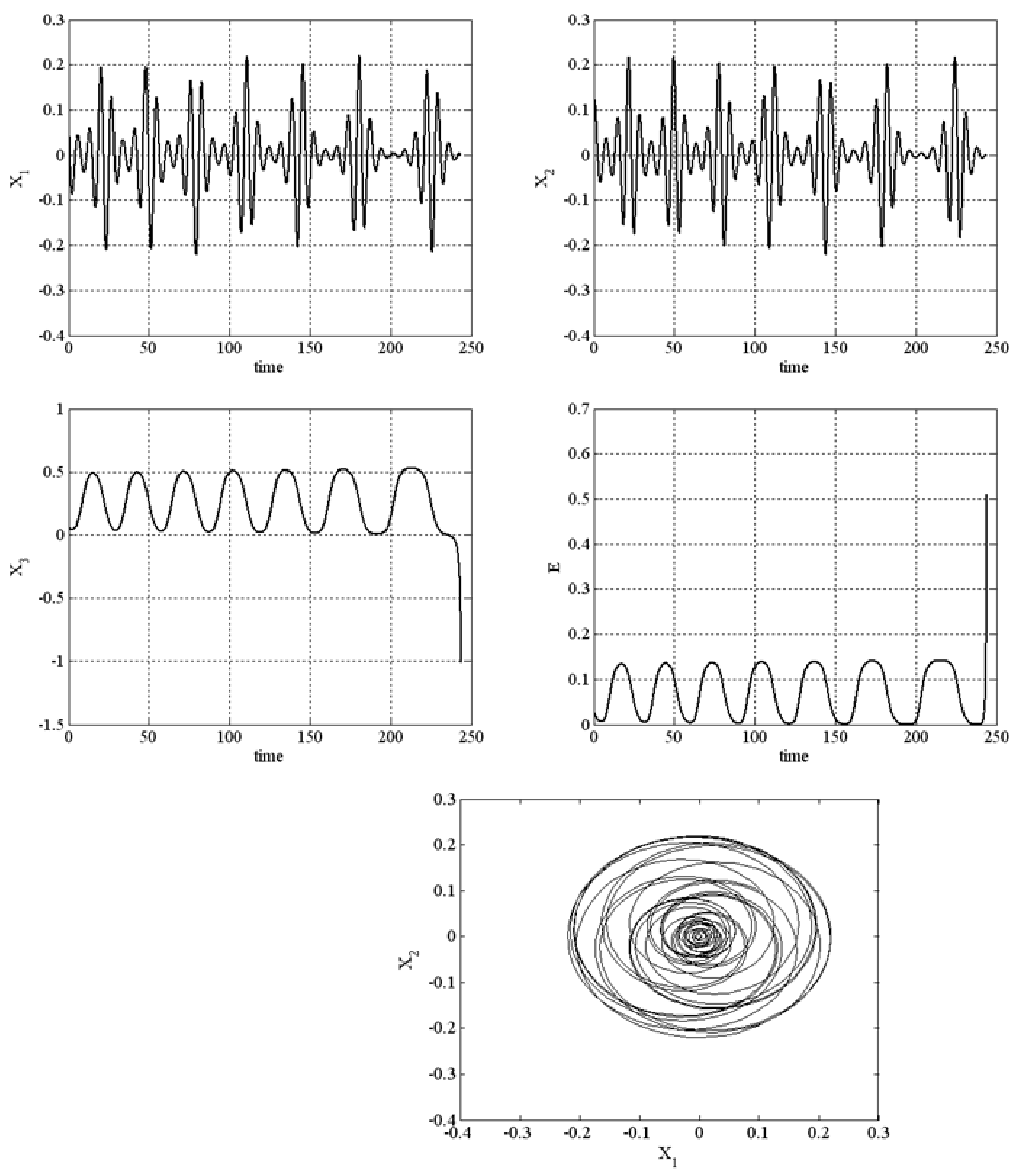
3. Numerical Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Analytical Calculation of Second Lyapunov Value—L2 (λ0)


Appendix B
Derivation of
Appendix C
First Lyapunov Value—L1
Appendix D
Derivation of Equilibrium Points
References
- Guckenheimer, J.; Holmes, P.H. Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields; Springer: New York, NY, USA, 2002. [Google Scholar]
- Neimark, Y.; Landa, P. Stochastic and Chaotic Oscillations; Kluwer Academic Press: Dordrecht, The Netherlands, 1992. [Google Scholar]
- Shilnikov, L.; Shilnikov, A.; Turaev, D.; Chua, L. Methods of Qualitative Theory in Nonlinear Dynamics; Part II; World Scientific: London, UK, 2001. [Google Scholar]
- Phillipson, P.; Schuster, P. Analytics of bifurcation. Int. J. Bifurc. Chaos 1998, 8, 471–482. [Google Scholar] [CrossRef]
- Nikolov, S. First Lyapunov value and bifurcation behaviour of specific class three-dimensional systems. Int. J. Bifurc. Chaos 2004, 14, 2811–2823. [Google Scholar] [CrossRef]
- Mosekilde, E.; Mastrenko, Y.; Postnov, D. Chaotic Synchronization. Application for Living Systems; World Scientific: Singapore, 2002. [Google Scholar]
- Pikovsky, A.; Rosenblum, M.; Kurths, J. Synchronization, a Universal Concept in Nonlinear Science; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Tokuda, I.; Kurths, J.; Rosa, E. Learning phase synchronization from nonsynchronized chaotic regimes. Phys. Rev. Lett. 2002, 88, 014101. [Google Scholar] [CrossRef]
- Gonchenko, S.; Shilnikov, L.; Turaev, D. Dynamical phenomena in systems with structurally unstable Poincare homoclinic orbits. Chaos 1996, 6, 15–31. [Google Scholar] [CrossRef] [PubMed]
- Smale, S. Structurally stable systems are not dense. Amer. J. Math. 1966, 88, 491–496. [Google Scholar] [CrossRef]
- Smale, S. Dynamical Systems and Turbulence; Springer: Berlin, Germany, 1977. [Google Scholar]
- Andronov, A.; Witt, A.; Chaikin, S. Theory of Oscillations; Addison-Wesley: Boston, MA, USA, 1966. [Google Scholar]
- Bautin, N.; Leontovich, E. Methods and Approaches for Investigation of Two Dimensional Dynamical Systems; Nauka: Moscow, Russia, 1976. (In Russian) [Google Scholar]
- Bautin, N. Behaviour of Dynamical Systems near the Boundary of Stability; Nauka: Moscow, Russia, 1984. (In Russian) [Google Scholar]
- Nikolov, S.; Petrov, V. New results about route to chaos in Rossler system. Int. J. Bifurc. Chaos 2004, 14, 293–308. [Google Scholar] [CrossRef]
- Leonov, G.; Kuznetsov, N. Hidden attractors in dynamical systems. From hidden oscillations in Hilbert-Kolmogorov, Aizerman, and Kalman problems to hidden chaotic attractors in Chua circuits. Int. J. Bifurc. Chaos 2013, 23, 1330002. [Google Scholar] [CrossRef]
- Hopf, E. A mathematical example displaying features of turbulence. Commun. Pur. Appl. Math. 1948, 1, 303–322. [Google Scholar] [CrossRef]
- Langford, W. Periodic and steady-state mode interactions lead to tori. SIAM J. Appl. Math. 1979, 37, 22–48. [Google Scholar] [CrossRef]
- Hassard, B.; Kazarinoff, N.; Wan, Y. Theory and Applications of Hopf Bifurcation; CUP Archive: Cambridge, UK, 1981. [Google Scholar]
- Nikolov, S.; Bozhkov, B. Bifurcations and chaotic behaviour on the Lanford system. Chaos Solitons Fractals 2004, 21, 803–808. [Google Scholar] [CrossRef]
- Guo, G.; Wang, X.; Lin, X.; Wei, M. Steady-state and Hopf bifurcations in the Langford ODE and PDE systems. Nonlinear Anal. Real World Appl. 2017, 34, 343–362. [Google Scholar] [CrossRef]
- Yang, Q.; Yang, T. Complex dynamics in a generalized Langford system. Nonlinear Dyn. 2018, 91, 2241–2270. [Google Scholar] [CrossRef]
- Nikolov, S.; Vassilev, V. Completely integrable dynamical systems of Hopf-Langford type. Commun. Nonlinear Sci. Numer. Simul. 2021, 92, 105464. [Google Scholar] [CrossRef]
- Korn, G.; Korn, T. Mathematical Handbook for Scientists and Engineers; McGraw-Hill Book Company: New York, NY, USA, 1968. [Google Scholar]
- Pecora, L.; Carroll, T. Synchronization in chaotic systems. Phys. Rev. Lett. 1990, 64, 821–824. [Google Scholar] [CrossRef]
- Arrowsmith, D.; Place, C. Dynamical Systems. Differential Equations, Maps and Chaotic Behaviour; Chapman & Hall: London, UK, 1992. [Google Scholar]
- Petrov, V.; Peifer, M.; Timmer, J. Bistability and self-oscillations in cell cycle control. Int. J. Bifurc. Chaos 2006, 16, 1057–1066. [Google Scholar] [CrossRef]
- Leonov, G.; Kuznetsova, O. Lyapunov quantities and limit cycles of two-dimensional dynamical systems. Analytical methods and symbolic computation. Regul. Chaotic Dyn. 2010, 15, 354–377. [Google Scholar] [CrossRef]
- Liénard, A. Etude des oscillations entretenues. Rev. Gen. Électr. 1928, 23, 901–912, 946–954. [Google Scholar]
- Lakshmanan, M.; Chandrasekar, V. Generating finite dimensional integrable nonlinear dynamical systems. Eur. Phys. J. Spec. Top. 2013, 222, 665–688. [Google Scholar] [CrossRef][Green Version]
- Kudryashov, N.A.; Sinelshchikov, D.I. On the criteria for integrability of the Liénard equation. Appl. Math. Lett. 2016, 57, 114–120. [Google Scholar] [CrossRef]
- Chandrasekar, V.K.; Senthilvelan, M.; Lakshmanan, M. New aspects of integrability of force-free Duffing-van del Pol oscillator and related nonlinear systems. J. Phys. A Math. Gen. 2004, 37, 4527–4534. [Google Scholar] [CrossRef]
- Chandrasekar, V.K.; Senthilvelan, M.; Lakshmanan, M. On the general solution for the modified Emden-type equation. J. Phys. A Math. Theor. 2007, 40, 4717–4727. [Google Scholar] [CrossRef]
- Govinder, K.S. Analysis of modified Painlevé-Ince equations. Ricerche Mat. 2020, 1–11. [Google Scholar] [CrossRef]
- Ince, E.L. Ordinary Differential Equations; Dover Publications: New York, NY, USA, 1956. [Google Scholar]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nikolov, S.G.; Vassilev, V.M. Assessing the Non-Linear Dynamics of a Hopf–Langford Type System. Mathematics 2021, 9, 2340. https://doi.org/10.3390/math9182340
Nikolov SG, Vassilev VM. Assessing the Non-Linear Dynamics of a Hopf–Langford Type System. Mathematics. 2021; 9(18):2340. https://doi.org/10.3390/math9182340
Chicago/Turabian StyleNikolov, Svetoslav G., and Vassil M. Vassilev. 2021. "Assessing the Non-Linear Dynamics of a Hopf–Langford Type System" Mathematics 9, no. 18: 2340. https://doi.org/10.3390/math9182340
APA StyleNikolov, S. G., & Vassilev, V. M. (2021). Assessing the Non-Linear Dynamics of a Hopf–Langford Type System. Mathematics, 9(18), 2340. https://doi.org/10.3390/math9182340






