The Hybrid FEM-DBCI for the Solution of Open-Boundary Low-Frequency Problems
Abstract
1. Introduction
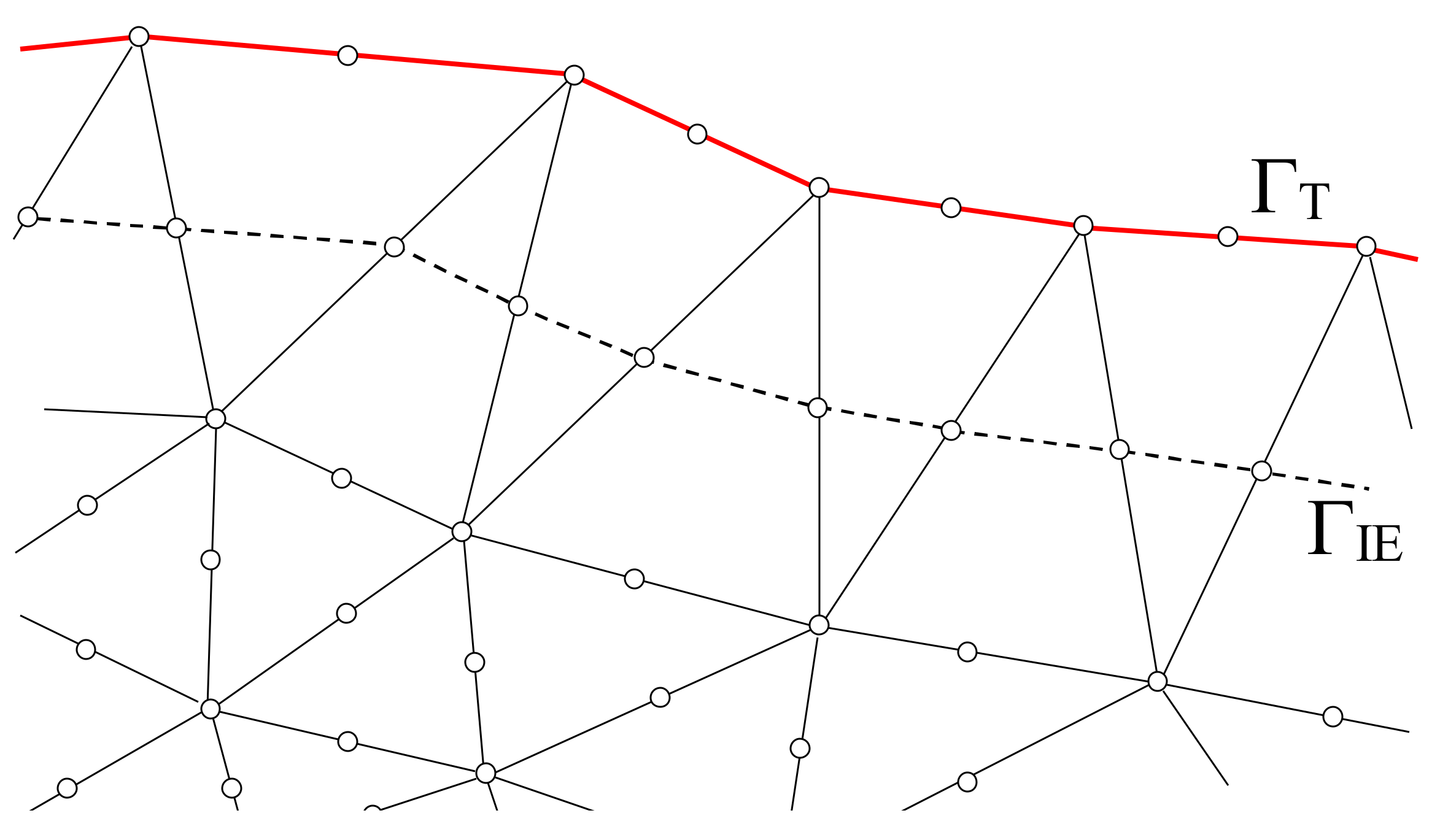
2. The FEM-DBCI Method for Electrostatics
3. The FEM-DBCI Method for Skin Effect
4. Solution of the Global Algebraic System
- (1)
- Set a zero initial guess for the Dirichlet condition on ΓT;
- (2)
- Solve the FEM equation (by means of the CG) to find ;
- (3)
- Compute .
- (a)
- Given the array ;
- (b)
- Solve the FEM equation with k0 = 0 (by means of the CG) to find ;
- (c)
- Compute .
5. An Analytical Example
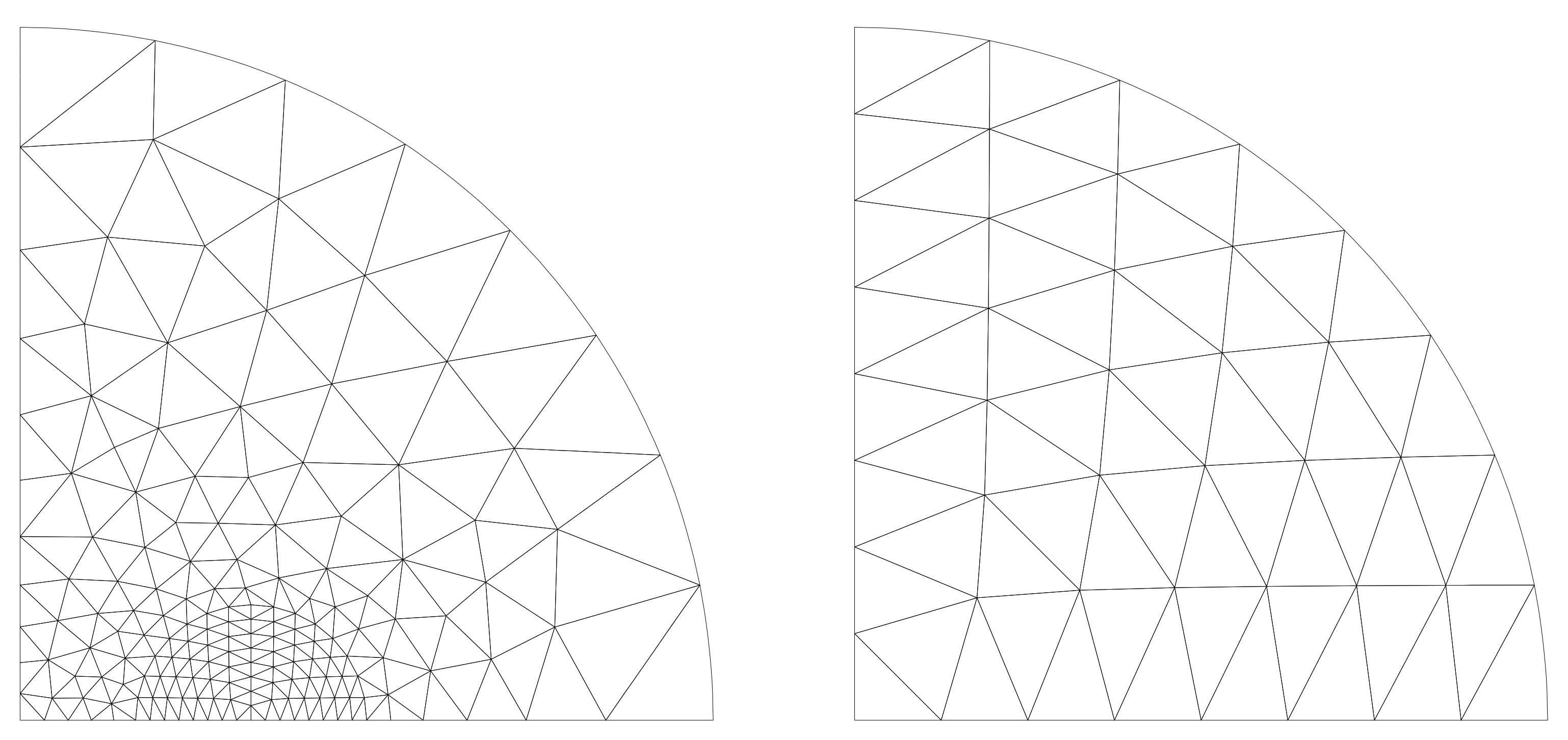
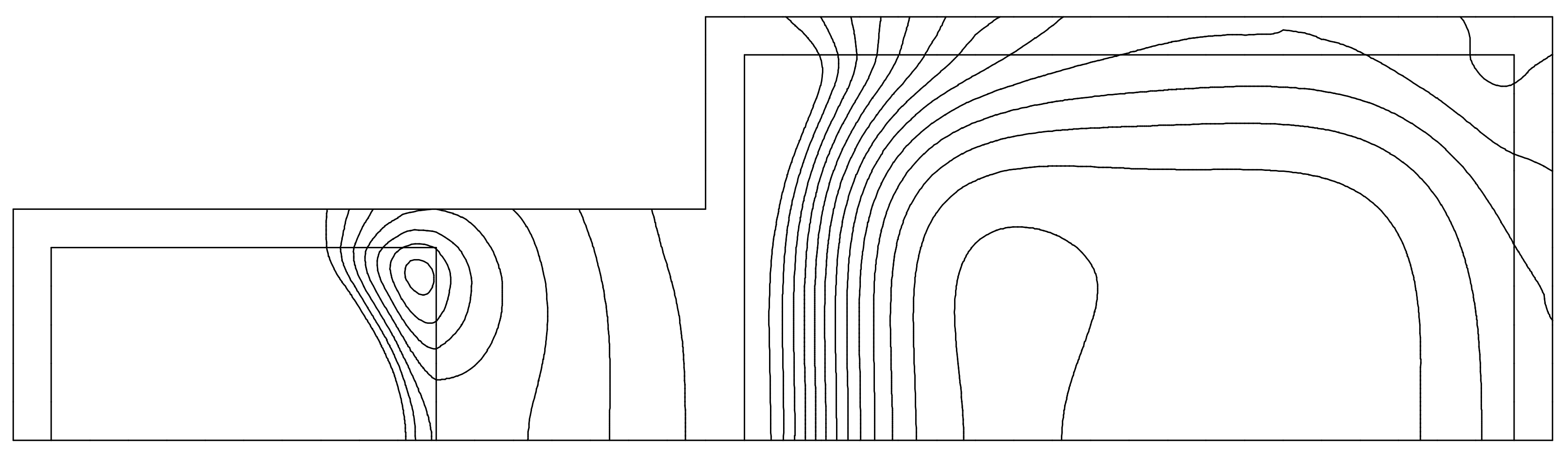
6. Numerical Examples
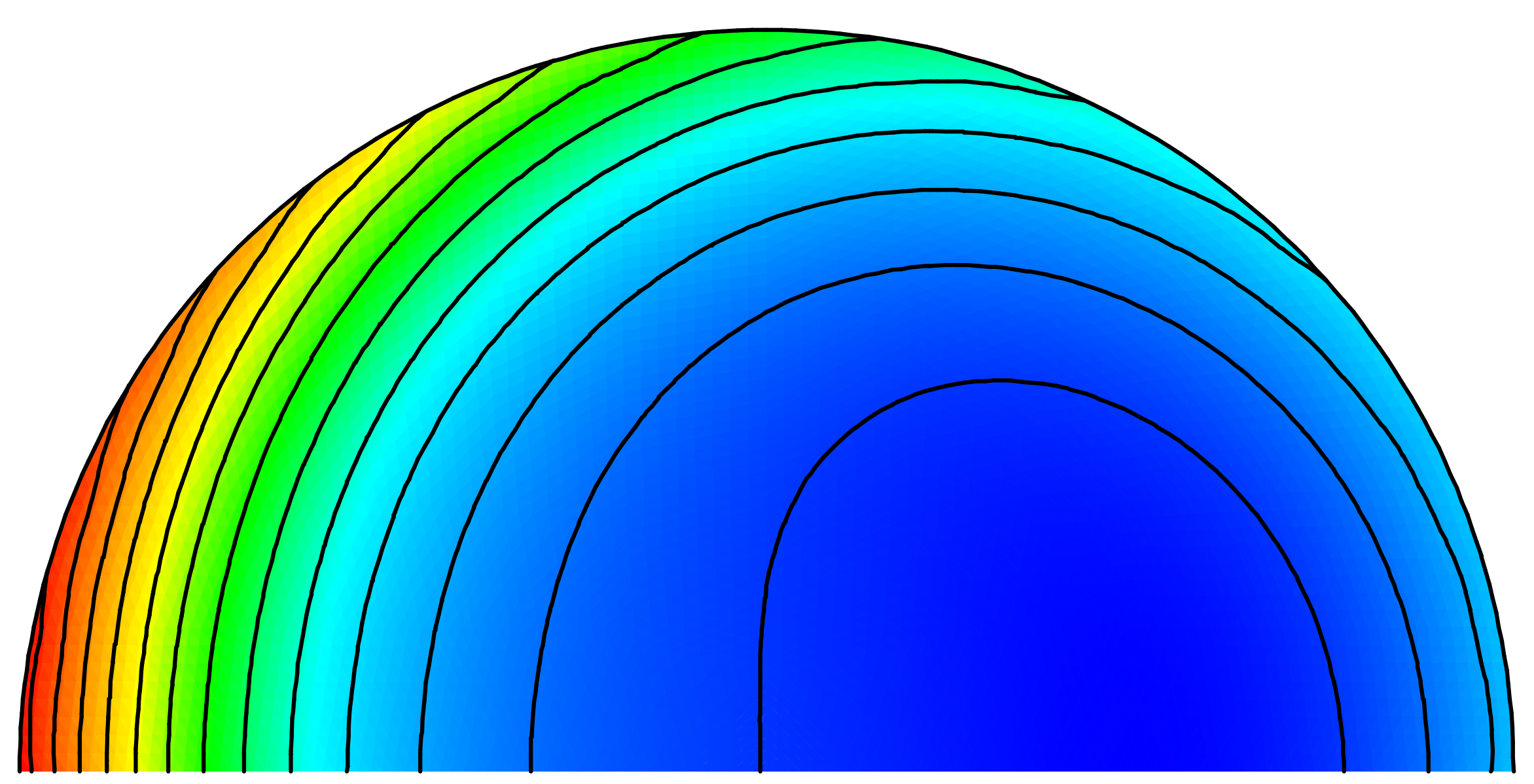
6.1. Capacitance of a Strip Line
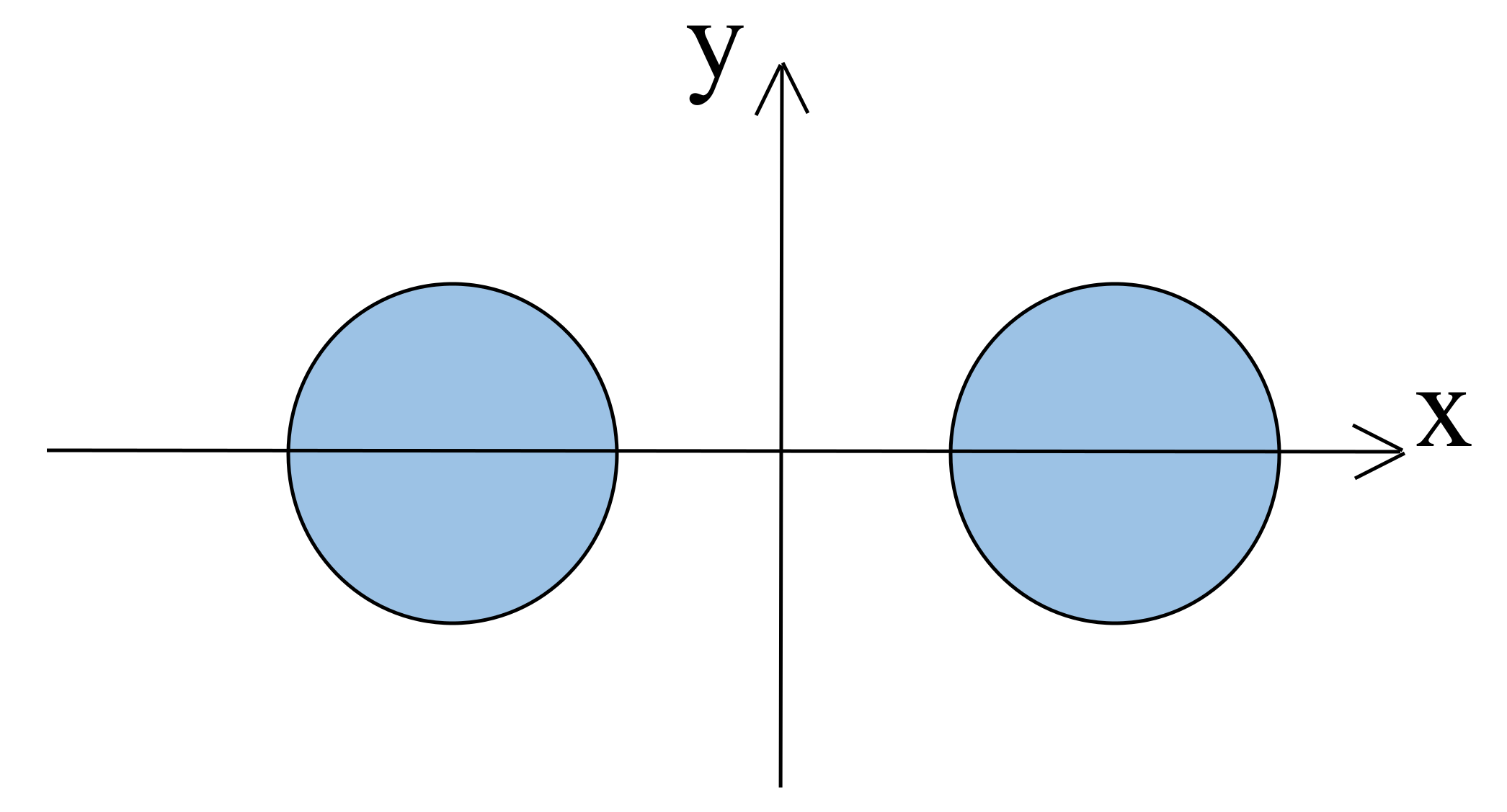
6.2. Two-Wire Transmission Line
6.3. Capacitance of a Cube Conductor
6.4. Skin Effect in a Two Wire Transmission Line
6.5. Skin Effect in a Non-Symmetric Transmission Line
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Silvester, P.P.; Ferrari, R.L. Finite Elements for Electrical Engineers; Cambridge University Press: Cambridge, UK, 1996. [Google Scholar]
- Jin, J. The Finite Element Method in Electromagnetics; John Wiley & Sons: New York, NY, USA, 2002. [Google Scholar]
- Chen, Q.; Konrad, A. A review of finite element open boundary techniques for static and quasi-static electromagnetic field problems. IEEE Trans. Magn. 1997, 33, 663–676. [Google Scholar] [CrossRef]
- Lowther, D.A.; Freeman, E.M.; Forghani, B. A sparse matrix open boundary method for finite element analysis. IEEE Trans. Magn. 1989, 25, 2810–2812. [Google Scholar] [CrossRef]
- Imhoff, J.F.; Meunier, G.; Sabonnadiere, J.C. Finite element modeling of open boundary problems. IEEE Trans. Magn. 1990, 26, 588–591. [Google Scholar] [CrossRef]
- Bettess, P. Infinite element. Int. J. Numer. Methods Eng. 1977, 11, 53–64. [Google Scholar] [CrossRef]
- Chen, Q.; Konrad, A.; Biringer, P.P. Computation of three-dimensional unbounded eddy current problems using asymptotic boundary conditions. IEEE Trans. Magn. 1995, 31, 1348–1351. [Google Scholar] [CrossRef]
- Engquist, B.; Majda, A. Absorbing boundary conditions for the numerical simulation of waves. Math. Comput. 1977, 31, 629–651. [Google Scholar] [CrossRef]
- Bayliss, A.; Gunzburger, M.; Turkel, E. Boundary conditions for the numerical solution of elliptic equations in exterior regions. SIAM J. Appl. Math. 1982, 42, 430–451. [Google Scholar] [CrossRef]
- Berenger, J.-P. A perfectly matched layer for the absorption of electromagnetic waves. J. Comput. Phys. 1994, 114, 185–200. [Google Scholar] [CrossRef]
- Aiello, G.; Alfonzetti, S.; Coco, S. Charge iteration: A procedure for the finite element computation of unbounded electrical fields. Int. J. Numer. Methods Eng. 1994, 37, 4147–4166. [Google Scholar] [CrossRef]
- Aiello, G.; Alfonzetti, S.; Borzì, G. A generalized minimal residual acceleration of the charge iteration procedure. J. Phys. III 1997, 7, 1955–1966. [Google Scholar] [CrossRef]
- Aiello, G.; Alfonzetti, S.; Rizzo, S.A.; Salerno, N. FEM-DBCI solution of open-boundary electrostatic problems in the presence of floating potential conductors. IEEE Trans. Magn. 2015, 52, 1–4. [Google Scholar] [CrossRef]
- Aiello, G.; Alfonzetti, S.; Coco, S.; Salerno, N. Finite element iterative solution to skin effect problems in open boundaries. Int. J. Numer. Model. 1996, 9, 125–143. [Google Scholar] [CrossRef]
- Aiello, G.; Alfonzetti, S.; Borzì, G.; Salerno, N. An improved solution scheme for open-boundary skin effect problems. IEEE Trans. Magn. 2001, 37, 3474–3477. [Google Scholar] [CrossRef]
- Aiello, G.; Alfonzetti, S.; Dilettoso, E. Finite element solution of eddy current problems in unbounded domains by means of the hybrid FEM−DBCI method. IEEE Trans. Magn. 2003, 39, 1409–1412. [Google Scholar] [CrossRef]
- Aiello, G.; Alfonzetti, S.; Salerno, N. Solution of skin-effect problems by means of the hybrid SDBCI method. COMPEL 2014, 33, 1935–1949. [Google Scholar] [CrossRef][Green Version]
- Aiello, G.; Alfonzetti, S.; Rizzo, S.A.; Salerno, N. Eddy Current Computation by the FEM-SDBCI Method. IEEE Trans. Magn. 2015, 52, 1–4. [Google Scholar] [CrossRef]
- Brebbia, C.A.; Telles, J.C.F.; Wrobel, L. Boundary Element Technique; Springer: Berlin/Heidelberg, Germany, 1984. [Google Scholar]
- Alfonzetti, S.; Salerno, N. A non-standard family of boundary elements for the hybrid FEM-BEM method. IEEE Trans. Magn. 2009, 45, 1312–1315. [Google Scholar] [CrossRef]
- Yuan, X.; Lynch, D.R.; Strohbehn, J.W. Coupling the finite element and moment methods for electromagnetic scattering from inhomogeneous objects. IEEE Trans. Antennas Propag. 1990, 38, 386–393. [Google Scholar] [CrossRef]
- Alfonzetti, S.; Borzì, G.; Salerno, N. Iteratively-improved Robin boundary conditions for the finite element solution of scattering problems in unbounded domains. Int. J. Numer. Methods Eng. 1998, 42, 601–629. [Google Scholar] [CrossRef]
- Alfonzetti, S.; Borzì, G. Finite element solution to electromagnetic scattering problems by means of the Robin boundary condition iteration method. IEEE Trans. Antennas Propag. 2002, 50, 132–140. [Google Scholar] [CrossRef]
- Alfonzetti, S.; Borzì, G.; Salerno, N. Electromagnetic scattering computation by means of the hybrid FEM-SRBCI method. IEEE Trans. Magn. 2015, 51, 7401504. [Google Scholar] [CrossRef]
- Liu, J.; Jin, J.M. A novel hybridization of higher order finite element and boundary integral methods for electromagnetic scattering. IEEE Trans. Antennas Propag. 2001, 50, 132–140. [Google Scholar]
- Aiello, G.; Alfonzetti, S.; Dilettoso, E.; Salerno, N. Improving the accuracy of the integral equation in the hybrid FEM-DBCI method for open boundary electrostatic problems. IEEE Trans. Magn. 2006, 42, 579–582. [Google Scholar] [CrossRef]
- Graglia, R.D. Static and dynamic potential integrals for linearly varying source distributions in two- and three-dimensional problems. IEEE Trans. Antennas Propag. 1987, 35, 662–669. [Google Scholar] [CrossRef]
- Konrad, A. Integrodifferential finite element formulation of two-dimensional steady-state skin effect problems. IEEE Trans. Magn. 1982, 18, 284–292. [Google Scholar] [CrossRef]
- Golub, G.H.; van Loan, C.F. Matrix Computations; Johns Hopkins University Press: Baltimore, MD, USA, 1996. [Google Scholar]
- Aiello, G.; Alfonzetti, S.; Coco, S.; Salerno, N. A theoretical study of charge iteration. COMPEL 1996, 15, 22–46. [Google Scholar] [CrossRef]
- Aiello, G.; Alfonzetti, S.; Coco, S.; Salerno, N. Overrelaxing the charge iteration procedure. IEEE Trans. Magn. 1996, 32, 694–697. [Google Scholar] [CrossRef]
- Saad, Y.; Schultz, M.H. GMRES: A generalized minimal residual algorithm for solving nonsymmetric linear systems. SIAM J. Sci. Stat. Comput. 1986, 7, 856–869. [Google Scholar] [CrossRef]
- Aiello, G.; Alfonzetti, S.; Salerno, N. Solution of skin effect problems by means of the DBCI-GMRES method. COMPEL 2002, 21, 474–475. [Google Scholar]
- Aiello, G.; Alfonzetti, S.; Borzì, G.; Dilettoso, E.; Salerno, N. A GMRES iterative solution of FEM-BEM global systems in skin effect problems. COMPEL 2008, 27, 1286–1295. [Google Scholar] [CrossRef]
- Aiello, G.; Alfonzetti, S.; Borzì, G.; Rizzo, S.A.; Salerno, N. A comparison between hybrid methods: FEM-BEM versus FEM-DBCI. COMPEL 2013, 32, 1900–1920. [Google Scholar] [CrossRef]
- Durand, E. Electrostatique; Masson Editeur: Paris, France, 1968. [Google Scholar]
- Mascagni, M.; Simonov, N.A. The random walk on the boundary method for calculating capacitance. J. Comput. Phys. 2004, 195, 465–473. [Google Scholar] [CrossRef]
- Alfonzetti, S.; Dilettoso, E.; Salerno, N. Simulated annealing with restarts for the optimization of electromagnetic devices. IEEE Trans. Magn. 2006, 42, 1115–1118. [Google Scholar] [CrossRef]
- Aiello, G.; Rizzo, S.A.; Alfonzetti, S.; Salerno, N. Optimization of the shape of an induction heating device in the presence of skin effect in the coils. COMPEL 2019, 39, 525–531. [Google Scholar] [CrossRef]
- Rokhlin, V. Rapid solution of integral equations of classical potential theory. J. Comput. Phys. 1985, 60, 187–207. [Google Scholar] [CrossRef]
- Engheta, N.; Murphy, W.D.; Rokhlin, V.; Vassiliou, M. The fast multipole method for electromagnetic scattering computation. IEEE Trans. Antennas Propag. 1992, 40, 634–641. [Google Scholar] [CrossRef]
- Hackbusch, W. Hierarchical Matrices: Algorithms and Analysis; Springer Series in Computational Mathematics; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar] [CrossRef]
- Aiello, G.; Alfonzetti, S.; Borzì, G.; Salerno, N. An overview of the ELFIN code for finite element research in electrical engineering. In Software for Electrical Engineering Analysis and Design; Konrad, A., Brebbia, C.A., Eds.; WIT Press: Southampton, UK, 1999. [Google Scholar]







| Dirichet | Neumann | Robin | |
|---|---|---|---|
| ΓT ≠ ΓIE | FEM-DBCI (LF) | – | FEM-RBCI FEM-AABC (HF) |
| ΓT ≡ ΓIE | FEM-SDBCI (LF) | FEM-BEM FEM-MoM (LF) (HF) | FEM-SRBCI (HF) |
| Method | GMRES Steps | CPU Time | Error Indicator ζ | Capacitance c/ε0 |
|---|---|---|---|---|
| FEM-DBCI (a) | 6 | 1.00 | 7.45 × 10−3 | 5.048423 |
| FEM-DBCI (b) | 6 | 1.02 | 6.71 × 10−3 | 5.047634 |
| FEM-DBCI (c) | 6 | 1.02 | 6.82 × 10−3 | 5.047570 |
| FEM-BEM | 8 | 2.01 | 2.19 × 10−3 | 5.047849 |
| Method | GMRES Steps | CPU Time (ms) | Normalized Capacitance |
|---|---|---|---|
| FEM-DBCI | 4 | 161.7 | 0.662214 |
| FEM-BEM | 18 | 1421.9 | 0.661590 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aiello, G.; Alfonzetti, S.; Rizzo, S.A.; Salerno, N. The Hybrid FEM-DBCI for the Solution of Open-Boundary Low-Frequency Problems. Mathematics 2021, 9, 1968. https://doi.org/10.3390/math9161968
Aiello G, Alfonzetti S, Rizzo SA, Salerno N. The Hybrid FEM-DBCI for the Solution of Open-Boundary Low-Frequency Problems. Mathematics. 2021; 9(16):1968. https://doi.org/10.3390/math9161968
Chicago/Turabian StyleAiello, Giovanni, Salvatore Alfonzetti, Santi Agatino Rizzo, and Nunzio Salerno. 2021. "The Hybrid FEM-DBCI for the Solution of Open-Boundary Low-Frequency Problems" Mathematics 9, no. 16: 1968. https://doi.org/10.3390/math9161968
APA StyleAiello, G., Alfonzetti, S., Rizzo, S. A., & Salerno, N. (2021). The Hybrid FEM-DBCI for the Solution of Open-Boundary Low-Frequency Problems. Mathematics, 9(16), 1968. https://doi.org/10.3390/math9161968









