RETRACTED: Resolving Indeterminacy Approach to Solve Multi-Criteria Zero-Sum Matrix Games with Intuitionistic Fuzzy Goals
Abstract
1. Introduction
1.1. Background
1.2. Literature Review
1.3. The Contribution and Structure of This article
2. Preliminaries
- (1)
- (2)
- (3)
- (4)
- if and only if and
- (1)
- (2)
- (3)
3. Zero-Sum Multi-Criteria Matrix Games
4. Application of IFS to Optimization Problem
4.1. Decision Making Approaches with I-Fuzzy Environment
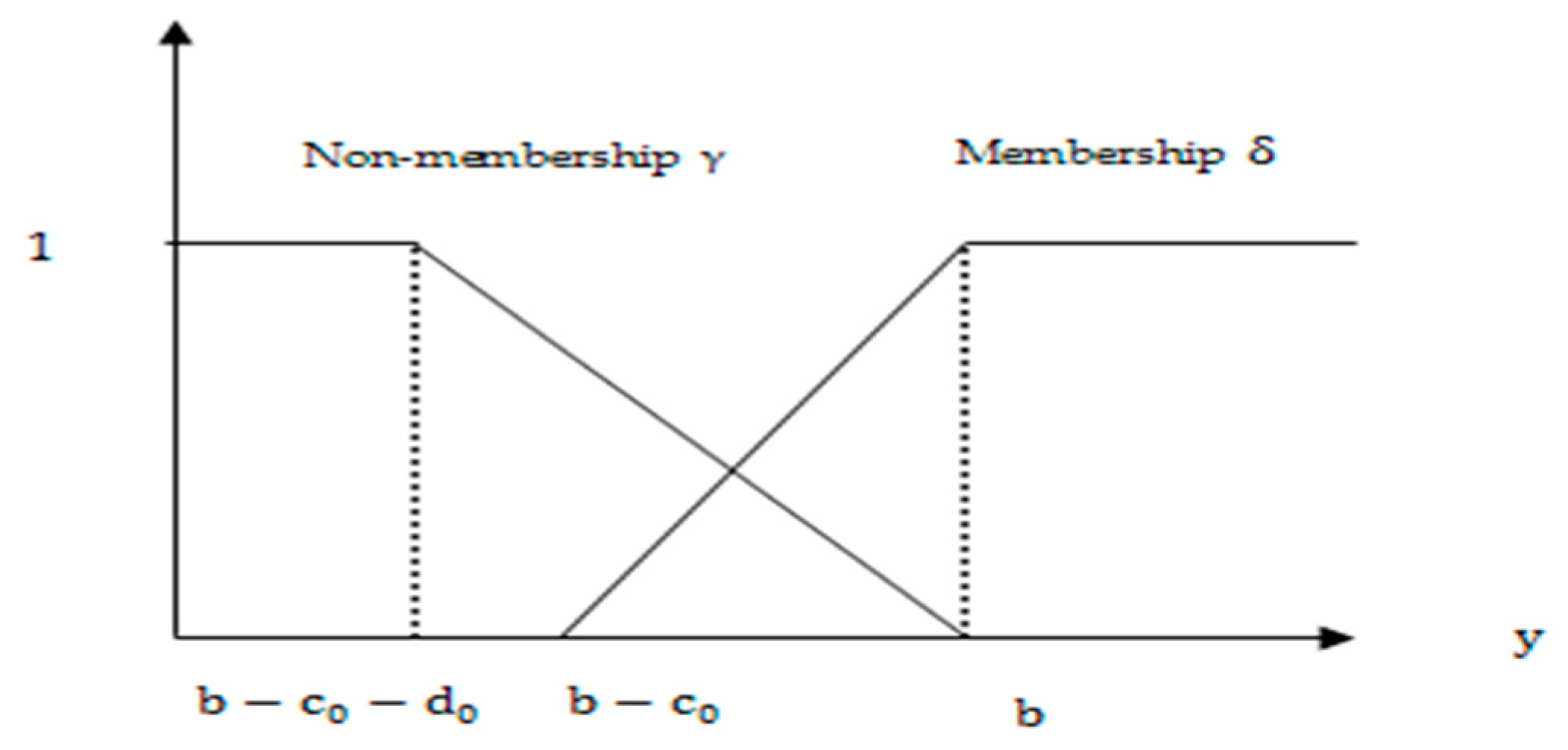
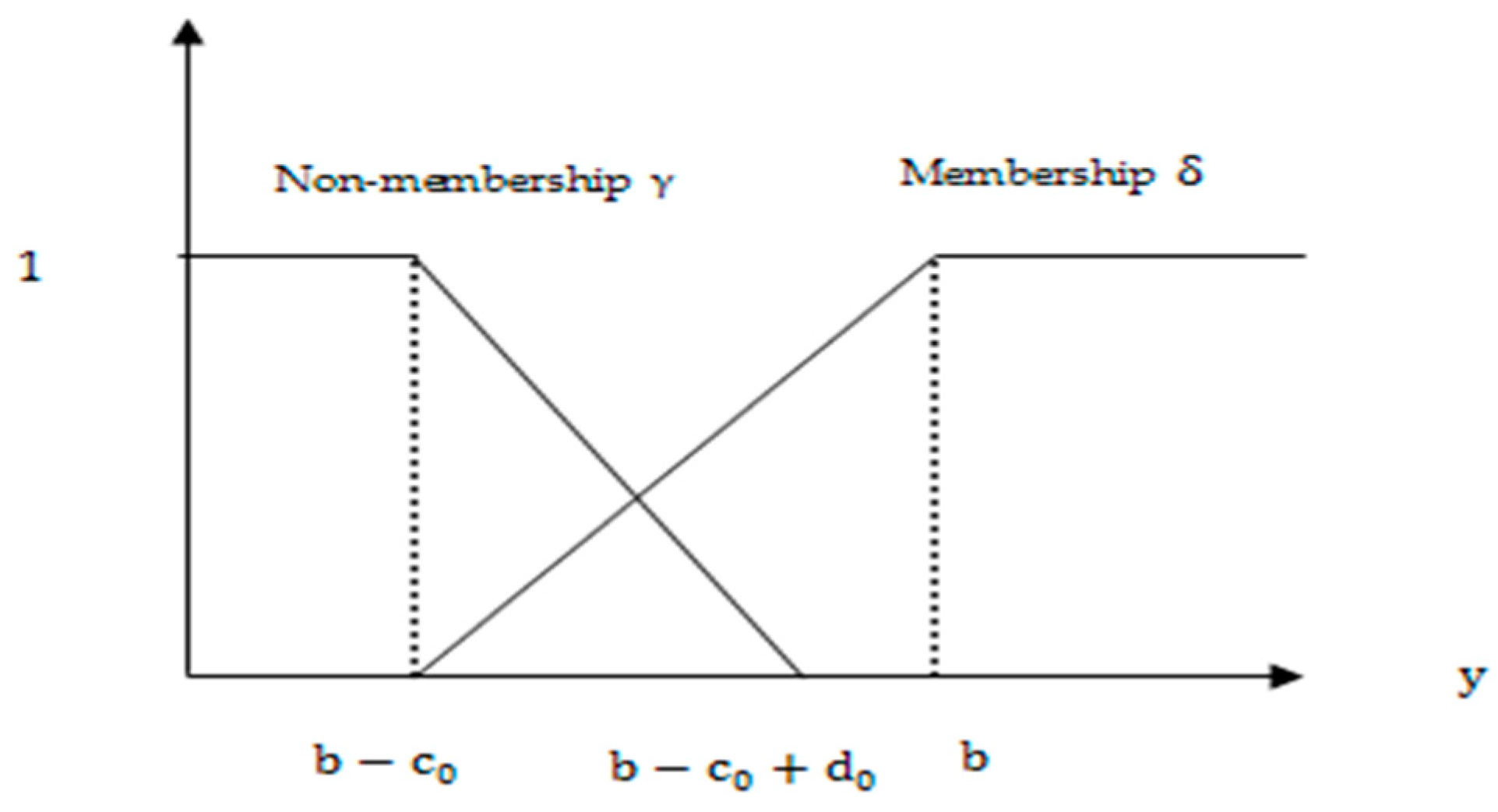
4.2. Interpretation of I-Fuzzy Inequalities
5. Mathematical Model of Multi-Criteria Zero-Sum Matrix Games with I-Fuzzy Goals
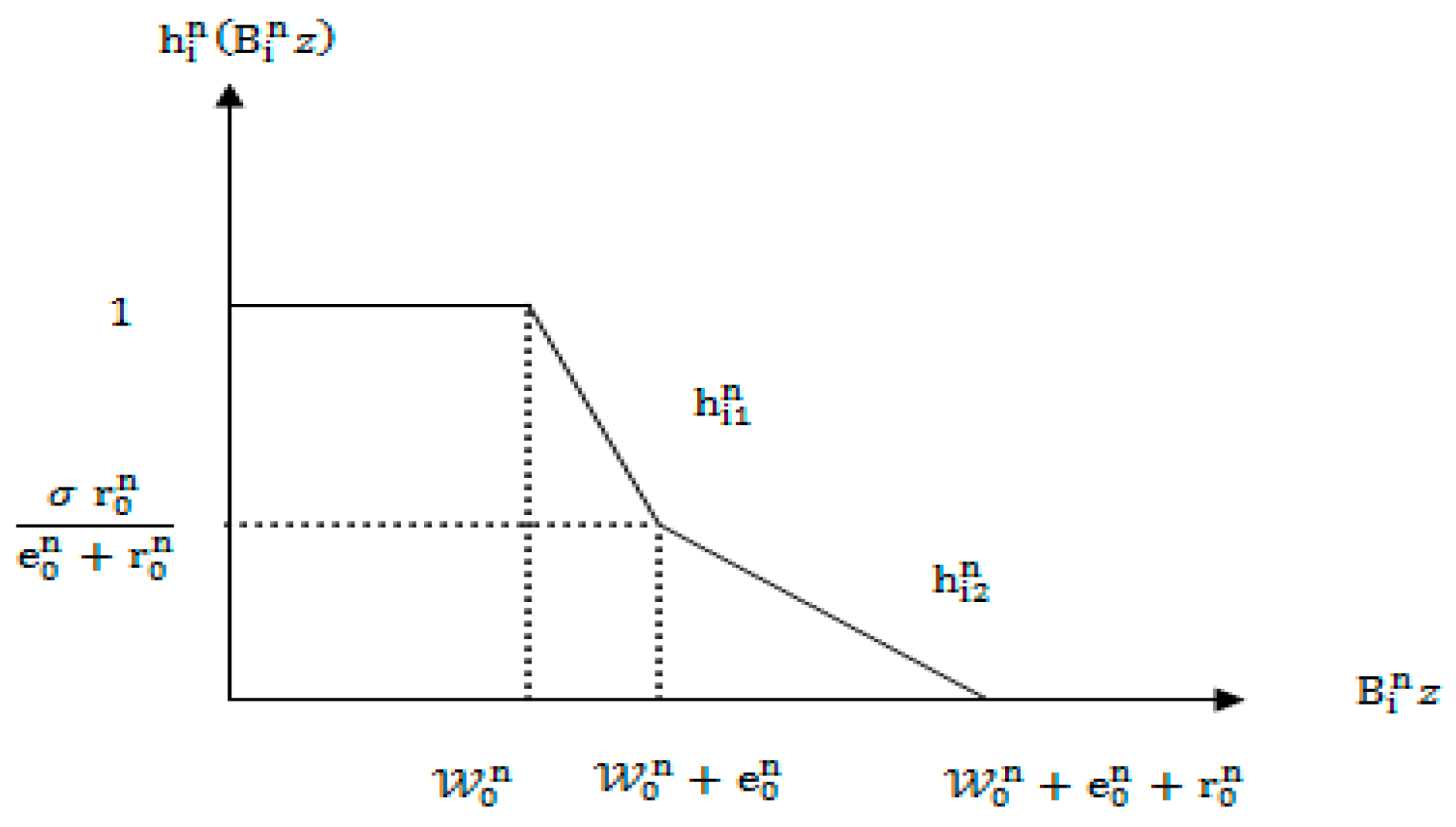
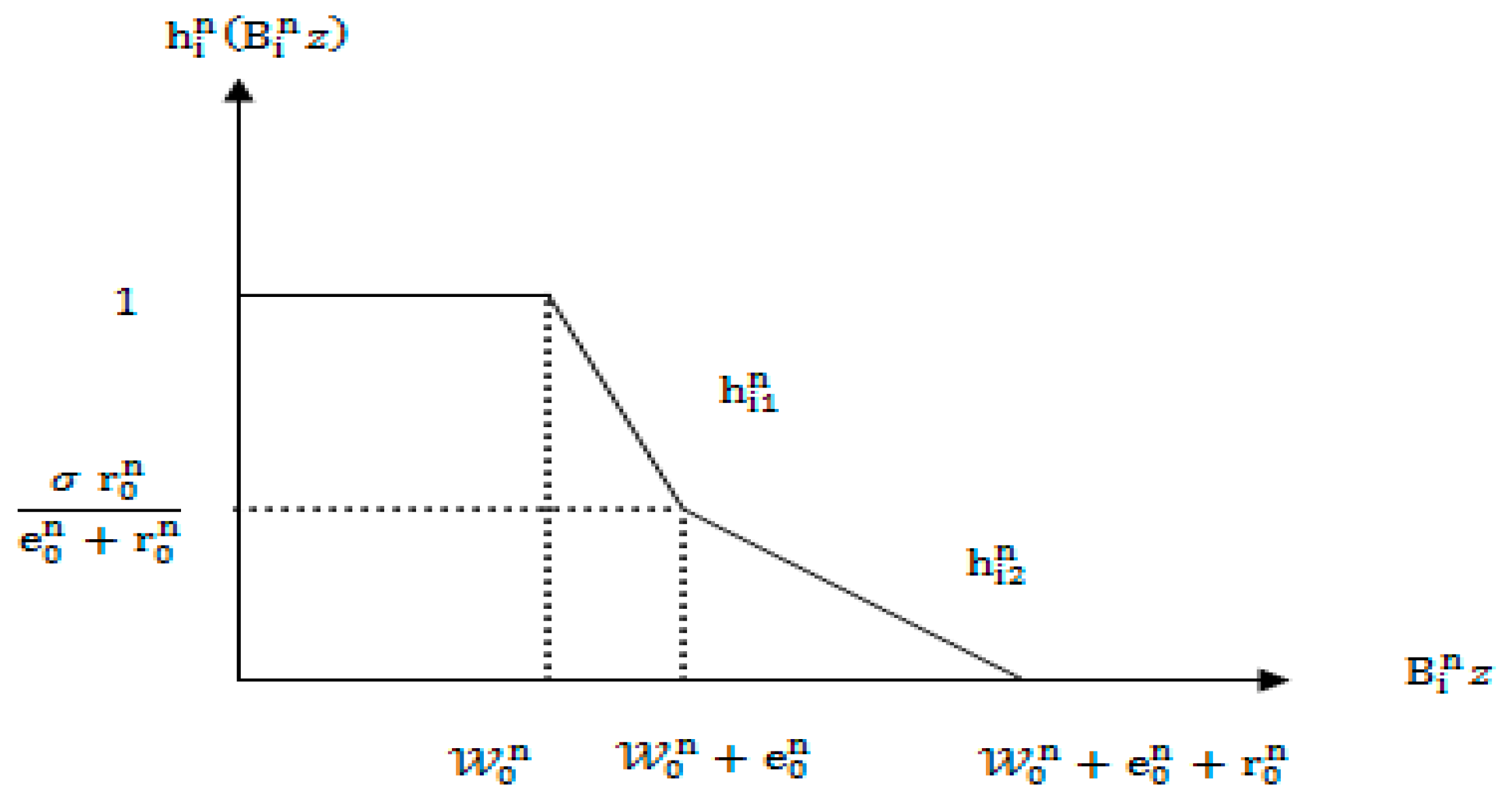
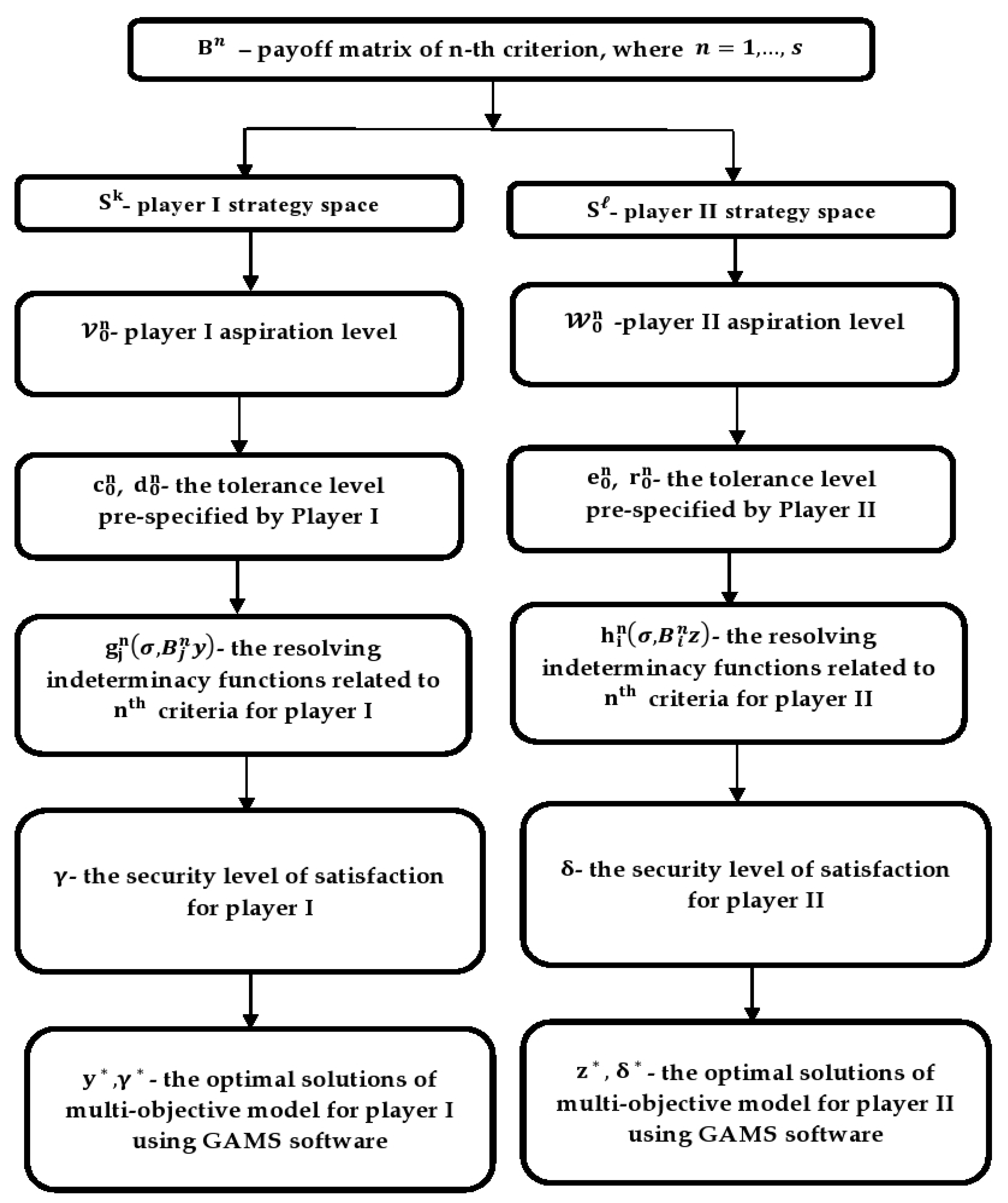
6. Resolving Indeterminacy Approach
6.1. Optimistic Approach
6.1.1. Optimization Model for Player I
6.1.2. Optimization Model for Player II
6.2. Pessimistic Approach
6.2.1. Optimization Model for Player I
6.2.2. Optimization Model for Player II
7. Numerical Examples and Computational Result Comparison
7.1. Example 1
7.1.1. Optimistic Approach
7.1.2. Pessimistic Approach
7.2. Example 2
7.2.1. Optimistic Approach
7.2.2. Pessimistic Approach
7.3. Results and Discussion
- The proposed approach is based on IFS, and it is evident that IFS suitably reflects the hesitation and uncertainty of human thinking; so, it provides more flexibility to the decision makers while expressing their decision.
- By comparing our results, as in Table 5, Table 6, Table 7 and Table 8, with Nishizaki et al. [10] and Aggarwal et al. [41], as in Table 9, Table 10 and Table 11, it can be easily seen that our proposed model produces much better optimal strategies with higher securities as, for player I, in our model, as opposed to and in case of Nishizaki’s and Aggarwal’s, respectively. Additionally, for player II, in our model, but and in Nishizaki and Aggarwal models, respectively.
8. Conclusions and Future Work
- Outlining the arithmetic operations and indeterminacy resolving functions of Atanassov’s I-fuzzy number.
- Proposing an effective algorithm based on the indeterminacy resolving function, I-fuzzy inequality relations, and Inuiguchi et al [42] algorithm.
- Constructing crisp models from the proposed I-fuzzy models.
- Solving the reduced crisp multi-objective linear programming models using the GAMS software [43].
- Conducting two numerical simulations to evaluate the applicability and effectiveness of the proposed approach.
- The numerical results confirm that the IFS outperform fuzzy set when studying uncertainty in game theory.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
- Step 1:
- Suppose the break points are .Compute , and and give them as , and , respectively, for . Then, , and , for .
- Step 2:
- Let and then obtain the value of .
- Step 3:
- Calculate and
- Step 4:
- For , and m = 1,2, findandThen compute
Appendix B
- Step 1:
- Suppose the break points areCompute , and and give them as , and , respectively, for . Then, , and , for
- Step 2:
- Let and then obtain the value of
- Step 3:
- Calculate and
- Step 4:
- For , and m = 1,2, findandThen compute
Appendix C
- Step 1:
- We haveand
- Step 2:
- Set and compute
- Step 3:
- Normalizing we obtain
- Step 4:
- Then for , and m = 1,2and
Appendix D
- Step 1:
- We haveand
- Step 2:
- Set and compute
- Step 3:
- Normalizing we obtain
- Step 4:
- Then for , and m = 1,2and
References
- Von Neumann, J.; Morgenstern, D. The Theory of Games in Economic Behavior; Princeton University Press: Princeton, NJ, USA, 1944; pp. 1–625. [Google Scholar]
- Owen, G. Game Theory, 2nd ed.; Academic Press: New York, NY, USA, 1982. [Google Scholar]
- Nash, J. Non-Cooperative Games. Ann. Math. 1951, 54, 286–295. [Google Scholar] [CrossRef]
- Blackwell, D. An Analog of the Minimax Theorem for Vector Payoffs. Pac. J. Math. 1956, 6, 1–8. [Google Scholar] [CrossRef]
- Cook, W.D. Zero-Sum Games with Multiple Goals. Nav. Res. Logist. Q. 1976, 23, 615–622. [Google Scholar] [CrossRef]
- Kumar, S. Max-Min Solution Approach for Multiobjective Matrix Game with Fuzzy Goals. Yugosl. J. Oper. Res. 2016, 26, 51–60. [Google Scholar] [CrossRef]
- Zeleny, M. Games with Multiple Payos. Int. J. Game Theory 1975, 4, 179–191. [Google Scholar] [CrossRef]
- Fernandez, F.R.; Puerto, J. Vector Linear Programming in Zero-Sum Multicriteria Matrix Games. J. Optim. Theory Appl. 1996, 89, 115–127. [Google Scholar] [CrossRef]
- Ghose, D.; Prasad, U.R. Solution Concepts in Two-Person Multicriteria Games. J. Optim. Theory Appl. 1989, 63, 167–189. [Google Scholar] [CrossRef]
- Nishizaki, I.; Sakawa, M. Fuzzy and Multiobjective Games for Conflict Resolution. Physica Verlag 2001, 64, 1–258. [Google Scholar]
- Corley, S.C. Games with Vector Payoffs. J. Optim. Theory Appl. 1985, 47, 463–475. [Google Scholar] [CrossRef]
- Fahem, K.; Radjef, M.S. Properly Efficient Nash Equilibrium in Multicriteria Noncooperative Games. Math. Methods Oper. Res. 2015, 82, 175–193. [Google Scholar] [CrossRef]
- Shapely, L.S. Equilibirum Points in Games with Vector Payoff. Nav. Res. Logist. Q. 1959, 6, 57–61. [Google Scholar] [CrossRef]
- Atanassov, K. Intuitionistic Fuzzy Sets. Fuzzy Sets Syst. 1986, 20, 87–96. [Google Scholar] [CrossRef]
- Atanassov, K. Intuitionistic Fuzzy Sets. Physica Heidelberg 1999, 1–137. [Google Scholar]
- De, S.K.; Biswas, R.; Roy, A.R. An Application of Intuitionistic Fuzzy Sets in Medical Diagnosis. Fuzzy Sets Syst. 2001, 117, 209–213. [Google Scholar] [CrossRef]
- Tyagi, S.K.; Akram, M. Human Reliability Evaluation for Offshore Platform Musters Using Intuitionistic Fuzzy Sets. IEEE Trans. Fuzzy Syst. 2013, 21, 1115–1122. [Google Scholar] [CrossRef]
- Li, D.F.; Nan, J.X. A Nonlinear Programming Approach to Matrix Games with Payoffs of Atanassov’s Intuitionistic Fuzzy Sets. Int. J. Uncertain. Fuzziness Knowl. Based Syst. 2009, 17, 585–607. [Google Scholar] [CrossRef]
- Nan, J.X.; Li, D.F.; Zhang, M.J. A Lexicographic Method for Matrix Games with Pay-Offs of Triangular Intuitionistic Fuzzy Numbers. Int. J. Comput. Intell. Syst. 2010, 3, 280–289. [Google Scholar] [CrossRef]
- Seikh, M.R.; Nayak, P.K.; Pal, M. Matrix Games in Intuitionistic Fuzzy Environment. Int. J. Math. Oper. Res. 2013, 5, 693–708. [Google Scholar] [CrossRef]
- Bandyopadhyay, S.; Nayak, P.K.; Pal, M. Solution of Matrix Game with Triangular Intuitionistic Fuzzy Pay-off Using Score Function. Open J. Optim. 2013, 2, 9–15. [Google Scholar] [CrossRef]
- Aggarwal, A.; Mehra, A.; Chandra, S. Application of Linear Programming with I-Fuzzy Sets to Matrix Games with I-Fuzzy Goals. Fuzzy Optim. Decis. Mak 2012, 11, 465–480. [Google Scholar] [CrossRef]
- Li, D.F.; Nan, J.X.; Tang, Z.P.; Chen, K.J.; Xiang, X.D.; Hong, F.X. A Bi-Objective Programming Approach to Solve Matrix Games with Payoffs of Atanassov’s Triangular Intuitionistic Fuzzy Numbers. Iran. J. Fuzzy Syst. 2012, 9, 93–110. [Google Scholar]
- Nayak, P.K.; Pal, M. Intuitionistic Fuzzy Optimization Technique for Nash Equilibrium Solution of Multi-Objective Bi-Matrix Game. J. Uncertain Syst. 2011, 5, 271–285. [Google Scholar]
- Jana, J.; Roy, S.K. Solution of Matrix Games with Generalised Trapezoidal Fuzzy Payoffs. Fuzzy Inf. Eng. 2018, 10, 213–224. [Google Scholar] [CrossRef]
- Nan, J.X.; Zhang, M.J.; Li, D.F. A Methodology for Matrix Games with Payoffs of Triangular Intuitionistic Fuzzy Number. J. Intell. Fuzzy Syst. 2014, 26, 2899–2912. [Google Scholar] [CrossRef]
- Seikh, R.; Nayak, P.K.; Pal, M. Matrix Games with Intuitionistic Fuzzy Pay-Offs. J. Inf. Optim. Sci. 2015, 36, 159–181. [Google Scholar] [CrossRef]
- Nan, J.; Li, D.; Zhang, M. The Linear Programming Approach to Matrix Games with Payoffs of Intuitionistic Fuzzy Sets. In Proceedings of the 2009 Second International Workshop on Computer Science and Engineering, Qingdao, China, 28–30 October 2009; pp. 603–607. [Google Scholar]
- Li, D. Decision and Game Theory in Management with Intuitionistic Fuzzy Sets; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Nan, J.X.; Zhang, M.J.; Li, D. Intuitionistic Fuzzy Programming Models for Matrix Games with Payoffs of Trapezoidal Intuitionistic Fuzzy Numbers. Int. J. Fuzzy Syst. 2014, 16, 444–456. [Google Scholar]
- Verma, T.; Kumar, A. A Note on a Methodology for Matrix Games with Payoffs of Triangular Intuitionistic Fuzzy Number. J. Intell. Fuzzy Syst. 2014, 27, 1689–1691. [Google Scholar] [CrossRef]
- Xing, Y.; Qiu, D. Solving Triangular Intuitionistic Fuzzy Matrix Game by Applying the Accuracy Function Method. Symmetry 2019, 11, 1258. [Google Scholar] [CrossRef]
- Verma, T.; Kumar, A.; Kacprzyk, J. A Novel Approach to the Solution of Matrix Games with Payoffs Expressed by Trapezoidal Intuitionistic Fuzzy Numbers. J. Autom. Mob. Robot. Intell. Syst. 2015, 9, 25–46. [Google Scholar] [CrossRef]
- Roy, S.K.; Bhaumik, A. Intelligent Water Management: A Triangular Type-2 Intuitionistic Fuzzy Matrix Games Approach. Water Resour. Manag. 2018, 32, 949–968. [Google Scholar] [CrossRef]
- Jana, J.; Roy, S.K. Dual Hesitant Fuzzy Matrix Games: Based on New Similarity Measure. Soft Comput. 2018, 23, 8873–8886. [Google Scholar] [CrossRef]
- Yu, D.; Li, D.-F.; Merigo, J.M. Dual Hesitant Fuzzy Group Decision Making Method and Its Application to Supplier Selection. Int. J. Mach. Learn. Cybern. 2016, 7, 819–831. [Google Scholar] [CrossRef]
- Liu, D.; Chen, X.; Peng, D. Cosine Distance Measure between Neutrosophic Hesitant Fuzzy Linguistic Sets and Its Application in Multiple Criteria Decision Making. Symmetry 2018, 10, 602. [Google Scholar] [CrossRef]
- Faizi, S.; Sałabun, W.; Rashid, T.; Wątróbski, J.; Zafar, S. Group Decision-Making for Hesitant Fuzzy Sets Based on Characteristic Objects Method. Symmetry 2017, 9, 136. [Google Scholar] [CrossRef]
- Joshi, D.K.; Beg, I.; Kumar, S. Hesitant Probabilistic Fuzzy Linguistic Sets with Applications in Multi-Criteria Group Decision Making Problems. Mathematics 2018, 6, 47. [Google Scholar] [CrossRef]
- Singh, S.; Garg, H. Symmetric Triangular Interval Type-2 Intuitionistic Fuzzy Sets with Their Applications in Multi Criteria Decision Making. Symmetry 2018, 10, 401. [Google Scholar] [CrossRef]
- Aggarwal, A.; Khan, I. Solving Multi-Objective Fuzzy Matrix Games via Multi-Objective Linear Programming Approach. Kybernetika 2016, 52, 153–168. [Google Scholar] [CrossRef]
- Inuiguchi, M.; Ichihashi, H.; Kume, Y. A Solution Algorithm for Fuzzy Linear Programming with Piecewise Linear Membership Functions. Fuzzy Sets Syst. 1990, 34, 15–31. [Google Scholar] [CrossRef]
- Mavrotas, G. Generation of Efficient Solutions in Multiobjective Mathematical Programming Problems Using GAMS. Tech. Rep. 2007, 167–189. [Google Scholar]
- Brikaa, M.G.; Zheng, Z.; Ammar, E.-S. Fuzzy Multi-Objective Programming Approach for Constrained Matrix Games with Payoffs of Fuzzy Rough Numbers. Symmetry 2019, 11, 702. [Google Scholar] [CrossRef]
- Li, D.F. A Ratio Ranking Method of Triangular Intuitionistic Fuzzy Numbers and Its Application to MADM Problems. Comput. Math. Appl. 2010, 60, 1557–1570. [Google Scholar] [CrossRef]
- Khan, I.; Aggarwal, A.; Mehra, A. Solving I-Fuzzy Bi-Matrix Games with I-Fuzzy Goals by Resolving Indeterminacy. J. Uncertain Syst. 2016, 10, 204–222. [Google Scholar]
- Angelov, P.P. Optimization in an Intuitionistic Fuzzy Environment. Fuzzy Sets Syst. 1997, 86, 299–306. [Google Scholar] [CrossRef]
- Dubey, D.; Chandra, S.; Mehra, A. Fuzzy Linear Programming under Interval Uncertainity Based on IFS Representation. Fuzzy Set Syst. 2012, 188, 68–87. [Google Scholar] [CrossRef]
- Yager, R. Some Aspects of Intuitionistic Fuzzy Sets. Fuzzy Optim. Decis. Mak. 2009, 8, 67–90. [Google Scholar] [CrossRef]
- Yang, T.; Ignizio, J.P.; Kim, H.J. Fuzzy Programming with Nonlinear Membership Functions: Piecewise Linear Approximation. Fuzzy Sets Syst. 1991, 41, 39–53. [Google Scholar] [CrossRef]
- Campos, L. Fuzzy Linear Programming Models to Solve Fuzzy Matrix Games. Fuzzy Sets Syst. 1989, 32, 275–289. [Google Scholar] [CrossRef]


| # | ||||
|---|---|---|---|---|
| 1 | 0.7917 | 0.2083 | 0.6743 | 1 |
| 2 | 0.8147 | 0.1803 | 0.6626 | 1.0249 |
| 3 | 0.925 | 0.1443 | 0.6408 | 1.041 |
| 4 | 0.983 | 0.1163 | 0.6259 | 1.0695 |
| # | ||||
|---|---|---|---|---|
| 1 | 0.36 | 0.64 | 1 | 0.79 |
| 2 | 0.374 | 0.626 | 0.9883 | 0.811 |
| 3 | 0.388 | 0.612 | 0.976 | 0.832 |
| 4 | 0.402 | 0.598 | 0.965 | 0.853 |
| # | ||||
|---|---|---|---|---|
| 1 | 0.8316 | 0.3291 | 0.5529 | 1 |
| 2 | 0.941 | 0.3011 | 0.541 | 1.0359 |
| 3 | 0.962 | 0.2621 | 0.5157 | 1.0526 |
| 4 | 0.991 | 0.2331 | 0.4874 | 1.0955 |
| # | ||||
|---|---|---|---|---|
| 1 | 0.472 | 0.556 | 0.9447 | 0.9354 |
| 2 | 0.458 | 0.542 | 0.9355 | 0.9515 |
| 3 | 0.472 | 0.528 | 0.9263 | 0.9676 |
| 4 | 0.486 | 0.514 | 0.9171 | 0.9838 |
| # | ||||||
|---|---|---|---|---|---|---|
| 1 | 0.5724637 | 0.2463768 | 0.1811594 | 0.4331723 | 0.4161490 | 0.7741020 |
| 2 | 0.875 | 0.125 | 0.00058216 | 0.5138888 | 0.3125 | 0.7608695 |
| 3 | 0.8098214 | 0.125 | 0.0651785 | 0.4994047 | 0.3404336 | 0.7580357 |
| 4 | 0.7446428 | 0.125 | 0.1303571 | 0.4849206 | 0.3683673 | 0.7552018 |
| 5 | 0.6794642 | 0.125 | 0.1955357 | 0.4704365 | 0.396301 | 0.752368 |
| 6 | 0.6142857 | 0.125 | 0.2607142 | 0.4559523 | 0.4242346 | 0.749534 |
| # | ||||||
|---|---|---|---|---|---|---|
| 1 | 0.625 | 0.0000683 | 0.375 | 0.7410714 | 0.4910714 | 0.44375 |
| 2 | 0.6287389 | 0.0186948 | 0.3525662 | 0.7354629 | 0.50122 | 0.44375 |
| 3 | 0.6366255 | 0.0581276 | 0.3052468 | 0.7236331 | 0.5226264 | 0.44375 |
| 4 | 0.6445121 | 0.0975604 | 0.2579274 | 0.7118032 | 0.5440328 | 0.44375 |
| 5 | 0.6523986 | 0.1369933 | 0.210608 | 0.6999734 | 0.5654392 | 0.44375 |
| 6 | 0.6602852 | 0.1764261 | 0.1632886 | 0.6881435 | 0.5868456 | 0.44375 |
| # | ||||||
|---|---|---|---|---|---|---|
| 1 | 0.875 | 0.125 | 0.0000416 | 0.7265625 | 0.065625 | 0.6634615 |
| 2 | 0.8132227 | 0.125 | 0.0617772 | 0.7188403 | 0.130491 | 0.6579966 |
| 3 | 0.7514455 | 0.125 | 0.1235544 | 0.7111182 | 0.1953571 | 0.6525317 |
| 4 | 0.6896682 | 0.125 | 0.1853316 | 0.703396 | 0.2602232 | 0.6470668 |
| 5 | 0.6278911 | 0.125 | 0.2471088 | 0.6956738 | 0.3250892 | 0.6416019 |
| 6 | 0.5661139 | 0.125 | 0.308886 | 0.6879517 | 0.3899553 | 0.636137 |
| # | ||||||
|---|---|---|---|---|---|---|
| 1 | 0.625 | 0.00000629 | 0.375 | 0.6380208 | 0.503125 | 0.2060439 |
| 2 | 0.6301808 | 0.0259042 | 0.3439149 | 0.6221544 | 0.5375776 | 0.2060439 |
| 3 | 0.634845 | 0.0492253 | 0.3159295 | 0.6078703 | 0.5685947 | 0.2060439 |
| 4 | 0.6395092 | 0.0725464 | 0.2879442 | 0.5935861 | 0.5996118 | 0.2060439 |
| 5 | 0.6441735 | 0.0958675 | 0.2599589 | 0.5793019 | 0.6306288 | 0.2060439 |
| 6 | 0.6488377 | 0.1191886 | 0.2319735 | 0.5650177 | 0.6616459 | 0.2060439 |
| # | ||||||
|---|---|---|---|---|---|---|
| 1 | 0.875 | 0.125 | 0.0 | 0.4531 | 0.0375 | 0.5769 |
| 2 | 0.8098 | 0.125 | 0.0651 | 0.4368 | 0.0766 | 0.5719 |
| 3 | 0.7446 | 0.125 | 0.1303 | 0.4205 | 0.1157 | 0.5668 |
| 4 | 0.6794 | 0.125 | 0.1955 | 0.4042 | 0.1548 | 0.5618 |
| 5 | 0.6142 | 0.125 | 0.2607 | 0.3879 | 0.1939 | 0.5568 |
| 6 | 0.5491 | 0.125 | 0.3258 | 0.3716 | 0.2330 | 0.5518 |
| # | ||||||
|---|---|---|---|---|---|---|
| 1 | 0.625 | 0.0 | 0.375 | 0.5468 | 0.2875 | 0.1442 |
| 2 | 0.6299 | 0.0249 | 0.3451 | 0.5337 | 0.3064 | 0.1442 |
| 3 | 0.6349 | 0.0498 | 0.3152 | 0.5207 | 0.3253 | 0.1442 |
| 4 | 0.6399 | 0.0747 | 0.2853 | 0.5076 | 0.3442 | 0.1442 |
| 5 | 0.6449 | 0.0996 | 0.2554 | 0.4945 | 0.3632 | 0.1442 |
| 6 | 0.6499 | 0.1245 | 0.2255 | 0.4814 | 0.3821 | 0.1442 |
| Player I | Player II | ||||||
|---|---|---|---|---|---|---|---|
| 0.3860 | 0.1250 | 0.48897 | 0.33088 | 0.25595 | 0.3469 | 0.3972 | 0.5804 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Brikaa, M.G.; Zheng, Z.; Ammar, E.-S. RETRACTED: Resolving Indeterminacy Approach to Solve Multi-Criteria Zero-Sum Matrix Games with Intuitionistic Fuzzy Goals. Mathematics 2020, 8, 305. https://doi.org/10.3390/math8030305
Brikaa MG, Zheng Z, Ammar E-S. RETRACTED: Resolving Indeterminacy Approach to Solve Multi-Criteria Zero-Sum Matrix Games with Intuitionistic Fuzzy Goals. Mathematics. 2020; 8(3):305. https://doi.org/10.3390/math8030305
Chicago/Turabian StyleBrikaa, M. G., Zhoushun Zheng, and El-Saeed Ammar. 2020. "RETRACTED: Resolving Indeterminacy Approach to Solve Multi-Criteria Zero-Sum Matrix Games with Intuitionistic Fuzzy Goals" Mathematics 8, no. 3: 305. https://doi.org/10.3390/math8030305
APA StyleBrikaa, M. G., Zheng, Z., & Ammar, E.-S. (2020). RETRACTED: Resolving Indeterminacy Approach to Solve Multi-Criteria Zero-Sum Matrix Games with Intuitionistic Fuzzy Goals. Mathematics, 8(3), 305. https://doi.org/10.3390/math8030305





