Dominant Cubic Coefficients of the ‘1/3-Rule’ Reduce Contest Domains

Abstract

Antagonistic exploitation in competition with a cooperative strategy defines a social dilemma, whereby eventually overall fitness of the population decreases. Frequency-dependent selection between two non-mutating strategies in a Moran model of random genetic drift yields an evolutionary rule of biological game theory. When a singleton fixation probability of co-operation exceeds the selectively neutral value being the reciprocal of population size, its relative frequency in the population equilibrates to less than 1/3. Maclaurin series of a singleton type fixation probability function calculated at third order enables the convergent domain of the payoff matrix to be identified. Asymptotically dominant third order coefficients of payoff matrix entries were derived. Quantitative analysis illustrates non-negligibility of the quadratic and cubic coefficients in Maclaurin series with selection being inversely proportional to population size. Novel corollaries identify the domain of payoff matrix entries that determines polarity of second order terms, with either non-harmful or harmful contests. Violation of this evolutionary rule observed with non-harmful contests depends on the normalized payoff matrix entries and selection differential. Significant violations of the evolutionary rule were not observed with harmful contests.

1. Introduction

Selection between game strategies, on the order of magnitude as the reciprocal of population size, accords with partial differential equations, or diffusion theory, of population genetics [1,2,3,4]. Cubic expansion of a singleton-type fixation probability function herein definitively calibrates selection that yields a reduced domain of convergence of the ‘1/3-rule’ from biological evolutionary game theory. The Moran model of random genetic drift in continuous-time (with overlapping generations) [5,6] akin to the discrete-time Wright-Fisher model (with intermittent generations) [7,8] remain significant elementary models of theoretical population genetics [9]. The game-theoretical model herein does not require the assumption of a large population size, in contrast to the diffusion theory. Research on population genetics that mathematically reinvigorates evolutionary game theory intrigues the mainstream of theoretical biology [10,11,12].

Consider an evolutionary game in a finite population analogous to the haploid Moran model of population genetics [13,14,15]. The finite population of size N consists of i players of type C (co-operators) and N-i players of type D (defectors). These types do not mutate and thus represent pure strategies. The game involves pair-wise interactions being advantageous or disadvantageous for the two players according to a payoff matrix

$$\begin{array}{cc} & \begin{array}{cc}C& D\end{array}\\ \begin{array}{c}C\\ D\end{array}& \left(\begin{array}{cc}\alpha & \beta \\ \gamma & \delta \end{array}\right)\end{array}$$

(1)

According to the first row of this payoff matrix, a co-operator receives payoff α against another co-operator and receives payoff β against a defector. In the second row of the matrix, a defector receives payoff γ against a co-operator and receives payoff δ against another defector.

The corresponding evolutionary game represents a social dilemma when α > δ, which ensures that the exploitation of co-operators by defectors eventually yields an overall population fitness decrease as defectors approach fixation. Denote the selective parameter, $0\le \varpi \le 1$, being due to the payoff differential in the game. Let the fitness of co-operation be $f_i=1-\varpi +\varpi F_i$, where $F_i=\frac{1}{N-1}\left[\alpha \left(i-1\right)+\beta \left(N-i\right)\right]$. Let the fitness of defection be $g_i=1-\varpi +\varpi G_i$, where $G_i=\frac{1}{N-1}\left[\gamma i+\delta \left(N-i-1\right)\right]$. Thus, $F_i$ and $G_i$ define the expected payoffs to players of type C and type D, respectively. Let ${\rho }_C\left(\varpi \right)=1/\left[1+h\left(\varpi \right)\right]$ denote the fixation probability of a singleton type C individual in the population of size N, where $h\left(\varpi \right)={{\displaystyle \sum }}_{j=1}^{N-1}{{\displaystyle \prod }}_{i=1}^j\frac{g_i}{f_i}$. This elementary result of stochastic processes thus extends to frequency-dependent selection in the Moran model [16]. Details of this derivation can be found in review [17], and the introduction section of previous work [6].

The fixation probability of a singleton type C in the population yields an evolutionary rule [16], obtained in the limit of a large population size. The third order Maclaurin series of ${\rho }_C$ yields ${\rho }_C\left(\varpi \right)\approx {\rho }_C\left(0\right)+\varpi {\left[{\rho }_C^{\prime }\left(\varpi \right)\right]}_{\varpi =0}+\frac{{\varpi }^2}{2}{\left[{\rho }_C^{″}\left(\varpi \right)\right]}_{\varpi =0}+\frac{{\varpi }^3}{6}{\left[{\rho }_C^{‴}\left(\varpi \right)\right]}_{\varpi =0}$, where prime denotes differentiation with respect to $\varpi$. Derivatives must be evaluated at $\varpi =0$, whereas convergence of the resultant second- and third-order terms in the limit of a large population size requires non-zero values, $\varpi =\sigma /N$. Where σ denotes non-negative selection intensity. Linear truncation of the Maclaurin series yields the ‘1/3-rule’ [16], ${\rho }_C\left(\varpi \right)>\frac{1}{N}\Rightarrow \frac{\delta -\beta }{\alpha -\beta -\gamma +\delta }<\frac{1}{3}$, since ${\left[{\rho }_C^{\prime }\left(\varpi \right)\right]}_{\varpi =0}=\frac{\alpha }{6}\left(1-\frac{2}{N}\right)+\frac{\beta }{6}\left(2-\frac{1}{N}\right)-\frac{\gamma }{6}\left(1+\frac{1}{N}\right)-\frac{\delta }{6}\left(2-\frac{4}{N}\right)$. Note that ${\rho }_C\left(0\right)={\rho }_D\left(0\right)=\frac{1}{N}$, the singleton-type fixation probability with selective neutrality in a population of size N. In this evolutionary rule, the population relative frequency of co-operation equilibrates to less than one-third. The potential significance of higher-order expansions was recognized within a few years of the rule’s inception [18,19]. Theoretical socio-biologists consider the two-player game rule axiomatic for a variety of modelling environments. Topical research on fixation probability distributions include the following examples: unorthodox and non-reflexive game strategies that challenge the supremacy of tit-for-tat in the iterated Prisoners’ Dilemma [20]; congruency of various individual interactions within multi-player games that do not alter fixation probability distributions [21]; equilibration rates for genetic or strategy mutation processes when realized independently of random genetic drift [22]. It therefore garners substantial interest to study the significance and utility of non-linear fixation probability in evolutionary games.

In Section 2, all dominant third-order Maclaurin series coefficients for ${\rho }_C\left(\varpi \right)$ will be obtained. These calculations prove to supplant the heuristic coefficients proposed earlier [19]. Specific comments on these corrections and imminent research are deferred to the Discussion section.

2. Third-Order Extension of ‘1/3-Rule’ Calibrates Selection

2.1. Description of Cubic Coefficients Extending the ‘1/3-Rule’

The calculations in this Section culminate in proof of Theorem 1 that describes the cubic limiting dominant term of the inequality from which the ‘1/3-rule’ derives. This requires the Maclaurin series of ${\rho }_C\left(\varpi \right)$ up to third order. The second order expansion and its quadratic coefficients at finite population size were obtained in earlier work [23]. Theorem 1 implicitly describes the convergent domain where the ‘1/3-rule’ remains valid in the limit of a large population size.

Theorem 1.

Let ${\rho }_C\left(\varpi \right)>\frac{1}{N}$. Extension of the ‘1/3-rule’ inequality based on the Maclaurin series of${\rho }_C\left(\varpi \right)$at third order in the limit of a large population size yields

$$\begin{gathered} \frac{\delta -\beta }{\alpha -\beta -\gamma +\delta }<\frac{1}{3}+\frac{\sigma }{\alpha -\beta -\gamma +\delta }\left[\frac{{\alpha }^2}{180}+\frac{4{\beta }^2}{45}+\frac{{\gamma }^2}{180}+\frac{4{\delta }^2}{45}+\frac{13\alpha \beta }{180}-\frac{\alpha \gamma }{90}-\frac{13\alpha \delta }{180}-\frac{13\beta \gamma }{180}-\frac{8\beta \delta }{45}+\frac{13\gamma \delta }{180}\right]+ \\ \frac{{\sigma }^2}{3\left(\alpha -\beta -\gamma +\delta \right)}[-\frac{101{\alpha }^3}{2520}-\frac{32{\beta }^3}{315}+\frac{101{\gamma }^3}{2520}+\frac{32{\delta }^3}{315}-\frac{13{\alpha }^2\beta }{84}+\frac{101{\alpha }^2\gamma }{840}+\frac{13{\alpha }^2\delta }{84}-\frac{57\alpha {\beta }^2}{280}-\frac{101\alpha {\gamma }^2}{840}- \\ \frac{57\alpha {\delta }^2}{280}-\frac{13\beta {\gamma }^2}{84}-\frac{32\beta {\delta }^2}{105}+\frac{57\gamma {\beta }^2}{280}+\frac{57\gamma {\delta }^2}{280}+\frac{32\delta {\beta }^2}{105}+\frac{13\delta {\gamma }^2}{84}+\frac{13\alpha \beta \gamma }{42}+\frac{57\alpha \beta \delta }{140}-\frac{13\alpha \gamma \delta }{42}-\frac{57\beta \gamma \delta }{140}] \end{gathered}$$

(2)

Remark 1.

Equal payoff matrix entries, $\alpha =\beta =\gamma =\delta$, corresponds to selective neutrality. In that case, the fixation probability then equals 1/N, the zeroth order term in the Maclaurin series. Furthermore, at finite population size N, the entire set of second-order coefficients sum to zero. Similarly, the asymptotic third-order coefficients derived in Section 3 that comprise the proof of Theorem 1 sum to zero.

Remark 2.

According to Inequality (2), the total value of the second- and third-order terms shown being negative reduces the corresponding upper bound of the inequality such that the ‘1/3-rule’ holds. Alternatively, the total value of the second- and third-order terms shown being positive increases the corresponding upper bound of the inequality which strictly violates the ‘1/3-rule’.

2.2. Dominant Cubic Coefficients of Singleton Fixation Probability Maclaurin Series

Consecutive differentiation of the singleton fixation probability yields

$${{\rho }^{\prime }}_C\left(\varpi \right)=-\frac{h^{\prime }\left(\varpi \right)}{N^2}{|}_{\left(\varpi =0\right)}=N^{-2}{{\displaystyle \sum }}_{i=1}^{N-1}{{\displaystyle \sum }}_{j=1}^i\left(F_j-G_j\right);$$

(3)

$${{\rho }^{″}}_C\left(\varpi \right)=-\frac{h^{″}\left(\varpi \right)}{N^2}+\frac{2{\left[h^{\prime }\left(\varpi \right)\right]}^2}{N^3}{|}_{\left(\varpi =0\right)},$$

$$\text{where},h^{″}\left(\varpi \right){|}_{\left(\varpi =0\right)}={{\displaystyle \sum }}_{i=1}^{N-1}\left[{\left\{{{\displaystyle \sum }}_{j=1}^i\left(G_j-F_j\right)\right\}}^2+{{\displaystyle \sum }}_{j=1}^i\left\{F_j^2-G_j^2-2\left(F_j-G_j\right)\right\}\right];$$

(4)

$${{\rho }^{‴}}_C\left(\varpi \right)=-\frac{h^{‴}\left(\varpi \right)}{N^2}+\frac{6h^{\prime }\left(\varpi \right)h^{″}\left(\varpi \right)}{N^3}-\frac{6{\left[h^{\prime }\left(\varpi \right)\right]}^3}{N^4}{|}_{\left(\varpi =0\right)},$$

$$\begin{gathered} \text{where},h^{‴}\left(\varpi \right)={{\displaystyle \sum }}_{i=1}^{N-1}[\underset{\mathcal{O}\left(i^6\right){\left(N-1\right)}^{-3}}{\underbrace{{\left\{{{\displaystyle \sum }}_{j=1}^i{G}_j-F_j\right\}}^3}}+3\underset{\mathcal{O}\left(i^3\right){\left(N-1\right)}^{-2}}{\underbrace{\left\{{{\displaystyle \sum }}_{j=1}^i{F}_j^2-G_j^2-2\left(F_j-G_j\right)\right\}}}\underset{\mathcal{O}\left(i^2\right){\left(N-1\right)}^{-1}}{\underbrace{\left\{{{\displaystyle \sum }}_{j=1}^i{G}_j-F_j\right\}}}+ \\ 2\left\{{{\displaystyle \sum }}_{j=1}^i\underset{\mathcal{O}\left(i^4\right){\left(N-1\right)}^{-3}}{\underbrace{G_j^3-F_j^3}}-3\underset{\mathcal{O}\left(i^3\right){\left(N-1\right)}^{-2}}{\underbrace{\left(G_j^2-F_j^2\right)}}+3\underset{\mathcal{O}\left(i^2\right){\left(N-1\right)}^{-1}}{\underbrace{\left(G_j-F_j\right)}}\right\}]. \end{gathered}$$

(5)

Efficient calculation of the requisite third derivative ${\rho }_C^{‴}\left(\varpi \right)$ from Equation (5) obtains via orders of magnitude in the corresponding summations. Note that the summation of index powers can be calculated with the use of descending factorials ([24], Equation (2.50)). For instance, after some algebra, ${{\displaystyle \sum }}_{i=1}^{N-1}{i}^5={{\displaystyle \sum }}_{i=1}^{N-1}{i}_{\left(5\right)}+10i_{\left(4\right)}+25i_{\left(3\right)}+15i_{\left(2\right)}+i=\frac{1}{6}{N}_{\left(6\right)}+2N_{\left(5\right)}+\frac{25}{4}{N}_{\left(4\right)}+\frac{15}{3}{N}_{\left(3\right)}+\frac{1}{2}{N}_{\left(2\right)},$ where $i_{\left(m\right)}=i\left(i-1\right)\cdots \left(i-m+1\right)$. Hence, after further algebra, ${{\displaystyle \sum }}_{i=1}^{N-1}{i}^5=\frac{N^2{\left(N-1\right)}^2\left(4N^2-4N-2\right)}{24}$. Similarly, further algebra yields ${{\displaystyle \sum }}_{i=1}^{N-1}{i}^6=\frac{N\left(N-1\right)\left(2N-1\right)\left(3N^4-6N^3+3N+1\right)}{42}$. Thus, summation of the sixth index power equals $1, 65, 794, 4890, \dots$; when $N=2, 3, 4, 5, \dots$. Notice the leading-order terms of these summations that will be useful in the following calculations: ${{\displaystyle \sum }}_{i=1}^{N-1}i~\frac{N^2}{2}$, ${{\displaystyle \sum }}_{i=1}^{N-1}{i}^2~\frac{N^3}{3}$, ${{\displaystyle \sum }}_{i=1}^{N-1}{i}^3~\frac{N^4}{4}$, ${{\displaystyle \sum }}_{i=1}^{N-1}{i}^4~\frac{N^5}{5},{{\displaystyle \sum }}_{i=1}^{N-1}{i}^5~\frac{N^6}{6},$ and ${{\displaystyle \sum }}_{i=1}^{N-1}{i}^6~\frac{N^7}{7}$.

In Equation (5), the cubed inner summation ${\left\{{{\displaystyle \sum }}_{j=1}^i{G}_j-F_j\right\}}^3$ yields an outer summation magnitude $\mathcal{O}\left(N^4\right)$ that represents one dominant term. The second term of $h^{‴}\left(\varpi \right)$ in Equation (5), $3\left\{{{\displaystyle \sum }}_{j=1}^i{F}_j^2-G_j^2-2\left(F_j-G_j\right)\right\}\left\{{{\displaystyle \sum }}_{j=1}^i{G}_j-F_j\right\}$ yields a product of inner summations that yields an outer summation magnitude $\mathcal{O}\left(N^3\right)$. The final term of $h^{‴}\left(\varpi \right)$ in Equation (5) yields an outer summation magnitude $\mathcal{O}\left(N^2\right)$.

2.3. Part 1 of Dominant Terms in ${\rho }_C^{‴}\left(\varpi \right)$

The first part of the dominant third-order term in the Maclaurin series of ${\rho }_C\left(\varpi \right)$ in Equation (5) was found to be

$$\begin{gathered} {{\displaystyle \sum }}_{i=1}^{N-1}{\left\{{{\displaystyle \sum }}_{j=1}^i{G}_j-F_j\right\}}^3={\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}{\left\{{{\displaystyle \sum }}_{j=1}^i\gamma j+\delta \left(N-j-1\right)-\alpha \left(j-1\right)-\beta \left(N-j\right)\right\}}^3 \\ ={\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}{\left\{\gamma \frac{i\left(i+1\right)}{2}+\delta \left[\left(N-1\right)i-\frac{i\left(i+1\right)}{2}\right]-\alpha \frac{i\left(i-1\right)}{2}-\beta \left[Ni-\frac{i\left(i+1\right)}{2}\right]\right\}}^3 \end{gathered}$$

(6)

where Equation (6) results from expansion of the inner summation index $j=1, 2, \dots , i$. Expansion of the cubic exponent in Equation (6) yields

$$\begin{array}{l}{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}\{{\gamma }^3\frac{i^3{\left(i+1\right)}^3}{8}+{\delta }^3\left[{\left(N-\frac{3}{2}\right)}^3{i}^3-\frac{3}{2}{\left(N-\frac{3}{2}\right)}^2{i}^4+\frac{3}{4}\left(N-\frac{3}{2}\right)i^5-\frac{1}{8} i^6\right]-\\ {\alpha }^3\frac{i^3{\left(i-1\right)}^3}{8}-{\beta }^3\left[{\left(N-\frac{1}{2}\right)}^3{i}^3-\frac{3}{2}{\left(N-\frac{1}{2}\right)}^2{i}^4+\frac{3}{4}\left(N-\frac{1}{2}\right)i^5-\frac{1}{8} i^6\right]+3\left[{\gamma }^2\delta \frac{i^2{\left(i+1\right)}^2}{4}\right[(N-\\ \frac{3}{2})i-\frac{i^2}{2}]-{\gamma }^2\alpha \frac{i^2{\left(i+1\right)}^2}{4}\frac{i\left(i-1\right)}{2}-{\gamma }^2\beta \frac{i^2{\left(i+1\right)}^2}{4}[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}]-{\delta }^2\alpha {\left[\left(N-\frac{3}{2}\right)i-\frac{i^2}{2}\right]}^2\frac{i\left(i-1\right)}{2}-\\ {\delta }^2\beta {[\left(N-\frac{3}{2}\right)i-\frac{i^2}{2}]}^2\left[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}\right]-{\alpha }^2\beta \frac{i^2{\left(i-1\right)}^2}{4}\left[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}\right]]+3\left[\gamma {\delta }^2\frac{i\left(i+1\right)}{2}\right[\left(N-\frac{3}{2}\right)i-\\ \frac{i^2}{2}{]}^2+\gamma {\alpha }^2\frac{i\left(i+1\right)}{2}\frac{i^2{\left(i-1\right)}^2}{4}+\gamma {\beta }^2\frac{i\left(i+1\right)}{2}[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}]+\delta {\alpha }^2\left[\right(N-\frac{3}{2})i-\frac{i^2}{2}]\frac{i^2{\left(i-1\right)}^2}{4}+\delta {\beta }^2\left[\right(N-\\ \frac{3}{2})i-\frac{i^2}{2}][\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}{]}^2-\alpha {\beta }^2\frac{i\left(i-1\right)}{2}{\left[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}\right]}^2]-6[\gamma \delta \alpha \frac{i\left(i+1\right)}{2}[\left(N-\frac{3}{2}\right)i-\frac{i^2}{2}]\frac{i\left(i-1\right)}{2}+\\ \gamma \delta \beta \frac{i\left(i+1\right)}{2}[\left(N-\frac{3}{2}\right)i-\frac{i^2}{2}]\left[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}\right]-\delta \alpha \beta \left[\left(N-\frac{3}{2}\right)i-\frac{i^2}{2}\right]\frac{i\left(i-1\right)}{2}\left[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}\right]-\\ \gamma \alpha \beta \frac{i\left(i+1\right)}{2}\frac{i\left(i-1\right)}{2}\left[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}\right]\left]\right\}\end{array}$$

(7)

Inspection of Equation (7) yields the corresponding dominant coefficients in the Maclaurin series of singleton fixation probability at finite population size. These dominant cubic coefficients can be separated into four subgroups.

2.3.1. Dominant Single Cubic Coefficients

$${\gamma }^3:{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}\frac{i^6}{8}~\frac{N^4}{56}\Rightarrow \frac{N^2}{56}$$

(8)

$$\begin{gathered} {\delta }^3:{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}[{\left(N-\frac{3}{2}\right)}^3{i}^3-\frac{3}{2}{\left(N-\frac{3}{2}\right)}^2{i}^4+\frac{3}{4}\left(N-\frac{3}{2}\right)i^5-\frac{1}{8}{i}^6] \\ ~{\left(N-1\right)}^{-3}[N^3·\frac{N^4}{4}-3N^2·\frac{N^5}{10}+3N·\frac{N^6}{24}-\frac{N^7}{56}]=\frac{2N^4}{35}\Rightarrow \frac{2N^2}{35} \end{gathered}$$

(9)

Note the dominant coefficients of ${\alpha }^3\equiv -{\gamma }^3$ and ${\beta }^3\equiv -{\delta }^3$.

2.3.2. Dominant Paired Cubic Coefficients (i)

$${\gamma }^2\delta : 3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}\frac{i^4}{4}\left[\left(N-\frac{3}{2}\right)i-\frac{i^2}{2}\right]~3{\left(N-1\right)}^{-3}\left(N·\frac{N^6}{24}-\frac{N^7}{56}\right)=\frac{N^4}{14}\Rightarrow \frac{N^2}{14}$$

(10)

$${\gamma }^2\alpha : -3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}\frac{i^6}{8}~-\frac{3N^4}{56}\Rightarrow -\frac{3N^2}{56}$$

(11)

$${\gamma }^2\beta : -3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}\frac{i^4}{4}\left[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}\right]~-3{\left(N-1\right)}^{-3}\left(N·\frac{N^6}{24}-\frac{N^7}{56}\right)=-\frac{N^4}{14}\Rightarrow -\frac{N^2}{14}$$

(12)

$$\begin{gathered} {\delta }^2\alpha : -3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}{\left[\left(N-\frac{3}{2}\right)i-\frac{i^2}{2}\right]}^2\frac{i^2}{2}=-3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}[N^2\frac{i^4}{2}-N\frac{i^5}{2}+ \\ \frac{i^6}{8}]~-3{\left(N-1\right)}^{-3}\left(N^2·\frac{N^5}{10}-N·\frac{N^6}{12}+\frac{N^7}{56}\right)=-\frac{29N^4}{280}\Rightarrow -\frac{29N^2}{280} \end{gathered}$$

(13)

$${\delta }^2\beta : -3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}{\left[\left(N-\frac{3}{2}\right)i-\frac{i^2}{2}\right]}^2\left[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}\right]$$

$$~-3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}\left[N^2{i}^2-N{i}^3+\frac{i^4}{4}\right]\left[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}\right]$$

$$~-3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}\left[N^3{i}^3-N^2\frac{i^4}{2}-N^2{i}^4+N\frac{i^5}{2}+N\frac{i^5}{4}-\frac{i^6}{8} \right]$$

$$~-3{\left(N-1\right)}^{-3}\left[N^3·\frac{N^4}{4}-N^2·\frac{N^5}{10}-N^2·\frac{N^5}{5}+N·\frac{N^6}{12}+N·\frac{N^6}{24}-\frac{N^7}{56}\right]=-\frac{6N^4}{35}\Rightarrow -\frac{6N^2}{35}$$

(14)

Note the dominant coefficient of ${\alpha }^2\beta \equiv {\gamma }^2\beta$.

2.3.3. Dominant Paired Cubic Coefficients (ii)

Interchange of factors in summation terms previously calculated yields the dominant coefficients $\gamma {\delta }^2\equiv -{\delta }^2\alpha$, $\gamma {\alpha }^2\equiv 3{\gamma }^3$, $\delta {\alpha }^2\equiv {\gamma }^2\delta$ and $\alpha {\beta }^2\equiv -\gamma {\beta }^2$. Furthermore,

$$\begin{gathered} \gamma {\beta }^2 : 3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}\frac{ i^2}{2}{\left[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}\right]}^2=3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}\frac{i^2}{2}[{\left(N-\frac{1}{2}\right)}^2{i}^2-\left(N-\frac{1}{2}\right)i^3+\frac{i^4}{4}] \\ ~3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}[N^2\frac{i^4}{2}-N\frac{ i^5}{2}+\frac{i^6}{8}]~3{\left(N-1\right)}^{-3}\left(N^2·\frac{N^5}{10}-N·\frac{N^6}{12}+\frac{N^7}{56}\right)=\frac{29N^4}{280}\Rightarrow \frac{29N^2}{280} \end{gathered}$$

(15)

$$\begin{gathered} \delta {\beta }^2 : 3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}[\left(N-\frac{3}{2}\right)i-\frac{i^2}{2}]{\left[\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}\right]}^2=3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}[\left(N-\frac{3}{2}\right)i- \\ \frac{i^2}{2}\left]\right[{\left(N-\frac{1}{2}\right)}^2{i}^2-\left(N-\frac{1}{2}\right)i^3+\frac{i^4}{4}] \\ ~3{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}[N^3{i}^3-N^2{i}^4+N\frac{i^5}{4}-N^2\frac{i^4}{2}+N\frac{i^5}{2}-\frac{i^6}{8} ] \\ ~3{\left(N-1\right)}^{-3}[N^3·\frac{N^4}{4}-N^2·\frac{N^5}{5}+N·\frac{N^6}{24}-N^2·\frac{N^5}{10}+N·\frac{N^6}{12}-\frac{N^7}{56}]=\frac{6N^4}{35}\Rightarrow \frac{6N^2}{35} \end{gathered}$$

(16)

2.3.4. Dominant Triple Cubic Coefficients

Note the dominant coefficients of $\gamma \delta \alpha \equiv -2{\gamma }^2\delta$, $\delta \alpha \beta \equiv -2\alpha {\beta }^2,$ and $\gamma \alpha \beta \equiv -2{\gamma }^2\beta$. Furthermore,

$$\begin{gathered} \gamma \delta \beta : -6{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}\frac{i^2}{2}[\left(N-\frac{3}{2}\right)i-\frac{i^2}{2}][\left(N-\frac{1}{2}\right)i-\frac{i^2}{2}]~-6{\left(N-1\right)}^{-3}{{\displaystyle \sum }}_{i=1}^{N-1}\frac{i^2}{2}[N^2{i}^2- \\ N\frac{i^3}{2}-N\frac{i^3}{2}+\frac{i^4}{4}] \\ ~-6{\left(N-1\right)}^{-3}\left(N^2·\frac{N^5}{10}-N·\frac{N^6}{12}+\frac{N^7}{56}\right)=-\frac{29N^4}{140}\Rightarrow -\frac{29N^2}{140}. \end{gathered}$$

(17)

2.4. Part 2 of Dominant Terms in ${\rho }_C^{‴}\left(\varpi \right)$

From the top line of Equation (5), part 2 of the dominant third-order term requires calculation of $6N^{-3}{h}^{\prime }\left(\varpi \right)h^{″}\left(\varpi \right){|}_{\left(\varpi =0\right)}$. The corresponding second derivative coefficients were calculated exactly in earlier work ([23]; therein Equations (5), (8), (11), (14), (17), (20), (23), (26), (29), and (32)). The first derivative coefficients are well-known ([6], Equation (2.3)). Thus, the required product has leading-order coefficients given by

$$N^2[\gamma +2\delta -\alpha -2\beta ]\left[\frac{{\alpha }^2}{20}+\frac{2{\beta }^2}{15}+\frac{{\gamma }^2}{20}+\frac{2{\delta }^2}{15}+\frac{3\alpha \beta }{20}-\frac{\alpha \gamma }{10}-\frac{3\alpha \delta }{20}-\frac{3\beta \gamma }{20}-\frac{4\beta \delta }{15}+\frac{3\gamma \delta }{20}\right].$$

(18)

Carry through the algebra of Equation (18) to obtain

$$\begin{gathered} N^2[-\frac{{\alpha }^3}{20}-\frac{4{\beta }^3}{15}+\frac{{\gamma }^3}{20}+\frac{4{\delta }^3}{15}-\frac{5{\alpha }^2\beta }{20}+\frac{3{\alpha }^2\gamma }{20}+\frac{5{\alpha }^2\delta }{20}-\frac{13\alpha {\beta }^2}{30}+\frac{13\gamma {\beta }^2}{30}+\frac{12\delta {\beta }^2}{15}-\frac{3\alpha {\gamma }^2}{20}-\frac{5\beta {\gamma }^2}{20}+\frac{5\delta {\gamma }^2}{20}- \\ \frac{13\alpha {\delta }^2}{30}-\frac{12\beta {\delta }^2}{15}+\frac{13\gamma {\delta }^2}{30}+\frac{\alpha \beta \gamma }{2}+\frac{13\alpha \beta \delta }{15}-\frac{\alpha \gamma \delta }{2}-\frac{13\beta \gamma \delta }{15}] \end{gathered}$$

(19)

Thus, Equation (19) describes part 2 of the dominant cubic coefficients.

2.5. Part 3 of Dominant Terms in ${\rho }_C^{‴}\left(\varpi \right)$

From the top line of Equation (5), part 3 of the dominant third-order term requires calculation of $-6N^{-4}{\left[h^{\prime }\left(\varpi \right){|}_{\left(\varpi =0\right)}\right]}^3$. This product of the first derivative simplifies in leading order to yield

$$\begin{gathered} -{\left(\frac{N}{6}\right)}^2{\left(\gamma +2\delta -\alpha -2\beta \right)}^3 \\ ={\left(\frac{N}{6}\right)}^2\left[{\alpha }^3+8{\beta }^3-{\gamma }^3-8{\delta }^3+3\left(\alpha {\gamma }^2+2\beta {\gamma }^2+4\alpha {\delta }^2+8\beta {\delta }^2+2{\alpha }^2\beta -2{\gamma }^2\delta \right)-3\right({\alpha }^2\gamma + \\ 2{\alpha }^2\delta +4{\beta }^2\gamma +8{\beta }^2\delta +4\gamma {\delta }^2-4\alpha {\beta }^2)-6\left(2\alpha \beta \gamma +4\alpha \beta \delta -2\alpha \gamma \delta -4\beta \gamma \delta \right)] \end{gathered}$$

(20)

Thus, Equation (20) describes part 3 of the dominant cubic coefficients.

2.6. Simplification of Third-Order Maclaurin Series

The Maclaurin series at third order after cancellations of the zeroth order term, and the common factor $\varpi$ of the first-, second-, and third-order terms, yields the simplified inequality $0<{\rho }_C^{\prime }\left(\varpi \right){|}_{\left(\varpi =0\right)}+\frac{\varpi }{2}\left[{\rho }_C^{″}\left(\varpi \right){|}_{\left(\varpi =0\right)}\right]+\frac{{\varpi }^2}{6}\left[{\rho }_C^{‴}\left(\varpi \right){|}_{\left(\varpi =0\right)}\right]$. Next, the first derivative common factor ⅙ cancels out as divisor of the second and third derivatives in derivation of the extended ‘1/3-rule’. Standard form of the ‘1/3-rule’ requires a slight rearrangement of the first-order term in the simplified inequality just derived

$$\begin{gathered} 0<\alpha +2\beta -\gamma -2\delta \\ 0<\alpha +3\beta -\beta -\gamma -3\delta +\delta \\ 3\left(\delta -\beta \right)<\alpha -\beta -\gamma +\delta \\ \frac{\delta -\beta }{\alpha -\beta -\gamma +\delta }<\frac{1}{3}. \end{gathered}$$

(21)

Therefore, Inequality (21) shows common factor $3\left(\alpha -\beta -\gamma +\delta \right)$ as the other divisor of the second- and third-order terms in the simplified Maclaurin series. Gather like dominant cubic coefficients from the calculations of Section 2.3, Section 2.4 and Section 2.5, which in total yield those of Inequality (2).

This completes the proof of Theorem 1, Q.E.D.

3. Corollaries of Theorem 1

Inequalities derived from Theorem 1 yield conditions that prove violations of the rule extension at second order occur. The corresponding qualitative statements made characterize reduced domains of convergence in which the ‘1/3-rule’ remains valid.

3.1. Non-Harmful Contests

When $\alpha <\gamma$ and $\beta <\delta$, defectors dominate due to their relatively higher payoffs. The payoff scenario $\beta <\delta$ implies non-harmful contests, or strong exploitation of co-operators by defectors. Note that a regularity condition such that $\alpha +\delta >\beta +\gamma$ ensures non-degenerate equilibrium of the deterministic evolution of the game in this case.

Corollary 1.

Let $\alpha =c\gamma , \beta =d\delta$, where $0<c, d<1$. In this case, by Theorem 1 and Remark 2, at second order, the ‘1/3-rule’ extension term remains positive when

$$\frac{\beta }{\delta }<\frac{cd\left(1-d\right)}{\left(1-c\right)}$$

(22)

Proof of Corollary 1.

With the substitutions described, the second-order term of Inequality (2) equals

$$\frac{\sigma }{180[\left(1-d\right)\delta -\left(1-c\right)\gamma ]}\left[{\left(1-c\right)}^2{\gamma }^2+16{\left(1-d\right)}^2{\delta }^2+13\left(1-c\right)\left(1-d\right)\gamma \delta \right]$$

(23)

Expression (23) remains positive when $\left(1-d\right)\delta >\left(1-c\right)\gamma$. Thus, $\delta >\frac{\left(1-c\right)\gamma }{\left(1-d\right)}=\frac{\left(1-c\right)\alpha }{c\left(1-d\right)}>\frac{\left(1-c\right)\delta }{c\left(1-d\right)}=\frac{\left(1-c\right)\beta }{cd\left(1-d\right)}$. Hence, Inequality (22) pertains when the ‘1/3-rule’ exceeds its upper bound value.

Corollary 1 being therefore proven, Q.E.D. □

3.2. Harmful Contests

When $\alpha <\gamma$ and $\beta >\delta ,$ defector pairs endure combative attrition in contests. The payoff scenario $\beta >\delta$ implies harmful contests or weak exploitation of co-operators by defectors. Note that a regularity condition such that $\alpha +\delta <\beta +\gamma$ ensures non-degenerate equilibrium of the deterministic evolution of the game in this case.

Corollary 2.

Let $\alpha =c\gamma ,\delta =b\beta$, where $0<b,c<1$. In this case, by Theorem 1 and Remark 2, at second order, the ‘1/3-rule’extension term remains negative when

$$\frac{\beta }{\gamma }<\sqrt{\frac{16\frac{c^2}{b^2}{\left(1-b\right)}^2+{\left(1-c\right)}^2}{13\frac{b}{c}\left(1-b\right)\left(1-c\right)}}$$

(24)

Proof of Corollary 2.

With the substitutions described, the second-order term of Inequality (2) equals

$$\frac{\sigma }{180[\left(1-b\right)\beta +\left(1-c\right)\gamma ]}\left[13\left(1-b\right)\left(1-c\right)\beta \gamma -16{\left(1-b\right)}^2{\beta }^2-{\left(1-c\right)}^2{\gamma }^2\right]$$

(25)

Expression (25) remains negative when $13\left(1-b\right)\left(1-c\right)\beta \gamma <16{\left(1-b\right)}^2{\beta }^2+{\left(1-c\right)}^2{\gamma }^2$. Furthermore, $\alpha >\delta$ equates to $\gamma >\frac{b}{c}\beta$. Thus, $13{\beta }^2\frac{b}{c}\left(1-b\right)\left(1-c\right)<16{\gamma }^2\frac{c^2}{b^2}{\left(1-b\right)}^2+{\gamma }^2{\left(1-c\right)}^2$. Hence, Inequality (24) pertains when the ‘1/3-rule’ does not exceed its upper bound value.

Corollary 2 being therefore proven, Q.E.D. □

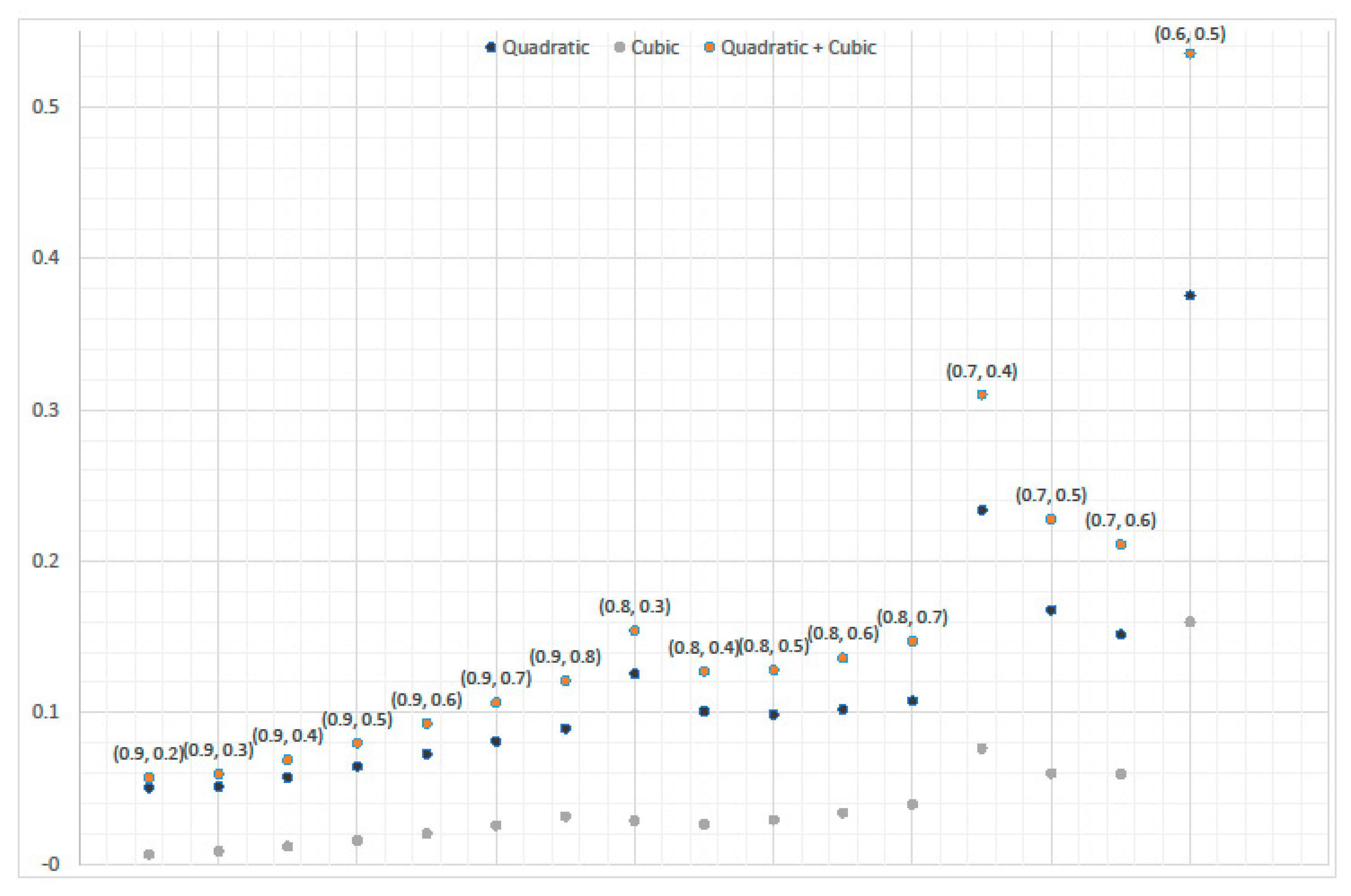
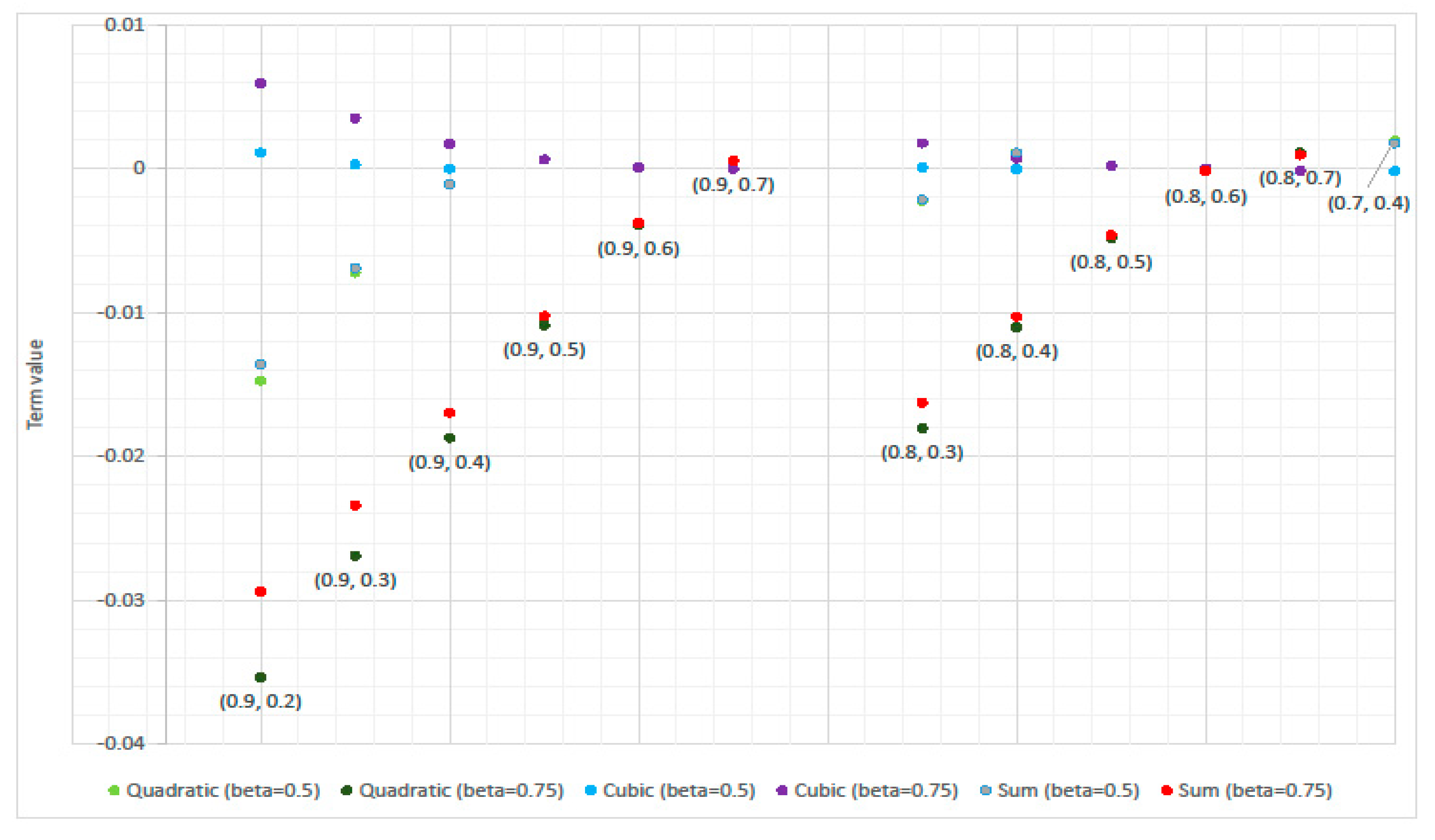
4. Quantify Maclaurin Series of ${\rho }_C\left(\varpi \right)$ Second- and Third-Order Terms

Recall from Section 3, where polarity of the second-order term from Inequality (2) accords with Corollaries 1 and 2. Consider the payoff matrix given by Expression (1), in which the number of pairwise comparisons $\left(\begin{array}{c}4\\ 2\end{array}\right)=6$. These six inequalities yield the following constraints: (i) $\alpha >\delta$; (ii) $\delta >\beta$; (iii) $\gamma >\delta$; (iv) $\gamma >\alpha$; (v) $\alpha >\beta$; and (vi) $\gamma >\beta$. Conditions (i) and (ii) refer to the social dilemma and non-harmful contests, respectively. Condition (iii) refers to rivalry attrition between defectors. Condition (iv) the exploitative gain defectors reap from cooperators. Condition (v) the cost cooperators suffer due to the loss of cooperation when they encounter a defector. Condition (vi) the gain of defectors compared to the loss of cooperators from dissimilar strategy encounter. Note that condition (ii) only allows for an inequality sign reversal, which then imposes harmful contests, and that $\beta$ takes positive values.

Define three constants, $0\le c_1, c_2,c_3\le 1$, such that condition (iii) implies $\delta =c_3\gamma$, (iv) $\alpha =c_1\gamma$, and (vi) $\beta =c_2\gamma$. Furthermore, conditions (i) and (ii) imply $c_1>c_3>c_2$. Substitution into the deterministic equilibrium relative frequency of cooperation $\frac{\delta -\beta }{\alpha -\beta -\gamma +\delta }=\frac{c_3-c_2}{c_1-c_2-1+c_3}\Rightarrow c_1+c_3>1$, when $c_2=0$. Note also that the ‘1/3-rule’ inequality at first order may be written $c_1+2c_2>1+2c_3$, which yields the contradiction $c_1>1+2(c_3-c_2)$. This contradiction implies that a linear truncation error must exist in the derivation of the ‘1/3-rule’. These non-negligible higher-order terms are quantified in Figure 1 and Figure 2.

4.1. Non-Harmful Contests When $\beta =0$

Corollary 1 quantified concordantly in Figure 1 that illustrates substantial violations of the rule exist unless $\sigma \ll 1$.

Figure 1 illustrates that smaller cubic terms were observed in comparison with non-negligible quadratic terms and therefore demonstrates convergence was reasonable at third order of the Maclaurin series. Clearly, violations of the ‘1/3-rule’ were observed in all of the corresponding domain shown.

4.2. Harmful Contests, Examples (i) $\beta =0.5$ and (ii) $0.75$

Corollary 2 being supported concordantly in Figure 2 that illustrates no significant violations of the rule were found.

Figure 2 demonstrates that the ‘1/3-rule’ holds without significant violation at least with the given values of β, since the negative quadratic terms dominate the positive cubic terms. These evaluations represent preliminary results due to the limited parameter values explored. It does seem likely that no domain of significant violation of the ‘1/3-rule’ exists when $\beta >\delta$.

5. Discussion

Theorem 1 restricts the ‘1/3-rule’ such that the selective differential $\varpi ~N^{-\left(1+p\right)}$, where $0\le p\le 1$. Theorem 1 sharpens the calibration of the selection intensity obtained in preceding work [6], which concludes that the general validity of the rule required extremely weak selection, where $p=1$. Such calibrations can be deduced that render any corresponding violations of the evolutionary rule at second and third order of negligible magnitude. In Corollary 1, the quotient of contested payoffs $\beta /\delta$ suggests a reduced domain of convergence of the ‘1/3-rule’ with non-harmful contests. The derived Inequality (22) of Corollary 1 yields a functional upper bound for the quotient of the only two payoff matrix entries that determine the value of the second order term in this case. Note that this idea gives credence to a preceding observation ([23], Remark (III) of Theorem 1). In Corollary 2, a quotient $\beta /\gamma$ that measures maximal exploitation determines polarity of the ‘1/3-rule’ extension term at second order with harmful contests. In this case, the two payoff matrix entries that determine the polarity of the second order term were proven β and γ. The payoff matrix entry inequalities $\delta <\beta$ and $\alpha <\gamma$, thus yield two positive constants less than one $0<b, c<1$, which eliminate α and δ. These two input constants evaluate the polarity functional, which was the derived Inequality (24). Recall, β represents the cost co-operators suffer due to loss of co-operation when they encounter a defector, whereas γ represents the benefit (advantageous payoff) when a defector exploits a co-operator. Quantitative analyses of asymptotic quadratic and cubic coefficients clarified these qualitative statements on selection intensity according to Theorem 1 (refer to Figure 1 and Figure 2). Namely, the ‘1/3-rule’ with harmful contests was found to be robust to selection intensity. In non-harmful contests, increased selection intensity raised the issue of divergence of the evolutionary rule due to an upper bound value greater than unity. The foundation of the model reveals constraints reducing its domain of convergence, since an intrinsic condition must apply such that $1+2\left(c_3-c_2\right)\le c_1$. This obtains from the deterministic relative frequency of co-operation, which yields the standard ‘1/3-rule’. Thus, non-harmful contests $\delta >\beta$ (equivalently, $c_3>c_2$) yields a contradiction of the intrinsic condition. Otherwise, harmful contests $\delta <\beta$ (equivalently, $c_3<c_2$) yields a valid model provided $c_1+2c_2\ge 1+2c_3$. For all these reasons, the higher order convergence behaviour of this evolutionary rule requires further investigation.

Earlier work of others ([19], Equations (A.11) and (A.13)) propose cubic coefficients from singleton fixation probability series that do not match those obtained herein with Inequality (2). Namely, Equation (A.11) uses the Moran model to get coefficients $\left[\left(N+1\right)\left(3N^2-2\right)u^3+15\left(N+1\right)N{u}^{2}v+30\left(N+1\right)u{v}^2+30v^3\right]\frac{\left(N-1\right)}{60},$ where $u=\left(a-b-c+d\right)/\left(N-1\right)$ and $v=\left(Nb-Nd-a+d\right)/\left(N-1\right).$ With respect to the standard Moran model herein, Equation (A.11) yields incorrect leading-order coefficients deflated at least by an order of magnitude in N. Their generalizations ([19], Equations (A.11) and (A.13)) also include an ill-defined heuristic multiplicative factor ${\left[f^{\prime }\left(0\right)\right]}^2$, where $f\left(\beta \pi \right)$ defines any function of the product of selection intensity $\beta$ and payoff π (in their notation). Whilst their Appendix Equation (A.4) does present the correct third-order expansion of the singleton fixation probability, which agrees with the calculus herein, subsequently, a different population process in Equation (A.11) and a specialized case of the Moran model in Equation (A.13) do not compare with the results herein.

The results of the current work suggest that the precise calibration of selection intensity and non-linear approximation of fixation probability be worthwhile research objectives. Two areas of interest already recognized are the applicability of diffusion theory to evolutionary games [4] and the generalization of pairwise interactions [25]. Another research problem discussed in an earlier review [10] relates fixation probability and the ‘1/3-rule’ to the structured coalescent process of population genetics [26]. Potentially, this could be interesting, since the results herein impact on the correct form of the ‘1/3-rule’ beyond linear truncation.

Finally, a parallel literature on social dilemmas within evolutionary economics was recently comprehensively reviewed [27]. The ‘risk-dominance’ inequality being treated in some detail therein corresponds to the linearized quotient of singleton fixation probabilities [6], whereas the standard ‘1/3-rule’ corresponds to one singleton fixation probability linearized [23]. A higher-order ‘risk-dominance’ extension amenable to finite population size analysis was recently studied [11]. Overall, the deepest scientific perspective must be that of evolved human co-operation that has consequentially revitalized research in statistical physics [28].