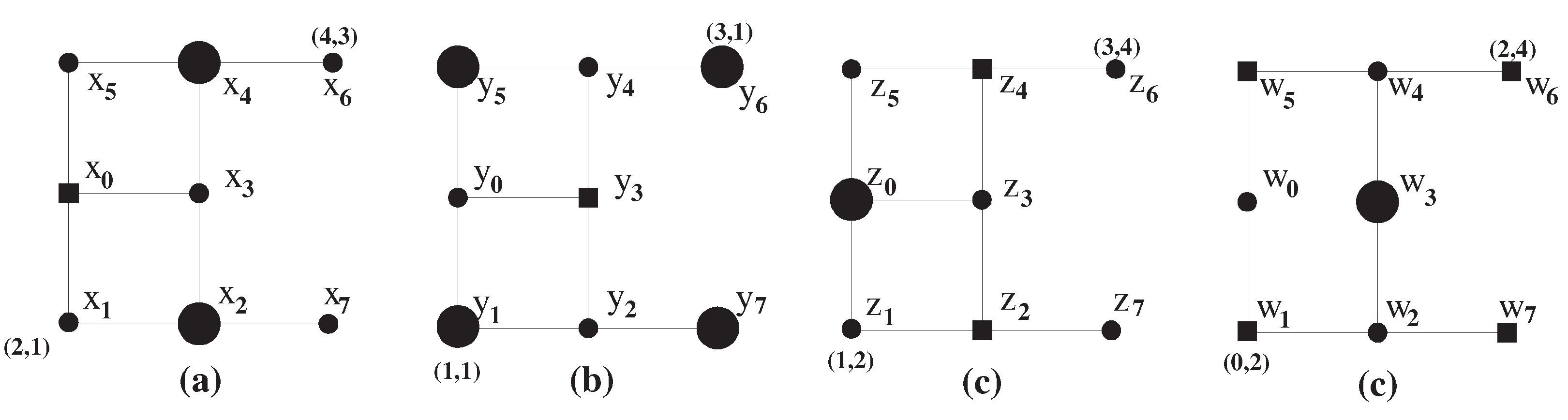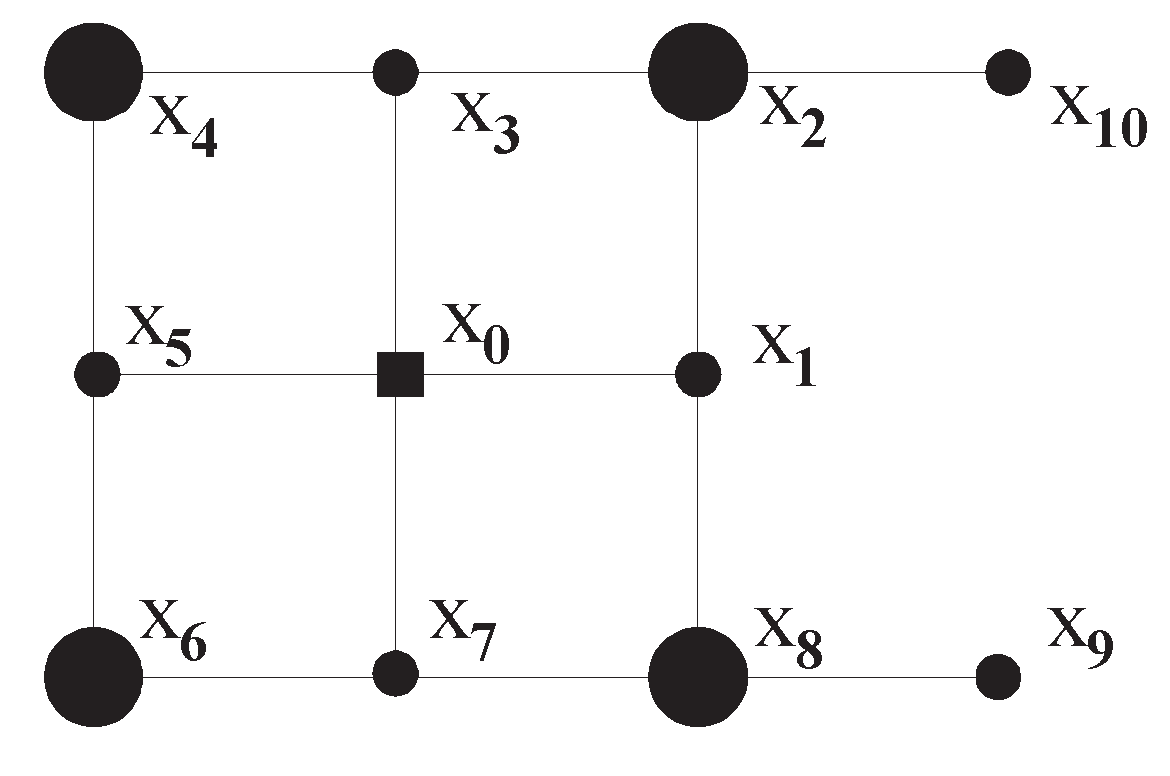1. Introduction
To make the paper self-contained, we recall that a mathematical object
X has the
FPP if every well-behaved mapping
f from
X to itself has a point
such that
. Reference [
1] studied a certain fixed point Theorem in semimetric spaces and further, Reference [
2] explored a coincidence point and common fixed point theorems in the product spaces of quasi-ordered metric spaces. Unlike the study of the fixed point property (
FPP, for short) for retractable topological spaces, since the research of the
FPP of non-retractable topological spaces still remains, the present paper addresses the issue.
A well-known connection exists between Alexandroff topology with
-separation axiom and order theory via the so-called specialization order and the down-sets [
3,
4]. More precisely, Alexandroff topologies on
X, denoted by
which are induced by the preordered sets
(for more details, see
Section 3), are in one-to-one correspondence with preorders on
X [
3]. This approach is often used to describe a topological space on which every continuous mapping has a fixed point. But another approach is in order theory, where a partially ordered set (or a poset for brevity)
P is said to have the
FPP if every increasing function on
P has a fixed point.
The paper will study the
FPP from the viewpoints of both order theory and Alexandroff topology and adopt the results into the study of the
FPP for Khalimsky topological spaces, which can play an important role in both pure and applied mathematics. In fixed point theory from the viewpoint of order theory, the well-known issue existed, as follows: If
X and
Y are ordered sets with the
FPP, does
have
FPP? This was of interest in the theory of ordered sets [
5]. It was conjectured for a long time (see Reference [
5]) that the product of two finite ordered sets with the
FPP has the
FPP. This has been referred to as the
Product Problem or the
Product Conjecture. Motivated by the results in Reference [
6], the conjecture was settled positively [
7] if
P is finite so that this became a theorem when Roddy [
7] proved the conjecture true in 1994.
Let us recall the well-known theorem [
8,
9] that a lattice
L has the
FPP if and only if
L is a complete lattice. Under this situation, for each order-preserving self-map
g of
L, the set
is a complete lattice. Furthermore, motivated by the Tarski-Davis theorem [
8,
9] on a lattice and Kuratowski’s question [
10,
11] on the product property of the
FPP on a peano continuum (or a compact, connected and locally connected metric space), many works dealt with the
FPP for ordered sets and topological spaces. Some of these include References [
3,
10,
12,
13,
14,
15,
16,
17,
18]. Rival [
19] considered irreducible points in arbitrary ordered sets, as follows: For a poset
, consider two distinct points
. If
and there is no
such that
, then
y is said to be an upper cover of
x and
x is called a lower cover of
y. Given a finite poset
, a point in
P is called irreducible if and only if it has exactly one upper cover or exactly one lower cover. Let
be irreducible and assume that
x has a unique lower cover. Then Rival [
19] proved the following:
[Rival theorem] For a poset P and let be irreducible. Then P has the FPP if and only if has the FPP.
In poset theory, consider a poset
P and two points
. Then
a is called retractable to
b (see Definition 3.1 of Reference [
20]) if and only if
and
and
, where under the Hasse diagram of
P,
and
mean the down set of
a and the upper set of
b in the poset
P, respectively.
Besides, Schröder (see Theorem 3.3 of Reference [
20]) proved the following:
[Schröder theorem] For a poset , assume that is retractable to . P has the FPP if and only if (1) has the FPP and (2) One of and has the FPP.
Motivated by these results, we can study the FPP for some Alexandroff (topological) spaces with -separation axiom (-A-space, for brevity if there is no danger of ambiguity) because a -A-space induces a poset and vice versa, as mentioned above. At the moment we have the following query.
[Question] How can we study the FPP of the poset (or the -A-space) in case a given poset (or a -A-space) is related to neither the Rival Theorem nor the Schröder theorem?
In
Section 4, we will address the question (see Theorem 3). In topology, it is well known that in general the product property of the
FPP does not hold [
16,
17,
21]. Comparing with the
FPP in References [
5,
16,
17,
21], its Khalimsky topological version has its own feature. Since the term “Khalimsky” will be often used in this paper, hereafter we will use the terminology
-’ instead of “Khalimsky” if there is no danger of confusion. To study the product property of the
FPP for
K-topological spaces, we need to recall basic notions associated with both
K-topology and fixed point theory.
Comparing a
K-topological space with spaces dealt with in earlier papers [
16,
17,
21], we can obtain a poset derived from the given
K-topological space. Every two points of a poset
need not be retractable point and further, each element of
need not be irreducible either (see Property 1). Moreover, this poset need not be a lattice (see Lemma 1 in the present paper). Hence we cannot use the Tarski-Davis [
8,
9], Rival [
19] and Schr
öder [
20] theorems to study the
FPP for
K-topological spaces. Henceforth, we need to study fixed point theory for
K-topological spaces in some different approaches from those of References [
16,
17,
21] (see Theorem 3).
Let
,
and
be the set of natural numbers, integers, and real numbers, respectively. Owing to Borsuk’s or Brouwer’s fixed point theorem [
22], it is well known that a compact Euclidean
n-dimensional cube
has the
FPP. However, in digital topology it is clear that any digital plane
followed from the Rosenfeld model on
does not have the
FPP related to digitally
k-continuous maps [
23] (for more details, see References [
24,
25,
26,
27]). Besides, it turns out that not every
-
A-space has the
FPP [
28]. For instance, not every
K-topological space has the
FPP [
28]. That is why in this paper we give particular attention to the
FPP for
K-topological spaces and its product property and further, to the further study of the
FPP for some points deleted
K-topological planes.
The rest of this paper is organized as follows.
Section 2 provides some terminology from
K-topology.
Section 3 investigates a poset structure derived from an
n-dimensional
K-topological space and studies some properties of a
K-continuous map from the viewpoint of order theory.
Section 4 studies the product property of the
FPP for
K-topological spaces and further, investigates various properties of fixed points of
K-continuous self-maps of a non-
K-retractable space.
Section 5 concludes the paper with summary and a further work.
2. Preliminaries
A poset consists of a set
and a reflexive, antisymmetric and transitive binary relation ≤, denoted by
. In this paper we will refer to the underlying set
X as the ordered set and many notions are followed from References [
29,
30]. Subsets
inherit the order relation from
X in terms of a restriction to
S. A homomorphism between ordered sets
P and
Q is an order-preserving map, that is, a map
having the following property:
(in
P) implies
(in
Q). In addition, we say that an ordered set
has the fixed point property if every order-preserving self-map
f of
X has a point
such that
and further, we denote
. Besides, for a poset
L,
L is called a lattice if and only if any two elements of
L have a supremum and an infimum.
L is called a complete lattice if and only if any subset of
L has a supremum and an infimum [
3].
An Alexandroff (
A-, for brevity) topological space
is said to be a topological space of which each point
has the smallest open neighborhood in
[
31]. As an example of an
A-topological space is the Khalimsky (
K-,for short) line topology. To be precise,
K-line topology on
, denoted by
, is induced by the set
as a subbase [
31] (see also Reference [
4]), where for
,
. Furthermore, the product topology on
induced by
is said to be the
Khalimsky product topology on
(or the Khalimsky
n-dimensional space), denoted by
. Hereafter, for a subset
we will denote by
,
, a subspace induced by
and it is called a
K-topological space. The study of these spaces includes References [
4,
28,
32,
33,
34,
35,
36,
37,
38,
39].
Let us examine the structure of
more precisely. A point
is
pure open if all coordinates are odd and
pure closed if each of the coordinates is even [
4] and the other points in
is called
mixed [
4]. These points are showed like following symbols: The symbols ▪, a black jumbo dot, • mean a pure closed point, a pure open point and a mixed point (see
Figure 1,
Figure 2,
Figure 3,
Figure 4 and
Figure 5), respectively.
In relation to the further statement of a mixed point in
, for the points
(resp.
), we call the point
p closed-open (resp.
open-closed) [
37]. In terms of this perspective, we clearly observe that the
smallest (open) neighborhood of the point
of
, denoted by
, is the following:
Similarly, for and , we can also consider in .
Definition 1 ([
4,
33]).
For , we say that two distinct points x and y in X are K-adjacent in if . Let us recall the following terminology for studying K-topological spaces.
Definition 2 ([
33,
40]).
For we define the followings.- (1)
Two distinct pointsare called K-path connected (or K-connected) if there is the sequence (or a path)on X withsuch thatandare K-adjacent,. This sequence is called a K-path. Furthermore, the number l is called the length of this K-path.
- (2)
A simple K-path in X is the K-pathin X such thatandare K-adjacent if and only if.
- (3)
We say that a simple closed K-curve with l elementsin X, denoted by, is the K-path such thatandare K-adjacent if and only if.
Let us now recall the
K-continuity of a map between two
K-topological spaces [
32] as follows: For two spaces
and
, a function
is said to be
K-continuous at a point
if the following property holds
Furthermore, we say that a map is K-continuous if it is K-continuous at every point . This approach can be reasonable because each subspace of is an Alexandroff space.
In addition, we recall the notion of
K-homeomorphism (see
Figure 1) as follows: For two spaces
and
, a map
is called a
K-homeomorphism if
h is a
K-continuous bijection and further,
is
K-continuous.
Using the
K-continuity of the map
f, we obtain the
K-topological category, denoted by
KTC, consisting of the following two data [
39]:
The set of objects , denoted by ;
For every ordered pair of objects and , the set of all K-continuous maps as morphisms.
Assume that
has the
FPP. If
is a
K-homeomorphism, then
Y is also proved to have the
FPP [
28].
3. An Ordered Space Derived from a Khalimsky Topological Space
Recently, it turns out that not every
K-topological space
has the
FPP such as (
) and
[
28]. Hereafter, we assume that a
K-topological space
is finite,
K-connected and further, not a singleton. Besides, we will study the
FPP of
K-topological spaces by using an order-theoretical approach. In a Hasse diagram for a finite ordered set, elements
x and
y satisfy the relation
if and only if
or there is an upward path from
x to
y that may go through other elements of the set, but for which all segments are traversed in the upward direction (see
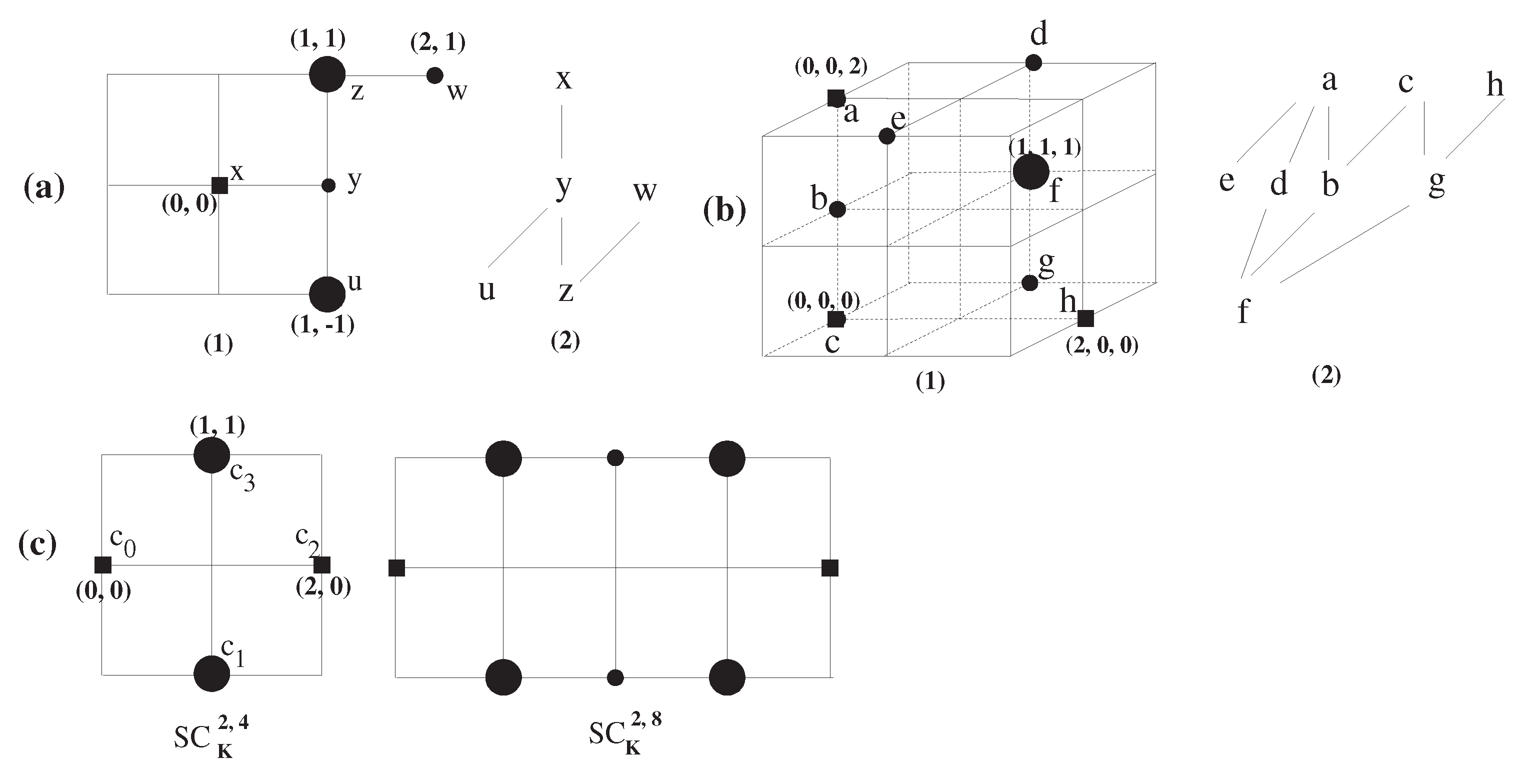
Figure 2a,b). Let
be a poset. For
, put
as a down set of
x in
.
Using the set of (3), we develop an induced topology on X generated by the set as a base and we denoted by the topological space.
In this paper we say that an order-topological space (ordered space, for short) is a set which is both a topological space and a poset. In
KTC, as special cases of the results in References [
5,
7,
16,
17,
21], as follows:
Remark 1. For two posetsand a function, f is an order-preserving map if and only if f is continuous between the induced Alexandroff topological spaces.
Proof. Assume that
f preserves order from
to
and let
be an open base set, where
means the down set of
y in the given poset (for more details, see the property (3)). We need to show that
is open in
X. Let us now take any
. In case
, we have
so that
. Therefore
. Hence, for any
we have
and further,
which implies that
is open.
Conversely, assume that f is continuous between the induced Alexandroff topologies and let for some . Suppose . Let . Therefore but A is open, due to the continuity of f, we obtain that is open. Hence . But , so , which implies that so that it invokes a contradiction. □
For
, we ask if the ordered space
induced by
is equal to the
K-topological space
. To be specific, based on the poset
derived from
, to establish the topology
on
X, we may have the following set as a subbase for the topology of
(see the property (1))
where
For instance, for
, to establish the topology
induced by the poset
derived from
, we have the set
as a subbase. Hence it is clear that the topology
on
is equal to the
K-topological space
.
By using this approach, for an integral interval , the ordered space induced by is equal to the K-interval denoted by .
According to this approach, for we obtain the following:
Corollary 1. For,is equal to, whereis a subspace for X relative to the topology.
Lemma 1. A K-topological spaceis an ordered space which need not be a lattice.
Proof. Under the Khalimsky n-dimensional space , we define a relation derived from , as follows:
For two points
of
we say that
Apart from this approach (4), we can also consider this relation
” in terms of the specialization order in Reference [
4]. More precisely, we say that
if and only if
, where the notation “
” means the closure operator. However, if we take the specialization order for establishing a relation “≤” for a poset, then this relation is different from the relation of (4). Since
satisfies the
-separation axiom, the relation “≤” of (4) is reflexive, antisymmetric(due to the
-separation axiom) and transitive. Furthermore, it is clear that the subspace
also induces a poset structure. However, the induced ordered space
need not be a lattice. For instance, consider the
K-topological spaces
and
in
Figure 2a,b. Then, according to the property (4), we obtain their Hasse diagrams of which the induced posets
and
are not lattices (see
Figure 2a,b(2)). □
The following is obtained by using the property of (3) and the relation of (4), which can be effectively used in studying the
FPP of ordered spaces in
Section 4.
Lemma 2. The ordered spaceis a-A-space and further, a semi-space.
Proof. Let us prove that the ordered space supports the -separation axiom. Consider any two distinct points . Note that, owing to the antisymmetric property of the relation ≤, we have or . Therefore or . Thus satisfies the -separation axiom because for every point we have .
Let us now prove that
is Alexandroff. Indeed, we suffice to show that an arbitrary intersection of base open sets is open. So assume that
is a subset of
X such that
and let
. Then, owing to the transitive property of the relation ≤, it is clear that
and thus
and therefore the intersection
A is open, which implies that
is an Alexandroff space. □
By using the notion of (4), Remark 1 and Lemma 2, we obtain the following:
Corollary 2. A K-continuous map between two K-topological spaces is equivalent to an order preserving map between posets derived from the given K-topological spaces.
4. The FPP of Non--Retractable Spaces
There was the well-known conjecture [
41] wondering if the fact that
X and
Y have the
FPP implies that the product space
has the property. This note contains an affirmative answer in the case that
X and
Y are compact ordered space
(with the order topology) [
7]. Besides, Cohen [
29] rephrased the result as follows.
As referred to in the above, since the present paper deals with only finite and connected spaces which is not a singleton, in
all spaces
are assumed to be both compact and connected. Motivated by the property (5) (or an immediate consequence of Reference [
7]), we have the following:
Remark 2. Assume that bothandhave the FPP. Then the product spacehas the FPP.
Proof. Under the hypothesis, it is clear that both
and
are compact (i.e., finite) connected ordered spaces. Owing to the results of Reference [
7], since both
and
derived from the given
K-topological spaces are finite posets, they have the
FPP. Hence, by Corollary 1, the product space
has the
FPP because it is equal to the ordered space
. By Corollary 1 and Lemma 1, the proof is completed (see the property (5)). □
Theorem 1 ([
28,
37]).
A simple K-path in has the FPP. Owing to Theorem 1, it is clear that a
K-interval obviously has the
FPP because a simple
K-path in
is
K-homeomorphic to a
K-interval
(see the last part of
Section 2).
By Theorem 1, since the K-interval has the FPP and further, is compact and connected, we have the following:
Remark 3. (1) References [35,37] dealt with the FPP of a K-interval and further, Reference [28] proved the FPP of a K-path. Besides, Reference [35] proved the FPP for finite K-topological plane in terms of an implicit function Theorem (see Theorem 9.2.4 on page 78 [35]). (2) Letbe a finite n-dimensional cube as a K-topological subspace. Then, by Remark 2 and Theorem 1,has the FPP.
The notion of retraction in order theory has been used in studying the
FPP [
19]. More precisely, let
be a poset. As mentioned in
Section 1, a point
is called retractable to
[
20] if and only if for all
implies
and
implies
[
20]. In other words, for an ordered set, we say that an order-preserving self-map
r of
P is called a retraction [
20] if and only if
. We will say that
is a retract of
P if and only if there is a retraction
with
[
20].
Based on the Schr
öder Theorem in
Section 1, Reference [
30] further studied the
FPP for product spaces in terms of the retractability from an order theoretical view. Since an ordered space is both a poset and a topological space, we will use the
K-retraction in Reference [
27] for studying the
FPP for
K-topological spaces.
Definition 3 ([
27]).
In KTC, a K-continuous map is a K-retraction if- (1)
is a K-topological subspace ofand
- (2)
for all.
Then we call X a K-retract of.
Example 1. Consider the K-topological spacesandin Figure 3. Then we observe thatis a K-retract of. As a special case of the Theorem in Reference [
7], we see the following: Let
be a
K-topological space having the
FPP. For
, if a map
is a
K-retraction, then
has the
FPP.
In this paper a K-topological plane is defined, as follows:
Definition 4. A spaceofis said to be a finite K-topological plane ifis K-homeomorphic to, wherefor some.
The following exploration involving a K-retract will be essentially used to prove main theorems of this paper.
Theorem 2. Let X be a finite K-topological plane. Not every one pointdeleted subspace of X is a K-retract of X.
Proof. We may consider
for some
such as
Based on this situation, we may examine the K-retract property of a spaces X with the following case according to the first case of (6).
Assume
and
in
Figure 4a. Let us consider the
K-topological spaces
and
. Then we observe that
is not a
K-retract of
. □
Thus this example guarantees that not every one point deleted subspace of a finite K-topological plane X is a K-retract of X.
Using Remark 3(2), owing to the FPP of a retraction, we obtain the following:
Corollary 3. Consider a K-topological spacecontained in a K-cubeas a subspace such thatis a K-retract of. Thenhas the FPP.
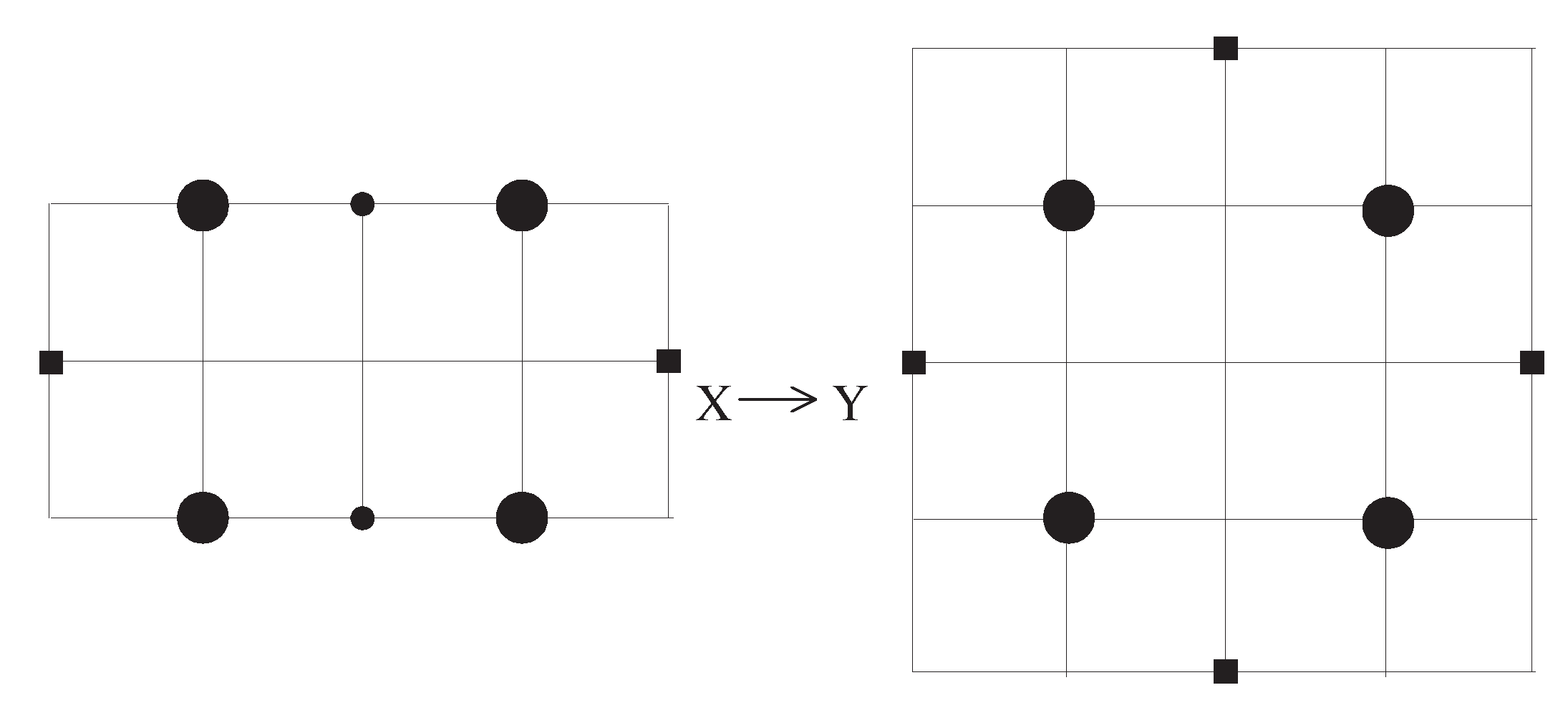
Example 2. (1) As referred to in Theorem 2, since the spaceis K-retractable ontoin Figure 4b, owing to the K-retract property of the FPP (see Corollary 3), it turns out thathas the FPP. Hence we conclude thathas the FPP. (2) As referred to in Theorem 2, since the spaceis K-retractable ontoin Figure 4c and further, owing to the K-retract property of the FPP, it turns out thathas the FPP. Hence we conclude thathas the FPP. In relation to the two theorems initiated by Rival and Schröder referred to in
Section 1, we can obviously have the following property.
Property 1. Consider the setin Figure 4a with the K-topological structure denoted byor a posetinduced by the space. Then the pointin the posetis neither irreducible point nor retractable to any point. Proof. We first prove that the point
is not irreducible in
. Assume an element
such that
. Then we may take
in
, for example,
. In case
, there is an element
such that
, which implies that
a is not irreducible in
. Next, we prove that
a is not retractable to any point
. To be precise, in
since
, for any
we obtain
which implies that
a is not retractable to the point
b. To be specific, for any element
the set
is not comparable with
. In case
, the element
a is not retractable to
b either because the points
a and
b also satisfies the property (7). □
In view of Property 1, we cannot address the question posed in
Section 1 by using the two theorems in
Section 1. Thus, by using the
FPP for the
K-topological category, let us now investigate the
FPP for some points deleted
K-topological spaces which are not
K-retracts of some
K-topological planes.
Theorem 3. Consider the K-topological plane. Let, where p is a pure closed point in. While the spaceis not K-retractable onto,has the FPP.
Proof. Without loss of generality, we may consider
and
in
Figure 4a. As referred to in Theorem 2, the given space
is not a
K-retract of any
K-topological plane such as
. Thus we cannot adopt Corollary 3 into the study of the
FPP of
. Hence we need to prove the
FPP for
from the viewpoint of Khalimsky topological category. Let us consider any
K-continuous self-map of
. In particular, consider the point
(see
Figure 4a) and assume any
K-continuous self-map
f sending the point
.
In case , the proof is completed.
In case is mapped into a pure open point in A such as . Then, owing to the K-continuity of f (see the property (2)), we have so that is a fixed point of f.
In case is mapped into a mixed point in A such as . Then, owing to the K-continuity of f, we should have or . If it happens to the former, the point t is a fixed point of f, and if it happens to the latter, the point or is a fixed point of f because . □
Example 3. By using the method similar to the proof of Theorem 3, while the given K-topological spaceis not a K-retract of any K-topological plane such as, where, we observe thathas the FPP.