Lower and Upper Solutions for Even Order Boundary Value Problems

Abstract

In this paper, we prove the existence of solutions of nonlinear boundary value problems of arbitrary even order using the lower and upper solutions method. In particular, we point out the fact that the existence of a pair of lower and upper solutions of a considered problem could imply the existence of solution of another one with different boundary conditions. We consider Neumann, Dirichlet, mixed and periodic boundary conditions.

1. Introduction

In the literature, the existence of solutions for nonlinear boundary value problems has been widely studied. This is due to the fact that almost all the physical phenomena, as well as many others in economics, biology or chemistry, are modeled by this kind of problems.

Some standard techniques which are frequently used to prove the existence of solutions for these problems are monotone iterative techniques (see [1,2,3]), lower and upper solutions method (see [4,5]) or fixed points theorems (see [1,6]). In all the aforementioned cases, the properties satisfied by the related Green’s function (and, in most of the cases, its constant sign) are the basic tool to prove the existence results. This clearly justifies the necessity of doing a careful study of linear problems and, in particular, of Green’s functions.

In a recent paper [7], we found some strong connections between Green’s functions of various separated two point boundary value conditions and Green’s functions of periodic problem. The key idea developed in that reference is the fact that the expression of the Green’s function related to each two-points case can be expressed as a linear combination of the Green’s function of periodic problems.

From those expressions relating various Green’s functions, we could obtain some comparison results which ensure that, in some cases, the Green’s function related to an even-order linear equation under some boundary conditions is bigger in every point than the Green’s function related to the same equation under another type of conditions.

The particular case of the Hill’s equation has also been considered in [8,9].

We show in this paper how the previous study can be applied to solve nonlinear boundary value problems. To do this, we use the relations found in [7,8] and apply the method of lower and upper solutions.

The method of lower and upper solutions is a classical tool for proving the existence of solutions of nonlinear boundary value problems. Roughly speaking, this method works as follows: the existence of a well-ordered pair of lower and an upper solutions ensures the existence of a solution lying between them. This way, we have information not only about the existence of a solution for our boundary value problem, but also about its location.

Some references that the reader may consult to find more information about this theory in a more general framework are [5,10,11,12,13,14,15].

The novelty in our approach with respect to others presented in the literature is that we are able to ensure the existence of solution of a problem by means of lower and upper solutions of another problem with different boundary conditions. To the best of our knowledge, this approach is new in the literature.

This paper is organized in the following way: First, Section 2 presents the set of problems and boundary conditions that we consider throughout the remaining of the articl and also gives some previous results. Section 3 includes the results proving the existence of solutions via lower and upper solutions method. Section 4 provides an example in which we prove the existence of solutions of the Dirichlet problem via lower and upper solutions of Neumann problem. Finally, in Section 5, we introduce another approach to ensure the existence of solutions for a certain boundary value problem, considering in this case an upper solution for other boundary conditions and some superlinear condition on the nonlinearity at $\pm \infty$.

2. Preliminary results

Consider the $2n$-order general linear operator

$$Lu(t)\equiv u^{(2n)}(t)+a_{2n-1}(t)u^{(2n-1)}(t)+\cdots +a_1(t)u^{\prime }(t)+a_0(t)u(t),t\in I\equiv [0,T],$$

with $a_k:I\to \mathbb{R},a_k\in L^{\alpha }(I),\alpha \ge 1$, $k=0,\dots ,2n-1$.

We note that a particular case of previous operator is the very well-known Hill’s operator:

$$Lu(t)\equiv u^{″}(t)+a_0(t)u(t),t\in I.$$

We work with the space

$$W^{2n,1}(I)=\left\{u\in {\mathcal{C}}^{2n-1}(I):u^{(2n-1)}\in \mathcal{A}\mathcal{C}(I)\right\},$$

where $\mathcal{A}\mathcal{C}(I)$ denotes the set of absolutely continuous functions on I. In particular, we consider $X\subset W^{2n,1}(I)$ a Banach space such that the following definition is satisfied.

Definition 1.

Given a Banach space X, operator L is said to be nonresonant on X if and only if the homogeneous equation

$$Lu(t)=0a.e.t\in I,u\in X,$$

has only the trivial solution.

Example 1.

Consider the periodic problem with constant coefficients, namely

$$\left\{\begin{array}{c}{u}^{(2n)}(t)+\lambda u(t)=0,t\in [0,T]\hfill \\ u^{(i)}(0)=u^{(i)}(T),i=0,\dots ,2n-1.\hfill \end{array}\right.$$

It is not difficult to verify that, if $n=2k+1$ for some $k=0,1,\dots$, the problem is nonresonant if and only if $\lambda \ne {\left(\frac{2m\pi }{T}\right)}^{2n}$, $m=0,1,\dots$, and that, if $n=2k$ for some $k=0,1,\dots$, the problem is nonresonant if and only if $\lambda \ne -{\left(\frac{2m\pi }{T}\right)}^{2n}$, $m=0,1,\dots$.

It is very well known that, if $\sigma \in error(I)$ and operator L is nonresonant on X, then the nonhomogeneous problem

$$Lu(t)=\sigma (t)a.e.t\in I,u\in X,$$

has a unique solution given by

$$u(t)=(L^{-1}\sigma )(t)={\int }_0^{T}G[T](t,s)\sigma (s)ds,\forall t\in I,$$

where $G[T]$ denotes the Green’s function related to operator L on X and it is uniquely determined.

We introduce now an auxiliary linear operator, whose coefficients are defined from the ones of operator L as follows:

$$\tilde{L}u(t)\equiv u^{(2n)}(t)+{\widehat{a}}_{2n-1}(t)u^{(2n-1)}(t)+{\tilde{a}}_{2n-2}(t)u^{(2n-2)}(t)+\cdots +{\widehat{a}}_1(t)u^{\prime }(t)+{\tilde{a}}_0(t)u(t),t\in J\equiv [0,2T],$$

where ${\tilde{a}}_{2k}$ and ${\widehat{a}}_{2k+1}$, $k=0,\dots ,n-1$, are, respectively, the even and odd extensions of $a_{2k}$ and $a_{2k+1}$ to J, that is,

$${\tilde{a}}_{2k}(t)=\left\{\begin{array}{cc}{a}_{2k}(t),\hfill & t\in [0,T],\hfill \\ a_{2k}(2T-t),\hfill & t\in [T,2T],\hfill \end{array}\right.$$

and

$${\widehat{a}}_{2k+1}(t)=\left\{\begin{array}{cc}{a}_{2k+1}(t),\hfill & t\in [0,T],\hfill \\ -a_{2k+1}(2T-t),\hfill & t\in (T,2T].\hfill \end{array}\right.$$

We work with some problems related to operator L (and, consequently, defined on the interval $[0,T]$) and some others related to operator $\tilde{L}$ (and, consequently, defined on $[0,2T]$). In the sequel, we describe the different problems and boundary conditions we are dealing with:

• Neumann problem on the interval $[0,T]$:

  $$\left\{\begin{array}{c}Lu(t)=\sigma (t),a.e.t\in I,\hfill \\ u^{(2k+1)}(0)=u^{(2k+1)}(T)=0,k=0,\dots ,n-1.\hfill \end{array}\right.$$
  The Green’s function related to this problem is denoted by $G_N[T]$.
• Dirichlet problem on the interval $[0,T]$:

  $$\left\{\begin{array}{c}Lu(t)=\sigma (t),a.e.t\in I,\hfill \\ u^{(2k)}(0)=u^{(2k)}(T)=0,k=0,\dots ,n-1.\hfill \end{array}\right.$$
  The Green’s function related to this problem is denoted by $G_D[T]$.
• Mixed problem 1 on the interval $[0,T]$:

  $$\left\{\begin{array}{c}Lu(t)=\sigma (t),a.e.t\in I,\hfill \\ u^{(2k+1)}(0)=u^{(2k)}(T)=0,k=0,\dots ,n-1.\hfill \end{array}\right.$$
  The Green’s function related to this problem is denoted by $G_{M_1}[T]$.
• Mixed problem 2 on the interval $[0,T]$:

  $$\left\{\begin{array}{c}Lu(t)=\sigma (t),a.e.t\in I,\hfill \\ u^{(2k)}(0)=u^{(2k+1)}(T)=0,k=0,\dots ,n-1.\hfill \end{array}\right.$$
  The Green’s function related to this problem is denoted by $G_{M_2}[T]$.
• Periodic problem on the interval $[0,2T]$:

  $$\left\{\begin{array}{c}\tilde{L}u(t)=\tilde{\sigma }(t),a.e.t\in J,\hfill \\ u^{(k)}(0)=u^{(k)}(2T),k=0,\dots ,2n-1.\hfill \end{array}\right.$$
  The Green’s function related to this problem is denoted by $G_P[2T]$.
• Neumann problem on the interval $[0,2T]$:

  $$\left\{\begin{array}{c}\tilde{L}u(t)=\tilde{\sigma }(t),a.e.t\in J,\hfill \\ u^{(2k+1)}(0)=u^{(2k+1)}(2T)=0,k=0,\dots ,n-1.\hfill \end{array}\right.$$
  The Green’s function related to this problem is denoted by $G_N[2T]$.
• Dirichlet problem on the interval $[0,2T]$:

  $$\left\{\begin{array}{c}\tilde{L}u(t)=\tilde{\sigma }(t),a.e.t\in J,\hfill \\ u^{(2k)}(0)=u^{(2k)}(2T)=0,k=0,\dots ,n-1.\hfill \end{array}\right.$$
  The Green’s function related to this problem is denoted by $G_D[2T]$.

Now, we compile some results which compare the values that several Green’s functions take point by point. In [7], the following equalities have been proved:

$$\begin{array}{ccc}\hfill G_N[T](t,s)+G_D[T](t,s)& =& 2G_P[2T](t,s),\forall (t,s)\in I\times I,\hfill \end{array}$$

(1)

$$\begin{array}{ccc}\hfill G_N[T](t,s)-G_D[T](t,s)& =& 2G_P[2T](2T-t,s),\forall (t,s)\in I\times I.\hfill \end{array}$$

(2)

From previous equalities, the following results were deduced.

Corollary 1.

([7] Corollary 4)

1. 

If $G_P[2T]\ge 0$ on $J\times J$, then $G_N[T](t,s)\ge \left|G_D[T](t,s)\right|\forall (t,s)\in I\times I$.

2. 

If $G_P[2T]\le 0$ on $J\times J$, then $G_N[T](t,s)\le -\left|G_D[T](t,s)\right|\forall (t,s)\in I\times I$.

Corollary 2.

([7] Corollary 5)

1. 

If $G_N[2T]\ge 0$ on $J\times J$, then $G_N[T](t,s)\ge \left|G_{M_1}[T](t,s)\right|\forall (t,s)\in I\times I$.

2. 

If $G_N[2T]\le 0$ on $J\times J$, then $G_N[T](t,s)<-\left|G_{M_1}[T](t,s)\right|\forall (t,s)\in I\times I$.

3. 

If $G_D[2T]\le 0$ on $J\times J$, then $G_{M_2}[T](t,s)<-\left|G_D[T](t,s)\right|\forall (t,s)\in I\times I$.

4. 

If $G_D[2T]\ge 0$ on $J\times J$, then $G_{M_2}[T](t,s)\ge \left|G_D[T](t,s)\right|\forall (t,s)\in I\times I$.

Previous results can be improved for the particular case of Hill’s equation.

Corollary 3.

([8] Corollary 4.10) Suppose that $n=1$ and $a_1\equiv 0$.

1. 

If $G_P[2T]\ge 0$ on $J\times J$, then $G_N[T](t,s)\ge -G_D[T](t,s)\ge 0\forall (t,s)\in I\times I$.

2. 

If $G_P[2T]\le 0$ on $J\times J$, then $G_N[T](t,s)<G_D[T](t,s)\le 0\forall (t,s)\in I\times I$.

Corollary 4.

([8] Corollary 4.13) Suppose that $n=1$ and $a_1\equiv 0$.

1. 

If $G_N[2T]\ge 0$ on $J\times J$, then $G_N[T](t,s)\ge -G_{M_1}[T](t,s)\ge 0\forall (t,s)\in I\times I$.

2. 

If $G_N[2T]\le 0$ on $J\times J$, then $G_N[T](t,s)<G_{M_1}[T](t,s)\le 0\forall (t,s)\in I\times I$.

3. 

If $G_D[2T]\le 0$ on $J\times J$, then $G_{M_2}[T](t,s)<G_D[T](t,s)\le 0\forall (t,s)\in I\times I$.

To finish with this preliminary section, we need to introduce a new differential operator.

For any $\lambda \in \mathbb{R}$, consider operator $L[\lambda ]$ defined from operator L in the following way

$$L[\lambda ]u(t)\equiv u^{(2n)}(t)+a_{2n-1}(t)u^{(2n-1)}(t)+\cdots +a_1(t)u^{\prime }(t)+(a_0(t)+\lambda )u(t),t\in I.$$

(3)

In particular, note that $L\equiv L[0]$. When working with this operator, to stress the dependence of the Green’s function on the parameter $\lambda$, we denote by $G[\lambda ,T]$ the Green’s function related to $L[\lambda ]$, with the corresponding subscripts including the boundary conditions. Note that $G[T]\equiv G[0,T]$.

3. Existence of Solutions of Nonlinear Problems

We show how to ensure the existence of solution of some nonlinear problems. To do this, following the line of Cabada, Cid and Sanchez [16], we use the relations found in Corollaries 1 and 2 and the method of lower and upper solutions.

In particular, we consider nonlinear problems that fulfill the following schedule

$$Lu(t)=f(t,u(t)),t\in I,u\in X,$$

(4)

with L nonresonant on the Banach space X.

It is clear that solutions of the previous problem correspond with the fixed points in X of the following integral operator

$$\mathcal{T}u(t):=\left(L^{-1}(f(·,u(·)))\right)(t)={\int }_0^{T}G(t,s)f(s,u(s))ds.$$

In particular, when the Banach space X is $X_{N,T}$, $X_{D,T}$, $X_{M_1,T}$ or $X_{M_2,T}$ (where these spaces denote the subsets of $W^{2n,1}(I)$, which include in each case the corresponding boundary conditions), we obtain, respectively, the following nonlinear problems:

• Neumann problem:

  $$Lu(t)=f(t,u(t)),t\in I,u\in X_{N,T},$$

  (5)
• Dirichlet problem:

  $$Lu(t)=f(t,u(t)),t\in I,u\in X_{D,T},$$

  (6)
• Mixed problem 1:

  $$Lu(t)=f(t,u(t)),t\in I,u\in X_{M_1,T},$$

  (7)
• Mixed problem 2:

  $$Lu(t)=f(t,u(t)),t\in I,u\in X_{M_2,T};$$

  (8)

each of them with its corresponding equivalent integral operator. For the purpose of finding fixed points of the integral operators, we use the following definitions.

Definition 2.

We say that a function $\alpha \in X$ is a lower solution of the problem in Equation (4) if

$$\begin{array}{c}\hfill L\alpha (t)\ge f(t,\alpha (t))for\mathrm{a}.\mathrm{e}.t\in I.\end{array}$$

Analogously, a function $\beta \in X$ is said to be an upper solution of the problem in Equation (4) if

$$\begin{array}{c}\hfill L\beta (t)\le f(t,\beta (t))for\mathrm{a}.\mathrm{e}.t\in I.\end{array}$$

Previous definitions are adapted to each of the considered problems by simply changing X by any of the suitable Banach spaces $X_{N,T}$, $X_{D,T}$, $X_{M_1,T}$ or $X_{M_2,T}$.

Before proving existence results for some of the problems, we introduce the conditions that are used in the remainder of the paper.

First, we ask the nonlinearity f to satisfy the following property:

$(L_0)$

The function $f:I\times \mathbb{R}\to \mathbb{R}$ is a ${\mathrm{L}}^1$-Carathéodory function, that is,

–

$f(·,x)$ is measurable for all $x\in \mathbb{R}$.

–

$f(t,·)$ is continuous for a. e. $t\in I$.

–

For every $R>0$ there exists ${\phi }_R\in {\mathrm{L}}^1(I)$ such that

$$\left|f(t,x)\right|\le {\phi }_R(t),$$

for all $x\in [-R,R]$ and a. e. $t\in I$.

Moreover, given two continuous functions $\alpha$ and $\beta$, we state the following conditions:

$(L_1)$

There exists some $\lambda \in \mathbb{R}$ such that for a. e. $t\in I$ and all $x\in [\alpha (t),\beta (t)]$, it holds that

$$f(t,\alpha (t))+\lambda \alpha (t)\ge f(t,x)+\lambda x\ge f(t,\beta (t))+\lambda \beta (t),$$

and

$$f(t,\alpha (t))+\lambda \alpha (t)\ge 0\ge f(t,\beta (t))+\lambda \beta (t).$$

$(L_2)$

There exists some $\lambda \in \mathbb{R}$ such that for a. e. $t\in I$ and all $x\in [\beta (t),\alpha (t)]$, it holds that

$$f(t,\alpha (t))+\lambda \alpha (t)\ge f(t,x)+\lambda x\ge f(t,\beta (t))+\lambda \beta (t),$$

and

$$f(t,\alpha (t))+\lambda \alpha (t)\ge 0\ge f(t,\beta (t))+\lambda \beta (t).$$

Now, we are in conditions to prove the existence results for the problem in Equation (6).

Theorem 1.

Assume that condition $(L_0)$ holds and let α and β be lower and upper solutions of the Neumann problem in Equation (5), respectively, such that $\alpha (t)\le \beta (t)$ for all $t\in I$. Moreover, assume that there exists some λ for which $G_P[\lambda ,2T]\le 0$ on $J\times J$, $G_D[\lambda ,T]\le 0$ on $I\times I$ and $(L_1)$ holds. Then, there exists a solution u of the Dirichlet problem in Equation (6) such that

$$\alpha (t)\le u(t)\le \beta (t),forallt\in I.$$

Proof. 

Let $\lambda$ be such that $G_P[\lambda ,2T]\le 0$ on $J\times J$, $G_D[\lambda ,T]\le 0$ on $I\times I$ and condition $(L_1)$ holds. Consider the problem

$$L[\lambda ]u(t)=f(t,u(t))+\lambda u(t),t\in I,u\in X_{D,T},$$

(9)

with $L[\lambda ]$ defined in Equation (3). It is clear that $L[\lambda ]u(t)\equiv Lu(t)+\lambda u(t)$ and, as a consequence, the solutions of the problem in Equation (9) coincide with the solutions of Equation (6). In addition, these solutions correspond with fixed points of the following integral operator

$${\mathcal{T}}_D[\lambda ]u(t)={\int }_0^T{G}_D[\lambda ,T](t,s)\left(f(s,u(s))+\lambda u(s)\right)ds.$$

(10)

We divide the proof into several steps:

Step 1:${\mathcal{T}}_D[\lambda ]:\mathcal{C}(I)\to \mathcal{C}(I)$ is a completely continuous operator:

This can be proved using standard techniques, taking into account that the Green’s function has enough regularity (in particular, $G_D[\lambda ,T]\in {\mathcal{C}}^{2n-2}(I\times I)$).

Step 2:$\alpha \le {\mathcal{T}}_D[\lambda ]\alpha$ and $\beta \ge {\mathcal{T}}_D[\lambda ]\beta$.

From Corollary 1, we know that $G_P[\lambda ,2T]\le 0$ on $J\times J$ implies that

$$G_N[\lambda ,T](t,s)\le -\left|G_D[\lambda ,T](t,s)\right|,\mathrm{for}\mathrm{all}t,s\in I.$$

(11)

On the other hand, the fact that $\alpha \in W^{n,1}(I)$ and $L\alpha (t)\ge f(t,\alpha (t))$ for a. e. $t\in I$ means that there exists a nonnegative function $g\in {\mathrm{L}}^1(I)$, such that

$$L\alpha (t)+\lambda \alpha (t)=f(t,\alpha (t))+\lambda \alpha (t)+g(t),\mathrm{for}\mathrm{a}.\mathrm{e}.t\in I.$$

Therefore, since $\alpha \in X_{N,T}$, it holds that

$$\begin{array}{c}\hfill \alpha (t)={\int }_0^T{G}_N[\lambda ,T](t,s)\left(f(s,\alpha (s))+\lambda \alpha (s)\right)ds+{\int }_0^T{G}_N[\lambda ,T](t,s)g(s)ds.\end{array}$$

From Equation (11), it is deduced that $G_N[\lambda ,T]$ is nonpositive. Thus, $G_N[\lambda ,T](t,s)g(s)$ is nonpositive for a. e. $t,s\in I$ and thus the second integral in previous expression is less than or equal to zero. Moreover, we also deduce from Equation (11) that $G_N[\lambda ,T]\le G_D[\lambda ,T]$ on $I\times I$. Therefore, taking into account that (from $(L_1)$) $f(s,\alpha (s))+\lambda \alpha (s)\ge 0$ for a. e. $s\in I$, we obtain the following inequalities for all $t\in I$:

$$\begin{array}{cc}\hfill \alpha (t)& \le {\int }_0^T{G}_N[\lambda ,T](t,s)\left(f(s,\alpha (s))+\lambda \alpha (s)\right)ds\hfill \\ & \le {\int }_0^T{G}_D[\lambda ,T](t,s)\left(f(s,\alpha (s))+\lambda \alpha (s)\right)ds={\mathcal{T}}_D[\lambda ]\alpha (t).\hfill \end{array}$$

Reasoning analogously with $\beta$, we conclude that $\beta \ge {\mathcal{T}}_D[\lambda ]\beta$ on I.

Step 3:${\mathcal{T}}_D[\lambda ]([\alpha ,\beta ])\subset [\alpha ,\beta ]$, where

$$[\alpha ,\beta ]\equiv \left\{u\in \mathcal{C}(I):\alpha (t)\le u(t)\le \beta (t),\mathrm{for}\mathrm{all}t\in I\right\}.$$

We decompose operator ${\mathcal{T}}_D[\lambda ]$ as a composition of two operators. First, consider the Nemytskii operator $N[\lambda ]:\mathcal{C}(I)\to \mathcal{C}(I)$ defined in the following way

$$N[\lambda ]u(t)=f(t,u(t))+\lambda u(t),\mathrm{for}\mathrm{a}.\mathrm{e}.t\in I.$$

On the other hand, consider operator $K[\lambda ]:\mathcal{C}(I)\to \mathcal{C}(I)$ defined as

$$K[\lambda ]\sigma (t)={\int }_0^T{G}_D[\lambda ,T](t,s)\sigma (s)ds,\mathrm{for}\mathrm{all}t\in I.$$

It is clear that

$${\mathcal{T}}_D[\lambda ]=K[\lambda ]\circ N[\lambda ].$$

Moreover, let us see that operator $K[\lambda ]$ is nonincreasing in $[\alpha ,\beta ]$. Indeed, take ${\sigma }_1,{\sigma }_2\in \mathcal{C}(I)$ such that ${\sigma }_1(t)\le {\sigma }_2(t)$ for all $t\in I$. Then, since $G_D[\lambda ,T]$ is nonpositive, it holds that

$$G_D[\lambda ,T](t,s){\sigma }_1(s)\ge G_D[\lambda ,T](t,s){\sigma }_2(s),\mathrm{for}\mathrm{a}.\mathrm{e}.t,s\in I$$

and therefore

$$K[\lambda ]{\sigma }_1(t)={\int }_0^T{G}_D[\lambda ,T](t,s){\sigma }_1(s)ds\ge {\int }_0^T{G}_D[\lambda ,T](t,s){\sigma }_2(s)ds=K[\lambda ]{\sigma }_2(t),\mathrm{for}\mathrm{all}t\in I.$$

Now, let $u\in [\alpha ,\beta ]$. From $(L_1)$, we have that

$$f(t,\alpha (t))+\lambda \alpha (t)\ge f(t,u(t))+\lambda u(t)\ge f(t,\beta (t))+\lambda \beta (t)$$

and so

$$\alpha (t)\le {\mathcal{T}}_D[\lambda ]\alpha (t)\le {\mathcal{T}}_D[\lambda ]u(t)\le {\mathcal{T}}_D[\lambda ]\beta (t)\le \beta (t)$$

and we conclude that ${\mathcal{T}}_D[\lambda ]u\in [\alpha ,\beta ]$ for all $u\in [\alpha ,\beta ]$.

Step 4: Operator ${\mathcal{T}}_D[\lambda ]$ has a fixed point in $X_{D,T}$.

Since the interval $[\alpha ,\beta ]$ is a closed, convex, bounded and nonempty subset of the Banach space X, operator ${\mathcal{T}}_D[\lambda ]$ is completely continuous and it holds that ${\mathcal{T}}_D[\lambda ]([\alpha ,\beta ])\subset [\alpha ,\beta ]$, then we are in the suitable conditions to apply Schauder’s fixed point Theorem ([17]) which ensures us the existence of a fixed point of ${\mathcal{T}}_D[\lambda ]$ on $[\alpha ,\beta ]$. Obviously, this fixed point satisfies Dirichlet boundary conditions and therefore it is a solution of the problem in Equation (6).

Remark 1.

Note that the functions α and β considered in previous theorem are not required to belong to $X_{D,T}$, that is, they may not be lower and upper solutions of Dirichlet problem, that is, the equalities ${\alpha }^{(2k)}(0)=0$, ${\alpha }^{(2k)}(T)=0$, ${\beta }^{(2k)}(0)=0$, ${\beta }^{(2k)}(T)=0$ may fail for some values of k.

In an analogous way, we can prove the following result when $G_P[\lambda ,2T]$ is nonnegative and hypothesis $(L_2)$ holds.

Theorem 2.

Assume that condition $(L_0)$ holds and let α and β be lower and upper solutions of the Neumann problem in Equation (5), respectively, such that $\alpha (t)\ge \beta (t)$ for all $t\in I$. Moreover, assume that there exists some λ for which $G_P[\lambda ,2T]\ge 0$ on $J\times J$, $G_D[\lambda ,T]\ge 0$ on $I\times I$ and $(L_2)$ holds. Then, there exists a solution u of the Dirichlet problem in Equation (6) such that

$$\beta (t)\le u(t)\le \alpha (t),forallt\in I.$$

Remark 2.

We must note that when $G_D[\lambda ,T]$ has constant sign, there exist α and β, lower and upper solutions of Dirichlet problem, respectively, and it is satisfied that

$$f(t,\alpha (t))+\lambda \alpha (t)\ge f(t,x)+\lambda x\ge f(t,\beta (t))+\lambda \beta (t)$$

for a. e. $t\in I$ and all $x\in [\alpha (t),\beta (t)]$, then there exists a solution of the Dirichlet problem in Equation (6) (see [16]). In this case, by adding the hypotheses on the sign of $f(t,\alpha (t))+\lambda \alpha (t)$ and $f(t,\beta (t))+\lambda \beta (t)$, we can ensure the existence of a solution for the problem in Equation (6) when we have lower and upper solutions of the Neumann problem in Equation (5).

Now, using the inequalities in Corollary 2, we can obtain similar results to prove the existence of solutions of Mixed 1 and Dirichlet problems.

Theorem 3.

Assume that condition $(L_0)$ holds and let α and β be lower and upper solutions of the problem in Equation (5), respectively, such that $\alpha (t)\le \beta (t)$ for all $t\in I$. Moreover, assume that there exists some λ for which $G_N[\lambda ,2T]\le 0$ on $J\times J$, $G_{M_1}[\lambda ,T]\le 0$ on $I\times I$ and $(L_1)$ holds. Then, there exists a solution u of the Mixed 1 problem in Equation (7) such that

$$\alpha (t)\le u(t)\le \beta (t),forallt\in I.$$

Theorem 4.

Assume that condition $(L_0)$ holds and let α and β be lower and upper solutions of the problem in Equation (5), respectively, such that $\alpha (t)\ge \beta (t)$ for all $t\in I$. Moreover, assume that there exists some λ for which $G_N[\lambda ,2T]\ge 0$ on $J\times J$, $G_{M_1}[\lambda ,T]\ge 0$ on $I\times I$ and $(L_2)$ holds. Then, there exists a solution u of the Mixed 1 problem in Equation (7) such that

$$\beta (t)\le u(t)\le \alpha (t),forallt\in I.$$

Theorem 5.

Assume that condition $(L_0)$ holds and let α and β be lower and upper solutions of the problem in Equation (8), respectively, such that $\alpha (t)\le \beta (t)$ for all $t\in I$. Moreover, assume that there exists some λ for which $G_D[\lambda ,2T]\le 0$ on $J\times J$, $G_D[\lambda ,T]\le 0$ on $I\times I$ and $(L_1)$ holds. Then, there exists a solution u of the Dirichlet problem in Equation (6) such that

$$\alpha (t)\le u(t)\le \beta (t),forallt\in I.$$

Theorem 6.

Assume that condition $(L_0)$ holds and let α and β be lower and upper solutions of the problem in Equation (8), respectively, such that $\alpha (t)\ge \beta (t)$ for all $t\in I$. Moreover, assume that there exists some λ for which $G_D[\lambda ,2T]\ge 0$ on $J\times J$, $G_D[\lambda ,T]\ge 0$ on $I\times I$ and $(L_2)$ holds. Then, there exists a solution u of the Dirichlet problem in Equation (6) such that

$$\beta (t)\le u(t)\le \alpha (t),forallt\in I.$$

Particular Case: Hill’s Equation

We briefly comment in this section some particularities which may occur when dealing with the case $n=1$ but are not true, in general, for any arbitrary n.

In particular, when considering Definition 2, for the general case it is required that both the lower and the upper solution belong to the Banach spaces $X_{N,T},X_{D,T},X_{M_1,T}$ or $X_{M_2,T}$. For the case $n=1$, it is possible to weaken these definitions by introducing some inequalities in the boundary conditions.

Apart from these generalizations with respect to the definitions of lower and upper solutions, some details also need to be changed in the theorems of existence of solution when $n=1$ and $a_1\equiv 0$. In particular, as a consequence of ([8] Corollary 4.8), it holds that the constant sign of $G_P[\lambda ,2T]$ implies that $G_D[\lambda ,T]$ is nonpositive, thus this hypothesis can be eliminated from Theorem 1. The same way, the constant sign of $G_N[\lambda ,2T]$ implies that $G_{M_1}[\lambda ,T]$ is nonpositive and we can remove this hypothesis from Theorem 3. Finally, the hypothesis that $G_D[\lambda ,T]$ is nonpositive can also be eliminated from Theorem 5, as it can be deduced from the constant sign of $G_D[\lambda ,2T]$.

Furthermore, due to these relations between the constant sign of different Green’s functions, Theorems 2, 4 and 6 do not make sense for the case $n=1$ as their hypotheses are never fulfilled in such case.

Finally, it must be pointed out that for the special case of considering Hill’s equation ($n=1$ and $a_1\equiv 0$), there exists a large number of explicit criteria to ensure the constant sign of the Green’s functions. These criteria have been proved in [6,18,19,20,21,22,23,24] and are compiled in ([9] Sections 3.3.3 and 3.3.4). Obviously, using the aforementioned criteria, it would be possible to reformulate Theorems 1–6 in other terms involving explicit conditions over the coefficients of the equation.

4. An Example

We include in this section an example in which Theorem 1 can be applied.

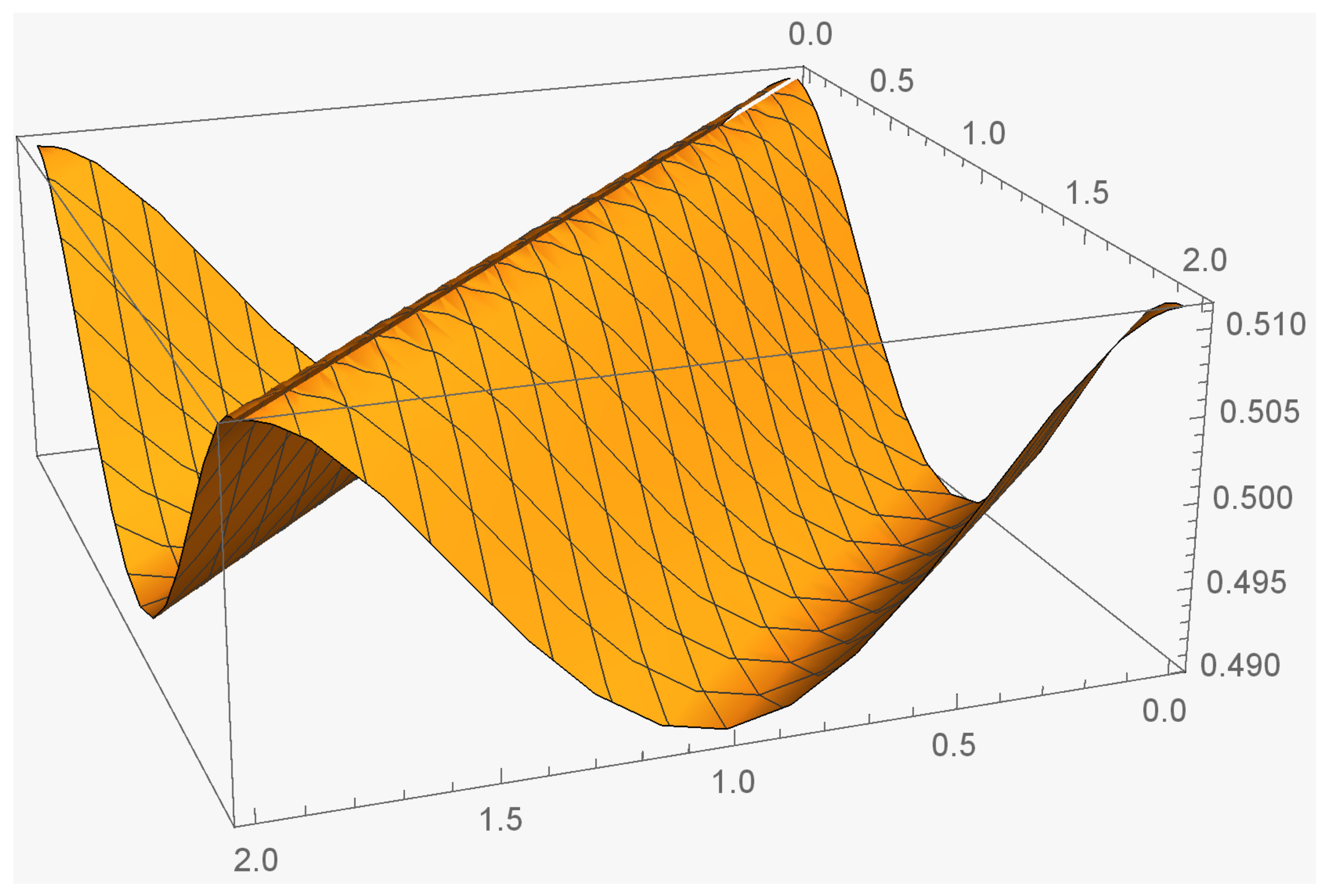
Consider the following nonlinear Dirichlet problem on $[0,1]$

$$\left\{\begin{array}{c}{u}^{(4)}(t)+u(t)=t^2\left(\frac{1}{10}+arctan(u(t))\right),t\in [0,1],\hfill \\ u(0)=u(1)=0,u^{″}(0)=u^{″}(1)=0.\hfill \end{array}\right.$$

(12)

Using [25], we can calculate the Green’s function related to the periodic problem on $[0,2]$

$$\left\{\begin{array}{c}{u}^{(4)}(t)+u(t)=0,t\in [0,2],\hfill \\ u(0)=u(1),u^{\prime }(0)=u^{\prime }(1),u^{″}(0)=u^{″}(1),u^{‴}(0)=u^{‴}(1),\hfill \end{array}\right.$$

(13)

which is nonnegative on $[0,2]\times [0,2]$. This function is represented in Figure 1.

The same way, using [25], we can calculate the Green’s function related to the homogeneous Dirichlet problem

$$\left\{\begin{array}{c}{u}^{(4)}(t)+u(t)=0,t\in [0,1],\hfill \\ u(0)=u(1)=0,u^{″}(0)=u^{″}(1)=0,\hfill \end{array}\right.$$

(14)

which is also nonnegative on $[0,1]\times [0,1]$. This function is represented in Figure 2.

Observe that, with the notation given in Theorem 2, we are choosing $\lambda =0$.

Now, we see that $\alpha (t)=1$ and $\beta (t)=-1$ are lower and upper solutions, respectively, of the Neumann problem

$$\left\{\begin{array}{c}{u}^{(4)}(t)+u(t)=t^2\left(\frac{1}{10}+arctan(u(t))\right),t\in [0,1],\hfill \\ u^{\prime }(0)=u^{\prime }(1)=0,u^{‴}(0)=u^{‴}(1)=0.\hfill \end{array}\right.$$

Indeed,

$$\left\{\begin{array}{c}{\alpha }^{(4)}(t)+\alpha (t)=1\ge t^2\left(\frac{1}{10}+arctan(\alpha (t))\right)=\left(\frac{1}{10}+\frac{\pi }{4}\right)t^2,\mathrm{for}\mathrm{all}t\in [0,1],\hfill \\ {\alpha }^{\prime }(0)={\alpha }^{\prime }(1)=0,{\alpha }^{‴}(0)={\alpha }^{‴}(1)=0.\hfill \end{array}\right.$$

and

$$\left\{\begin{array}{c}{\beta }^{(4)}(t)+\beta (t)=-1\le t^2\left(\frac{1}{10}+arctan(\beta (t))\right)=-\left(-\frac{1}{10}+\frac{\pi }{4}\right)t^2,\mathrm{for}\mathrm{all}t\in [0,1],\hfill \\ {\beta }^{\prime }(0)={\beta }^{\prime }(1)=0,{\beta }^{‴}(0)={\beta }^{‴}(1)=0.\hfill \end{array}\right.$$

Moreover, it holds that for $x\in [-1,1]$, $arctan(x)\in \left[\frac{-\pi }{4},\frac{\pi }{4}\right]$ and so

$$\left(\frac{1}{10}+\frac{\pi }{4}\right)t^2\ge \left(\frac{1}{10}+arctan(x)\right)t^2\ge \left(\frac{1}{10}-\frac{\pi }{4}\right)t^2.$$

Finally, we have that $f(t,\alpha (t))=\left(\frac{1}{10}+\frac{\pi }{4}\right)t^2\ge 0$ and $f(t,\beta (t))=\left(\frac{1}{10}-\frac{\pi }{4}\right)t^2\le 0$ for all $t\in [0,1]$. Thus, hypothesis $(L_2)$ is satisfied.

Therefore, we are in conditions to apply Theorem 2, which warrants the existence of a solution u of the Dirichlet problem in Equation (12) such that

$$-1\le u(t)\le 1,\mathrm{for}\mathrm{all}t\in [0,1].$$

Note that $\alpha$ and $\beta$ are not lower and upper solutions of the Dirichlet problem, as they are defined in Definition 2.

5. An Alternative Approach

In this section, we present another method to prove the existence of solution of the general $2n$th-order problem, where the method of lower and upper solutions is combined with the Krasnoselskii’s fixed point Theorem. We follow the line of Cabada and Cid [26], substituting the hypothesis of the existence of an upper solution of the corresponding problem, by the existence of an upper solution of a different problem.

We consider two subsections, the first one for the case when the Dirichlet Green’s function is nonpositive, and the second one for the converse case.

5.1. Negative Case

We assume that there exists some $\lambda$ and some subinterval $[a,b]\subset I$ for which the following condition holds:

$(C_0)$

$G_P[\lambda ,2T](2T-t,s)\le 0$ for $(t,s)\in I\times I$ and $G_P[\lambda ,2T](2T-t,s)<0$ for $(t,s)\in [a,b]\times I$.

In this case, from Equation (2), we have

$$G_D[\lambda ,T](t,s)-G_N[\lambda ,T](t,s)>0\forall (t,s)\in [a,b]\times I.$$

Therefore, we may define

$$\begin{array}{cc}\hfill m_1& =\underset{t\in [a,b],s\in I}{min}\left\{G_D[\lambda ,T](t,s)-G_N[\lambda ,T](t,s)\right\}>0,\hfill \\ \hfill m_2& =\underset{t,s\in I\times I}{max}\left\{G_D[\lambda ,T](t,s)-G_N[\lambda ,T](t,s)\right\}\hfill \end{array}$$

and

$${\gamma }_2=\frac{m_1}{m_2}\in (0,1].$$

Let $K_2$ be the following cone

$$K_2=\left\{u\in W^{2n,1}(I);u(t)\ge 0,\forall t\in I,\underset{t\in [a,b]}{min}u(t)\ge {\gamma }_2{\parallel u\parallel }_{\infty }\right\}.$$

Let us denote by ⪯ the partial ordering induced by the cone: $x⪯y$ if and only if $y-x\in K_2$. We use the notation $x\prec y$ for $y-x\in K_2\backslash \{\theta \}$, where $\theta$ denotes the zero element of the Banach space.

We use the following fixed point theorem due to Krasnoselskii.

Theorem 7.

([27] Theorem 13.D) Let N be a real Banach space with order cone K. Suppose that the operator $\mathcal{T}:K\to K$ is completely continuous and a cone expansion, that is, there exist $0<r<R$ such that

$$\mathcal{T}x\ngeqq xforallx\in K,\parallel x\parallel =r$$

and

$$\mathcal{T}x\nleqq xforallx\in K,\parallel x\parallel =R.$$

Then, $\mathcal{T}$ has a fixed point on K with $r<\parallel x\parallel <R$.

First, we prove the following preliminary result, which is the line of ([26] Theorem 2.1).

Lemma 1.

Suppose that there exists $\lambda \in \mathbb{R}$ for which condition $(C_0)$ is fulfilled and operator ${\mathcal{T}}_D[\lambda ]$, given in Equation (10), is nondecreasing and completely continuous. Let

$$S=\{x\in K_2;{\mathcal{T}}_D[\lambda ]x⪯x\}$$

and suppose that:

$(i)$

There exists $\beta \in int(K_2)$ such that ${\mathcal{T}}_N[\lambda ]\beta ⪯\beta$, with

$${\mathcal{T}}_N[\lambda ]x(t)={\int }_0^T{G}_N[\lambda ,T](t,s)(f(s,x(s))+\lambda x(s))ds.$$

$(ii)$

There exists some $R>0$ such that $S\cap \{x\in K_2;\parallel x\parallel =R\}=\varnothing$.

$(iii)$

$f(t,\beta (t))+\lambda \beta (t)\le 0$ for a. e. $t\in I$.

Then, there exists $x\in K_2$, $x\ne \theta$ such that ${\mathcal{T}}_D[\lambda ]x=x$.

Proof. 

Since $\beta \in int(K_2)$, there exists some $r>0$ such that $\overline{B(\beta ,r)}\subset K_2$.

We distinguish two cases:

(I)

There exists some $x\in K_2$, with ${\parallel x\parallel }_{\infty }=r$, such that ${\mathcal{T}}_D[\lambda ]x⪰x$.

Since ${\parallel x\parallel }_{\infty }=r$, then $\beta -x\in \overline{B(\beta ,r)}\subset K_2$, which implies that $x⪯\beta$.

Consider the sequence $\{x_n\}$ defined in the following way:

$$\left\{\begin{array}{c}{x}_0=x,\hfill \\ x_n={\mathcal{T}}_D[\lambda ]x_{n-1}.\hfill \end{array}\right.$$

Since $x⪯\beta$ and ${\mathcal{T}}_D[\lambda ]$ is nondecreasing, it occurs that

$$0\prec x⪯x_n={\mathcal{T}}_D^n[\lambda ]x⪯{\mathcal{T}}_D^n[\lambda ]\beta ,\forall n\in \mathbb{N}.$$

Let us prove now that

$${\mathcal{T}}_D[\lambda ]\beta ⪯{\mathcal{T}}_N[\lambda ]\beta .$$

Clearly, from $(C_0)$ and Equation (2), for all $t\in I$, it holds that

$${\mathcal{T}}_N[\lambda ]\beta (t)-{\mathcal{T}}_D[\lambda ]\beta (t)={\int }_0^T(G_N[\lambda ,T](t,s)-G_D[\lambda ,T](t,s))(f(s,\beta (s))+\lambda \beta (s))ds\ge 0.$$

Moreover, for all $t\in I$,

$$\begin{array}{cc}\hfill {\mathcal{T}}_N[\lambda ]\beta (t)-{\mathcal{T}}_D[\lambda ]\beta (t)& ={\int }_0^T(G_N[\lambda ,T](t,s)-G_D[\lambda ,T](t,s))(f(s,\beta (s))+\lambda \beta (s))ds\hfill \\ & \le m_2{\int }_0^T|f(s,\beta (s))+\lambda \beta (s)|ds,\hfill \end{array}$$

which implies that

$$\parallel {\mathcal{T}}_N[\lambda ]\beta -{\mathcal{T}}_D{[\lambda ]\beta \parallel }_{\infty }\le m_2{\int }_0^T|f(s,\beta (s))+\lambda \beta (s)|ds$$

and, since

$$\begin{array}{cc}\hfill {\mathcal{T}}_N[\lambda ]\beta (t)-{\mathcal{T}}_D[\lambda ]\beta (t)& ={\int }_0^T(G_N[\lambda ,T](t,s)-G_D[\lambda ,T](t,s))(f(s,\beta (s))+\lambda \beta (s))ds\hfill \\ & \ge m_1{\int }_0^T|f(s,\beta (s))+\lambda \beta (s)|ds,\forall t\in [a,b],\hfill \end{array}$$

we have that

$$\underset{t\in [a,b]}{min}\left\{{\mathcal{T}}_N[\lambda ]\beta (t)-{\mathcal{T}}_D[\lambda ]\beta (t)\right\}\ge {\gamma }_2{\parallel {\mathcal{T}}_N[\lambda ]\beta -{\mathcal{T}}_D[\lambda ]\beta \parallel }_{\infty }.$$

Therefore, it is fulfilled that

$${\mathcal{T}}_D[\lambda ]\beta ⪯{\mathcal{T}}_N[\lambda ]\beta ⪯\beta$$

and so

$$0\prec x⪯x_n={\mathcal{T}}_D^n[\lambda ]x⪯{\mathcal{T}}_D^n[\lambda ]\beta ⪯\beta ,\forall n\in \mathbb{N}.$$

As a consequence, $x_n⪯\beta$ for all $n\in \mathbb{N}$ and this implies that $\parallel x_n{\parallel }_{\infty }\le {\parallel \beta \parallel }_{\infty }$ and so the sequence $\{x_n\}$ is bounded.

In addition, since ${\mathcal{T}}_D[\lambda ]$ is completely continuous, it holds that $\{{\mathcal{T}}_D[\lambda ]x_n\}=\{x_n\}$ is relatively compact and, consequently, there exists a subsequence $\{x_{n_k}\}$ which converges to $x^{*}$. Thus, since ${\mathcal{T}}_D[\lambda ]$ is nondecreasing, $x_{n_k}⪯x^{*}$ for all $k\in \mathbb{N}$, and so,

$$x_{n_k}⪯x_n⪯x^{*},\forall n\ge n_k.$$

Then,

$$\parallel x^{*}-x_n{\parallel }_{\infty }\le {\parallel x^{*}-x_{n_k}\parallel }_{\infty },$$

from which we deduce that $\{x_n\}$ converges to $x^{*}$ and, from the continuity of ${\mathcal{T}}_D[\lambda ]$, $x^{*}={\mathcal{T}}_D[\lambda ]x^{*}$, that is, $x^{*}$ is a fixed point of ${\mathcal{T}}_D[\lambda ]$ and, moreover,

$$\theta ⪯x⪯x^{*}⪯\beta .$$

(II)

${\mathcal{T}}_D[\lambda ]x\ngeqq x$ for all $x\in K_2$ such that ${\parallel x\parallel }_{\infty }=r$.

From condition $(ii)$, we know that there exists some constant $R>r$ such that ${\mathcal{T}}_D[\lambda ]x\nleqq x$ for all $x\in K_2$ such that ${\parallel x\parallel }_{\infty }=R$.

Then, from Theorem 7, we conclude the existence of a fixed point of ${\mathcal{T}}_D[\lambda ]$.

□

Remark 3.

As pointed out in ([26] Remark 2.1), it must be observed that in case S is bounded, condition $(ii)$ in previous lemma holds trivially.

Remark 4.

The proof of Lemma 1 follows the line of that of Theorem 2.1 in [26], except for the new part in which we prove that ${\mathcal{T}}_D[\lambda ]\beta ⪯{\mathcal{T}}_N[\lambda ]\beta$. For the reader’s convenience, we have decided to include the whole proof and not only the modified part.

In the sequel, to deduce an existence result for the nonlinear Dirichlet problem in Equation (6), we introduce the following condition

$(C_1)$

There exists a nonpositive function $\varphi \in {\mathrm{L}}^1(I)$ and a constant ${\gamma }_1\in (0,1]$ such that

$$G_D[\lambda ,T](t,s)\ge \varphi (s),\forall t\in I,\mathrm{a}.\mathrm{e}.s\in I$$

and

$$G_D[\lambda ,T](t,s)\le {\gamma }_1\varphi (s),\forall t\in [a,b],\mathrm{a}.\mathrm{e}.s\in I.$$

Let K be the following cone

$$K=\left\{u\in W^{2n,1}(I);u(t)\ge 0,\forall t\in I,\underset{t\in [a,b]}{min}x(t)\ge \gamma {\parallel x\parallel }_{\infty }\right\},$$

with

$$\gamma =min\{{\gamma }_1,{\gamma }_2\},$$

and ${\gamma }_1$ given in $(C_1)$.

Theorem 8.

Suppose that there exists $\lambda \in \mathbb{R}$ such that $G_D[\lambda ,T]\le 0$ on $I\times I$ and $(C_0)$–$(C_1)$ and the following assumptions hold:

$(C_2)$

$f(t,·)$ is nonincreasing for a. e. $t\in I$.

$(C_3)$

$f(t,x)+\lambda x\le 0$ for a. e. $t\in I$ and all $x\ge 0$.

$(C_4)$

$\underset{x\to \infty }{lim}\frac{f(t,x)}{x}=-\infty$ uniformly in t.

$(C_5)$

There exists $\beta \in int(K)$ such that ${\mathcal{T}}_N[\lambda ]\beta ⪯\beta$.

Then, there exists a nonnegative solution $u\in K$ for the Dirichlet problem in Equation (6).

Proof. 

Consider operator ${\mathcal{T}}_D[\lambda ]$ given in Equation (10).

We divide the proof into several steps.

Step 1:${\mathcal{T}}_D[\lambda ]:\mathcal{C}(I)\to \mathcal{C}(I)$ is a completely continuous operator:

This can be proved using standard techniques, taking into account that the Green’s function has enough regularity (in particular, $G_D[\lambda ,T]\in {\mathcal{C}}^{2n-2}(I\times I)$).

Step 2:${\mathcal{T}}_D[\lambda ](K)\subset K$:

Let $x\in K$. Then, ${\mathcal{T}}_D[\lambda ]x(t)\ge 0$ for all $t\in I$ as a direct consequence of $(C_3)$ and the non positiveness of $G_D$ on $I\times I$.

Now, from $(C_3)$ and $(C_5)$, for all $t\in I$:

$$\begin{array}{c}\hfill {\mathcal{T}}_D[\lambda ]x(t)={\int }_0^T{G}_D[\lambda ,T](t,s)\left(f(s,x(s))+\lambda x(s)\right)ds\le {\int }_0^T\varphi (s)\left(f(s,x(s))+\lambda x(s)\right)ds\end{array}$$

and so

$$\begin{array}{c}\hfill \parallel {\mathcal{T}}_D{[\lambda ]x\parallel }_{\infty }\le {\int }_0^T\varphi (s)\left(f(s,x(s))+\lambda x(s)\right)ds.\end{array}$$

Moreover, for $t\in [a,b]$

$$\begin{array}{c}\hfill {\mathcal{T}}_D[\lambda ]x(t)={\int }_0^T{G}_D[\lambda ,T](t,s)\left(f(s,x(s))+\lambda x(s)\right)ds\ge {\gamma }_1{\int }_0^T\varphi (s)\left(f(s,x(s))+\lambda x(s)\right)ds.\end{array}$$

Therefore,

$$\underset{t\in [a,b]}{min}{\mathcal{T}}_D[\lambda ]x(t)\ge {\gamma }_1{\int }_0^T\varphi (s)\left(f(s,x(s))+\lambda x(s)\right)ds\ge {\gamma }_1\parallel {\mathcal{T}}_D{[\lambda ]x\parallel }_{\infty }\ge \gamma {\parallel {\mathcal{T}}_D[\lambda ]x\parallel }_{\infty },$$

and, clearly, ${\mathcal{T}}_D[\lambda ]x\in K$.

Step 3:${\mathcal{T}}_D[\lambda ]$ is a nondecreasing operator:

Take $x_1,x_2\in \mathcal{C}(I)$ such that $x_1⪰x_2$. From $(C_2)$, it is easy to see that ${\mathcal{T}}_D[\lambda ]x_1(t)\ge {\mathcal{T}}_D[\lambda ]x_2(t)$ for all $t\in I$. Moreover, with a similar argument to the one made in Step 2, one can check that

$$\underset{t\in [a,b]}{min}\left\{{\mathcal{T}}_D[\lambda ]x_1(t)-{\mathcal{T}}_D[\lambda ]x_2(t)\right\}\ge \gamma {\parallel {\mathcal{T}}_D[\lambda ]x_1-{\mathcal{T}}_D[\lambda ]x_2\parallel }_{\infty },$$

and so ${\mathcal{T}}_D[\lambda ]x_1⪰{\mathcal{T}}_D[\lambda ]x_2$.

Step 4:$S=\{x\in K;{\mathcal{T}}_D[\lambda ]x⪯x\}$ is bounded.

Take $M<0$ such that

$$M+\lambda <\frac{1}{{\displaystyle \underset{t\in [a,b]}{max}\left\{{\int }_a^b{G}_D[\lambda ,T](t,s)ds\right\}}}.$$

From condition $(C_4)$, there exists some positive constant $\alpha$ such that

$$f(t,x)>Mx,\forall x>\alpha .$$

Take $x\in K$ such that ${\displaystyle \underset{t\in [a,b]}{min}\{x(t)\}>\alpha }$. Then,

$$f(t,x(t))<Mx(t),\forall t\in [a,b].$$

Let now $t_0\in [a,b]$ be such that ${\displaystyle x(t_0)=\underset{t\in [a,b]}{min}x(t)}$. It occurs that for $t\in [a,b]$

$$\begin{array}{cc}\hfill {\mathcal{T}}_D[\lambda ]x(t)& ={\int }_0^T{G}_D[\lambda ,T](t,s)\left(f(s,x(s))+\lambda x(s)\right)ds\hfill \\ & \ge {\int }_a^b{G}_D[\lambda ,T](t,s)\left(f(s,x(s))+\lambda x(s)\right)ds\hfill \\ & >{\int }_a^b{G}_D[\lambda ,T](t,s)(M+\lambda )x(s)ds\hfill \\ & \ge (M+\lambda )x(t_0){\int }_a^b{G}_D[\lambda ,T](t,s)ds>x(t_0).\hfill \end{array}$$

In particular,

$${\mathcal{T}}_D[\lambda ]x(t_0)>x(t_0),$$

and so ${\mathcal{T}}_D[\lambda ]x\nleqq x$.

Therefore, $x\notin S$. We conclude that whenever $x\in S$ it must occur that ${\displaystyle \underset{t\in [a,b]}{min}x(t)\le \alpha }$ and thus

$$\alpha \ge \underset{t\in [a,b]}{min}x(t)\ge \gamma {\parallel x\parallel }_{\infty },$$

and we deduce that S is bounded.

Then, as a consequence from Lemma 1, we conclude that operator ${\mathcal{T}}_D[\lambda ]$ has a nontrivial fixed point in the cone K, which is a nontrivial solution for the Dirichlet problem in Equation (6). □

As a consequence of previous result, we obtain the following corollary.

Corollary 5.

Suppose that there exists $\lambda \in \mathbb{R}$ such that $G_D[\lambda ,T]\le 0$ on $I\times I$ and $(C_0)$–$(C_1)$ and the following assumptions hold:

$(C_2)$

$f(t,·)$ is nonincreasing for a. e. $t\in I$.

$(C_3)$

$f(t,x)+\lambda x\le 0$ for a. e. $t\in I$ and all $x\ge 0$.

$(C_4)$

$\underset{x\to \infty }{lim}\frac{f(t,x)}{x}=-\infty$ uniformly in t.

$(C_5^{*})$

$G_N[\lambda ,T]\le 0$ on $I\times I$ and there exists $\beta \in int(K)$ an upper solution of the Neumann problem in Equation (5).

Then, there exists a nonnegative solution $u\in K$ for the Dirichlet problem in Equation (6).

Proof. 

The proof follows from the fact that, as it has been made in the proof of Theorem 1, condition $(C_5^{*})$ implies that ${\mathcal{T}}_N[\lambda ]\beta ⪯\beta$. □

We must note that for, the second-order equation (that is, $n=1$), it occurs that if $G_P[\lambda ,2T]\le 0$ on $J\times J$, then $G_P[\lambda ,2T]<0$ on $J\times J$ and so $[a,b]=J$ in the second part of condition $(C_0)$. This is not true, in general, for the even-order equation when $n>1$. We show now an example in which $G_P[\lambda ,2T]\le 0$ on $J\times J$ and condition $(C_0)$ holds and another one in which $G_P[\lambda ,2T]\le 0$ on $J\times J$ but condition $(C_0)$ does not hold.

Example 2.

Consider the fourth-order periodic problem on $[0,2T]=[0,1]$

$$\left\{\begin{array}{c}{u}^{(4)}(t)+Mu(t)=0,t\in [0,1]\hfill \\ u^{(i)}(0)=u^{(i)}(1),i=0,\dots ,3.\hfill \end{array}\right.$$

In this case, as it has been proved in ([4] Lemma 2.10 and Remark 2.6), the related Green’s function is strictly negative for $M\in \left(-{(2\pi \mu )}^4,0\right)$, where $\mu \approx 0.7528094$ is the unique solution in $\left(\frac{1}{2},1\right)$ of the equation

$$-tanhm\pi =tanm\pi .$$

Moreover, for $M=-{(2\pi \mu )}^4$, the Green’s function is nonpositive on $[0,1]\times [0,1]$ and vanishes only on the diagonal of its square of definition and on the points $(0,1)$ and $(1,0)$. Therefore, if we take $[a,b]\subset (0,1/2)$, our method can be applied for $M\in \left[-{(2\pi \mu )}^4,0\right)$.

Example 3.

Consider now the sixth-order periodic problem on $[0,2T]=[0,1]$

$$\left\{\begin{array}{c}{u}^{(6)}(t)+Mu(t)=0,t\in [0,1]\hfill \\ u^{(i)}(0)=u^{(i)}(1),i=0,\dots ,5.\hfill \end{array}\right.$$

In this case, as proved in ([28] Theorem 4.2), the related Green’s function is strictly negative for $M\in \left(-{(2\pi \overline{\mu })}^6,0\right)$, where $\overline{\mu }\approx 1.010105$ is the unique solution in $\left(\frac{\sqrt{3}}{2},\frac{2}{\sqrt{3}}\right)$ of the equation

$$sinhm\pi \left(2sinhm\pi /2cos\sqrt{3}m\pi /2+\sqrt{3}coshm\pi /2sin\sqrt{3}m\pi /2\right)=cos\sqrt{3}m\pi -coshm\pi .$$

Moreover, for $M=-{(2\pi \overline{\mu })}^6$, the Green’s function is nonpositive on $[0,1]\times [0,1]$ and vanishes only on the lines

$$\{(t,s)\in [0,1]\times [0,1];s=t+1/2\}$$

and

$$\{(t,s)\in [0,1]\times [0,1];s=t-1/2\}.$$

Therefore, our method can be applied for $M\in \left(-{(2\pi \mu )}^6,0\right)$ but not for $M=-{(2\pi \overline{\mu })}^6$ as it is not possible to find any interval $[a,b]\subset \left[0,\frac{1}{2}\right]$ such that $G_P$ is strictly positive on $(1-t,s)$ for all $(t,s)\in [a,b]\times \left[0,\frac{1}{2}\right]$.

5.2. Positive Case

We formulate now the corresponding results for the case when the Dirichlet Green’s function is nonnegative.

We assume in this case that there exist some $\lambda$ and some subinterval $[a,b]\subset I$ for which the following conditions hold:

$({\tilde{C}}_0)$

$G_P[\lambda ,2T](2T-t,s)\ge 0$ for $(t,s)\in I\times I$ and $G_P[\lambda ,2T](2T-t,s)>0$ for $(t,s)\in [a,b]\times I$.

$({\tilde{C}}_1)$

There exists a nonnegative function $\tilde{\varphi }\in {\mathrm{L}}^1(I)$ and a constant ${\tilde{\gamma }}_1\in (0,1]$ such that

$$G_D[\lambda ,T](t,s)\ge \tilde{\varphi }(s),\forall t\in I,\mathrm{a}.\mathrm{e}.s\in I$$

and

$$G_D[\lambda ,T](t,s)\le {\tilde{\gamma }}_1\tilde{\varphi }(s),\forall t\in [a,b],\mathrm{a}.\mathrm{e}.s\in I.$$

In this case, from Equation (2),

$$G_N[\lambda ,T](t,s)-G_D[\lambda ,T](t,s)>0\forall (t,s)\in [a,b]\times I.$$

Therefore, we take now

$$\begin{array}{cc}\hfill {\tilde{m}}_1& =\underset{t\in [a,b],s\in I}{min}\left\{G_N[\lambda ,T](t,s)-G_D[\lambda ,T](t,s)\right\}>0,\hfill \\ \hfill {\tilde{m}}_2& =\underset{t,s\in I\times I}{max}\left\{G_N[\lambda ,T](t,s)-G_D[\lambda ,T](t,s)\right\}\hfill \end{array}$$

and

$${\tilde{\gamma }}_2=\frac{{\tilde{m}}_1}{{\tilde{m}}_2}\in (0,1],$$

and we consider again ${\tilde{K}}_2$ the following cone

$${\tilde{K}}_2=\left\{u\in W^{2n,1}(I);u(t)\ge 0,\forall t\in I,\underset{t\in [a,b]}{min}x(t)\ge {\tilde{\gamma }}_2{\parallel x\parallel }_{\infty }\right\}.$$

Now, the existence results are the ones below.

Lemma 2.

Suppose that there exists $\lambda \in \mathbb{R}$ for which condition $({\tilde{C}}_0)$ is fulfilled and operator ${\mathcal{T}}_D[\lambda ]$, given in Equation (10), is nondecreasing and completely continuous. Let

$$S=\{x\in {\tilde{K}}_2;{\mathcal{T}}_D[\lambda ]x⪯x\}$$

and suppose that:

$(i)$

There exists $\beta \in int({\tilde{K}}_2)$ such that ${\mathcal{T}}_N[\lambda ]\beta ⪯\beta$, with

$${\mathcal{T}}_N[\lambda ]x(t)={\int }_0^T{G}_N[\lambda ,T](t,s)(f(s,x(s))+\lambda x(s))ds.$$

$(ii)$

There exists some $R>0$ such that $S\cap \{x\in {\tilde{K}}_2;\parallel x\parallel =R\}=\varnothing$.

$(iii)$

$f(t,\beta (t))+\lambda \beta (t)\ge 0$ for a. e. $t\in I$.

Then, there exists $x\in {\tilde{K}}_2$, $x\ne \theta$ such that ${\mathcal{T}}_D[\lambda ]x=x$.

Now, by defining

$$\tilde{K}=\left\{u\in W^{2n,1}(I);u(t)\ge 0,\forall t\in I,\underset{t\in [a,b]}{min}x(t)\ge \tilde{\gamma }{\parallel x\parallel }_{\infty }\right\},$$

with $\tilde{\gamma }=min\{{\tilde{\gamma }}_1,{\tilde{\gamma }}_2\}$, we have the following existence result for the nonlinear Dirichlet problem in Equation (6).

Theorem 9.

Suppose that there exists $\lambda \in \mathbb{R}$ such that $G_D[\lambda ,T]\ge 0$ on $I\times I$ and $({\tilde{C}}_0)$–$({\tilde{C}}_1)$ and the following assumptions hold:

$({\tilde{C}}_2)$

$f(t,·)$ is nondecreasing for a. e. $t\in I$.

$({\tilde{C}}_3)$

$f(t,x)+\lambda x\ge 0$ for a. e. $t\in I$ and all $x\ge 0$.

$({\tilde{C}}_4)$

$\underset{x\to \infty }{lim}\frac{f(t,x)}{x}=\infty$ uniformly in t.

$({\tilde{C}}_5)$

There exists $\beta \in int(\tilde{K})$ such that ${\mathcal{T}}_N[\lambda ]\beta ⪯\beta$.

Then, there exists a nonnegative solution $u\in \tilde{K}$ for the Dirichlet problem in Equation (6).

We also have the following corollary.

Corollary 6.

Suppose that there exists $\lambda \in \mathbb{R}$ such that $G_D[\lambda ,T]\ge 0$ on $I\times I$ and $({\tilde{C}}_0)$–$({\tilde{C}}_1)$ and the following assumptions hold:

$({\tilde{C}}_2)$

$f(t,·)$ is nondecreasing for a. e. $t\in I$.

$({\tilde{C}}_3)$

$f(t,x)+\lambda x\ge 0$ for a. e. $t\in I$ and all $x\ge 0$.

$({\tilde{C}}_4)$

$\underset{x\to \infty }{lim}\frac{f(t,x)}{x}=\infty$ uniformly in t.

$({\tilde{C}}_5^{*})$

$G_N[\lambda ,T]\ge 0$ on $I\times I$ and there exists $\beta \in int(\tilde{K})$ an upper solution of the Neumann problem in Equation (5).

Then, there exists a nonnegative solution $u\in \tilde{K}$ for the Dirichlet problem in Equation (6).

We must note that the results in this subsection can never be applied for the second-order equation, since in such a case the Dirichlet Green’s function can not be nonnegative. For higher-order equations, as in previous subsection, it is easy to find examples in which condition $(\widetilde{C_0})$ holds and also examples in which it does not hold.