Rheological Behavior of Rockmass Supported with Rockbolts Based on Viscoelastic Analysis Method
Abstract
:1. Introduction
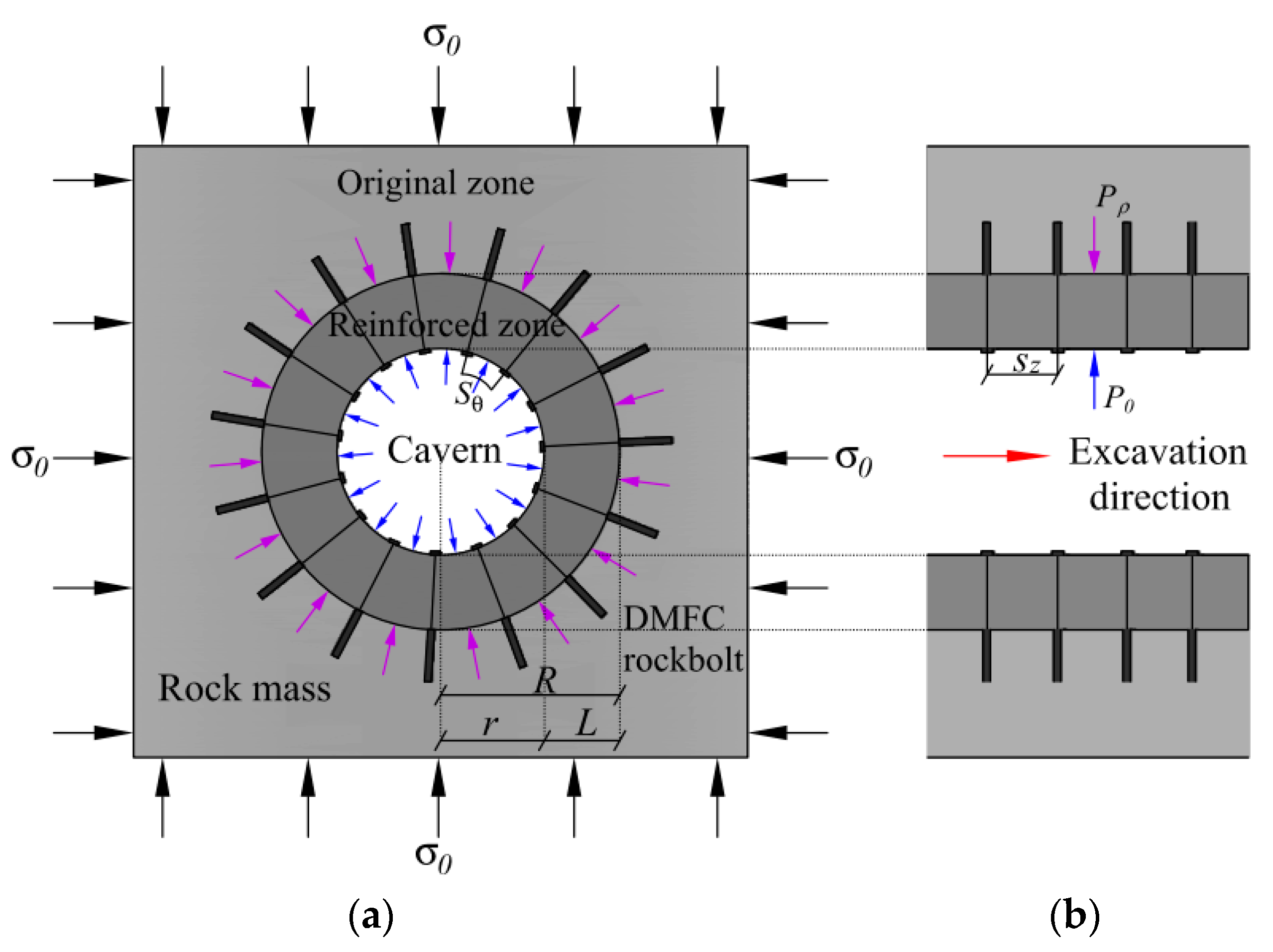
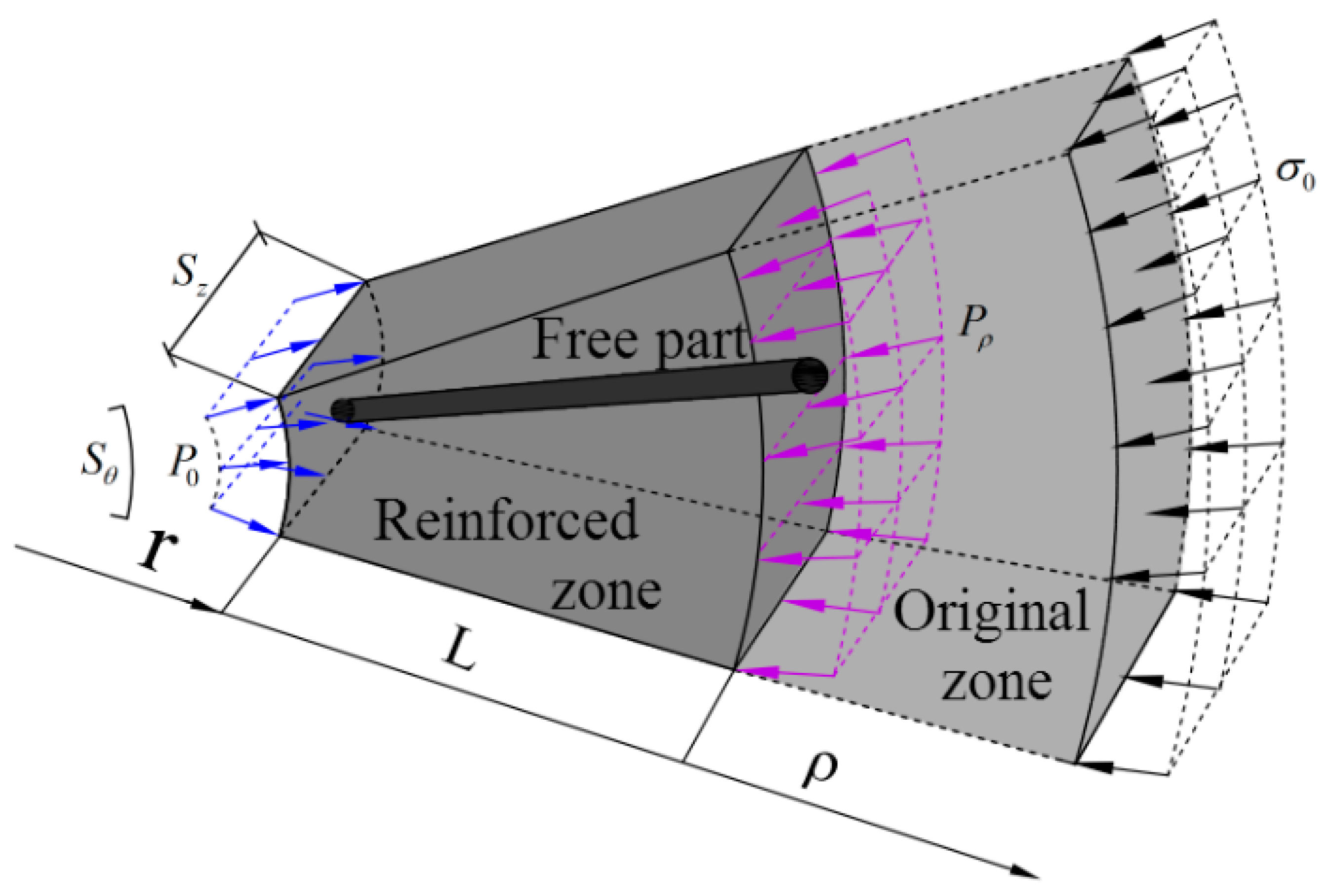
2. Interaction Model
3. Viscoelastic Analytical Solutions in K–K Constitutive Model
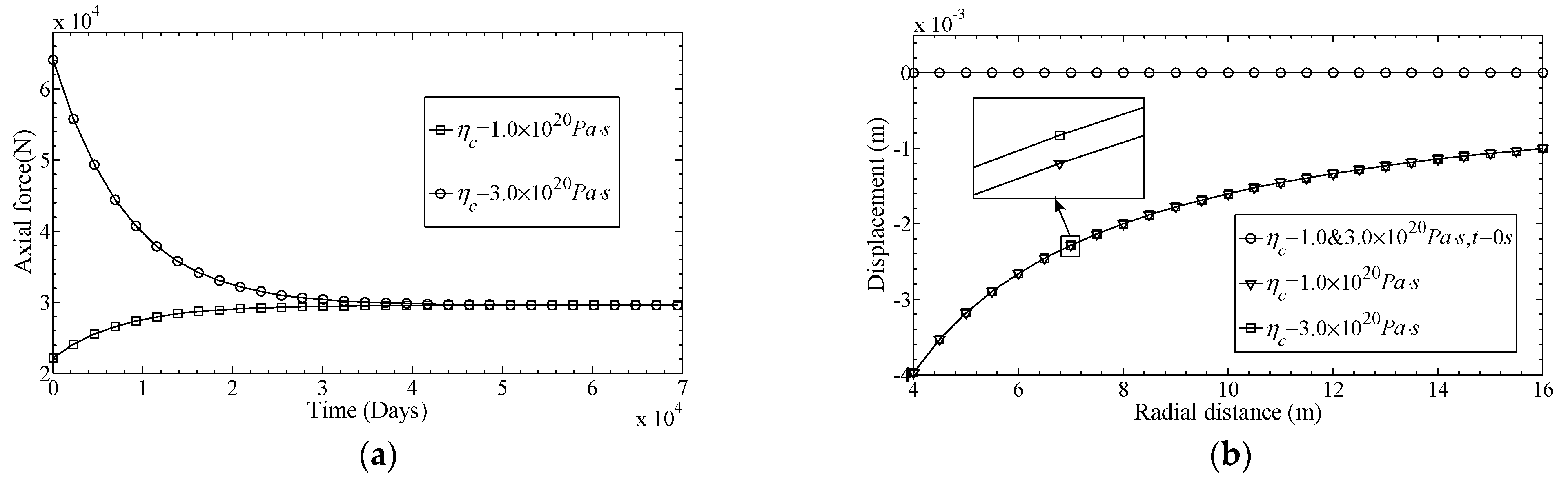
4. Analysis of Analytical Solutions
4.1. K-K Rheology Model
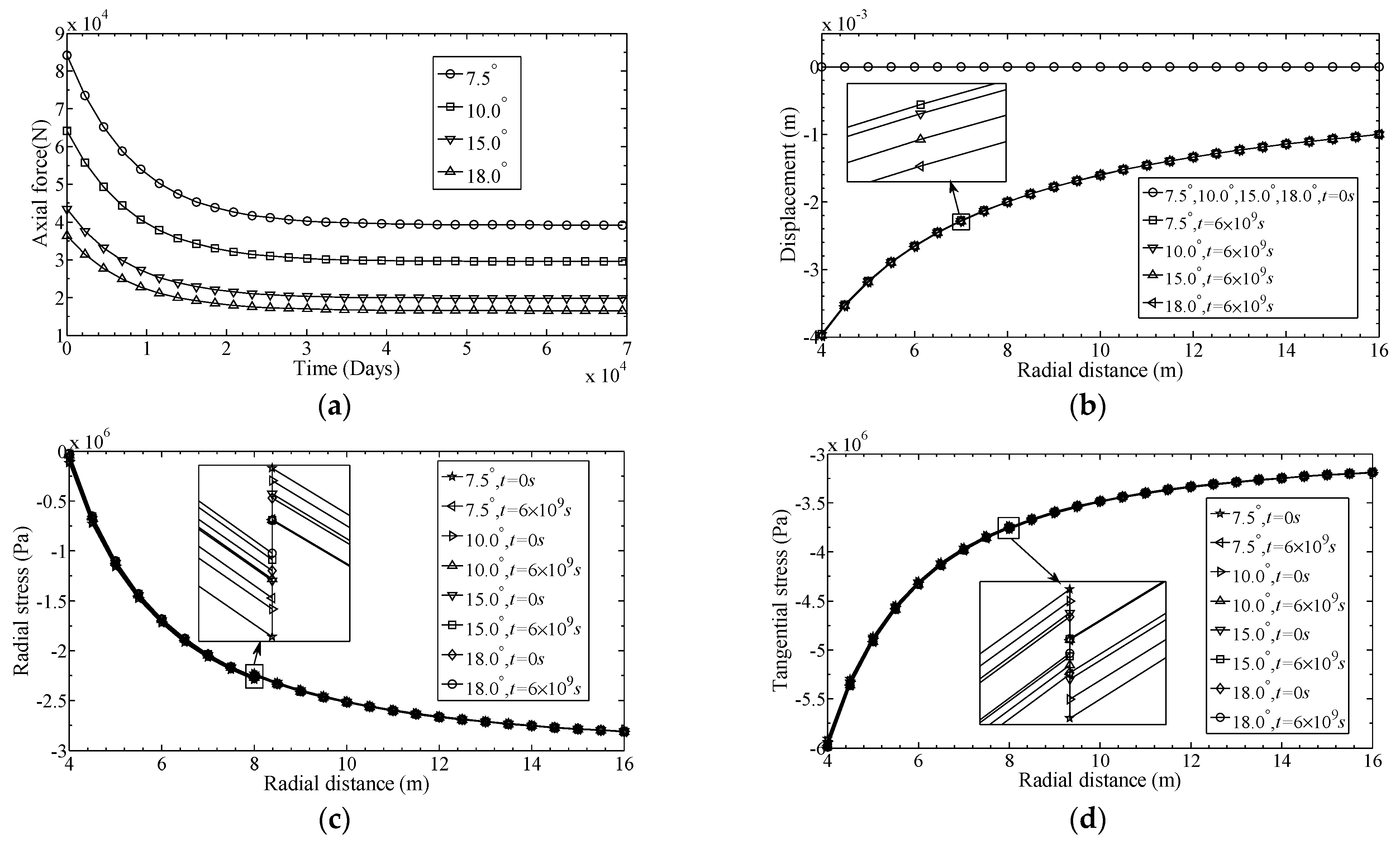
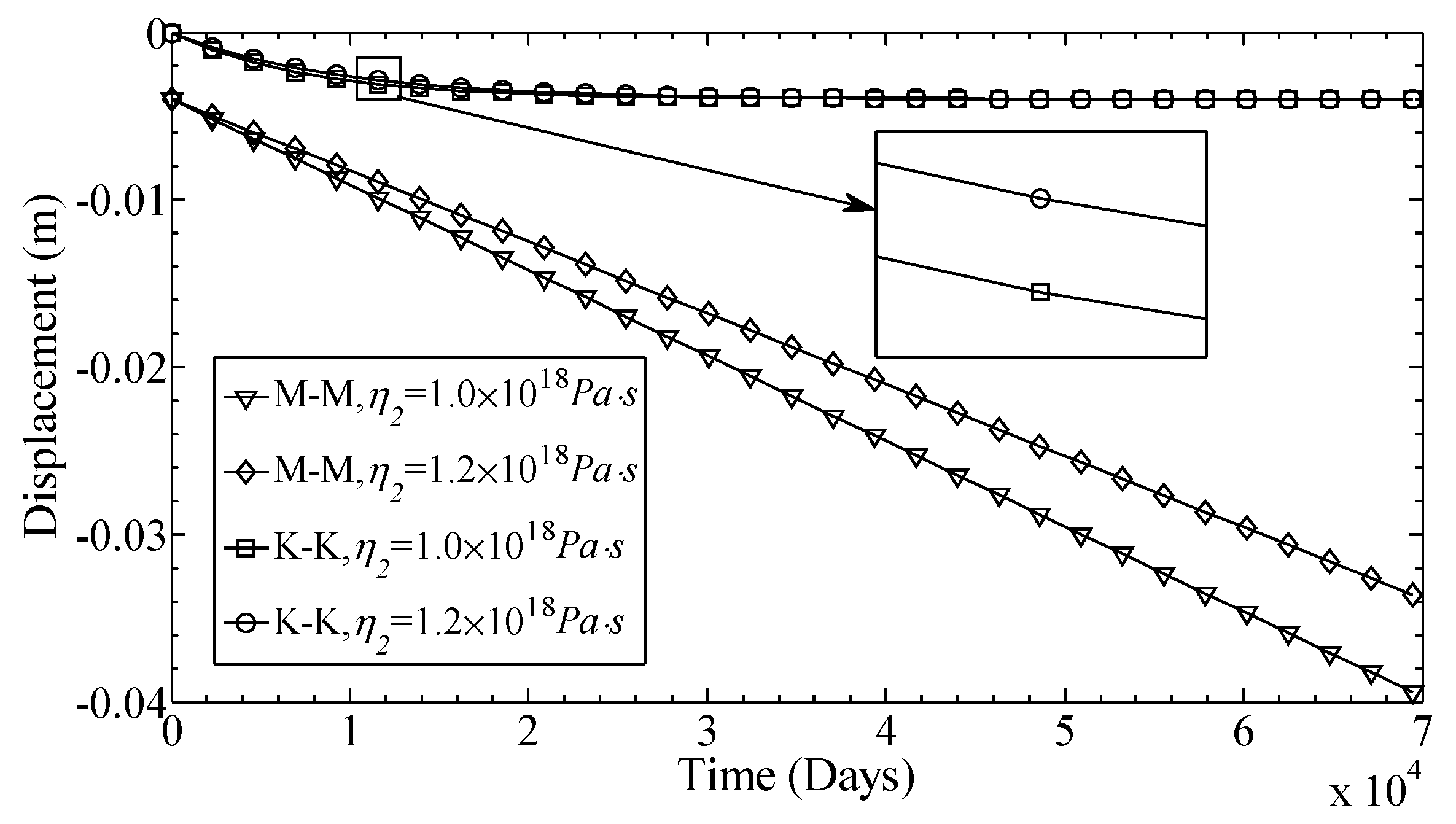
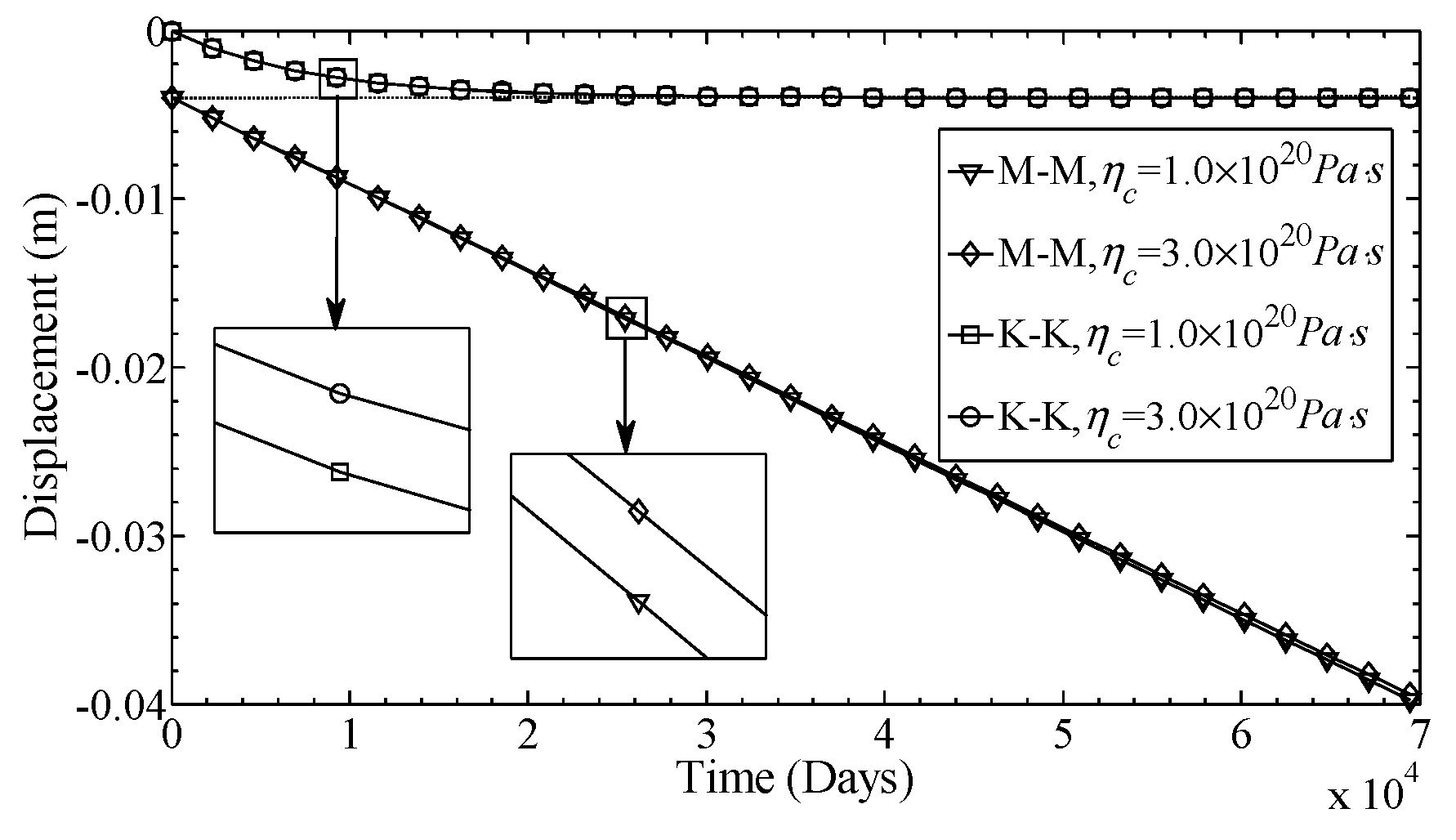
4.2. Different Constitutive Models for Rock Mass and Rockbolts
5. Conclusions
- (1)
- Based on this study, the time-dependent features of rock mass–rockbolt rheology coupling is considered in the long-term design and maintenance of caverns, and the theoretical model can be used to evaluate the rheological mechanical properties of the interaction between the rock mass and DMFC rockbolts, and then the long-term mechanical behavior of the rockbolt supporting system can be predicted;
- (2)
- The evolution of the axial force of a rockbolt is closely related to the viscosity coefficient of rockbolts . When using the M–M model, if is larger than a certain value (), the axial force of rockbolts increases with time, and eventually approaches a limit value. Conversely, the axial force decreases with time, and gradually approaches a minimum value. When using the K–K model, the variation of the axial force is opposite to the result from the M–M model. If the is larger than a certain value, the axial force decreases with time, and eventually approaches the corresponding elastic solution. In contrast, the axial force increases with time, and approaches the value of the corresponding elastic solution. In addition, when the viscosity coefficient of a rockbolt is relatively large, the axial force of the rockbolt may increase at first and then decrease;
- (3)
- The results show a marked difference when the Kelvin model is used as opposed to the Maxwell model; the reason for the difference is that the properties of these two models are different. The Maxwell model can describe the materials’ transient, relaxation and viscous flow, while the Kelvin model can only describe the elastic after-effect and deformation limit;
- (4)
- In this study, analytical solutions of the theoretical model under the circumstances of the K–K model and M–M model are given. This solution derivation methodology may also be applied to other types of rheological models; for example, the reasonable and simplified method for the theoretical model of a CFC rockbolt can be further studied based on this model, which lays a foundation of the preliminary research for solving the theoretical model of a CFC rockbolt. Additional details regarding rockbolt and rock mass coupling properties should be investigated using the appropriate rheological models in future work. Furthermore, the coupling model nonlinear viscoelastic behavior is also the next work.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B
Appendix C
References
- Sharifzadeh, M.; Abolfazl, T.; Mohammad, A.M. Time-dependent behavior of tunnel lining in weak rock mass based on displacement back analysis method. Tunn. Undergr. Space Technol. 2013, 38, 348–356. [Google Scholar] [CrossRef]
- Zhu, W.S.; Zhao, J. Stability Analysis and Modelling of Underground Excavations in Fractured Rocks; Elsevier Science: Amsterdam, The Netherlands, 2004. [Google Scholar]
- Wang, G.; Liu, C.Z.; Jiang, Y.J.; Wu, X.Z.; Wang, S.G. Rheological Model of DMFC Rockbolt and Rockmass in a Circular Tunnel. Rock Mech. Rock Eng. 2015, 48, 2319–2357. [Google Scholar] [CrossRef]
- Sun, J. Rock rheological mechanics and its advance in engineering applications. Chin. J. Rock Mech. Eng. 2007, 26, 1081–1106. [Google Scholar]
- Evert, H.; Ted, B. Underground Excavations in Rock; CRC Press: London, UK, 1980. [Google Scholar]
- Stillborg, B. Professional Users Handbook for Rock Bolting; Trans Tech Publications: Stafa-Zurich Switzerland, 1994. [Google Scholar]
- Windsor, C.R. Rock reinforcement systems. Int. J. Rock Mech. Min. Sci. 1997, 34, 919–951. [Google Scholar] [CrossRef]
- Bobet, A.; Einstein, H.H. Tunnel reinforcement with rockbolts. Tunn. Undergr. Space Technol. 2011, 26, 100–123. [Google Scholar] [CrossRef]
- Li, C.; Stillborg, B. Analytical models for rock bolts. Int. J. Rock Mech. Min. Sci. 1999, 36, 1013–1029. [Google Scholar] [CrossRef]
- Cai, Y.; Tetsuro, E.; Jiang, Y.J. A rock bolt and rock mass interaction model. Int. J. Rock Mech. Min. Sci. 2004, 41, 1055–1067. [Google Scholar] [CrossRef]
- Zhang, Y.J.; Liu, Y.P. 3D viscoelastic-viscoplastic FEM analysis for bolted orthotropic rockmass. Chin. J. Rock Mech. Eng. 2002, 21, 1770–1775. [Google Scholar]
- Zhu, Z.D. Rheologic analysis of action mechanism of underground roadway bolts. J. Shandong Univ. Sci. Technol. 1997, 16, 148–153. [Google Scholar]
- Wu, G.J.; Chen, W.Z.; Wang, Y.G. Study of distribution and variation of anchor stress based on creep effect of rock mass. Rock Soil Mech. 2010, 31, 150–155. [Google Scholar]
- Goodman, R. Introduction to Rock Mechanics, 2nd ed.; Wiley: New York, NY, USA, 1989. [Google Scholar]
- Nomikos, P.; Rahmannejad, R.; Sofianos, A. Supported axisymmetric tunnels within linear viscoelastic Burgers rocks. Rock Mech. Rock Eng. 2011, 44, 553–564. [Google Scholar] [CrossRef]
- Ladanyi, B.; Gill, D. Tunnel lining design in creeping rocks. In Proceedings of the Symposium on Design and Performance of Underground Excavations ISRM, Cambridge, UK, 3–6 September 1984. [Google Scholar]
- Sulem, J.; Panet, M.; Guenot, A. An analytical solution for time-dependent displacements in circular tunnel. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1984, 24, 155–164. [Google Scholar] [CrossRef]
- Ladanyi, B. Time dependent response of rock around tunnel. In Comprehensive Rock Engineering: Principles, Practice & Projects; Hudson, J., Ed.; Pergamon Press: Oxford, UK, 1999; Volume 2, pp. 77–112. [Google Scholar]
- Panet, M. Understanding deformations in tunnels. In Comprehensive Rock Engineering: Principles, Practice & Projects; Hudson, J., Ed.; Pergamon Press: Oxford, UK, 1993; Volume 2, pp. 663–690. [Google Scholar]
- Ghaboussi, J.; Gioda, G. On the time-dependent effects in advancing tunnels. Int. J. Numer. Methods Geomech. 1977, 2, 249–269. [Google Scholar] [CrossRef]
- Gioda, G. A finite element solution of non-linear creep problems in rock. Int. J. Rock Mech. Sci. Geomech. Abstr. 1981, 8, 35–46. [Google Scholar] [CrossRef]
- Peila, D.; Oreste, P.; Rabajuli, G.; Trabucco, E. The pre-tunnel method, a new Italian technology for full-face tunnel excavation: A numerical approach to design. Tunn. Undergr. Space Technol. 1995, 10, 367–374. [Google Scholar] [CrossRef]
- Pan, Y.W.; Dong, J.J. Time-dependent tunnel convergence—I. Formulation of the model. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1991, 28, 469–475. [Google Scholar] [CrossRef]
- Phienwej, N.; Thakur, P.K.; Cording, E.J. Time-dependent response of tunnels considering creep effect. Int. J. Geomech. 2007, 7, 296–306. [Google Scholar] [CrossRef]
- Li, J.J.; Zheng, B.L.; Xu, C.Y. Numerical analyses of creep behavior for prestressed anchor rods. Chin. Q. Mech. 2007, 28, 124–128. [Google Scholar]
- Tang, H.; Wang, D.P.; Huang, R.Q.; Pei, X.J.; Chen, W.L. A new rock creep model based on variable-order fractional derivatives and continuum damage mechanics. Bull. Eng. Geol. Environ. 2018, 77, 375–383. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Li, Y.P. Rock Rheology Theory and Numerical Simulation; Beijing Science Press: Beijing, China, 2008. [Google Scholar]
- Kovári, K. History of the sprayed concrete lining method—Part I: Milestones up to the 1960s. Tunn. Undergr. Space Technol. 1992, 18, 57–69. [Google Scholar] [CrossRef]
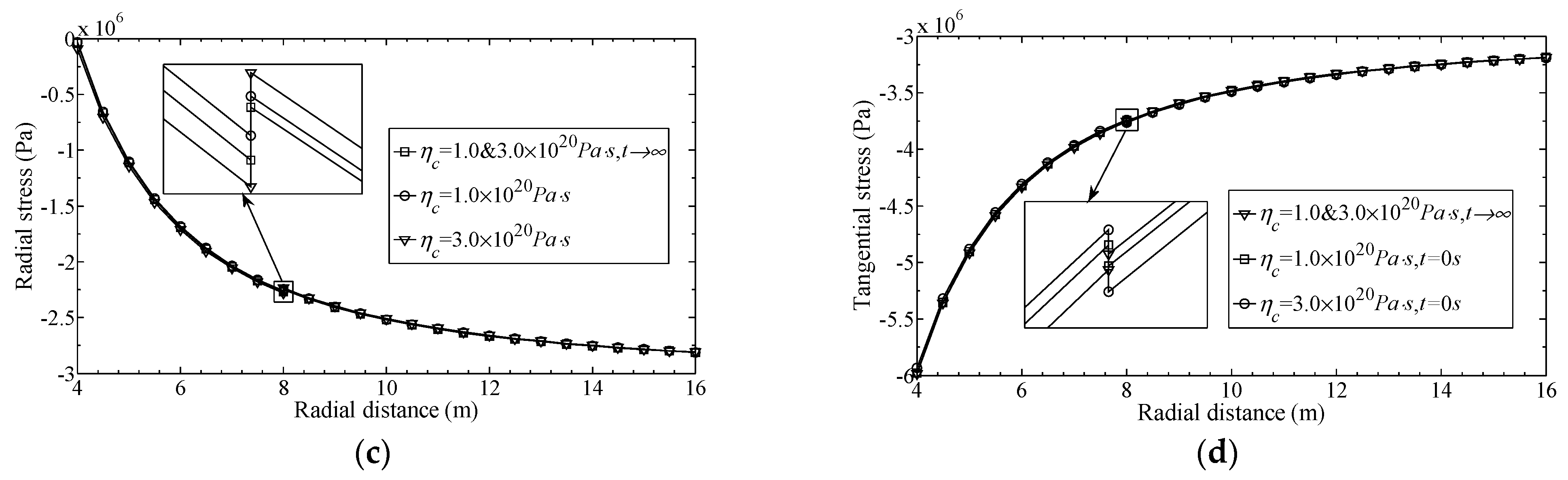


| Experiment No. | ηC (Pa·s) | EC (Pa) | η2 (Pa·s) | K (Pa) | G0 (Pa) | σ0 (Pa) | r (m) | R (m) | AC (m2) | L (m) | Sθ (m) | SZ (m) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A | 1.0 × 1020 | 2.0 × 1011 | 1.0 × 1018 | 2.2 × 109 | 1.5 × 109 | −3.0 × 106 | 4 | 8 | 3.0 × 10-4 | 4 | 0.6981 | 1 |
| B | 3.0 × 1020 | 2.0 × 1011 | 1.0 × 1018 | 2.2 × 109 | 1.5 × 109 | −3.0 × 106 | 4 | 8 | 3.0 × 10-4 | 4 | 0.6981 | 1 |
| C | 3.0 × 1020 | 2.0 × 1011 | 1.0 × 1018 | 2.2 × 109 | 1.5 × 109 | −3.0 × 106 | 4 | 8 | 3.0 × 10-4 | 4 | 1.0472 | 1 |
| D | 3.0 × 1020 | 2.0 × 1011 | 1.0 × 1018 | 2.2 × 109 | 1.5 × 109 | −3.0 × 106 | 4 | 8 | 3.0 × 10-4 | 4 | 1.2566 | 1 |
| E | 3.0 × 1020 | 2.0 × 1011 | 1.0 × 1018 | 2.2 × 109 | 1.5 × 109 | −3.0 × 106 | 4 | 8 | 3.0 × 10-4 | 4 | 0.5236 | 1 |
| F | 3.0 × 1020 | 2.0 × 1011 | 0.9 × 1018 | 2.2 × 109 | 1.5 × 109 | −3.0 × 106 | 4 | 8 | 3.0 × 10-4 | 4 | 0.6981 | 1 |
| G | 3.0 × 1020 | 2.0 × 1011 | 1.1 × 1018 | 2.2 × 109 | 1.5 × 109 | −3.0 × 106 | 4 | 8 | 3.0 × 10-4 | 4 | 0.6981 | 1 |
| H | 3.0 × 1020 | 2.0 × 1011 | 1.2 × 1018 | 2.2 × 109 | 1.5 × 109 | −3.0 × 106 | 4 | 8 | 3.0 × 10-4 | 4 | 0.6981 | 1 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, G.; Han, W.; Liu, C.; Wang, K.; Luan, H. Rheological Behavior of Rockmass Supported with Rockbolts Based on Viscoelastic Analysis Method. Mathematics 2018, 6, 199. https://doi.org/10.3390/math6100199
Wang G, Han W, Liu C, Wang K, Luan H. Rheological Behavior of Rockmass Supported with Rockbolts Based on Viscoelastic Analysis Method. Mathematics. 2018; 6(10):199. https://doi.org/10.3390/math6100199
Chicago/Turabian StyleWang, Gang, Wei Han, Chuanzheng Liu, Ke Wang, and Hengjie Luan. 2018. "Rheological Behavior of Rockmass Supported with Rockbolts Based on Viscoelastic Analysis Method" Mathematics 6, no. 10: 199. https://doi.org/10.3390/math6100199
APA StyleWang, G., Han, W., Liu, C., Wang, K., & Luan, H. (2018). Rheological Behavior of Rockmass Supported with Rockbolts Based on Viscoelastic Analysis Method. Mathematics, 6(10), 199. https://doi.org/10.3390/math6100199






