Preparational Uncertainty Relations for N Continuous Variables
Abstract
:1. Introduction
2. Lower Bounds of Uncertainty Functionals
2.1. Extrema of Uncertainty Functionals
2.2. Consistency Conditions
3. Inequalities for Two or More Continuous Variables
3.1. Inequalities without Correlation Terms
3.2. Inequalities with Correlation Terms
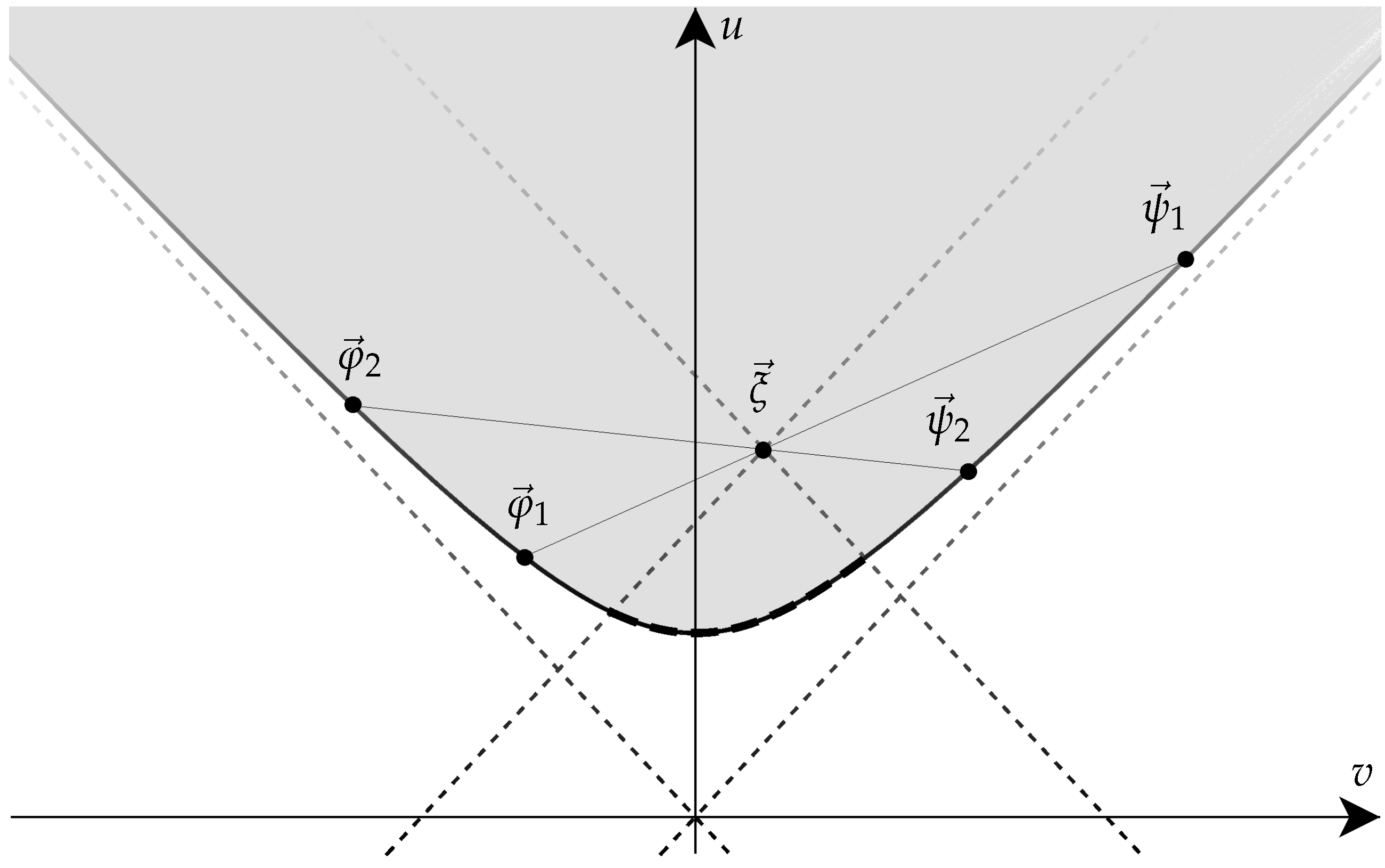
4. The Uncertainty Region
4.1. More Than One Continuous Variable:
4.1.1. The Uncertainty Region Has a Convex Boundary
4.1.2. The Uncertainty Region Has No Pure-State Holes
4.1.3. All Moments Arise as Convex Combinations of Two Pure States
4.2. One Continuous Variable:
4.2.1. The Uncertainty Region Has a Convex Boundary
4.2.2. The Uncertainty Region Has No Pure-State Holes
4.2.3. All Moments Arise as Convex Combinations of Two Pure States
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Heisenberg, W. Über den anschaulichen Inhalt der quantentheoretischen Kinematik und Mechanik. Z. Phys. 1927, 43, 172–198. [Google Scholar]
- Kennard, E.H. Zur Quantenmechanik einfacher Bewegungstypen. Z. Phys. 1927, 44, 326–352. [Google Scholar] [CrossRef]
- Weyl, H. The Theory of Groups and Quantum Mechanics; Robertson, H.P., Ed.; Dover: New York, NY, USA, 1931. [Google Scholar]
- Robertson, H.P. The Uncertainty Principle. Phys. Rev. 1929, 34, 163–164. [Google Scholar] [CrossRef]
- Schrödinger, E. Zum Heisenbergschen Unschäfeprinzip. Sitzungsber. Preuss. Akad. Wiss. Phys.-Math. Klasse 1930, 19, 296–323. [Google Scholar]
- Arthurs, E.; Kelly, J.L. On the Simultaneous Measurement of a Pair of Conjugate Observables. Bell Syst. Tech. J. 1965, 44, 725–729. [Google Scholar] [CrossRef]
- Busch, P.; Lahti, P.; Werner, R.F. Proof of Heisenberg’s error-disturbance relation. Phys. Rev. Lett. 2013, 111, 160405. [Google Scholar] [CrossRef] [PubMed]
- Ozawa, M. Universally valid reformulation of the Heisenberg uncertainty principle on noise and disturbance in measurement. Phys. Rev. A 2003, 67, 042105. [Google Scholar] [CrossRef]
- Bennett, C.H.; Brassard, G. Qyantum cryptography: Public key distribution and coin tossing. In Proceedings of IEEE International Conference on Computers, Systems and Signal Processing, Bangalore, India, 10–12 December 1984; pp. 175–179.
- Duan, L.-M.; Giedke, G.; Cirac, J.I.; Zoller, P. Inseparability Criterion for Continuous Variable Systems. Phys. Rev. Lett. 2000, 84, 2722–2725. [Google Scholar] [CrossRef] [PubMed]
- Simon, R. Peres-Horodecki Separability Criterion for Continuous Variable Systems. Phys. Rev. Lett. 2000, 84, 2726–2729. [Google Scholar] [CrossRef] [PubMed]
- Jackiw, R. Minimum Uncertainty Product, Number-Phase Uncertainty Product, and Coherent States. J. Math. Phys. 1968, 9, 339–346. [Google Scholar] [CrossRef]
- Kechrimparis, S.; Weigert, S. Universality in Uncertainty Relations for a Quantum Particle. J. Phys. A 2016. in print. [Google Scholar]
- Tasca, D.S.; Walborn, S.P.; Ribeiro, P.H.S.; Toscano, F. Detection of transverse entanglement in phase space. Phys. Rev. A 2008, 78, 010304R. [Google Scholar] [CrossRef]
- Toscano, F.; Saboia, A.; Avelar, A.T.; Walborn, S.P. Systematic construction of genuine-multipartite- entanglement criteria in continuous-variable systems using uncertainty relations. Phys. Rev. A 2015, 92, 052316. [Google Scholar] [CrossRef]
- Paul, E.C.; Tasca, D.S.; Rudnicki, Ł.; Walborn, S.P. Detecting entanglement of continuous variables with three mutually unbiased bases. Phys. Rev. A 2016, 94, 012303. [Google Scholar] [CrossRef]
- Busch, P.; Schönbeck, T.P.; Schroeck, F., Jr. Quantum observables: Compatibility versus commutativity and maximal information. J. Math. Phys. 1987, 28, 2866–2872. [Google Scholar] [CrossRef]
- Bialynicki-Birula, I.; Bialynicka-Birula, Z. Uncertainty Relation for Photons. Phys. Rev. Lett. 2012, 108, 140401. [Google Scholar] [CrossRef] [PubMed]
- Rudnicki, Ł. Heisenberg uncertainty relation for position and momentum beyond central potentials. Phys. Rev. A 2012, 85, 022112. [Google Scholar] [CrossRef]
- Weigert, S. Landscape of uncertainty in Hilbert space for one-particle states. Phys. Rev. A 1996, 53, 2084–2088. [Google Scholar] [CrossRef] [PubMed]
- Williamson, J. On the Algebraic Problem Concerning the Normal Forms of Linear Dynamical Systems. Am. J. Math. 1936, 58, 141–163. [Google Scholar] [CrossRef]
- Simon, R.; Mukunda, N.; Dutta, B. Quantum-noise matrix for multimode systems: U(n) invariance, squeezing, and normal forms. Phys. Rev. A 1994, 49, 1567–1583. [Google Scholar] [CrossRef] [PubMed]
- Arvind; Dutta, B.; Mukunda, N.; Simon, R. The real symplectic groups in quantum mechanics and optics. Pramana 1995, 45, 471–497. [Google Scholar] [CrossRef]
- Adesso, G.; Ragy, S.; Lee, A.R. Continuous Variable Quantum Information: Gaussian States and Beyond. Open Syst. Inf. Dyn. 2014, 21, 1440001. [Google Scholar] [CrossRef]
- Robertson, H.P. An Indeterminacy Relation for Several Observables and Its Classical Interpretation. Phys. Rev. 1934, 46, 794–801. [Google Scholar] [CrossRef]
- Weedbrook, C.; Pirandola, S.; Garcia-Patron, R.; Cerf, N.J.; Ralph, T.C.; Shapiro, J.H.; Lloyd, S. Gaussian quantum information. Rev. Mod. Phys. 2012, 84, 621–669. [Google Scholar] [CrossRef]
- Boyd, S.; Vanderberghe, L. Convex Optimization; Cambridge University Press: Cambridge, UK, 2004; p. 74. [Google Scholar]
- Dammeier, L.; Schwonnek, R.; Werner, R.F. Uncertainty relations for angular momentum. New J. Phys. 2015, 17, 093046. [Google Scholar] [CrossRef]
- Dodonov, V.V.; Kurmyshev, E.Y.; Man’ko, V.I. Generalized uncertainty relation and correlated coherent states. Phys. Lett. A 1980, 79, 150–152. [Google Scholar] [CrossRef]
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kechrimparis, S.; Weigert, S. Preparational Uncertainty Relations for N Continuous Variables. Mathematics 2016, 4, 49. https://doi.org/10.3390/math4030049
Kechrimparis S, Weigert S. Preparational Uncertainty Relations for N Continuous Variables. Mathematics. 2016; 4(3):49. https://doi.org/10.3390/math4030049
Chicago/Turabian StyleKechrimparis, Spiros, and Stefan Weigert. 2016. "Preparational Uncertainty Relations for N Continuous Variables" Mathematics 4, no. 3: 49. https://doi.org/10.3390/math4030049
APA StyleKechrimparis, S., & Weigert, S. (2016). Preparational Uncertainty Relations for N Continuous Variables. Mathematics, 4(3), 49. https://doi.org/10.3390/math4030049






