Maximum Principle-Preserving Computational Algorithm for the 3D High-Order Allen–Cahn Equation
Abstract
1. Introduction
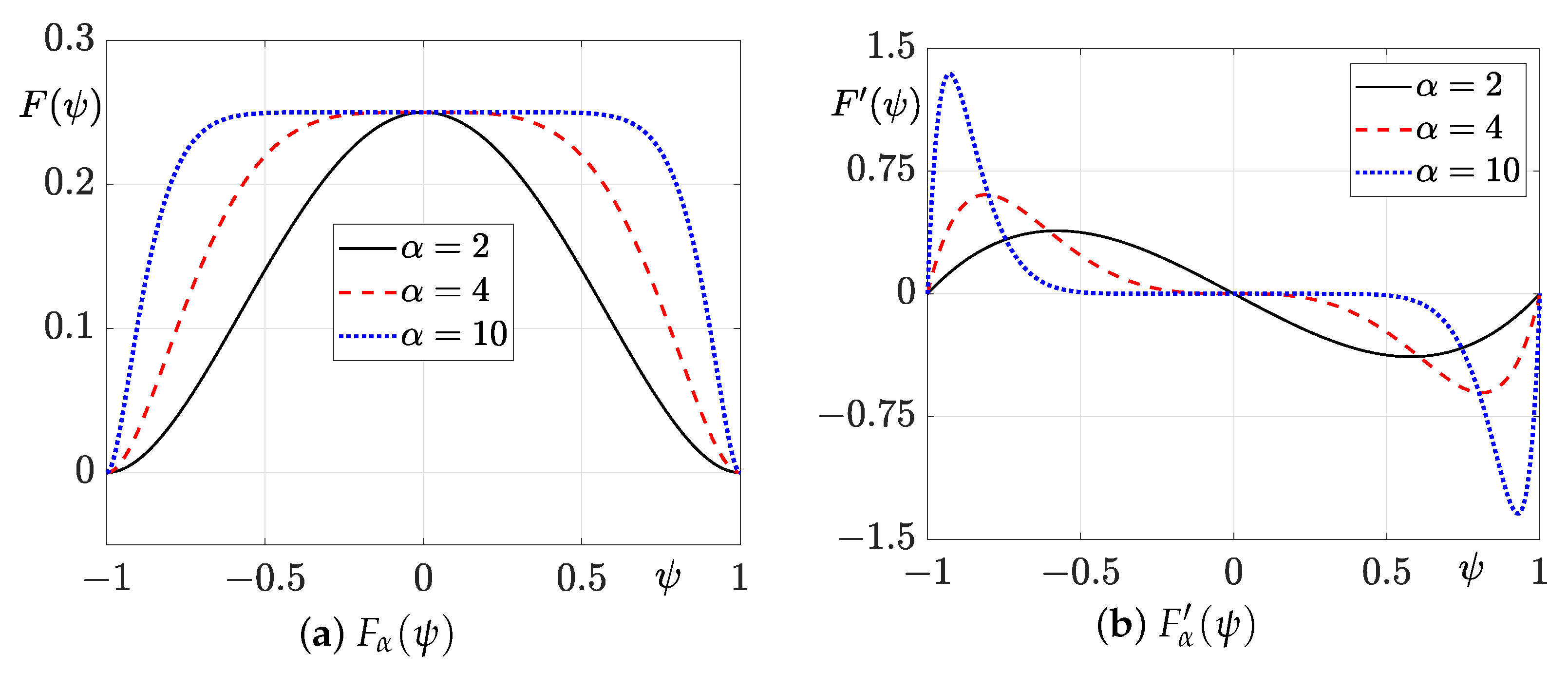
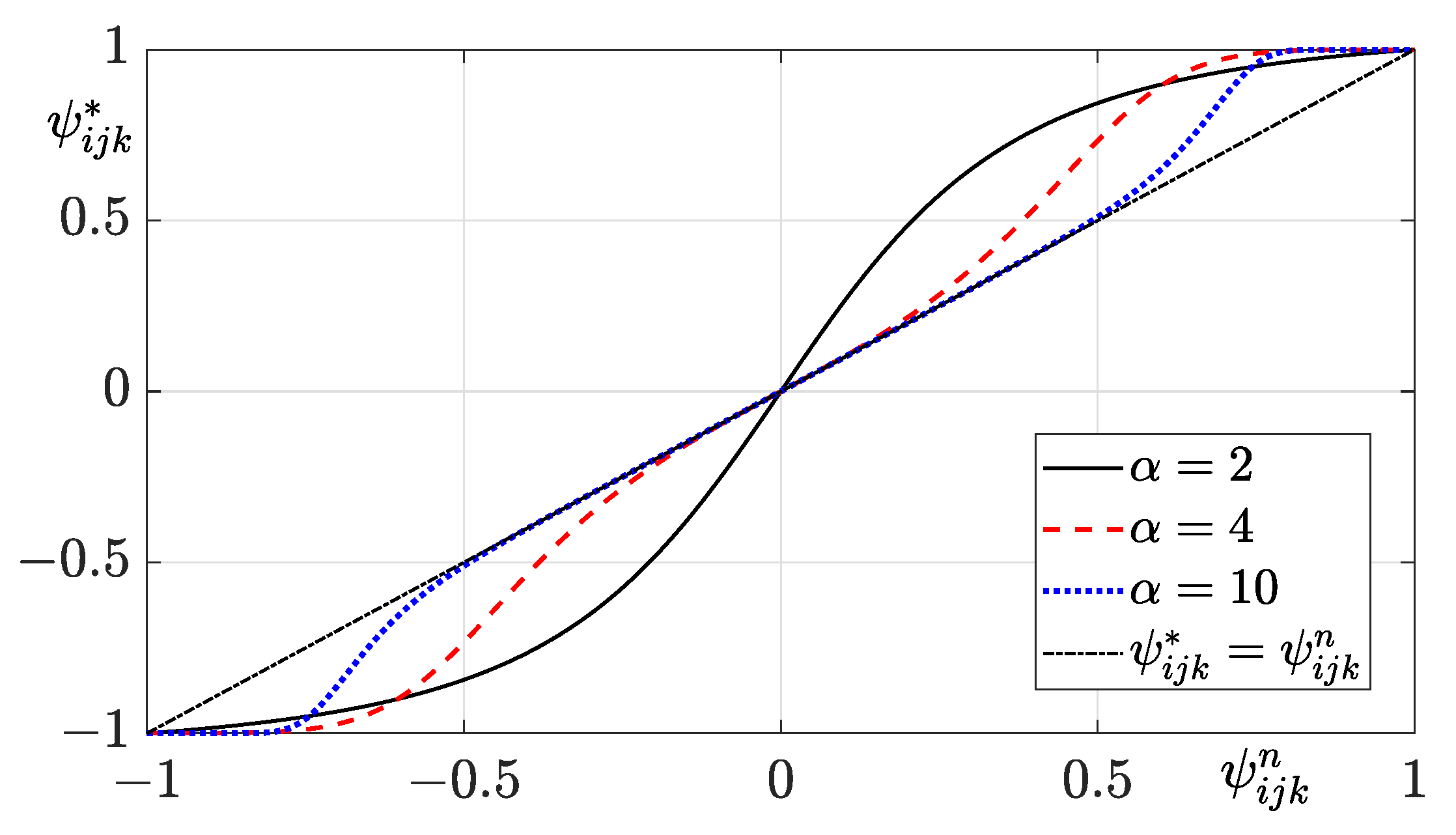
2. Computational Method
3. Numerical Experiments
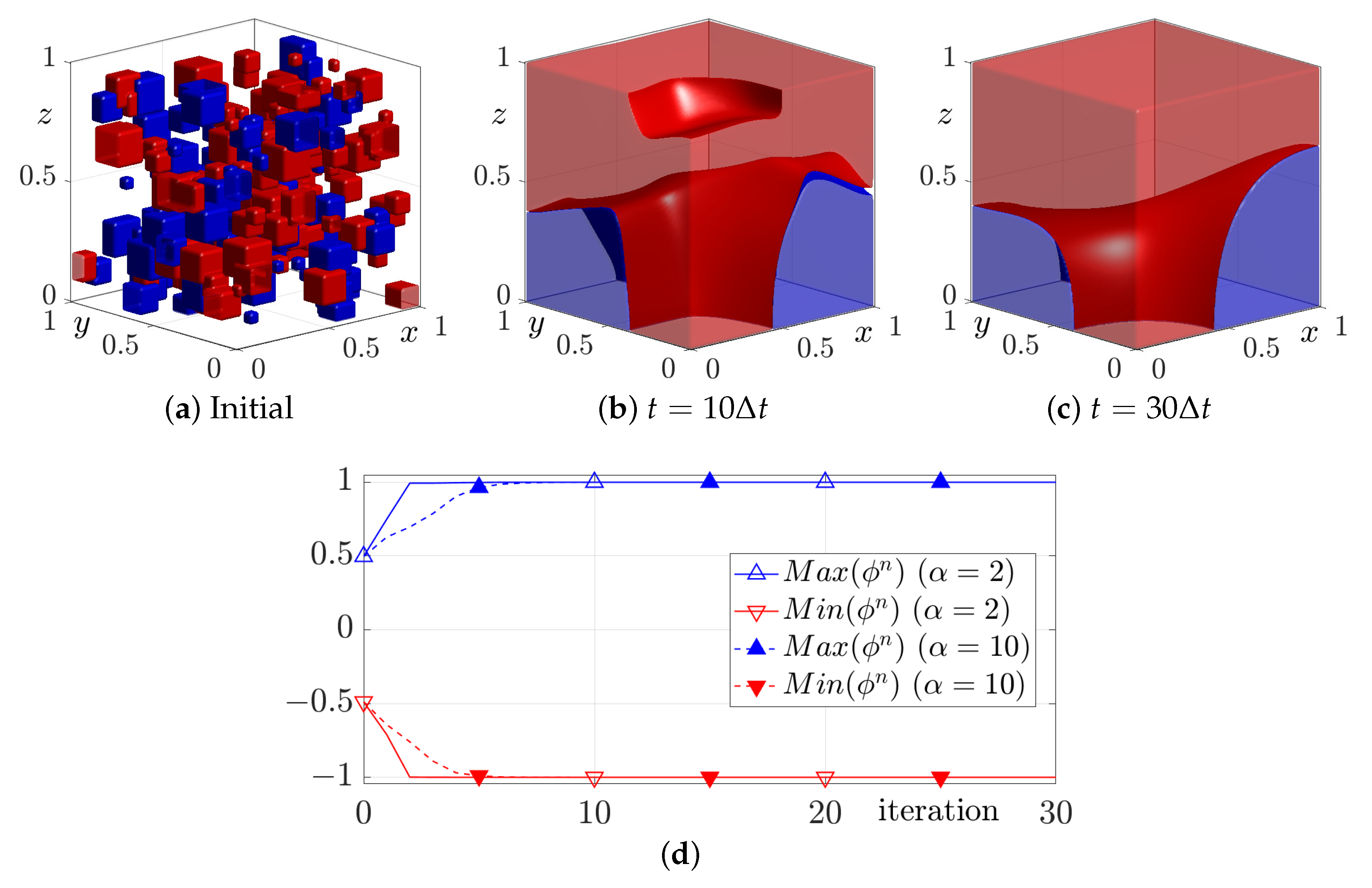
3.1. Stability Test
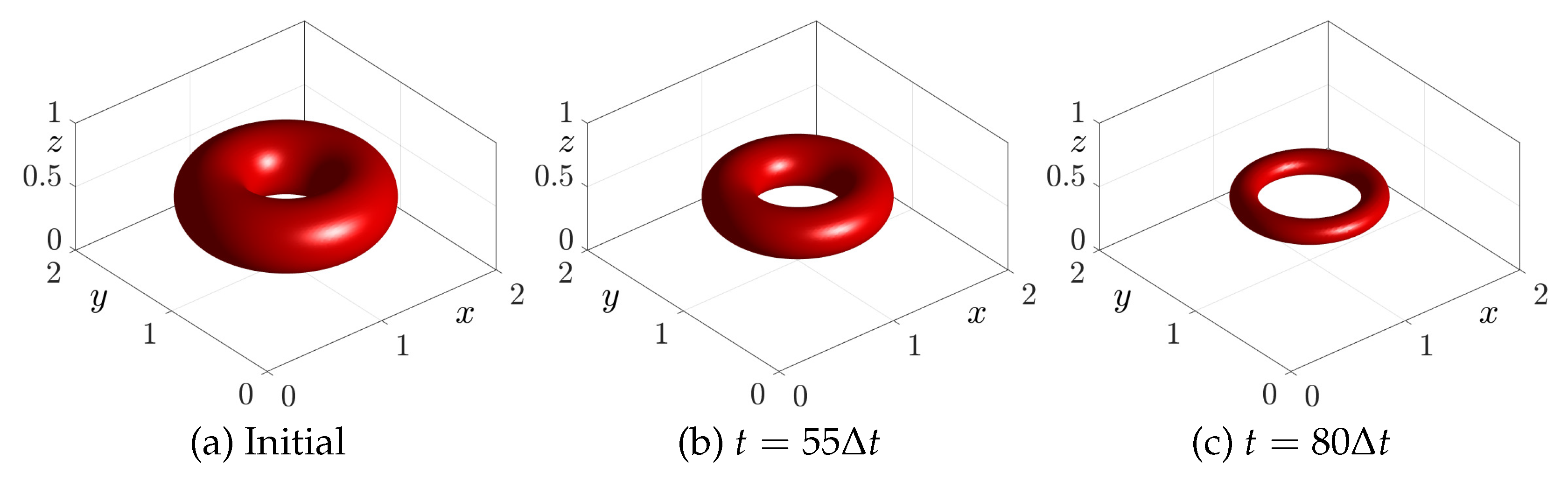
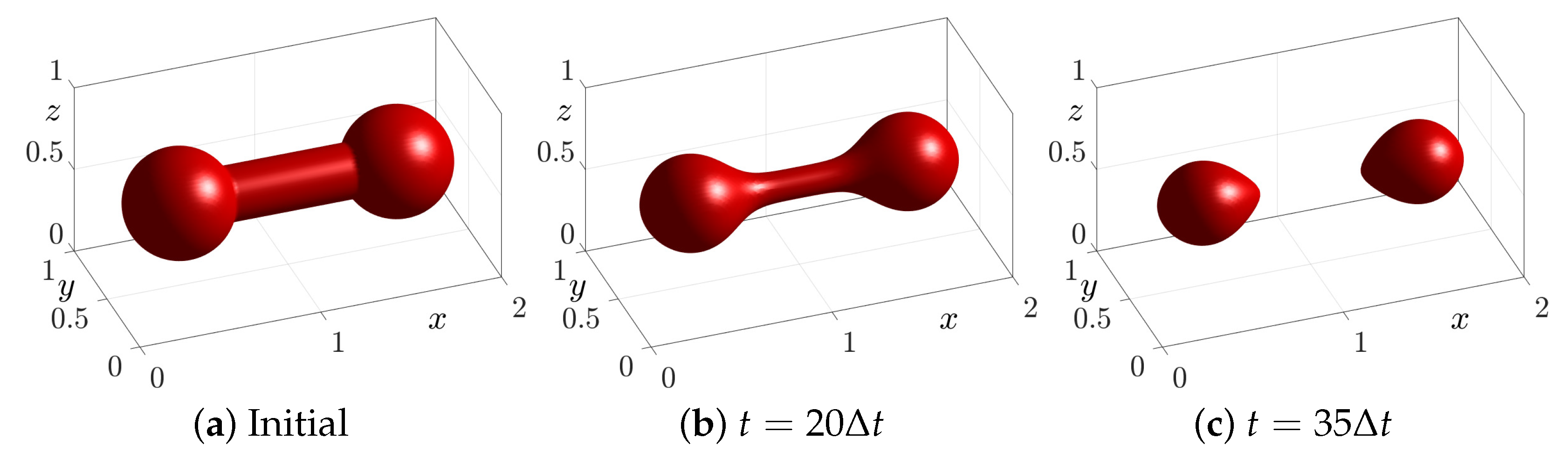
3.2. Motion by Mean Curvature
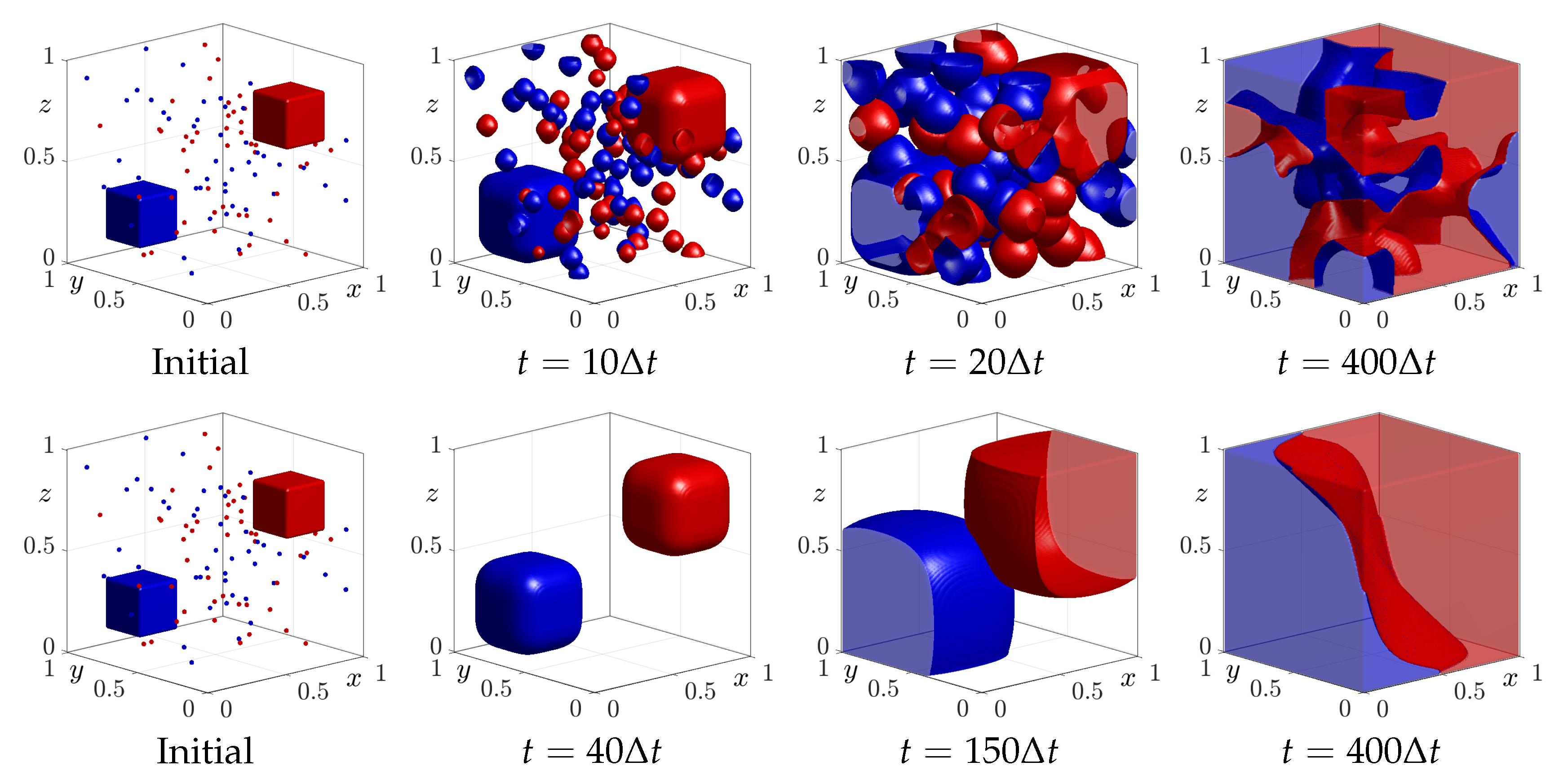
3.3. Binary Data Classification with Noisy Data
3.4. Comparison with the Fully Explicit Method
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Allen, S.M.; Cahn, J.W. A microscopic theory for antiphase boundary motion and its application to antiphase domain coarsening. Acta Metall. 1979, 27, 1085–1095. [Google Scholar] [CrossRef]
- Kim, J. Maximum principle preserving the unconditionally stable method for the Allen–Cahn equation with a high-order potential. Electron. Res. Arch. 2025, 33, 433–446. [Google Scholar] [CrossRef]
- Ham, S.; Li, Y.; Jeong, D.; Lee, C.; Kwak, S.; Hwang, Y.; Kim, J. An explicit adaptive finite difference method for the Cahn–Hilliard equation. J. Nonlinear Sci. 2022, 32, 80. [Google Scholar] [CrossRef]
- Emamjomeh, M.; Nabati, M.; Dinmohammadi, A. Numerical study of two operator splitting localized radial basis function method for Allen–Cahn problem. Eng. Anal. Bound. Elem. 2024, 163, 126–137. [Google Scholar] [CrossRef]
- Liu, S.; Peng, Y. A local region-based Chan–Vese model for image segmentation. Pattern Recognit. 2012, 45, 2769–2779. [Google Scholar] [CrossRef]
- Geng, Y.; Teng, Y.; Wang, Z.; Ju, L. A deep learning method for the dynamics of classic and conservative Allen–Cahn equations based on fully-discrete operators. J. Comput. Phys. 2024, 496, 112589. [Google Scholar] [CrossRef]
- Rihan, F.A.; Al-Mdallal, Q.M.; AlSakaji, H.J.; Hashish, A. A fractional-order epidemic model with time-delay and nonlinear incidence rate. Chaos Solitons Fractals 2019, 126, 97–105. [Google Scholar] [CrossRef]
- Hashemi, M.S.; Bayram, M.; Riaz, M.B.; Baleanu, D. Bifurcation analysis and exact solutions of the two-mode Cahn–Allen equation by a novel variable coefficient auxiliary equation method. Results Phys. 2024, 64, 107882. [Google Scholar] [CrossRef]
- Lv, Z.; Song, X.; Feng, J.; Xia, Q.; Xia, B.; Li, Y. Reduced-order prediction model for the Cahn–Hilliard equation based on deep learning. Eng. Anal. Bound. Elem. 2025, 172, 106118. [Google Scholar] [CrossRef]
- Li, R.; Gao, Y.; Chen, Z. Adaptive discontinuous Galerkin finite element methods for the Allen–Cahn equation on polygonal meshes. Numer. Algorithms 2024, 95, 1981–2014. [Google Scholar] [CrossRef]
- Beneš, M.; Chalupecký, V.; Mikula, K. Geometrical image segmentation by the Allen–Cahn equation. Appl. Numer. Math. 2004, 51, 187–205. [Google Scholar] [CrossRef]
- Xie, W.; Xia, Q.; Yu, Q.; Li, Y. An effective phase field method for topology optimization without the curvature effects. Comput. Math. Appl. 2023, 146, 200–212. [Google Scholar] [CrossRef]
- Sohaib, M.; Furati, K.M.; Shah, A. Space fractional Allen–Cahn equation and its applications in phase separation: A numerical study. Commun. Nonlinear Sci. Numer. Simul. 2024, 137, 108173. [Google Scholar] [CrossRef]
- Hu, X.; Xia, Q.; Xia, B.; Li, Y. A second-order accurate numerical method with unconditional energy stability for the Lifshitz–Petrich equation on curved surfaces. Appl. Math. Lett. 2025, 163, 109439. [Google Scholar] [CrossRef]
- Mohammadi, V.; Dehghan, M.; Mesgarani, H. The localized RBF interpolation with its modifications for solving the incompressible two-phase fluid flows: A conservative Allen–Cahn–Navier–Stokes system. Eng. Anal. Bound. Elem. 2024, 168, 105908. [Google Scholar] [CrossRef]
- Kim, H.; Lee, C.; Yoon, S.; Choi, Y.; Kim, J. A fast shape transformation using a phase-field model. Extrem. Mech. Lett. 2022, 52, 101633. [Google Scholar] [CrossRef]
- Choi, J.; Ham, S.; Kwak, S.; Hwang, Y.; Kim, J. Stability analysis of an explicit numerical scheme for the Allen–Cahn equation with high-order polynomial potentials. AIMS Math. 2024, 9, 19332–19344. [Google Scholar] [CrossRef]
- Guan, Z.; Lowengrub, J.S.; Wang, C.; Wise, S.M. Second order convex splitting schemes for periodic nonlocal Cahn–Hilliard and Allen–Cahn equations. J. Comput. Phys. 2014, 277, 48–71. [Google Scholar] [CrossRef]
- Guaraco, M.A. Min–max for phase transitions and the existence of embedded minimal hypersurfaces. J. Differ. Geom. 2018, 108, 91–133. [Google Scholar] [CrossRef]
- Song, X.; Xia, B.; Li, Y. An efficient data assimilation based unconditionally stable scheme for Cahn–Hilliard equation. Comput. Appl. Math. 2024, 43, 121. [Google Scholar] [CrossRef]
- Ju, L.; Li, X.; Qiao, Z. Stabilized exponential-SAV schemes preserving energy dissipation law and maximum bound principle for the Allen–Cahn type equations. J. Sci. Comput. 2022, 92, 66. [Google Scholar] [CrossRef]
- Peng, G.; Li, Y. An energy stable bound-preserving finite volume scheme for the Allen–Cahn equation based on operator splitting method. Comput. Math. Appl. 2025, 178, 47–60. [Google Scholar] [CrossRef]
- Zhang, H.; Qian, X.; Song, S. Third-order accurate, large time-stepping and maximum-principle-preserving schemes for the Allen–Cahn equation. Numer. Algorithms 2024, 95, 1213–1250. [Google Scholar] [CrossRef]
- Lee, D. Gradient-descent-like scheme for the Allen–Cahn equation. AIP Adv. 2023, 13, 8. [Google Scholar] [CrossRef]
- Li, Y.; Liu, R.; Xia, Q.; He, C.; Li, Z. First- and second-order unconditionally stable direct discretization methods for multi-component Cahn–Hilliard system on surfaces. J. Comput. Appl. Math. 2022, 401, 113778. [Google Scholar] [CrossRef]
- Yang, J.; Tan, Z.; Kim, J. Original variables based energy-stable time-dependent auxiliary variable method for the incompressible Navier–Stokes equation. Comput. Fluids 2022, 240, 105432. [Google Scholar] [CrossRef]
- Li, D.; Quan, C.; Xu, J. Stability and convergence of Strang splitting. Part I: Scalar Allen–Cahn equation. J. Comput. Phys. 2022, 458, 111087. [Google Scholar] [CrossRef]
- Li, D.; Quan, C.; Xu, J. Stability and convergence of Strang splitting. Part II: Tensorial Allen–Cahn equations. J. Comput. Phys. 2022, 454, 110985. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, J.; Hwang, Y. Maximum Principle-Preserving Computational Algorithm for the 3D High-Order Allen–Cahn Equation. Mathematics 2025, 13, 1085. https://doi.org/10.3390/math13071085
Kim J, Hwang Y. Maximum Principle-Preserving Computational Algorithm for the 3D High-Order Allen–Cahn Equation. Mathematics. 2025; 13(7):1085. https://doi.org/10.3390/math13071085
Chicago/Turabian StyleKim, Junseok, and Youngjin Hwang. 2025. "Maximum Principle-Preserving Computational Algorithm for the 3D High-Order Allen–Cahn Equation" Mathematics 13, no. 7: 1085. https://doi.org/10.3390/math13071085
APA StyleKim, J., & Hwang, Y. (2025). Maximum Principle-Preserving Computational Algorithm for the 3D High-Order Allen–Cahn Equation. Mathematics, 13(7), 1085. https://doi.org/10.3390/math13071085







