Variational Iteration and Linearized Liapunov Methods for Seeking the Analytic Solutions of Nonlinear Boundary Value Problems
Abstract
:1. Introduction
2. The Existence of Solutions
2.1. Existence Theorem
2.2. Application to an Example
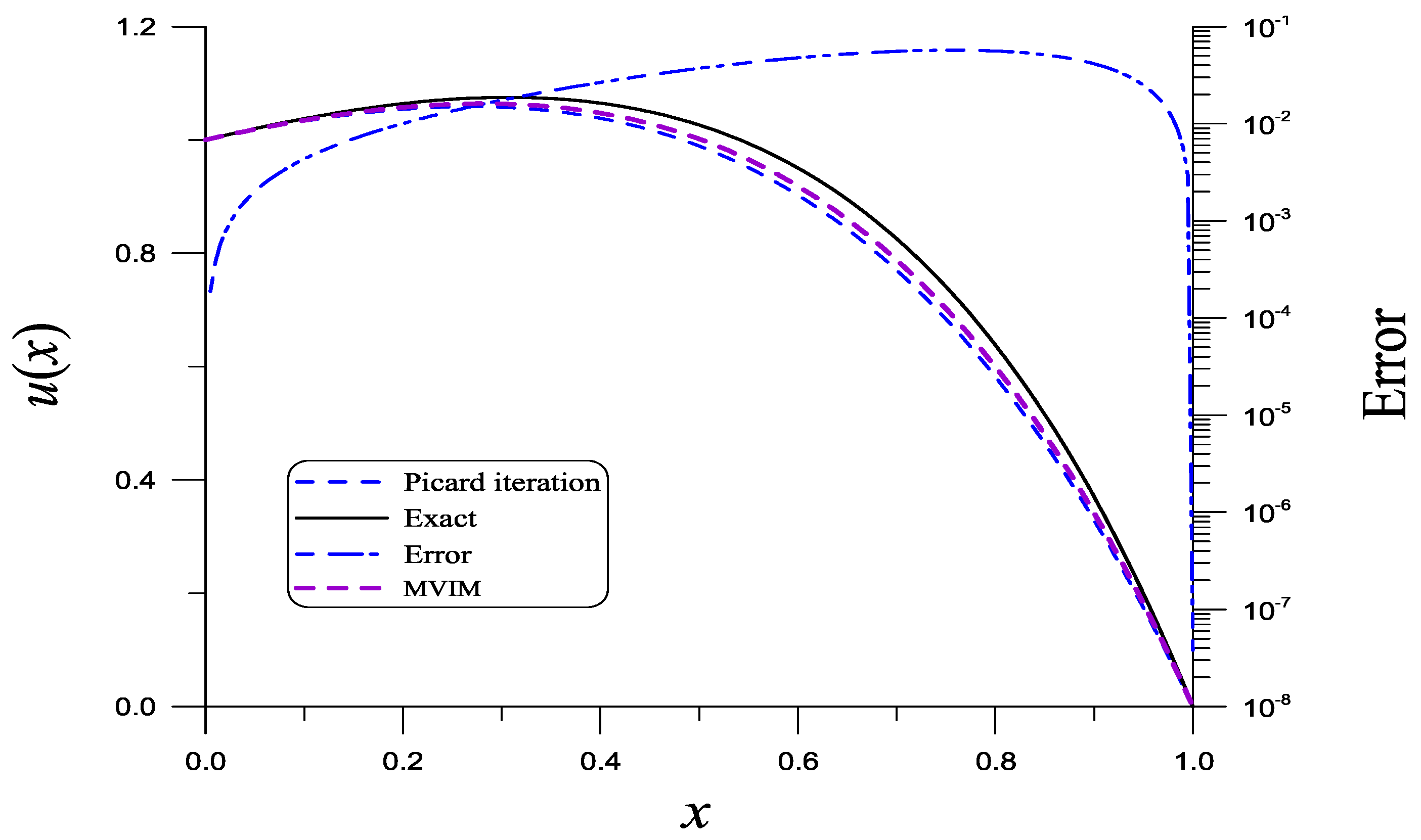
3. Slow Convergence of the Picard Iteration Method
4. Boundary Shape Function Method
5. A Modified Variational Iteration Method
5.1. Variational Iteration Method
5.2. A Modified VIM
5.3. Determination of the Right-Hand Values
5.4. Nonlocal Boundary Conditions
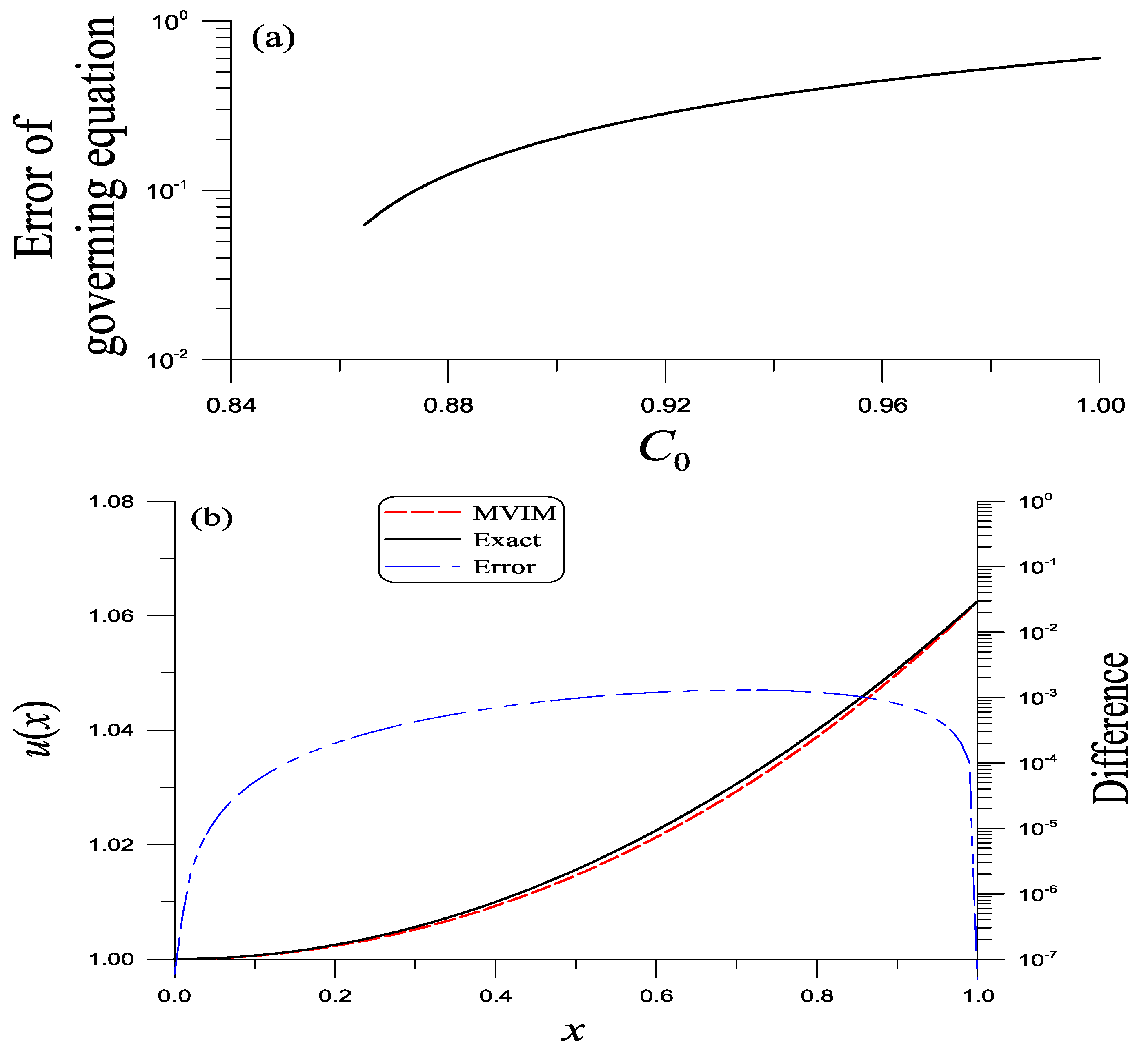
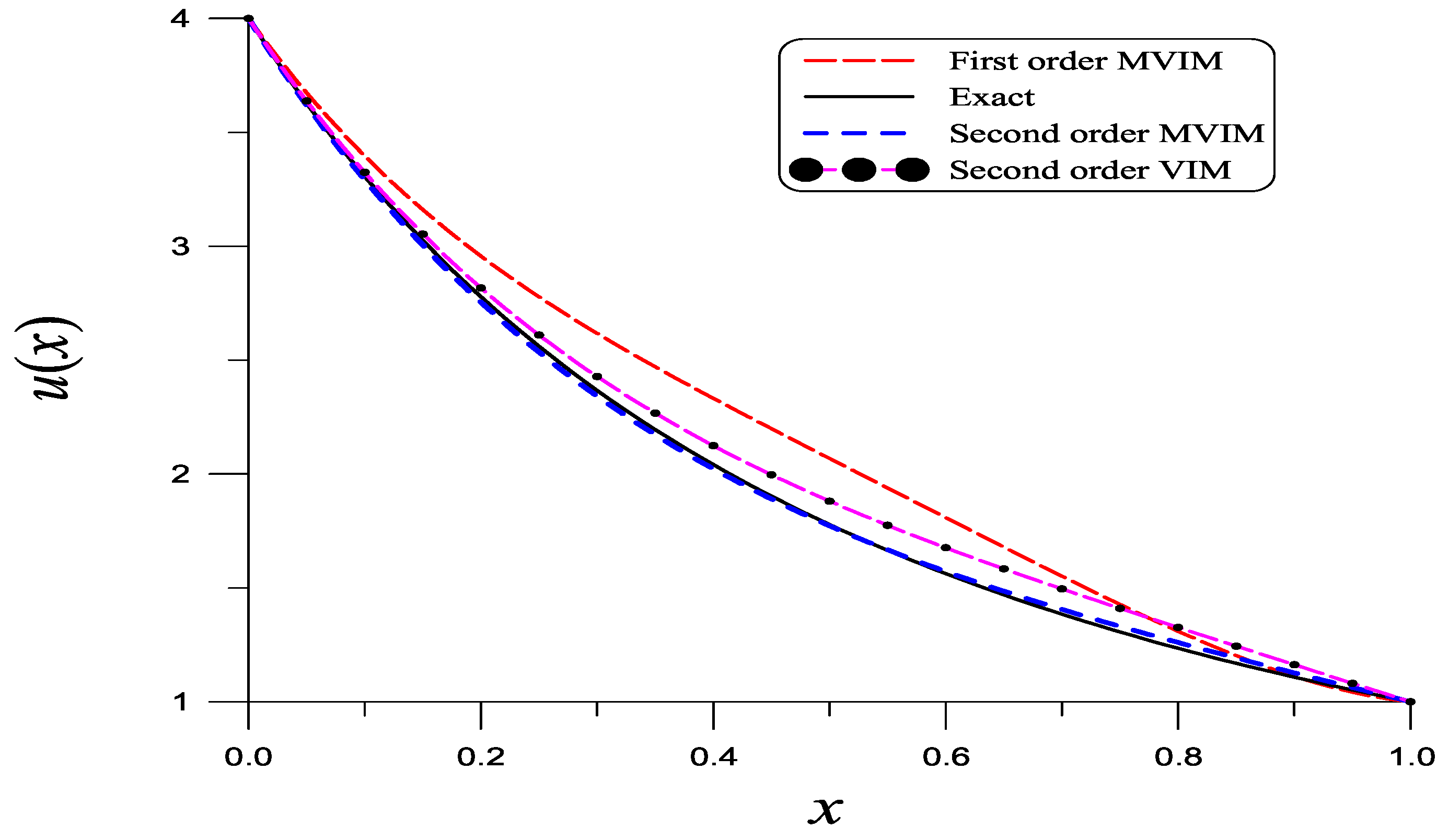
6. Example Testing for the MVIM
A Nonlocal BVP
7. Linearized Liapunov Method for Seeking Analytic Solutions
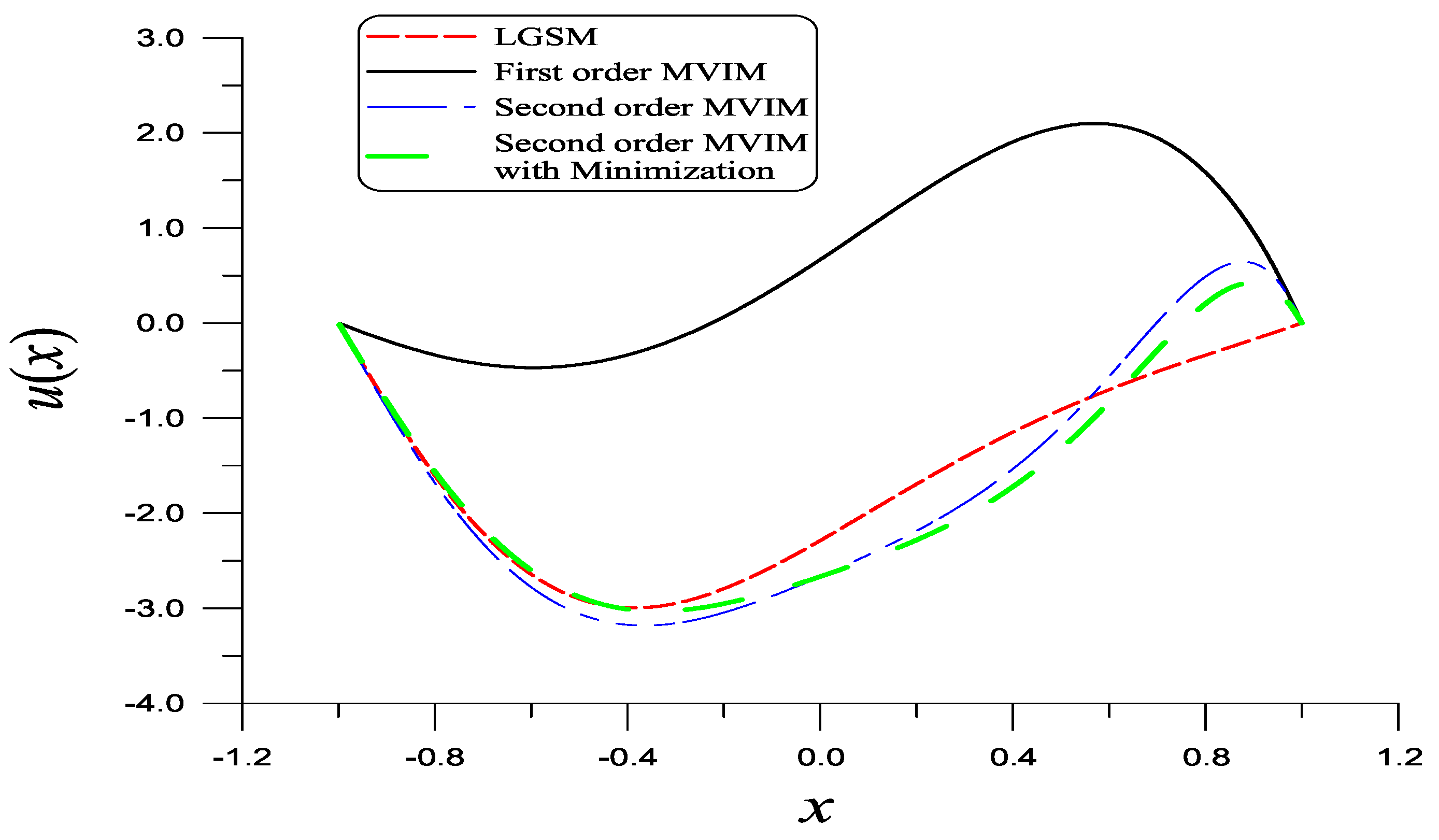
8. Examples Testing for the Linearized Liapunov Method
9. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| x | Exact | Equation (A6) | Equation (A5) | Equation (A7) |
|---|---|---|---|---|
| 0 | 1.00000 | 1.00000 | 1.00000 | 1.00000 |
| 0.1 | 1.03753 | 1.05255 | 1.03468 | 1.03025 |
| 0.2 | 1.06402 | 1.08885 | 1.05740 | 1.04716 |
| 0.3 | 1.07501 | 1.10347 | 1.06351 | 1.04601 |
| 0.4 | 1.06482 | 1.09023 | 1.04724 | 1.02132 |
| 0.5 | 1.02626 | 1.04222 | 1.00164 | 0.966968 |
| 0.6 | 0.950319 | 0.951926 | 0.918457 | 0.876260 |
| 0.7 | 0.825678 | 0.811284 | 0.788167 | 0.741986 |
| 0.8 | 0.638204 | 0.611764 | 0.599927 | 0.556525 |
| 0.9 | 0.370281 | 0.344429 | 0.341633 | 0.311926 |
| 1.0 | 0 | 0 | 0 |
Appendix B
References
- Cash, J.R. On the numerical integration of nonlinear two-point boundary value problems using iterated deferred corrections, Part 1: A survey and comparison of some one-step formulae. Comput. Math. Appl. 1986, 12, 1029–1048. [Google Scholar] [CrossRef]
- Cash, J.R. On the numerical integration of nonlinear two-point boundary value problems using iterated deferred corrections, Part 2: The development and analysis of highly stable deferred correction formulae. SIAM J. Numer. Anal. 1988, 25, 862–882. [Google Scholar] [CrossRef]
- Cash, J.R.; Wright, R.W. Continuous extensions of deferred correction schemes for the numerical solution of nonlinear two-point boundary value problems. Appl. Numer. Math. 1998, 28, 227–244. [Google Scholar] [CrossRef]
- Ascher, U.M.; Mattheij, R.M.M.; Russell, R.D. Numerical Solution of Boundary Value Problems for Ordinary Differential Equations; SIAM: Philadelphia, PA, USA, 1995. [Google Scholar]
- Liu, C.S. The Lie-group shooting method for nonlinear two-point boundary value problems exhibiting multiple solutions. Comput. Model. Eng. Sci. 2006, 13, 149–163. [Google Scholar]
- Cabada, A.; Pouso, R.L. Existence theory for functional p-Laplacian equations with variable exponents. Nonlinear Anal. 2003, 52, 557–572. [Google Scholar] [CrossRef]
- Cabada, A.; O’Regan, D.; Pouso, R.L. Second order problems with functional conditions including Sturm-Liouville and multipoint conditions. Math. Nachr. 2008, 281, 1254–1263. [Google Scholar] [CrossRef]
- Mawhin, J.; Thompson, H.B. Bounding surfaces and second order quasilinear equations with compatible nonlinear functional boundary conditions. Adv. Nonlinear Stud. 2011, 11, 157–172. [Google Scholar] [CrossRef]
- Erbe, L.H. Nonlinear boundary value problems for second order differential equations. J. Diff. Equ. 1970, 7, 459–472. [Google Scholar] [CrossRef]
- Mawhin, J.; Schmitt, K. Upper and lower solutions and semilinear second order elliptic equations with non-linear boundary conditions. Proc. Roy. Soc. Edinburgh 1984, 97, 199–207. [Google Scholar] [CrossRef]
- De Coster, C.; Habets, P. Two-Point Boundary Value Problems: Lower And Upper Solutions; Elsevier: New York, NY, USA, 2006. [Google Scholar]
- He, J.H. Variational iteration method – a kind of non-linear analytical technique: Some examples. Int. J. Non-linear Mech. 1999, 34, 699–708. [Google Scholar] [CrossRef]
- He, J.H. Variational iteration method for autonomous ordinary systems. Appl. Math. Comput. 2000, 114, 115–123. [Google Scholar] [CrossRef]
- Herisanu, N.; Marinca, V. A modified variational iteration method for strongly nonlinear problems. Nonlinear Sci. Lett. A 2010, 1, 183–192. [Google Scholar]
- Turkyilmazoglu, M. An optimal variational iteration method. Appl. Math. Lett. 2011, 24, 762–765. [Google Scholar] [CrossRef]
- Wang, X.; Atluri, S.N. A unification of the concepts of the variational iteration, Adomian decomposition and Picard iteration methods; and a local variational iteration method. Comput. Model. Eng. Sci. 2016, 111, 567–585. [Google Scholar]
- Chang, S.H. Convergence of variational iteration method applied to two-point diffusion problems. Appl. Math. Model. 2016, 40, 6805–6810. [Google Scholar] [CrossRef]
- Chang, S.H. A variational iteration method involving Adomian polynomials for a strongly nonlinear boundary value problem. East Asian J. Appl. Math. 2019, 9, 153–164. [Google Scholar]
- Farkas, M. Periodic Motions; Springer: New York, NY, USA, 1994. [Google Scholar]
- Liapunov, A.M. Sur une série dans la théorie des équations différentielles linéaires du second ordre à coefficients périodiques. Zap. Akad. Nauk Fiz.-Mat. Otd. 8th series 1902, 13, 1–70. [Google Scholar]
- He, J.H. Homotopy perturbation method: A new nonlinear analytical technique. Appl. Math. Comput. 2003, 135, 73–79. [Google Scholar] [CrossRef]
- He, J.H. Homotopy perturbation method for solving boundary value problems. Phy. Lett. A 2006, 350, 87–88. [Google Scholar] [CrossRef]
- Noor, M.A.; Mohyud-Din, S.T. Homotopy perturbation method for solving sixth-order boundary value problems. Comput. Math. Appl. 2008, 55, 2953–2972. [Google Scholar] [CrossRef]
- Chun, C.; Sakthivel, R. Homotopy perturbation technique for solving two-point boundary value problems–comparison with other methods. Comput. Phy. Communi. 2010, 181, 1021–1024. [Google Scholar] [CrossRef]
- Khuri, S.A.; Sayfy, A. Generalizing the variational iteration method for BVPs: Proper setting of the correction functional. Appl. Math. Lett. 2017, 68, 68–75. [Google Scholar] [CrossRef]
- Liu, C.S.; Chang, C.W. Boundary shape function method for nonlinear BVP, automatically satisfying prescribed multipoint boundary conditions. Bound. Value Prob. 2020, 2020, 139. [Google Scholar] [CrossRef]
- Coddington, E.A.; Levinson, N. Theory of Ordinary Differential Equations; McGraw-Hill: New York, NY, USA, 1955. [Google Scholar]
- Reid, W.T. Ordinary Differential Equations; John Wiley & Son: New York, NY, USA, 1971. [Google Scholar]
- Zhou, Z.; Shen, J. A second-order boundary value problem with nonlinear and mixed boundary conditions: Existence, uniqueness, and approximation. Abstr. Appl. Anal. 2010, 2010, 287473. [Google Scholar] [CrossRef]
- Liu, C.S.; Chang, J.R. Boundary shape functions methods for solving the nonlinear singularly perturbed problems with Robin boundary conditions. Int. J. Nonlinear Sci. Numer. Simul. 2020, 21, 797–806. [Google Scholar] [CrossRef]
- Rani, G.S.; Jayan, S.; Nagaraja, K.V. An extension of golden section algorithm for n-variable functions with MATLAB code. IOP Conf. Ser. Mater. Sci. Eng. 2018, 577, 012175. [Google Scholar] [CrossRef]
- Lu, J. Variational iteration method for solving two-point boundary value problems. J. Comput. Appl. Math. 2007, 207, 92–95. [Google Scholar] [CrossRef]
- Adomian, G.; Elrod, M.; Rach, R. A new approach to boundary value equations and application to a generalization of Airy’s equation. J. Math. Anal. Appl. 1989, 140, 554–568. [Google Scholar] [CrossRef]
- Ha, S.N. A nonlinear shooting method for two-point boundary value problems. Comput. Math. Appl. 2001, 42, 1411–1420. [Google Scholar] [CrossRef]
- Ha, S.N.; Lee, C.R. Numerical study for two-point boundary value problems using Green’s functions. Comput. Math. Appl. 2002, 44, 1599–1608. [Google Scholar] [CrossRef]
- Liu, C.S.; Kuo, C.L.; Chang, C.W. Decomposition-linearization-sequential homotopy methods for nonlinear differential/integral equations. Mathematics 2024, 12, 3557. [Google Scholar] [CrossRef]
- Liu, C.S.; Chang, C.W. Lie-group shooting/boundary shape function methods for solving nonlinear boundary value problems. Symmetry 2022, 14, 778. [Google Scholar] [CrossRef]
- Chang, C.W.; Chang, J.R.; Liu, C.S. The Lie-group shooting method for solving classical Blasius flat-plate problem. Comput. Mater. Contin. 2008, 7, 139–153. [Google Scholar]
- Cortell, R. Numerical solutions of the classical Blasius flat-plate problem. Appl. Math. Comput. 2005, 170, 706–710. [Google Scholar] [CrossRef]
- Zheng, L.C.; Chen, X.H.; Chang, X.X. Analytical approximants for a boundary layer flow on a stretching moving surface with a power velocity. Int. J. Appl. Mech. Eng. 2004, 9, 795–802. [Google Scholar]
- Khuri, S.A.; Sayfy, A. Self-adjoint singularly perturbed boundary value problems: An adaptive variational approach. Math. Meth. Appl. Sci. 2013, 36, 1070–1079. [Google Scholar] [CrossRef]



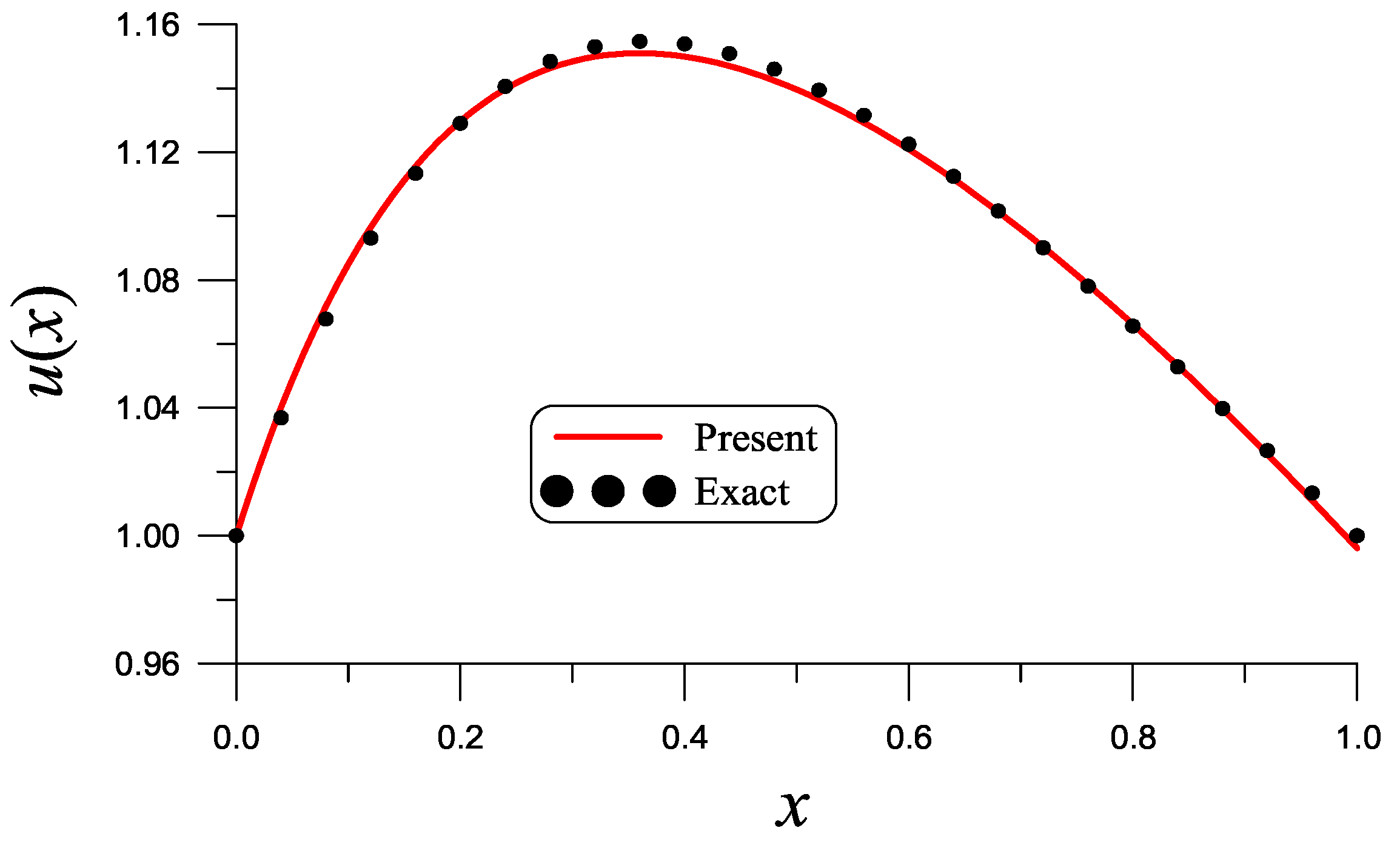
| x | Exact | Present | Lu [32] |
|---|---|---|---|
| 0 | 1.00000 | 1.00000 | 0.8646 |
| 0.1 | 1.00063 | 1.00057 | 0.8665 |
| 0.2 | 1.00250 | 100230 | 0.8723 |
| 0.3 | 1.00563 | 1.00520 | 0.8820 |
| 0.4 | 1.01000 | 1.00930 | 0.8956 |
| 0.5 | 1.01563 | 1.01465 | 0.9131 |
| 0.6 | 1.02250 | 1.02130 | 0.9346 |
| 0.7 | 1.03062 | 1.02932 | 0.9603 |
| 0.8 | 1.04000 | 1.03880 | 0.9901 |
| 0.9 | 1.05062 | 1.04982 | 1.0241 |
| 1.0 | 1.06250 | 1.06250 | 1.0625 |
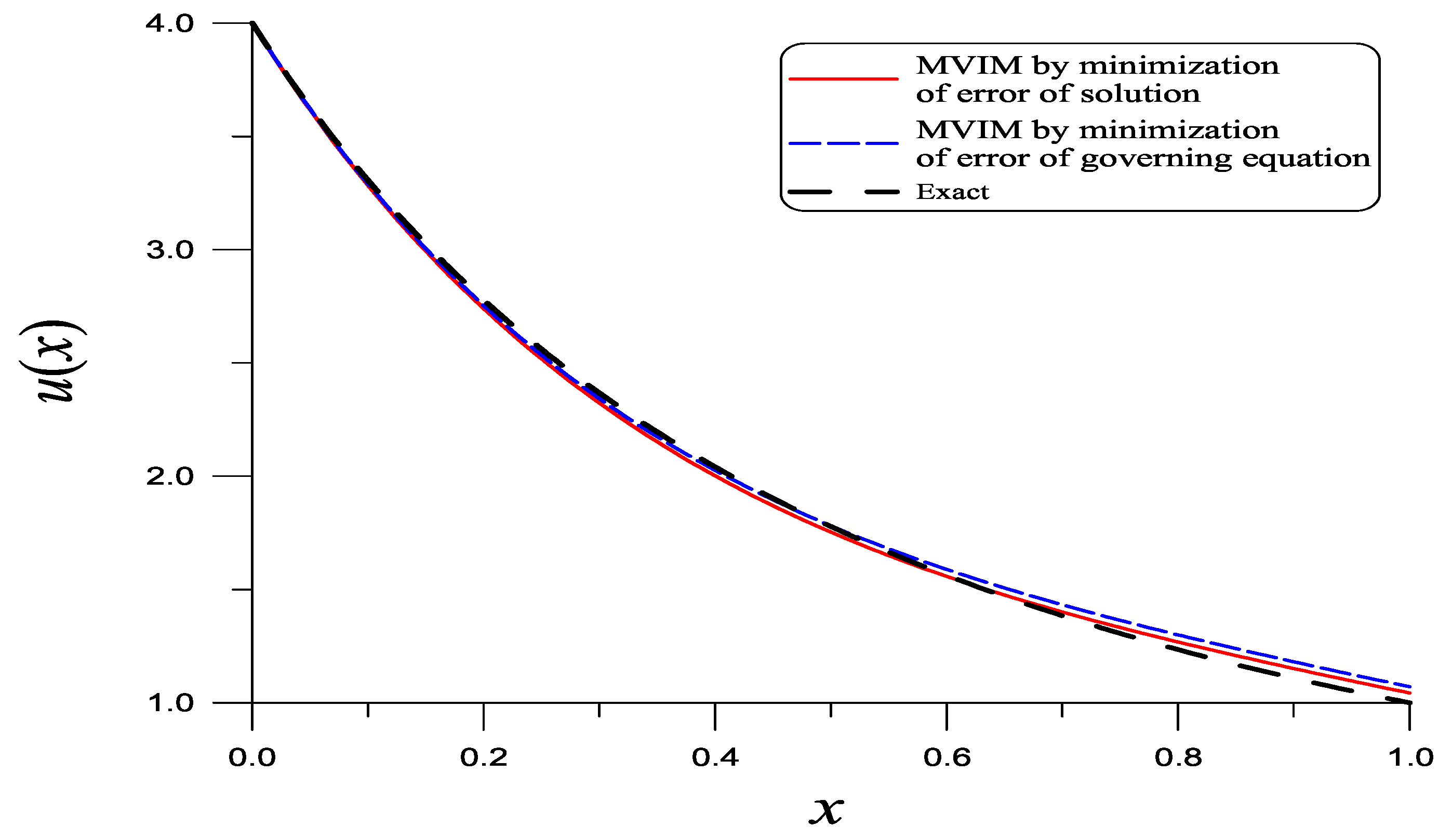
| x | Exact | Present | Absolute Error |
|---|---|---|---|
| 0 | 4.0000 | 4.0000 | 0.0 |
| 0.1 | 3.3058 | 3.3135 | 0.0077 |
| 0.2 | 2.7778 | 2.8073 | 0.0295 |
| 0.3 | 2.3669 | 2.4295 | 0.0626 |
| 0.4 | 2.0408 | 2.1372 | 0.1964 |
| 0.5 | 1.7778 | 1.8961 | 0.1183 |
| 0.6 | 1.5625 | 1.6810 | 0.1185 |
| 0.7 | 1.3841 | 1.4751 | 0.0910 |
| 0.8 | 1.2346 | 1.2706 | 0.0360 |
| 0.9 | 1.1080 | 1.0685 | 0.0395 |
| 1.0 | 1.0000 | 0.8785 | 0.1215 |
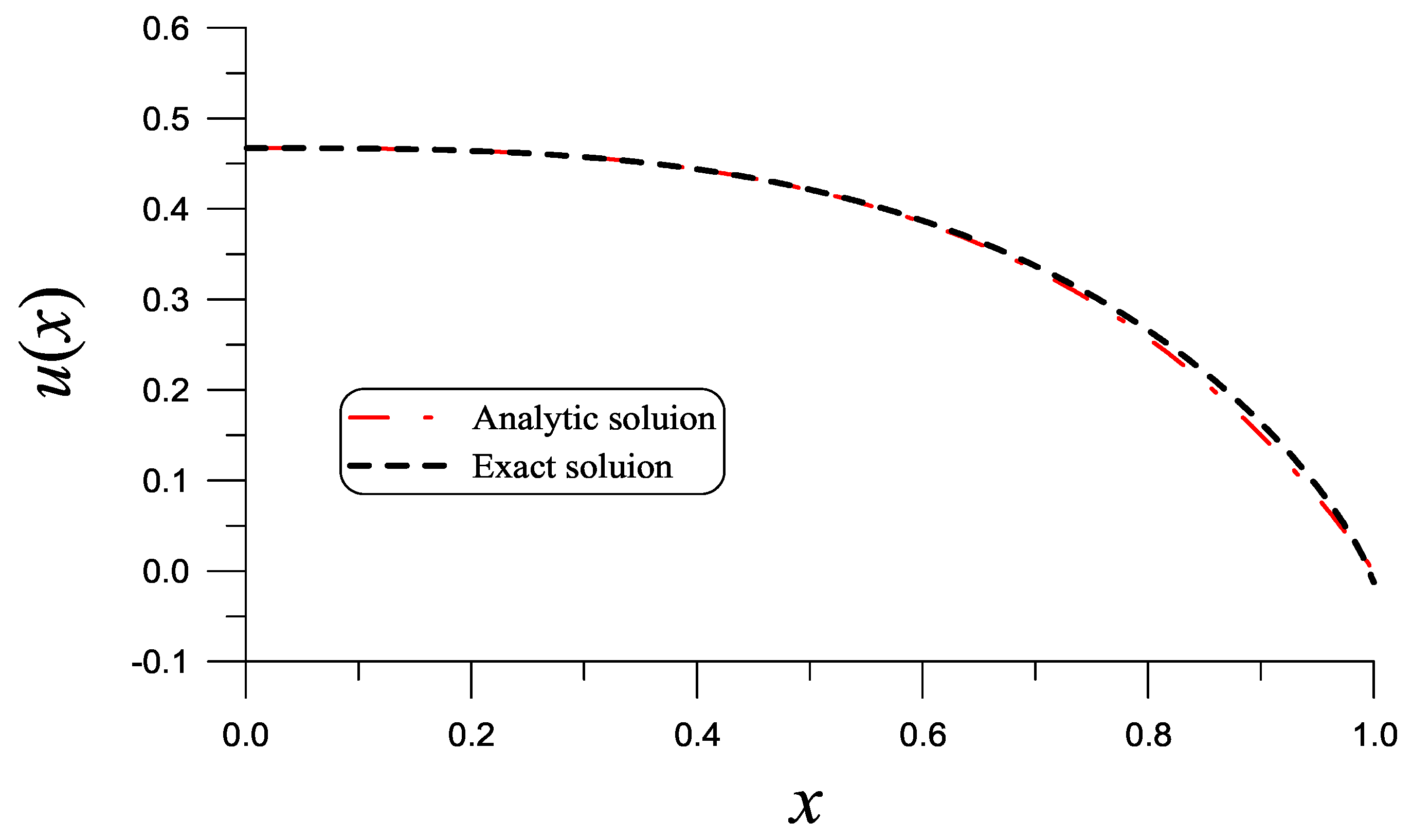
| x | Equation (115) | Equation (116) | Exact | Equation (125) |
|---|---|---|---|---|
| 0.1 | −0.658247 | −0.654982 | −0.654333 | −0.826110 |
| 0.2 | −0.481409 | −0.479186 | −0.478722 | −0.600393 |
| 0.4 | −0.118469 | −0.119156 | −0.119050 | −0.136309 |
| 0.5 | 0.064340 | 0.061822 | 0.061794 | 0.095388 |
| 0.7 | 0.430574 | 0.424832 | 0.424726 | 0.540575 |
| 0.9 | 0.804495 | 0.799604 | 0.799879 | 0.902546 |
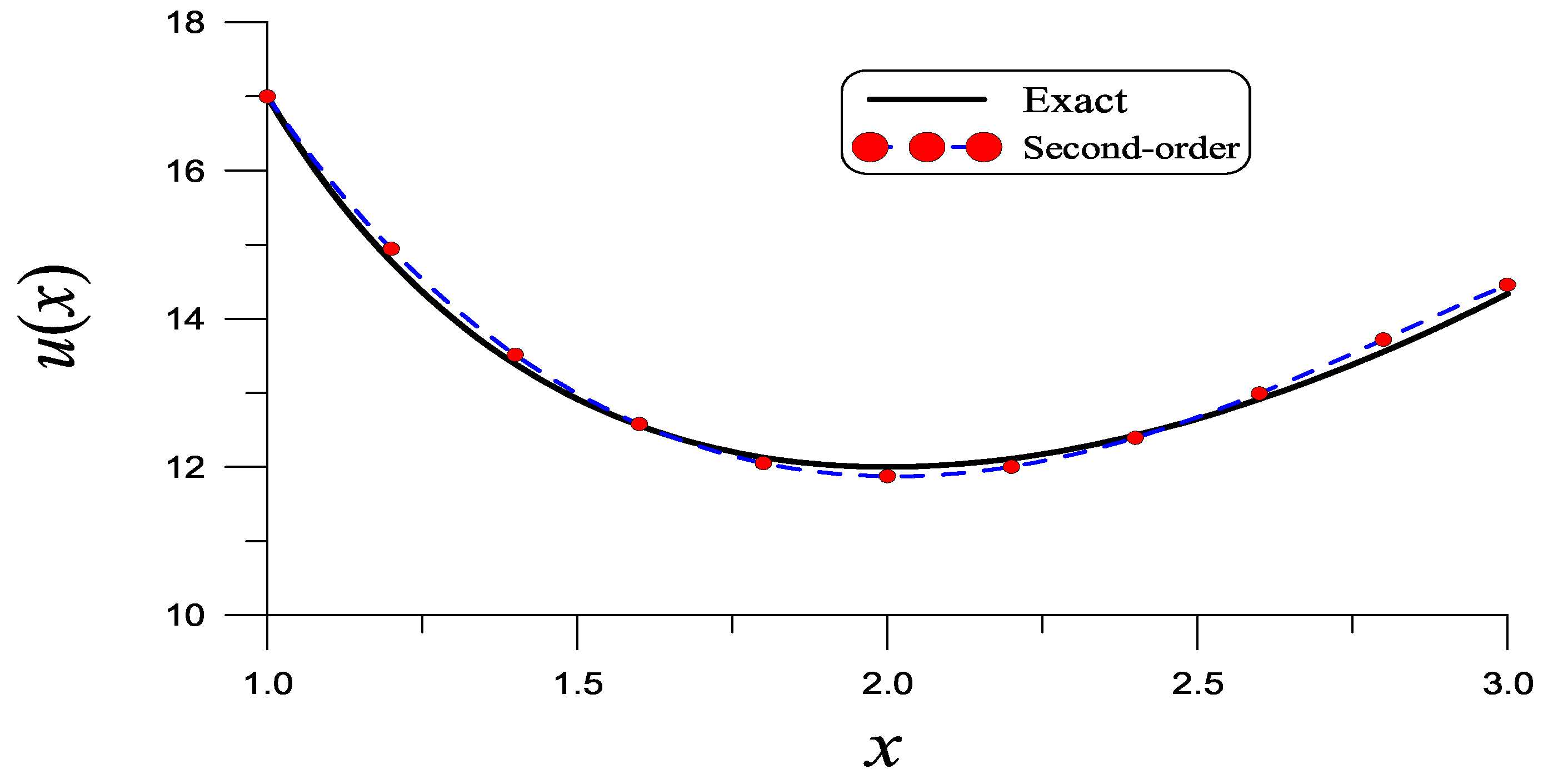
| x | 1.1 | 1.4 | 1.8 | 2.0 | 2.4 | 2.7 | 3 |
| Exact | 15.755 | 13.389 | 12.129 | 12 | 12.427 | 13.216 | 14.333 |
| Present | 15.883 | 13.513 | 12.053 | 11.875 | 12.394 | 13.346 | 14.458 |
| RE |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, C.-S.; Li, B.; Kuo, C.-L. Variational Iteration and Linearized Liapunov Methods for Seeking the Analytic Solutions of Nonlinear Boundary Value Problems. Mathematics 2025, 13, 354. https://doi.org/10.3390/math13030354
Liu C-S, Li B, Kuo C-L. Variational Iteration and Linearized Liapunov Methods for Seeking the Analytic Solutions of Nonlinear Boundary Value Problems. Mathematics. 2025; 13(3):354. https://doi.org/10.3390/math13030354
Chicago/Turabian StyleLiu, Chein-Shan, Botong Li, and Chung-Lun Kuo. 2025. "Variational Iteration and Linearized Liapunov Methods for Seeking the Analytic Solutions of Nonlinear Boundary Value Problems" Mathematics 13, no. 3: 354. https://doi.org/10.3390/math13030354
APA StyleLiu, C.-S., Li, B., & Kuo, C.-L. (2025). Variational Iteration and Linearized Liapunov Methods for Seeking the Analytic Solutions of Nonlinear Boundary Value Problems. Mathematics, 13(3), 354. https://doi.org/10.3390/math13030354








