Semi-Analytical Closed-Form Solutions for Dynamical Rössler-Type System

Abstract

Mathematical models and numerical simulations are necessary to understand the functions of biological rhythms, to comprehend the transition from simple to complex behavior and to delineate the conditions under which they arise. The aim of this work is to investigate the R$\ddot{\mathrm{o}}$ssler-type system. This system could be proposed as a theoretical model for biological rhythms, generalizing this formula for chaotic behavior. It is assumed that the R$\ddot{\mathrm{o}}$ssler-type system has a Hamilton–Poisson realization. To semi-analytically solve this system, a Bratu-type equation was explored. The approximate closed-form solutions are obtained using the Optimal Parametric Iteration Method (OPIM) using only one iteration. The advantages of this analytical procedure are reflected through a comparison between the analytical and corresponding numerical results. The obtained results are in a good agreement with the numerical results, and they highlight that our procedure is effective, accurate and usefully for implementation in applicationssuch as an oscillator with cubic and harmonic restoring forces, the Thomas–Fermi equation and the Lotka–Voltera model with three species.

1. Introduction

Many applications in medicine, outdoor weather control applications, secure communication techniques and so on are based on the study of chaotic dynamic systems. This work explored a Bratu-type equation [1] to semi-analytically solve a R$\ddot{\mathrm{o}}$ssler-type system [2]. Various numerical methods use the Bratu problem as a test bed, and the generalization of this problem has led to the variety of applications in which it arises, such as radiative heat transfer, thermal reactions, chemical reactor theory, nanotechnology and so on.

Raja et al. [3] used the genetic algorithm (GA) and the active set method (ASM) to solve Bratu-type equations with applications in electrically conducting solids and various other physical phenomena. Caglar et al. [4] reported the dynamic behaviors of the solution of Bratu’s equation by computing the corresponding Lyapunov exponent, power spectra and cobweb diagrams modeling the chaotic regimes. Jalilian [5] obtained smooth approximate solutions using the non-polynomial spline method in comparison with those obtained using B-spline, Laplace and decomposition methods. Kafri et al. [6] developed an iterative algorithm combining Green’s function and fixed-point iterative schemes to solve the nonlinear Bratu’s boundary value problem. Hichar et al. [7] reported some solutions to this equation and its applications in electrostatics and plasma physics. Mohsen [8] discussed different iterative schemes, such as the finite-difference method and nonstandard finite-difference methods with a simple sinusoidal starting function, obtaining a simple solution for the Bratu problem. Wazwaz [9] applied the Adomian decomposition method to determine exact solutions for Bratu-type equations. Syam et al. [10] numerically solved Bratu’s equation using the Laplace–Adomain decomposition method. Boyd [11] built an analytical solution by using a series of even Chebyshev polynomials and comparing them with the corresponding numerical results obtained using the collocation or pseudospectral method. Abd et al. [12] applied some perturbation expansion methods to analytically solve the one-dimensional Bratu problem. Abbasbandy et al. [13] solved the Bratu equation using the Lie-group shooting method. Deniz et al. [14] proposed a new approach, namely the Optimal Perturbation Iteration Method, for solving Bratu-type problems. Keshavarz et al. [15] developed an efficient numerical method, namely the Taylor wavelet method, for obtaining analytical solutions for the Bratu-type equations. Abdelhakem et al. [16] solved some nonlinear problems using two spectral Legendre’s derivative algorithms. Singh et al. [17] adopted an efficient technique (the Haar wavelet method) for numerically solving Bratu-type equations. Mohsen [18] used Green’s function for obtaining the integral solution to Bratu’s equation. Temimi et al. [19] proposed a new computational scheme for the classical Bratu equation. Jator et al. [20] explored numerical solutions by applying the Block Nystr$\ddot{\mathrm{o}}$m Method. Behl et al. [21] presented a family of iterative methods to solve Bratu’s equation. Tomar et al. [22] introduced an efficient analytical iterative method that obtains a semi-analytical solution for the Bratu-type problem. Karamollahi et al. [23] used Hermite interpolation to approximate dual solutions for the strongly nonlinear one-dimensional Bratu problem. Jator et al. [24] introduced a new numerical scheme to solve Bratu’s problem. Singh et al. [25] applied the Homotopy Perturbation Method (HPM) to solve a class of Bratu’s equations. Aydinlik et al. [26] approached the Bratu-type equation based on the Smooth Composite Chebyshev Finite-Difference Method. Aksoy et al. [27] and obtained different perturbation–iteration solutions to the Bratu-type equations. Venkatesh et al. [28] used the Legendre wavelet method to solve Bratu-type initial value problems. Ragb et al. [29] introduced a numerical scheme based on differential quadrature methods for solving the Bratu problem. Masood et al. [30] tested Mexican Hat Wavelet neural networks for solving Bratu-type nonlinear systems. Ahmad et al. [31] proposed an efficient algorithm to obtain better solutions for Bratu differential equations.

Recently, Karimov et al. [32,33] proposed a novel technique by studying the synchronization between a circuit modeling the R$\ddot{\mathrm{o}}$ssler chaotic system. Ding et al. [34] investigated the existence of a Shilnikov-type homoclinic orbit of in the R$\ddot{\mathrm{o}}$ssler system and spiral chaos using the series expressions of the solution.

The impact of complex dynamic systems/nonlinear differential equations on real-world applications has led to the development of new mathematical methods for approaching dynamical behaviors and that consider the existence of chaos caused by the influence of several physical parameters. For example, Zhou [35] examined the asymptotic behavior of the Nicholson model with neutral-type delays, Zhou [36] studied a four-dimensional predator–prey chemostat model that considered the dynamical mechanism of cyclic persistence and the existence of periodic solutions. The difference between a classical and anomalous diffusion equation was analyzed by Zhao et al. [37].

Nonlinear wave dynamics, such as solitons, breathers, rogue waves and semi-rational solutions on periodic backgrounds, which are important for many physical systems, were investigated by Li et al. [38], who pointed out their dynamic behaviors.

In general, with chaotic systems, the exact solution can no longer be controlled, but their dynamic properties are analyzed with specific mathematical methods, such as bifurcation routes, Poincare maps, frequency spectra, amplitude modulation, topological horseshoes, the existence of a heteroclinic orbit or homoclinic orbit, equilibrium, Lyapunov exponent spectra, a dissipative system, phase portraits, bifurcation diagrams and Hopf bifurcation. These properties characterize the chaotic behaviors of dynamical systems.

The organization of the remainder of this paper is as follows. In Section 2, a short presentation of Bratu’s problem and the R$\ddot{\mathrm{o}}$sller-type system is provided. Section 3 describes the Optimal Parametric Iteration Method and semi-analytical solutions. Section 4 contains the numerical results, and Section 5 summarizes the concluding remarks.

2. Preliminary

2.1. Bratu’s Problem

The original one-dimensional Bratu nonlinear boundary value problem is presented in [1]:

$$\begin{array}{c}{u}^{\prime \prime }\left(t\right)+\lambda e^{u\left(t\right)}=0,\lambda >0\hfill \\ u\left(0\right)=0,u\left(1\right)=0.\hfill \end{array}$$

(1)

The exact solution of the nonlinear problem of Equation (1) is [39]

$$u\left(t\right)=-2ln\left[\frac{cosh\left(0.5\right(t-0.5\left)\theta \right)}{cosh(\theta /4)}\right],$$

where $\theta$ is the solution of the equation $\theta =\sqrt{2\lambda }cosh(\theta /4)$, and $\lambda$ is a parameter that describes the number of possible solutions, for $\lambda <{\lambda }_c$ and no solution for $\lambda >{\lambda }_c$, ${\lambda }_c=3.513830719$.

2.2. The R$\ddot{o}$sller-Type System

The R$\ddot{\mathrm{o}}$sller-type system is written as follows [2]:

$$\left\{\begin{array}{c}\dot{x}=-y-z\hfill \\ \dot{y}=x+ay\hfill \\ \dot{z}=b-cz+xz\hfill \end{array}\right.,a,b,c\in \mathbb{R}.$$

(2)

This subsection emphasizes the approximate closed-form solution of system (2) in the completely integrable case ($a=b=c=0$) studied in [40,41,42]:

$$\left\{\begin{array}{c}\dot{x}=-y-z\hfill \\ \dot{y}=x\hfill \\ \dot{z}=xz\hfill \end{array}\right..$$

(3)

System (3) is a Hamiltonian mechanical system with a Hamiltonian–Poisson structure characterized by the constants of motion given by

$$\begin{array}{c}H(x,y,z)=\frac{1}{2}(x^2+y^2)+z\mathrm{the}\mathrm{Hamiltonian},\hfill \\ C(x,y,z)=z{e}^{-y}\mathrm{the}\mathrm{Casimir}.\hfill \end{array}$$

(4)

In considering the initial conditions

$$x\left(0\right)=x_0,y\left(0\right)=y_0,z\left(0\right)=z_0,$$

(5)

the exact solutions of Equations (3) and (5) are written as the intersection of the surfaces:

$$\left\{\begin{array}{c}\frac{1}{2}(x^2+y^2)+z=\frac{1}{2}(x_0^2+y_0^2)+z_0\hfill \\ z{e}^{-y}=z_0{e}^{-y_0}\hfill \end{array}\right..$$

(6)

In taking into account Equation (4), it is more convenient to consider the following transformation:

$$\left\{\begin{array}{c}y-y_0=u\hfill \\ z=z_0{e}^u\hfill \\ x=\dot{u}\hfill \end{array}\right.,$$

(7)

which describes the closed-form solutions of Equations (3) and (5). The unknown smooth function u from Equation (7) is the solution of the nonlinear initial value problem

$$\left\{\begin{array}{c}\ddot{u}+u+z_0{e}^u+y_0=0\hfill \\ u\left(0\right)=0,\dot{u}\left(0\right)=x_0,\hfill \end{array}\right.$$

(8)

obtained from the first item of Equation (3).

The obtained problem given by Equation (8) is a Bratu-type nonlinear initial value problem with a linear control.

Section 3 presents some semi-analytical solutions of Equation (8) obtained using the OPIM procedure and that are denoted by $\overline{u}$ or ${\overline{u}}_{OPIM}$.

There are some analytical methods for solving nonlinear differential equations as follows: the optimal iteration parametrization method (OIPM) [43], the optimal homotopy asymptotic method (OHAM) [44,45,46], the optimal homotopy perturbation method (OHPM) [47,48,49] and the modified optimal parametric iteration method [50].

In taking into consideration Equation (7), a semi-analytical closed-form solutions of Equations (3) and (5) are

$$\left\{\begin{array}{c}\overline{x}=\dot{\overline{u}}\hfill \\ \overline{y}=\overline{u}+y_0\hfill \\ \overline{z}=z_0{e}^{\overline{u}}\hfill \end{array}\right.,$$

(9)

where $\overline{u}$ is a semi-analytical solution of Equation (8).

3. The Optimal Parametric Iteration Method

3.1. Preliminary

Some iteration procedures for solving different nonlinear problems were developed in [51,52,53,54,55].

The idea of the Optimal Parametric Iteration Method is to consider the following second-order nonlinear differential equation:

$$\begin{array}{c}{\displaystyle \mathcal{L}\left[u\left(t\right)\right]+\mathcal{N}[t,u\left(t\right),\dot{u}\left(t\right),\ddot{u}\left(t\right)]-g\left(t\right)=0,t\in \mathcal{I}\subset \mathbb{R},}\hfill \end{array}$$

(10)

subject to the initial conditions

$$\begin{array}{c}{\displaystyle \mathcal{B}[u\left(t\right),\dot{u}\left(t\right)]=0,}\hfill \end{array}$$

(11)

where $\mathcal{L}$ is a linear operator; $\mathcal{N}$, a nonlinear operator; $\mathcal{B}$, a boundary operator; g, a known function; u, an unknown smooth function depending on the independent variable t; and $\dot{u}\left(t\right)=\frac{du}{dt}$.

For real values $\alpha$, $\beta$ and $\gamma$, by applying the well-known Taylor formula for an analytic function F, we obtain

$$\begin{array}{c}F(t,u+\alpha ,\dot{u}+\beta ,\ddot{u}+\gamma )=F(t,u,\dot{u},\ddot{u})+\frac{\alpha }{1!}{F}_u(t,u,\dot{u},\ddot{u})+\hfill \\ +\frac{\beta }{1!}{F}_{\dot{u}}(t,u,\dot{u},\ddot{u})+\frac{\gamma }{1!}{F}_{\ddot{u}}(t,u,\dot{u},\ddot{u})+\cdots ,\hfill \end{array}$$

(12)

where $F_u=\frac{\partial F}{\partial u}$. Instead of solving nonlinear differential Equation (10), one can solve another equation, making recourse to Equation (12) and to the following scheme, namely, the Optimal Parametric Iteration Method (OPIM), introduced by Marinca et al. [43]:

$$\begin{array}{c}{\displaystyle \mathcal{L}\left[u_{n+1}\left(t\right)\right]+\mathcal{N}[t,u_n,{\dot{u}}_n,{\ddot{u}}_n]+{\alpha }_n(t,C_i){\mathcal{N}}_u[t,u_n,{\dot{u}}_n,{\ddot{u}}_n]+}\hfill \\ +{\beta }_n(t,C_j){\mathcal{N}}_{\dot{u}}[t,u_n,{\dot{u}}_n,{\ddot{u}}_n]+{\gamma }_n(t,C_k){\mathcal{N}}_{\ddot{u}}[t,u_n,{\dot{u}}_n,{\ddot{u}}_n]+\cdots -g\left(t\right)=0\hfill \\ {\displaystyle \mathcal{B}[u_{n+1}\left(t\right),{\dot{u}}_{n+1}\left(t\right)]=0}\hfill \end{array},n\ge 0,$$

(13)

where ${\alpha }_n(t,C_i)$, ${\beta }_n(t,C_j)$ and ${\gamma }_n(t,C_k)$ are auxiliary continuous functions; ${\mathcal{N}}_F=\frac{\partial \mathcal{N}}{\partial F}$ (obtained from the Taylor series expansion of the nonlinear operator $\mathcal{N}[t,u\left(t\right),\dot{u}\left(t\right),\ddot{u}\left(t\right)]$); $u_{n+1}\left(t\right)$ is the (n + 1)-th-order approximate solution of Equations (10) and (11), denoted by $\overline{u}\left(t\right)$ or ${\overline{u}}_{OPIM}\left(t\right)$; and $u_0\left(t\right)$ is the initial approximation, a solution of the following linear differential problem:

$$\begin{array}{c}{\displaystyle \mathcal{L}\left[u_0\left(t\right)\right]-g\left(t\right)=0}\hfill \\ {\displaystyle \mathcal{B}[u_0\left(t\right),{\dot{u}}_0\left(t\right)]=0.}\hfill \end{array}$$

(14)

The real constants $C_i$, $C_j$ and $C_k$ are unknown convergence-control parameters and can be optimally computed.

The $(n+1)$-order approximate solution of Equations (10) and (11) is well determined if the convergence-control parameters are known.

In the OPIM, the linear operator $\mathcal{L}$ is arbitrarily chosen, not the physical parameters. There are situations when the choice of physical parameters give rise to the chaotic behavior of the dynamic system. This happens in the cases of choosing higher values for the damping factor or exceeding the optimal resonance conditions, as well as in the case of arbitrarily choosing the initial conditions.

If $u_0\left(t\right)$ is the initial approximation of Equation (14), the nonlinear operators $\mathcal{N}[t,u_0,{\dot{u}}_0,{\ddot{u}}_0]$, ${\mathcal{N}}_u[t,u_0,{\dot{u}}_0,{\ddot{u}}_0]$, ${\mathcal{N}}_{\dot{u}}[t,u_0,{\dot{u}}_0,{\ddot{u}}_0]$ and ${\mathcal{N}}_{\ddot{u}}[t,u_0,{\dot{u}}_0,{\ddot{u}}_0]$ that appear in Equation (13) have the form

$$\begin{array}{c}\sum _{i=1}^{n_{max}}{h}_i\left(t\right)g_i\left(t\right),\hfill \end{array}$$

(15)

where $n_{max}$ is a positive integer, and $h_i\left(t\right)$ and $g_i\left(t\right)$ are known functions that depend on $u_0\left(t\right)$.

For $u_{n+1}$ an $(n+1)$-order approximate solution of Equations (10) and (11), the validation of this procedure is highlighted by computing the residual function given by

$$\begin{array}{c}{\displaystyle \mathcal{R}\left(t\right)=\mathcal{L}\left[u_{n+1}\left(t\right)\right]+\mathcal{N}[t,u_{n+1}\left(t\right),{\dot{u}}_{n+1}\left(t\right),{\ddot{u}}_{n+1}\left(t\right)]-g\left(t\right),t\in \mathcal{I}\subset \mathbb{R},}\hfill \end{array}$$

(16)

such that ${\displaystyle \mathcal{R}\left(t\right)<<1}$ for all $t\in \mathcal{I}$.

Using the linearly independent functions $h_1,h_2,\cdots ,h_m$, we introduce some types of approximate solutions of Equation (10).

Definition 1.

A sequence of functions ${\left\{s_m\left(t\right)\right\}}_{m\ge 1}$ of the form

$${\displaystyle s_m\left(t\right)=\sum _{i=1}^m{\alpha }_m^i·h_i\left(t\right),m\ge 1,{\alpha }_m^i\in \mathbb{R},}$$

(17)

is called an OPIM sequence of Equation (10).

The functions of the OPIM sequences are called OPIM functions of Equation (10).

The OPIM sequences ${\left\{s_m\left(t\right)\right\}}_{m\ge 1}$ with the property

$${\displaystyle \underset{m\to \infty }{lim}\mathcal{R}(t,s_m\left(t\right))=0}$$

are called convergent to the solution of Equation (10), where $\mathcal{R}(t,u(t\left)\right)=\mathcal{L}\left[u\right(t\left)\right]+$$\mathcal{N}[t,u\left(t\right),\dot{u}\left(t\right),\ddot{u}\left(t\right)]-g\left(t\right)$.

Definition 2.

The OPIM functions $\tilde{F}$ satisfying the conditions

$$\left|\mathcal{R}(t,\tilde{F}\left(t\right))\right|<\epsilon ,\mathcal{B}\left(\tilde{F}(t,C_i),\frac{d\tilde{F}(t,C_i)}{dt}\right)=0$$

(18)

are called ε-approximate OPIM solutions of Equation (10).

Definition 3.

The OPIM functions $\tilde{F}$ satisfying the conditions

$${\displaystyle \underset{0}{\overset{\infty }{\int }}{\mathcal{R}}^2(t,\tilde{F}\left(t\right))dt\le \epsilon ,\mathcal{B}\left(\tilde{F}(t,C_i),\frac{d\tilde{F}(t,C_i)}{dt}\right)=0}$$

(19)

are called weak ε-approximate OPIM solutions of Equation (10) on the real interval $(0,\infty )$.

Remark 1.

An ε-approximate OPIM solution of Equation (10) is also a weak ε-approximate OPIM solution. It follows that the set of weak ε-approximate OPIM solutions of Equation (10) also contains the approximate OPIM solutions of Equation (10).

The existence of weak $\epsilon$-approximate OPIM solutions results from the theorem presented above.

Theorem 1.

Equation (10) admits a sequence of weak ε-approximate OPIM solutions.

Proof. 

This is similar to the Theorem from [56].

Firstly, the OPIM sequences ${\left\{s_m\right\}}_{m\ge 1}$ are built by considering the approximate OPIM solutions of the type

$${\displaystyle \overline{F}\left(t\right)=\sum _{i=1}^n{C}_m^i·h_i\left(t\right),\mathrm{where}m\ge 1\mathrm{is}\mathrm{fixed}\mathrm{arbitrarily}.}$$

(20)

The unknown parameters $C_m^i$, $i\in \{1,2,\cdots ,n\}$ will be determined.

Upon introducing the approximate solutions $\overline{F}$ into Equation (10), the expression yields

$$\mathcal{R}(t,C_m^i):=\mathcal{R}(t,\overline{F})=\mathcal{L}[\overline{F}\left(t\right)]+\mathcal{N}[t,\overline{F}\left(t\right),\dot{\overline{F}}\left(t\right),\ddot{\overline{F}}\left(t\right)]-g\left(t\right).$$

By attaching the real functional

$${\displaystyle {\mathcal{J}}_1\left(C_m^i\right)=\underset{0}{\overset{\infty }{\int }}{\mathcal{R}}^2(t,C_m^i)dt}$$

(21)

to Equation (10) and imposing the initial conditions, we can determine $l\in \mathbb{N}$, $l\le m$ such that $C_m^1$, $C_m^2$, …, $C_m^l$ are computed as $C_m^{l+1}$, $C_m^{l+2}$, …, $C_m^n$.

The values of ${\tilde{C}}_m^{l+1}$, ${\tilde{C}}_m^{l+2}$, …, ${\tilde{C}}_m^n$ are computed by replacing $C_m^1$, $C_m^2$, …, $C_m^l$ in Equation (21), which provide the minimum of functional (21).

By means of the initial conditions, the values ${\tilde{C}}_m^1$, ${\tilde{C}}_m^2$, …, ${\tilde{C}}_m^l$ as functions of ${\tilde{C}}_m^{l+1}$, ${\tilde{C}}_m^{l+2}$, …, ${\tilde{C}}_m^n$ are determined.

In using the constants ${\tilde{C}}_m^1$, ${\tilde{C}}_m^2$, …, ${\tilde{C}}_m^n$ thus determined, the following OPIM functions

$${\displaystyle s_m\left(t\right)=\sum _{i=1}^n{\tilde{C}}_m^i·h_i\left(t\right)}$$

(22)

are constructed.

The next step is to show that the above OPIM functions $s_m\left(t\right)$ are weak $\epsilon$-approximate OPIM solutions of Equation (10).

Therefore, the OPIM functions $s_m\left(t\right)$ are computed, and in taking into account that the $\overline{F}$ given by (20) are OPIM functions for Equation (10), it follows that

$${\displaystyle 0\le \underset{0}{\overset{\infty }{\int }}{\mathcal{R}}^2(t,s_m\left(t\right))dt\le \underset{0}{\overset{\infty }{\int }}{\mathcal{R}}^2(t,\overline{F}\left(t\right))dt,\forall m\ge 1.}$$

Thus,

$${\displaystyle 0\le \underset{m\to \infty }{lim}\underset{0}{\overset{\infty }{\int }}{\mathcal{R}}^2(t,s_m\left(t\right))dt\le \underset{m\to \infty }{lim}\underset{0}{\overset{\infty }{\int }}{\mathcal{R}}^2(t,\overline{F}\left(t\right))dt.}$$

Since $\overline{F}\left(t\right)$ is convergent to the solution of Equation (10), we obtain

$${\displaystyle \underset{m\to \infty }{lim}\underset{0}{\overset{\infty }{\int }}{\mathcal{R}}^2(t,s_m\left(t\right))dt=0.}$$

It follows that for all $\epsilon >0$, there exists $m_0\ge 1$ such that for all $m\ge 1$ and $m>m_0$, the sequence $s_m\left(t\right)$ is a weak $\epsilon$-approximate OPIM solution of Equation (10). □

Remark 2.

The proof of the above theorem provides us a way to determine a weak ε-approximate OPIM solution of Equation (10), $\overline{F}$. Moreover, in taking into account Remark 1, if $\left|\mathcal{R}\right(t,\overline{F}\left)\right|<\epsilon$, then $\overline{F}$ is also an ε-approximate OPIM solution of the considered equation.

Remark 3.

(1) The integration of Equation (13) produces secular terms of the forms $tcos\left({\omega }_{0}t\right)$, $tsin\left({\omega }_{0}t\right)$, $t^{2}cos\left({\omega }_{0}t\right)$, $t^{2}sin\left({\omega }_{0}t\right)$, $tcos\left(2{\omega }_{0}t\right)$, $tsin\left(2{\omega }_{0}t\right)$ and so on. For the nonlinear oscillator, the secular terms that appear through integration generate the resonance phenomenon. Consequently, the secular terms have to be avoided.

(2) The OPIM method was successfully applied in the case of ODEs with boundary conditions, see Ref. [57], such as the following:

(a) A thin film flow of a fourth-grade fluid down a vertical cylinder:

$$\begin{array}{c}{\displaystyle \eta f^{\prime \prime }\left(\eta \right)+f^{\prime }\left(\eta \right)+k\eta +2b\left[{\left(f^{\prime }\left(\eta \right)\right)}^2+3\eta \left(f^{\prime }{\left(\eta \right)}^2{f}^{\prime \prime }\left(\eta \right)\right)\right]=0}\hfill \\ {\displaystyle f\left(1\right)=0,f^{\prime }\left(d\right)=0,}\hfill \end{array}$$

(23)

where $f^{\prime }\left(\eta \right)=\frac{df}{d\eta }$. The linear operator is chosen as $\mathcal{L}\left[f\left(\eta \right)\right]=\eta f^{\prime \prime }\left(\eta \right)+f^{\prime }\left(\eta \right)+k\eta$.

(b) Thermal radiation on an MHD flow over a stretching porous sheet:

$$\begin{array}{c}{\displaystyle f^{\prime \prime \prime }\left(\eta \right)+f\left(\eta \right)f^{\prime \prime }\left(\eta \right)-f^{\prime }{\left(\eta \right)}^2-M{f}^{\prime }\left(\eta \right)=0}\hfill \\ {\displaystyle {\theta }^{\prime \prime }\left(\eta \right)+\left(a-b{e}^{-\gamma \eta }\right){\theta }^{\prime }\left(\eta \right)-c{e}^{-\gamma \eta }\theta \left(\eta \right)=0}\hfill \\ {\displaystyle f\left(1\right)=\lambda ,f^{\prime }\left(0\right)=1,\theta \left(0\right)=1}\hfill \\ f^{\prime }\left(\eta \right)\to 0,\theta \left(\eta \right)\to 0as\eta \to \infty .\hfill \end{array}$$

(24)

where the initial guess is chosen as ${\theta }_0\left(\eta \right)=0$ and $f_0\left(\eta \right)=\lambda +\frac{1}{\lambda }\left(1-e^{-\gamma \eta }\right)$, with $\gamma =\frac{1}{2}\left(\lambda +\sqrt{{\lambda }^2+4M+4}\right)$.

(c) An oscillator with cubic and harmonic restoring forces:

$$\begin{array}{c}{\displaystyle u^{\prime \prime }\left(t\right)+u\left(t\right)+a{u}^3\left(t\right)+bsinu\left(t\right)=0}\hfill \\ {\displaystyle u\left(0\right)=A,u^{\prime }\left(0\right)=0.}\hfill \end{array}$$

(25)

where linear operator is chosen as $\mathcal{L}\left[u\left(t\right)\right]=u^{\prime \prime }\left(t\right)+u\left(t\right)$.

(d) The Thomas–Fermi equation:

$$\begin{array}{c}{\displaystyle y^{\prime \prime }\left(x\right)=\sqrt{\frac{y^3\left(x\right)}{x}}\iff x{\left[y^{\prime \prime }\left(x\right)\right]}^2-y^3\left(x\right)=0}\hfill \\ {\displaystyle y\left(0\right)=1,y\left(x\right)\to 0\mathrm{as}x\to \infty .}\hfill \end{array}$$

(26)

where linear operator is chosen as $\mathcal{L}\left[y\left(x\right)\right]=y^{\prime \prime }\left(x\right)-{\lambda }^{2}y\left(x\right)$, and the nonlinear operator yields $\mathcal{N}\left[y\left(x\right)\right]=x{\left[y^{\prime \prime }\left(x\right)\right]}^2-y^3\left(x\right)+y^{\prime \prime }\left(x\right)-{\lambda }^{2}y\left(x\right)$.

(e) The Lotka–Voltera model with three species:

$$\begin{array}{c}{\displaystyle x^{\prime }\left(t\right)=x(1-x-\alpha y-\beta z)}\hfill \\ {\displaystyle y^{\prime }\left(t\right)=y(1-\beta x-y-\alpha z)}\hfill \\ {\displaystyle z^{\prime }\left(t\right)=z(1-\alpha x-\beta y-z)}\hfill \\ {\displaystyle x\left(0\right)=a,y\left(0\right)=b,z\left(0\right)=c.}\hfill \end{array}$$

(27)

where the initial approximations are chosen as $x_0\left(t\right)=a{e}^{-t}$, $y_0\left(t\right)=b{e}^{-t}$ and $z_0\left(t\right)=c{e}^{-t}$, or $x_0\left(t\right)=a{e}^{-2t}$, $y_0\left(t\right)=b$ and $z_0\left(t\right)=c{e}^{-t}$, and so on.

3.2. Semi-Analytical Solutions Using OPIM Technique

The applicability of the OPIM procedure for the nonlinear differential problems given by Equation (8) using only one iteration is presented in details below.

$$\begin{array}{c}{\displaystyle \mathcal{L}\left[u\left(t\right)\right]=\ddot{u}\left(t\right)+{\omega }_0^{2}u\left(t\right)}\hfill \\ {\displaystyle \mathcal{N}[t,u\left(t\right),\dot{u}\left(t\right),\ddot{u}\left(t\right)]=\left(1-{\omega }_0^2\right)u\left(t\right)+z_0{e}^{u\left(t\right)}+y_0,t>0.}\hfill \end{array}$$

(28)

For obtaining a semi-analytical solution of Equation (8), it is more convenient to use the approximation in series:

$${\displaystyle e^{u\left(t\right)}=\sum _{i=0}^{\infty }\frac{1}{i!}{u}^i\left(t\right)=1+\frac{1}{1!}u\left(t\right)+\frac{1}{2!}{u}^2\left(t\right)+\dots +\frac{1}{N_{max}!}{u}^{N_{max}}\left(t\right)+\dots .}$$

(29)

In taking into consideration the linear operator given by Equation (28), the initial approximation $u_0\left(t\right)$, which is the solution of Equation (14), is

$$\begin{array}{c}{\displaystyle u_0\left(t\right)={\tilde{C}}_{0}sin\left({\omega }_{0}t\right),}\hfill \end{array}$$

(30)

with ${\tilde{C}}_0=\frac{\dot{u}\left(0\right)}{{\omega }_0}$.

In using Equation (28), a simple computation yields the following expressions:

$$\begin{array}{c}{\displaystyle {\mathcal{N}}_u[t,u,\dot{u},\ddot{u}]=1-{\omega }_0^2+z_0{e}^{u\left(t\right)},{\mathcal{N}}_{\dot{u}}[t,u,\dot{u},\ddot{u}]=0,{\mathcal{N}}_{\ddot{u}}[t,u,\dot{u},\ddot{u}]=0.}\hfill \end{array}$$

(31)

In returning to Equation (13), there are a lot of possibilities to choose from for the following auxiliary functions:

$$\begin{array}{c}{\displaystyle {\alpha }_n(t,{\tilde{B}}_i)={\tilde{B}}_{1}cos\left({\omega }_{0}t\right)+{\tilde{C}}_{1}sin\left({\omega }_{0}t\right),}\hfill \end{array}$$

(32)

or $\begin{array}{c}{\displaystyle {\alpha }_n(t,C_i)={\tilde{B}}_{2}cos\left(2{\omega }_{0}t\right)+{\tilde{C}}_{2}sin\left(2{\omega }_{0}t\right),}\hfill \end{array}$ and so on.

In taking into account Equations (29) and (32), for $N_{max}=2$, a simple integration of Equation (13), using only one iteration $n=1$, yields

$$\begin{array}{c}{\displaystyle u_1\left(t\right)=\frac{-192y_0-192z_0-48z_0{\tilde{C}}_0^2-96{\tilde{C}}_0{\tilde{C}}_1{z}_0}{192{\omega }_0^2}+}\hfill \\ +\frac{1}{192{\omega }_0^2}\left(192y_0+192z_0+64{\tilde{C}}_0^2{z}_0+3{\tilde{B}}_1{\tilde{C}}_0^2{z}_0+128{\tilde{C}}_0{\tilde{C}}_1{z}_0\right)cos\left({\omega }_{0}t\right)+\hfill \\ +\frac{1}{192{\omega }_0^2}\left(-96{\tilde{C}}_0-96{\tilde{C}}_1-96{\tilde{C}}_0{z}_0-64{\tilde{B}}_1{\tilde{C}}_0{z}_0-96{\tilde{C}}_1{z}_0-27{\tilde{C}}_0^2{\tilde{C}}_1{z}_0+192x_0{\omega }_0+\right.\hfill \\ \left.+96{\tilde{C}}_0{\omega }_0^2+96{\tilde{C}}_1{\omega }_0^2\right)sin\left({\omega }_{0}t\right)+\frac{1}{192{\omega }_0^2}\left(-16{\tilde{C}}_0^2{z}_0-32{\tilde{C}}_0{\tilde{C}}_1{z}_0\right)cos\left(2{\omega }_{0}t\right)-\hfill \\ -\frac{3{\tilde{B}}_1{\tilde{C}}_0^2{z}_0}{192{\omega }_0^2}cos\left(3{\omega }_{0}t\right)+\frac{32{\tilde{B}}_1{\tilde{C}}_0{z}_0}{192{\omega }_0^2}sin\left(2{\omega }_{0}t\right)-\frac{3{\tilde{C}}_0^2{\tilde{C}}_1{z}_0}{192{\omega }_0^2}sin\left(3{\omega }_{0}t\right)+\frac{1}{192{\omega }_0^2}\left(96{\tilde{C}}_0{\omega }_0+\right.\hfill \\ \left.+96{\tilde{C}}_1{\omega }_0+96{\tilde{C}}_0{z}_0{\omega }_0+96{\tilde{C}}_1{z}_0{\omega }_0+36{\tilde{C}}_0^2{\tilde{C}}_1{z}_0{\omega }_0-96{\tilde{C}}_0{\omega }_0^3-96{\tilde{C}}_1{\omega }_0^3\right)tcos\left({\omega }_{0}t\right)+\hfill \\ +\frac{1}{192{\omega }_0^2}\left(-96{\tilde{B}}_1{\omega }_0-96{\tilde{B}}_1{z}_0{\omega }_0-12{\tilde{B}}_1{\tilde{C}}_0^2{z}_0{\omega }_0+96{\tilde{B}}_1{\omega }_0^3\right)tsin\left({\omega }_{0}t\right).\hfill \end{array}$$

(33)

Neglecting secular terms yields

$$\begin{array}{c}{\displaystyle {\tilde{C}}_1=\frac{{\tilde{C}}_0}{2},{\omega }_0=\frac{\sqrt{8+8z_0+{\tilde{C}}_0^2{z}_0}}{2\sqrt{2}}.}\hfill \end{array}$$

(34)

Therefore, for $N_{max}=2$, the first approximation $u_1\left(t\right)$ is a linear combination of the function set

$$\{1,cos\left({\omega }_{0}t\right),sin\left({\omega }_{0}t\right),cos\left(2{\omega }_{0}t\right),sin\left(2{\omega }_{0}t\right),cos\left(3{\omega }_{0}t\right),sin\left(3{\omega }_{0}t\right)\},$$

$$u_1\left(t\right)=B_0+B_{1}cos\left({\omega }_{0}t\right)+C_{1}sin\left({\omega }_{0}t\right)+B_{2}cos\left(2{\omega }_{0}t\right)+C_{2}sin\left(2{\omega }_{0}t\right)+$$

$$+B_{3}cos\left(3{\omega }_{0}t\right)+C_{3}sin\left(3{\omega }_{0}t\right),$$

where the parameters ${\omega }_0$, $B_0$, $B_i$, $C_i$ and $i=\overline{1,3}$ depending on the ${\tilde{C}}_0$, ${\tilde{B}}_1$ and ${\tilde{C}}_1$ will be optimally identified.

In taking into account Equations (29) and (32), for $N_{max}=4$, a simple integration of Equation (13), using only one iteration $n=1$, yields

$$\begin{array}{c}{\displaystyle u_1\left(t\right)=\frac{-23040y_0-23040z_0-5760{\tilde{C}}_0^2{z}_0-360{\tilde{C}}_0^4{z}_0-11520{\tilde{C}}_0{\tilde{C}}_1{z}_0-1440{\tilde{C}}_0^3{\tilde{C}}_1{z}_0}{23040{\omega }_0^2}+}\hfill \\ +\frac{1}{23040{\omega }_0^2}\left(23040y_0+23040z_0+7680{\tilde{C}}_0^2{z}_0+360{\tilde{B}}_1{\tilde{C}}_0^2{z}_0+512{\tilde{C}}_0^4{z}_0+20{\tilde{B}}_1{\tilde{C}}_0^4{z}_0+\right.\hfill \\ +\left.15360{\tilde{C}}_0{\tilde{C}}_1{z}_0+2048{\tilde{C}}_0^3{\tilde{C}}_1{z}_0\right)cos\left({\omega }_{0}t\right)+\frac{1}{23040{\omega }_0^2}\left(-11520{\tilde{C}}_0-11520{\tilde{C}}_1-\right.\hfill \\ -11520{\tilde{C}}_0{z}_0-7680{\tilde{B}}_1{\tilde{C}}_0{z}_0-1080{\tilde{C}}_0^3{z}_0-512{\tilde{B}}_1{\tilde{C}}_0^3{z}_0-11520{\tilde{C}}_1{z}_0-3240{\tilde{C}}_0^2{\tilde{C}}_1{z}_0-\hfill \\ -\left.200{\tilde{C}}_0^4{\tilde{C}}_1{z}_0+23040x_0{\omega }_0+11520{\tilde{C}}_0{\omega }_0^2+11520{\tilde{C}}_1{\omega }_0^2\right)sin\left({\omega }_{0}t\right)+\frac{1}{23040{\omega }_0^2}\left(-1920{\tilde{C}}_0^2{z}_0-\right.\hfill \\ -\left.160{\tilde{C}}_0^4{z}_0-3840{\tilde{C}}_0{\tilde{C}}_1{z}_0-640{\tilde{C}}_0^3{\tilde{C}}_1{z}_0\right)cos\left(2{\omega }_{0}t\right)+\frac{1}{23040{\omega }_0^2}\left(3840{\tilde{B}}_1{\tilde{C}}_0{z}_0+\right.\hfill \\ +\left.320{\tilde{B}}_1{\tilde{C}}_0^3{z}_0\right)sin\left(2{\omega }_{0}t\right)+\frac{1}{23040{\omega }_0^2}\left(-360{\tilde{B}}_1{\tilde{C}}_0^2{z}_0-45/2{\tilde{B}}_1{\tilde{C}}_0^4{z}_0\right)cos\left(3{\omega }_{0}t\right)+\hfill \\ +\frac{1}{23040{\omega }_0^2}\left(-120{\tilde{C}}_0^3{z}_0-360{\tilde{C}}_0^2{\tilde{C}}_1{z}_0-75/2{\tilde{C}}_0^4{\tilde{C}}_1{z}_0\right)sin\left(3{\omega }_{0}t\right)+\frac{1}{23040{\omega }_0^2}\left(8{\tilde{C}}_0^4{z}_0+\right.\hfill \\ +\left.32{\tilde{C}}_0^3{\tilde{C}}_1{z}_0\right)cos\left(4{\omega }_{0}t\right)-\frac{32{\tilde{B}}_1{\tilde{C}}_0^3{z}_0}{23040{\omega }_0^2}sin\left(4{\omega }_{0}t\right)+\frac{5/2{\tilde{B}}_1{\tilde{C}}_0^4{z}_0}{23040{\omega }_0^2}cos\left(5{\omega }_{0}t\right)+\hfill \\ +\frac{5/2{\tilde{C}}_0^4{\tilde{C}}_1{z}_0}{23040{\omega }_0^2}sin\left(5{\omega }_{0}t\right)+\frac{1}{23040{\omega }_0^2}\left(11520{\tilde{C}}_0{\omega }_0+11520{\tilde{C}}_1{\omega }_0+11520{\tilde{C}}_0{z}_0{\omega }_0+\right.\hfill \\ +1440{\tilde{C}}_0^3{z}_0{\omega }_0+11520{\tilde{C}}_1{z}_0{\omega }_0+4320{\tilde{C}}_0^2{\tilde{C}}_1{z}_0{\omega }_0+300{\tilde{C}}_0^4{\tilde{C}}_1{z}_0{\omega }_0-11520{\tilde{C}}_0{\omega }_0^3-\hfill \\ -\left.11520{\tilde{C}}_1{\omega }_0^3\right)tcos\left({\omega }_{0}t\right)+\frac{1}{23040{\omega }_0^2}\left(-11520{\tilde{B}}_1{\omega }_0-11520{\tilde{B}}_1{z}_0{\omega }_0-\right.\hfill \\ -\left.1440{\tilde{B}}_1{\tilde{C}}_0^2{z}_0{\omega }_0-60{\tilde{B}}_1{\tilde{C}}_0^4{z}_0{\omega }_0+11520{\tilde{B}}_1{\omega }_0^3\right)tsin\left({\omega }_{0}t\right).\hfill \end{array}$$

(35)

Neglecting secular terms yields

$$\begin{array}{c}{\displaystyle {\tilde{C}}_1=\frac{{\tilde{C}}_0^3}{4\left(12+{\tilde{C}}_0^2\right)},{\omega }_0=\frac{\sqrt{192+192z_0+24{\tilde{C}}_0^2{z}_0+{\tilde{C}}_0^4{z}_0}}{8\sqrt{3}},}\hfill \end{array}$$

(36)

and so on.

Therefore, for $N_{max}=4$, the first approximation $u_1\left(t\right)$ is a linear combination of the function set

$$\{1,cos\left({\omega }_{0}t\right),sin\left({\omega }_{0}t\right),cos\left(2{\omega }_{0}t\right),sin\left(2{\omega }_{0}t\right),\dots ,cos\left(5{\omega }_{0}t\right),sin\left(5{\omega }_{0}t\right)\},$$

$$u_1\left(t\right)=B_0+B_{1}cos\left({\omega }_{0}t\right)+C_{1}sin\left({\omega }_{0}t\right)+B_{2}cos\left(2{\omega }_{0}t\right)+C_{2}sin\left(2{\omega }_{0}t\right)+$$

$$+B_{3}cos\left(3{\omega }_{0}t\right)+C_{3}sin\left(3{\omega }_{0}t\right)+B_{4}cos\left(4{\omega }_{0}t\right)+C_{4}sin\left(4{\omega }_{0}t\right)+B_{5}cos\left(5{\omega }_{0}t\right)+C_{5}sin\left(5{\omega }_{0}t\right),$$

where the parameters ${\omega }_0$, $B_0$, $B_i$, $C_i$ and $i=\overline{1,5}$ depending on the ${\tilde{C}}_0$, ${\tilde{B}}_1$ and ${\tilde{C}}_1$ will be optimally identified.

Generally, for a fixed number $N_{max}\in {\mathbb{N}}^{*}$, the first approximation $u_1\left(t\right)$ is a linear combination of the function set

$$\{1,cos\left(i{\omega }_{0}t\right),sin\left(i{\omega }_{0}t\right),i=1,2,\dots ,N_{max}+1\},$$

$$\begin{array}{c}{\displaystyle u_1\left(t\right)=B_0+\sum _{i=1}^{N_{max}+1}{B}_{i}cos\left(i{\omega }_{0}t\right)+C_{i}sin\left(i{\omega }_{0}t\right),}\hfill \end{array}$$

(37)

where the parameters ${\omega }_0$, $B_0$, $B_i$, $C_i$ and $i=\overline{1,N_{max}+1}$ depending on the ${\tilde{C}}_0$, ${\tilde{B}}_1$ and ${\tilde{C}}_1$ will be optimally identified.

Thus, in using only one iteration, the OPIM solution is well determined as ${\overline{u}}_{OPIM}\left(t\right)=u_1\left(t\right)$ by Equation (37).

Furthermore, using two iterations, the OPIM solution is computed as ${\overline{u}}_{OPIM}\left(t\right)=u_2\left(t\right)$ using Equation (37), and so on.

The initial conditions given by Equation (8) yield

$$\begin{array}{c}{\displaystyle B_0=-\sum _{i=1}^{N_{max}+1}{B}_i,{\omega }_0=\frac{x_0}{{\displaystyle \sum _{i=1}^{N_{max}+1}}i{C}_i}.}\hfill \end{array}$$

(38)

4. Numerical Results and Validation

The R$\ddot{\mathrm{o}}$ssler-type system studied admits periodic solutions independently of the initial conditions, as it was proved in [2].

The results of the numerical study are provided in this section.

Firstly, the absolute errors ${ϵ}_u=|u_{numerical}-{\overline{u}}_{OPIM}|$ are examined for different values of the $N_{max}$ index in Table 1 and Figure 1.

Table 1. Values of the absolute errors: ${ϵ}_u=|u_{numerical}-{\overline{u}}_{OPIM}|$ for initial conditions $x_0=0.25$, $y_0=0.75$ and $z_0=1.05$ and different values of the index $N_{max}$; ${\overline{u}}_{OPIM}$ analytic approximate solution of Equation (8) obtained from Equations (37), (38) and (A1)–(A4).

t	${\mathit{N}}_{\mathit{max}}=16$	${\mathit{N}}_{\mathit{max}}=20$	${\mathit{N}}_{\mathit{max}}=24$	${\mathit{N}}_{\mathit{max}}=30$
0	5.68434 × ${10}^{-13}$	8.12291 $\times {10}^{-22}$	4.54747 $\times {10}^{-13}$	7.10543 $\times {10}^{-15}$
1/2	1.56141 $\times {10}^{-5}$	8.68649 $\times {10}^{-6}$	2.21405 $\times {10}^{-6}$	4.85037 $\times {10}^{-7}$
1	1.48405 $\times {10}^{-5}$	1.41248 $\times {10}^{-7}$	2.6783 $\times {10}^{-6}$	5.0592 $\times {10}^{-7}$
3/2	6.04191 $\times {10}^{-5}$	3.44 $\times {10}^{-6}$	1.44322 $\times {10}^{-6}$	6.4853 $\times {10}^{-7}$
2	3.3371 $\times {10}^{-7}$	9.82754 $\times {10}^{-6}$	3.82636 $\times {10}^{-7}$	5.22813 $\times {10}^{-7}$
5/2	6.32447 $\times {10}^{-5}$	3.4972 $\times {10}^{-6}$	3.48186 $\times {10}^{-6}$	2.96143 $\times {10}^{-7}$
3	3.62685 $\times {10}^{-5}$	1.43278 $\times {10}^{-5}$	7.30771 $\times {10}^{-7}$	7.67939 $\times {10}^{-7}$
7/2	4.35175 $\times {10}^{-5}$	4.90013 $\times {10}^{-7}$	7.79294 $\times {10}^{-7}$	7.20673 $\times {10}^{-7}$
4	4.14164 $\times {10}^{-5}$	1.68089 $\times {10}^{-5}$	1.48269 $\times {10}^{-6}$	4.27381 $\times {10}^{-7}$
9/2	3.19588 $\times {10}^{-5}$	1.68538 $\times {10}^{-5}$	2.11227 $\times {10}^{-6}$	1.03521 $\times {10}^{-6}$
5	1.32596 $\times {10}^{-4}$	1.90962 $\times {10}^{-5}$	2.91648 $\times {10}^{-6}$	9.71333 $\times {10}^{-7}$
11/2	1.90914 $\times {10}^{-5}$	3.46814 $\times {10}^{-6}$	1.51662 $\times {10}^{-6}$	4.52946 $\times {10}^{-7}$
6	1.3966 $\times {10}^{-4}$	2.01006 $\times {10}^{-5}$	3.01251 $\times {10}^{-6}$	8.10717 $\times {10}^{-7}$
13/2	3.33686 $\times {10}^{-5}$	8.48484 $\times {10}^{-7}$	4.2035 $\times {10}^{-7}$	9.86731 $\times {10}^{-7}$
7	1.15607 $\times {10}^{-4}$	1.38123 $\times {10}^{-5}$	8.79764 $\times {10}^{-8}$	7.25709 $\times {10}^{-7}$
15/2	3.18879 $\times {10}^{-5}$	9.57228 $\times {10}^{-6}$	2.69659 $\times {10}^{-6}$	1.10401 $\times {10}^{-6}$
8	5.01597 $\times {10}^{-5}$	1.00957 $\times {10}^{-5}$	5.19338 $\times {10}^{-7}$	1.25896 $\times {10}^{-6}$
17/2	6.856 $\times {10}^{-5}$	5.66887 $\times {10}^{-6}$	2.75871 $\times {10}^{-6}$	9.05699 $\times {10}^{-7}$
9	3.01073 $\times {10}^{-5}$	5.25215 $\times {10}^{-6}$	5.99213 $\times {10}^{-7}$	1.1485 $\times {10}^{-6}$
19/2	6.08924 $\times {10}^{-5}$	1.05709 $\times {10}^{-6}$	6.60261 $\times {10}^{-7}$	1.42172 $\times {10}^{-6}$
10	4.58559 $\times {10}^{-5}$	2.81366 $\times {10}^{-6}$	1.80462 $\times {10}^{-6}$	1.40391 $\times {10}^{-6}$

Figure 1. Profile of the absolute errors: ${ϵ}_u=|u_{numerical}-{\overline{u}}_{OPIM}|$ for initial conditions $x_0=0.25$, $y_0=0.75$, $z_0=1.05$ and $N_{max}=30$; the ${\overline{u}}_{OPIM}$ analytic approximate solution of Equation (8) obtained from Equations (37), (38) and (A4).

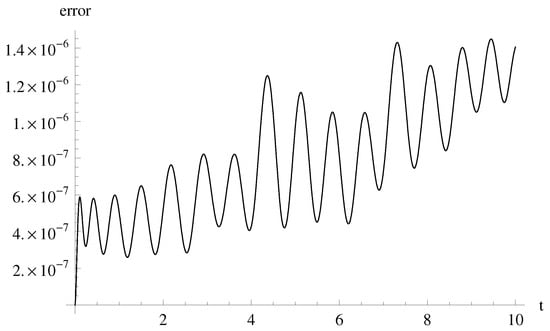
From Table 1, it can be observed that when the $N_{max}$ index increases, the magnitude of the absolute errors then decreases, until ${10}^{-7}$.

In choosing arbitrary values for the initial conditions $x_0=0.25$, $y_0=0.75$ and $z_0=1.05$, the profiles of the approximate analytic solutions ${\overline{u}}_{OPIM}$, ${\overline{x}}_{OPIM}$, ${\overline{y}}_{OPIM}$ and ${\overline{z}}_{OPIM}$ are represented in Figure 2 and Figure 3, highlighting the accuracy of the analytical procedure.

Figure 2. Profile of the approximate analytic solution ${\overline{u}}_{OPIM}$ of Equation (8) obtained from Equations (37), (38) and (A4) for initial conditions $x_0=0.25$, $y_0=0.75$, $z_0=1.05$ and $N_{max}=30$; OPIM solution (dotted line) and numerical solution (solid line).

Figure 3. Profile of the closed-form solutions ${\overline{x}}_{OPIM}$, ${\overline{y}}_{OPIM}$ and ${\overline{z}}_{OPIM}$ given by Equations (9) and (A4) for initial conditions $x_0=0.25$, $y_0=0.75$, $z_0=1.05$ and $N_{max}=30$; OPIM solution (dotted line) and numerical solution (solid line).

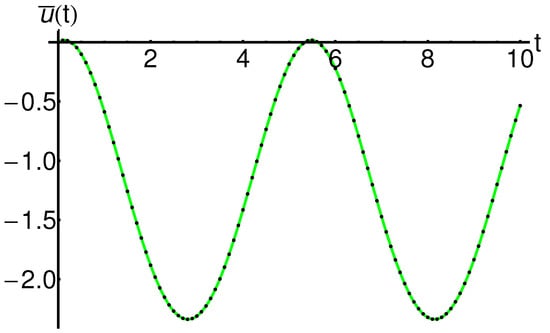
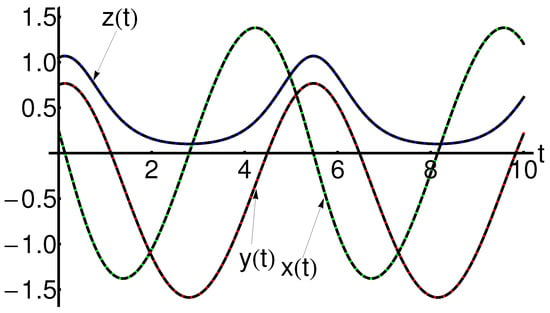
Comparisons between the semi-analytic closed-form solutions ${\overline{u}}_{OPIM}$ and ${\overline{x}}_{OPIM}$ and their corresponding numerical solutions are presented in Table 2 and Figure 2 and Table 3 and Figure 3, respectively. The precision and efficiency of the OPIM method (using just one iteration) compared to the iterative method described in [58] (using six iterations) are represented in Table 4 and Figure 4, respectively.

Table 2. The approximate analytic solution ${\overline{u}}_{OPIM}$ of Equation (8) given by Equations (37) and (38) and the corresponding numerical solution for initial conditions $x_0=0.25$, $y_0=0.75$, $z_0=1.05$ and the index $N_{max}=30$ (absolute errors: ${ϵ}_u=|u_{numerical}-{\overline{u}}_{OPIM}|$).

t	${\mathit{u}}_{\mathit{numerical}}$	${\overline{\mathit{u}}}_{\mathit{OPIM}}$	${\mathit{ϵ}}_{\mathit{u}}$
0	−8.12291 $\times {10}^{-22}$	−7.10542 $\times {10}^{-15}$	7.10542 $\times {10}^{-15}$
1/2	−0.1009833721	−0.1009828870	4.85037 $\times {10}^{-7}$
1	−0.5875537666	−0.5875532607	5.05920 $\times {10}^{-7}$
3/2	−1.2588228636	−1.2588222150	6.48530 $\times {10}^{-7}$
2	−1.8816621245	−1.8816616017	5.22813 $\times {10}^{-7}$
5/2	−2.2683521270	−2.2683518309	2.96142 $\times {10}^{-7}$
3	−2.3108289294	−2.3108281614	7.67938 $\times {10}^{-7}$
7/2	−1.9975070462	−1.9975063255	7.20673 $\times {10}^{-7}$
4	−1.4151229407	−1.4151225133	4.27380 $\times {10}^{-7}$
9/2	−0.7351867600	−0.7351857248	1.03520 $\times {10}^{-6}$
5	−0.1849885987	−0.1849876273	9.71333 $\times {10}^{-7}$

Table 3. The approximate analytic solution ${\overline{x}}_{OPIM}$ given by Equation (9) and corresponding numerical solution for initial conditions $x_0=0.25$, $y_0=0.75$, $z_0=1.05$ and the index $N_{max}=30$ (absolute errors: ${ϵ}_x=|x_{numerical}-{\overline{x}}_{OPIM}|$).

t	${\mathit{x}}_{\mathit{numerical}}$	${\overline{\mathit{x}}}_{\mathit{OPIM}}$	${\mathit{ϵ}}_{\mathit{x}}$
0	0.25	0.2499999249	7.50267 $\times {10}^{-8}$
1/2	−0.6367781227	−0.6367801438	2.02113 $\times {10}^{-6}$
1	−1.2376076804	−1.2376093968	1.71634 $\times {10}^{-6}$
3/2	−1.3673785582	−1.3673786474	8.91577 $\times {10}^{-8}$
2	−1.0603932046	−1.0603910929	2.11171 $\times {10}^{-6}$
5/2	−0.4497705143	−0.4497711768	6.62540 $\times {10}^{-7}$
3	0.2837908478	0.2837896153	1.23259 $\times {10}^{-6}$
7/2	0.9401129558	0.9401146702	1.71439 $\times {10}^{-6}$
4	1.3313637430	1.3313651699	1.42692 $\times {10}^{-6}$
9/2	1.3107276683	1.3107251137	2.55464 $\times {10}^{-6}$
5	0.8126645233	0.8126672218	2.69845 $\times {10}^{-6}$

Table 4. Values of the approximate analytical solution ${\overline{x}}_{OPIM}\left(t\right)$ (A4), the iterative solution $x_{iter}\left(t\right)$ Equation (41) and the corresponding numerical solution.

t	${\mathit{x}}_{\mathit{numerical}}$	${\overline{\mathit{x}}}_{\mathit{OPIM}}$	${\mathit{x}}_{\mathit{iter}}$
0	0.25	0.2499999249	0.25
0.35	−0.3847286943	−0.3847266999	−0.3823724677
0.7	−0.9282966040	−0.9282953550	−0.9683700083
1.05	−1.2724487484	−1.2724506529	−1.4370077000
1.4	−1.3794335466	−1.3794320704	−1.7324569333
1.75	−1.2616050449	−1.2616062739	−1.8234993489
2.1	−0.9572932068	−0.9572918609	−1.7129807750
2.45	−0.5195989669	−0.5196005038	−1.4472651645
2.8	−0.0116471526	−0.0116453617	−1.1256885333
3.15	0.4978649029	0.4978635857	−0.9100128968
3.5	0.9401129558	0.9401146702	−1.0338802083

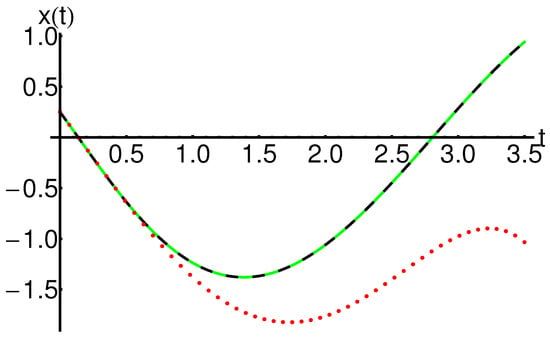
Figure 4. Profiles of the approximate analytical solution ${\overline{x}}_{OPIM}\left(t\right)$ of Equation (3) given by Equation (A4), the iterative solution $x_{iter}\left(t\right)$ given by Equation (41) and the corresponding numerical solution; OPIM solution (dashed black line), iterative solution (dotted red line) and numerical solution (solid green line).

The profiles of the approximate analytic solution ${\overline{u}}_{OPIM}$ and each of ${\overline{x}}_{OPIM}$, ${\overline{y}}_{OPIM}$ and ${\overline{z}}_{OPIM}$ have a periodic behavior, as depicted in Figure 2 and Figure 3.

The numerical values of the convergence-control parameters for ${\overline{u}}_{OPIM}$ for different values of the $N_{max}$ index are presented in detail in Appendix A.

OPIM Solutions versus Iterative Solutions

To emphasize the advantages of the presented method, the iterative solutions were obtained using the iterative method [58].

If system (3) is integrated over the interval $[0,t]$, it results in the following:

$$\begin{array}{c}{\displaystyle x\left(t\right)=x\left(0\right)+\underset{0}{\overset{t}{\int }}\left(-y\left(s\right)-z\left(s\right)\right)ds}\hfill \\ {\displaystyle y\left(t\right)=y\left(0\right)+\underset{0}{\overset{t}{\int }}x\left(s\right)ds}\hfill \\ {\displaystyle z\left(t\right)=z\left(0\right)+\underset{0}{\overset{t}{\int }}x\left(s\right)z\left(s\right)ds}\hfill \end{array}.$$

(39)

The iterative procedure leads to the following:

$$\begin{array}{c}{\displaystyle x_0\left(t\right)=x\left(0\right),x_1\left(t\right)=N_1(x_0,y_0,z_0)=\underset{0}{\overset{t}{\int }}\left(-y_0\left(s\right)-z_0\left(s\right)\right)ds,}\hfill \\ {\displaystyle y_0\left(t\right)=y\left(0\right),y_1\left(t\right)=N_2(x_0,y_0,z_0)=\underset{0}{\overset{t}{\int }}{x}_0\left(s\right)ds,}\hfill \\ {\displaystyle z_0\left(t\right)=z\left(0\right),z_1\left(t\right)=N_3(x_0,y_0,z_0)=\underset{0}{\overset{t}{\int }}{x}_0\left(s\right)z_0\left(s\right)ds,}\hfill \\ \cdots \hfill \\ x_m\left(t\right)=N_1\left({\displaystyle \sum _{i=0}^{m-1}{x}_i,\sum _{i=0}^{m-1}{y}_i,\sum _{i=0}^{m-1}{z}_i}\right)-N_1\left({\displaystyle \sum _{i=0}^{m-2}{x}_i,\sum _{i=0}^{m-2}{y}_i,\sum _{i=0}^{m-2}{z}_i}\right),\hfill \\ y_m\left(t\right)=N_2\left({\displaystyle \sum _{i=0}^{m-1}{x}_i,\sum _{i=0}^{m-1}{y}_i,\sum _{i=0}^{m-1}{z}_i}\right)-N_2\left({\displaystyle \sum _{i=0}^{m-2}{x}_i,\sum _{i=0}^{m-2}{y}_i,\sum _{i=0}^{m-2}{z}_i}\right),\hfill \\ z_m\left(t\right)=N_3\left({\displaystyle \sum _{i=0}^{m-1}{x}_i,\sum _{i=0}^{m-1}{y}_i,\sum _{i=0}^{m-1}{z}_i}\right)-N_3\left({\displaystyle \sum _{i=0}^{m-2}{x}_i,\sum _{i=0}^{m-2}{y}_i,\sum _{i=0}^{m-2}{z}_i}\right),\hfill \\ m\ge 2.\hfill \end{array}$$

(40)

The solutions of Equation (3), using the iterative algorithm, can be written as

$${\displaystyle x_{iter}\left(t\right)=\sum _{m=0}^{\infty }{x}_m\left(t\right),y_{iter}\left(t\right)=\sum _{m=0}^{\infty }{y}_m\left(t\right),z_{iter}\left(t\right)=\sum _{m=0}^{\infty }{z}_m\left(t\right),}$$

The iterative solutions $x_{iter}\left(t\right)$ become the following after five iterations, when considering the initial conditions $x\left(0\right)=0.25$, $y\left(0\right)=0.75$ and $z\left(0\right)=1.05$ (presented in Table 4) and when taking into account algorithm (40):

$$\begin{array}{c}{\displaystyle x_{iter}\left(t\right)=\sum _{m=0}^6{x}_m\left(t\right)=0.25-1.8t-0.125t^2+0.23t^3+0.0104166666t^4-0.015t^5}\hfill \\ \\ {\displaystyle y_{iter}\left(t\right)=\sum _{m=0}^6{y}_m\left(t\right)=0.75+0.25t-0.899t^2-0.04166t^3+0.07499t^4+0.0020833t^5}\hfill \\ {\displaystyle z_{iter}\left(t\right)=\sum _{m=0}^6{z}_m\left(t\right)=1.05+0.2625t-0.912187499t^2-0.27726562499t^3+}\hfill \\ +0.4637021484t^4+0.16374291992t^5-0.1719049153t^6-0.06765617675t^7+\hfill \\ +0.0480402343t^8+0.01614275537t^9-0.0062000939t^{10}-0.00178433279t^{11}+\hfill \\ +0.0002424569t^{12}+0.00006936427t^{13}+4.047309\times {10}^{-6}{t}^{14}+6.8359375\times {10}^{-8}{t}^{15}.\hfill \end{array}$$

(41)

Figure 4 and Table 4, respectively, present a parallel between the OPIM solutions ${\overline{x}}_{OPIM}$ and the corresponding iterative solutions $x_{iter}$ given in Equation (41). This comparative analysis highlights the efficiency and the accuracy of the modified OPIM method using only one iteration.

The improved precision and efficiency of the OPIM method (using just one iteration), compared to those of the iterative method described in [58] (using six iterations), are observed in the presented comparison.

The advantages of the OPIM method versus other methods are efficiency, convergence control (in the sense that the residual functions are smaller than 1), the non-existence of small parameters and the writing of the solutions in an effective form.

5. Conclusions

A new analytical approach, the OPIM, for solving second-order nonlinear differential equations was developed, which uses only one iteration.

The analytical approximate solutions were built for a class of nonlinear dynamical systems that possess a Hamilton–Poisson structure.

The obtained results were validated by graphically comparing them with the corresponding numerical solutions.

The accuracy of the results is illustrated through graphical and tabular representations.

These comparisons prove the precision of the applied method in the sense that the analytical solutions are approaching the exact solution.

The achieved results have high potential, and they encourage the study of dynamical systems with similar properties.