The Stability of Solutions of the Variable-Order Fractional Optimal Control Model for the COVID-19 Epidemic in Discrete Time

Abstract

This article introduces a discrete-time fractional variable order over a SEIQR model, incorporated for COVID-19. Initially, we establish the well-possedness of solution. Further, the disease-free and the endemic equilibrium points are determined. Moreover, the local asymptotic stability of the model is analyzed. We develop a novel discrete fractional optimal control problem tailored for COVID-19, utilizing a discrete mathematical model featuring a variable order fractional derivative. Finally, we validate the reliability of these analytical findings through numerical simulations and offer insights from a biological perspective.

1. Introduction

COVID-19, first identified in Wuhan, the capital of Hubei Province, China, in 2019 [1], is an acute respiratory disease. Since 2002, severe acute respiratory syndrome (SARS-CoV) and Middle East respiratory syndrome (MERS-CoV) have been responsible for outbreaks in humans, despite primarily infecting animals [2]. As per the International Committee on Taxonomy of Viruses (ICTV), coronaviruses are classified within the sub-family Coronavirinae, which is a part of the family Coronaviridae and the order Nidovirales. The sub-family Coronavirinae encompasses four biological groups: $\alpha ,\beta ,\gamma$, and $\delta$-coronaviruses [3,4]. Studies indicate that all coronaviruses have their origins in animals [3,5]. Moreover, recent research findings [6] suggest that although the precise origin of SARS-CoV-2 cannot be definitively determined, the potential for laboratory origin cannot be easily ruled out. $\alpha$-coronaviruses, like HumanCoV-NL63 and HumanCoV-229E, usually result in mild infections in humans. However, SADS-CoV (Swine acute diarrhea syndrome coronavirus), which utilizes swines as intermediate carriers, does not induce infectious symptoms in humans. While both HCoV-OC43 and HCoV-HKU1 belong to the $\beta$-coronavirus category, they typically pose no serious threat to humans [7]. However, the perception of highly pathogenic coronaviruses changed significantly following the outbreaks of SARS-CoV (severe acute respiratory syndrome coronavirus) in 2003 and MERS-CoV (Middle East respiratory syndrome coronavirus) in 2012 [7].

Mathematical models that depict infectious diseases are pivotal in both theoretical understanding and practical application [8,9,10,11,12,13]. Developing and scrutinizing models of this nature aids in comprehending the mechanisms of transmission and disease characteristics. This understanding facilitates the formulation of effective strategies for prediction, prevention, and control, ultimately safeguarding population health. To date, numerous mathematical models for infectious diseases, formulated using differential equations, have been constructed and analyzed to study the virus spread [12,13,14,15]. Recently, mathematical models for the COVID-19 epidemic have attracted considerable interest from mathematicians, biologists, epidemiologists, pharmacists, and chemists, producing noteworthy and vital outcomes [15,16,17,18,19,20]. Furthermore, these investigations have extended to encompass fractional-order models, as evidenced by studies like [21,22,23].

Recent research has extensively explored optimal control strategies for managing COVID-19 and its co-infections [24,25,26,27,28,29,30]. Fractional variable-order optimal control problems (V-FOCPs) have been formulated using various definitions of fractional derivatives, such as Riemann–Liouville and Caputo derivatives, with illustrative examples provided in [31,32,33]. Moreover, the discrete-time fractional optimal control model has been investigated in studies like [34,35,36]. Additionally, research has delved into optimal control problems for variable fractional systems [32].

Model Formulation

We shall present a detailed proposal for the discrete-time variable-order fractional COVID-19 system. Motivated by [37,38], we introduce the following notation: Let $S\left(t\right)$, $E\left(t\right)$, $I\left(t\right)$, $Q\left(t\right)$, and $R\left(t\right)$ denote the susceptible, exposed, infected, isolated, and removed populations at time $t\ge 0$, respectively. The total population is represented by $N\left(t\right)=S\left(t\right)+E\left(t\right)+I\left(t\right)+Q\left(t\right)+R\left(t\right)$. All parameters $(\chi ,\beta ,\nu ,\rho ,\lambda ,\delta ,\mu ,\gamma ,\psi ,\varphi ,{\phi }_1,\tau ,{\phi }_2)$ are positive real numbers which are provided in Table 1. Here, $\chi$ represent the recruitment rate. The proposed model is presented as follows:

$$\begin{array}{ccc}\hfill {\Delta }^{\alpha \left(t\right)}S\left(t\right)& =& \chi -\beta \nu {\displaystyle \frac{SI}{N+\rho I}}+\lambda R\left(t\right)-(\delta +\mu )S\left(t\right),\hfill \\ \hfill {\Delta }^{\alpha \left(t\right)}E\left(t\right)& =& \beta \nu {\displaystyle \frac{SI}{N+\rho I}}-(\gamma +\mu )E\left(t\right),\hfill \\ \hfill {\Delta }^{\alpha \left(t\right)}I\left(t\right)& =& \gamma E\left(t\right)-(\psi +\varphi +{\phi }_1+\mu )I\left(t\right),\hfill \\ \hfill {\Delta }^{\alpha \left(t\right)}Q\left(t\right)& =& \psi I\left(t\right)-(\tau +{\phi }_2+\mu )Q\left(t\right),\hfill \\ \hfill {\Delta }^{\alpha \left(t\right)}R\left(t\right)& =& \delta S\left(t\right)+\varphi I\left(t\right)+\tau Q\left(t\right)-(\lambda +\mu )R\left(t\right),\hfill \end{array}$$

(1)

with the initial conditions

$$S\left(0\right)=S_0,E\left(0\right)=E_0,I\left(0\right)=I_0,Q\left(0\right)=Q_0,R\left(0\right)=R_0.$$

Table 1. Description of the model parameters.

Parameters	Description	Value	Reference
$\chi$	Recruitment rate	assumed	–
$\rho$	Saturation factor	assumed	–
$\nu$	Contact rate	9.0631	[38]
$\beta$	The transmission probabilities	0.2761	[38]
$\delta$	Self protection rate	0.0439	[38]
$\lambda$	Transmission rate from temporarily removed to susceptible population	0.0028	[38]
$\gamma$	Rate of progression from exposed group to the infected group	0.1736	[38]
$\psi$	Isolation rate	0.516	[38]
${\phi }_1$	Death rate in infected group caused by COVID-19	0.018	[38]
${\phi }_2$	Death rate in isolated group caused by COVID-19	$3.7559\times {10}^{-5}$	[38]
$\tau$	Recovery rate	0.0534	[38]
$\varphi$	Self recovery rate	$6.1462\times {10}^{-6}$	[38]
$\mu$	Natural death rate	$3.2811\times {10}^{-5}$	[38]

Here, the delta variable-order fractional difference of model (1) is given in sense of Caputo where $\alpha \left(t\right)\in (0,1).$

This paper is organized as follows. In Section 2, we provide definitions of variable-order fractional calculus in discrete-time along with some important auxiliaries related to VOFDD. Section 3 discusses the existence and uniqueness conditions of solutions and presents stability theorems for equilibrium points. Optimal control analysis is covered in Section 4. Section 5 outlines the used numerical scheme. Section 6 presents numerical simulations and results. Finally, Section 7 concludes our contribution.

2. Preliminaries

In this context, we introduce certain definitions and notations referenced from the papers [39,40]. Let ${\mathbb{N}}_a$ denote the set $\{a,a+1,a+2,\dots \}$ and ${\mathbb{N}}_a^T$ represent the set $\{a,a+1,a+2,\dots ,T\}$.

Definition 1. 

Let $\alpha \left(t\right)>0$ and $\sigma \left(s\right)=s+1$. For $u\left(t\right)$ defined on ${\mathbb{N}}_a$, the delta variable-order fractional sum of order $\alpha \left(t\right)$ is defined by

$$\begin{array}{c}\hfill {\Delta }_a^{-\alpha \left(t\right)}u\left(t\right)=\frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}\sum _{s=a}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}u\left(s\right),\end{array}$$

(2)

where $t^{\left(\alpha \right(t\left)\right)}$ is the discrete factorial functional given by $t^{\left(\alpha \right(t\left)\right)}=\frac{\mathsf{\Gamma }(t+1)}{\mathsf{\Gamma }(t-\alpha (t)+1)}$.

Definition 2. 

For $u\left(t\right)$ defined on defined on ${\mathbb{N}}_a,\alpha \left(t\right)>0,\alpha \notin \mathbb{N}$, the delta Caputo variable-order fractional difference is defined by

$$\begin{array}{c}\hfill {}^C\Delta _a^{\alpha \left(t\right)}u\left(t\right)={\Delta }_a^{-(m-\alpha (t\left)\right)}{\Delta }^{m}u\left(t\right)=\frac{1}{\mathsf{\Gamma }(m-\alpha (t\left)\right)}\sum _{s=a}^{t-(m-\alpha (t\left)\right)}{(t-\sigma \left(s\right))}^{(m-\alpha (t)-1)}{\Delta }^{m}u\left(s\right),\end{array}$$

(3)

where $t\in {\mathbb{N}}_{a+m-\alpha \left(t\right)},m=\left[\alpha \left(t\right)\right]+1.$ Note that the forward difference operator is defined by $\Delta u\left(t\right)=u(t+1)-u\left(t\right)$.

Theorem 1 

([41]). Let $s\in {\mathbb{N}}_{a+1}$, then the following hold

$$\sum _{k=a+1}^s{(s-k+1)}^{\alpha \left(s\right)-1}={\displaystyle \frac{{(s-a)}^{\alpha \left(s\right)}}{\alpha \left(s\right)}}.$$

(4)

Theorem 2 

([42,43]). Consider the following fractional variable-order discrete system

$${\Delta }^{\alpha \left(t\right)}x=f\left(x\right),x\left(0\right)=x_0,$$

(5)

with $x\in {\mathbb{R}}^n,\underline{\alpha }=\inf \alpha \left(t\right)$ and $\overline{\alpha }=\sup \alpha \left(t\right)$ where $0<\underline{\alpha }<\alpha \left(t\right)<\overline{\alpha }<1$. The equilibrium points of the system (5) are solutions to the equation $f\left(x\right)=0$.

An equilibrium is locally asymptotically stable if all the eigenvalues ${\lambda }_i$$(i=1,2,\dots ,n)$ of the Jacobian matrix $J=\Delta f$ evaluated at the equilibrium satisfy

$$|arg\left({\lambda }_i\right)|<{\displaystyle \frac{\pi }{2}}\underline{\alpha }.$$

(6)

On the other hand, if $|arg\left({\lambda }_i\right)|>{\displaystyle \frac{\pi }{2}}\overline{\alpha },$ then the equilibrium point is unstable.

Theorem 3 

([44]). Consider the polynomial equation

$$p\left(\lambda \right)={\lambda }^2+a_1\lambda +a_2.$$

1. 

For $n=1,$ the condition for stability is $a_1>0$.

2 

For $n=2,$ the condition for stability either Routh–Hurwitz conditions [45] $(a_1>0,a_2>0)$ or $a_1<0$, $4a_2>a_1^2$, ${tan}^{-1}(4a_2-a_1^2)>{\displaystyle \frac{\pi }{2}}\overline{\alpha }.$

Definition 3 

([46]). Given a system of characteristic equations in the form of n-order polynomials as follows

$$f\left(z\right)=d_1{z}^n+d_1{z}^{n-1}+\cdots +d_{n-1}z+d_n.$$

(7)

If all the real parts of equation from the root are negative, then

$$\frac{d_1}{d_0}>0,\frac{d_2}{d_0}>0,\cdots ,\frac{d_n}{d_0}>0.$$

(8)

Suppose $d_k$ are real numbers for $k=0,1,2,\cdots ,2n-1$ and $d_k$ are positive numbers. The Hurwitz matrix for Equation (7) is defined as a square matrix of size $n\times n$ as follows:

$$H_n=\left(\begin{array}{cccccc}{d}_1& d_3& d_5& d_7& \cdots & d_{2n-1}\\ d_0& d_2& d_4& d_6& \cdots & d_{2n-2}\\ 0& d_1& d_3& d_5& \cdots & d_{2n-3}\\ 0& d_0& d_2& d_4& \cdots & d_{2n-4}\\ ⋮& ⋮& ⋮& ⋮& ⋮& ⋮\\ 0& 0& 0& 0& \cdots & d_n\end{array}\right),$$

(9)

where $d_k=0$ for $k<0$ or $k>n.$ Therefore, the matrix element index is greater than n, or the negative index must be replaced by zero. The k-level Hurwitz determinant, denoted by $det{H}_k;k=1,2,\cdots ,n$, formed from the Hurwitz matrix (9), is defined as follows:

$$det{H}_n=\left|\begin{array}{cccccc}{d}_1& d_3& d_5& d_7& \cdots & d_{2}n-1\\ d_0& d_2& d_4{d}_6& & \cdots & d_{2}n-2\\ 0& d_1& d_3& d_5& \cdots & d_{2}n-3\\ 0& d_0& d_2& d_4& \cdots & d_{2}n-4\\ ⋮& ⋮& ⋮& ⋮& ⋮& ⋮\\ 0& 0& 0& 0& \cdots & d_n\end{array}\right|$$

Theorem 4 

([46]). The polynomial root (7) has a real part of its root that is negative if and only if the inequality (3) is fulfilled and

$$det{H}_1>0;det{H}_2>0;det{H}_3>0;\cdots ;det{H}_n>0.$$

(10)

Thus, the equilibrium point $\overline{z}$ is stable if and only if $det{H}_j>0$ for each $j=1,2,\cdots ,n$.

3. Properties of Solution

3.1. Non-Negativity and Boundedness of the Solutions

In this subsection, we discuss some properties related to the non negativity and boundedness of the solution of model (1). To this aim, we show the following result.

Theorem 5. 

All solutions of model (1) are non negative for any initial value $\left(S\right(0)$, $E\left(0\right)$, $I\left(0\right)$, $Q\left(0\right)$, $R\left(0\right))\in (0,0,0,0,0)\cup {\mathbb{R}}_{+}^5$ and the feasible region of model (1) is defined by $N=\{(S\left(t\right),E\left(t\right),I\left(t\right),Q\left(t\right),R\left(t\right))\in {\mathbb{R}}_{+}^5;0<S\left(t\right)+E\left(t\right)+I\left(t\right)+Q\left(t\right)+R\left(t\right)<{\displaystyle \frac{\chi }{\mu }}\}.$

Proof. 

Suppose a general fractional variable-order discrete time model of system (1) as

$$\begin{array}{ccc}\hfill {\Delta }^{\alpha \left(t\right)}{S\left(t\right)|}_{S=0}& =& \chi +\lambda R\left(t\right),\hfill \\ \hfill {\Delta }^{\alpha \left(t\right)}{E\left(t\right)|}_{E=0}& =& \beta \nu {\displaystyle \frac{SI}{N+\rho I}},\hfill \\ \hfill {\Delta }^{\alpha \left(t\right)}{I\left(t\right)|}_{I=0}& =& \gamma E\left(t\right),\hfill \\ \hfill {\Delta }^{\alpha \left(t\right)}{Q\left(t\right)|}_{Q=0}& =& \psi I\left(t\right),\hfill \\ \hfill {\Delta }^{\alpha \left(t\right)}{R\left(t\right)|}_{R=0}& =& \delta S\left(t\right)+\varphi I\left(t\right)+\tau Q\left(t\right).\hfill \end{array}$$

From the above results, it easy to deduce that the solutions $S\left(t\right),E\left(t\right),I\left(t\right),Q\left(t\right)$ and $R\left(t\right)$ are positive. Next, we have to show that the boundedness of the solution of model (1). We have,

$$\begin{array}{c}\hfill {\Delta }^{\alpha \left(t\right)}N\left(t\right)=\chi -\mu N\left(t\right)-{\phi }_{1}I\left(t\right)-{\phi }_{2}Q\left(t\right)<\chi -\mu N\left(t\right).\end{array}$$

According on the fractional order comparison Theorem in [47], we obtain

$$\begin{array}{c}\hfill N\left(t\right)<{\displaystyle \frac{\chi }{\mu }}+\left(N\left(0\right)-{\displaystyle \frac{\chi }{\mu }}\right)E_{\alpha \left(t\right),1}(-\mu t^{\alpha \left(t\right)})<{\displaystyle \frac{\chi }{\mu }},\end{array}$$

where $N\left(0\right)<{\displaystyle \frac{\chi }{\mu }}.$ □

3.2. Equilibrium Points and Basic Reproduction Number

First, to discover equilibria of the model (1) where $X={(S,E,I,Q,R)}^T$, we set

$${\Delta }^{\alpha \left(t\right)}X\left(t\right)=0.$$

(11)

We obtain the following algebraic system:

$$\begin{array}{ccc}\hfill \chi -\beta \nu {\displaystyle \frac{SI}{N+\rho I}}+\lambda R\left(t\right)-(\delta +\mu )S\left(t\right)& =& 0,\hfill \\ \hfill \beta \nu {\displaystyle \frac{SI}{N+\rho I}}-(\gamma +\mu )E\left(t\right)& =& 0,\hfill \\ \hfill \gamma E\left(t\right)-(\psi +\varphi +{\phi }_1+\mu )I\left(t\right)& =& 0,\hfill \\ \hfill \psi I\left(t\right)-(\tau +{\phi }_2+\mu )Q\left(t\right)& =& 0,\hfill \\ \hfill \delta S\left(t\right)+\varphi I\left(t\right)+\tau Q\left(t\right)-(\lambda +\mu )R\left(t\right)& =& 0.\hfill \end{array}$$

(12)

Using some algebraic calculations, we find two solutions of system (12). We have a disease-free equilibrium point noted by $P_0=(S_0,0,0,0,R_0),$ and an endemic equilibrium point denoted as $P_{*}=\left(S_{*},E_{*},I_{*},Q_{*},R_{*}\right).$ Here,

$$S_0=\frac{\chi (\lambda +\mu )}{\mu \left(\lambda +\delta +\mu \right)};R_0=\frac{\chi \delta }{\mu \left(\lambda +\delta +\mu \right)}.$$

More detailed discussion about an endemic equilibrium point are provided later. Now, we identify the basic reproduction number of the model (1) denoted by $R_0$ using the spectral radius of the next generation matrix as in [48].

Let $Y={(E,I,Q)}^T$. We have

$$\begin{array}{c}\hfill {\Delta }^{\alpha \left(t\right)}Y=\mathcal{F}\left(Y\right)-\mathcal{V}\left(Y\right)\end{array}$$

where

$$\mathcal{F}=\left(\begin{array}{c}\left(\beta \nu {\displaystyle \frac{SI}{N+\rho I}}\right)\\ 0\\ 0\end{array}\right.,\mathcal{V}=\left(\begin{array}{c}\left(\gamma +\mu \right)E\\ -\gamma E+\left(\psi +\varphi +{\phi }_1+\mu \right)I\\ -\psi I+\left(\tau +{\phi }_2+\mu \right)Q\end{array}\right).$$

The Jacobi matrix of the above at the disease-free state $P_0$ is given by

$$\mathbf{F}=\left(\begin{array}{ccc}0& {\displaystyle \frac{\beta \nu S_0}{N}}& 0\\ 0& 0& 0\\ 0& 0& 0\end{array}\right),\mathbf{V}=\left(\begin{array}{ccc}\gamma +\mu & 0& 0\\ -\gamma & \psi +\varphi +{\phi }_1+\mu & 0\\ 0& -\psi & \tau +{\phi }_2+\mu \end{array}\right).$$

Hence, the spectral radius is denoted by $\varsigma .$

$${\mathcal{R}}_0=\varsigma \left(F{V}^{-1}\right)=\frac{\beta \nu \gamma S_0}{N\left(\gamma +\mu \right)\left(\psi +\varphi +{\phi }_1+\mu \right)}.$$

Theorem 6. 

The proposed variable-order fractional model (1) has a unique disease endemic equilibrium point $P_{*}=\left(S_{*},E_{*},I_{*},Q_{*},R_{*}\right)$ if and only if ${\mathcal{R}}_0>1.$

Proof. 

We are able to obtain the endemic equilibrium $P_{*}=\left(S_{*},E_{*},I_{*},Q_{*},R_{*}\right)$, and it is written as

$$I_{*}=q_1{E}_{*};I{Q}_{*}=q_2{I}_{*};R_{*}=\frac{\left(\delta +\mu \right)S_{*}+\left(\gamma +\mu \right)E_{*}-\chi }{\lambda };S_{*}=\frac{\chi \left(\lambda +\mu \right)-\left(B_1-B_2\right)E_{*}}{\mu \left(\lambda +\delta +\mu \right)},$$

(13)

where

$$q_1=\frac{\gamma }{\psi +\varphi +{\phi }_1+\mu };q_2=\frac{\psi }{\tau +{\phi }_2+\mu };B_1=\left(\lambda +\mu \right)\left(\gamma +\mu \right);B_2=\lambda \varphi q_1+\lambda \tau q_1{q}_2.$$

(14)

We can obtain that

$$B_1-B_2=\frac{\left(\lambda +\mu \right)\left(\gamma +\mu \right)\left(\psi +\varphi +{\phi }_1+\mu \right)\left(\tau +{\phi }_2+\mu \right)-\lambda \varphi \gamma \left(\tau +{\phi }_2+\mu \right)-\lambda \tau \gamma \psi }{\left(\psi +\varphi +{\phi }_1+\mu \right)\left(\tau +{\phi }_2+\mu \right)}>0.$$

Further, from the above equations, we have

$$\beta \nu \frac{\left(\chi \left(\lambda +\mu \right)+\left(B_2-B_1\right)x\right)q_1{E}_{*}}{\mu \left(\lambda +\delta +\mu \right)\left(N+\rho q_1{E}_{*}\right)}=\left(\gamma +\mu \right)E_{*}.$$

Define

$$g\left(x\right)=\beta \nu \frac{\left(\chi \left(\lambda +\mu \right)+\left(B_2-B_1\right)x\right)q_{1}x}{\mu \left(\lambda +\delta +\mu \right)\left(N+\rho q_{1}x\right)}-\left(\gamma +\mu \right)x,$$

(15)

with $g\left(x\right)=0$, at $x\in \left[{\displaystyle 0,\frac{\chi \left(\lambda +\mu \right)}{B_1-B_2}}\right]$ where

$$\beta \nu \frac{\left(\chi \left(\lambda +\mu \right)+\left(B_2-B_1\right)x\right)q_{1}x}{\mu \left(\lambda +\delta +\mu \right)\left(N+\rho q_{1}x\right)}-\left(\gamma +\mu \right)x=0,$$

and $g\left(0\right)=0$, $g\left({\displaystyle \frac{\chi \left(\lambda +\mu \right)}{B_1-B_2}}\right)=\frac{\chi \left(\lambda +\mu \right)\left(\gamma +\mu \right)}{B_1-B_2}<0.$ Now,

$$\begin{array}{ccc}& & {\displaystyle g^{\prime }\left(x\right)=\frac{-\beta \nu \left(B_2-B_1\right)q_{1}x}{\mu \left(\lambda +\delta +\mu \right)\left(N+\rho q_{1}x\right)}+q_1\beta \nu \frac{SN}{{\left(N+\rho q_{1}x\right)}^2}-\left(\gamma +\mu \right),}\hfill \\ & & {\displaystyle g^{\prime }\left(0\right)=q_1\beta \nu \frac{S_{*}}{N}-\left(\gamma +\mu \right)=\left(\gamma +\mu \right)\left({\mathcal{R}}_0-1\right).}\hfill \end{array}$$

Here,

$${\mathcal{R}}_0=\frac{\beta \nu \gamma S_0}{N\left(\gamma +\mu \right)\left(\psi +\varphi +{\phi }_1+\mu \right)}.$$

Since $g\left(x\right)$ is a continuous differentiable function $x\in \left[{\displaystyle 0,\frac{\chi \left(\lambda +\mu \right)}{B_1-B_2}}\right].$ That $g\left(x\right)=0$ has a positive solution $E_{*}.$ If $g\left(x\right)>0$ ( ${\mathcal{R}}_0>1$) is proven. □

3.3. Existence and Uniqueness (E&U) of the Solution

In this subsection, we prove the existence and uniqueness of the solutions of our problem (1). The kernels $H_1,H_2,H_3,H_3,H_4$ and $H_5$ are defined by

$$\begin{array}{ccc}\hfill H_1(t,S\left(t\right))& =& \chi -\beta \nu {\displaystyle \frac{SI}{N+\rho I}}+\lambda R\left(t\right)-(\delta +\mu )S\left(t\right),\hfill \\ \hfill H_2(t,E\left(t\right))& =& \beta \nu {\displaystyle \frac{SI}{N+\rho I}}-(\gamma +\mu )E\left(t\right),\hfill \\ \hfill H_3(t,I\left(t\right))& =& \gamma E\left(t\right)-(\psi +\varphi +{\phi }_1+\mu )I\left(t\right),\hfill \\ \hfill H_4(t,Q\left(t\right))& =& \psi I\left(t\right)-(\tau +{\phi }_2+\mu )Q\left(t\right),\hfill \\ \hfill H_5(t,R\left(t\right))& =& \delta S\left(t\right)+\varphi I\left(t\right)+\tau Q\left(t\right)-(\lambda +\mu )R\left(t\right).\hfill \end{array}$$

Theorem 7. 

The Kernels $H_1,H_2,H_3,H_4$ and $H_5$ have the Lipschitz condition.

Proof. 

Depending on the fractional discrete variable-order calculus properties, a solution of (1) is given by

$$\begin{array}{ccc}\hfill S(t)& =& S(0)+{\Delta }^{-\alpha (t)}(\chi -\beta \nu {\displaystyle \frac{SI}{N+\rho I}}+\lambda R(t)-(\delta +\mu )S(t)),\hfill \\ \hfill E(t)& =& E(0)+{\Delta }^{-\alpha (t)}(\beta \nu {\displaystyle \frac{SI}{N+\rho I}}-(\gamma +\mu )E(t)),\hfill \\ \hfill I(t)& =& I(0)+{\Delta }^{-\alpha (t)}(\gamma E(t)-(\psi +\varphi +{\phi }_1+\mu )I(t)),\hfill \\ \hfill Q(t)& =& Q(0)+{\Delta }^{-\alpha (t)}(\psi I(t)-(\tau +{\phi }_2+\mu )Q(t)),\hfill \\ \hfill R(t)& =& R(0)+{\Delta }^{-\alpha (t)}(\delta S(t)+\varphi I(t)+\tau Q(t)-(\lambda +\mu )R(t)).\hfill \end{array}$$

(16)

By definition,

$$\begin{array}{ccc}\hfill S\left(t\right)& =& S\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(\chi -\beta \nu {\displaystyle \frac{SI}{N+\rho I}}+\lambda R\left(t\right)-(\delta +\mu )S\left(t\right)),\hfill \\ \hfill E\left(t\right)& =& E\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(\beta \nu {\displaystyle \frac{SI}{N+\rho I}}-(\gamma +\mu )E\left(t\right)),\hfill \\ \hfill I\left(t\right)& =& I\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(\gamma E\left(t\right)-(\psi +\varphi +{\phi }_1+\mu )I\left(t\right)),\hfill \\ \hfill Q\left(t\right)& =& Q\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(\psi I\left(t\right)-(\tau +{\phi }_2+\mu )Q\left(t\right)),\hfill \\ \hfill R\left(t\right)& =& R\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(\delta S\left(t\right)+\varphi I\left(t\right)+\tau Q\left(t\right)-(\lambda +\mu )R\left(t\right)).\hfill \end{array}$$

(17)

We consider the two functions $S\left(t\right)$ and $S^{*}\left(t\right)$. We have

$$\begin{array}{ccc}\hfill \parallel H_1(t,S(t))-H_1(t,S^{*}(t))\parallel & =& ∥(\beta \nu {\displaystyle \frac{I}{N+\rho I}}+(\delta +\mu ))(S(t)-S^{*}(t))∥.\hfill \end{array}$$

(18)

Suppose that

$$p_1=\parallel \beta \nu +(\delta +\mu )\parallel .$$

If ${\displaystyle \frac{I}{N+\rho I}}<1,$ we obtain

$$\parallel H_1(t,S\left(t\right))-H_1(t,S^{*}\left(t\right))\parallel <p_1\parallel S\left(t\right)-S^{*}\left(t\right)\parallel .$$

We use similar arguments for other functions, we obtain

$$\begin{array}{ccc}\hfill \parallel H_2(t,E\left(t\right))-H_2(t,E^{*}\left(t\right))\parallel & <& p_2\parallel E\left(t\right)-E^{*}\left(t\right)\parallel ,\hfill \\ \hfill \parallel H_3(t,I\left(t\right))-H_3(t,I^{*}\left(t\right))\parallel & <& p_3\parallel I\left(t\right)-I^{*}\left(t\right)\parallel ,\hfill \\ \hfill \parallel H_4(t,Q\left(t\right))-H_4(t,Q^{*}\left(t\right))\parallel & <& p_4\parallel Q\left(t\right)-Q^{*}\left(t\right)\parallel ,\hfill \\ \hfill \parallel H_5(t,R\left(t\right))-H_5(t,R^{*}\left(t\right))\parallel & <& p_5\parallel R\left(t\right)-R^{*}\left(t\right)\parallel .\hfill \end{array}$$

(19)

The respective Lipschitz constants to the functions $H_1,H_2,H_3,H_4$ and $H_5$ are $p_1$, $p_2$, $p_3$, $p_4$ and $p_5$. Therefore, the equations in (17) become

$$\begin{array}{ccc}\hfill S\left(t\right)& =& S\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}{H}_1(s,S\left(s\right)),\hfill \\ \hfill E\left(t\right)& =& E\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}{H}_2(s,A\left(s\right)),\hfill \\ \hfill I\left(t\right)& =& I\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}{H}_3(s,M\left(s\right)),\hfill \\ \hfill Q\left(t\right)& =& Q\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}{H}_4(s,C\left(s\right)),\hfill \\ \hfill R\left(t\right)& =& R\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}{H}_5(s,R\left(s\right)).\hfill \end{array}$$

(20)

The recursive formula is presented as

$$\begin{array}{ccc}\hfill S_n\left(t\right)& =& S\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}{H}_1(s,S_{n-1}\left(s\right)),\hfill \\ \hfill E_n\left(t\right)& =& E\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}{H}_2(s,E_{n-1}\left(s\right)),\hfill \\ \hfill I_n\left(t\right)& =& I\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}{H}_3(s,I_{n-1}\left(s\right)),\hfill \\ \hfill Q_n\left(t\right)& =& Q\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}{H}_4(s,Q_{n-1}\left(s\right)),\hfill \\ \hfill R_n\left(t\right)& =& R\left(0\right)+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}{H}_5(s,R_{n-1}\left(s\right)),\hfill \end{array}$$

(21)

the initial conditions are given by $S_0\left(t\right)=S\left(0\right)$, $E_0\left(t\right)=E\left(0\right)$, $I_0\left(t\right)=I\left(0\right)$, $Q_0\left(t\right)=Q\left(0\right)$ and $R_0\left(t\right)=R\left(0\right).$ Then, we take the expressions for difference of successive terms

$${\vartheta }_{S,n}\left(t\right)=S_n\left(t\right)-S_{n-1}\left(t\right)={\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(H_1(s,S_{n-1}\left(s\right))-H_1(s,S_{n-2}\left(s\right))),$$

$${\vartheta }_{E,n}\left(t\right)=E_n\left(t\right)-E_{n-1}\left(t\right)={\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(H_2(s,E_{n-1}\left(s\right))-H_2(s,E_{n-2}\left(s\right))),$$

$${\vartheta }_{I,n}\left(t\right)=I_n\left(t\right)-I_{n-1}\left(t\right)={\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(H_3(s,I_{n-1}\left(s\right))-H_3(s,I_{n-2}\left(s\right))),$$

$${\vartheta }_{Q,n}\left(t\right)=Q_n\left(t\right)-Q_{n-1}\left(t\right)={\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(H_4(s,Q_{n-1}\left(s\right))-H_4(s,Q_{n-2}\left(s\right))),$$

$${\Phi }_{R,n}\left(t\right)=R_n\left(t\right)-R_{n-1}\left(t\right)={\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(H_5(s,R_{n-1}\left(s\right))-H_5(s,R_{n-2}\left(s\right))),$$

where

$$\begin{array}{c}\hfill S_n\left(t\right)=\sum _{j=0}^n{\vartheta }_{S,j}\left(t\right);E_n\left(t\right)=\sum _{j=0}^n{\vartheta }_{E,j}\left(t\right);I_n\left(t\right)=\sum _{j=0}^n{\vartheta }_{I,j}\left(t\right);\\ \hfill Q_n\left(t\right)=\sum _{j=0}^n{\vartheta }_{Q,j}\left(t\right);R_n\left(t\right)=\sum _{j=0}^n{\vartheta }_{R,j}\left(t\right).\end{array}$$

Considering

$$\begin{array}{ccc}\hfill {\vartheta }_{S,n-1}\left(t\right)& =& S_{n-1}\left(t\right)-S_{n-2}\left(t\right),\hfill \\ \hfill {\vartheta }_{E,n-1}\left(t\right)& =& E_{n-1}\left(t\right)-E_{n-2}\left(t\right),\hfill \\ \hfill {\vartheta }_{I,n-1}\left(t\right)& =& I_{n-1}\left(t\right)-I_{n-2}\left(t\right),\hfill \\ \hfill {\vartheta }_{Q,n-1}\left(t\right)& =& Q_{n-1}\left(t\right)-Q_{n-2}\left(t\right),\hfill \\ \hfill {\vartheta }_{R,n-1}\left(t\right)& =& R_{n-1}\left(t\right)-R_{n-2}\left(t\right),\hfill \end{array}$$

(22)

we obtain the following:

$$\begin{array}{ccc}\hfill \parallel {\vartheta }_{S,n}\left(t\right)\parallel & <& {\displaystyle \frac{p_1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel {\vartheta }_{S,n-1}\left(s\right)\parallel ,\hfill \\ \hfill \parallel {\vartheta }_{E,n}\left(t\right)\parallel & <& {\displaystyle \frac{p_2}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel {\vartheta }_{E,n-1}\left(s\right)\parallel ,\hfill \\ \hfill \parallel {\vartheta }_{I,n}\left(t\right)\parallel & <& {\displaystyle \frac{p_3}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel {\vartheta }_{I,n-1}\left(s\right)\parallel ,\hfill \\ \hfill \parallel {\vartheta }_{Q,n}\left(t\right)\parallel & <& {\displaystyle \frac{p_4}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel {\vartheta }_{Q,n-1}\left(s\right)\parallel ,\hfill \\ \hfill \parallel {\vartheta }_{R,n}\left(t\right)\parallel & <& {\displaystyle \frac{p_5}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel {\vartheta }_{R,n-1}\left(s\right)\parallel .\hfill \end{array}$$

(23)

□

Theorem 8. 

The solution of model (1) exists for $t\in [0,\mathrm{T}]$ provided

$${\displaystyle \frac{k{p}_i}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}<1,i=1,\dots ,5.$$

Proof. 

Here, the function $S\left(t\right)$, $E\left(t\right)$, $I\left(t\right)$, $Q\left(t\right)$ and $R\left(t\right)$ are bounded, and the kernels $H_1$, $H_2$, $H_3$, $H_4$ and $H_5$ satisfy the Lipschitz condition. By using the recursive principle, the inequalities (23) involve

$$\begin{array}{ccc}\hfill \parallel {\vartheta }_{S,n}\left(t\right)\parallel & <& \parallel S_0\left(t\right)\parallel {\left({\displaystyle \frac{k{p}_1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\right)}^n,\hfill \\ \hfill \parallel {\vartheta }_{E,n}\left(t\right)\parallel & <& \parallel E_0\left(t\right)\parallel {\left({\displaystyle \frac{k{p}_2}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\right)}^n,\hfill \\ \hfill \parallel {\vartheta }_{I,n}\left(t\right)\parallel & <& \parallel I_0\left(t\right)\parallel {\left({\displaystyle \frac{k{p}_3}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\right)}^n,\hfill \\ \hfill \parallel {\vartheta }_{Q,n}\left(t\right)\parallel & <& \parallel Q_0\left(t\right)\parallel {\left({\displaystyle \frac{k{p}_4}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\right)}^n,\hfill \\ \hfill \parallel {\vartheta }_{R,n}\left(t\right)\parallel & <& \parallel R_0\left(t\right)\parallel {\left({\displaystyle \frac{k{p}_5}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\right)}^n.\hfill \end{array}$$

(24)

Applying a limit, as n approaches ∞, we obtain $\parallel {\vartheta }_{.,n}\left(t\right)\parallel ⟶0$. Hence, we have the existence of the solutions of Equation (1). □

Theorem 9. 

The solution of (1) is unique if $\parallel \aleph \left(t\right)\parallel \left(1-{\displaystyle \frac{kp}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\right)>0$ holds true.

Proof. 

Assume that there exists another solution to model (1), and it is given as ($S_2\left(t\right)$, $E_2\left(t\right)$, $I_2\left(t\right)$, $Q_2\left(t\right)$, $R_2\left(t\right)$) where

$$\aleph \left(t\right)=\left(\begin{array}{c}S\left(t\right)-S_2\left(t\right)\\ E\left(t\right)-E_2\left(t\right)\\ I\left(t\right)-I_2\left(t\right)\\ Q\left(t\right)-Q_2\left(t\right)\\ R\left(t\right)-R_2\left(t\right)\end{array}\right);p=\left(\begin{array}{c}{p}_1\\ p_2\\ p_3\\ p_4\\ p_5\end{array}\right).$$

$$\begin{array}{ccc}\hfill S\left(t\right)-S_2\left(t\right)& =& {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(L_1(s,S\left(s\right))-H_1(s,S_2\left(s\right))),\hfill \\ \hfill E\left(t\right)-E_2\left(t\right)& =& {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(L_2(s,E\left(s\right))-H_2(s,E_2\left(s\right))),\hfill \\ \hfill I\left(t\right)-I_2\left(t\right)& =& {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\left(L_3(s,I_{(}s)\right)-H_3(s,I_2\left(s\right))),\hfill \\ \hfill Q\left(t\right)-Q_2\left(t\right)& =& {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(L_4(s,Q\left(s\right))-H_4(s,Q_2\left(s\right))),\hfill \\ \hfill R\left(t\right)-R_2\left(t\right)& =& {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(L_5(s,R\left(s\right))-H_5(s,R_2\left(s\right))).\hfill \end{array}$$

(25)

Applying the norm on (25), we obtain

$$\begin{array}{ccc}\hfill \parallel S\left(t\right)-S_2\left(t\right)\parallel & =& \parallel {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(H_1(s,S\left(s\right))-H_1(s,S_2\left(s\right)))\parallel ,\hfill \\ \hfill \parallel E\left(t\right)-E_2\left(t\right)\parallel & =& \parallel {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(H_2(s,E\left(s\right))-H_2(s,E_2\left(s\right)))\parallel ,\hfill \\ \hfill \parallel I\left(t\right)-I_2\left(t\right)\parallel & =& \parallel {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\left(H_3(s,I_{(}s)\right)-H_3(s,I_2\left(s\right)))\parallel ,\hfill \\ \hfill \parallel Q\left(t\right)-Q_2\left(t\right)\parallel & =& \parallel {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(H_4(s,Q\left(s\right))-H_4(s,Q_2\left(s\right)))\parallel ,\hfill \\ \hfill \parallel R\left(t\right)-R_2\left(t\right)\parallel & =& \parallel {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}(H_5(s,R\left(s\right))-H_5(s,R_2\left(s\right)))\parallel .\hfill \end{array}$$

So

$$\begin{array}{ccc}\hfill \parallel S\left(t\right)-S_2\left(t\right)\parallel & =& {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel (H_1(s,S\left(s\right))-H_1(s,S_2\left(s\right)))\parallel ,\hfill \\ \hfill \parallel E\left(t\right)-E_2\left(t\right)\parallel & =& {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel (H_2(s,E\left(s\right))-H_2(s,E_2\left(s\right)))\parallel ,\hfill \\ \hfill \parallel I\left(t\right)-I_2\left(t\right)\parallel & =& {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel \left(H_3(s,I_{(}s)\right)-H_3(s,I_2\left(s\right)))\parallel ,\hfill \\ \hfill \parallel Q\left(t\right)-Q_2\left(t\right)\parallel & =& {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel (H_4(s,Q\left(s\right))-H_4(s,Q_2\left(s\right)))\parallel ,\hfill \\ \hfill \parallel R\left(t\right)-R_2\left(t\right)\parallel & =& {\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel (H_5(s,R\left(s\right))-H_5(s,R_2\left(s\right)))\parallel .\hfill \end{array}$$

(26)

Using the Lipschitz condition,

$$\begin{array}{ccc}\hfill \parallel S\left(t\right)-S_2\left(t\right)\parallel & <& {\displaystyle \frac{p_1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel S\left(t\right)-S_2\left(t\right)\parallel ,\hfill \\ \hfill \parallel E\left(t\right)-E_2\left(t\right)\parallel & <& {\displaystyle \frac{p_2}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel E\left(t\right)-E_2\left(t\right)\parallel ,\hfill \\ \hfill \parallel I\left(t\right)-I_2\left(t\right)\parallel & <& {\displaystyle \frac{p_3}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel I\left(t\right)-I_2\left(t\right)\parallel ,\hfill \\ \hfill \parallel Q\left(t\right)-Q_2\left(t\right)\parallel & <& {\displaystyle \frac{p_4}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel Q\left(t\right)-Q_2\left(t\right)\parallel ,\hfill \\ \hfill \parallel R\left(t\right)-R_2\left(t\right)\parallel & <& {\displaystyle \frac{p_5}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}\parallel R\left(t\right)-R_2\left(t\right)\parallel .\hfill \end{array}$$

(27)

In consequence,

$$\begin{array}{ccc}\hfill \parallel S\left(t\right)-S_2\left(t\right)\parallel (1-{\displaystyle \frac{k{p}_1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}})& <& 0,\hfill \\ \hfill \parallel E\left(t\right)-E_2\left(t\right)\parallel (1-{\displaystyle \frac{k{p}_2}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}})& <& 0,\hfill \\ \hfill \parallel I\left(t\right)-I_2\left(t\right)\parallel (1-{\displaystyle \frac{k{p}_3}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}})& <& 0,\hfill \\ \hfill \parallel Q\left(t\right)-Q_2\left(t\right)\parallel (1-{\displaystyle \frac{k{p}_4}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}})& <& 0,\hfill \\ \hfill \parallel R\left(t\right)-R_2\left(t\right)\parallel (1-{\displaystyle \frac{k{p}_5}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}})& <& 0.\hfill \end{array}$$

(28)

This is a contradiction, hence the result. □

3.4. The Stability of Equilibrium Points

In this subsection, we study the local asymptotic stability of the equilibrium points.

Theorem 10. 

The disease-free equilibrium $P_0=\left(S_0,0,0,0,R_0\right)$ of the suggested discrete fractional variable-order model is locally asymptotically stable if ${\mathcal{R}}_0<1$, and is unstable if ${\mathcal{R}}_0>1$.

Proof. 

The Jacobian matrix of model (1) estimated at $P_0$ is given by

$$\mathcal{J}\left(P_0\right)=\left(\begin{array}{ccccc}-\left(\delta +\mu \right)& 0& -\beta \nu \frac{S_0}{N}& 0& \lambda \\ 0& -\left(\mu +\gamma \right)& \beta \nu \frac{S_0}{N}& 0& 0\\ 0& \gamma & -\left(\psi +{\phi }_1+\varphi +\mu \right)& 0& 0\\ 0& 0& \psi & -\left(\tau +{\phi }_2+\mu \right)& 0\\ \delta & 0& \varphi & \tau & -\left(\lambda +\mu \right)\end{array}\right).$$

The characteristic polynomial of $\mathcal{J}$ is represented by

$$|\mathcal{J}\left(P_0\right)-\lambda \widehat{\mathcal{I}}|=\left(\lambda +\mu \right)\left(\lambda +\tau +{\phi }_1+\mu \right)\left(\lambda +\lambda +\delta +\mu \right)L\left({\lambda }^{\alpha \left(t\right)}\right)=0,$$

where

$$L\left(\lambda \right)={\left(\lambda \right)}^2+a_1\lambda +a_2.$$

Here,

$$a_1=\gamma +\psi +\phi +\varphi +2\mu ;a_2=(1-{\mathcal{R}}_0)(\gamma +\mu )(\psi +\phi +\varphi +\mu ).$$

There are five eigenvalues; ${\lambda }_1<0$; ${\lambda }_2<0$; ${\lambda }_3<0$ and ${\lambda }_4$, ${\lambda }_5$ are the solution of $L\left(\lambda \right)$.

If ${\mathcal{R}}_0<1$ then $a_1>0$ and $a_2>0$, $L\left(\lambda \right)$ has two real roots that are negative, then $P_0$ is locally asymptotically stable. Likewise, condition ${\mathcal{R}}_0>1$ that ${\lambda }_4{\lambda }_5<0$ holds that the equilibrium $P_0$ is unstable, and so the theorem is proven. □

Theorem 11. 

The endemic equilibrium $P_{*}$ is locally asymptotically stable if $R_0>1.$

Proof. 

The Jacobian matrix of model (1) at $P_{*}$ is

$$J\left(P_{*}\right)=\left[\begin{array}{ccccc}-b_1& 0& -\beta \nu l_2& 0& \lambda \\ \beta \nu l_1& -b_2& \beta \nu l_2& 0& 0\\ 0& \gamma & -b_3& 0& 0\\ 0& 0& \psi & -b_4& 0\\ \delta & 0& \varphi & \tau & -b_5\end{array}\right],$$

where

$$b_1=\beta \nu l_1+\delta +\mu ,b_2=\gamma +\mu ,b_3=\psi +\varphi +{\phi }_1+\mu ,$$

$$b_4=\tau +{\phi }_2+\mu ,b_5=\lambda +\mu ,l_1=\frac{I_{*}}{N+\rho I_{*}},l_2=\frac{S_{*}N}{{\left(N+\rho I_{*}\right)}^2}.$$

The characteristic equation is

$$\left|\lambda E-J\left(P_{*}\right)\right|={\lambda }^5+{\zeta }_1{\lambda }^4+{\zeta }_2{\lambda }^3+{\zeta }_3{\lambda }^2+{\zeta }_4\lambda +{\zeta }_5,$$

where

$$\begin{array}{cc}\hfill {\zeta }_1=& \sum _{i=1}^5{a}_i,\hfill \\ \hfill {\zeta }_2=& a_1\left({\xi }_1-a_1\right)+a_2\left({\xi }_1-a_1-a_2\right)+a_3\left(a_4+a_5\right)+a_4{a}_5-{\rho }^a{\theta }^a-\beta c^a{ϵ}^a{h}_2,\hfill \\ \hfill {\zeta }_3=& a_2\left[a_4{a}_5+a_1\left(a_4+a_5\right)\right]+a_3\left[a_4{a}_5+a_2\left(a_1+a_4+a_5\right)+a_1\left(a_4+a_5\right)\right]+a_1{a}_4{a}_5\hfill \\ \hfill & +{ϵ}^{\alpha }{\beta }^2{c}^{2\alpha }{h}_1{h}_2-{ϵ}^{\alpha }\beta c^{\alpha }{h}_2\left(a_1+a_4+a_5\right)-{\theta }^{\alpha }{\rho }^{\alpha }\left(a_2+a_3+a_4\right),\hfill \\ \hfill {\zeta }_4=& a_3\left[a_2\left(a_4{a}_5+a_1\left(a_4+a_5\right)\right)+a_1{a}_4{a}_5\right]+a_1{a}_2{a}_4{a}_5+\left(a_4+a_5\right){ϵ}^{\alpha }{\beta }^2{c}^{2x}{h}_1{h}_2\hfill \\ \hfill & -\left(a_4{a}_5+a_1{a}_5+a_1{a}_4\right){ϵ}^{\alpha }\beta c^{\alpha }{h}_2-\left(a_2{a}_4+a_3{a}_4+a_2{a}_3\right){\theta }^{\alpha }{\rho }^{\alpha }+{ϵ}^a{\theta }^{\alpha }\beta c^a\left({\rho }^{\alpha }{h}_2-{\gamma }^a{h}_1\right),\hfill \\ \hfill {\zeta }_5=& a_1{a}_2{a}_3{a}_4{a}_5+a_4{a}_5{ϵ}^{\alpha }{\beta }^2{c}^{2x}{h}_1{h}_2-a_1{a}_4{a}_5{ϵ}^{\alpha }\beta c^{\alpha }{h}_2-a_2{a}_3{a}_4{\theta }^{\alpha }{\rho }^{\alpha }\hfill \\ \hfill & +a_4{ϵ}^{\alpha }{\theta }^{\alpha }\beta c^{\alpha }\left({\rho }^{\alpha }{h}_2-{\gamma }^{\alpha }{h}_1\right)-{ϵ}^{\alpha }{\delta }^{\alpha }{\theta }^{\alpha }{\eta }^{\alpha }\beta c^{\alpha }{h}_1.\hfill \end{array}$$

If ${\zeta }_{\mathrm{i}}>0(i=2,3,4,5)$ and it is clear that ${\zeta }_1>0$, the sufficient conditions can be derived as follows:

$$\left|\begin{array}{cc}{\zeta }_1\hfill & 1\hfill \\ {\zeta }_3\hfill & {\zeta }_2\hfill \end{array}\right|>0,\left|\begin{array}{ccc}{\zeta }_1\hfill & 1\hfill & 0\hfill \\ {\zeta }_3\hfill & {\zeta }_2\hfill & {\zeta }_1\hfill \\ {\zeta }_5\hfill & {\zeta }_4\hfill & {\zeta }_3\hfill \end{array}\right|>0,\left|\begin{array}{cccc}{\zeta }_1& 1& 0& 0\\ {\zeta }_3& {\zeta }_2& {\zeta }_1& 1\\ {\zeta }_5& {\zeta }_4& {\zeta }_3& {\zeta }_2\\ 0& 0& {\zeta }_5& {\zeta }_4\end{array}\right|>0,$$

(29)

for which the equilibrium $P_{*}$ is locally asymptotically stable. □

4. Optimal Control Problem

A vaccine for the emerging coronavirus (COVID-19) has been developed, aiming to decrease the number of contacts between susceptible individuals and infected individuals to limit infection and mitigate the spread of the virus. This can be realized through various measures, including home quarantine, nucleic acid testing, and restrictions on residents’ movement. Mathematically, these measures can be represented by a coefficient denoted as u in this section, indicating the intensity of different control measures. Consequently, the model system of Equation (1) is modified as follows:

$$\begin{array}{ccc}\hfill {\Delta }^{\alpha \left(t\right)}S\left(t\right)& =& \chi -\beta {\nu }^{\alpha \left(t\right)}(1-u\left(t\right)){\displaystyle \frac{SI}{N+\rho I}}+{\lambda }^{\alpha \left(t\right)}R\left(t\right)-({\delta }^{\alpha \left(t\right)}+{\mu }^{\alpha \left(t\right)})S\left(t\right),\hfill \\ \hfill {\Delta }^{\alpha \left(t\right)}E\left(t\right)& =& \beta {\nu }^{\alpha \left(t\right)}(1-u\left(t\right)){\displaystyle \frac{SI}{N+\rho I}}-({\gamma }^{\alpha \left(t\right)}+{\mu }^{\alpha \left(t\right)})E\left(t\right),\hfill \\ \hfill {\Delta }^{\alpha \left(t\right)}I\left(t\right)& =& {\gamma }^{\alpha \left(t\right)}E\left(t\right)-({\psi }^{\alpha \left(t\right)}+{\varphi }^{\alpha \left(t\right)}+{\phi }_1^{\alpha \left(t\right)}+{\mu }^{\alpha \left(t\right)})I\left(t\right),\hfill \\ \hfill {\Delta }^{\alpha \left(t\right)}Q\left(t\right)& =& {\psi }^{\alpha \left(t\right)}I\left(t\right)-({\tau }^{\alpha \left(t\right)}+{\phi }_2^{\alpha \left(t\right)}+{\mu }^{\alpha \left(t\right)})Q\left(t\right),\hfill \\ \hfill {\Delta }^{\alpha \left(t\right)}R\left(t\right)& =& {\delta }^{\alpha \left(t\right)}S\left(t\right)+{\varphi }^{\alpha \left(t\right)}I\left(t\right)+{\tau }^{\alpha \left(t\right)}Q\left(t\right)-({\lambda }^{\alpha \left(t\right)}+{\mu }^{\alpha \left(t\right)})R\left(t\right).\hfill \end{array}$$

(30)

The corresponding discrete fractional optimal control problem with variable order in the Caputo sense is considered as follows:

$$J\left(u^{*}\right)=\underset{u\in \mathsf{\Omega }}{\min }J\left(u\right),$$

where J is defined by

$$J\left(u\right)=\sum _{k=0}^{N-1}{C}_{1}E\left(k\right)+C_{2}I\left(k\right)+C_{3}Q\left(k\right)+C_4{u}^2\left(k\right),$$

and the control space $\mathsf{\Omega }$ is defined by the set

$$\mathsf{\Omega }=\left\{u\in {\mathcal{R}}^n/0<u_{min}<u<u_{max}<1\right\}.$$

The coefficients $C_1,C_2,C_3$ and $C_4$ represent the positive weight constants of exposed, infected, isolated and control variables.

Theorem 12. 

Let $u^{*}$ denote the optimal control variable of the discrete fractional optimal control with variable order, and let $S^{*}$, $E^{*}$, $I^{*}$, $Q^{*}$, and $R^{*}$ represent the optimal state solution. Additionally, there exist adjoint variables ${\kappa }_i$, where $i=1,\dots ,5$, satisfying the following equations:

$$\begin{array}{ccc}\hfill {\Delta }^{\alpha (t)}{\kappa }_1(t)& =& -(\beta {\nu }^{\alpha (t)}(1-u){\displaystyle \frac{I}{N+\rho I}}+{\delta }^{\alpha (t)}+{\mu }^{\alpha (t)}){\kappa }_1(t)+\beta {\nu }^{\alpha (t)}(1-u){\displaystyle \frac{I}{N+\rho I}}{\kappa }_2(t)+{\delta }^{\alpha (t)}{\kappa }_5(t),\hfill \\ \hfill {\Delta }^{\alpha (t)}{\kappa }_2(t)& =& C_1-({\gamma }^{\alpha (t)}+{\mu }^{\alpha (t)}){\kappa }_2(t)+{\gamma }^{\alpha (t)}{\kappa }_2(t),\hfill \\ \hfill {\Delta }^{\alpha (t)}{\kappa }_3(t)& =& C_2-(\beta {\nu }^{\alpha (t)}(1-u){\displaystyle \frac{I}{{(N+\rho I)}^2}}{\kappa }_1(t)+(\beta {\nu }^{\alpha (t)}(1-u){\displaystyle \frac{SN}{{(N+\rho I)}^2}}{\kappa }_2(t)-\hfill \\ & & ({\psi }^{\alpha (t)}+{\varphi }^{\alpha (t)}+{\phi }_1^{\alpha (t)}+{\mu }^{\alpha (t)}){\kappa }_3(t)+{\psi }^{\alpha (t)}{\kappa }_4(t)+{\varphi }^{\alpha (t)}{\kappa }_5(t),\hfill \\ \hfill {\Delta }^{\alpha (t)}{\kappa }_4(t)& =& C_3-({\tau }^{\alpha (t)}+{\phi }_2^{\alpha (t)}+{\mu }^{\alpha (t)}){\kappa }_4(t)+{\tau }^{\alpha (t)}{\kappa }_5,\hfill \\ \hfill {\Delta }^{\alpha (t)}{\kappa }_5(t)& =& {\lambda }^{\alpha (t)}{\kappa }_1-({\lambda }^{\alpha (t)}+{\mu }^{\alpha (t)}){\kappa }_5,\hfill \end{array}$$

(31)

with ${\Delta }_T^{\alpha \left(t\right)}{\kappa }_i\left(t\right)=0$, $i=1,\cdots ,5.$ In addition, the optimal control $u^{*}$ is characterized by

$$u^{*}=\min \left(\max \left(\frac{\beta {\nu }^{\alpha \left(t\right)}SI({\kappa }_2-{\kappa }_1)}{2C_4(N+\rho I)},0\right),1\right).$$

(32)

Proof. 

We can determine the discrete optimal control $u^{*}$ by the application of a discrete version of Pontryagin’s maximum principle as in [49,50,51] to the discrete Hamiltonian function $\mathcal{M}$ as follows

$$\begin{array}{cc}\hfill \mathcal{M}=& C_{1}E+C_{2}I+C_{3}Q+C_4{u}^2+{\kappa }_1(\chi -\beta {\nu }^{\alpha (t)}(1-u){\displaystyle \frac{SI}{N+\rho I}}+{\lambda }^{\alpha (t)}R-({\delta }^{\alpha (t)}+{\mu }^{\alpha (t)})S)\hfill \\ \hfill & +{\kappa }_2(\beta {\nu }^{\alpha (t)}(1-u){\displaystyle \frac{SI}{N+\rho I}}-({\gamma }^{\alpha (t)}+{\mu }^{\alpha (t)})E)+{\kappa }_3({\gamma }^{\alpha (t)}E-({\psi }^{\alpha (t)}+{\varphi }^{\alpha (t)}+{\phi }_1^{\alpha (t)}+{\mu }^{\alpha (t)})I)\hfill \\ & +{\kappa }_4({\psi }^{\alpha (t)}I-({\tau }^{\alpha (t)}+{\phi }_2^{\alpha (t)}+{\mu }^{\alpha (t)})Q)+{\kappa }_5({\delta }^{\alpha (t)}S+{\varphi }^{\alpha (t)}I+{\tau }^{\alpha (t)}Q-({\lambda }^{\alpha (t)}+{\mu }^{\alpha (t)})R.\hfill \end{array}$$

By using the following formulations,

$${\Delta }_T^{\alpha \left(t\right)}{\kappa }_1={\displaystyle \frac{\partial H}{\partial S}};{\Delta }_T^{\alpha \left(t\right)}{\kappa }_2={\displaystyle \frac{\partial H}{\partial E}};{\Delta }_T^{\alpha \left(t\right)}{\kappa }_3={\displaystyle \frac{\partial H}{\partial I}};{\Delta }_T^{\alpha \left(t\right)}{\kappa }_4={\displaystyle \frac{\partial H}{\partial Q}};{\Delta }_T^{\alpha \left(t\right)}{\kappa }_5={\displaystyle \frac{\partial H}{\partial R}},$$

the discrete-time fractional adjoint system with variable order is given as

$$\begin{array}{ccc}\hfill {\Delta }^{\alpha (t)}{\kappa }_1(t)& =& -(\beta {\nu }^{\alpha (t)}(1-u){\displaystyle \frac{I}{N+\rho I}}+{\delta }^{\alpha (t)}+{\mu }^{\alpha (t)}){\kappa }_1(t)+\beta {\nu }^{\alpha (t)}(1-u){\displaystyle \frac{I}{N+\rho I}}{\kappa }_2(t)+{\delta }^{\alpha (t)}{\kappa }_5(t),\hfill \\ \hfill {\Delta }^{\alpha (t)}{\kappa }_2(t)& =& C_1-({\gamma }^{\alpha (t)}+{\mu }^{\alpha (t)}){\kappa }_2(t)+{\gamma }^{\alpha (t)}{\kappa }_2(t),\hfill \\ \hfill {\Delta }^{\alpha (t)}{\kappa }_3(t)& =& C_2-(\beta {\nu }^{\alpha (t)}(1-u){\displaystyle \frac{I}{{(N+\rho I)}^2}}{\kappa }_1(t)+(\beta {\nu }^{\alpha (t)}(1-u){\displaystyle \frac{SN}{{(N+\rho I)}^2}}{\kappa }_2(t)\hfill \\ & & -({\psi }^{\alpha (t)}+{\varphi }^{\alpha (t)}+{\phi }_1^{\alpha (t)}+{\mu }^{\alpha (t)}){\kappa }_3(t)+{\psi }^{\alpha (t)}{\kappa }_4(t)+{\varphi }^{\alpha (t)}{\kappa }_5(t),\hfill \\ \hfill {\Delta }^{\alpha (t)}{\kappa }_4(t)& =& C_3-({\tau }^{\alpha (t)}+{\phi }_2^{\alpha (t)}+{\mu }^{\alpha (t)}){\kappa }_4(t)+{\tau }^{\alpha (t)}{\kappa }_5,\hfill \\ \hfill {\Delta }^{\alpha (t)}{\kappa }_5(t)& =& {\lambda }^{\alpha (t)}{\kappa }_1-({\lambda }^{\alpha (t)}+{\mu }^{\alpha (t)}){\kappa }_5.\hfill \end{array}$$

(33)

The control equation is ${\displaystyle \frac{\partial H}{\partial u}}=0,$ and we have the optimal condition

$$\frac{\partial H}{\partial u}=2C_{4}u+\beta {\nu }^{\alpha \left(t\right)}{\displaystyle \frac{SI}{N+\rho I}}{\kappa }_1-\beta {\nu }^{\alpha \left(t\right)}{\displaystyle \frac{SI}{N+\rho I}}{\kappa }_2=0,$$

$$u={\displaystyle \frac{\beta {\nu }^{\alpha \left(t\right)}SI({\kappa }_2-{\kappa }_1)}{2(N+\rho I)C_4}},$$

and for the optimal control $u^{*}$, we have

$$u^{*}=\left\{\begin{array}{cc}0\hfill & {\displaystyle if\frac{\partial H}{\partial u}<0}\hfill \\ u\hfill & {\displaystyle if\frac{\partial H}{\partial u}=0}\hfill \\ 1\hfill & {\displaystyle if\frac{\partial H}{\partial u}>0}\hfill \end{array}\right.$$

and

$$u^{*}=\min \left(\max \left(\frac{\beta {\nu }^{\alpha \left(t\right)}SI({\kappa }_2-{\kappa }_1)}{2C_4(N+\rho I)},0\right),1\right).$$

□

5. Numerical Simulation

5.1. Numerical Strategy without Control

In this subsection, we solve the discrete-time fractional variable-order model defined by the system (1) using the Adams type predictor–corrector method proposed in [52].

$$\begin{array}{ccc}\hfill {\Delta }_t^{\alpha \left(t\right)}y\left(t\right)& =& f(t,y(t\left)\right),\hfill \\ \hfill y{\left(0\right)}^b& =& y_0^b,0<\alpha \left(t\right)<1,0<t<\tau ,\hfill \end{array}$$

(34)

where $b=0,1,\dots ,n-1$, and $n=\left[\alpha \right(t\left)\right].$ Analogous to the fractional order meaning, the above is equivalent to the Volterra equation

$$y\left(t\right)=\sum _{b=0}^{n-1}{y}_0^b{\displaystyle \frac{t^b}{b!}}+{\displaystyle \frac{1}{\mathsf{\Gamma }\left(\alpha \right(t\left)\right)}}\sum _{s=0}^{t-\alpha \left(t\right)}{(t-\sigma \left(s\right))}^{\left(\alpha \right(t)-1)}f(s,y\left(s\right)),$$

(35)

to obtain the numerical solutions of the suggested model. We take

$$h={\displaystyle \frac{\tau }{N}};t_z=zh;N=\bigcup \left[N_{k-1},N_k\right];z=N_{k-1},\dots ,N_k\in {\mathbb{Z}}^{+},$$

$\alpha \left(t\right)=\left({\alpha }_1,{\alpha }_2,\dots ,{\alpha }_k\right)$ by means of letting $y_z\approx y\left(t_z\right)$, the discretization of (1) is

$$\begin{array}{ccc}\hfill S_{q+1}& =& \sum _{z=0}^{[\alpha (k]}{S}_0^{(z)}{\displaystyle \frac{t_{q+1}^{(z)}}{z!}}+{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+2)}}\sum _{z=0}^q(p_{z,q+1})(\chi -\beta \nu {\displaystyle \frac{S_z{I}_z}{N+\rho I_z}}+\lambda R_z-(\delta +\mu )S_z)\hfill \\ & & +{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+2)}}\sum _{z=0}^q(p_{q+1,q+1})(\chi -\beta \nu {\displaystyle \frac{S_{q+1}^{PF}{I}_{q+1}^{PF}}{N+\rho I_{q+1}^{PF}}}+\lambda R_{q+1}^{PF}-(\delta +\mu )S_{q+1}^{PF}),\hfill \\ \hfill E_{q+1}& =& \sum _{z=0}^{[\alpha (k)]}{E}_0^{(z)}{\displaystyle \frac{t_{q+1}^{(z)}}{z!}}+{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+2)}}\sum _{z=0}^q(p_{z,q+1})(\beta \nu {\displaystyle \frac{S_z{I}_z}{N+\rho I_z}}-(\gamma +\mu )E_z)\hfill \\ & & +{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+2)}}\sum _{z=0}^q(p_{q+1,q+1})(\beta \nu {\displaystyle \frac{S_{q+1}^{PF}{I}_{q+1}^{PF}}{N+\rho I_{q+1}^{PF}}}-(\gamma +\mu )E_{q+1}^{PF}),\hfill \\ \hfill I_{q+1}& =& \sum _{z=0}^{[\alpha (k)]}{I}_0^{(z)}{\displaystyle \frac{t_{q+1}^{(z)}}{z!}}+{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+2)}}\sum _{z=0}^q(p_{z,q+1})(\gamma E_z-(\psi +\varphi +{\phi }_1+\mu )I_z)\hfill \\ & & +{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+2)}}\sum _{z=0}^q(p_{q+1,q+1})(\gamma E_{q+1}^{PF}(\psi +\varphi +{\phi }_1+\mu )I_{q+1}^{PF},\hfill \\ \hfill Q_{q+1}& =& \sum _{z=0}^{[\alpha (k)]}{Q}_0^{(z)}{\displaystyle \frac{t_{q+1}^{(z)}}{z!}}+{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+2)}}\sum _{z=0}^q(p_{z,q+1})(\psi I_z-(\tau +{\phi }_2+\mu )Q_z)\hfill \\ & & +{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+2)}}\sum _{z=0}^q(p_{q+1,q+1})(r_1^{\alpha (k)}{M}_{q+1}^{PF}(\psi I_{q+1}^{PF}-(\tau +{\phi }_2+\mu )Q_{q+1}^{PF}),\hfill \\ \hfill R_{q+1}& =& \sum _{z=0}^{[\alpha (k)]}{R}_0^{(z)}{\displaystyle \frac{t_{q+1}^{(z)}}{z!}}+{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+2)}}\sum _{z=0}^q(p_{z,q+1})(\delta S_z+\varphi I_z+\tau Q_z-(\lambda +\mu )R_z)\hfill \\ & & +{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+2)}}\sum _{z=0}^q(p_{q+1,q+1})(\delta S_{q+1}^{PF}+\varphi I_{q+1}^{PF}+\tau Q_{q+1}^{PF}-(\lambda +\mu )R_{q+1}^{PF}),\hfill \end{array}$$

(36)

where

$$p_{z,q+1}=\left\{\begin{array}{cc}{q}^{\alpha \left(k\right)+1}-(q-\alpha \left(k\right)){(q+1)}^{\alpha \left(k\right)},\hfill & \mathrm{if}z=0\hfill \\ {(q-z+2)}^{\alpha \left(k\right)+1}+{(q-z)}^{\alpha \left(k\right)+1}-2{(q-z+1)}^{\alpha \left(k\right)+1},\hfill & \mathrm{if}1<z<q\hfill \\ 1,\hfill & \mathrm{if}z=q+1.\hfill \end{array}\right.$$

(37)

The proposed prediction formula is calculated as follows:

$$\begin{array}{cc}\hfill S_{q+1}^{PF}=& {\displaystyle \sum _{z=0}^{[\alpha (k)]}{S}_0^{(z)}{\displaystyle \frac{t_{q+1}^{(z)}}{z!}}+{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+1)}}\sum _{z=0}^q(j_{z,q+1})(\chi -\beta \nu {\displaystyle \frac{S_z{I}_z}{N+\rho I_z}}+\lambda R_z-(\delta +\mu )S_z),}\hfill \\ \hfill E_{q+1}^{PF}=& {\displaystyle \sum _{z=0}^{[\alpha (k)]}{E}_0^{(z)}{\displaystyle \frac{t_{q+1}^{(z)}}{z!}}+{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+1)}}\sum _{z=0}^q(j_{z,q+1})(\beta \nu {\displaystyle \frac{S_z{I}_z}{N+\rho I_z}}-(\gamma +\mu )E_z),}\hfill \\ \hfill I_{q+1}^{PF}=& {\displaystyle \sum _{z=0}^{[\alpha (k)]}{I}_0^{(z)}{\displaystyle \frac{t_{q+1}^{(z)}}{z!}}+{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+1)}}\sum _{z=0}^q(j_{z,q+1})(\psi +\varphi +{\phi }_1+\mu )I_z),}\hfill \\ \hfill Q_{q+1}^{PF}=& {\displaystyle \sum _{z=0}^{[\alpha (k)]}{Q}_0^{(z)}{\displaystyle \frac{t_{q+1}^{(z)}}{z!}}+{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+1)}}\sum _{z=0}^q(j_{z,q+1})(\psi I_z-(\tau +{\phi }_2+\mu )Q_z),}\hfill \\ \hfill R_{q+1}^{PF}=& {\displaystyle \sum _{z=0}^{[\alpha (k)]}{R}_0^{(z)}{\displaystyle \frac{t_{q+1}^{(z)}}{z!}}+{\displaystyle \frac{h^{\alpha (k)}}{\mathsf{\Gamma }(\alpha (k)+1)}}\sum _{z=0}^q(j_{z,q+1})(\delta S_z+\varphi I_z+\tau Q_z-(\lambda +\mu )R_z)}\hfill \end{array}$$

(38)

where

$$j_{z,q+1}={(q+1-z)}^{\alpha \left(k\right)}-{(q-z)}^{\alpha \left(k\right)}.$$

5.2. Numerical Strategy with Control

In this subsection, to solve the (V-FOCP) in the discrete-time defined, we have

$$Y_{q+1}=\sum _{z=0}^{\left[\alpha \right(k\left)\right]}{Y}_0^{\left(z\right)}{\displaystyle \frac{t_{q+1}^{\left(z\right)}}{z!}}+{\displaystyle \frac{h^{\alpha \left(k\right)}}{\mathsf{\Gamma }\left(\alpha \right(k)+2)}}\sum _{z=0}^q\left(p_{z,q+1}\right)g(t_z,Y_z,u_q)+{\displaystyle \frac{h^{\alpha \left(k\right)}}{\mathsf{\Gamma }\left(\alpha \right(k)+2)}}\sum _{z=0}^q\left(p_{q+1,q+1}\right)g(t_{q+1},Y_{q+1}^{PF},u_{q+1}^{PF})$$

(39)

where $Y={\left(S,E,I,Q,R\right)}^T.$ We can rewrite the system of adjoint equations in the compact form with

$$L(t,Y,\kappa ,u)=\left[\begin{array}{c}{L}_1(t,Y,\kappa ,u)\\ L_2(t,Y,\kappa ,u)\\ L_3(t,Y,\kappa ,u)\\ L_4(t,Y,\kappa ,u)\\ L_5(t,Y,\kappa ,u)\end{array}\right].$$

We obtain

$${\Delta }_t^{\alpha \left(t\right)}\kappa (T_f-t)=L(T_f-t,Y(T_f-t),\kappa (T_f-t),u(T_f-t)).$$

(40)

We have a discritized control system as in [38] with following algorithm.

5.3. Solution Algorithm of V-FOCP in Discrete Time

Step 1: Consider the initial estimation control u and used the initial condition.

Step 2: Find the adjoint variable and the optimal states by solving control problem.

Step 3: Find the control $u^{*}$ using control function.

Step 4: Take $u_k=\frac{u^{*}+u_k}{2}$ to update the control.

Step 5: Stop the iteration when $\frac{\parallel u_k-u_{k-1}\parallel }{\parallel u_k\parallel }$ otherwise return to Step 2.

6. Numerical Results and Discussion

In this section, we use the parameters provided in Table 1 to discuss the introduced model (1) numerically. Additionally, the proposed model of fractional variable order in discrete time is numerically solved using the method outlined in the previous section. Moreover, the initial value conditions for the system (1) are set as follows:

$S\left(0\right)=5.5\times {10}^6$, $E\left(0\right)=4.25\times {10}^4$, $I\left(0\right)=\mathrm{18,000}$, $Q\left(0\right)=3000$, and $R\left(0\right)=4\times {10}^6$ as in [38]. The following values are assumed for $\alpha \left(t_k\right)$:

$${\alpha }_1=1$$

$${\alpha }_2=\left(\begin{array}{ccc}0.8& 0.85& 0.9\end{array}\right),{\alpha }_3=\left(\begin{array}{ccc}0.7& 0.75& 0.8\end{array}\right),{\alpha }_4=\left(\begin{array}{ccc}0.6& 0.65& 0.7\end{array}\right).$$

Figure 1, Figure 2 and Figure 3 depict the influence of different values of the variable fractional parameter ${\alpha }_k$ on the dynamics of subdivisions for the total population of COVID-19. We obtained interesting results by varying ${\alpha }_k$. Figure 1 illustrates changes in the susceptible population graph as the value of $\alpha$ varies, with a decrease in the number of days separating the two curves as $\alpha$ decreases. We observe a proportional relationship between the time taken to reach the peak point in the graphs for exposed $\left(E\right)$ and infected $\left(I\right)$ individuals and the changes in $\alpha$ as depicted in Figure 2 and Figure 3.

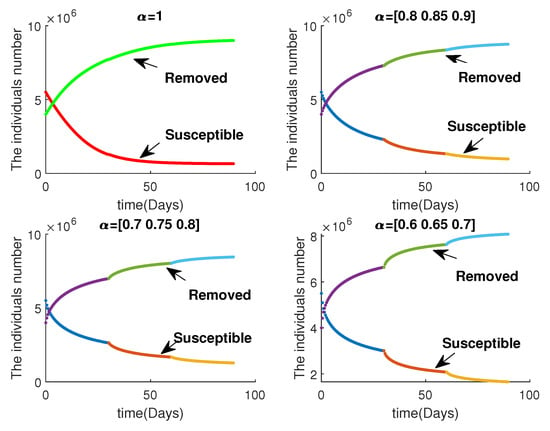
Figure 1. The behavior of the susceptible and removed population from some variable order $\alpha$.

Figure 2. The behavior of the exposed and infected population from some variable order $\alpha$.

Figure 3. The behavior of the isolated population from some variable order $\alpha$.

Figure 4, Figure 5, Figure 6, Figure 7 and Figure 8 present simulation results that demonstrate the significance of the control variable to control the pandemic. The optimal control measures have a positive impact on reducing the rate of infection and the number of individuals exposed to infection, as depicted in Figure 5. Additionally, the number of people recovering increases more than usual. However, the decline in the susceptible population slows down due to the reduction in the number of individuals at risk resulting from the control measures.

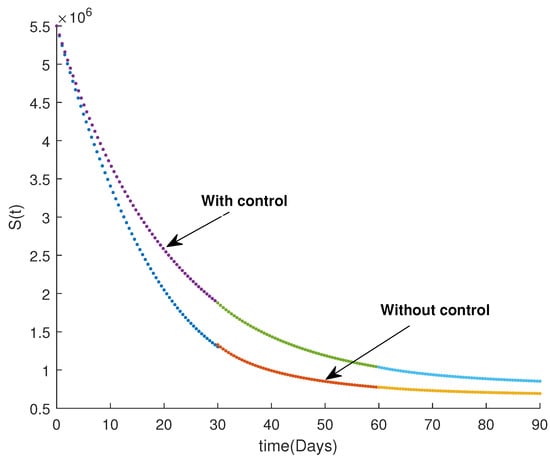
Figure 4. Susceptible group with control and without control for $\alpha =[0.99,0.95,0.93]$.

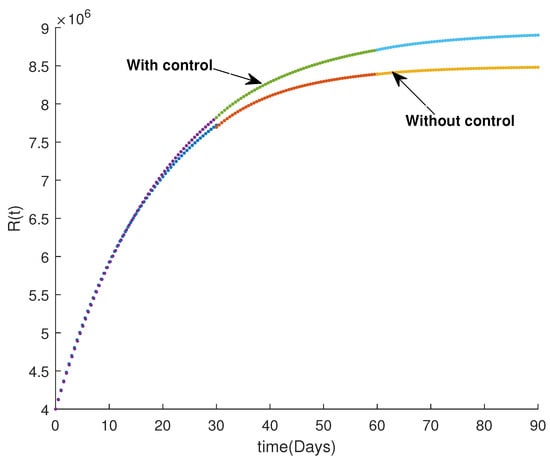
Figure 5. Recovered group with control and without control for $\alpha =[0.99,0.95,0.93]$.

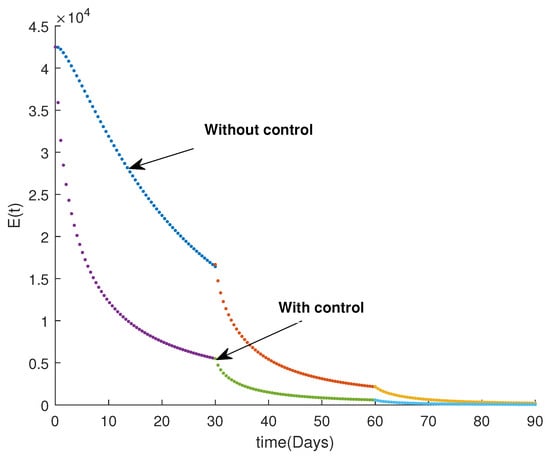
Figure 6. Exposed group with control and without control for $\alpha =[0.7,0.75,0.8]$.

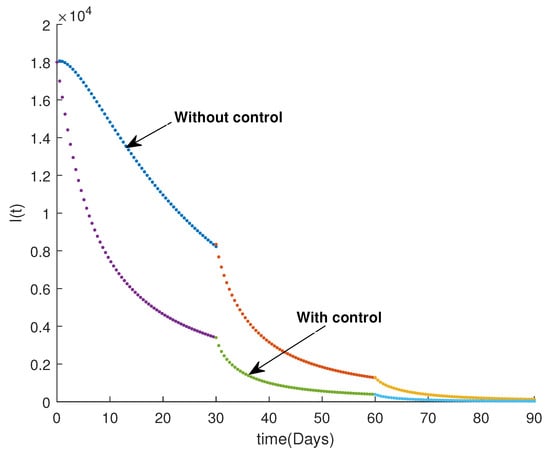
Figure 7. Infected group with control and without control for $\alpha =[0.7,0.75,0.8]$.

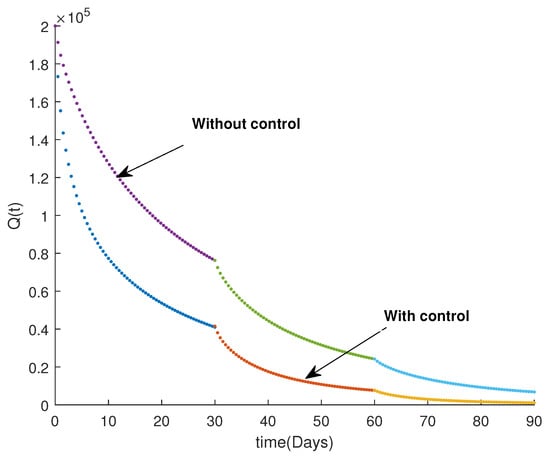
Figure 8. Isolated group with control and without control for $\alpha =[0.7,0.75,0.8]$.

7. Conclusions

In this study, we have conducted an analysis of a novel mathematical model for the ’SEIQR’ epidemic (COVID-19), incorporating an isolated class. This model is characterized by a discrete-time system of fractional variable order in the Caputo sense. We have examined the non-negativity and boundedness of the solution, as well as determined the reproduction number $R_0$ by computing the spectral radius of the next-generation matrix. Based on the threshold $R_0$, we have established the existence and stability of both the disease-free equilibrium and endemic equilibrium points. Moreover, we have applied an optimal control approach to a discrete-time COVID-19 model. A numerical scheme utilizing the Adam’s numerical method was employed for the Caputo fractional variable-order system. We tackled the Variable-Order Fractional Optimal Control Problem (VO-FOCP) in discrete time. Numerical simulations have been conducted to underscore the significance of control measures. It has been observed that upon implementation of control measures, the number of susceptible individuals increases, while the number of infected and recovered individuals decreases.