Ultrasound Computed Tomography Reflection Imaging with Coherence-Factor Beamforming for Breast Tumor Early Detection
Abstract
1. Introduction
2. Materials and Methods
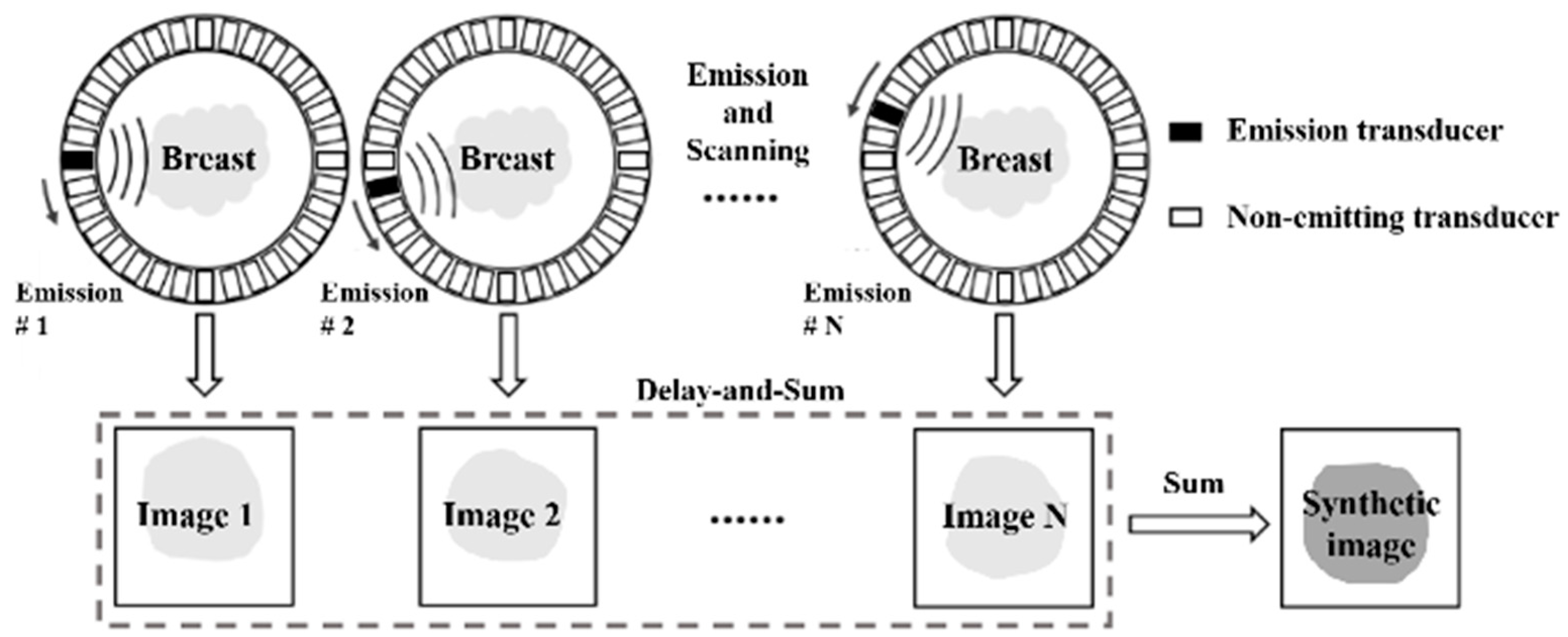
2.1. Previous Delay-and-Sum Beamforming in Synthetic Aperture USCT Reflection Imaging
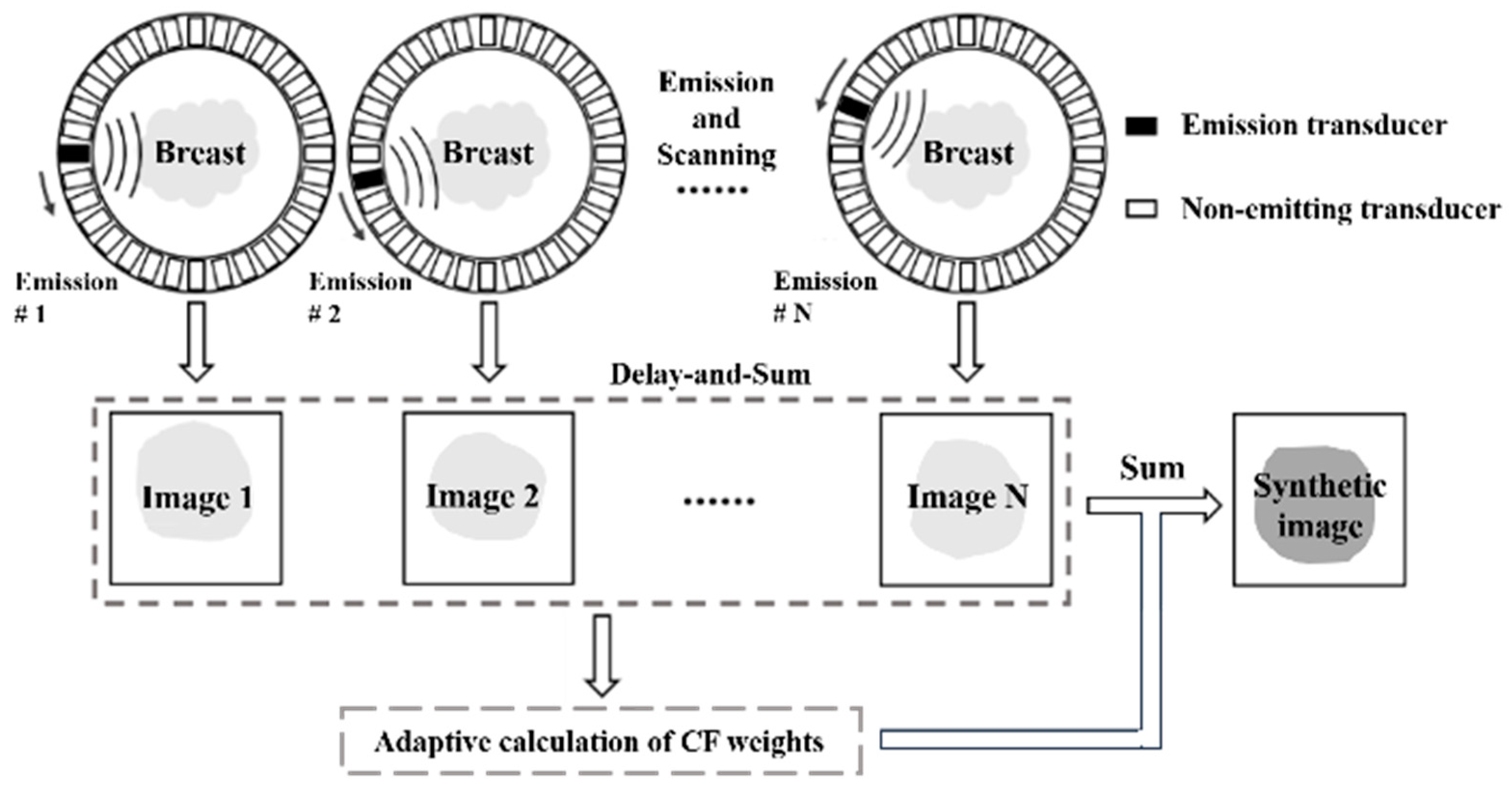
2.2. Coherence-Factor Beamforming in Synthetic Aperture USCT Reflection Imaging
3. USCT Prototype
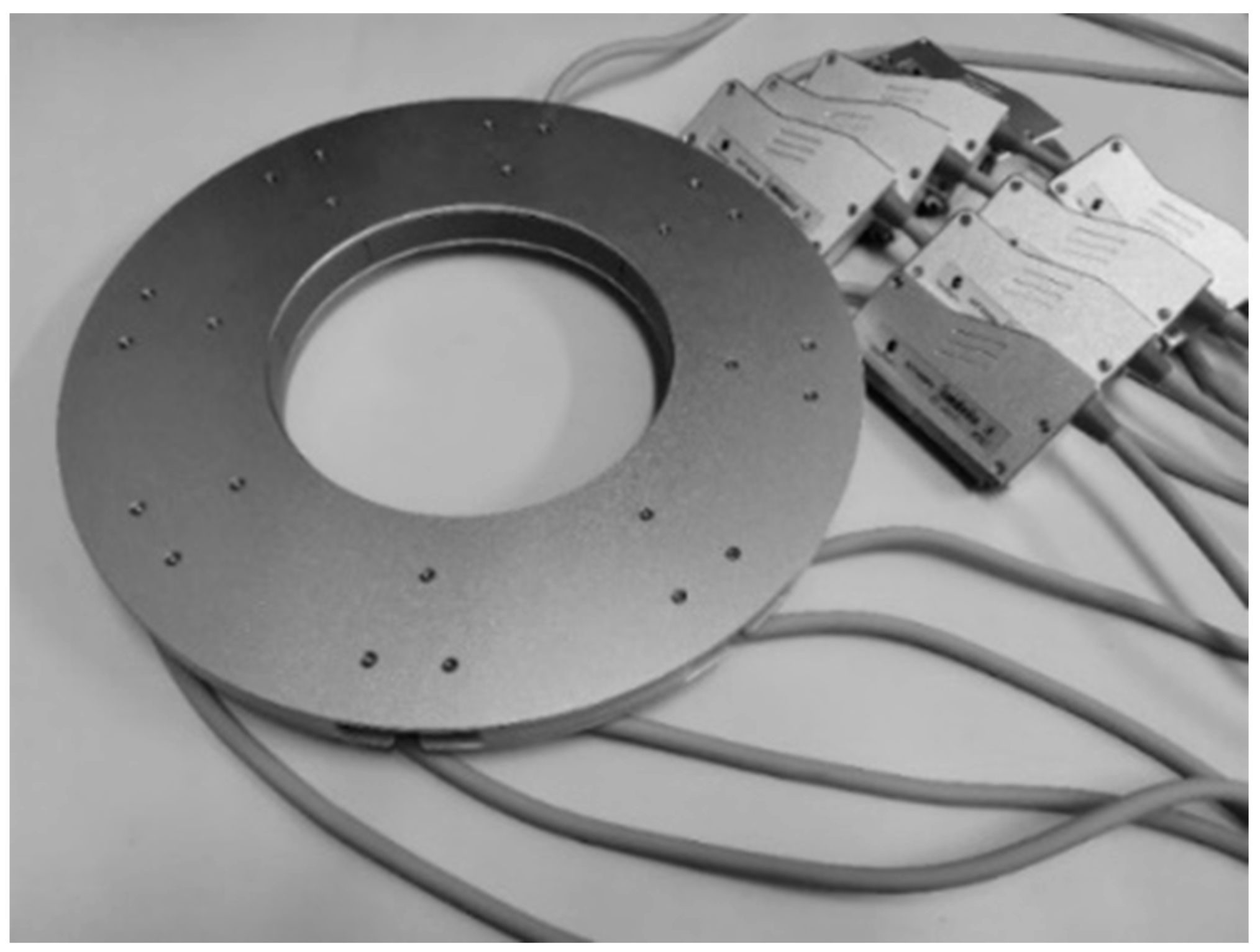
3.1. The Ring Transducer Array
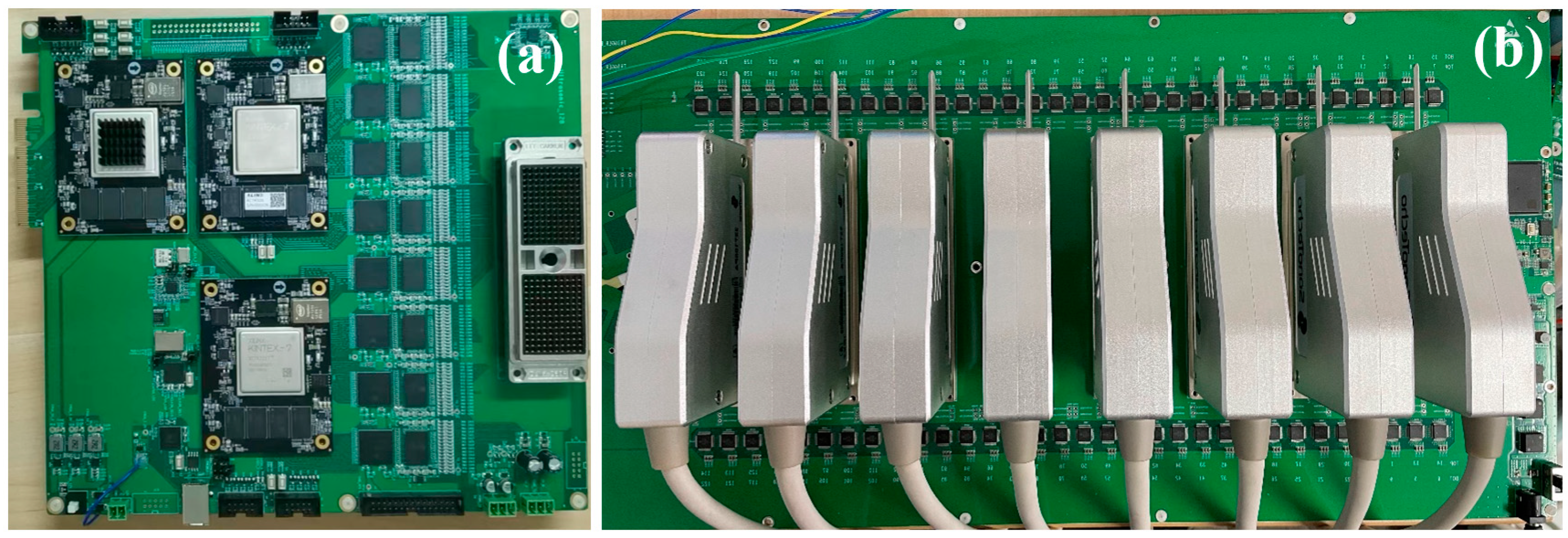
3.2. USCT Prototype Core Circuitry
4. Experiment Methods
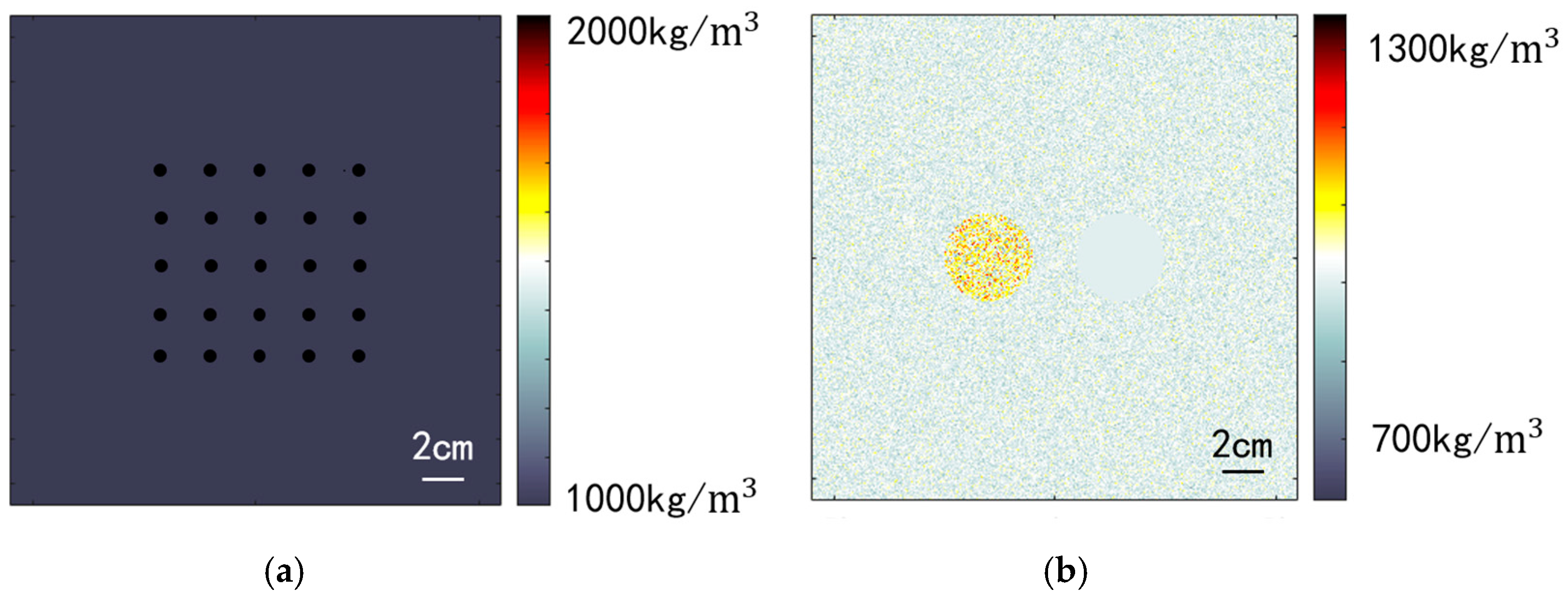
4.1. Simulation Setup
4.2. Experimental Setup
4.3. Evaluation Metrics
5. Results
5.1. Resolution and Contrast Comparison in Simulation
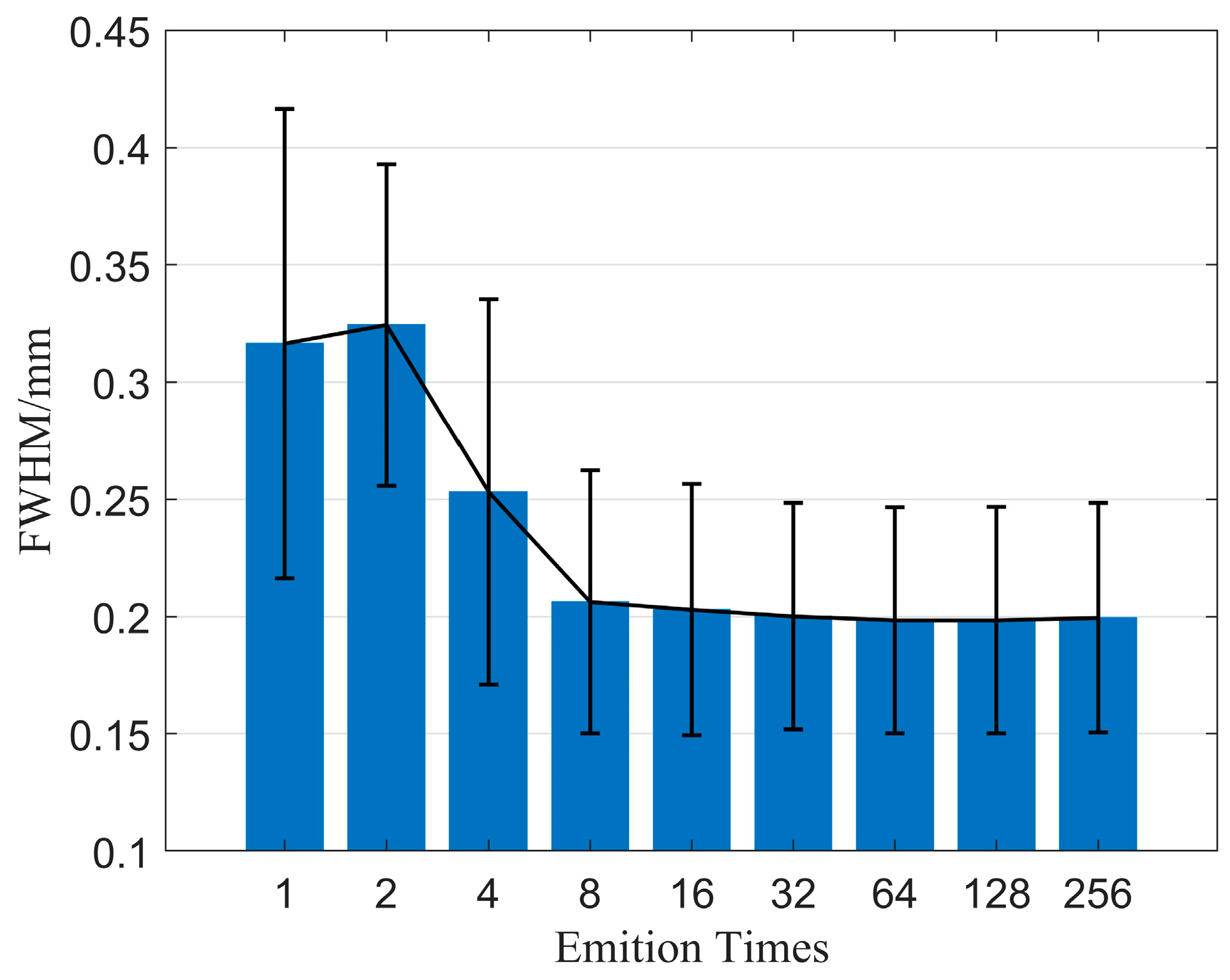
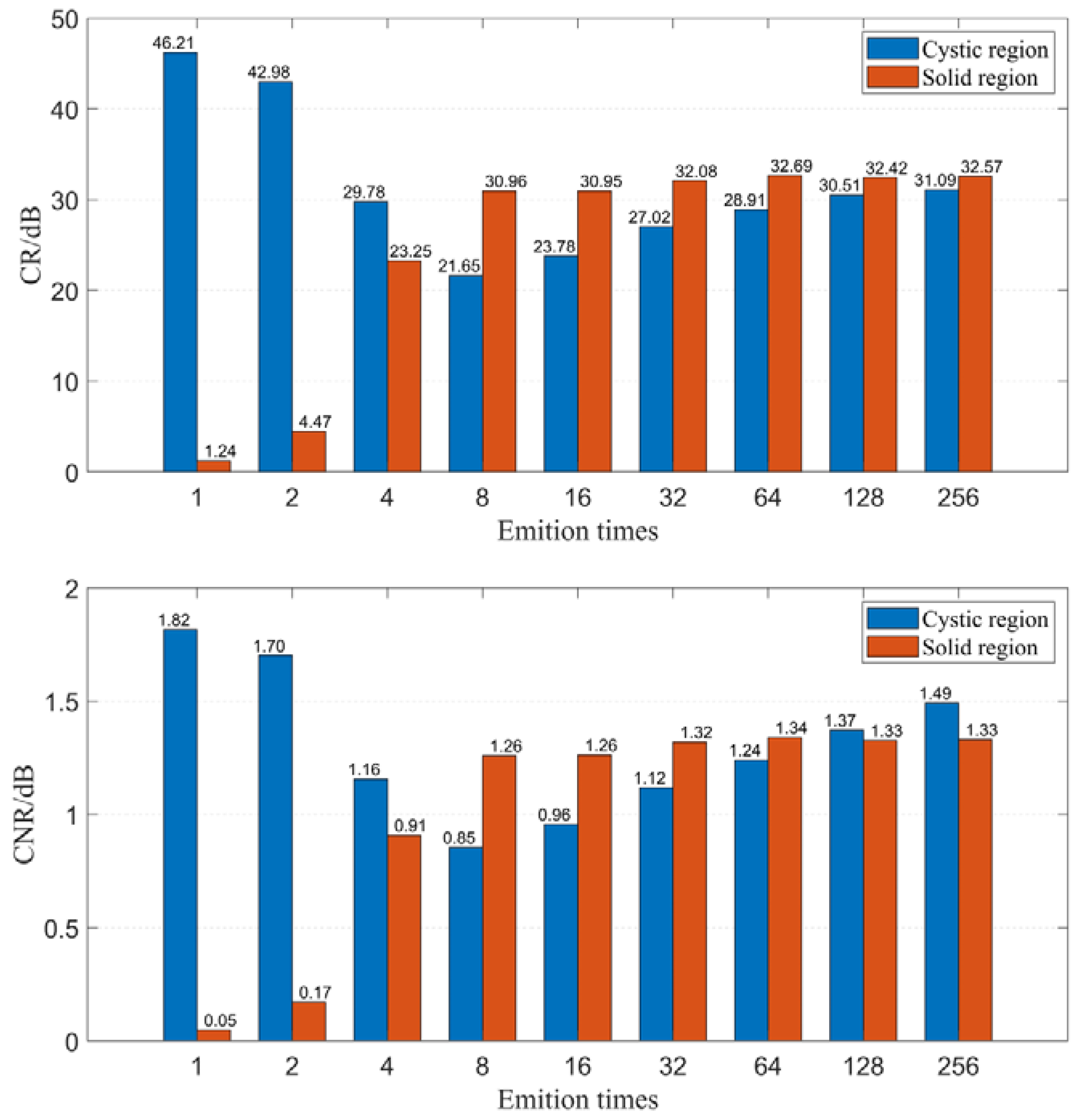
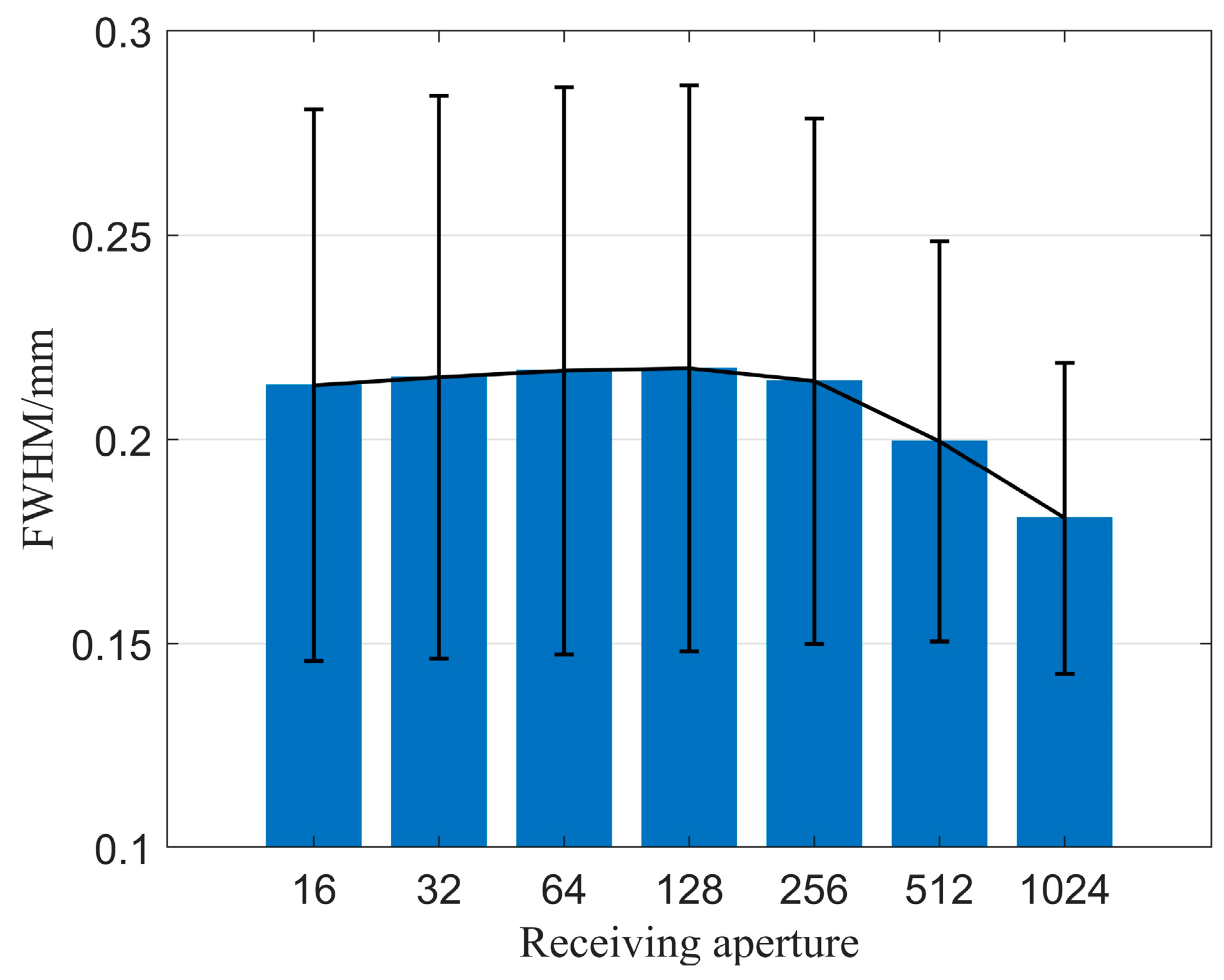
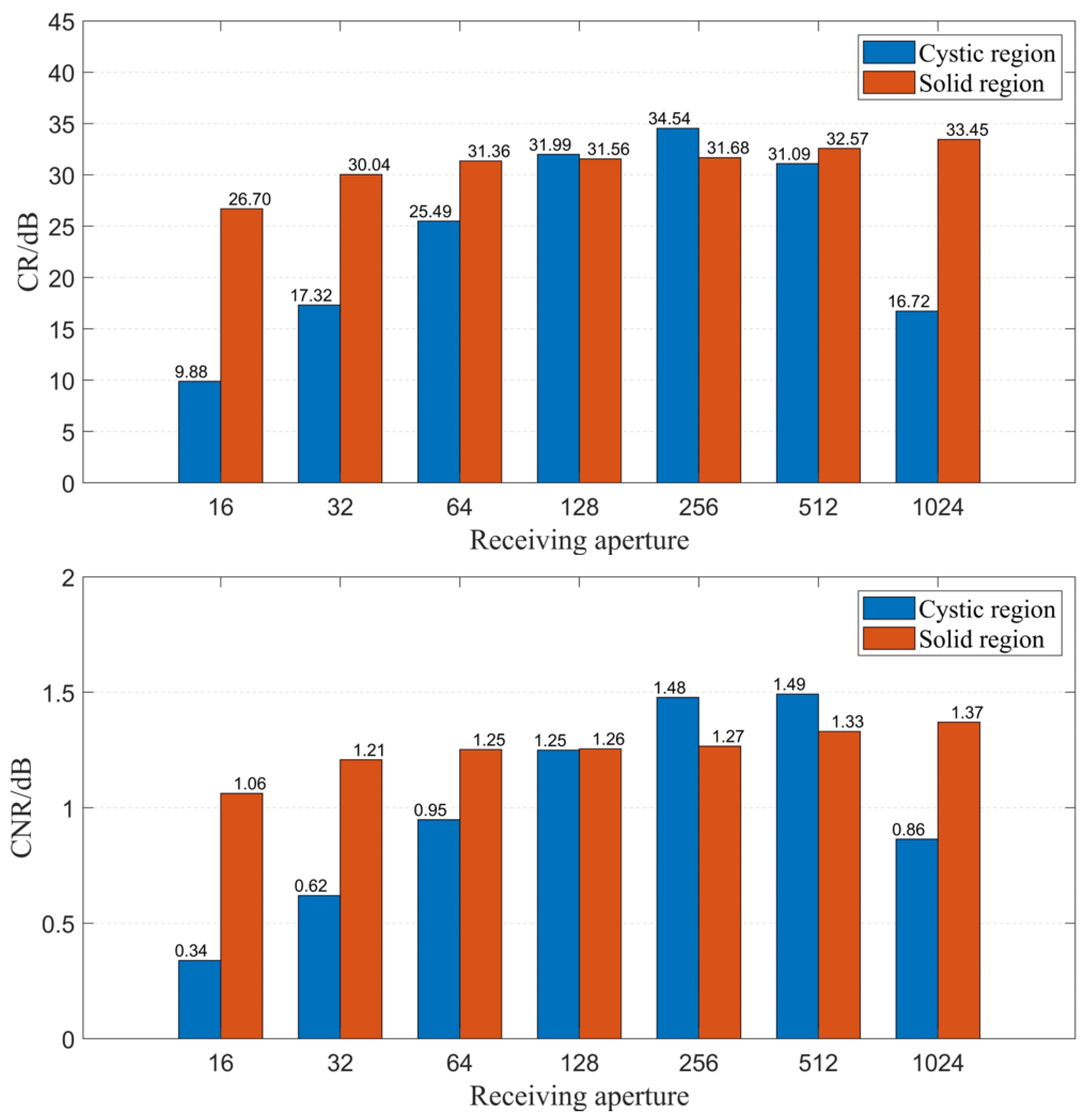
5.2. Imaging Parameters Affecting Simulation
5.3. Resolution and Contrast Comparison in Phantom Experiments
6. Discussion
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sung, H.; Ferlay, J.; Siegel, R.L.; Laversanne, M.; Soerjomataram, I.; Jemal, A.; Bray, F. Global cancer statistics 2020: GLOBOCAN estimates of incidence and mortality worldwide for 36 cancers in 185 countries. CA Cancer J. Clin. 2021, 71, 209–249. [Google Scholar] [CrossRef] [PubMed]
- Welch, H.G.; Schwartz, L.M.; Woloshin, S. Are Increasing 5-Year Survival Rates Evidence of Success Against Cancer? JAMA J. Am. Med. Assoc. (Chin. Ed.) 2000, 283, 2975–2978. [Google Scholar] [CrossRef] [PubMed]
- Huo, J.; Chen, W.; Li, N.; Shen, H.; Li, J.; Wang, Y.; Li, J.; Tian, J.; Zhou, B. Guidelines for Breast Cancer Screening, Early Diagnosis, and Early Treatment in Chinese Women (2021, Beijing). Chin. J. Oncol. 2021, 30, 161–191. [Google Scholar]
- Yaffe, M.J.; Mittmann, N.; Alagoz, O.; Trentham-Dietz, A.; Tosteson, A.N.; Stout, N.K. The effect of mammography screening regimen on incidence-based breast cancer mortality. J. Med. Screen. 2018, 25, 197–204. [Google Scholar] [CrossRef] [PubMed]
- Yao, Z.; Fan, S.; Nakajima, Y.; Qu, X. A Combined Regularization Method Using Prior Structural Information for Sound-speed Image Reconstruction of Ultrasound Computed Tomography. IEEE Access 2020, 8, 106832–106842. [Google Scholar] [CrossRef]
- Qu, X.; Yao, Z.; Tian, W.; Sun, J. Study on phase correction for USCT echo image by sound-speed image with different resolutions and noise levels. IEEE Access 2019, 9, 150657–150666. [Google Scholar] [CrossRef]
- Qu, X.; Fan, S.; Yao, Z.; Gao, S.; Sun, J.; Zhang, S.; Azuma, T. The effect of sound-speed-image resolution on phase aberration correction for ultrasound computed tomography. In Proceedings of the IEEE International Conference on Imaging Systems and Techniques (IST), Krakow, Poland, 16–18 October 2018. [Google Scholar]
- Duric, N.; Littrup, P.; Poulo, L.; Babkin, A.; Pevzner, R.; Holsapple, E.; Rama, O.; Glide, C. Detection of breast cancer with ultrasound tomography: First results with the Computed Ultrasound Risk Evaluation (CURE) prototype. Med. Phys. 2007, 34, 773–785. [Google Scholar] [CrossRef] [PubMed]
- Sree, S.V.; Ng EY, K.; Acharya, R.U.; Acharya, R.U.; Faust, O. Breast imaging: A survey. World J. Clin. Oncol. 2011, 2, 171. [Google Scholar] [CrossRef]
- Park, C.; Trumpour, T.; Aziz, A.; Bax, J.S.; Tessier, D.; Gardi, L.; Fenster, A. Cost-effective, portable, patient-dedicated three-dimensional automated breast ultrasound for point of care breast cancer screening. Sci. Rep. 2023, 13, 14390. [Google Scholar] [CrossRef]
- Luccas, V.; Azimbagirad, M.; Cabrelli, L.; Grillo, F.W.; Pavan, T.Z.; Otavio Murta, L., Jr.; Elias, J., Jr.; Carneiro, A.A.O. 3D automated breast ultrasound imaging: Equipment and image reconstruction. Res. Biomed. Eng. 2021, 37, 519–533. [Google Scholar] [CrossRef]
- Hendriks, G.; Chen, C.; Mann, R.; Hansen, H.H.; de Korte, C.L. Automated 3-D Ultrasound Elastography of the Breast: An in vivo Validation Study. Ultrasound Med. Biol. 2024, 50, 358–363. [Google Scholar] [CrossRef] [PubMed]
- Greenleaf, J.F.; Johnson, S.A.; Lent, A.H. Measurement of spatial distribution of refractive index in tissues by ultrasonic computer assisted tomography. Ultrasound Med. Biol. 1978, 3, 327–339. [Google Scholar] [CrossRef] [PubMed]
- Robinson, B.S.; Greenleaf, J.F. The scattering of ultrasound by cylinders: Implications for diffraction tomography. J. Acoust. Soc. Am. 1986, 80, 40–49. [Google Scholar] [CrossRef] [PubMed]
- Schmidt, S.; Duric, N.; Li, C.; Roy, O.; Huang, Z.-F. Modification of Kirchhoff migration with variable sound speed and attenuation for acoustic imaging of media and application to tomographic imaging of the breast. Med. Phys. 2011, 38, 998–1007. [Google Scholar] [CrossRef] [PubMed]
- Gemmeke, H.; Zapf, M.; Hopp, T.; Ruiter, N.V. Optimization of the aperture and transducers of a three-dimensional ultrasound computer tomography system. J. Acoust. Soc. Am. 2014, 135, 2178. [Google Scholar] [CrossRef]
- Hopp, T.; Zapf, M.; Gemmeke, H.; Ruiter, N.V. Experimental evaluation of straight ray and bent ray phase aberration correction for USCT SAFT imaging. In Proceedings of the Medical Imaging 2018: Ultrasonic Imaging and Tomography, Houston, TX, USA, 14–15 February 2018; Volume 10580, pp. 136–148. [Google Scholar]
- Hua, S.; Ding, M.; Weichi, M. Research on Ultrasound Inverse Scattering Imaging Based on Compressed Sensing. Beijing Biomed. Eng. 2015, 34, 24–31+37. [Google Scholar]
- Wang, S.; Song, J.; Peng, Y.; Zhou, L.; Ding, M.; Yuchi, M. Ultrasound computed tomography by frequency-shift low-pass filtering and least mean square adaptive filtering. In Proceedings of the Medical Imaging 2017: Ultrasonic Imaging and Tomography, Orlando, FL, USA, 15–16 February 2017; Volume 10139, pp. 40–46. [Google Scholar]
- Qu, X.; Azuma, T.; Yogi, T.; Azuma, S.; Takeuchi, H.; Tamano, S.; Takagi, S. Synthetic aperture ultrasound imaging with a ring transducer array: Preliminary ex vivo results. J. Med. Ultrason. 2016, 43, 461–471. [Google Scholar] [CrossRef]
- Stotzka, R.; Wuerfel, J.; Mueller, T.O.; Gemmeke, H. Medical imaging by ultrasound computer tomography. In Proceedings of the Medical Imaging 2002: Ultrasonic Imaging and Signal Processing, San Diego, CA, USA, 23–28 February 2002. [Google Scholar]
- Yang, M.; Gao, Y.; Tang, L.; Hou, J.; Hu, B. Wearable Eye-Tracking System for Synchronized Multimodal Data Acquisition. In IEEE Transactions on Circuits and Systems for Video Technology; IEEE: Piscataway, NJ, USA, 2023. [Google Scholar] [CrossRef]
- Treeby, B.E.; Cox, B.T. k-Wave: MATLAB toolbox for the simulation and reconstruction of photoacoustic wave fields. J. Biomed. Opt. 2010, 15, 021314. [Google Scholar] [CrossRef]
- Treeby, B.E.; Jaros, J.; Rendell, A.P.; Cox, B.T. Modeling nonlinear ultrasound propagation in heterogeneous media with power law absorption using ak-space pseudospectral method. J. Acoust. Soc. Am. 2012, 131, 4324–4336. [Google Scholar] [CrossRef]
- Treeby, B.E.; Jaros, J.; Rohrbach, D.; Cox, B.T. Modelling elastic wave propagation using the k-wave matlab toolbox. In Proceedings of the 2014 IEEE International Ultrasonics Symposium, Chicago, IL, USA, 3–6 September 2014; pp. 146–149. [Google Scholar]
- Treeby, B.E.; Budisky, J.; Wise, E.S.; Jaros, J.; Cox, B.T. Rapid calculation of acoustic fields from arbitrary continuous-wave sources. J. Acoust. Soc. Am. 2018, 143, 529–537. [Google Scholar] [CrossRef]
- Martin, E.; Jaros, J.; Treeby, B. Experimental validation of k-Wave: Nonlinear Wave Propagation in Layered, Absorbing Fluid Media. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2020, 67, 81–91. [Google Scholar] [CrossRef] [PubMed]
- Treeby, B.; Wise, E.; Kuklis, F.; Jaros, J.; Cox, B.T. Nonlinear Ultrasound Simulation in an Axisymmetric Coordinate System using k-space pseudospectral method. J. Acoust. Soc. Am. 2020, 148, 2288–2300. [Google Scholar] [CrossRef] [PubMed]
- Treeby, B. Modeling Nonlinear Wave Propagation on Nonuniform Grids using a Mapped k-Space Pseudospectral Method. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2013, 60, 2208–2213. [Google Scholar] [CrossRef] [PubMed]
- Firouzi, K.; Cox, B.; Treeby, B.; Saffari, N. A first-order k-space model for elastic wave propagation in heterogeneous media. J. Acoust. Soc. Am. 2012, 132, 1271–1283. [Google Scholar] [CrossRef] [PubMed]
- Treeby, B.; Cox, B. A k-space Green’s function solution for acoustic initial value problems in homogeneous media with power law absorption. J. Acoust. Soc. Am. 2011, 129, 3652–3660. [Google Scholar] [CrossRef] [PubMed]
- Cox, B.; Kasa, S.; Arridge, S.; Beard, P. k-space propagation models for acoustically heterogeneous media: Application to biomedical photoacoustics. J. Acoust. Soc. Am. 2007, 121, 3453–3464. [Google Scholar] [CrossRef]
- Alomariz, R.S. Plane Wave Imaging Beamforming Techniques for Medical Ultrasound Imaging; University of Leeds: Leeds, UK, 2017. [Google Scholar]
- Tao, Y.; Yang, M.; Li, H.; Wu, Y.; Hu, B. DepMSTAT: Multimodal Spatio-Temporal Attentional Transformer for Depression Detection. In IEEE Transactions on Knowledge and Data Engineering; IEEE: Piscataway, NJ, USA, 2024. [Google Scholar] [CrossRef]
- Yang, M.; Weng, Z.; Zhang, Y.; Tao, Y.; Hu, B. Three-Stream Convolutional Neural Network for Depression Detection with Ocular Imaging. IEEE Trans. Neural Syst. Rehabil. Eng. 2023, 31, 4921–4930. [Google Scholar] [CrossRef]


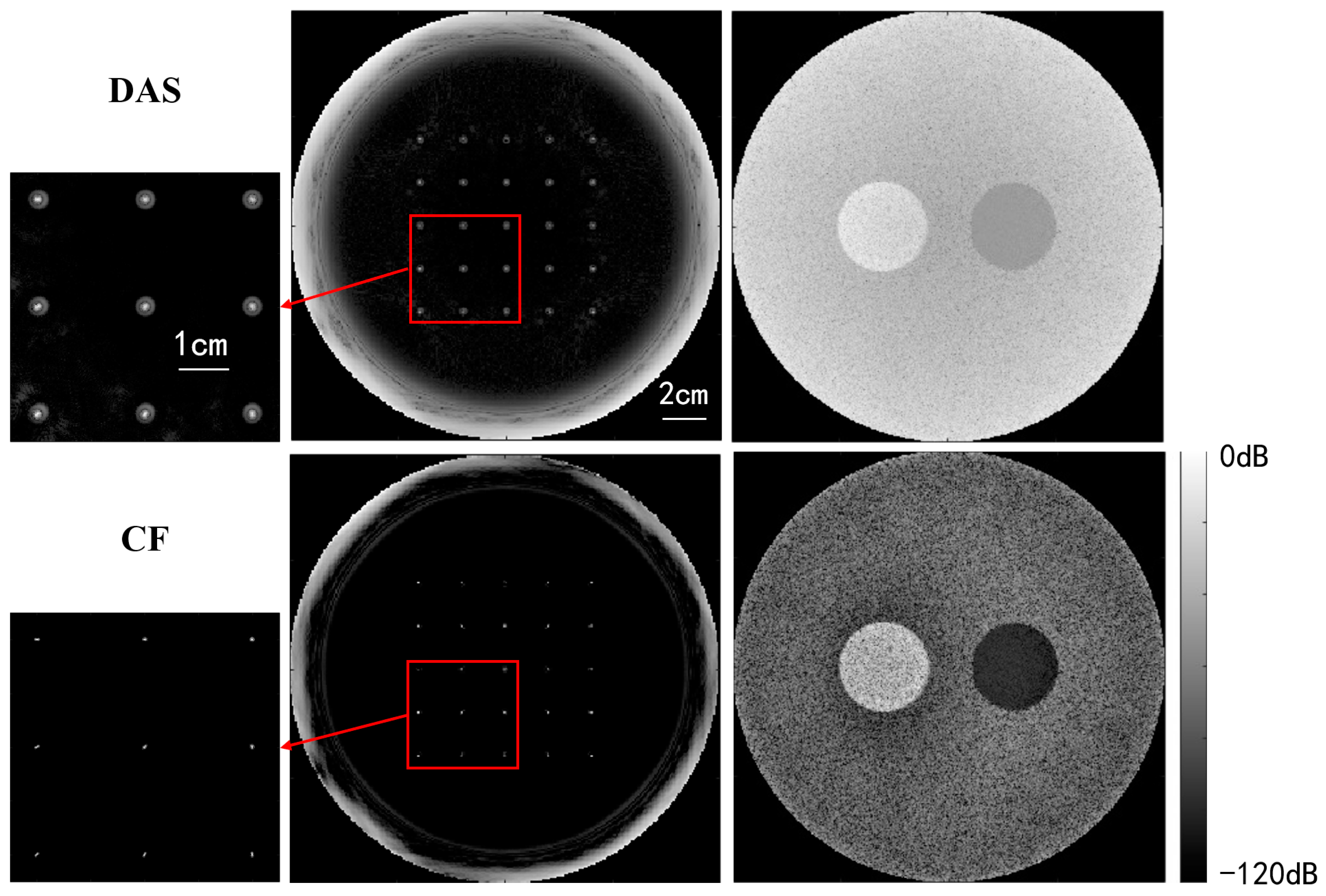
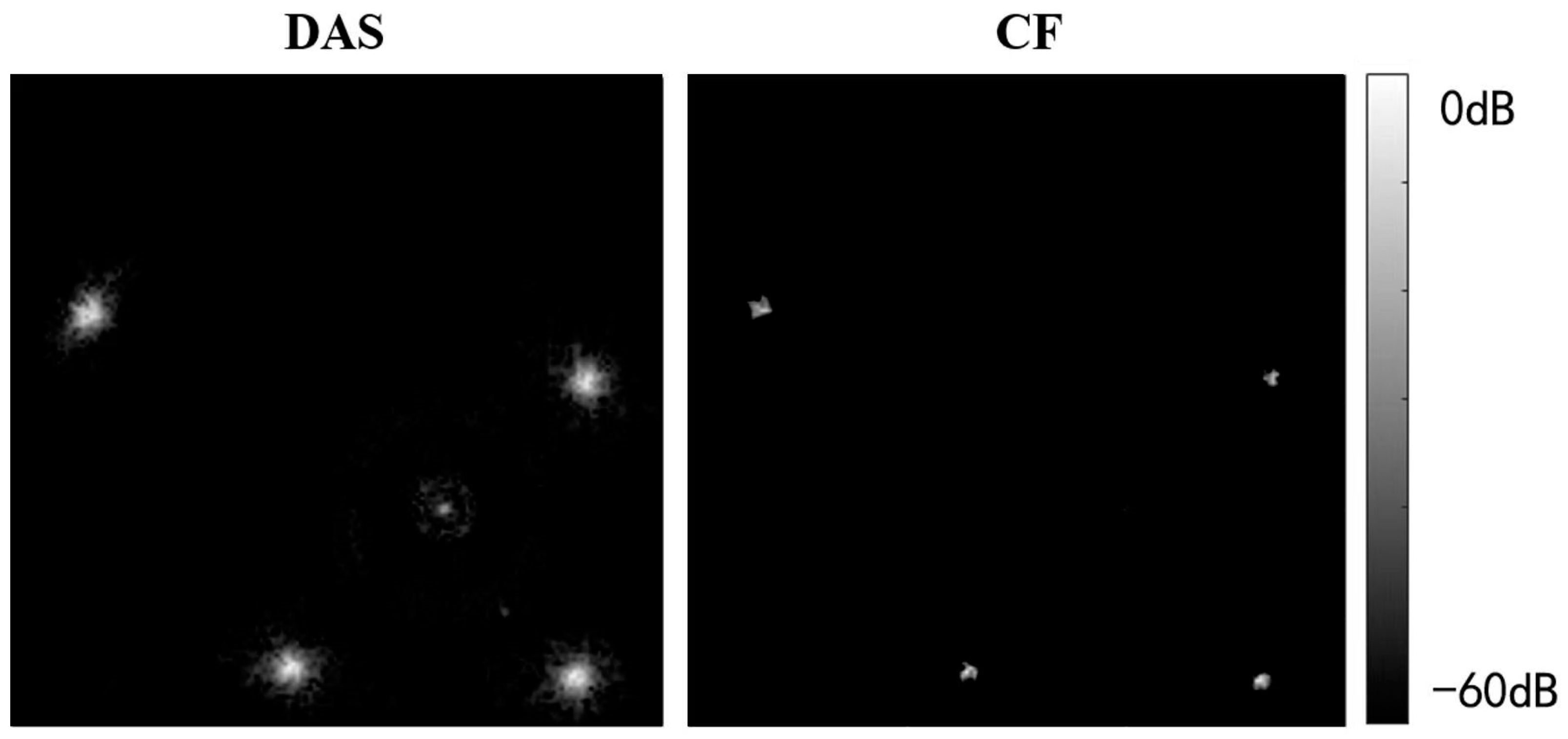

| Method | FWHM | CR | CNR | ||
|---|---|---|---|---|---|
| Cystic Region | Solid Region | Cystic Region | Solid Region | ||
| DAS | 0.372 | 6.57 | 15.3 | 0.78 | 1.49 |
| CF | 0.199 | 31.1 | 32.6 | 1.58 | 1.33 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hou, Z.; Yuan, R.; Wang, Z.; Wei, X.; Ren, C.; Zhou, J.; Qu, X. Ultrasound Computed Tomography Reflection Imaging with Coherence-Factor Beamforming for Breast Tumor Early Detection. Mathematics 2024, 12, 1106. https://doi.org/10.3390/math12071106
Hou Z, Yuan R, Wang Z, Wei X, Ren C, Zhou J, Qu X. Ultrasound Computed Tomography Reflection Imaging with Coherence-Factor Beamforming for Breast Tumor Early Detection. Mathematics. 2024; 12(7):1106. https://doi.org/10.3390/math12071106
Chicago/Turabian StyleHou, Zuoxun, Ruichen Yuan, Zihao Wang, Xiaorui Wei, Chujian Ren, Jiale Zhou, and Xiaolei Qu. 2024. "Ultrasound Computed Tomography Reflection Imaging with Coherence-Factor Beamforming for Breast Tumor Early Detection" Mathematics 12, no. 7: 1106. https://doi.org/10.3390/math12071106
APA StyleHou, Z., Yuan, R., Wang, Z., Wei, X., Ren, C., Zhou, J., & Qu, X. (2024). Ultrasound Computed Tomography Reflection Imaging with Coherence-Factor Beamforming for Breast Tumor Early Detection. Mathematics, 12(7), 1106. https://doi.org/10.3390/math12071106






