Queuing-Inventory System with Catastrophes in the Warehouse: Case of Rare Catastrophes
Abstract
1. Introduction
- Exact and approximate methods to study a QIS model with finite waiting rooms are developed.
- High-accuracy, closed-form, approximate formulas for calculating the steady-state probabilities and performance measures of the investigated QIS in the case of rare catastrophes are developed.
- By using developed approximate closed-form formulas, the performance measures of large-scale QISs are calculated and the expected total cost (ETC) is minimized by choosing the optimal value of reorder point.
2. The Model
3. Steady-State Analysis
3.1. An Exact Approach
- Case 1: When , the following is true:
- Case 2: When , the following is true:
- Case 3: When , the following is true:
- Case 4: When , the following is true:
- The mean number of items in the warehouse (i.e., the average inventory level) is calculated as a mathematical expectation of the appropriate random variable and is given by the following:
- Similar to (8), the average order size (i.e., the average size of replenished items from external source) is calculated as a mathematical expectation of the appropriate random variable and is calculated as follows:
- An inventory order is placed in two cases: (1) if the inventory level drops to the re-order point after completing customer service in states , or (2) if catastrophes occur in the states Therefore, the average reorder intensity is calculated as follows:
- The mean length of the queue is calculated as a mathematical expectation (an average value) of the appropriate random variable and is given by the following:
- Losing c-customers occurs in three cases: (1) if, at the time the c-customer arrives, the waiting room is full (with probability 1), i.e., the system is in one of the states , ; (2) if, at the time the c-customer arrives, the inventory level is zero and the waiting room is not full (with probability ), i.e., the system is in one of the states , (3) when an n-customer arrives, it displaces one c-customer. Therefore, the loss rate of c-customers is calculated as follows:
3.2. An Approximate Approach
- Case
- Case
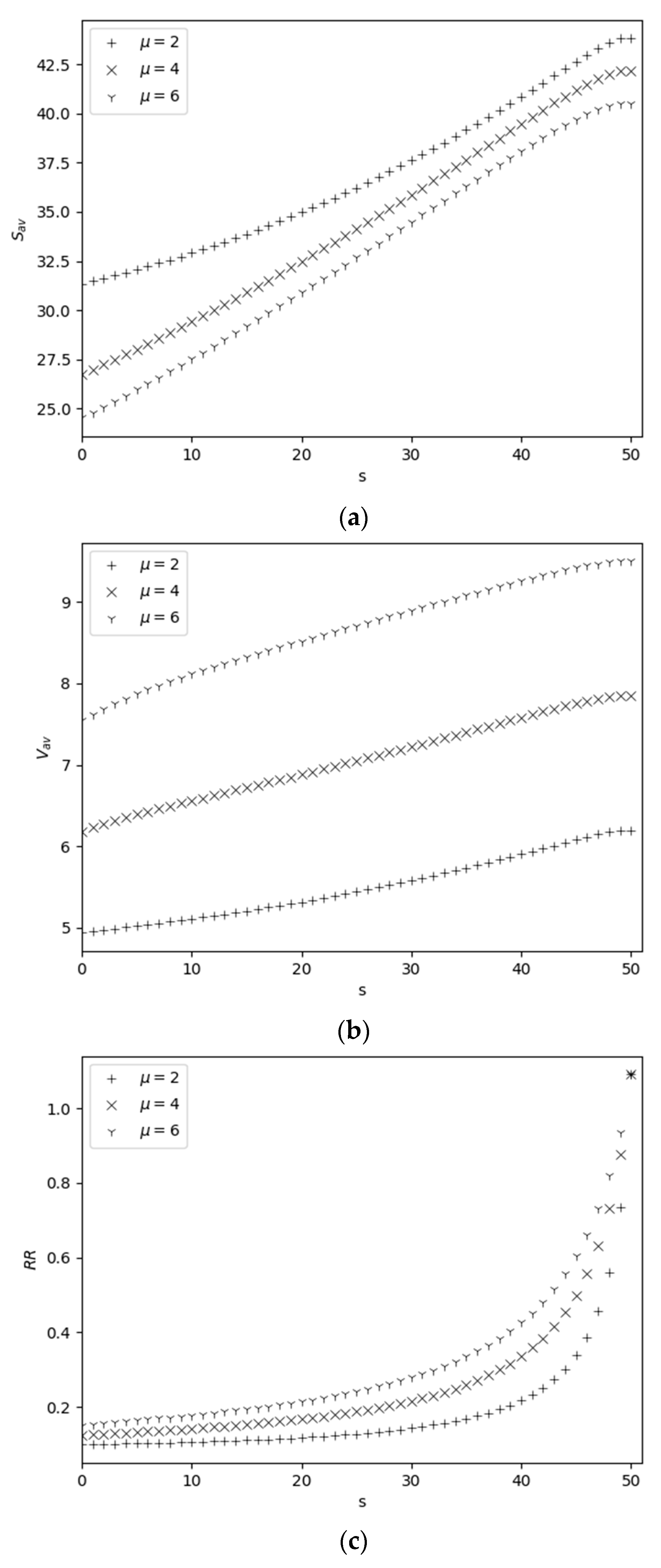
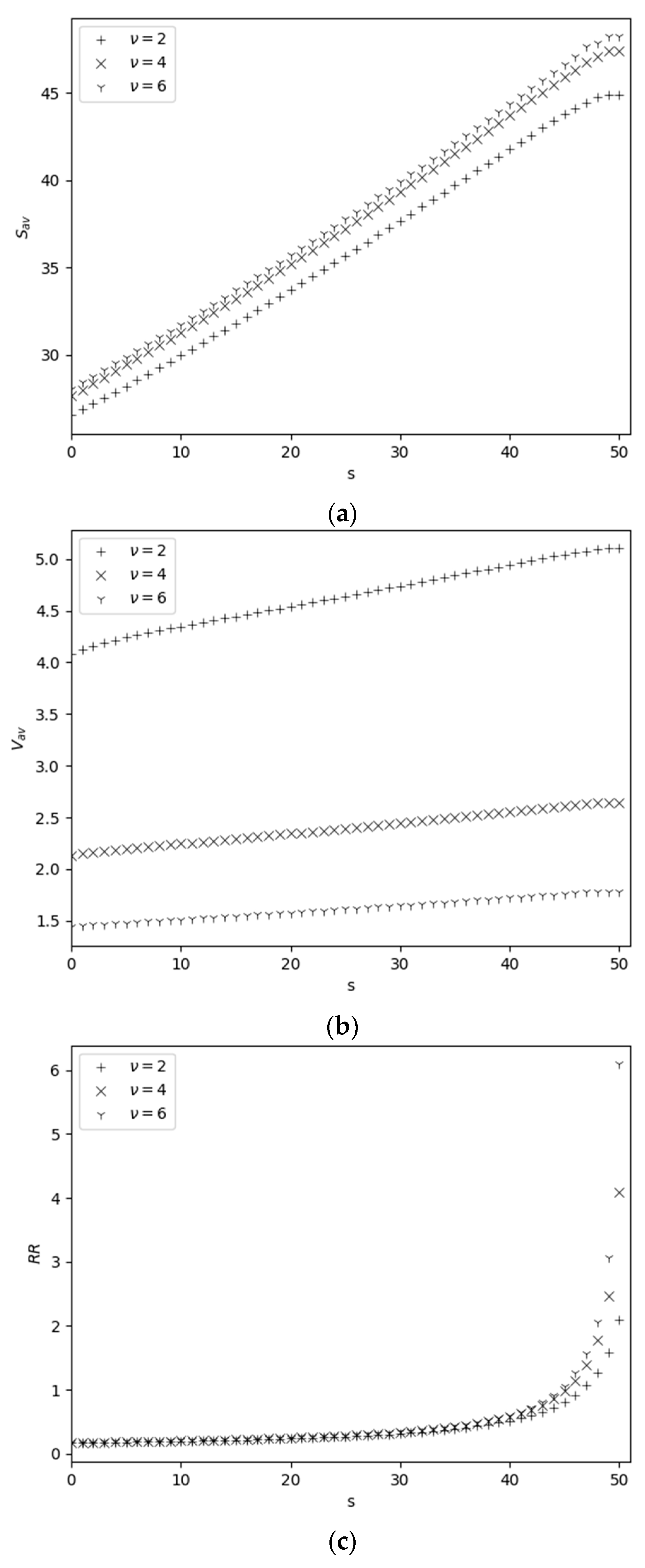
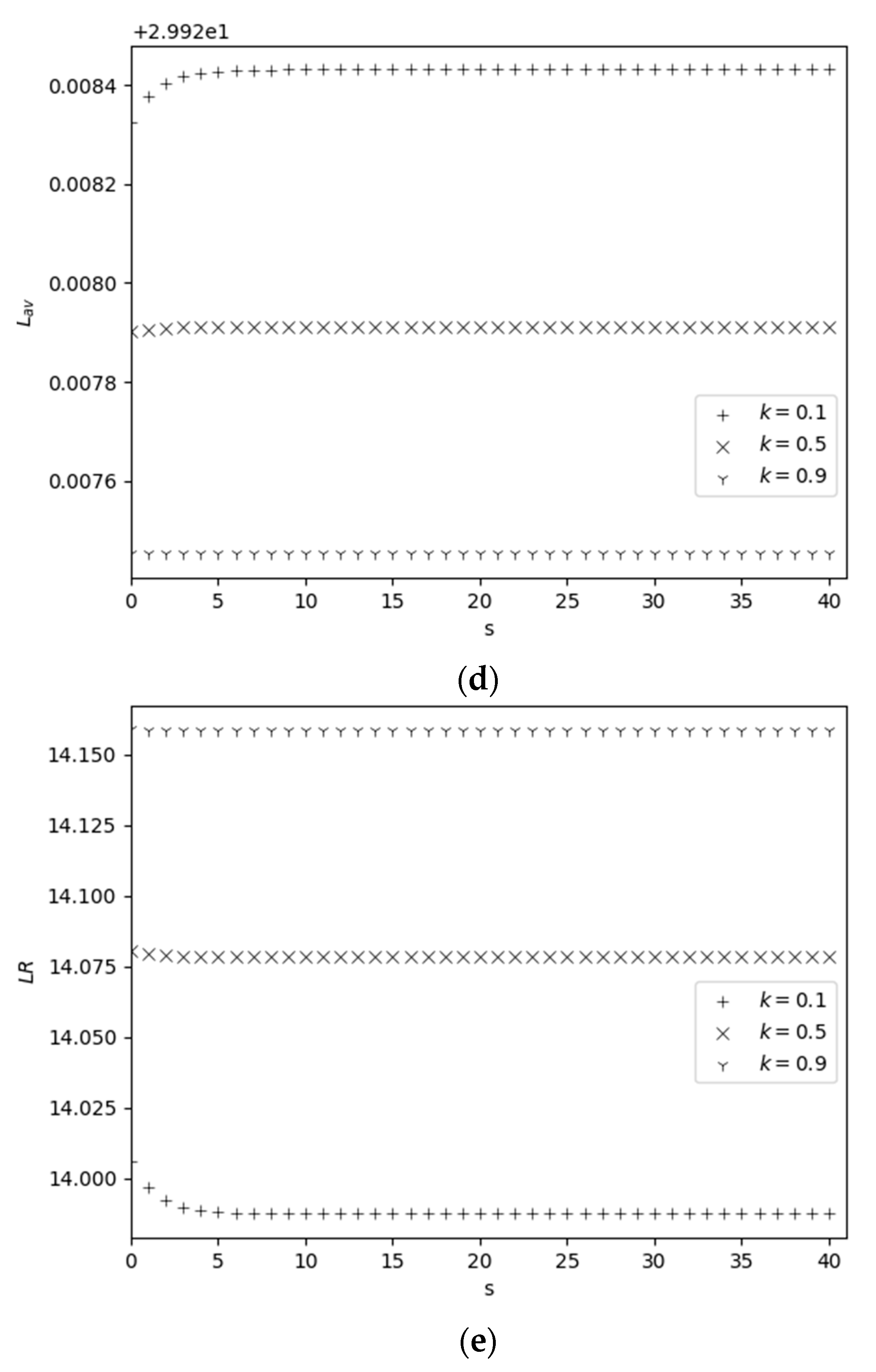
4. Numerical Experiments
4.1. Accuracy of the Developed Approximate Formulas
4.2. Behavior of Performance Measures versus Reorder Point
4.3. Optimization Problem
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chakravarthy, S.R. A catastrophic queueing model with delayed action. Appl. Math. Model. 2017, 46, 631–649. [Google Scholar] [CrossRef]
- Kumar, D.; Singh, B.G. Transient Solution of a Two Homogeneous Servers Markovian Queueing System with Environmental, Catastrophic and Restoration Effects. Int. J. Math. Stat. Stud. 2024, 12, 45–53. [Google Scholar] [CrossRef]
- Demircioglu, M.; Bruneel, H.; Wittevrongel, S. Analysis of a Discrete-Time Queueing Model with Disasters. Mathematics 2021, 9, 3283. [Google Scholar] [CrossRef]
- Kumar, B.K.; Arivudainambi, D. Transient solution of an M/M/1 queue with catastrophes. Comput. Math. Appl. 2000, 40, 1233–1240. [Google Scholar] [CrossRef]
- Jain, N.K.; Rakesh, K. Transient solution of a catastrophic-cum-restorative queuing problem with correlated arrivals and variable service capacity. Int. J. Inform. Manag. Sci. 2007, 18, 461–465. [Google Scholar]
- Jain, N.K.; Singh, B.G. A queue with varying catastrophic intensity. Int. J. Comput. Appl. Math 2010, 5, 41–46. [Google Scholar]
- Singh, B.G.; Niwas, B.R. Time-dependent analysis of a queueing system incorporating the effect of environment, catastrophe, and restoration. J. Reliab. Stat. Stud. 2015, 8, 29–40. [Google Scholar]
- Krishnamoorthy, A.; Joshua, A.N.; Mathew, A.P. The k-out-of-n: G System Viewed as a Multi-Server Queue. Mathematics 2024, 12, 210. [Google Scholar] [CrossRef]
- Rykov, V.; Kochueva, O.; Farkhadov, M. Preventive Maintenance of a k-out-of-n System with Applications in Subsea Pipeline Monitoring. J. Mar. Sci. Eng. 2021, 9, 85. [Google Scholar] [CrossRef]
- Melikov, A.; Mirzayev, R.R.; Nair, S.S. Numerical investigation of double source queuing-inventory systems with destructive customers. J. Comput. Syst. Sci. Int. 2022, 61, 581–598. [Google Scholar] [CrossRef]
- Melikov, A.; Poladova, L.; Edayapurath, S.; Sztrik, J. Single-Server Queuing-Inventory Systems with Negative Customers and Catastrophes in the Warehouse. Mathematics 2023, 11, 2380. [Google Scholar] [CrossRef]
- Neuts, M.F. Matrix-Geometric Solutions in Stochastic Models: An Algorithmic Approach; The Johns Hopkins University Press: Baltimore, MD, USA, 1981. [Google Scholar]
- Chakravarthy, S.R. Introduction to Matrix-Analytic Methods in Queues 1—Basics; John Wiley & Sons, Inc.: London, UK, 2022; Volume 1. [Google Scholar]
- Chakravarthy, S.R. Introduction to Matrix-Analytic Methods in Queues 2—Queues and Simulation; John Wiley & Sons, Inc.: London, UK, 2022; Volume 2. [Google Scholar]
- Dudin, A.N.; Klimenok, V.I.; Vishnevsky, V.M. The Theory of Queueing Systems with Correlated Flows; Springer: Basel, Switzerland, 2020. [Google Scholar]
- Amirthakodi, M.; Sivakumar, B. An inventory system with service facility and feedback customers. Int. J. Ind. Syst. Eng. 2019, 33, 374–411. [Google Scholar] [CrossRef]
- Amirthakodi, M.; Sivakumar, B. An inventory system with a service facility and finite orbit size for feedback customers. OPSEARCH 2015, 52, 225–255. [Google Scholar] [CrossRef]
- Amirthakodi, M.; Radhamani, V.; Sivakumar, B. A perishable inventory system with service facility and feedback customers. Ann Oper Res. 2015, 233, 25–55. [Google Scholar] [CrossRef]
- Sivakumar, B.; Elango, C.; Arivarignan, G. A Perishable Inventory System with Service Facilities and Batch Markovian Demands. Int. J. Pure Appl. Math. 2006, 32, 33–49. [Google Scholar]
- Devi, P.C.; Sivakumar, B.; Krishnamoorthy, A. Optimal Control Policy of an Inventory System with Postponed Demand. RAIRO-Oper. Res. 2016, 50, 145–155. [Google Scholar] [CrossRef]
- Varghese, D.T.; Shajin, D. State Dependent Admission of Demands in a Finite Storage System. Int. J. Pure Appl. Math. 2018, 118, 917–922. [Google Scholar]
- Jenifer, J.S.A.; Sangeetha, N.; Sivakumar, B. Optimal Control of Service Parameter for a Perishable Inventory System with Service Facility, Postponed Demands and Finite Waiting Hall. Int. J. Inform. Manag. Sci. 2014, 25, 349–370. [Google Scholar]
- Melikov, A.; Mirzayev, R.R.; Sztrik, J. Double Sources QIS with Finite Waiting Room and Destructible Stocks. Mathematics 2023, 11, 226. [Google Scholar] [CrossRef]
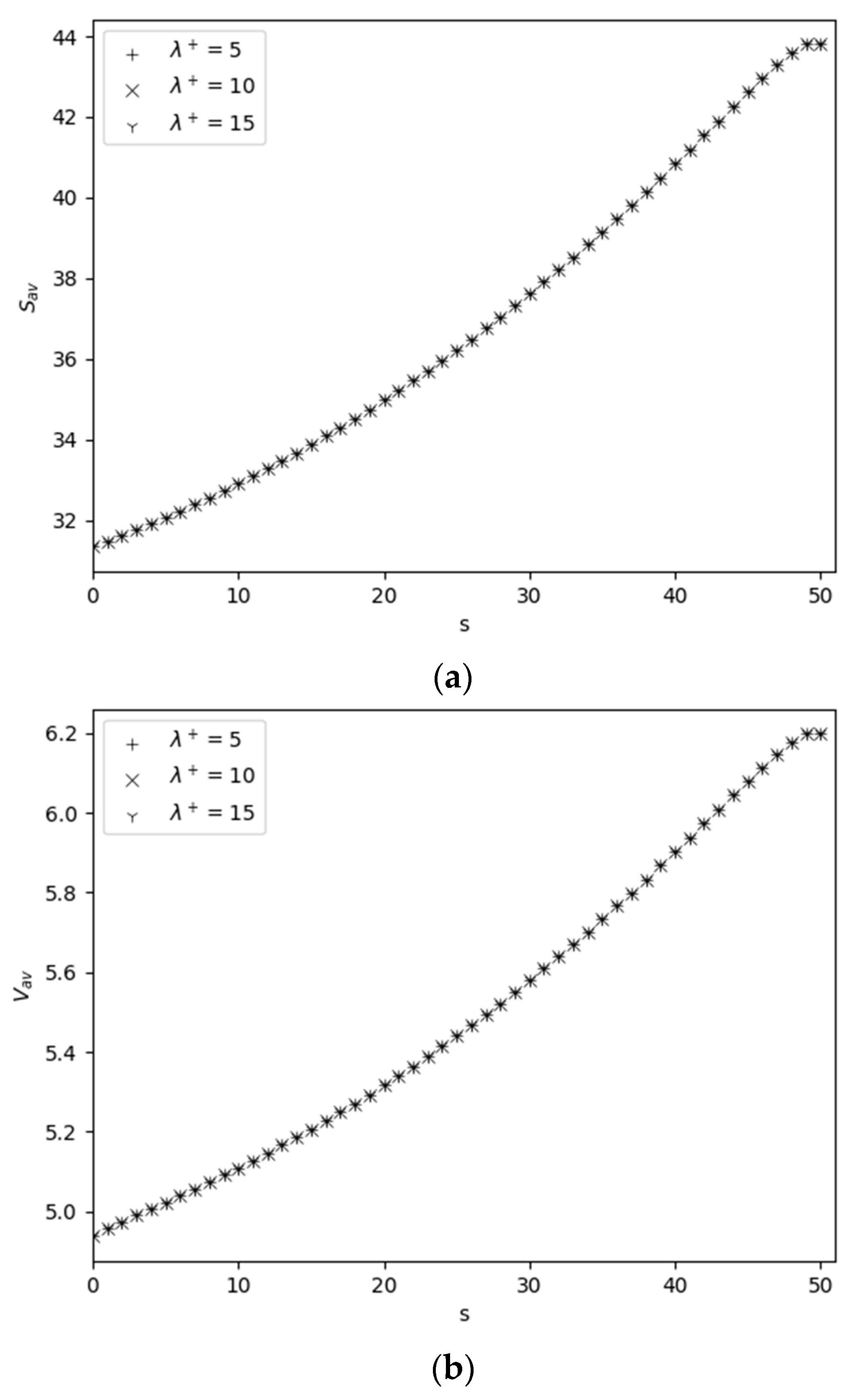
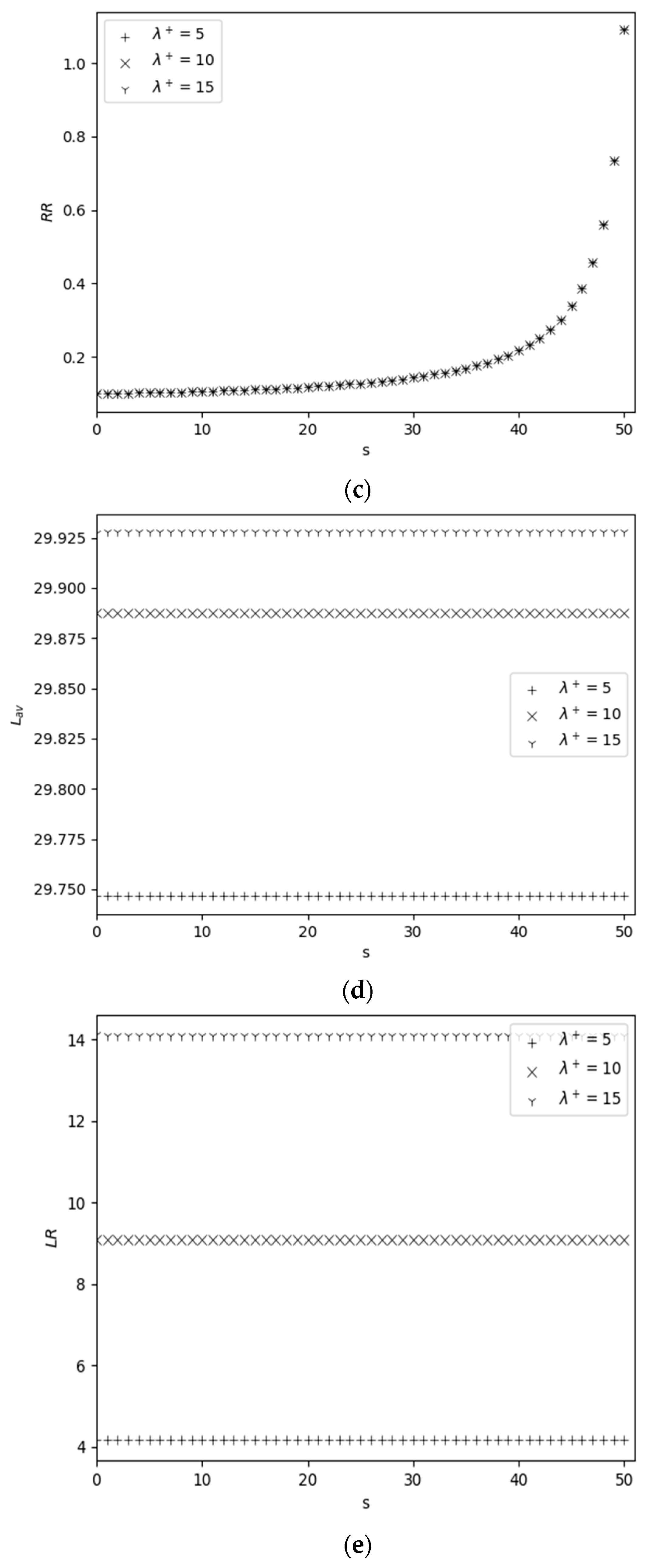
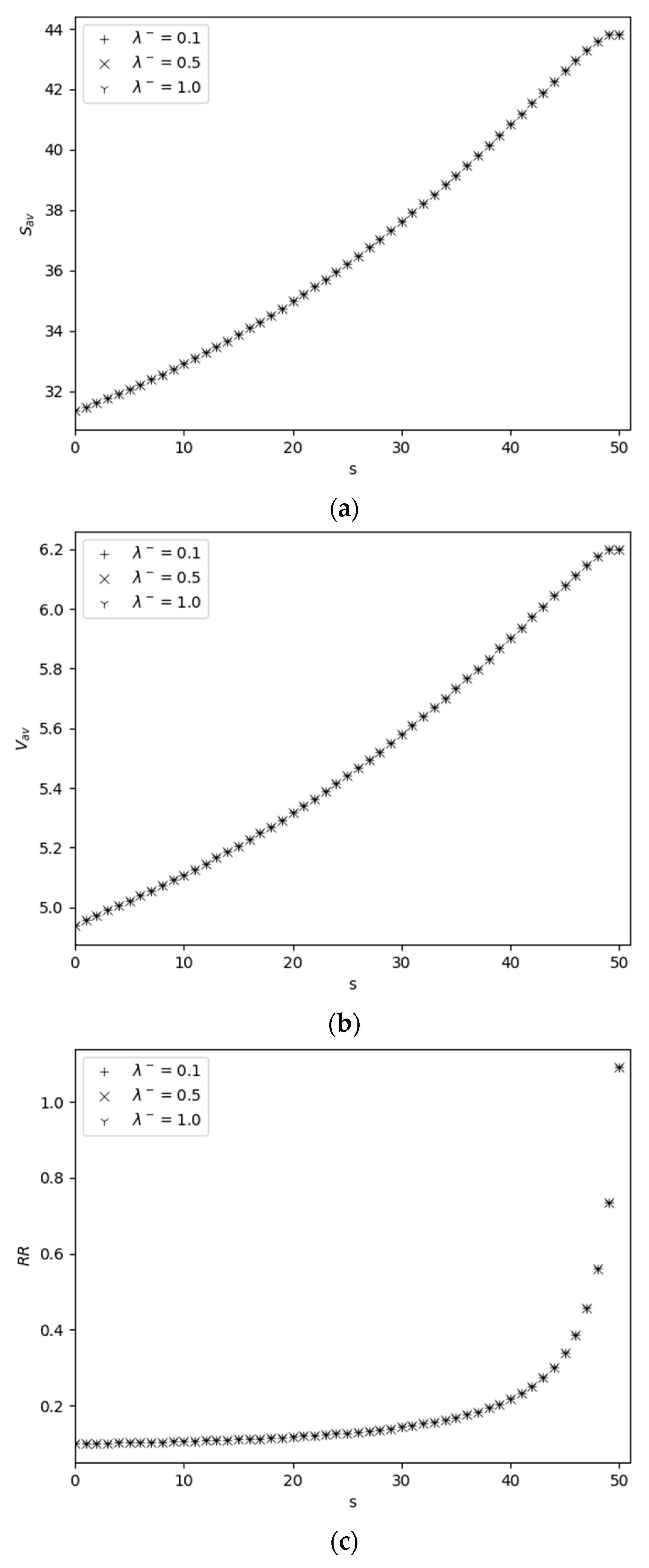
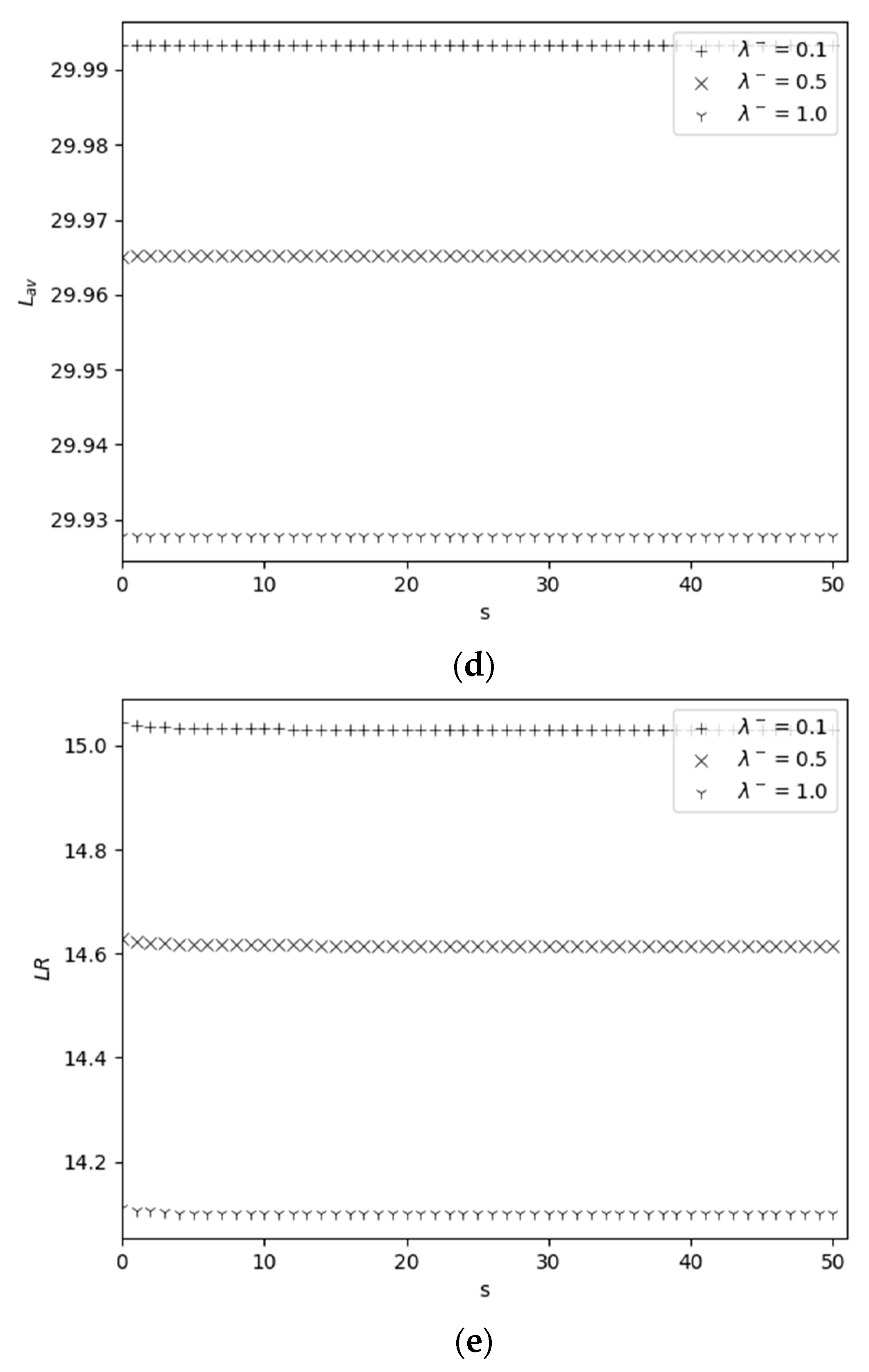



| Max of Error for SSPs | Error for | |||||
|---|---|---|---|---|---|---|
| 0 | ||||||
| 5 | ||||||
| 10 | ||||||
| 15 | ||||||
| 20 | ||||||
| 25 | ||||||
| 30 | ||||||
| 35 | ||||||
| 40 | ||||||
| 45 | ||||||
| 50 | 28.07176 | 1.439081 | 0.172690 | 29.92832 | 13.98755 | 18,981.34 |
| 55 | 31.23721 | 1.493466 | 0.162924 | 29.92834 | 13.98755 | 19,059.21 |
| 60 | 34.46487 | 1.548604 | 0.154860 | 29.92835 | 13.98755 | 19,138.86 |
| 65 | 37.75379 | 1.604545 | 0.148112 | 29.92836 | 13.98755 | 19,220.23 |
| 70 | 41.10292 | 1.661316 | 0.142398 | 29.92837 | 13.98755 | 19,303.25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Melikov, A.; Poladova, L.; Sztrik, J. Queuing-Inventory System with Catastrophes in the Warehouse: Case of Rare Catastrophes. Mathematics 2024, 12, 906. https://doi.org/10.3390/math12060906
Melikov A, Poladova L, Sztrik J. Queuing-Inventory System with Catastrophes in the Warehouse: Case of Rare Catastrophes. Mathematics. 2024; 12(6):906. https://doi.org/10.3390/math12060906
Chicago/Turabian StyleMelikov, Agassi, Laman Poladova, and Janos Sztrik. 2024. "Queuing-Inventory System with Catastrophes in the Warehouse: Case of Rare Catastrophes" Mathematics 12, no. 6: 906. https://doi.org/10.3390/math12060906
APA StyleMelikov, A., Poladova, L., & Sztrik, J. (2024). Queuing-Inventory System with Catastrophes in the Warehouse: Case of Rare Catastrophes. Mathematics, 12(6), 906. https://doi.org/10.3390/math12060906







