1. Introduction
The theory of
q-calculus emerged as a natural extension of traditional calculus, leading to the development of a rich and powerful mathematical framework. Over the years, it has proven to be an invaluable tool in various scientific fields, including mathematics, quantum physics, mechanics, algebra, approximation theory, and operator theory [
1,
2,
3]. Initially formulated by Jackson et al. [
4], the
q-calculus structure has undergone significant evolution, with contributions from numerous researchers in the field; see, for example, [
5,
6,
7,
8]. The introduction of
q-calculus paved the way for exploring
q-analogues of classical functions, allowing for new insights and applications in different areas of study.
The concepts and terminology associated with
q-calculus have been extensively explored in works by Andrews, Gasper, and Ernst, among others [
9,
10,
11]. These
q-analogues are crucial in studying and generalizing classical special functions, leading to a deeper understanding of their behavior in various contexts. The recent surge in research surrounding
q-special polynomials has further underscored their significance in theoretical and applied mathematics.
In particular, the study of
q-Appell polynomials [
12], a specialized class of polynomials, has garnered attention due to their versatility in numerous applications. Recent developments have also focused on the
q-truncated exponential polynomials [
13], which have been studied for their potential in problems within quantum mechanics, optics, and other domains where products of special functions are encountered. The current work introduces a hybrid family of the 2-variable
q-truncated exponential–Appell polynomials, offering new insights into their structure and applications.
This paper aims to provide a comprehensive exploration of these polynomials, presenting their series representation, addition and recursive formulas, q-recurrence relation, and integral representation, among other key properties. The results presented herein aim to further our understanding of the role of these polynomials in modern mathematical analysis and their practical applications.
This article comprises five sections.
Section 2 outlines the preliminaries, which include fundamental concepts of
q-calculus and related definitions.
Section 3 introduces the 2-variable
q-trunc. exp. Appell polynomials, detailing their series representation, addition formula, recursive formula,
q-recurrence relation,
q-integral representation, determinant form, and extended quasi-monomiality.
Section 4 examines specific instances within the
q-trunc. exp. Appell class. The graphical representation of these members is presented in
Appendix A. The article is concluded with an overview of the 2-variable
q-trunc. exp.
-polynomials.
2. Preliminaries
This section introduces the essential concepts and definitions that form the foundation for the study of 2-variable q-truncated exponential Appell polynomials. Key results from q-calculus are outlined below, all of which are central to the understanding of the polynomials discussed in subsequent sections.
The
q-shifted factorial
is defined by the following relation [
11]:
The
q-analogue of
is expressed as [
11]:
and the
q-factorial function is given by [
11]:
The
q-binomial coefficient
is defined as [
11]:
The exponential function has the following
q-analogue [
11]:
which, for the
q-commuting variables
and
, satisfies [
11]:
The
q-derivative, with respect to
, of a function
w is defined by [
11]:
For any two functions
and
, the following differential rules hold [
11]:
and
In particular, we have [
11]:
and
Therefore, the
order
q-derivative of the
q-exponential function is given by:
The
q-definite integral, with respect to
, of a function
w is defined as [
11]:
whereas the
q-definite integral for the
q-derivative of a function
is expressed as [
11]:
Heine’s binomial formula is given by [
14]:
We note that, as q approaches , all results in q-calculus align with those in classical calculus.
Appell polynomials, a distinguished category of polynomial sequences, were originally introduced by Appell [
15] in 1880. Al-Salam [
12] later made a significant contribution by extending these polynomials and investigating their diverse properties. The
q-Appell polynomials
are defined using the following generating equation [
12]:
where
is an analytic function at
and
.
The concept of monomiality provides a powerful method for studying the characteristics of specific special polynomials. Its origin traces back to the early 19th century when Steffensen [
16] introduced the term ‘poweroid’. Dattoli later refined and broadened this idea into quasi-monomiality [
17]. Subuhi Khan et al. have employed the principle of monomiality to propose and explore certain hybrid classes of special polynomials; see, for example [
18,
19,
20]. Very recently, the notion of monomiality has been extended for the
q-special polynomials [
21,
22].
For the
q-polynomials
, the
q-multiplicative operator
and the
q-derivative operator
satisfy the following relations [
21]:
and
respectively.
These
q-operators adhere to the following commutation and
q-differential relations [
21]:
and
or, alternatively,
respectively.
From Equations (
20)–(
22), it follows that:
Also, from Equation (
18), we observe that:
and
where
.
As a result, the generating relation for
is expressed as [
21]:
We revisit the definition of the
q-dilation operator [
23], which operates on any function
as:
and satisfies the property
.
The
q-truncated exponential polynomials (
q-TEPs) are commonly found in problems within optics and quantum mechanics, and they hold particular importance in the calculation of integrals that incorporate products of special functions. Very recently, Raza et al. [
13] studied the
q-truncated exponential polynomials. The
order
q-truncated exponential polynomials, denoted by
, are defined by the following generating function [
13]:
Motivated by the importance of q-trunc. exp. polynomials and q-Appell polynomials, a hybrid family associated with these q-special polynomials is introduced in the next section.
3. q-Truncated Exponential–Appell Polynomials
In this section, the q-trunc. exp. Appell polynomials are introduced and their properties including series definition, addition formula, recursive formula, q-recurrence relation, q-integral representation, determinant form, and extended quasi-monomiality are established.
The 2-variable trunc. exp. polynomials of order
m, denoted by
, are defined by the following generating relation [
24]:
To introduce a hybrid family of
q-special polynomials related to
q-Appell sequences, we first define the 2-variable
q-trunc. exp. polynomials (2V
q-TEP) of order
m, represented as
, using the following generating equation:
The 2-variable q-trunc. exp. polynomials play a crucial role in mathematical modeling and applied sciences. In quantum mechanics, they are used to describe interactions between two coupled quantum systems, aligning with q-deformed algebraic structures. Their applications in signal processing and optics involve analyzing two-variable dependencies, such as in wave functions and light propagation.
In view of generating Equations (
28) and (
30), we obtain the following specific cases of the 2V
q-TEP:
Now, we introduce the 2-variable q-trunc. exp. Appell polynomials (2Vq-TEAP) of order m.
Theorem 1. The 2-variable q-trunc. exp. Appell polynomials of order m, denoted by , are defined by the following generating equation: Proof. Using expansion (
5) in the
l.h.s. of Equation (
30) and then replacing powers of
,
,
, …with the correlating polynomials
,
,
, …, and summing up the terms in the resulting equation, we have
Using Equation (
16) and labeling the resultant 2V
q-TEAP in the
r.h.s. by
, assertion (
33) is obtained. □
Remark 1. For , the following consequence of Theorem (1) is deduced:
Corollary 1. For the q-trunc. exp. Appell polynomials of order m, , the following generating equation holds: Remark 2. Taking in Equation (33), the 2-variable q-trunc. exp. Appell polynomials of order m reduce to the 2-variable q-trunc. exp. polynomials (2Vq-TEP) of order m Remark 3. As , Equation (33) reduces to the 2-variable trunc. exp. Appell polynomials of order m, , which are defined by the following generating function [25]: Theorem 2. For the 2-variable q-trunc. exp. Appell polynomials , the following series representation holds true: Proof. Using Equations (
15) and (
16) in generating Equation (
33), we have
which, on applying the series rearrangement techniques and equating the corresponding powers of
on both the sides in the resultant equation, yields assertion (
36). □
Theorem 3. The 2-variable q-trunc. exp. Appell polynomials satisfy the following addition formula: Proof. Replacing
by
in generating Equation (
33), and then using Equation (
6), it follows that
Using Equations (
5) and (
33) in the
l.h.s. of the above equation, we have
which, on rearranging the series and then equating the similar powers of
on both sides, yields assertion (
37). □
Theorem 4. For the 2-variable q-trunc. exp. Appell polynomials , the following q-recurrence formula holds true: Proof. Taking the
q-partial derivative with respect to
in both sides of Equation (
17) and then rearranging the series in the
r.h.s, we have
Again, the
q-partial differentiation of Equation (
33) with respect to
gives
which, on using Equations (
8) and (
9) in the
l.h.s. and then separating each of the terms, takes the form
Utilizing Equations (
15), (
30), (
33), and (
39) in Equation (
40), it follows that
which, on rearranging the series and then equating the similar powers of
in both sides of resultant equation, yields assertion (
38). □
Theorem 5. For the 2-variable q-trunc. exp. Appell polynomials , the following q-recursive formula holds: Proof. Taking the
order
q-partial derivative with respect to
on both sides of generating Equation (
33) and then using Equation (
11) in the
l.h.s., it follows that
On rearranging the above series and then comparing the like powers of
in both sides of the resultant equation, we obtain assertion (
41). □
Corollary 2. For the 2-variable q-trunc. exp. Appell polynomials , following q-definite integral with respect to ξ holds: Proof. Taking
in formula (
41) and then performing
q-integration with respect to variable
in the resultant equation, we obtain integral (
42). □
Keleshteri and Mahmudov [
26], extending the methods of [
27,
28], derived the determinantal form of
q-Appell polynomials. Recognizing the significance of determinant representations in computational and applied contexts, the determinant representation of the 2V
q-TEAP
is established by proving the following result:
Theorem 6. For the 2-variable q-trunc. exp. Appell polynomials of order m , the following determinant expression holds:where are the 2-variable q-trunc. exp. polynomials of order m and is a numerical sequence with and given as: Proof. Consider the polynomial sequence
, defined as
such that
Using Equations (
17) and (
46) in Equation (
47), and simplifying, we find
so that Equations (
44) and (
45) hold.
Multiplying generating Equation (
33) by
and using Equation (
47), we have
which gives
where
are defined by Equation (
30).
Using the series rearrangement technique in the
r.h.s. of Equation (
49) and simplifying, the following system of equations with unknowns
, (
) is obtained:
The set of equations above constitutes a lower triangular system. Applying Cramer’s rule, we have
After transposing and making
j circular row exchanges in Equation (
51), assertion (
43) follows. □
Extending the notion of monomiality to examine various q-special polynomials is an extremely valuable technique. We will now discuss the quasi-monomiality of the 2Vq-TEAP.
Theorem 7. The 2-variable q-trunc. exp. Appell polynomials are quasi-monomial under the action of the following q-multiplicative and q-derivative operators:andrespectively. Proof. Taking the
q-partial derivative of both sides of Equation (
33) with respect to
using Equations (
8) and (
9), it follows that
which, in view of Equation (
27), becomes
Using Equation (
33) in the
l.h.s. of above equation and then comparing the like powers of
in both sides of the resulting equation, we find
which, in accordance with monomiality Equation (
18), yields assertion (
52).
In view of Equation (
19), we note that Equation (
41) (for
) proves assertion (
53). □
Remark 4. Using Equations (52) and (53) in monomiality Equation (21), the following q-differential equation for the 2-variable q-trunc. exp. Appell polynomials hold true: In the following section, certain members belonging to the 2-variable q-trunc. exp. Appell family are considered.
4. Examples
The
q-Bernoulli,
q-Euler, and
q-Genocchi polynomials [
29,
30,
31,
32] play a crucial role in numerous expansions and approximation formulas, contributing significantly to classical and numerical analysis. These members of the
q-Appell family and corresponding numbers are also useful in the analytic theory of numbers. By employing an appropriate choice of
, particular members of the
q-Appell family can be obtained.
We consider the following examples to provide the results for the corresponding members of the class of 2-variable q-trunc. exp. Appell polynomials :
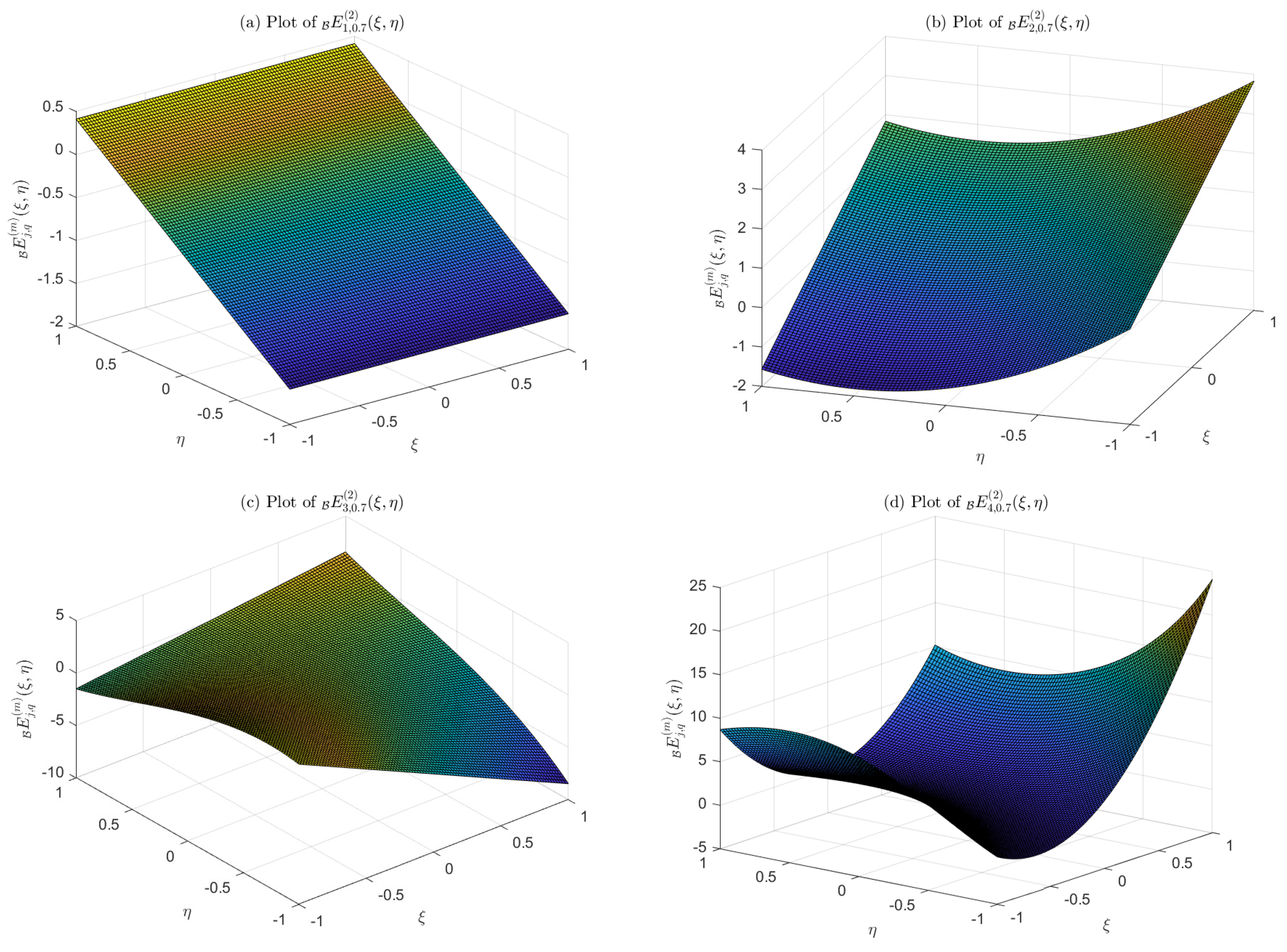
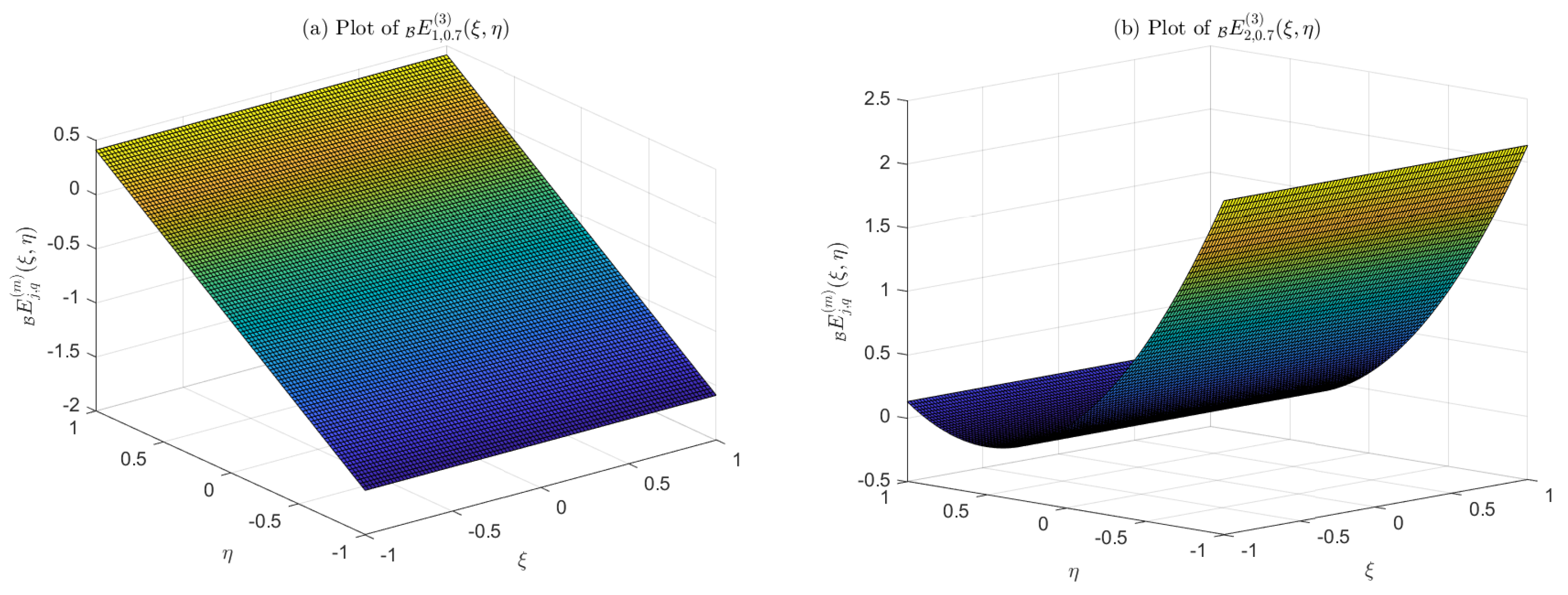
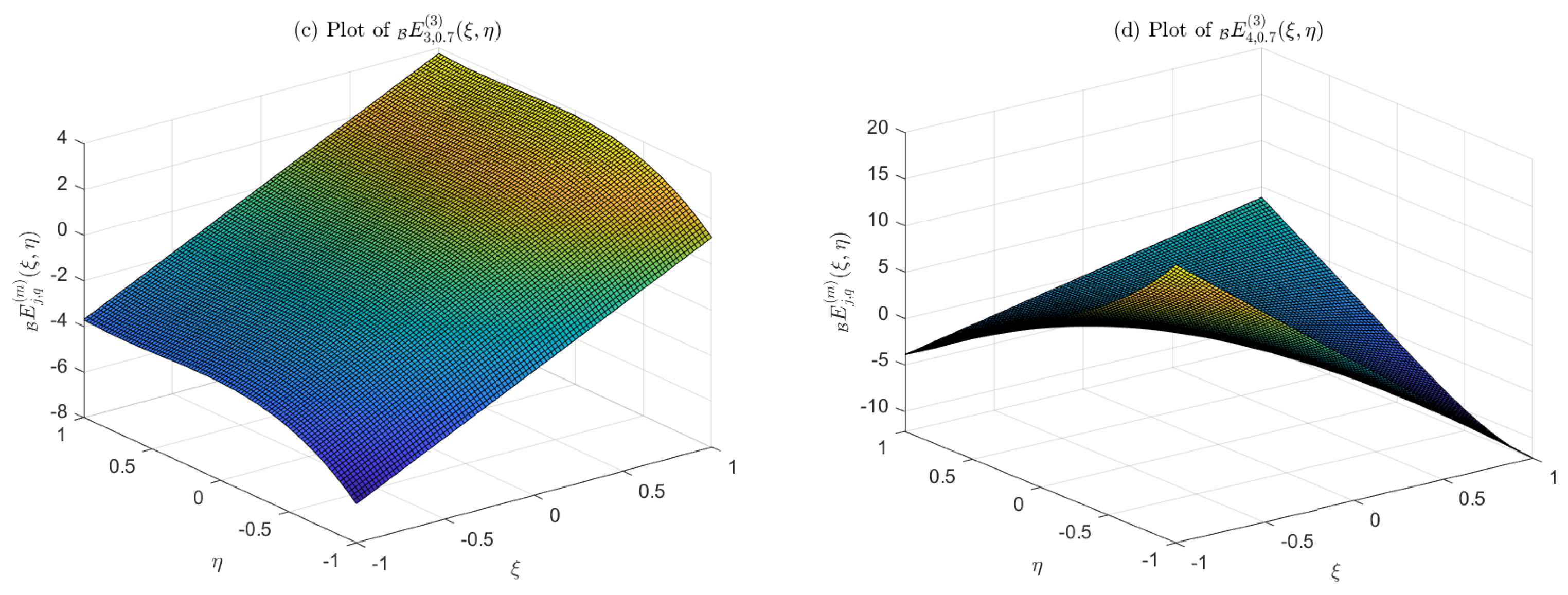
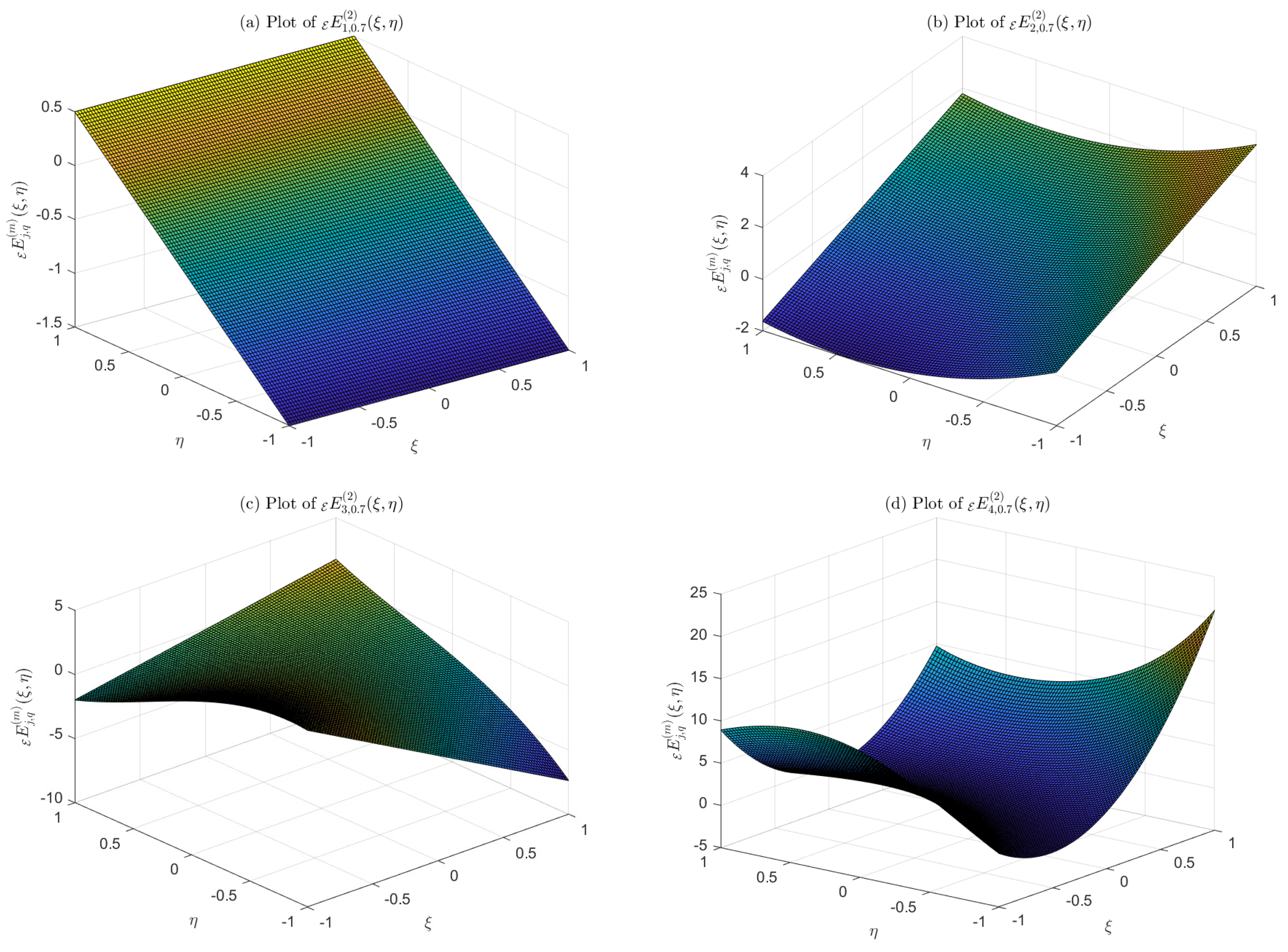
Example 1. Taking , that is, considering the q-Bernoulli polynomials [29,31,32] in generating Equation (33), we obtain the 2-variable q-trunc. exp. Bernoulli polynomials (2Vq-TEBP) of order m , defined by the following generating relation: From Equations (36) and (38), we find the following series representation and q-recurrence relation for the 2Vq-TEBP :andrespectively. Further, we obtain the following expressions of q-multiplicative and q-derivative operators for :andrespectively. Consequently, the q-differential equation satisfied by is given as: Example 2. Taking for the q-Euler polynomials [31,32] in Equations (33) and (36), we obtain the 2-variable q-trunc. exp. Euler polynomials (2Vq-TEEP) of order m , defined by the following generating relation:and series expansion: Similarly, the q-recurrence relation for the 2Vq-TEEP is obtained as: Further, it follows that the 2Vq-TEEP are quasi-monomial under the action of the following q-multiplicative and q-derivative operators:andrespectively, and satisfy the following q-differential equation: Example 3. Taking for the q-Genocchi polynomials [30] in Equations (33) and (36), we obtain the following generating function and series expansion for the 2-variable q-trunc. exp. Genocchi polynomials (2Vq-TEGP) of order m :andrespectively. Also, from Equation (38), we find the following q-recurrence relation for : Further, in view of Equations (52)–(54), we note that the 2Vq-TEGP are quasi-monomial under the action of the following q-multiplicative and q-derivative operators:andrespectively, and they satisfy the following q-differential equation: In the next section, some concluding remarks and future directions are given.
5. Conclusions and Future Directions
In this article, we introduced the 2-variable
q-trunc. exp. Appell polynomials and established their several properties. The results for the 2-variable
q-trunc. exp. Bernoulli,
q-trunc. exp. Euler, and
q-trunc. exp. Genocchi polynomials are established, whereas the graphical representation of these polynomials will be considered in
Appendix A. These findings contribute to the understanding of
q-series and special functions, with potential applications in diverse fields such as combinatorics, number theory, and mathematical physics. Therefore, we introduce another new family associated with
q-truncated exponential polynomials:
Based on the definition of the
-polynomials [
33], first, we introduce a novel set of
q-special polynomials, known as the
q-
-polynomials
, through the following generating function:
Using Equation (
5) and
q-cosine expansion [
11] in the
l.h.s. of Equation (
74), and simplifying, we obtain the following series representation for the
q-
-polynomials
:
Now, using a similar strategy as in Theorem 1, we obtain the
q-trunc. exp.
-polynomials of order
m,
, defined by the following generating relation:
Using Equations (
15) and (
74) in the
l.h.s. of Equation (
76), and simplifying, we obtain the following series representation for the
q-trunc. exp.
-polynomials of order
m,
:
In our forthcoming investigation, we will examine properties such as addition formulas, recursive formulas, q-recurrence relations, q-integral representations, determinant forms, extended quasi-monomiality, and other related results for the q-trunc. exp. -polynomials of order m.