A Finite-Time Disturbance Observer for Tracking Control of Nonlinear Systems Subject to Model Uncertainties and Disturbances
Abstract
1. Introduction
- (1)
- Firstly, a novel FTDOB with a simple structure is first introduced to exactly estimate the lumped disturbance caused by model uncertainties and external disturbances in system dynamics. In addition, the constructed DOB ensures the finite-time convergence of the estimation error.
- (2)
- Secondly, in light of the constructed FTDOB, a DOB-based control strategy is developed to achieve high-accuracy tracking performance by compensating the influence of the lumped disturbance in a feed-forward manner in the control design process.
- (3)
- Finally, the stability of the recommended observer and overall closed-loop system is analyzed by the Lyapunov theory, and the effectiveness of the proposed controller is confirmed by both comparative simulation and experiment results.
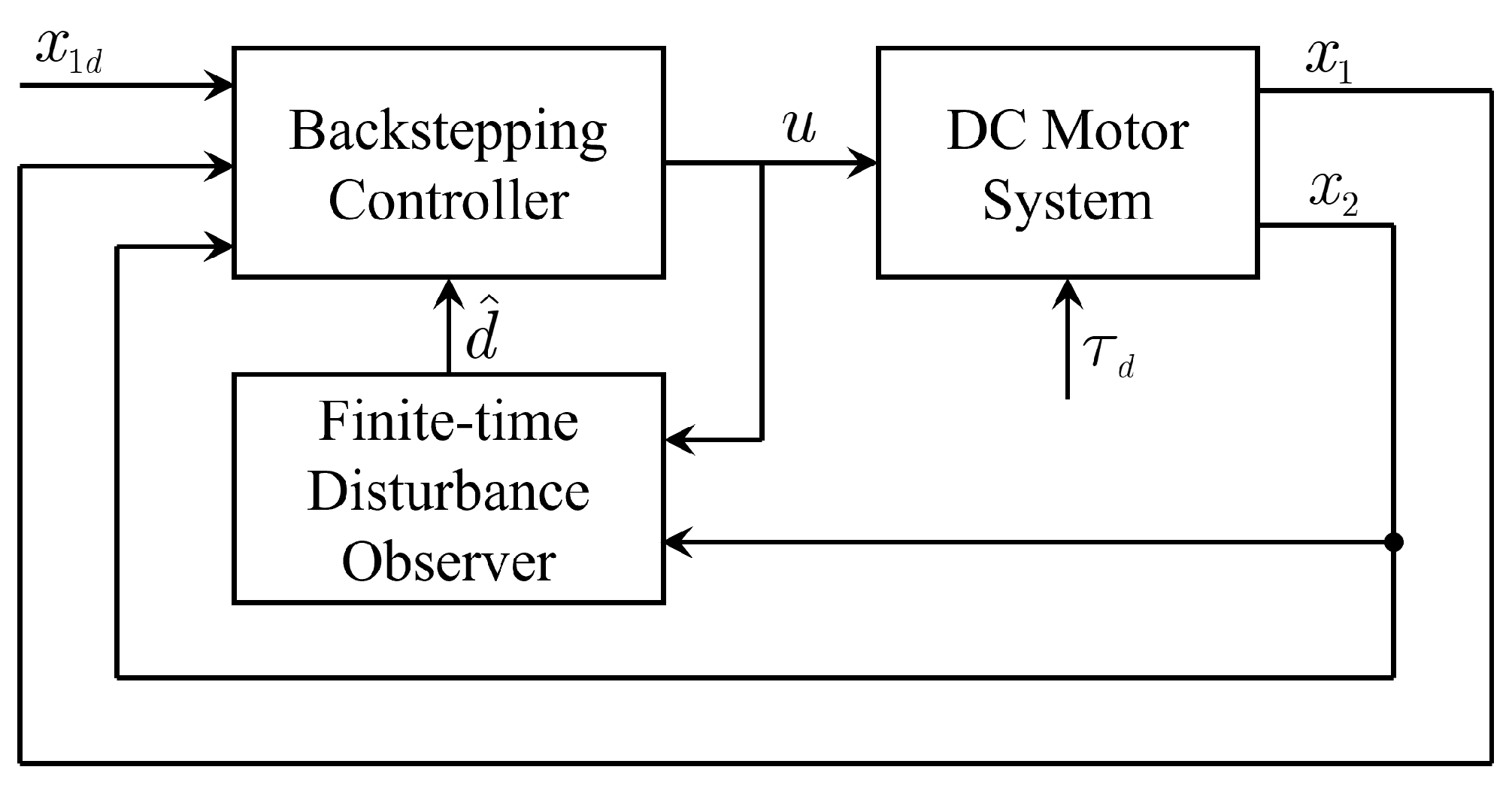
2. Problem Formulation
3. Disturbance-Observer Control Design
3.1. Finite-Time Disturbance Observer Design
3.2. Disturbance Observer-Based Control Design
4. Stability Analysis
5. Simulation and Experimental Verification
5.1. Numerical Simulations
- (a)
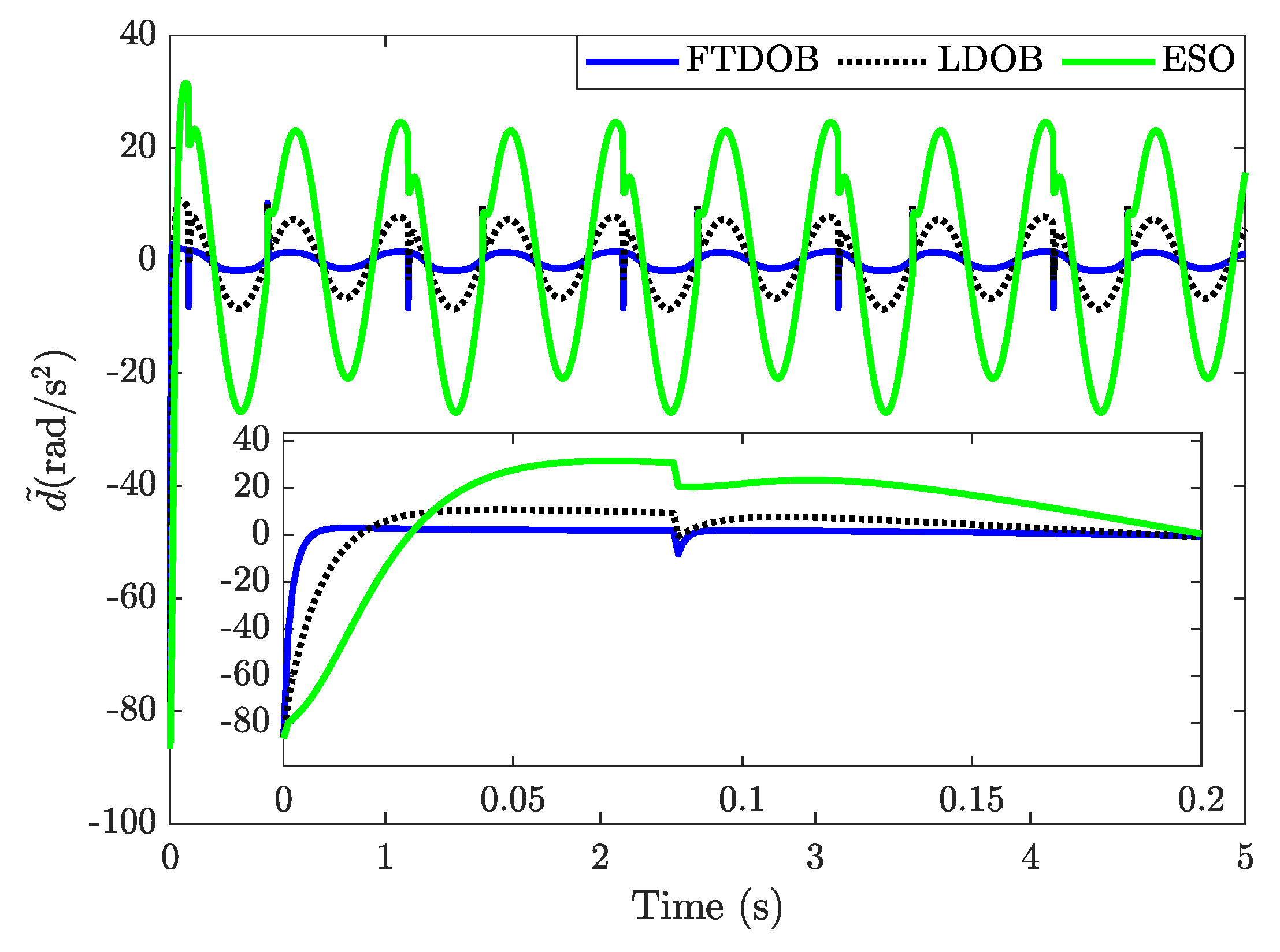
- FTDOB: The proposed DOB (30), with observer gains being chosen as and , and the tuning parameter selected as .
- (b)
- LDOB: A linear disturbance observer, whose dynamics are given bywhere is the observer gain.
- (c)
- ESO: An extended state observer, which is adopted to estimate the lumped disturbance in (32), is designed aswhere stands for the observer gain, is the estimate of , and denotes the estimation of the lumped disturbance .
5.1.1. Open-Loop Control Structure
5.1.2. Closed-Loop Control Structure
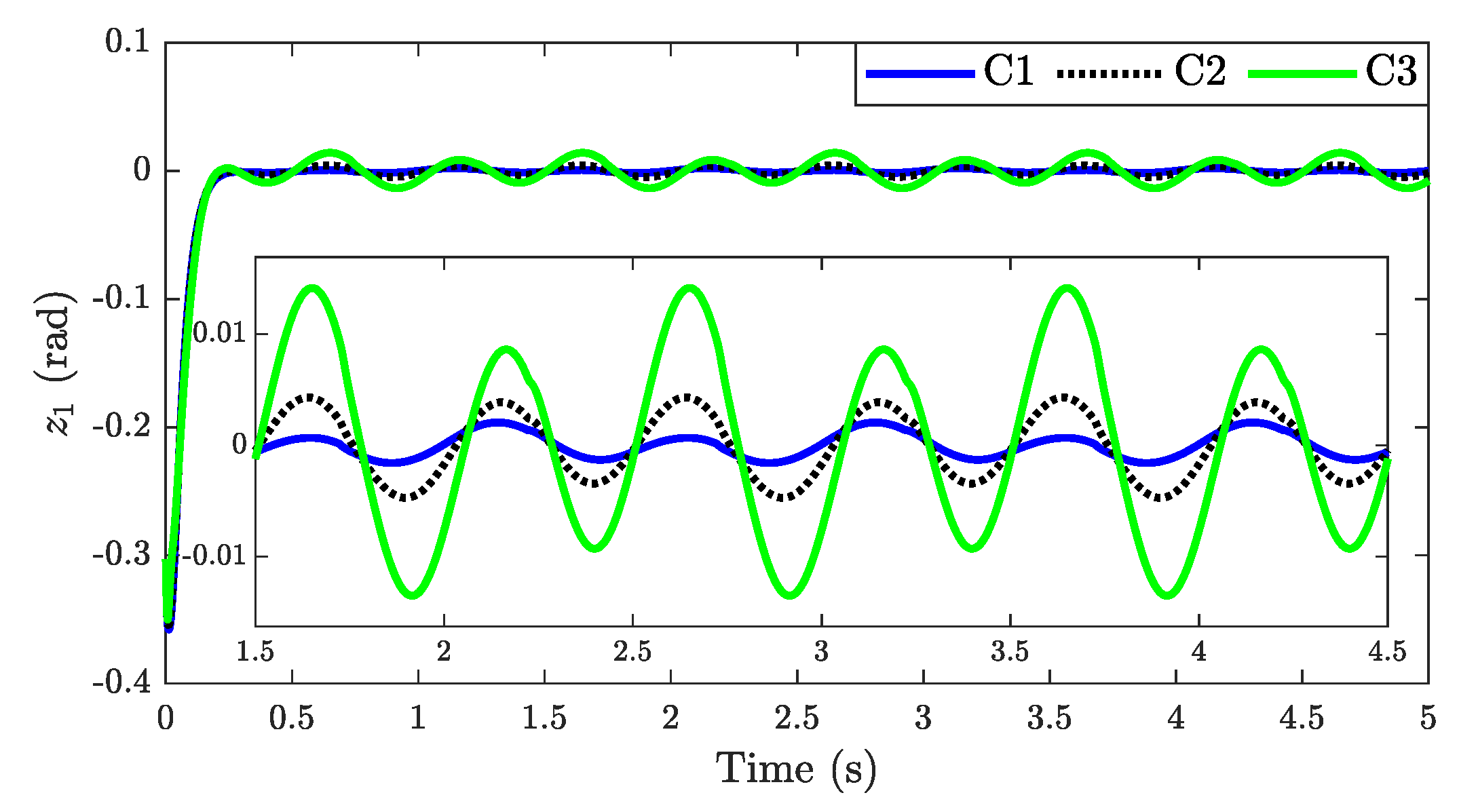
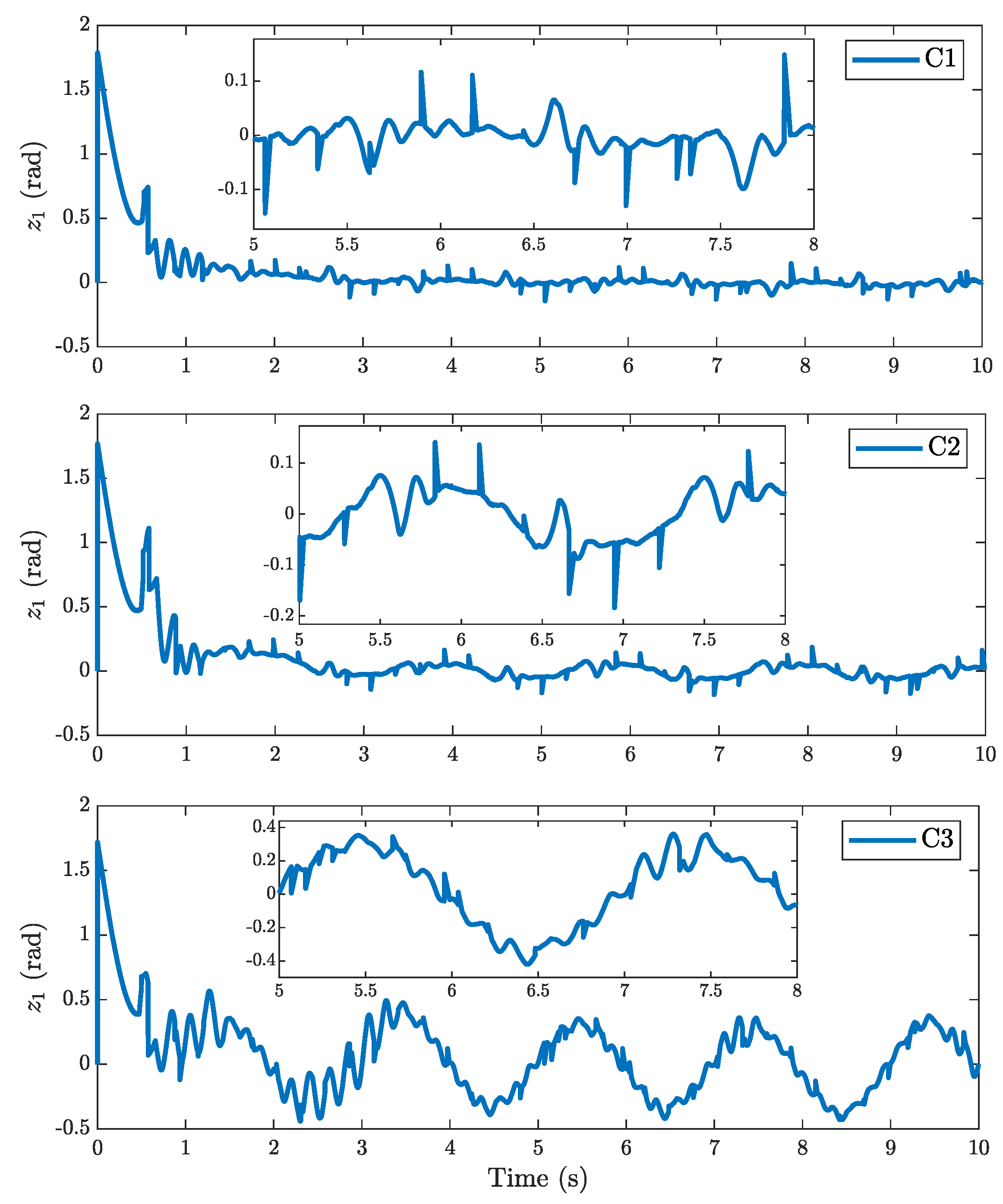
5.2. Experimental Validation
- (a)
- C1: The control algorithm with the proposed disturbance observer being designed to estimate the lumped disturbance in (36) as follows:where , , and observer gains are chosen as and .
- (b)
- C2 [34]: The linear disturbance observer (LDOB)-based controller (LDOB-BC) in which the dynamics of the LDOB are represented as:where z is the auxiliary variable and k reflexes the observer gain that is selected as .
- (c)
- C3 [35]: An extended state observer (ESO)-based controller, in which the dynamics of the constructed ESO are as follows:where and are estimates of and , respectively; denotes the estimation of ; and stands for the observer bandwidth to be selected. Herein, the observer bandwidth is chosen as .
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| ADRC | Active Disturbance Rejection Control |
| BSC | Backstepping Control |
| ESO | Extended State Observer |
| LDOB | Linear Disturbance Observer |
| FTDOB | Finite-Time Disturbance Observer |
References
- Zhang, J.; Nie, P.; Chen, Y.; Zhang, B. A Joint Acceleration Estimation Method Based on a High-Order Disturbance Observer. IEEE Robot. Autom. Lett. 2022, 7, 12615–12622. [Google Scholar] [CrossRef]
- Wang, C.; Guo, L.; Wen, C.; Zhu, Y.; Qiao, J. Adaptive Anti-Disturbance Control for a Class of Uncertain Nonlinear Systems with Composite Disturbances. IEEE Trans. Cybern. 2023, 54, 4241–4254. [Google Scholar] [CrossRef] [PubMed]
- Yang, G.; Yao, J. Multilayer neurocontrol of high-order uncertain nonlinear systems with active disturbance rejection. Int. J. Robust Nonlinear Control. 2024, 34, 2972–2987. [Google Scholar] [CrossRef]
- Zhang, J.; Jiang, W.; Ge, S.S. Adaptive Fuzzy Control for Uncertain Strict-Feedback Nonlinear Systems with Full-State Constraints Using Disturbance Observer. IEEE Trans. Syst. Man Cybern. Syst. 2023, 53, 6145–6156. [Google Scholar] [CrossRef]
- Liu, X.; Su, X.; Li, R.; Shi, P. Anti-disturbance sliding mode control for uncertain nonlinear systems. Int. J. Control. 2023, 96, 3001–3011. [Google Scholar] [CrossRef]
- Edwards, C.; Spurgeon, S.K. Sliding Mode Control: Theory And Applications, 1st ed.; CRC Press: Boca Raton, FL, USA, 1998. [Google Scholar]
- Landau, I.D.; Lozano, R.; M’Saad, M.; Karimi, A. Adaptive Control: Algorithms, Analysis and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Liu, Z.-G.; Xue, L.; Sun, Z.-Y. A new robust adaptive tracking strategy to uncertain time-delay nonlinear systems with a general form. Automatica 2022, 146, 110560. [Google Scholar] [CrossRef]
- Xu, H.; Yu, D.; Liu, Y.J. Observer-Based Fuzzy Adaptive Predefined Time Control for Uncertain Nonlinear Systems with Full-State Error Constraints. IEEE Trans. Fuzzy Syst. 2024, 32, 1370–1382. [Google Scholar] [CrossRef]
- Lin, F.; Xue, G.; Qin, B.; Li, S.; Liu, H. Event-triggered finite-time fuzzy control approach for fractional-order nonlinear chaotic systems with input delay. Chaos Solitons Fractals 2023, 175, 114036. [Google Scholar] [CrossRef]
- Nguyen, M.H.; Dao, H.V.; Ahn, K.K. Adaptive Robust Position Control of Electro-Hydraulic Servo Systems with Large Uncertainties and Disturbances. Appl. Sci. 2022, 12, 2. [Google Scholar] [CrossRef]
- Yu, G.; Reis, J.; Silvestre, C. Quadrotor Neural Network Adaptive Control: Design and Experimental Validation. IEEE Robot. Autom. Lett. 2023, 8, 2574–2581. [Google Scholar] [CrossRef]
- Truong, H.V.A.; Nguyen, M.H.; Tran, D.T.; Ahn, K.K. A novel adaptive neural network-based time-delayed estimation control for nonlinear systems subject to disturbances and unknown dynamics. ISA Trans. 2023, 142, 214–227. [Google Scholar] [CrossRef]
- Lin, F.; Xue, G.; Li, S.; Liu, H.; Pan, Y.; Cao, J. Finite-time sliding mode fault-tolerant neural network control for nonstrict-feedback nonlinear systems. Nonlinear Dyn. 2023, 111, 17205–17227. [Google Scholar] [CrossRef]
- Liu, Z.G.; Shi, Y.Y.; Sun, W.; Su, S.F. Direct Fuzzy Adaptive Regulation for High-Order Delayed Systems: A Lyapunov–Razumikhin Function Method. IEEE Trans. Cybern. 2024, 54, 1894–1906. [Google Scholar] [CrossRef]
- Han, J.-Q. Nonlinear design methods for control systems. IFAC Proc. Vol. 1999, 32, 1531–1536. [Google Scholar] [CrossRef]
- Nguyen, M.H.; Ahn, K.K. Output Feedback Robust Tracking Control for a Variable-Speed Pump-Controlled Hydraulic System Subject to Mismatched Uncertainties. Mathematics 2023, 11, 8. [Google Scholar] [CrossRef]
- Peng, Z.; Jiang, Y.; Liu, L.; Shi, Y. Path-Guided Model-Free Flocking Control of Unmanned Surface Vehicles Based on Concurrent Learning Extended State Observers. IEEE Trans. Syst. Man Cybern. Syst. 2023, 53, 4729–4739. [Google Scholar] [CrossRef]
- Chen, Z.; Guo, Q.; Li, T.; Yan, Y. Output Constrained Control of Lower Limb Exoskeleton Based on Knee Motion Probabilistic Model with Finite-Time Extended State Observer. IEEE/Asme Trans. Mechatron. 2023, 28, 2305–2316. [Google Scholar] [CrossRef]
- Yang, W.; Ding, S.; Ding, C. Fast Supertwisting Sliding Mode Control With Antipeaking Extended State Observer for Path-Tracking of Unmanned Agricultural Vehicles. IEEE Trans. Ind. Electron. 2024, 71, 12973–12982. [Google Scholar] [CrossRef]
- Sebastian, G.; Li, Z.; Crocher, V.; Kremers, D.; Tan, Y.; Oetomo, D. Interaction Force Estimation Using Extended State Observers: An Application to Impedance-Based Assistive and Rehabilitation Robotics. IEEE Robot. Autom. Lett. 2019, 4, 1156–1161. [Google Scholar] [CrossRef]
- Fan, Q.; Chen, W.; Huang, W.; Xie, L.; Bi, K.; Zhu, Y. Combined Magnetic Field Decoupling and Disturbance Rejection Control of Microrobots Based on Extended State Observer. IEEE Robot. Autom. Lett. 2022, 7, 4032–4039. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, J.; Gu, S.; Zhao, L.; Li, Z. Modelling and Angle Tracking Control for Multi-Chamber Soft Bending Pneumatic Muscle. IEEE Robot. Autom. Lett. 2023, 8, 7647–7654. [Google Scholar] [CrossRef]
- Ohishi, K.; Nakao, M.; Ohnishi, K.; Miyachi, K. Microprocessor-Controlled DC Motor for Load-Insensitive Position Servo System. IEEE Trans. Ind. Electron. 1987, 1, 44–49. [Google Scholar] [CrossRef]
- Lee, W.; Chung, W.K. Disturbance-Observer-Based Compliance Control of Electro-Hydraulic Actuators with Backdrivability. IEEE Robot. Autom. Lett. 2019, 4, 1722–1729. [Google Scholar] [CrossRef]
- Lee, D.; Back, J.; Oh, S. Workspace Nonlinear Disturbance Observer for Robust Position Control of Flexible Joint Robots. IEEE Robot. Autom. Lett. 2024, 9, 4495–4502. [Google Scholar] [CrossRef]
- Xi, R.D.; Xiao, X.; Ma, T.N.; Yang, Z.X. Adaptive Sliding Mode Disturbance Observer Based Robust Control for Robot Manipulators Towards Assembly Assistance. IEEE Robot. Autom. Lett. 2022, 7, 6139–6146. [Google Scholar] [CrossRef]
- Li, K.; Li, Y. Disturbance-Observer Adaptive Fuzzy Performance Constraint Control for Vehicular Platoon System. IEEE Trans. Syst. Man Cybern. Syst. 2024, 54, 2462–2470. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, J.; Zhang, O.; Zhao, Y.; Chen, W.; Gao, Y. Adaptive Disturbance Observer-Based Fixed-Time Tracking Control for Uncertain Robotic Systems. IEEE Trans. Ind. Electron. 2024, 71, 14823–14831. [Google Scholar] [CrossRef]
- Que, N.; Deng, W.; Zhou, N.; Yao, J. Disturbance Observer Based Prescribed-Time Tracking Control of Nonlinear Systems with Non-Vanishing Uncertainties. IEEE Trans. Circuits Syst. II Express Briefs 2024, 71, 3131–3135. [Google Scholar] [CrossRef]
- Gao, Z.; Zhang, Y.; Guo, G. Prescribed-Time Control of Vehicular Platoons Based on a Disturbance Observer. IEEE Trans. Circuits Syst. Express Briefs 2022, 69, 3789–3793. [Google Scholar] [CrossRef]
- Yu, S.; Yu, X.; Shirinzadeh, B.; Man, Z. Continuous finite-time control for robotic manipulators with terminal sliding mode. Automatica 2005, 41, 1957–1964. [Google Scholar] [CrossRef]
- Mitrinović, D.S.; Pečarić, J.E.; Fink, A.M. Young’s Inequality. In Classical and New Inequalities in Analysis; Mathematics and Its Applications (East European Series); Springer: Dordrecht, The Netherlands, 1993; Volume 61. [Google Scholar]
- Won, D.; Kim, W.; Shin, D.; Chung, C.C. High-Gain Disturbance Observer-Based Backstepping Control with Output Tracking Error Constraint for Electro-Hydraulic Systems. IEEE Trans. Control. Syst. Technol. 2015, 23, 787–795. [Google Scholar] [CrossRef]
- Yao, J.; Jiao, Z.; Ma, D. Adaptive Robust Control of DC Motors with Extended State Observer. IEEE Trans. Ind. Electron. 2014, 61, 3630–3637. [Google Scholar] [CrossRef]




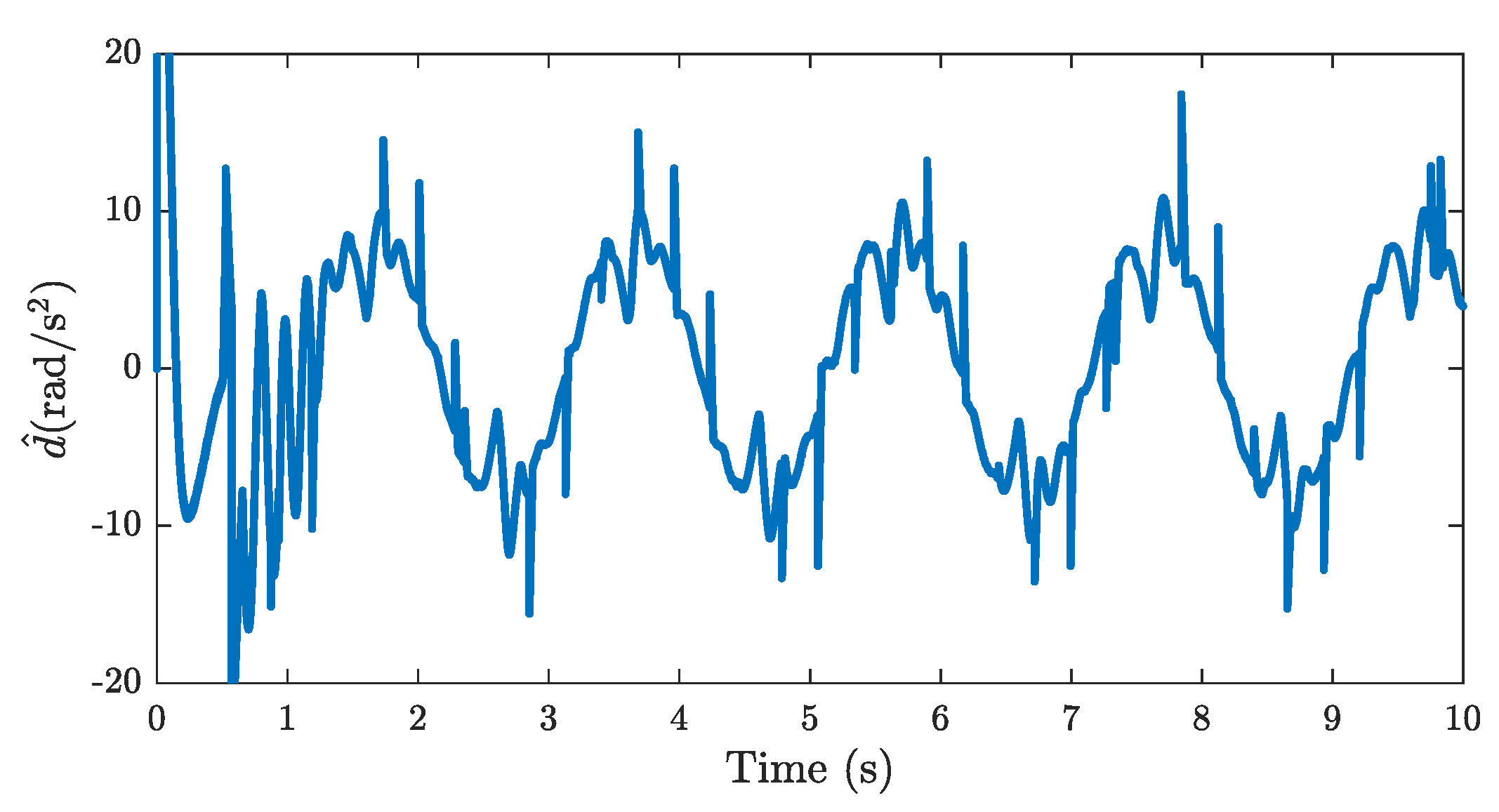
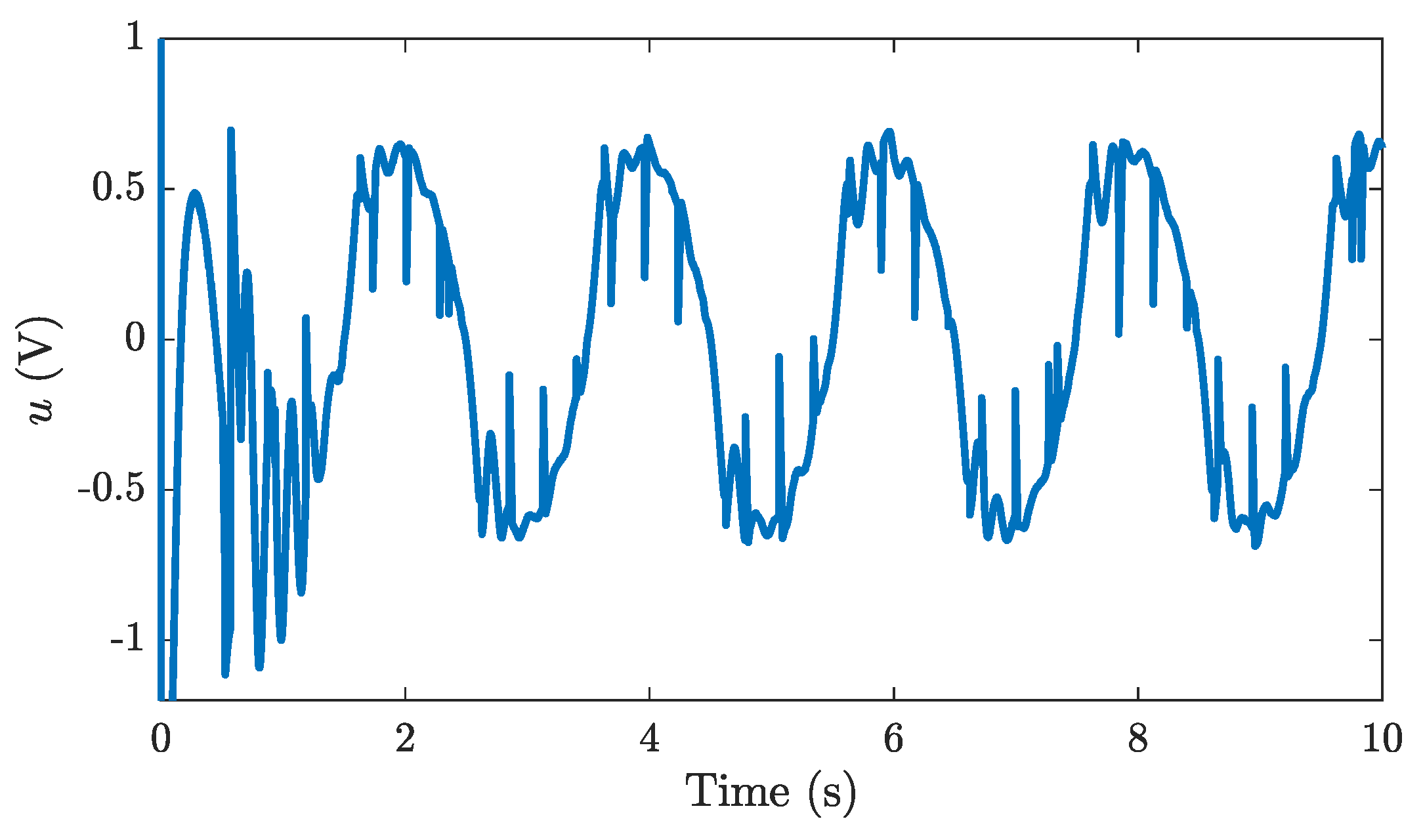
| Controller | Me (rad) | e (rad) | e (rad) |
|---|---|---|---|
| C1 | |||
| C2 | |||
| C3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nguyen, M.H.; Ahn, K.K. A Finite-Time Disturbance Observer for Tracking Control of Nonlinear Systems Subject to Model Uncertainties and Disturbances. Mathematics 2024, 12, 3512. https://doi.org/10.3390/math12223512
Nguyen MH, Ahn KK. A Finite-Time Disturbance Observer for Tracking Control of Nonlinear Systems Subject to Model Uncertainties and Disturbances. Mathematics. 2024; 12(22):3512. https://doi.org/10.3390/math12223512
Chicago/Turabian StyleNguyen, Manh Hung, and Kyoung Kwan Ahn. 2024. "A Finite-Time Disturbance Observer for Tracking Control of Nonlinear Systems Subject to Model Uncertainties and Disturbances" Mathematics 12, no. 22: 3512. https://doi.org/10.3390/math12223512
APA StyleNguyen, M. H., & Ahn, K. K. (2024). A Finite-Time Disturbance Observer for Tracking Control of Nonlinear Systems Subject to Model Uncertainties and Disturbances. Mathematics, 12(22), 3512. https://doi.org/10.3390/math12223512









