Optimal Replenishment Strategy for a High-Tech Product Demand with Non-Instantaneous Deterioration under an Advance-Cash-Credit Payment Scheme by a Discounted Cash-Flow Analysis
Abstract
1. Introduction
2. Literature Review
2.1. Research on Non-Instantaneous Deteriorating Items
2.2. Research on Ramp-Type Demand
2.3. Research on Generalized Payments
- Cash or cash-credit payment: In 1913, Harris [22] proposed the EOQ model, in which the buyer must pay cash on delivery (i.e., cash payment). However, in the existing competitive market, most companies offer their products with various credit terms (i.e., credit payment) to stimulate sales and remain competitive. Teng [23] presented cash-credit payments for retailers with poor-credit customers, in which partial cash payment reduces default risk and partial credit payment stimulates sales. Yang et al. [24] explored the optimal retailer order and credit policies when suppliers offer either a cash discount or delayed payment based on the order quantity.
- Credit payment: Substantial research has investigated credit payment, which involves the provision of a permissible delay in payment. In 1985, Goyal [25] developed an EOQ model for credit payment circumstances that neglected differences in the sale price and purchase cost. Shah [26] considered a stochastic inventory model with permissible delay in payments. Teng [27] presented an EOQ ordering policy with a conditionally permissible delay in payments. Gupta and Wang [28] explored a stochastic inventory model with trade credit. Taleizadeh et al. [29] investigated an EOQ model with partially delayed payment and partial backordering. Mahata and De [30] proposed an EOQ inventory system for items with a price-dependent demand rate under a retailer partial trade credit policy to reduce default risk. Huang [31] extended Goyal’s model to develop an EOQ model, in which the supplier offers the retailer a permissible delay period (i.e., upstream trade credit), and the retailer, in turn, provides a trade credit period (i.e., downstream trade credit) to its customers. This is a two-level trade credit policy. Since then, many models have been developed in numerous directions. For example, Yang explored an inventory model for a ramp-type demand with two-level trade credit financing linked to order quantity [20]. Subsequently, Yang [32] presented an optimal ordering policy for deteriorating items with limited storage capacity under two-level trade credit linked to the order quantity using a discounted cash flow (DCF) analysis. Relevant articles can be found in the references of these studies. Moradi et al. [33] proposed an inventory model for imperfect quality items, integrating the impacts of learning effects and partial trade credit. Lin et al. [34] focused on optimizing ordering policies and credit terms for items whose demand varies with inventory levels under the condition of a trade credit limit. Pal et al. [35] examined a two-warehouse inventory model that accounts for non-instantaneous deterioration, incorporating credit policy, inflation, demand dependent on price and time, and partial backlogging.
- Advance or advance-credit payment: Inventory models with advance payment have rarely been studied. Zhang [36] proposed an optimal advance payment scheme involving fixed prepayment costs. Gupta et al. [37] presented an application of a genetic algorithm for producing an inventory model with advance payment and interval-valued inventory costs. Maiti et al. [38] considered advance payment in an inventory model with a stochastic lead time and price-dependent demand. Taleizadeh [39] proposed an EOQ model with partial backordering and advance payments for evaporating items. Teng et al. [40] adopted an inventory lot size policy for deteriorating items with expiration dates as well as advance payment. Khan et al. [41] explored the effects of full and partial advance payments with discount facilities for deteriorating products when the demand is both price- and stock-dependent. Zhang et al. [42] developed an EOQ model with full advance payment and partial-advanced–partial-delayed payment. Zia and Taleizadeh [43] devised a lot sizing model with back-ordering under hybrid linked-to-order multiple advance payments and delayed payment. Diabat et al. [44] proposed an advance-credit payment for a lot sizing model with partial downstream delayed payment, partial upstream advance payment, and partial backordering for deteriorating items. Duary et al. [45] explored a price discount inventory model with advance and delayed payments for deteriorating items under capacity constraints and partially backlogged shortages.
- Advance-cash-credit payment: In addition to the aforementioned studies, Li et al. [46] presented pricing and lot sizing policies for perishable products with an ACC payment, which were examined using a DCF analysis. Li et al. [47] considered an ACC payment with a time-dependent demand. Wu et al. [48] provided inventory policies for perishable products with expiration dates and ACC payment schemes. Li et al. [49] studied optimal pricing, lot sizing, and back-ordering decisions for when a seller demands an ACC payment. Li et al. [50] proposed lot sizing and pricing decisions for perishable products with three-echelon supply chains and ACC payments when the demand depends on the price and stock age. Li et al. [51] developed EOQ-based pricing and customer credit decisions for generalized supplier payments (i.e., ACC payments). Tsao et al. [52] provided a supply chain network design for an ACC payment. Feng et al. [53] investigated the optimal sale price, replenishment cycle, and payment time for ACC payments from a seller’s perspective. Feng et al. [54] explored pricing and lot sizing for fresh goods when the demand depends on the unit price, displayed stock, and product age under generalized payment. Shi et al. [55] also used ACC payment schemes to demonstrate an optimal retailer strategy for perishable products with increasing demand under a two-level trade credit. Recently, Tsao et al. [56] developed a model for a single supplier–manufacturer chain to identify the optimal replenishment cycle time and predictive maintenance effort needed to minimize the total cost’s present value, given that the manufacturer receives an ACC payment from the supplier. Chang and Tseng [57] formulated EOQ models to analyze how ACC payment schemes and carbon emission policies affect replenishment and pricing strategies for perishable goods.
3. Assumptions and Notation
- Items can deteriorate after being in stock for a period. No replacement or repair of deteriorating items is assumed to occur during the period.
- Each cost considered is assumed to be continuously compounded throughout the analysis. The cash flows associated with product transactions are assumed to be instantaneous.
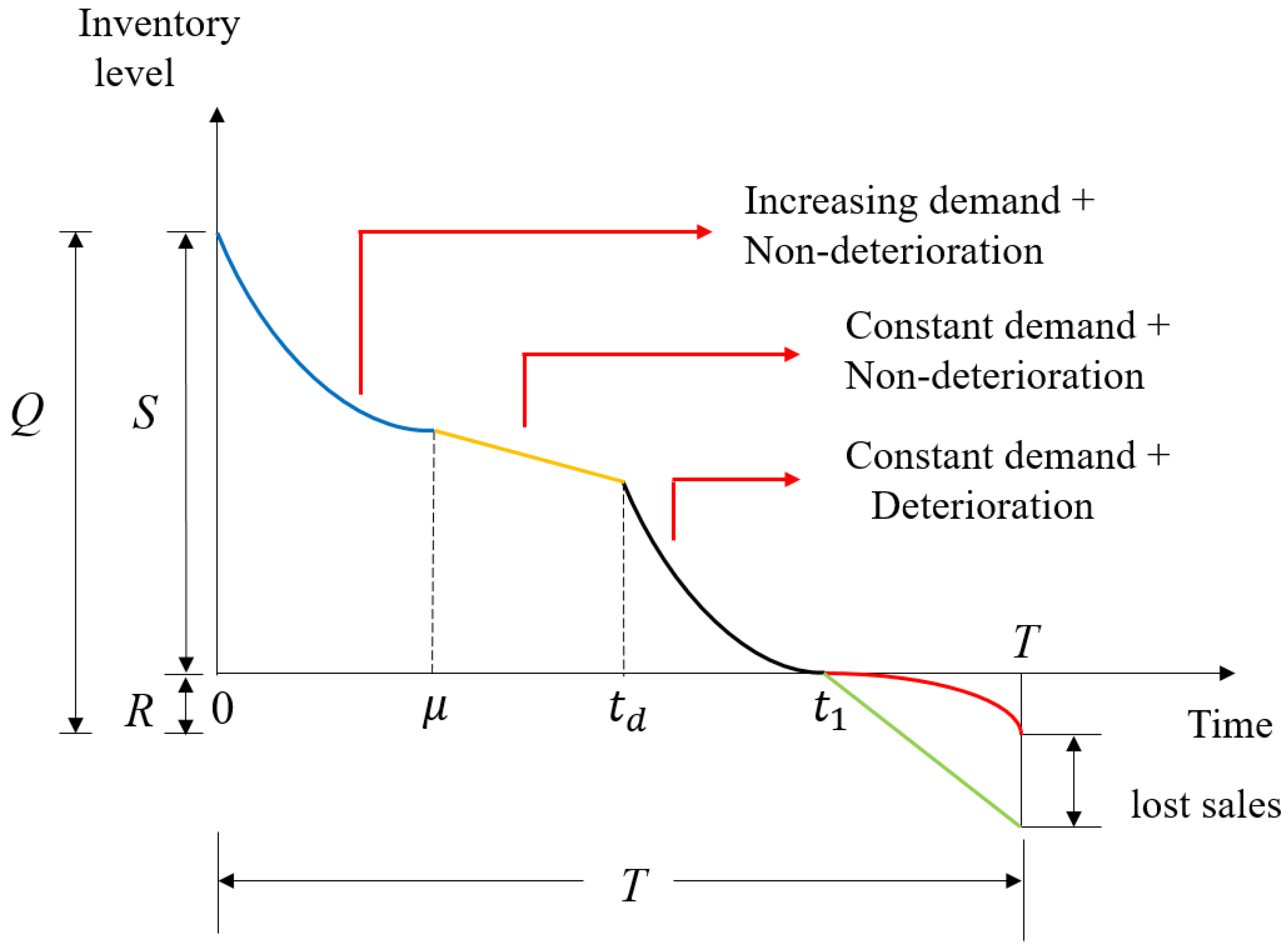
- Allowance for shortages is permitted. Unfulfilled demand is stored for later fulfillment, and the proportion of backlogged shortages is a continuously differentiable and decreasing function of t, which is denoted by , where t represents the time until the next replenishment, 0 1, and . If = 1 (or 0) for all t values, then shortages are completely backlogged (or lost). If 0 < < 1, then the shortage is partially backlogged and partially lost.
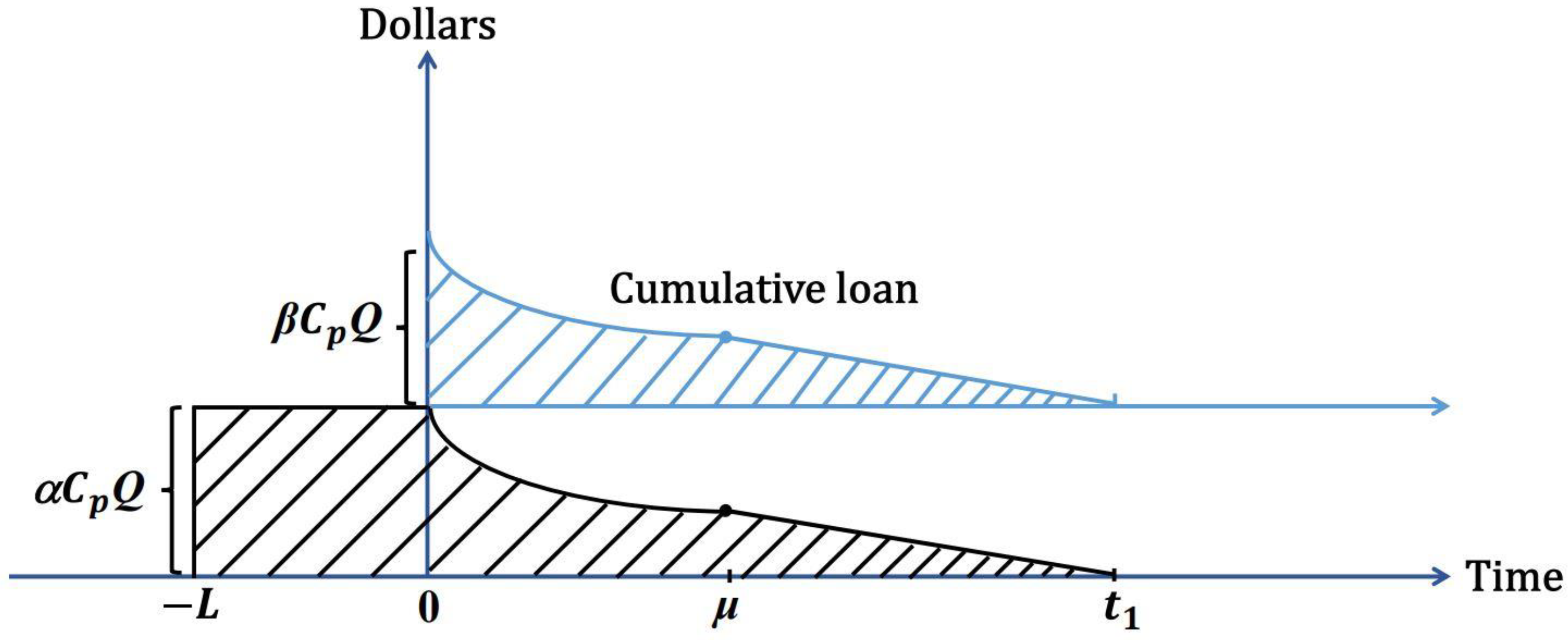
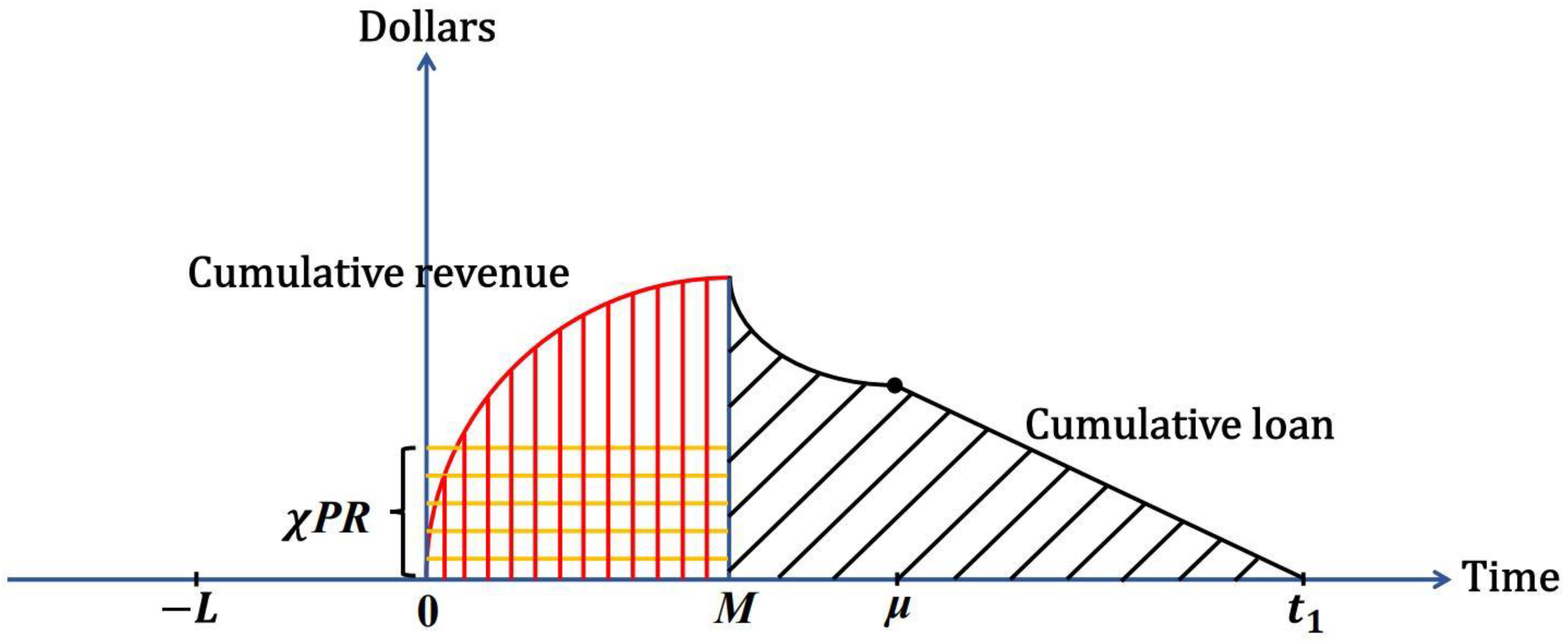
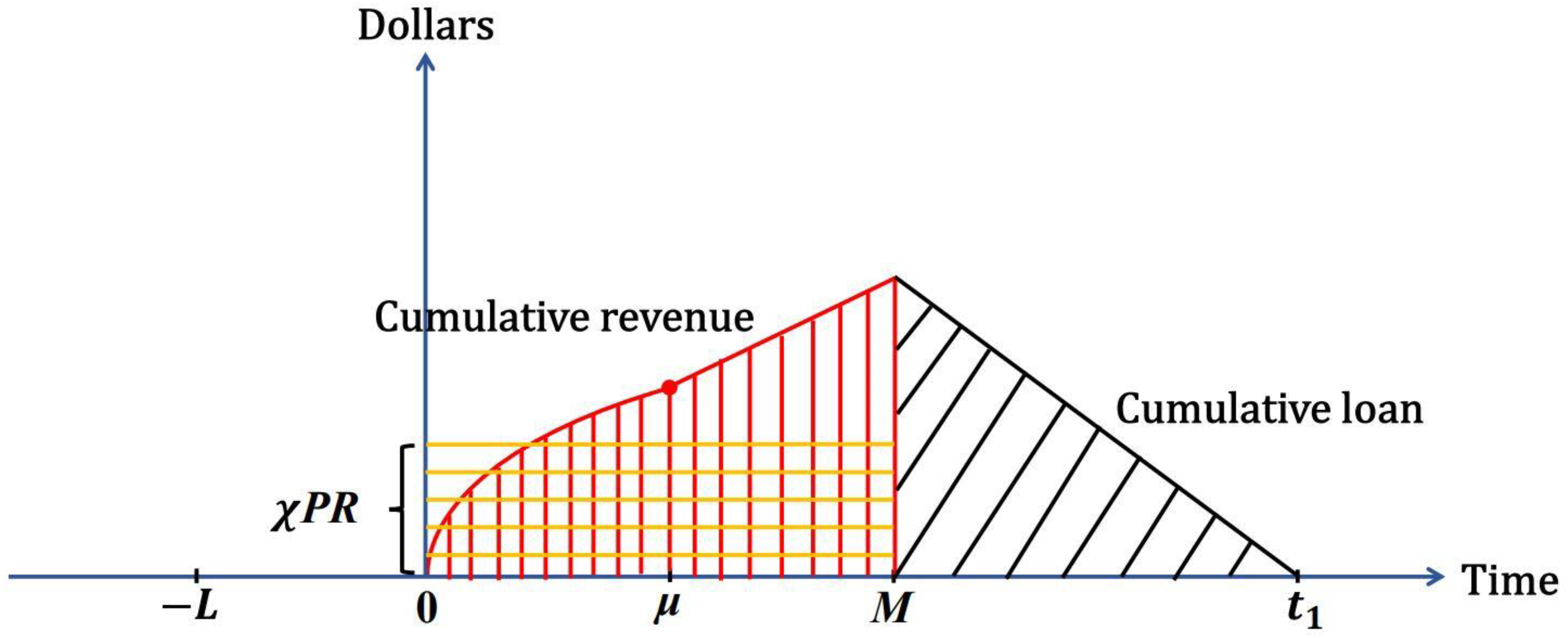
- The buyer pays the seller a fraction α of the total purchase cost in advance as a deposit for L years. The buyer then pays another fraction β of the total purchase cost in cash upon receiving the order quantity Q units at time 0. The seller grants an upstream credit period of M for the remainder fraction of the total purchase cost (i.e., .
4. Mathematical Models
4.1. Scenario 1. (i.e., the Demand Growth Period Is Not Longer than the Stable Quality Period)
4.2. Scenario 2. (i.e., the Demand Growth Period Is Not Shorter than the Stable Quality Period)
5. Solutions to Proposed Models
5.1. Scenario 1:
5.2. Scenario 2:
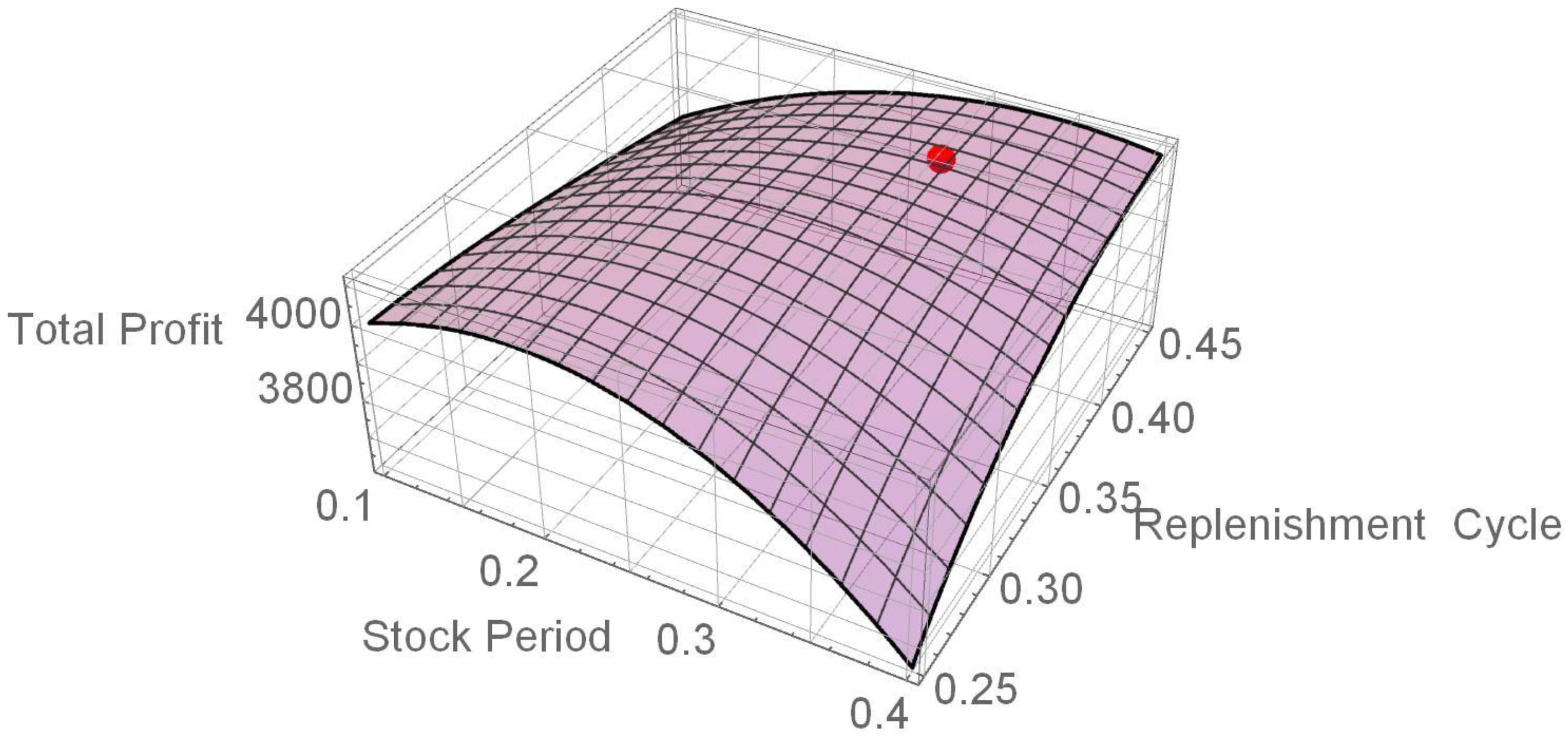
6. Numerical Examples
7. Sensitivity Analysis
- When , the optimal profit and the backordered quantity R are greater, whereas the stock period , the replenishment cycle T, and the ordered quantity Q are lower.
- When , the replenishment cycle T, the optimal profit , the ordered quantity Q, and the backordered quantity R are all greater, whereas the stock period is shorter.
- When , the stock period , the replenishment cycle T, the optimal profit , and the ordered quantity Q are all greater, whereas the backordered quantity R is lower when but greater when .
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Walpole, R.E.; Myers, R.H. Probability and Statistics for Engineering and Scientists; Macmillan: New York, NY, USA, 1978. [Google Scholar]
- Ouyang, L.Y.; Wu, K.S.; Yang, C.T. A study on an inventory model for non-instantaneous deteriorating items with permissible delay in payments. Comput. Ind. Eng. 2006, 51, 637–651. [Google Scholar] [CrossRef]
- Geetha, K.V.; Uthayakumar, R. Economic design of an inventory policy for non-instantaneous deteriorating items under permissible delay in payments. J. Comput. Appl. Math. 2010, 233, 2492–2505. [Google Scholar] [CrossRef]
- Maihami, R.; Kamalabadi, I.N. Joint pricing and inventory control for non-instantaneous deteriorating items with partial backlogging and time and price dependent demands. Int. J. Prod. Econ. 2012, 136, 116–122. [Google Scholar] [CrossRef]
- Soni, H.; Patel, K. Optimal pricing and inventory policies for non-instantaneous deteriorating items with permissible delay in payment: Fuzzy expected value model. Int. J. Ind. Eng. Comput. 2012, 3, 281–300. [Google Scholar] [CrossRef]
- Chang, C.T.; Cheng, M.C.; Ouyang, L.Y. Optimal pricing and ordering policies for non-instantaneously deteriorating items under order-size-dependent delay in payment. Appl. Math. Model. 2015, 39, 747–763. [Google Scholar] [CrossRef]
- Tiwari, S.; Cárdenas-Barrón, L.E.; Khanna, A.; Jaggi, C.K. Impact of trade credit and inflation on retailer’s ordering policies for non-instantaneous deteriorating items in a two-warehouse environment. Int. J. Prod. Econ. 2016, 176, 154–169. [Google Scholar] [CrossRef]
- Jaggi, C.K.; Tiwari, S.; Goel, S.K. Credit financing in economic ordering policies for non-instantaneous deteriorating items with price dependent demand and two storage facilities. Ann. Oper. Res. 2017, 248, 253–280. [Google Scholar] [CrossRef]
- Lashgari, M.; Taleizadeh, A.A.; Sadjadi, S.J. Ordering policies for non-instantaneous deteriorating items under hybrid partial prepayment, partial trade credit and partial backordering. J. Oper. Res. Soc. 2018, 69, 1167–1196. [Google Scholar] [CrossRef]
- Tavassoli, S.; Manavizadeh, N.; Rezzaei, A.; Rabbani, M. A lot-sizing model for non-instantaneous deteriorating products under advance payment and non-linear partial backlogging. Iran. J. Manag. Stud. 2022, 15, 85–110. [Google Scholar] [CrossRef]
- Pathak, K.; Yadav, A.S.; Agarwal, P. Optimizing two-warehouse inventory for shelf-life stock with time-varying bi-quadratic demand under shortages and inflation. Math. Model. Eng. Probl. 2024, 11, 446–456. [Google Scholar] [CrossRef]
- Manna, S.K.; Chaudhrui, K.S. An EOQ model with ramp type demand rate, time dependent deterioration rate, unit production cost and shortages. Eur. J. Oper. Res. 2006, 171, 557–566. [Google Scholar] [CrossRef]
- Deng, P.S.; Lin, R.H.J.; Chu, P. A Note on the inventory models for deteriorating items with ramp type demand rate. Eur. J. Oper. Res. 2007, 178, 112–120. [Google Scholar] [CrossRef]
- Panda, S.; Senapati, S.; Basu, M. Optimal replenishment policy for perishable seasonal products in a season with ramp-type dependent demand. Comput. Ind. Eng. 2008, 54, 301–314. [Google Scholar] [CrossRef]
- Agrawal, S.; Banerjee, S. Two-warehouse inventory model with ramp-type demand and partially backlogged shortages. Int. J. Syst. Sci. 2011, 42, 1115–1126. [Google Scholar] [CrossRef]
- Skouri, K.; Konstantaras, I.; Manna, S.K. Inventory models with ramp type demand rate, time dependent deterioration rate, unit production cost and shortages. Ann. Oper. Res. 2011, 191, 73–95. [Google Scholar] [CrossRef]
- Agrawal, S.; Banerjee, S.; Papachristos, S. Inventory model with deteriorating items, ramp-type demand and partially backlogged shortages for a two-warehouse system. Appl. Math. Model. 2013, 37, 8912–8929. [Google Scholar] [CrossRef]
- Halim, M.A. Weibull distributed deteriorating inventory model with ramp type demand and fully backlogged shortage. Asian J. Math. Comput. Res. 2017, 19, 148–157. Available online: https://www.researchgate.net/publication/319330183 (accessed on 1 September 2024).
- Shi, Y.; Zhang, Z.; Zhou, F.; Shi, Y. Optimal ordering policies for a single deteriorating item with ramp-type demand rate under permissible delay in payments. J. Oper. Res. Soc. 2019, 70, 1848–1868. [Google Scholar] [CrossRef]
- Yang, H.L. An inventory model for ramp-type demand with two-level trade credit financing linked to order quantity. Open J. Bus. Manag. 2019, 7, 427–446. [Google Scholar] [CrossRef][Green Version]
- Viswanath, J.; Thilagavathi, R.; Karthi, K.; Mahadal, M. A study of a two storage single product inventory system with ramp type demand, n-phase prepayment and purchase for exigency. Mathematics 2023, 11, 1728. [Google Scholar] [CrossRef]
- Harris, F.W. How many parts to make at once. Fact. Mag. Manag. 1913, 10, 135–136, 152. [Google Scholar] [CrossRef]
- Teng, J.T. Optimal ordering policies for a retailer who offers distinct trade credits to its good and bad credit customers. Int. J. Prod. Econ. 2009, 119, 415–423. [Google Scholar] [CrossRef]
- Yang, C.T.; Pan, Q.; Ouyang, L.Y.; Teng, J.T. Retailer’s optimal order and credit policies when a supplier offers either a cash discount or delay payment linked to order quantity. Eur. J. Ind. Eng. 2013, 7, 370–392. [Google Scholar] [CrossRef]
- Goyal, S.K. Economic order quantity under conditions of permissible delay in payments. J. Oper. Res. Soc. 1985, 36, 335–338. [Google Scholar] [CrossRef]
- Shah, N.H. Probabilistic time-scheduling model for an exponentially decaying inventory when delay in payments is permissible. Int. J. Prod. Econ. 1993, 32, 77–82. [Google Scholar] [CrossRef]
- Teng, J.T. On the economic order quantity under conditions of permissible delay in payments. J. Oper. Res. Soc. 2002, 53, 915–918. Available online: https://www.jstor.org/stable/822919 (accessed on 1 September 2024). [CrossRef]
- Gupta, D.; Wang, L. A stochastic inventory model with trade credit. Manuf. Serv. Oper. Manag. 2009, 11, 4–18. [Google Scholar] [CrossRef]
- Taleizadeh, A.A.; Pentico, D.W.; Jabalamei, M.S.; Aryanezhad, M. An EOQ model with partial delayed payment and partial backordering. Omega 2013, 41, 354–368. [Google Scholar] [CrossRef]
- Mahata, G.C.; De, S.K. An EOQ inventory system of ameliorating items for price dependent demand rate under retailer partial trade credit policy. Opsearch 2016, 53, 889–916. [Google Scholar] [CrossRef]
- Huang, Y.F. Optimal retailer’s ordering policies in the EOQ model under trade credit financing. J. Oper. Res. Soc. 2003, 54, 1011–1015. [Google Scholar] [CrossRef]
- Yang, H.L. Optimal ordering policy for deteriorating items with limited storage capacity under two-level trade credit linked to order quantity by a discounted cash-flow analysis. Open J. Bus. Manag. 2019, 7, 919–940. [Google Scholar] [CrossRef]
- Moradi, S.; Gholamian, M.R.; Sepehri, A. An inventory model for imperfect quality items considering learning effects and partial trade credit policy. Opsearch 2023, 60, 276–325. [Google Scholar] [CrossRef]
- Lin, F.; Shi, Y.; Zhou, X. Optimizing order policy and credit term for items with inventory-level-dependent demand under trade credit limit. J. Manag. Sci. Eng. 2023, 8, 413–429. [Google Scholar] [CrossRef]
- Pal, S.; Manna AKAli, I.; Roy, P.; Shaikh, A.A. A two-warehouse inventory model with credit policy and inflation effect. Decis. Anal. J. 2024, 10, 100406. [Google Scholar] [CrossRef]
- Zhang, A.X. Optimal advance payment scheme involving fixed pre-payment costs. Omega 1996, 24, 577–582. [Google Scholar] [CrossRef]
- Gupta, R.K.; Bhunia, A.K.; Goyal, S.K. An application of genetic algorithm in solving an inventory model with advance payment and interval valued inventory costs. Math. Comput. Model. 2009, 49, 893–905. [Google Scholar] [CrossRef]
- Maiti, A.K.; Maiti, M.K.; Maiti, M. Inventory model with stochastic lead-time and price-dependent demand incorporating advance payment. Appl. Math. Model. 2009, 33, 2433–2443. [Google Scholar] [CrossRef]
- Taleizadeh, A.A. An EOQ model with partial backordering and advance payments for an evaporating item. Int. J. Prod. Econ. 2014, 155, 185–193. [Google Scholar] [CrossRef]
- Teng, J.T.; Cárdenas-Barrón, L.E.; Chang, H.J.; Wu, J.; Hu, Y. Inventory lot-size policies for deteriorating items with expiration dates and advance payments. Appl. Math. Model. 2016, 40, 8605–8616. [Google Scholar] [CrossRef]
- Khan, M.A.A.; Shaikh, A.A.; Panda, G.; Konstantaras, I.; Cárdenas-Barrón, L.E. The effect of advance payment with discount facility on supply decisions of deteriorating products whose demand is both price and stock dependent. Int. Trans. Oper. Res. 2020, 27, 1343–1367. [Google Scholar] [CrossRef]
- Zhang, Q.; Tsao, Y.C.; Chen, T.H. Economic order quantity under advance payment. Appl. Math. Model. 2014, 38, 5910–5921. [Google Scholar] [CrossRef]
- Zia, N.P.; Taleizadeh, A.A. A lot-sizing model with backordering under hybrid linked-to-order multiple advance payments and delayed payment. Transp. Res. Part E Logist. Transp. Rev. 2015, 82, 19–37. [Google Scholar] [CrossRef]
- Diabat, A.; Taleizadeh, A.A.; Lashgari, M. A lot sizing model with partial downstream delayed payment, partial upstream advance payment, and partial backordering for deteriorating items. J. Manuf. Syst. 2017, 45, 322–342. [Google Scholar] [CrossRef]
- Duary, A.; Das, S.; Arif, M.G.; Abualnaja, K.M.; Khan, M.A.A.; Zakarya, M.; Shaikh, A.A. Advance and delay in payments with price-discount inventory model for deteriorating items under capacity constraint and partially backlogged shortages. Alex. Eng. J. 2022, 61, 1735–1745. [Google Scholar] [CrossRef]
- Li, R.; Chan, Y.L.; Chang, C.T.; Cárdenas-Barrón, L.E. Pricing and lot-sizing policies for perishable products with advance-cash-credit payments by a discounted cash-flow analysis. Int. J. Prod. Econ. 2017, 193, 578–589. [Google Scholar] [CrossRef]
- Li, R.; Skouri, K.; Teng, J.T.; Yang, W.G. Seller’s optimal replenishment policy and payment term among advance, credit, and credit payments. Int. J. Prod. Econ. 2018, 197, 35–42. [Google Scholar] [CrossRef]
- Wu, J.; Teng, J.T.; Chan, Y.L. Inventory policies for perishable products with expiration dates and advance-cash-credit payment schemes. Int. J. Syst. Sci. Oper. Logist. 2018, 5, 310–326. [Google Scholar] [CrossRef]
- Li, R.; Liu, Y.; Teng, J.T.; Tsao, Y.C. Optimal pricing, lot-sizing and backordering decisions when a seller demands for an advance-cash-credit payment scheme. Eur. J. Oper. Res. 2019, 278, 283–295. [Google Scholar] [CrossRef]
- Li, R.; Teng, J.T.; Chang, C.T. Lot-sizing and pricing decisions for perishable products under three-echelon supply chains when demand depends on price and stock-age. Ann. Oper. Res. 2021, 307, 303–328. [Google Scholar] [CrossRef]
- Li, R.; Yang, H.L.; Shi, Y.; Teng, J.T.; Lai, K.K. EOQ-based pricing and customer credit decisions under general supplier payments. Eur. J. Oper. Res. 2021, 289, 652–665. [Google Scholar] [CrossRef]
- Tsao, Y.C.; Muthi’ah, A.D.; Vu, T.L.; Arvitrida, N.I. Supply chain network design under advance-cash-credit payment. Ann. Oper. Res. 2021, 305, 251–272. [Google Scholar] [CrossRef]
- Feng, L.; Skouri, K.; Wang, W.C. Optimal selling price, replenishment cycle and payment time among advance, cash, and credit payments from the seller’s perspective. Ann. Oper. Res. 2022, 315, 791–812. [Google Scholar] [CrossRef] [PubMed]
- Feng, L.; Wang, W.C.; Teng, J.T.; Cárdenas-Barrón, L.E. Pricing and lot-sizing decision for fresh goods when demand depends on unit price, displaying stocks and product age under generalized payments. Eur. J. Oper. Res. 2022, 296, 940–952. [Google Scholar] [CrossRef]
- Shi, Y.; Zhang, Z.; Tiwari, S.; Tao, Z. Retailer’s optimal strategy for a perishable product with increasing demand under various payment schemes. Ann. Oper. Res. 2022, 315, 899–929. [Google Scholar] [CrossRef]
- Tsao, Y.C.; Pantisoontorn, A.; Vu, T.L.; Chen, T.H. Optimal production and predictive maintenance decisions for deteriorated products under advance-cash-credit payments. Int. J. Prod. Econ. 2024, 269, 109132. [Google Scholar] [CrossRef]
- Chang, C.T.; Tseng, Y.T. The impact of payment schemes and carbon emission policies on replenishment and pricing decisions for perishable products in a Supply Chain. Mathematics 2024, 12, 1033. [Google Scholar] [CrossRef]



| References | Demand Pattern | Deterioration (Instantaneous/ Non-Instantaneous/ Others) | Payment Policy | Discounted Cash-Flow | ||
|---|---|---|---|---|---|---|
| Advance | Cash | Credit | ||||
| Agrawal and Banerjee [15] | Ramp-type | |||||
| Agrawal et al. [17] | Ramp-type | V Instantaneous | ||||
| Chang et al. [6] | Price-dependent | Non-instantaneous | V | |||
| Chang and Tseng [57] | Price and stock-age dependent | Instantaneous | V | V | V | V |
| Diabat et al. [44] | Stock-dependent | Instantaneous | V | V | ||
| Duary et al. [45] | Time-dependent | Instantaneous | V | V | ||
| Feng et al. [53] | Price and payment time-dependent | V | V | V | ||
| Feng et al. [54] | Price, stock, product age dependent | V | V | V | ||
| Geetha and Uthayakumar [3] | Constant | Non-instantaneous | V | |||
| Gupta et al. [37] | Constant | V | ||||
| Gupta and Wang [28] | Stochastic demand | V | ||||
| Halim [18] | Ramp-type | Weibull distributed deterioration | ||||
| Huang [31] | Constant | V | ||||
| Jaggi et al. [8] | Price-dependent | Non-instantaneous | V | |||
| Khan et al. [41] | Price and stock- dependent | Instantaneous | V | |||
| Lashgari et al. [9] | Constant | Non-instantaneous | V | V | ||
| Li et al. [46] | Price-dependent | Instantaneous | V | V | V | V |
| Li et al. [47] | Payment time- dependent | V | V | V | ||
| Li et al. [49] | Price-dependent | V | V | V | ||
| Li et al. [50] | Price and stock-age | Instantaneous | V | V | V | V |
| Li et al. [51] | Price and credit period dependent | V | V | V | ||
| Lin et al. [34] | Stock-dependent | V | ||||
| Maihami and Kamalabadi [4] | Price-dependent | Non-instantaneous | ||||
| Mahata and De [30] | Price-dependent | Instantaneous | V | |||
| Maiti et al. [38] | Price-dependent | V | ||||
| Manna and Chaudhrui [12] | Ramp-type | Time-dependent deterioration | ||||
| Ouyang et al. [2] | Price-dependent | Non-instantaneous | V | |||
| Panda et al. [14] | Ramp-type | Instantaneous | ||||
| Pal et al. [35] | Price and time dependent | Non-instantaneous | V | V | ||
| Pathak et al. [11] | Biquadratic time-dependent | Non-instantaneous | V | |||
| Shah [26] | Stochastic demand | Instantaneous | V | |||
| Shi et al. [55] | Increasing demand | Instantaneous | V | V | V | |
| Shi et al. [19] | Ramp-type | Instantaneous | V | |||
| Skouri et al. [16] | Ramp-type | Instantaneous | ||||
| Soni and Patel [5] | Price-dependent | Non-instantaneous | V | |||
| Taleizadeh [39] | Constant | V | ||||
| Tavassoli et al. [10] | Constant | Non-instantaneous | V | |||
| Teng et al. [40] | Constant | Instantaneous | V | |||
| Tiwari et al. [7] | Constant | Non-instantaneous | V | V | ||
| Tsao et al. [56] | Constant | Instantaneous | V | V | V | V |
| Viswanath et al. [21] | Ramp-type | Instantaneous | V | |||
| Wu et al. [48] | Constant | Instantaneous | V | V | V | |
| Yang et al. [24] | Credit period-dependent | V | V | |||
| Yang [20] | Ramp-type | V | ||||
| Yang [32] | Time-varying | Instantaneous | V | V | ||
| Zhang et al. [42] | Constant | V | ||||
| Zia and Taleizadeh [43] | Constant | V | V | |||
| Present paper | Ramp-type | Non-instantaneous | V | V | V | V |
| Parameters | Decision Variables | Optimal Solution | |||||
|---|---|---|---|---|---|---|---|
| M | T | Q | R | ||||
| 0.25 | 0.25 | 0.05 | 0.2351 | 0.3167 | 3906.80 | 65.12 | 16.52 |
| 0.10 | 0.2672 | 0.3573 | 4018.80 | 75.56 | 18.86 | ||
| 0.15 | 0.3055 | 0.4079 | 4115.93 | 88.39 | 22.08 | ||
| 0.20 | 0.3499 | 0.4667 | 4202.62 | 103.44 | 25.94 | ||
| 0.25 | 0.3973 | 0.5298 | 4282.00 | 119.97 | 30.24 | ||
| 0.30 | 0.4502 | 0.5997 | 4353.45 | 138.69 | 35.03 | ||
| 0.35 | 0.5038 | 0.6707 | 4420.27 | 158.29 | 40.11 | ||
| Trend | |||||||
| Parameters | Decision Variables | Optimal Solution | |||||
|---|---|---|---|---|---|---|---|
| M | T | Q | R | ||||
| (a) | |||||||
| 0.15 | 0.25 | 0.05 | 0.3116 | 0.4106 | 4092.63 | 89.05 | 21.40 |
| 0.10 | 0.3091 | 0.4090 | 4098.38 | 88.66 | 21.58 | ||
| 0.15 | 0.3062 | 0.4068 | 4104.62 | 88.16 | 21.72 | ||
| 0.20 | 0.3061 | 0.4076 | 4110.04 | 88.33 | 21.92 | ||
| 0.25 | 0.3055 | 0.4079 | 4115.93 | 88.39 | 22.08 | ||
| 0.30 | 0.2900 | 0.3928 | 4122.43 | 85.04 | 22.21 | ||
| 0.35 | 0.2902 | 0.3930 | 4131.34 | 85.07 | 22.19 | ||
| 0.40 | 0.2904 | 0.3931 | 4140.23 | 85.09 | 22.17 | ||
| Trend | |||||||
| (b) | |||||||
| 0.15 | 0.10 | 0.05 | 0.3077 | 0.4074 | 4090.31 | 88.41 | 21.53 |
| 0.10 | 0.3052 | 0.4057 | 4096.10 | 88.02 | 21.71 | ||
| 0.15 | 0.3022 | 0.4035 | 4102.37 | 87.51 | 21.86 | ||
| 0.20 | 0.3021 | 0.4043 | 4107.80 | 87.68 | 22.05 | ||
| 0.25 | 0.3016 | 0.4046 | 4113.71 | 87.73 | 22.21 | ||
| 0.30 | 0.2861 | 0.3896 | 4120.41 | 84.38 | 22.35 | ||
| 0.35 | 0.2863 | 0.3897 | 4129.32 | 84.41 | 22.45 | ||
| 0.40 | 0.2865 | 0.3899 | 4138.24 | 84.43 | 22.52 | ||
| Trend | |||||||
| (a) | ||||||||
| Parameters | Decision variables | Optimal solution | ||||||
| M | T | Q | R | |||||
| 0.15 | 0.25 | 0.25 | 20 | 0.2623 | 0.3496 | 4169.00 | 75.60 | 18.92 |
| 30 | 0.2847 | 0.3798 | 4141.45 | 82.23 | 20.56 | |||
| 40 | 0.3055 | 0.4079 | 4115.93 | 88.39 | 22.08 | |||
| 50 | 0.3250 | 0.4342 | 4092.07 | 94.15 | 23.51 | |||
| 60 | 0.3434 | 0.4590 | 4069.57 | 99.59 | 24.85 | |||
| Trend | ||||||||
| (b) | ||||||||
| Parameters | Decision variables | Optimal solution | ||||||
| M | T | Q | R | |||||
| 0.15 | 0.10 | 0.25 | 20 | 0.2580 | 0.3458 | 4167.28 | 74.79 | 19.02 |
| 30 | 0.2807 | 0.3763 | 4139.45 | 81.51 | 20.68 | |||
| 40 | 0.3016 | 0.4046 | 4113.71 | 87.73 | 22.21 | |||
| 50 | 0.3212 | 0.4311 | 4089.66 | 93.55 | 23.65 | |||
| 60 | 0.3397 | 0.4561 | 4067.01 | 99.04 | 25.00 | |||
| Trend | ||||||||
| (a) | ||||||||
| Parameters | Decision variables | Optimal solution | ||||||
| M | T | Q | R | |||||
| 0.15 | 0.25 | 0.25 | 6 | 0.3290 | 0.4233 | 4983.05 | 91.93 | 20.39 |
| 8 | 0.3172 | 0.4153 | 4549.22 | 90.09 | 21.20 | |||
| 10 | 0.3055 | 0.4079 | 4115.93 | 88.39 | 22.08 | |||
| 12 | 0.2941 | 0.4012 | 3683.22 | 86.83 | 23.07 | |||
| 14 | 0.2829 | 0.3952 | 3251.11 | 85.43 | 24.18 | |||
| Trend | ||||||||
| (b) | ||||||||
| Parameters | Decision variables | Optimal solution | ||||||
| M | T | Q | R | |||||
| 0.15 | 0.10 | 0.25 | 6 | 0.3266 | 0.4213 | 4981.48 | 91.57 | 20.47 |
| 8 | 0.3140 | 0.4126 | 4547.29 | 89.58 | 21.30 | |||
| 10 | 0.3016 | 0.4046 | 4113.71 | 87.73 | 22.21 | |||
| 12 | 0.2894 | 0.3972 | 3680.78 | 86.02 | 23.22 | |||
| 14 | 0.2774 | 0.3906 | 3248.53 | 84.46 | 24.35 | |||
| Trend | ||||||||
| (a) | ||||||||
| Parameters | Decision variables | Optimal solution | ||||||
| M | T | Q | R | |||||
| 0.15 | 0.25 | 0.25 | 20 | 0.2775 | 0.4024 | 1962.26 | 86.83 | 26.76 |
| 25 | 0.2929 | 0.4043 | 3038.38 | 87.46 | 23.97 | |||
| 30 | 0.3055 | 0.4079 | 4115.93 | 88.39 | 22.08 | |||
| 35 | 0.3162 | 0.4121 | 5194.40 | 89.41 | 20.73 | |||
| 40 | 0.3254 | 0.4164 | 6273.49 | 90.42 | 19.70 | |||
| Trend | ||||||||
| (b) | ||||||||
| Parameters | Decision variables | Optimal solution | ||||||
| M | T | Q | R | |||||
| 0.15 | 0.10 | 0.25 | 20 | 0.2727 | 0.9384 | 1960.50 | 85.99 | 26.92 |
| 25 | 0.2886 | 0.4007 | 3036.36 | 86.72 | 24.11 | |||
| 30 | 0.3016 | 0.4046 | 4113.71 | 87.73 | 22.21 | |||
| 35 | 0.3126 | 0.4090 | 5192.01 | 88.81 | 20.85 | |||
| 40 | 0.3221 | 0.4136 | 6270.97 | 89.89 | 19.81 | |||
| Trend | ||||||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, H.-L.; Chang, C.-T.; Tseng, Y.-T. Optimal Replenishment Strategy for a High-Tech Product Demand with Non-Instantaneous Deterioration under an Advance-Cash-Credit Payment Scheme by a Discounted Cash-Flow Analysis. Mathematics 2024, 12, 3160. https://doi.org/10.3390/math12193160
Yang H-L, Chang C-T, Tseng Y-T. Optimal Replenishment Strategy for a High-Tech Product Demand with Non-Instantaneous Deterioration under an Advance-Cash-Credit Payment Scheme by a Discounted Cash-Flow Analysis. Mathematics. 2024; 12(19):3160. https://doi.org/10.3390/math12193160
Chicago/Turabian StyleYang, Hui-Ling, Chun-Tao Chang, and Yao-Ting Tseng. 2024. "Optimal Replenishment Strategy for a High-Tech Product Demand with Non-Instantaneous Deterioration under an Advance-Cash-Credit Payment Scheme by a Discounted Cash-Flow Analysis" Mathematics 12, no. 19: 3160. https://doi.org/10.3390/math12193160
APA StyleYang, H.-L., Chang, C.-T., & Tseng, Y.-T. (2024). Optimal Replenishment Strategy for a High-Tech Product Demand with Non-Instantaneous Deterioration under an Advance-Cash-Credit Payment Scheme by a Discounted Cash-Flow Analysis. Mathematics, 12(19), 3160. https://doi.org/10.3390/math12193160






