Fractional Calculus for Non-Discrete Signed Measures

Abstract

In this paper, we suggest a first-ever construction of fractional integral and differential operators based on signed measures including a vector-valued case. The study focuses on constructing the fractional power of the Riemann–Stieltjes integral with a signed measure, using semigroup theory. The main result is a theorem that provides the exact form of a semigroup for the Riemann–Stieltjes integral with a measure having a countable number of extrema. This article provides examples of semigroups based on integral operators with signed measures and discusses the fractional powers of differential operators with partial derivatives.

1. Introduction

1.1. Short Historic and Bibliographic Overview

The basic goal of fractional calculus is to derive a formula that, for a given operator L and a range of parameters $\alpha$, will produce a set of operators $\left\{L^{\alpha }\right\}$ endowed with characteristics typical of powers. Specifically, $L^n$ should align with the conventional iterated power $L^n=L·L·\dots ·L$ (n times) when $\alpha$ is a positive integer n, and the law of indices $L^{\alpha +\beta }=L^{\alpha }{L}^{\beta }$ should be applicable whenever $L^{\alpha }$, $L^{\beta }$, and $L^{\alpha +\beta }$ exist.

It is widely recognized that there are several methods available for defining fractional powers of $L={\displaystyle \frac{d}{dx}}$, which correspond to fractional derivatives and integrals (see, for example, [1,2,3]). Besides, there are multiple techniques available for the creation of operator families $\left\{L^{\alpha }\right\}$, with each technique being suitable for a distinct category of operators. For instance, several researchers such as Balakrishnan [4], Krasnoselski [5], Komatsu [6], Westphal [7], and Yosida [8] have explored the challenge of formulating an expression for ${(-L)}^{\alpha }$ in scenarios where L represents a closed operator within a Banach space.

The Riemann–Stieltjes integral, as follows:

$$\underset{a}{\overset{t}{\int }}f\left(y\right)dF\left(y\right),t\in [a,b],$$

(1)

extends the concept of the Riemann integral by allowing integration with respect to a more general class of functions called Stieltjes integrators F. The simplest existence theorem states that if f is continuous and F is of bounded variation on $[a,b]$, then the Riemann–Stieltjes integral exists. The corresponding to Equation (1) differential operator is ${\displaystyle \frac{d}{dF\left(t\right)}}$. The Riemann–Stieltjes integral has various applications in Probability Theory, Control Theory, Finance and Economics, Signal Processing, Function Approximation, etc.

Let us consider the approach to the conception of fractional integrals and derivatives of a function f with respect to another function F. The fractional integral of a function with respect to another function was introduced by Hj. Holmgren (see [9]). Fractional integrals of a function by another in the complex plane were studied by T. Osler [10,11].

In [12,13,14], for the space $X=C[a,b]$ of continuous functions on $[a,b]$ with the topology of the uniform convergence and for a positive and continuous function $p\left(t\right)$ in $[a,b]$, $a<b$, the following operator was used:

$$\left(I_{a+,p}^{\alpha }f\right)\left(t\right)={\displaystyle \frac{1}{\Gamma \left(\alpha \right)}}\underset{a}{\overset{t}{\int }}f\left(\tau \right){\left(\underset{\tau }{\overset{t}{\int }}p\left(s\right)ds\right)}^{\alpha -1}p\left(\tau \right)d\tau ,$$

(2)

where $\alpha >0$, $t\in [a,b]$, $f\in C[a,b]$ was studied. This operator is realised as a negative fractional power of ${\displaystyle \frac{1}{p\left(t\right)}}{\displaystyle \frac{d}{dt}}$ on $[a,b]$:

$$\left(I_{a+,p}^{\alpha }f\right)\left(t\right)={\left({\displaystyle \frac{1}{p\left(t\right)}}{\displaystyle \frac{d}{dt}}\right)}^{-\alpha }.$$

Let F be a strictly increasing function having a continuous derivative. Practically the same definition as Equation (2) was given in [1], p. 326, formula 18.25 in the following form:

$$\left(I_{a+,F}^{\alpha }f\right)\left(t\right)={\displaystyle \frac{1}{\Gamma \left(\alpha \right)}}\underset{a}{\overset{t}{\int }}f\left(\tau \right){\left(F\left(t\right)-F\left(\tau \right)\right)}^{\alpha -1}dF\left(\tau \right),\alpha >0.$$

(3)

The fractional derivative of a function f with respect to another function F is defined for $\alpha \in (0,1)$ as:

$$\left(D_{a+,F}^{\alpha }f\right)\left(t\right)={\displaystyle \frac{1}{F^{\prime }\left(t\right)}}{\displaystyle \frac{d}{dt}}\left(I_{a+,F}^{1-\alpha }f\right)\left(t\right).$$

(4)

When $F\left(t\right)>0$, we can also consider the Marchaud fractional derivative of order $\alpha \in (0,1)$:

$$\left({\mathbf{D}}_{+,F}^{\alpha }f\right)\left(t\right)={\displaystyle \frac{1}{\Gamma (-\alpha )}}\underset{0}{\overset{\infty }{\int }}(f\left(t\right)-f(t-\tau )){\left(F\left(\tau \right)\right)}^{-\alpha -1}dF\left(\tau \right).$$

(5)

Formulas (3) and (4) are valid only when $F\left(t\right)$ is a strictly increasing function. But in applications, function F can be not monotone and even discrete. Applications of a signed measure in differential equations were considered in [15]. A probabilistic interpretation of a signed measure can be found in [16]. Integrals of a function with respect to a signed measure are used in stochastics to model and analyze complex systems, such as financial markets, where both positive and negative quantities exist (see [17]).

In this study, we will concentrate on constructing the fractional power of the Riemann–Stieltjes integral, expressed as Equation (1), where function $F\left(t\right)$ is continuous and has a bounded variation on $[a,b]$. We will use the formulas of U. Westphal and semigroup theory.

It is worth mentioning that there exist different concepts of the general fractional calculus operators defined in a completely different form compared to those mentioned above. In particular, we refer to [2], where general fractional calculus considered with probabilistic applications and to [18,19,20,21,22,23].

1.2. Preliminaries: Powers Based on Semigroups

In this article, we explore a convenient method for constructing the fractional power of an operator using semigroups. Such an approach was carried out by U. Westphal in [7] (see also [3]).

Let $T_t$, $0\le t$ be a contraction semigroup in a real or complex Banach space X, and A be its infinitesimal generator.

In [7], the fractional power ${(-A)}^{\alpha }$, $0<\alpha <1$ was defined by the following formula:

$${(-A)}^{\alpha }f={\displaystyle \frac{1}{\Gamma (-\alpha )}}\underset{0}{\overset{\infty }{\int }}{t}^{-\alpha -1}(T_t-I)f\left(x\right)dt,f\in D\left(A\right).$$

(6)

In the case $1<\alpha <\ell$, $\ell =2,3,\dots$ Equation (6) can be written with the usage of “finite differences” ${(I-T_t)}^{\ell }$:

$${(-A)}^{\alpha }f={\displaystyle \frac{1}{C_{\alpha }(\ell )}}\underset{0}{\overset{\infty }{\int }}{t}^{-\alpha -1}{(I-T_t)}^{\ell }f\left(x\right)dt,$$

(7)

where $C_{\alpha }(\ell )=\Gamma (-\alpha )A_{\alpha }(\ell )$, $A_{\alpha }(\ell )={\displaystyle \sum _{k=0}^{\ell }}{(-1)}^{k-1}\left({\displaystyle \genfrac{}{}{0pt}{}{\ell }{k}}\right)={\displaystyle \sum _{k=0}^{\ell }}{(-1)}^{k-1}{\displaystyle \frac{\ell !}{k!(\ell -k)!}}.$

The negative power of the operator $(-A)$ for $0<\alpha <1$ can be defined by the following equation:

$${(-A)}^{-\alpha }f={\displaystyle \frac{1}{\Gamma \left(\alpha \right)}}\underset{0}{\overset{\infty }{\int }}{t}^{\alpha -1}{T}_{t}f\left(x\right)dt.$$

(8)

In order to get fractional integral of the order $\alpha$ greater than 1, one can just apply the iterated integral to Equation (8).

Example 1.

If the operator $A=\left(-{\displaystyle \frac{d}{dx}}\right)$, then A is the generating operator of the strongly continuous semigroup $T_{t}f\left(x\right)=f(x-t)$ on $L^2[0,\infty )$.

By Equation (6), we get:

$${\left({\displaystyle \frac{d}{dx}}\right)}^{\alpha }f\left(x\right)={\displaystyle \frac{1}{\Gamma (-\alpha )}}\underset{0}{\overset{\infty }{\int }}{t}^{-\alpha -1}(f(x-t)-f\left(x\right))dt,0<\alpha <1.$$

Easy calculations confirm that:

$${(I-T_t)}^{\ell }f\left(x\right)=\sum _{k=0}^{\ell }{(-1)}^k\left({\displaystyle \genfrac{}{}{0pt}{}{\ell }{k}}\right)f(x-kt)=\left({\Delta }_t^{\ell }f\right)\left(x\right)$$

is a finite difference. So, for $\alpha >1$, $\alpha$ not an integer, $\ell =\left[\alpha \right]+1$, we can write:

$${\left({\displaystyle \frac{d}{dx}}\right)}^{\alpha }f\left(x\right)={\displaystyle \frac{1}{C_{\alpha }(\ell )}}\underset{0}{\overset{\infty }{\int }}{t}^{-\alpha -1}{(I-T_t)}^{\ell }f\left(x\right)dt={\displaystyle \frac{1}{C_{\alpha }(\ell )}}\underset{0}{\overset{\infty }{\int }}{\displaystyle \frac{\left({\Delta }_t^{\ell }f\right)\left(x\right)}{t^{\alpha +1}}}dt.$$

Therefore, ${\left({\displaystyle \frac{d}{dx}}\right)}^{\alpha }$ represents the Marchaud fractional derivatives (see [1], p. 111).

For $\alpha >0$, the negative power of the operator ${\displaystyle \frac{d}{dx}}$ can be defined by equality Equation (8):

$${\left({\displaystyle \frac{d}{dx}}\right)}^{-\alpha }f\left(x\right)={\displaystyle \frac{1}{\Gamma \left(\alpha \right)}}\underset{0}{\overset{\infty }{\int }}{t}^{\alpha -1}f(x-t)dt={\displaystyle \frac{1}{\Gamma \left(\alpha \right)}}\underset{-\infty }{\overset{x}{\int }}{(x-t)}^{\alpha -1}f\left(t\right)dt=\left(I_{+}^{\alpha }f\right)\left(x\right).$$

So, we obtain the fractional Riemann–Liouville integral on the whole real axis (see Formula (5.2) from [1], p. 94).

If we want to get fractional Riemann–Liouville integral on half-axis (see Formula (5.1) from [1], p. 94):

$$\left(I_{0+}^{\alpha }f\right)\left(x\right)={\displaystyle \frac{1}{\Gamma \left(\alpha \right)}}\underset{0}{\overset{x}{\int }}{(x-t)}^{\alpha -1}f\left(t\right)dt,0<x,$$

we should take:

$$T_{t}f\left(x\right)=\left\{\begin{array}{c}f(x-t),t\le x;\hfill \\ f\left(0\right),t>x\hfill \end{array}\right.$$

for such functions that $f\left(0\right)=0$.

1.3. Detailed Content

The rest of the article is organized as follows. In Section 2, the focus is on integral operators of the form $I_{g}f\left(x\right)=\underset{a}{\overset{x}{\int }}f\left(y\right)g\left(y\right)dy$ and their inverse operators (derivatives) $D_{g}f\left(x\right)={\displaystyle \frac{d}{g\left(x\right)dx}}f\left(x\right)$. We provide examples of semigroups based on integral operators with power measure and with cosine measure.

Section 3 describes a mathematical result related to the construction of an operator semigroup based on $If\left(t\right)=\underset{a}{\overset{t}{\int }}f\left(y\right)dF\left(y\right)$, where $F\left(y\right)$ is continuous of locally finite variation and such that for a discrete set $a_k\in \mathbb{R}$, $k\in \mathbb{Z}$, $\left|k\right|<K$ with $K\in \mathbb{N}$ or $K=\infty$, F is strictly increasing on $[a_{2k},a_{2k+1}]$ and strictly decreasing on $[a_{2k-1},a_{2k}]$ for all k. In this section, we also present examples and corollaries related to fractional powers of operators, including their probabilistic representations.

Section 4 presents new fractional operators, focusing on examples of fractional powers of integral operators with signed measures. It presents calculations for fractional powers of specific integral operators, such as $L=D_k=-{\displaystyle \frac{d}{(k+1)x^{k}dx}}$ and $L=D_s={\displaystyle \frac{d}{sinxdx}}$, and their corresponding Feller processes. The text also explores fractional powers of differential operators with partial derivatives and discusses applications in defining fractional Poisson and Heisenberg brackets in classical and quantum mechanics.

2. Simple Examples of Semigroups Based on Integral Operators with Signed Measures

Let us consider an integral operator:

$$I_{g}f\left(x\right)=\underset{a}{\overset{x}{\int }}f\left(y\right)g\left(y\right)dy$$

and its inverse operator (derivative):

$$D_{g}f\left(x\right)={\displaystyle \frac{d}{g\left(x\right)dx}}f\left(x\right).$$

In order to define fractional powers of $I_g$ and $D_g$ using Formulas (6)–(8), we need to build the semigroup $T_t$ generated by $D_g$, and it must be a contraction semigroup.

If g is strictly positive, then $G\left(x\right)=1/g\left(x\right)$ is as well. The group (even group, not just semigroup) $T_t$ generated by G has explicit representation (solution of the first-order linear pde via characteristics):

$$T_{t}f\left(x\right)=f\left(X_x\left(t\right)\right),$$

where $X_x\left(t\right)$ is the solution of the ode $\dot{x}=G\left(x\right)$ with initial condition x at a.

Now, interesting thing occurs when g changes sign and G gets infinite values. On first sight, one may think that everything breaks down, but remarkably it is not. The group may be still well defined. Assume that zeros of g are discrete and degenerate in the sense that $g^{\prime }$ does not vanish there. Then, the dynamics $\dot{x}=G\left(x\right)$ moves from any $x_0$ monotonically to the nearest zero of g, where $g^{\prime }$ is negative (by assumed non-degeneracy it always takes finite time to reach this point) and then stays there for ever after. Well-defined contraction semigroup and Formulas (6)–(8) applies.

Consider some examples.

Example 2.

Integral operator:

$$I_{k}f\left(x\right)=-\underset{0}{\overset{x}{\int }}(k+1)y^{k}f\left(y\right)dy$$

with an odd integer k has an inverse operator (derivative):

$$D_{k}f\left(x\right)=-{\displaystyle \frac{d}{(k+1)x^{k}dx}}f\left(x\right).$$

To calculate the semigroup via characteristics, one has to solve the ODE:

$$(k+1)x^k\dot{x}=-1,or{x}^{k+1}=-t+x_0^{k+1},$$

where $x_0$ is an initial point. We obtain that the semigroup $T_t=exp\left(t{D}_k\right)$ is given by:

$$T_{t}f\left(x\right)=\left\{\begin{array}{c}f\left(0\right),t\ge x^{k+1},\hfill \\ f(\mathrm{sgn}\left(x\right){(x^{k+1}-t)}^{1/(k+1)}),t\le x^{k+1}.\hfill \end{array}\right.$$

This semigroup is Feller (strongly continuous) in $C_{\infty }\left(\mathbb{R}\right)$ with the domain of the generator consisting of continuously differentiable functions from $C\left(\mathbb{R}\right)$ s.t. $f^{\prime }\left(x\right)=o\left(x^k\right)$ for $x\to \infty$ and a finite limit $\underset{x\to 0}{lim}{\displaystyle \frac{f^{\prime }\left(x\right)}{x^k}}$ exists.

Example 3.

Integral operator:

$$I_{s}f\left(x\right)=\underset{0}{\overset{x}{\int }}sinyf\left(y\right)dy.$$

has an inverse operator (derivative):

$$D_{s}f\left(x\right)={\displaystyle \frac{d}{sinxdx}}f\left(x\right).$$

In order to calculate the semigroup via characteristics, one has to solve the ODE:

$$sinx\dot{x}=1,ord\cos x=-dt.$$

Due to the periodicity of the sine, solutions preserve the intervals $[2\pi k,2\pi (k+1\left)\right]$, $k\in \mathbb{Z}$. The solution starting at $x\in [0,2\pi ]$ is:

$$X_x\left(t\right)=\left\{\begin{array}{c}\pi ,t\ge 1+\cos x,\hfill \\ arccos(\cos x-t),t\le 1+\cos x,x\in (0,\pi ],\hfill \\ 2\pi -arccos(\cos x-t),t\le 1+\cos x,x\in [\pi ,2\pi ).\hfill \end{array}\right.$$

The semigroup $T_t=exp\left(t{D}_s\right)$ is Feller on each interval $[2\pi k,2\pi (k+1\left)\right]$ given by:

$$T_{t}f\left(x\right)=\left\{\begin{array}{c}f(2\pi k+\pi ),t\ge 1+\cos x,\hfill \\ f(2\pi k+arccos(\cos x-t\left)\right),t\le 1+\cos x,x\in [2\pi k,2\pi k+\pi ],\hfill \\ f\left(2\pi \right(k+1)-arccos(\cos x-t\left)\right),t\le 1+\cos x,x\in [2\pi k+\pi ,2\pi (k+1\left)\right].\hfill \end{array}\right.$$

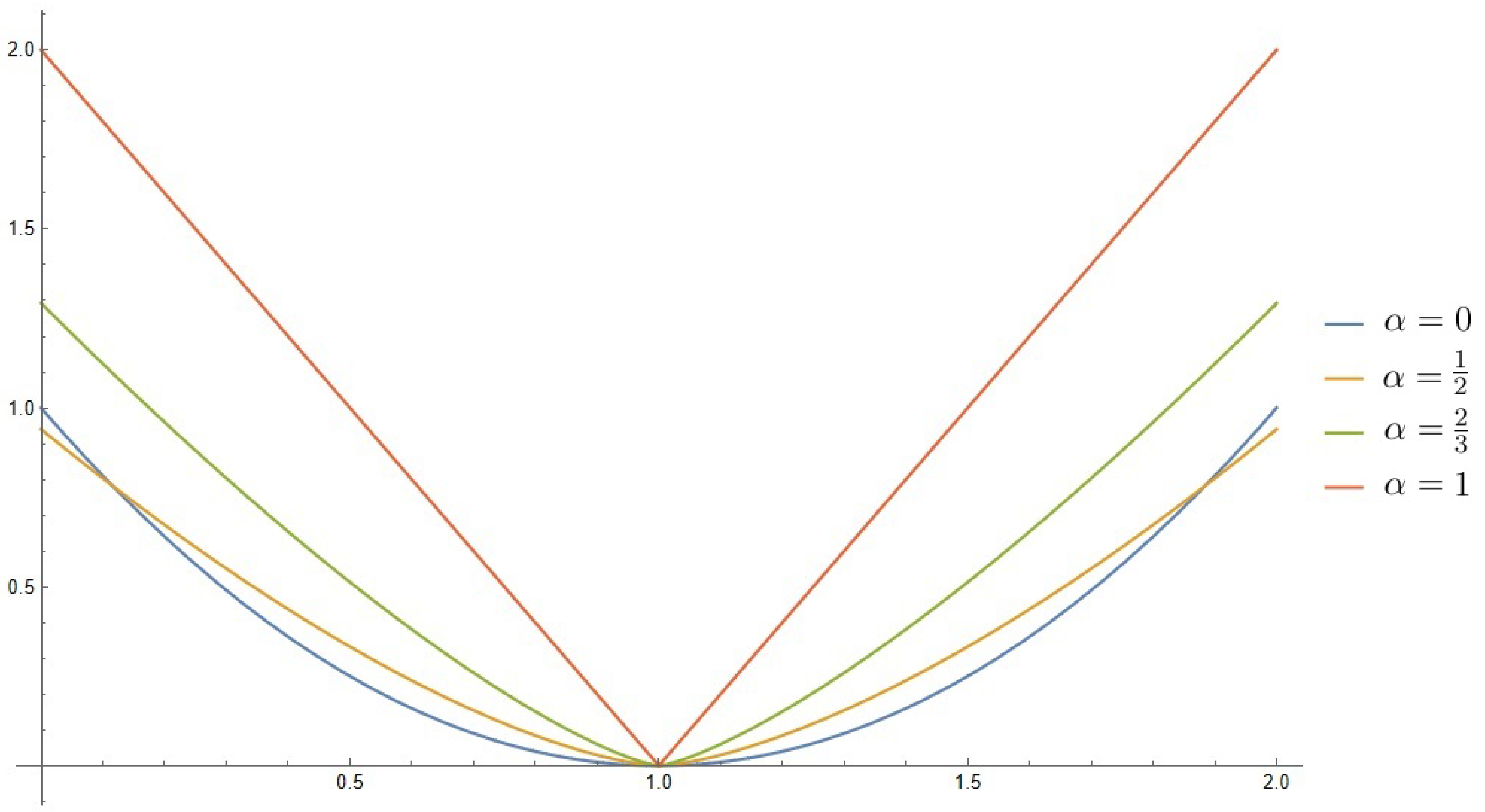
3. Main Result of the General Semigroup Operator

Let us consider the integral $If\left(t\right)=\underset{a}{\overset{t}{\int }}f\left(y\right)dF\left(y\right)$ with some $a\in \mathbb{R}$. Assume that F is continuous of locally finite variation and such that for a discrete set $a_k\in \mathbb{R}$, $k\in \mathbb{Z}$, $\left|k\right|<K$ with $K\in \mathbb{N}$ or $K=\infty$, F is strictly increasing on $[a_{2k},a_{2k+1}]$ and strictly decreasing on $[a_{2k-1},a_{2k}]$ for all k. Let S be some appropriate function. Then the inverse differential operator solving equation $IG=S$ is given by the formula:

$$G\left(x\right)=\left(LS\right)\left(x\right)={\displaystyle \frac{dS\left(x\right)}{dF\left(x\right)}}=\underset{\delta \to 0}{lim}{\displaystyle \frac{S(x+\delta )-S\left(x\right)}{F(x+\delta )-F\left(x\right)}},$$

(9)

which holds for any continuous G and all points x. By our assumptions on F, the derivative $F^{\prime }\left(x\right)$ exists and does not vanish for almost all points x. For these points:

$$G\left(x\right)=LS\left(x\right)={\displaystyle \frac{S^{\prime }\left(x\right)}{F^{\prime }\left(x\right)}}.$$

Theorem 1.

Under the assumptions on F given above, the operator L generates a strongly continuous semigroup on the set of functions that are continuous away from the set $\left\{a_{2k}\right\}$ with left and right limits at these points. The semigroup has invariant spaces $C\left([a_{2k},a_{2k+2}]\right)$, where it acts by the formula:

$$T_{t}S\left(x\right)=\left\{\begin{array}{c}S\left[{(F-F\left(a_{2k}\right))}^{-1}(t+F\left(x\right)-F\left(a_{2k}\right))\right],x\in [a_{2k},a_{2k+1}],t\le F\left(a_{2k+1}\right)-F\left(x\right),\hfill \\ S\left[{(F-F\left(a_{2k+2}\right))}^{-1}(t+F\left(x\right)-F\left(a_{2k+2}\right))\right],x\in [a_{2k+1},a_{2k+2}],t\le F\left(a_{2k+1}\right)-F\left(x\right),\hfill \\ S\left(a_{2k+1}\right),x\in [a_{2k},a_{2k+2}],t\ge F\left(a_{2k+1}\right)-F\left(x\right).\hfill \end{array}\right.$$

(10)

Remark 1.

Let us stress for clarity that ${(F-F\left(a_{2k}\right))}^{-1}$ denote the inverse functions to $F\left(x\right)-F\left(a_{2k}\right)$, which are well defined and continuous on $x\in [a_{2k},a_{2k+1}]$, due to the assumed continuity and strong monotonicity of F on these intervals.

Proof. 

In order to see where Formula (10) comes from, let us look at $x\in [a_{2k},a_{2k+1}]$ and assume that $F^{\prime }\left(x\right)$ is well defined and positive for all $x\in (a_{2k},a_{2k+1})$. To shorten formulas, let us assume $F\left(a_{2k}\right)=0$. Observe then that the action of the semigroup $S_t\left(x\right)=T_{t}S\left(x\right)$, generated by L, on a function $S\left(x\right)$ is given by the solution of the linear first-order partial differential equation:

$${\displaystyle \frac{\partial S_t}{\partial t}}\left(x\right)=L{S}_t\left(x\right)={\displaystyle \frac{1}{F^{\prime }\left(x\right)}}{\displaystyle \frac{\partial S_t\left(x\right)}{\partial x}}.$$

The solution to this Cauchy problem can be defined via characteristics, which solve the following equation:

$$\dot{X}={\displaystyle \frac{1}{F^{\prime }\left(X\right)}}.$$

Namely, denoting $X_x\left(t\right)$ the solution to this equation starting from a point x, we will have:

$$S_t\left(x\right)=S\left(X_x\left(t\right)\right).$$

Solving the equation $\dot{X}=1/F^{\prime }\left(X\right)$ yields $F^{\prime }\left(X\right)\dot{X}=1$, or $F\left(X_x\left(t\right)\right)=t+F\left(x\right)$. Thus, $X_x\left(t\right)=F^{-1}(t+F\left(x\right))$ yielding for $S_t\left(x\right)$ the first line of Equation (10). The specific feature of our problem is that this holds only for finite times $t<F\left(a_{2k+1}\right)-F\left(x\right)$. For $t=F\left(a_{2k+1}\right)-F\left(x\right)$, and thus, $X_x\left(t\right)=a_{2k+1}$, the equation $\dot{X}=1/F^{\prime }\left(X\right)$ is not defined at all, since $F^{\prime }\left(x\right)$ either does not exist or equals to zero. It is then natural to define $X_x\left(t\right)=a_{2k}$ for $t\ge F\left(a_{2k+1}\right)-F\left(x\right)$, because the point $a_{2k}$ is stable in the sense that the vector field $1/F^{\prime }\left(x\right)$ points towards this point both from the right and from the left. For $x=a_{2k}$, where the equation is also not defined, the formula for $X_x\left(t\right)$ is obtained by continuity having in mind that this point is repulsive for characteristic equation. Furthermore, Equation (10) follows.

Now, once Formula (10) is obtained (by whatever heuristical reasons and with whatever simplifying assumptions), it is straightforward to see that it does specify a strongly continuous semigroup under the assumptions of the theorem. One just has to show that whenever the generator is defined, it is given by Equation (9). Furthermore, this is mostly straightforward. In fact, from Equation (9):

$$\left(LS\right)\left(x\right)=\underset{\delta \to 0}{lim}{\displaystyle \frac{S(x+\delta )-S\left(x\right)}{F(x+\delta )-F\left(x\right)}}.$$

Let us denote $ϵ=F(x+\delta )-F\left(x\right)$. By the continuity of F, $ϵ\to 0$ as $\delta \to 0$. Moreover, $x+\delta =F^{-1}(F\left(x\right)+ϵ)$. Hence:

$$LS\left(x\right)=\underset{ϵ\to 0}{lim}{\displaystyle \frac{S\left[F^{-1}(ϵ+F\left(x\right))\right]-S\left(x\right)}{ϵ}}=\underset{ϵ\to 0}{lim}{\displaystyle \frac{T_{t}S\left(x\right)-S\left(x\right)}{ϵ}},$$

which is the defining formula for the generator of $T_t$. The proof is complete. □

Example 4.

Let:

$$F\left(x\right)=\left\{\begin{array}{cc}x\hfill & if 0\le x\le 1;\hfill \\ 2-x\hfill & if 1<x\le 2\hfill \end{array}\right.$$

and $f\in C[0,2]$ such that $f\left(1\right)=0$. Then, $a_0=0$, $a_1=1$, $a_2=2$ and the semigroup constructed by Equation (10) is as follows:

$$T_{t}f\left(x\right)=\left\{\begin{array}{cc}& f\left(x+t\right),x\in [0,1),t\le 1-x,\hfill \\ & f\left(x-t\right),x\in [1,2],t\le x-1,\hfill \\ & 0,x\in [0,2],t>1-F\left(x\right).\hfill \end{array}\right.$$

Corollary 1.

Under the assumptions of the theorem:

$${(-L)}^{\alpha }S\left(x\right)={\displaystyle \frac{1}{\Gamma (-\alpha )}}\underset{0}{\overset{\infty }{\int }}{t}^{-\alpha -1}(T_{t}f\left(x\right)-f\left(x\right))dt$$

is well defined with $T_t$ given by Equation (10). The corresponding fractional integrals:

$${(-L)}^{-\alpha }f\left(x\right)={\displaystyle \frac{1}{\Gamma \left(\alpha \right)}}\underset{0}{\overset{\infty }{\int }}{t}^{\alpha -1}{T}_{t}f\left(x\right)dt$$

are well defined for functions $f\left(x\right)$ that vanish at the points $x=a_{2k+1}$ for all k.

Corollary 2.

The fractional powers ${(-L)}^{\alpha }$ are seen to be given in the Lévy–Khintchine form, so that they generate Feller semigroups and Feller processes, say $X_x^{\alpha }\left(t\right)$. Therefore, the potential operators ${(-L)}^{-\alpha }$ can be expressed in probabilistic representation as path integrals:

$$-{(-L)}^{-\alpha }f\left(x\right)=\mathbf{E}\underset{0}{\overset{{\tau }_x}{\int }}f\left(X_x^{\alpha }\left(t\right)\right)dt,$$

where ${\tau }_x$ is the time the process $X_x^{\alpha }\left(t\right)$ reaches one of the points $a_{2k}$.

4. New Fractional Operators

In this section, we consider some examples of fractional powers.

4.1. Examples of Fractional Powers of Integral Operators with Signed Measures

Example 5.

Using semigroup from Example 2 and Formula (6), we get that the fractional power $\alpha \in (0,1)$ of $L=D_k=-{\displaystyle \frac{d}{(k+1)x^{k}dx}}$ with an odd integer k and with inverse sign is:

$$\begin{array}{ccc}\hfill -{(-L)}^{\alpha }f\left(x\right)& =& -{\left({\displaystyle \frac{d}{(k+1)x^{k}dx}}\right)}^{\alpha }f\left(x\right)\hfill \\ & =& -{\displaystyle \frac{1}{\Gamma (-\alpha )}}\underset{0}{\overset{\infty }{\int }}{t}^{-\alpha -1}(T_t-I)f\left(x\right)dt\hfill \\ & =& -{\displaystyle \frac{1}{\Gamma (-\alpha )}}\underset{0}{\overset{x^{k+1}}{\int }}{t}^{-\alpha -1}[f(\mathrm{sgn}\left(x\right){(x^{k+1}-t)}^{1/(k+1)})-f\left(x\right)]dt\hfill \\ & +& {\displaystyle \frac{x^{-\alpha (k+1)}}{\Gamma (1-\alpha )}}(f\left(0\right)-f\left(x\right)).\hfill \end{array}$$

(11)

This is clearly the generator of a Feller process, say $X_x^{\alpha }\left(t\right)$, whose potential operator $-{(-L)}^{-\alpha }$ is calculated via the semigroup and represents the fractional integral. Namely, $-{(-L)}^{-\alpha }$, the analog of Riemann–Liouville fractional integral is defined for continuous functions vanishing at zero as:

$$-{(-L)}^{-\alpha }f\left(x\right)=\mathbf{E}\underset{0}{\overset{{\tau }_x}{\int }}f\left(X_x^{\alpha }\left(t\right)\right)dt,$$

where ${\tau }_x$ is the time the process $X_x^{\alpha }\left(t\right)$ reaches zero.

Formula (8) we can use only if $f\left(0\right)=0$, then applying Equation (8), we get for $0<\alpha <1$:

$$\begin{array}{ccc}\hfill {(-L)}^{-\alpha }f& =& {\left({\displaystyle \frac{d}{d{x}^{k+1}}}\right)}^{-\alpha }f\left(x\right)\hfill \\ & =& {\displaystyle \frac{1}{\Gamma \left(\alpha \right)}}\underset{0}{\overset{\infty }{\int }}{t}^{\alpha -1}{T}_{t}f\left(x\right)dt\hfill \\ & =& {\displaystyle \frac{1}{\Gamma \left(\alpha \right)}}\underset{0}{\overset{x^{k+1}}{\int }}{t}^{\alpha -1}f\left(\mathrm{sgn}\left(x\right){(x^{k+1}-t)}^{1/(k+1)}\right)dt.\hfill \end{array}$$

(12)

Remark 2.

If $x>0$ putting $\mathrm{sgn}\left(x\right){(x^{k+1}-t)}^{1/(k+1)}=\tau$ in Equations (11) and (12) we get:

$$\begin{array}{ccc}\hfill {(-L)}^{\alpha }f& =& {\left({\displaystyle \frac{d}{d{x}^{k+1}}}\right)}^{\alpha }f\left(x\right)\hfill \\ & =& {\displaystyle \frac{1}{\Gamma (-\alpha )}}\underset{0}{\overset{x}{\int }}{(x^{k+1}-{\tau }^{k+1})}^{-\alpha -1}\left(f\left(\tau \right)-f\left(x\right)\right)d\tau \hfill \\ & +& {\displaystyle \frac{x^{-\alpha (k+1)}}{\Gamma (1-\alpha )}}(f\left(x\right)-f\left(0\right))\hfill \end{array}$$

and

$${(-L)}^{-\alpha }f={\left({\displaystyle \frac{d}{d{x}^{k+1}}}\right)}^{-\alpha }f\left(x\right)={\displaystyle \frac{1}{\Gamma \left(\alpha \right)}}\underset{0}{\overset{x}{\int }}{(x^{k+1}-{\tau }^{k+1})}^{\alpha -1}f\left(\tau \right)d{\tau }^{k+1}$$

that are the Erdélyi–Kober derivative in the Marchaud form and (3) for $a=0$, $g\left(x\right)=x^{k+1}$, respectively.

Example 6.

Using semigroup from Example 3 and Formula (6) the fractional power of $L=D_s={\displaystyle \frac{d}{sinxdx}}$ with inverse sign is given for $f\in C\left(\right[2\pi k,2\pi (k+1)\left]\right)$ by:

$$\begin{array}{cc}\hfill -{(-L)}^{\alpha }f\left(x\right)& =-{\left(-{\displaystyle \frac{d}{sinxdx}}\right)}^{\alpha }f\left(x\right)\hfill \\ \hfill & =-{\displaystyle \frac{1}{\Gamma (-\alpha )}}\underset{0}{\overset{1+\cos x}{\int }}{t}^{-\alpha -1}[f(2\pi k+arccos(\cos x-t))-f\left(x\right)]dt\hfill \\ \hfill & +{\displaystyle \frac{{(1+\cos x)}^{-\alpha }}{\Gamma (1-\alpha )}}(f\left(0\right)-f\left(x\right))\hfill \end{array}$$

for $x\in [2\pi k,2\pi k+\pi ]$ and symmetrically for $x\in [2\pi k+\pi ,2\pi (k+1\left)\right]$.

This is clearly the generator of a Feller process, say $X_x^{\alpha }\left(t\right)$, whose potential operator $-{(-L^{\alpha })}^{-1}$ is calculated via the semigroup and represents the fractional integral. Namely, $-{(-L^{\alpha })}^{-1}$, the analog of Riemann–Liouville fractional integral is defined for functions $f\in C\left(\right[2\pi k,2\pi (k+1)\left]\right)$ vanishing at $2\pi k+\pi$ as:

$$-{(-L^{\alpha })}^{-1}f\left(x\right)=\mathbf{E}\underset{0}{\overset{{\tau }_x}{\int }}f\left(X_x^{\alpha }\left(t\right)\right)dt,$$

where ${\tau }_x$ is the time the process $X_x^{\alpha }\left(t\right)$ reaches $2\pi k+\pi$.

Example 7.

Let us consider an integral operator $I_{F}f\left(x\right)=\underset{0}{\overset{x}{\int }}f\left(y\right)dF\left(y\right)$ and its inverse operator (derivative) $D_{F}f\left(x\right)={\displaystyle \frac{d}{dF\left(x\right)}}f\left(x\right),$ where $F\left(x\right)$ is from Example 4. Let $f\left(1\right)=0$, then by Equation (6), we get:

$$\begin{array}{cc}\hfill {\left(-{\displaystyle \frac{d}{dF\left(x\right)}}\right)}^{\alpha }f\left(x\right)& ={\displaystyle \frac{1}{\Gamma (-\alpha )}}[I_{[0,1]}\left(x\right)\underset{0}{\overset{1-x}{\int }}{t}^{-\alpha -1}(f(x+t)-f\left(x\right))dt\hfill \\ \hfill & +I_{[1,2]}\left(x\right)\underset{0}{\overset{x-1}{\int }}{t}^{-\alpha -1}\left(f(x-t)\right)-f\left(x\right))dt+]\hfill \\ \hfill & ={\displaystyle \frac{1}{\Gamma (-\alpha )}}[I_{[0,1]}\left(x\right)\underset{x}{\overset{1}{\int }}{(t-x)}^{-\alpha -1}(f\left(t\right)-f\left(x\right))dt\hfill \\ \hfill & -I_{[1,2]}\left(x\right)\underset{1}{\overset{x}{\int }}{(x-t)}^{-\alpha -1}(f\left(t\right)-f\left(x\right))dt].\hfill \end{array}$$

For $f\left(x\right)={(x-1)}^2$, $\alpha =0;{\displaystyle \frac{1}{2}};{\displaystyle \frac{2}{3}};1$ plots of ${\left(-{\displaystyle \frac{d}{dF\left(x\right)}}\right)}^{\alpha }f\left(x\right)$ are presented in Figure 1.

4.2. Fractional Powers of Differential Operators with Partial Derivatives

In the remaining brief comments of the paper, we like to demonstrate that the semigroup method used above for integrals with signed measure can be effectively and easily applied in many other situations.

For instance, let us look at differential operators of first order with partial derivatives:

$$LS\left(x\right)=g\left(x\right){\displaystyle \frac{\partial S}{\partial x}}=\sum _{j=1}^n{g}_j\left(x\right){\displaystyle \frac{\partial S}{\partial x_j}},$$

with g Lipschitz. Let $X_x\left(t\right)$ denote the solution of the equation $\dot{x}=g\left(x\right)$ starting from x at time zero. Then, L generates a conservative Feller semigroup in $C_{\infty }\left({\mathbb{R}}^n\right)$:

$$T^{t}f\left(x\right)=f\left(X_x\left(t\right)\right).$$

The power of this operator can be written in the following form:

$$\begin{array}{cc}{(-L)}^{\alpha }f\left(x\right)={\displaystyle \frac{1}{\Gamma (-\alpha )}}\underset{0}{\overset{\infty }{\int }}{t}^{-\alpha -1}(T_t-I)f\left(x\right)dt\hfill & ={\displaystyle \frac{1}{\Gamma (-\alpha )}}\underset{0}{\overset{\infty }{\int }}{t}^{-\alpha -1}[f\left(X_x\left(t\right)\right)-f\left(x\right)]dt\hfill \end{array}$$

(13)

for Lipschitz (sufficiently Holder) f. This operator (with inverse sign) also generates a Feller semigroup in $C_{\infty }\left({\mathbb{R}}^n\right)$. The corresponding fractional integral (potential operator of the semigroup) is:

$${(-L)}^{-\alpha }f\left(x\right)={\displaystyle \frac{1}{\Gamma \left(\alpha \right)}}\underset{0}{\overset{\infty }{\int }}{t}^{\alpha -1}{T}_{t}f\left(x\right)dt={\displaystyle \frac{1}{\Gamma \left(\alpha \right)}}\underset{0}{\overset{\infty }{\int }}{t}^{\alpha -1}f\left(X_x\left(t\right)\right)dt.$$

Operator ${(-L)}^{-\alpha }$ can be define for functions f supported on some domain $\Omega$ such that $X_x\left(t\right)$ exits $\Omega$ in finite time.

4.3. Fractional Poisson and Heisenberg Brackets

As an insightful example, we can use the construction of the previous section to define the fractional power of the Poisson bracket:

$$LS(x,p)=\{H,S\}={\displaystyle \frac{\partial H}{\partial p}}{\displaystyle \frac{\partial S}{\partial x}}-{\displaystyle \frac{\partial H}{\partial x}}{\displaystyle \frac{\partial S}{\partial p}}$$

for a Hamiltonian function $H(x,p)$ as the operator:

$${(-L)}^{\alpha }f\left(x\right)={\displaystyle \frac{1}{\Gamma (-\alpha )}}{\int }_0^{\infty }{t}^{-\alpha -1}\left[f{(X,P)}_{x,p}\left(t\right)\right)-f(x,p)]dt,$$

where ${(X,P)}_{x,p}\left(t\right)$ solves the corresponding Hamiltonian system.

Similarly, one can define fractional Heisenberg operators of quantum mechanics. The standard Heisenberg equation has the following form:

$$\dot{A}\left(t\right)=L_H\left(A\right)=-i[H,A\left(t\right)],$$

where $A\left(t\right),H$ are self-adjoint operators in a Hilbert space, H being called a Hamiltonian.

An operator $A\to i[A,H]$ is known to generate a semigroup $T_t$ acting on the space of operators as:

$$T_{t}A=exp\{-iHt\}Aexp\left\{iHt\right\}.$$

Thus, one obtains the fractional power:

$${(-L_H)}^{\alpha }A={\displaystyle \frac{1}{\Gamma (-\alpha )}}{\int }_0^{\infty }{t}^{-\alpha -1}(\exp \{-iHt\}Aexp\left\{iHt\right\}-A)dt.$$

This operator was constructed in a slightly different way (via resolvents) in paper [24]. Different approaches to classical and quantum fractional brackets ware developed in [25] and [26]. Fractional Heisenberg equations represent one approach to building fractional quantum mechanics. Another approach develops the theory of fractional Schrödinger equation, see, e.g., [27] for closed quantum systems and [28] for open quantum systems.

5. Conclusions

In this article, for the first time, fractional integrals and derivatives were constructed with respect to a signed measure. An approach based on semigroup theory was used. The suggested fractional powers have stochastic and quantum mechanic applications.