Event-Triggered Output Feedback H∞ Control for Markov-Type Networked Control Systems

Abstract

This paper studies the output feedback $H\infty$ control problem of event-triggered Markov-type networked control systems. Firstly, a new Lyapunov–Krasovskii functional is constructed, which contains an event-triggered scheme, Markovian jump system, and quantified information. Secondly, the upper bound of the weak infinitesimal generation operator of the Lyapunov–Krasovskii function is estimated by combining Wirtinger’s-based integral inequality and reciprocally convex inequality. Finally, based on the Lyapunov stability theory, the closed-loop stability criterion of event-triggered Markov-type networked control systems and the design method of the output feedback $H\infty$ controller for the disturbance attenuation level $\gamma$ are given in the terms of linear matrix inequalities. The effectiveness and superiority of the proposed method are verified using three numerical examples.

1. Introduction

In recent years, as a special kind of random mixed system, the Markovian jump system has shown an advantage of better describing physical systems with sudden change. It has been widely used in industrial control systems, network control systems, fault detection systems, and other fields [1,2,3,4,5,6,7]. In [1], the authors based their research on the method of combining the auxiliary function-based double integral inequality and the extended Wirtinger inequality and Jensen inequality and studied the delay-dependent stability of a Markovian jump network delay system with partial information on transition probabilities. In [2], the authors studied the design of a reduced-order unknown input observer for a one-sided Lipschitz nonlinear continuous-time descriptor Markovian jump system. In [3], the authors studied the sliding mode control problem of continuous-time Markovian jump systems with partially unknown and uncertain transition rate matrices. It has attracted many scholars to devote themselves to the stability analysis and controller research of Markovian jump systems. In [4], the authors, based on the properties of Markovian jump systems, considered packet loss as a Bernoulli stochastic process with constant probability, independence, and identical distribution. A method to design $H\infty$ filters for networked control systems with network delay and packet loss was proposed. In [5], the author proposed a state feedback controller for solving the discrete-time model reference tracking control problem of external disturbances and Markov jump systems under input constraints. At the same time, a state feedback controller was proposed to ensure that the system state vector accurately tracks the given state reference model vector. In [6], the authors introduced a logarithmic quantizer into the design of a state feedback controller and input the uncertainty into the quantization error matrix represented by an interval matrix. The quantization control problem of a continuous-time uncertain Markovian jump system with mixed delays and partial known transition probabilities was studied. In [7], the authors established a sufficient condition for exponential almost sure stability by applying a method to its stochastic transfer matrix and studied exponential almost sure stability of continuous-time Markovian jump systems with additional stochastic switching performance and stability problems. In [7], the author established sufficient conditions for exponential almost certain stability by applying a method to its random transition matrix and studied the exponential almost certain stability of continuous-time Markovian jump systems with additional random switching performance and stability problems.

With the rapid development of computer and network technology, a network was introduced into the traditional control system to form a networked control system. The network control system has the advantages of high cost-effectiveness, simple installation and maintenance, and high reliability, and it is is widely used in aerospace, defense industry, telemedicine, etc. In a practical network control system, the components of the control system (controller, controlled object, actuator et al.) lie in different positions and the signals of each part are transmitted through the communication network. The signal is in the transmission process, and due to the limitation of network bandwidth, the network control system inevitably has problems such as network delay, packet loss, and timing disorder [8,9,10,11,12]. In order to overcome the latency of communication networks and solve the problem of limited network resources, signal quantization technology has emerged in recent years [13,14,15,16]. For example, in [13], the author considered the joint design of quantizers and estimators to study the state estimation problem of linear dynamic systems. In [14], the stabilization condition of a Markovian jump system with partial transition probability and input quantization was studied. In [15], the authors studied the stabilization of switched linear systems with sampling and quantized output feedback. In [16], the author assumed that each mode of the system was stable and observable, and each mode had feedback gain. The stabilization problem of switched linear systems with sampled data and quantized output feedback was studied.

Recently, a new event-triggered scheme based on different traditional time-triggered schemes has been proposed in the literature, which can be used as an alternative method to minimize the use of communication resources. Compared with a traditional time-triggered scheme, an event-triggered scheme can significantly reduce the sampling frequency of samplers and unnecessary data transmission while maintaining control system performance. In the case of limited communication bandwidth, reducing unnecessary data transmission can improve the efficiency of data transmission and reduce the problem of data transmission delay in communication networks. In recent years, the event-triggered scheme has attracted the attention of domestic scholars [17,18,19,20,21,22,23,24,25]. For example, in [17], the authors studied the design of an event-triggered controller for networked control systems with network delay. In [18], the author studied event-triggered controller design for networked control systems via a new integral inequality of a Lyapunov–Krasovskii functional. In [19], the authors studied the event-triggered output feedback $H\infty$ control problem of a networked control system with non-uniform sampling. In [20], the authors studied the control design problem of the event-triggered linear network system of state quantification and control input quantification. In [21], the authors studied the event-triggered static output feedback control of the network control system. In order to reduce the utilization of limited network bandwidth, in [22], the authors studied the $H\infty$ control problem of the networked Markovian jump system with an event-triggered scheme. In [23], the authors proposed a centralized/distributed hybrid event-triggering scheme for nonlinear multi-agent systems and studied the leader–follower consistency problem of nonlinear stochastic multi-agent systems. In [24], in order to save the limited network communication bandwidth of multi-agent systems, the author proposed a novel event-triggered network consensus mechanism and studied the event-triggered guarantee cost consensus of discrete-time singular multi-agent systems with switching topology. In [25], the authors studied consensus problems for a class of uncertain nonlinear multi-agent systems by using neural networks and event-driven mechanisms.

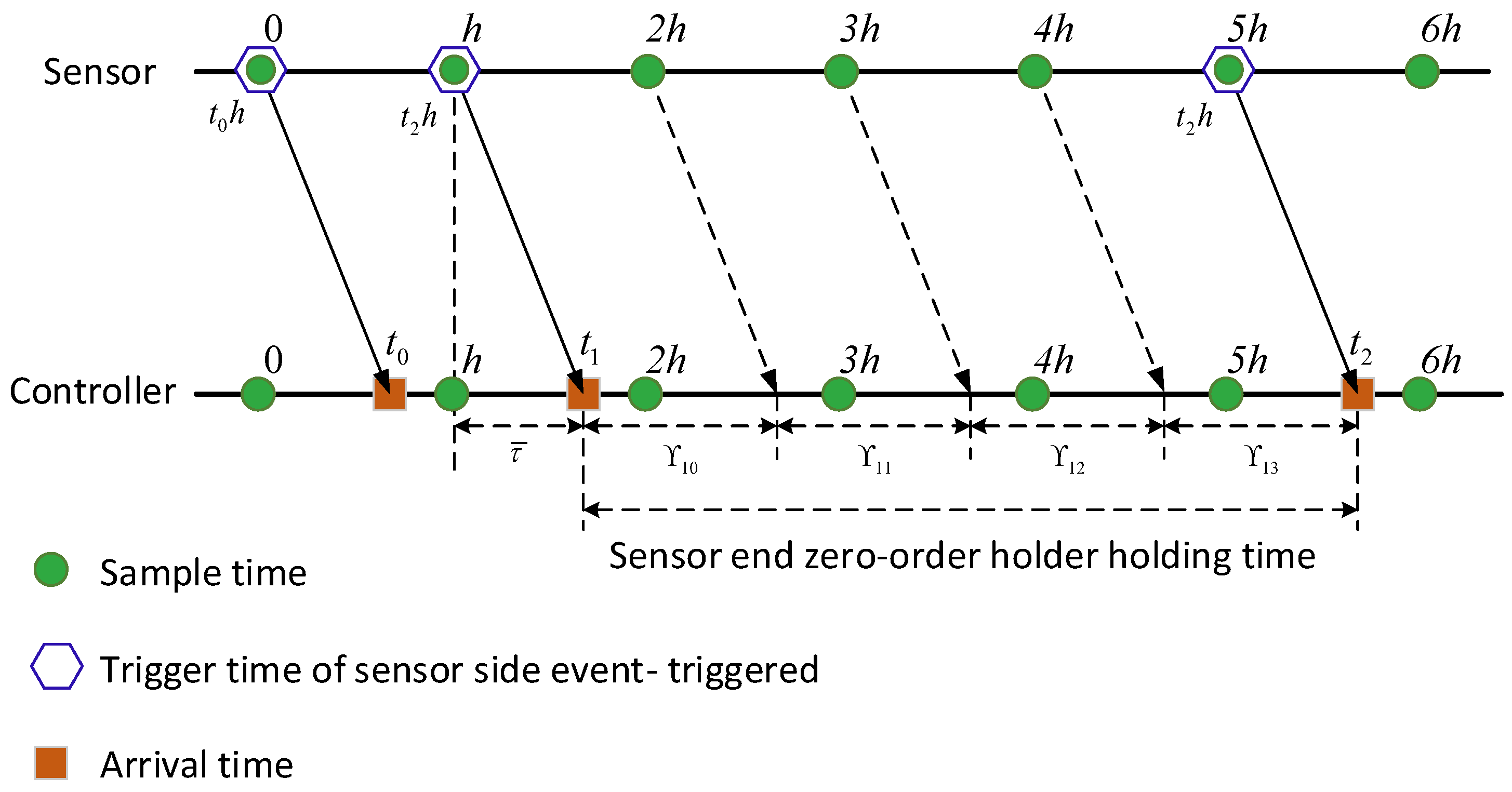
Through the analysis of the literature above, it was found that there are still some shortcomings in the research on network delay in networked control systems. Due to the limitation of network bandwidth resources, the existing research often adopts time-triggered and event-triggered schemes to improve the utilization of bandwidth resources and to reduce network delay. At the same time, when estimating the upper bound of Lyapunov–Krasovskii functional derivatives, there may be situations where too many matrix variables are introduced and the network delay is amplified. Inspired by the above discussion, this paper focuses on the output feedback $H\infty$ control problem of event-triggered Markov-type network control systems. The main contributions are as follows: (1) In order to solve the problem of network delay caused by limited network bandwidth resources, by introducing an event-triggered mechanism and signal quantization, the transmission of redundant information in the network can be reduced, the transmission efficiency of information in the network can be improved, and the network delay can be reduced. (2) When constructing the Lyapunov–Krasovskii function, considering the influence of network delay on the stability of the system and the influence of different modes on the Markov switching strategy in a small switching time region, the Lyapunov–Krasovskii function contains Markov jump information, network delay information, and the coupling relationship between network delay and the upper bound of network delay. (3) When estimating the upper bound of the weak infinitesimal generator operator of the Lyapunov–Krasovskii function, the combination of Wirtinger’s-based integral inequality and the reciprocally convex inequality is used to estimate the weak infinitesimal generator operator of the Lyapunov–Krasovskii function. It can reduce the matrix variable and the computational complexity, and it can avoid the network delay being amplified to reduce the conservatism of the system.

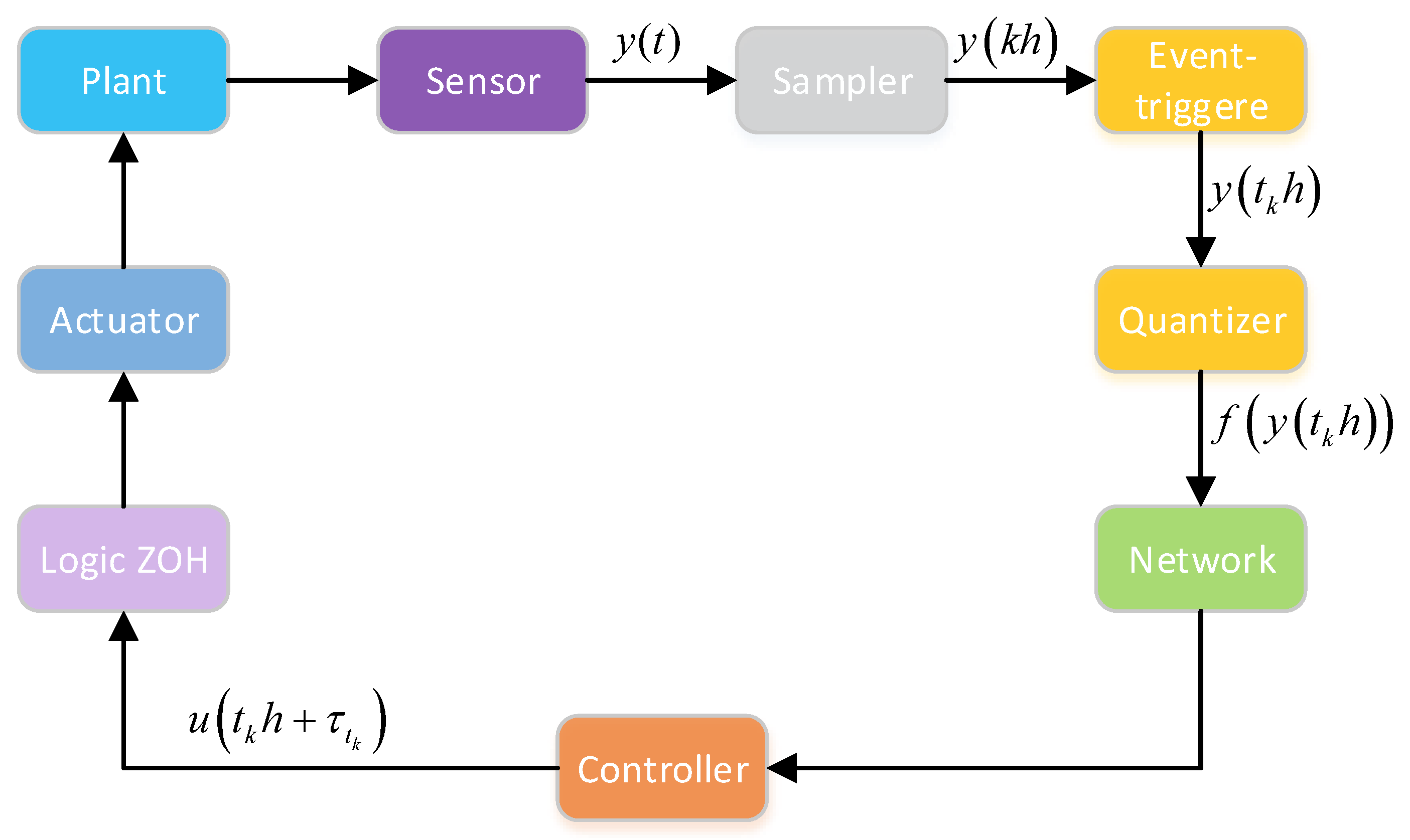
The structure of this paper is as follows. In Section 2, a new Markov-type networked control systems model with external disturbances is established, considering the effects of event triggering and quantization. In Section 3, the output feedback $H\infty$ control problem of event-triggered Markov-type networked control systems is studied. In Section 4, two numerical examples are given to verify the effectiveness and superiority of the method. In Section 5, the conclusion of this article is summarized, and future research directions and work are emphasized.

Notation: In this paper, ${\mathbb{R}}^n$ and ${\mathbb{R}}^{n\times m}$ denote the n-dimensional Euclidean space and the set of $n\times n$ real matrices; the notation $P>0$$(P\ge 0)$ means that P is a real symmetric and positive-definite (semi-positive-definite) matrix; the notation $\epsilon \{·\}$ stands for the mathematical expectation; the superscripts ‘−1’ and ‘T’ stand for the inverse and transpose of a matrix, respectively; symmetric terms in a symmetric matrix are denoted by ‘∗’. For any square matrices A and B, it is defined that $diag(A,B)=\left[\begin{array}{cc}A& 0\\ \ast & B\end{array}\right]$. The notation I stands for the identity matrix.

2. Problem Formulation

2.1. System Description

The structure of a Markov-type network control system under an event-triggered mechanism is shown in Figure 1, where the plant is characterized as a Markovian jump system with a time-varying delay and is represented by the following:

$$\left\{\begin{array}{c}\dot{x}\left(t\right)=A\left(r_t\right)x\left(t\right)+B\left(r_t\right)u\left(t\right)+B_w\left(r_t\right)\omega \left(t\right),\hfill \\ y\left(t\right)=C_1\left(r_t\right)x\left(t\right),\hfill \\ z\left(t\right)=C_2\left(r_t\right)x\left(t\right)+D\left(r_t\right)u\left(t\right),\hfill \end{array}\right.$$

(1)

where $x\left(t\right)\in R^n$ is the state variable, $y\left(t\right)\in R^m$ is the measured output, $u\left(t\right)\in R^w$ is the controlled input, $z\left(t\right)\in R^p$ is the controlled output, $\omega \left(t\right)\in R^q$ is the external disturbance with $\omega \left(t\right)\in L_2[0,\infty )$, respectively, and $A\left(r_t\right)$, $B\left(r_t\right)$, $B_w\left(r_t\right)$, $C_1\left(r_t\right)$, $C_2\left(r_t\right)$, and $D\left(r_t\right)$ are known real constant matrices with appropriate dimensions. $\{r_t,t\ge 0\}$ is a homogeneous finite-state Markov jump process of right continuous trajectory values in the finite set $S=\{1,2,\cdots ,r\}$. The transition rate matrix $\Pi =\left({\pi }_{ij}\right)(i,j\in {\mathbb{R}}^{S\times S})$ is given by

$$Pr\{r_{t+\Delta }=j|r_t=i\}=\left\{\begin{array}{cc}{\pi }_{ij}\Delta +o(\Delta ),\hfill & i\ne j,\hfill \\ 1+{\pi }_{ij}\Delta +o(\Delta ),\hfill & i=j,\hfill \end{array}\right.$$

(2)

where $\Delta >0$, ${lim}_{\Delta \to 0}{\displaystyle \frac{0(\Delta )}{\Delta }}$, and $\pi \ge 0$ for $i\ne j$ is the transition rate from mode i at time t to mode j at time $t+\Delta$, ${\pi }_{ii}=-{\sum }_{j=1,j\ne i}^r{\pi }_{ij}$.

For the Markov-type network control system shown in Figure 1, in order to facilitate the subsequent analysis, we make the following assumptions:

• The sensor is time-triggered. The measurement output is sampled periodically with a constant period h. The set of periodic sampling intervals is denoted by $S_1=\left\{jh\right|j=1,2,\cdots \}$.
• The controller and actuator adopt an event-triggered mechanism. The zero-order hold (ZOH) logic is used to hold the control input when there is no latest control signal arriving at the actuator. The set of transmission sequences is described by $S_2=\left\{t_{k}h\right|t_k\in \mathbb{N}\}\subseteq S_1$. All transmitted signals are time-sampled.
• ${\tau }_{t_k}$ represents the network delay, which is calculated from the time $t_k$ when the event generator releases the sampled signal to the controller side through the communication network, where $\overline{\tau }$ represents the upper bound of ${\tau }_{t_k}$.

Remark 1. 

If all the sampling data are transmitted to the actuator through the network, then $S_1=S_2$, which is the triggered mechanism transformed into a time-triggered one. Furthermore, the ZOH logic is issued to store the latest data packet, which means that the control input of the actuator will remain unchanged until the new data packet is transmitted to the ZOH logic again, and the actuator will not change. If some disordered data packets contain outdated information, they will be actively discarded by the ZOH logic.

2.2. Modeling Based on the Event-Triggered Scheme

As we all know, in the networked control systems’ output feedback control, the output value of the plant is transmitted to the network controller through the communication network. Based on the information received by the plant, the controller transmits the control output back through the communication network (located in the plant side) actuator. Due to the limitation of the communication network in Figure 1, an event generator and quantizer $f(·)$ are applied. An event generator is introduced between the sensor and the quantizer $f(·)$. The sensor data $y\left(kh\right)$ sampled by the sampler are transmitted to the event generator. Whether the data after the event generator needs to be transmitted to the new output of the plan through a quantizer and the information sent to the network controller depends on the following conditions [17]:

$${(y\left(t_{k}h\right)-y(t_{k}h+lh))}^T\Omega \left(r_{tkh+lh}\right)(y\left(t_{k}h\right)-y(t_{k}h+lh))\le \sigma \left(r_{t_{k}h+lh}\right){\left(y(t_{k}h+lh)\right)}^T\Omega \left(r_{tkh+lh}\right)\left(y(t_{k}h+lh)\right),$$

(3)

where $\sigma \left(r_{t_{k}h+lh}\right)\in [0,1)$, $\Omega \left(r_{t_{k}h+lh}\right)$ is a symmetric positive-definite matrix, $l=1,2,\cdots ,$$y\left(r_{t_{k}h+lh}\right)$ denotes the latest sampling output of the sampler at the current time, $y\left(t_{k}h\right)$ represents the latest transmitted data that do not meet the event-triggered threshold condition. If the current sampled data violates the event-triggered threshold condition (3), the data are transmitted to the controller through the network. For the convenience of later analysis, the interval of the ZOH logic is divided into several subintervals in Figure 2:

$$[t_{k}h+{\tau }_{t_k},t_{k+1}+{\tau }_{t_k+l+1}]=\bigcup _{l=1}^d{{\rm Y}}_{l,k},$$

(4)

where ${{\rm Y}}_{l,k}=[t_{k}h+lh+{\tau }_{t_k+l},t_{k+l}h+lh+h+{\tau }_{t_k+l+1}],l=1,2,\cdots ,d$.

It is defined that the allowable equivalent time delay of the network is $\eta \left(t\right)=t-t_{k}h-lh$ and that $0\le {\tau }_{t_k}\le \eta \left(t\right)\le h+\overline{\tau }\equiv {\eta }_M$ is obtained.

In the process of stability analysis and the derivation of the stability criterion, the event-triggered threshold condition (3) is included, and the state error is expressed as $e_k\left(t\right)=x\left(t_{k}h\right)-x(t_{k}h+lh)$. Thus, for $t\in [t_{k}h+{\tau }_{t_k},t_{k+1}h+{\tau }_{t_k+l+1}]$, the event-triggered threshold condition (3) can be rewritten as follows:

$$e_k^T\left(t\right)C_1^T\left(r_t\right)\Omega \left(r_{t_{k}h+lh}\right)C_1\left(r_t\right)e_k\left(t\right)\le \sigma (r_{t_{k}h}+lh)x^T(t-\eta \left(t\right))C_1^T\left(r_t\right)\Omega \left(r_{t_{k}h+lh}\right)C_1\left(r_t\right)x(t-\eta \left(t\right)).$$

(5)

Remark 2. 

The $\sigma (r_{t_{k}h}+lh)$ parameter is used to determine the frequency at which the sampled signal is released. $\sigma (r_{t_{k}h}+lh)$ means that all sampling signals are released at each sampling instant. At the time, the event-triggered scheme (3) becomes a periodic sampling scheme.

Remark 3. 

Under the event-triggered scheme, we assume that the release time is $t_{1}h,t_{2}h,\cdots$, the time delay of network communication is ${\tau }_k$, and the measured value will reach the actuator at $t_{1}h+{\tau }_1,t_{2}h+{\tau }_2,\cdots$, after quantification.

Remark 4. 

The role of the ZOH logic on the actuator side is to store the latest control output, which means that outdated information will be actively discarded by the ZOH logic. When the actuator does not arrive at the latest valid information, the logical zero-order holder keeps the control signal unchanged until the output of the logical zero-order holder is updated to new data.

In order to further decrease the restriction of network bandwidth, a quantizer $f(·)$ is introduced and defined as $f\left(y\left(t_{k}h\right)\right)=[f_1\left(y_1\left(t_{k}h\right)\right),f_2\left(y_2\left(t_{k}h\right)\right),\cdots ,f_m\left(y_m\left(t_{k}h\right)\right)]$, where $f_s\left(y_s\left(t_{k}h\right)\right),(s=1,2,\cdots ,m)$ is expressed as follows:

$$f_s\left(y_s\left(t_{k}h\right)\right)=\left\{\begin{array}{cc}{u}_l^{\left(s\right)},\hfill & y_s\left(t_{k}h\right)>0,\hfill \\ 0,\hfill & y_s\left(t_{k}h\right)=0,\hfill \\ -f_s(-y_s\left(t_{k}h\right)),\hfill & y_s\left(t_{k}h\right)<0,\hfill \end{array}\right.$$

(6)

with ${\displaystyle \frac{1}{1+{\delta }_{f_s}}}{u}_l^{\left(s\right)}<y_s\left(t_{k}h\right)\le {\displaystyle \frac{1}{1-{\delta }_{f_s}}}{u}_l^{\left(s\right)}$, ${\delta }_{f_s}={\displaystyle \frac{1-{\rho }_{f_s}}{1+{\rho }_{f_s}}}(0<{\rho }_{f_s}<1)$. ${\rho }_{f_s}$ is the quantization density, which is a constant. Similar to [26,27], we define the set of quantized energy levels as $u_s=\{\pm u_l^{\left(s\right)},u_l^{\left(s\right)}={\rho }_{f_s}^l{u}_0^{\left(s\right)},l=\pm 1,\pm 2,\cdots \}\cup \{\pm u_0^{\left(s\right)}\}\cup \left\{0\right\}$ with $u_0^{\left(s\right)}$.

According to the above analysis, the event-triggered scheme, quantization scheme, and network delay in the communication network are considered. Using sector demarcation method [28], the measured output received by the controller can be expressed as

$$\tilde{y}\left(t\right)=f\left(y\left(t_{k}h\right)\right)=(I+{\Delta }_f)C_1\left(r_t\right)[x(t-\eta \left(t\right))+e_k\left(t\right)],$$

(7)

with ${\Delta }_f=diag\{{\Delta }_{f_1},{\Delta }_{f_2},\cdots ,{\Delta }_{f_m}\},{\Delta }_{f_s}\in [-{\delta }_{f_s},{\delta }_{f_s}],s=1,2,\cdots ,m$.

An output feedback controller is defined as follows:

$$u\left(t\right)=K\left(r_t\right)(I+{\Delta }_f)C_1\left(r_t\right)[x(t-\eta \left(t\right))+e_k\left(t\right)],$$

(8)

where $K\left(r_t\right)$ is the undetermined matrix.

Remark 5. 

The influence of quantization can be transformed into norm-bounded uncertainties, and ${\Delta }_f$ can explain the gain change in a realistic way. By applying the sector-bound method, we can eliminate ${\Delta }_f$ and obtain δ-independent conditions.

We will substitute Formula (8) into Formula (1) to obtain the following closed-loop networked control systems:

$$\left\{\begin{array}{c}\dot{x}\left(t\right)=A\left(r_t\right)x\left(t\right)+B\left(r_t\right)K\left(r_t\right)(I+{\Delta }_f)C_1\left(r_t\right)[x(t-\eta \left(t\right))+e_k\left(t\right)]+Bw\left(r_t\right)\omega \left(t\right),\hfill \\ z\left(t\right)=C_2\left(r_t\right)x\left(t\right)+D\left(r_t\right)K\left(r_t\right)(I+{\Delta }_f)C_1\left(r_t\right)[x(t-\eta \left(t\right))+e_k\left(t\right)].\hfill \end{array}\right.$$

(9)

In this paper, in order to facilitate the description, when $r_t=i,i\in S$, the matrix $N\left(r_t\right)$ is represented by $N_i$; for example, $A\left(r_t\right)$ is represented by $A_i$, $B\left(r_t\right)$ by $B_i$, $C_1\left(r_t\right)$ by $C_{1i}$, $C_2\left(r_t\right)$ by $C_{2i}$, $K\left(r_t\right)$ by $K_i$, $B_w\left(r_t\right)$ by $B_{wi}$, and so on.

Remark 6. 

It can be seen that not all the sampled data are transmitted to the controller through the network through the quantizer on the side of the sensor and the controller. Due to the introduction of the event generator, only the sampling data that do not meet the event-triggered threshold will be sent to the quantizer.

In the last part of this section, we will list some relevant definitions and lemmas used in the stability analysis and controller design process later on.

Definition 1 

([29]). For the convenience of later analysis, the solution $x(x_0,t,t_r)$ of system (1) with $r_0\in S$ is denoted by $x\left(t\right)$. It is known that $\{x_t,r_t\}$ is a Markov process with an initial state $(x_0,r_0)$, and its weak infinitesimal generator operator, action on function V, is defined.

$$\mathcal{L}V(x_t,t,r_t)=\underset{\Delta \to 0^{+}}{lim}{\displaystyle \frac{1}{\Delta }}[\epsilon \left(V(x(t+\Delta ),t+\Delta ,r_{t+\Delta })\right|x\left(t\right),t,r_t)-V(x\left(t\right),t,r_t)].$$

Definition 2. 

Given a scalar $\gamma >0$, if system (1) is asymptotically stable, system (1) has a given $H\infty$ disturbance attenuation level γ; under zero initial conditions, for any $\omega \left(t\right)\in L_2[0,\infty ),\omega \ne 0$, there is

$${\parallel z\left(t\right)\parallel }_{{\epsilon }_2}<\gamma {\parallel \omega \left(t\right)\parallel }_2,$$

The system (1) is asymptotically stable and satisfies $H\infty$ performance index γ.

Lemma 1 

([30]). For a given matrix $R>0$, the following inequality holds for all continuously differentiable functions ω in $[a,b]\to {\mathbb{R}}^p$, as follows:

$${\int }_a^b{\dot{w}}^T\left(u\right)R\dot{w}\left(u\right)du\ge {\displaystyle \frac{1}{b-a}}{\Omega }_0^{T}R{\Omega }_0+{\displaystyle \frac{3}{b-a}}{\Omega }_1^{T}R{\Omega }_1,$$

where

$${\Omega }_0=w\left(b\right)-w\left(a\right),{\Omega }_1=w\left(b\right)+w\left(a\right)-{\displaystyle \frac{2}{b-a}}{\int }_a^{b}w\left(u\right)du.$$

Lemma 2 

([31]). For given a scalar α in the interval $(0,1)$, a given positive matrix R in ${\mathbb{R}}^p$, two matrices $W_1$ and $W_2$ in ${\mathbb{R}}^{p\times q}$. It is defined that, for all vectors ξ in ${\mathbb{R}}^q$, the function $\Theta (\alpha ,R)$ is given by the following:

$$\Theta (\alpha ,R)={\displaystyle \frac{1}{\alpha }}{\xi }^T{W}_1^{T}R{W}_1\xi +{\displaystyle \frac{1}{1-\alpha }}{\xi }^T{W}_2^{T}R{W}_2\xi .$$

Then, if a matrix X in ${\mathbb{R}}^{p\times q}$ such as $\left[\begin{array}{cc}R& X\\ \ast & R\end{array}\right]>0$ is existed, the following inequality holds:

$$\underset{\alpha \in (0,1)}{min}\Theta (\alpha ,R)\ge {\left[\begin{array}{c}{W}_1\xi \\ W_2\xi \end{array}\right]}^T\left[\begin{array}{cc}R& X\\ \ast & R\end{array}\right]\left[\begin{array}{c}{W}_1\xi \\ W_2\xi \end{array}\right].$$

Lemma 3 

([32]). Given matrices $D,E$, and $F\left(t\right)$ of appropriate dimensions with $R>0$ satisfying $F^T\left(t\right)F\left(t\right)\le I$, for any $\epsilon >0$, the following inequality holds:

$$DEF+E^T{F}^{T}D\le \epsilon D{D}^T+{\epsilon }^{-1}{E}^{T}E.$$

3. Main Results

3.1. Stability Analysis of Event-Triggered Markov-Type Networked Control Systems

In this section, the stability analysis of output feedback $H\infty$ control for event-triggered Markov-type networked control systema is studied. A new Lyapunov–Krasovskii functional was constructed using the Wirtinger integral inequality and the cross convex combination method. Based on the Lyapunov stability theory, the closed-loop stability criterion of event-triggered Markov-type networked control systems is given.

Theorem 1.

For given a scalar $\gamma ,{\eta }_M,{\sigma }_i$ and the control matrix $K_i$, if there exist matrices $P_i>0$, $Q,R,{\Omega }_i>0(i\in S)$, and if the following LMI holds, then under the event-triggered threshold condition (3), the closed-loop system (9) is asymptotically stable with $H\infty$ performance index γ.

$${\Xi }_i=\left[\begin{array}{ccc}{\Xi }_{11i}& \ast & \ast \\ {\Xi }_{21i}& -I& \ast \\ {\Xi }_{31i}& 0& -P_i{R}^{-1}{P}_i\end{array}\right]<0,$$

(10)

where

$${\Xi }_{11i}=\left[\begin{array}{ccccccc}{\Phi }_{11i}& \ast & \ast & \ast & \ast & \ast & \ast \\ {\Phi }_{21i}& {\Phi }_{22i}& \ast & \ast & \ast & \ast & \ast \\ 0& -{\displaystyle \frac{2}{{\eta }_M}}R& -{\displaystyle \frac{4}{{\eta }_M}}R& \ast & \ast & \ast & \ast \\ {\Phi }_{41i}& 0& 0& -C_{1i}^T{\Omega }_i{C}_{1i}& \ast & \ast & \ast \\ B_{\omega i}^T{P}_i& 0& 0& 0& -{\gamma }^{2}I& \ast & \ast \\ {\displaystyle \frac{6}{{\eta }_M}}R& {\displaystyle \frac{6}{{\eta }_M}}R& 0& 0& 0& -{\displaystyle \frac{12}{{\eta }_M}}R& \ast \\ 0& {\displaystyle \frac{6}{{\eta }_M}}R& {\displaystyle \frac{6}{{\eta }_M}}R& 0& 0& 0& -{\displaystyle \frac{12}{{\eta }_M}}R\end{array}\right],$$

$${\Phi }_{11i}=A_i^T{P}_i+P_i{A}_i+Q+\sum _{j=1}^r{\pi }_{ij}{P}_i-{\displaystyle \frac{4}{{\eta }_M}}R,$$

$${\Phi }_{21i}=C_{1i}^T{(I+\Delta f)}^T{K}_i^T{B}_i^T{P}_i-{\displaystyle \frac{2}{{\eta }_M}}R,$$

$${\Phi }_{22i}={\sigma }_i{C}_{1i}^T{\Omega }_i{C}_{1i}-{\displaystyle \frac{8}{{\eta }_M}}R,$$

$${\Phi }_{41i}=C_{1i}^T{(I+\Delta f)}^T{K}_i^T{B}_i^T{P}_i,$$

$${\Xi }_{21i}=[C_{2i},D_i{K}_i(I+\Delta )C_{1i},0,D_i{K}_i(I+\Delta )C_{1i},0,0,0],$$

$${\Xi }_{31i}=\sqrt{{\eta }_M}[P_i{A}_i,P_i{B}_i{K}_i(I+\Delta )C_{1i},0,P_i{B}_i{K}_i(I+\Delta )C_{1i},P_i{B}_{wi},0,0],$$

Proof. 

We construct a stochastic Lyapunov–Krasovskii function candidate as

$$\begin{array}{cc}\hfill V(x_t,t,r_t)=& V_1(x_t,t,r_t)+V_2(x_t,t,r_t)+V_3(x_t,t,r_t),\hfill \end{array}$$

(11)

where

$$V_1(x_t,t,r_t)=x^T\left(t\right)P\left(r_t\right)x\left(t\right),$$

$$V_2(x_t,t,r_t)={\int }_{t-{\eta }_M}^t{x}^T\left(s\right)Qx\left(s\right)ds,$$

$$V_3(x_t,t,r_t)={\int }_{t-{\eta }_M}^t{\int }_s^t{\dot{x}}^T\left(v\right)R\dot{x}\left(v\right)dvds,$$

with $P\left(r_t\right)>0,Q>0,S>0,R>0,\xi \left(t\right)=[x^T,x^T(t-\eta \left(t\right)),x^T(t-{\eta }_M),e_k^T\left(t\right),{\omega }^T\left(t\right),$${\displaystyle \frac{1}{\eta \left(t\right)}}{\int }_{t-{\eta }_M}^t{x}^T\left(s\right)ds,{\displaystyle \frac{1}{{\eta }_M-\eta \left(t\right)}}{\int }_{t-{\eta }_M}^{t-\eta \left(t\right)}{x}^T\left(s\right)ds]$. Taking the derivative of $V(x_t,t,r_t)$ along the trajectory of the system (9) for $i,i\in S$ is found as the weak infinitesimal generator operator:

$$\begin{array}{cc}\hfill \mathcal{L}V\left(t\right)& =2{\dot{x}}^T\left(t\right)P_{i}x\left(t\right)+x^T\left(t\right)\sum _{j=1}^r{\pi }_{ij}{P}_{j}x\left(t\right)+x^T\left(t\right)Qx\left(t\right)+x^T(t-{\eta }_M)Qx(t-{\eta }_M)\hfill \\ \hfill & +{\eta }_M{\dot{x}}^T\left(t\right)R\dot{x}\left(t\right)-{\int }_{t-{\eta }_M}^t{\dot{x}}^T\left(t\right)R\dot{x}\left(t\right)ds.\hfill \end{array}$$

(12)

By introducing the event-triggered threshold condition into Formula (12), we get

$$\begin{array}{cc}\hfill \mathcal{L}V\left(t\right)& =2{\dot{x}}^T\left(t\right)P_{i}x\left(t\right)+x^T\left(t\right)\sum _{j=1}^r{\pi }_{ij}{P}_{j}x\left(t\right)+x^T\left(t\right)Qx\left(t\right)+x^T(t-{\eta }_M)Qx(t-{\eta }_M)\hfill \\ \hfill & +{\eta }_M{\dot{x}}^T\left(t\right)R\dot{x}\left(t\right)-{\int }_{t-{\eta }_M}^t{\dot{x}}^T\left(t\right)R\dot{x}\left(t\right)ds-e_k^T\left(t\right)C_{1i}^T{\Omega }_i{C}_{1i}{e}_k^T\left(t\right)\hfill \\ \hfill & +e_k^T\left(t\right)C_{1i}^T{\Omega }_i{C}_{1i}{e}_k^T\left(t\right).\hfill \end{array}$$

(13)

The term ${\eta }_M{\dot{x}}^T\left(t\right)R\dot{x}\left(t\right)$ in Formula (13) can be processed as follows:

$$\begin{array}{cc}\hfill & {\eta }_M{\dot{x}}^T\left(t\right)R\dot{x}\left(t\right)\hfill \\ \hfill & =\sqrt{{\eta }_M}{\{A_{i}x\left(t\right)+B_i{K}_i(I+{\Delta }_f)C_{1i}[x(t-\eta \left(t\right))+e_k\left(t\right)]+B_{wi}\omega \left(t\right)\}}^{T}R\sqrt{{\eta }_M}\{A_{i}x\left(t\right)\hfill \\ \hfill & +B_i{K}_i(I+{\Delta }_f)C_{1i}[x(t-\eta \left(t\right))+e_k\left(t\right)]+B_{wi}\omega \left(t\right)\}\hfill \\ & ={\xi }^T\left(t\right){\left\{\sqrt{{\eta }_M}[A_i,B_i{K}_i(I+{\Delta }_f)C_{1i},0,B_i{K}_i(I+{\Delta }_f)C_{1i},B_{wi},0,0]\right\}}^T\hfill \\ \hfill & \times R\left\{\sqrt{{\eta }_M}[A_i,B_i{K}_i(I+{\Delta }_f)C_{1i},0,B_i{K}_i(I+{\Delta }_f)C_{1i},B_{wi},0,0]\right\}\xi \left(t\right).\hfill \end{array}$$

(14)

where we define ${\psi }_{31i}=\sqrt{{\eta }_M}[A_i,B_i{K}_i(I+{\Delta }_f)C_{1i},0,B_i{K}_i(I+{\Delta }_f)C_{1i},B_{wi},0,0]$. In order to facilitate the use of the LMI later, we introduce $P_i$ in ${\psi }_{31i}$, and there is ${\Xi }_{31i}=\sqrt{{\eta }_M}[P_i{A}_i,P_i{B}_i{K}_i(I+{\Delta }_f)C_{1i},0,P_i{B}_i{K}_i(I+{\Delta }_f)C_{1i},P_i{B}_{wi},0,0]$.

Considering the influence of network delay on system stability, Lemma 1 is applied to estimate the upper bound of the single integral term ${\int }_{t-{\eta }_M}^t{\dot{x}}^T\left(s\right)Rx\left(s\right)ds$, and the processing procedure is as follows:

$$\begin{array}{cc}\hfill & -{\int }_{t-{\eta }_M}^t{\dot{x}}^T\left(s\right)Rx\left(s\right)ds\hfill \\ \hfill & =-{\int }_{t-\eta \left(t\right)}^t{\dot{x}}^T\left(s\right)Rx\left(s\right)ds-{\int }_{t-{\eta }_M}^{t-\eta \left(t\right)}{\dot{x}}^T\left(s\right)Rx\left(s\right)ds\hfill \\ \hfill & \le -{\xi }^T\left(t\right)\left({\displaystyle \frac{1}{\eta \left(t\right)}}{\left[\begin{array}{c}{G}_1\\ G_2\end{array}\right]}^T\overline{R}\left[\begin{array}{c}{G}_1\\ G_2\end{array}\right]+{\displaystyle \frac{1}{{\eta }_M-\eta \left(t\right)}}{\left[\begin{array}{c}{G}_3\\ G_4\end{array}\right]}^T\overline{R}\left[\begin{array}{c}{G}_3\\ G_4\end{array}\right]\right)\xi \left(t\right),\hfill \end{array}$$

(15)

where

$$\overline{R}=\left[\begin{array}{cc}R& 0\\ \ast & 3R\end{array}\right],$$

$$G_1=[I,-I,0,0,0,0,0],G_2=[0,I,I,0,0,2I,0],$$

$$G_3=[0,I,-I,0,0,0,0],G_4=[0,I,I,0,0,0,-2I].$$

In dealing with inequality (15), Lemma 2 is applied, and the process is as follows:

$$\begin{array}{cc}\hfill -& {\int }_{t-{\eta }_M}^t{\dot{x}}^T\left(s\right)Rx\left(s\right)ds\hfill \\ \hfill =& -{\int }_{t-\eta \left(t\right)}^t{\dot{x}}^T\left(s\right)Rx\left(s\right)ds-{\int }_{t-{\eta }_M}^{t-\eta \left(t\right)}{\dot{x}}^T\left(s\right)Rx\left(s\right)ds\hfill \\ \hfill \le & -{\xi }^T\left(t\right)\left({\displaystyle \frac{1}{\eta \left(t\right)}}{\left[\begin{array}{c}{G}_1\\ G_2\end{array}\right]}^T\overline{R}\left[\begin{array}{c}{G}_1\\ G_2\end{array}\right]+{\displaystyle \frac{1}{{\eta }_M-\eta \left(t\right)}}{\left[\begin{array}{c}{G}_3\\ G_4\end{array}\right]}^T\overline{R}\left[\begin{array}{c}{G}_3\\ G_4\end{array}\right]\right)\xi \left(t\right)\hfill \\ \hfill \le & -{\xi }^T\left(t\right)\left({\displaystyle \frac{1}{{\eta }_M}}{\left[\begin{array}{c}{G}_{12}\\ G_{34}\end{array}\right]}^T\tilde{R}\left[\begin{array}{c}{G}_{12}\\ G_{34}\end{array}\right]\right)\xi \left(t\right),\hfill \end{array}$$

(16)

where

$$G_{12}=\left[\begin{array}{c}{G}_1\\ G_2\end{array}\right],G_{34}=\left[\begin{array}{c}{G}_3\\ G_4\end{array}\right],\tilde{R}=\left[\begin{array}{cccc}R& 0& 0& 0\\ \ast & 3R& 0& 0\\ \ast & \ast & R& 0\\ \ast & \ast & \ast & 3R\end{array}\right].$$

Substituting the event-triggered threshold condition (3) and Equations (9), (14), and inequality (16) into Equation (13), the following inequality can be obtained:

$$\begin{array}{cc}\hfill \mathcal{L}V\left(t\right)=& 2{\dot{x}}^T\left(t\right)P_{i}x\left(t\right)+x^T\left(t\right)\sum _{j=1}^r{\pi }_{ij}{P}_{j}x\left(t\right)+x^T\left(t\right)Qx\left(t\right)+x^T(t-{\eta }_M)Qx(t-{\eta }_M)\hfill \\ \hfill -& {\int }_{t-{\eta }_M}^t{\dot{x}}^T\left(t\right)R\dot{x}\left(t\right)ds{\sigma }_i{x}^T(t-\eta \left(t\right))C_{1i}^T{\Omega }_i{C}_{1i}x(t-\eta \left(t\right))-e_k^T\left(t\right)C_{1i}^T{\Omega }_i{C}_{1i}{e}_k^T\left(t\right)\hfill \\ \hfill +& {\xi }^T\left(t\right){\left\{\sqrt{{\eta }_M}[A_i,B_i{K}_i(I+{\Delta }_f)C_{1i},0,B_i{K}_i(I+{\Delta }_f)C_{1i},B_{wi},0,0]\right\}}^T\hfill \\ \hfill \times & R\left\{\sqrt{{\eta }_M}[A_i,B_i{K}_i(I+{\Delta }_f)C_{1i},0,B_i{K}_i(I+{\Delta }_f)C_{1i},B_{wi},0,0]\right\}\xi \left(t\right)\hfill \\ \hfill \le & -{\xi }^T\left(t\right)\left({\displaystyle \frac{1}{\eta \left(t\right)}}{\left[\begin{array}{c}{G}_1\\ G_2\end{array}\right]}^T\overline{R}\left[\begin{array}{c}{G}_1\\ G_2\end{array}\right]+{\displaystyle \frac{1}{{\eta }_M-\eta \left(t\right)}}{\left[\begin{array}{c}{G}_3\\ G_4\end{array}\right]}^T\overline{R}\left[\begin{array}{c}{G}_3\\ G_4\end{array}\right]\right)\xi \left(t\right)\hfill \\ \hfill \le & -{\xi }^T\left(t\right)\left({\displaystyle \frac{1}{{\eta }_M}}{\left[\begin{array}{c}{G}_{12}\\ G_{34}\end{array}\right]}^T\tilde{R}\left[\begin{array}{c}{G}_{12}\\ G_{34}\end{array}\right]\right)\xi \left(t\right).\hfill \end{array}$$

(17)

Here, we define

$$J=\epsilon \left\{{\int }_0^{\infty }[z^T\left(t\right)z\left(t\right)-{\gamma }^2{\omega }^T\left(t\right)\omega \left(t\right)]ds.\right\}$$

(18)

Since the system (9) is asymptotically stable, under the condition of zero initial state, according to Definition (2), we can obtain

$$\epsilon \left\{{\int }_0^{\infty }\mathcal{L}V\left(t\right)ds\right\}=\underset{t\to 0^{+}}{lim}V\left(t\right)-V\left(0\right)=0.$$

(19)

Further processing can be obtained, as follows:

$$\begin{array}{cc}\hfill J& \le \epsilon \left\{{\int }_0^{\infty }[z^T\left(t\right)z\left(t\right)-{\gamma }^2{\omega }^T\left(t\right)\omega \left(t\right)]ds\right\}\hfill \\ \hfill & \le \epsilon \left\{{\int }_0^{\infty }{\xi }^T\left(t\right)[{\Xi }_{11i}+{\Xi }_{21i}^T{\Xi }_{21i}+{\Xi }_{31i}^T{P}_i{R}^{-1}{P}_i{\Xi }_{31i}]ds.\right\}\hfill \end{array}$$

(20)

Applying the Schur complement and Definition (2) may draw such conclusion (10), which guarantees $\mathcal{L}V\left(t\right)\le {\gamma }^2{\omega }^T\left(t\right)\omega \left(t\right)-z^T\left(t\right)z\left(t\right)$ in (20). The closed-loop system (9) is asymptotically stable with $H\infty$ norm-bound $\gamma$ if (10) holds. This completes the proof. □

3.2. Controller Design for Event-Triggered Markov-Type Networked Control Systems

In this section, the problem of output feedback $H\infty$ controller design for event-triggered Markov-type networked control system is studied. Based on the stability analysis of the event-triggered Markov-type networked control system, the controller design problem of the system is transformed into the stability problem of the closed-loop system. We studied the design and implementation of an output feedback $H\infty$ controller for event-triggered Markov-type network control systems. An output feedback $H\infty$ controller for event-triggered Markov-type network control systems was proposed.

The problem of how to design and implement the output feedback $H\infty$ controller of event-triggered Markov-type networked control systems is studied. The output feedback $H\infty$ controller of event-triggered Markov-type networked control systems is proposed.

Theorem 2. 

Given the constant $\gamma ,{\sigma }_i,{\eta }_M,{\epsilon }_1,{\epsilon }_2$ and a sufficiently small positive scalar $s_i$, if there are positive definite matrices $X_i,\overline{Q},R,\overline{{\Omega }_i},i,\in S$, the following LMIs hold. Under the event-triggered threshold condition (3), the closed-loop system (9) is asymptotically stable and meets the $H\infty$ performance index γ. The output feedback gain matrix is $K_i=Z_i{Y}_i^{-1}$.

$$\left[\begin{array}{cccc}{\overline{\Xi }}_i& \ast & \ast & \ast \\ {\overline{\Sigma }}_{21i}& -{\epsilon }_{2}I& \ast & \ast \\ {\overline{\Sigma }}_{31i}& 0& -{\epsilon }_{2}I& \ast \\ {\overline{\Sigma }}_{41i}& 0& 0& {\Lambda }_i\end{array}\right]<0,$$

(21)

$$s_i\to 0^{+},\left[\begin{array}{cc}{s}_{i}I& \ast \\ Y_i{C}_{1i}-C_{1i}{X}_i& I\end{array}\right]<0,$$

(22)

where

$${\overline{\Xi }}_i=\left[\begin{array}{ccc}{\overline{\Xi }}_{11i}& \ast & \ast \\ {\overline{\Xi }}_{21i}& -I& \ast \\ {\overline{\Xi }}_{31i}& 0& -2{\epsilon }_1{X}_i+{\epsilon }_1^2\overline{R}\end{array}\right],$$

$${\overline{\Xi }}_{11i}=\left[\begin{array}{ccccccc}{\overline{\Phi }}_{11i}& \ast & \ast & \ast & \ast & \ast & \ast \\ {\overline{\Phi }}_{21i}& {\sigma }_i{\overline{\Omega }}_i-{\displaystyle \frac{8}{{\eta }_M}}\overline{R}& \ast & \ast & \ast & \ast & \ast \\ 0& -{\displaystyle \frac{2}{{\eta }_M}}\overline{R}& -{\displaystyle \frac{4}{{\eta }_M}}\overline{R}& \ast & \ast & \ast \\ C_{1i}^T{Z}_i^T{B}_i^T& 0& 0& C_{1i}^T{Z}_i^T{B}_i^T& \ast & \ast & \ast \\ B_{wi}^T& 0& 0& 0& -{\gamma }^{2}I& \ast & \ast \\ {\displaystyle \frac{6}{{\eta }_M}}\overline{R}& {\displaystyle \frac{6}{{\eta }_M}}\overline{R}& 0& 0& 0& -{\displaystyle \frac{12}{{\eta }_M}}\overline{R}& \ast \\ 0& {\displaystyle \frac{6}{{\eta }_M}}\overline{R}& {\displaystyle \frac{6}{{\eta }_M}}\overline{R}& 0& 0& 0& -{\displaystyle \frac{12}{{\eta }_M}}\overline{R}\end{array}\right],$$

$${\overline{\Phi }}_{11i}=X_i{A}_i^T+A_i{X}_i+\overline{Q}+{\pi }_{ij}{X}_i-{\displaystyle \frac{4}{{\eta }_M}}\overline{R},$$

$${\overline{\Phi }}_{21i}=C_{1i}^T{Z}_i^T{B}_i^T-{\displaystyle \frac{2}{{\eta }_M}}\overline{R},$$

$${\overline{\Xi }}_{21i}=[C_{2i}{X}_i,D_i{Z}_i{C}_{1i},0,D_i{Z}_i{C}_{1i},0,0,0],$$

$${\overline{\Xi }}_{31i}=\sqrt{{\eta }_M}[A_i{X}_i, B_i{Z}_i{C}_{1i},0,B_i{Z}_i{C}_{1i},B_{wi},0,0],$$

$${\overline{\Sigma }}_{21i}=[{\epsilon }_2{B}_i^T,0,0,0,0,0,0,{\epsilon }_2{D}_i^T,\epsilon \sqrt{{\eta }_M}{B}_i^T],$$

$${\overline{\Sigma }}_{31i}=[0,{\sigma }_f{Z}_i{C}_{1i},0,{\sigma }_f{Z}_i{C}_{1i},0,0,0,0,0],$$

$${\overline{\Sigma }}_{41i}=[{\Theta }_i,0,0,0,0,0,0,0,0],$$

$${\Theta }_i^T=[\sqrt{{\pi }_{i1}}{X}_i,\cdots ,\sqrt{{\pi }_{ii-1}}{X}_i,\sqrt{{\pi }_{ii+1}}{X}_i,\cdots ,\sqrt{{\pi }_{ir}}{X}_i],$$

$${\widehat{\Lambda }}_i=diag\{-X_i,\cdots ,-X_{i-1},-X_{i+1},\cdots ,-X_r\}.$$

Proof. 

According to

$$(R-{\epsilon }_1^{-1}{P}_i)R^{-1}(R-{\epsilon }_1^{-1}{P}_i)\ge 0,$$

We can get:

$$-2{\epsilon }_1{P}_i+{\epsilon }_1^{2}R\ge -P_i{R}^{-1}{P}_i.$$

The $-P_i{R}^{-1}{P}_i$ in inequality (10) is replaced by $-2{\epsilon }_1{P}_i+{\epsilon }_1^{2}R$, and the following inequality can be obtained:

$$\begin{array}{c}\hfill {\Xi }_i=\left[\begin{array}{ccc}{\Xi }_{11i}& \ast & \ast \\ {\Xi }_{21i}& -I& \ast \\ {\Xi }_{31i}& 0& -P_i{R}^{-1}{P}_i\end{array}\right]<\left[\begin{array}{ccc}{\Xi }_{11i}& \ast & \ast \\ {\Xi }_{21i}& -I& \ast \\ {\Xi }_{31i}& 0& -2{\epsilon }_1{P}_i+{\epsilon }_1^{2}R\end{array}\right].\end{array}$$

(23)

It can be seen that Inequality (11) can be equivalently expressed in the following form:

$${\widehat{\Xi }}_i+sym\left\{H_{Bi}^T{H}_{fi}\right\}+H_I^T\sum _{j=1,j\ne i}^r{\pi }_{ij}{P}_j{H}_I<0$$

(24)

where

$${\widehat{\Xi }}_i=\left[\begin{array}{ccc}{\widehat{\Xi }}_{11i}& \ast & \ast \\ {\widehat{\Xi }}_{21i}& -I& \ast \\ {\widehat{\Xi }}_{31i}& 0& -2{\epsilon }_1{P}_i+{\epsilon }_1^{2}R\end{array}\right],$$

$${\widehat{\Xi }}_{11i}=\left[\begin{array}{ccccccc}{\widehat{\Phi }}_{11i}& \ast & \ast & \ast & \ast & \ast & \ast \\ {\widehat{\Phi }}_{21i}& {\widehat{\Phi }}_{21i}& \ast & \ast & \ast & \ast & \ast \\ 0& -{\displaystyle \frac{2}{{\eta }_M}}R& -{\displaystyle \frac{4}{{\eta }_M}}R& \ast & \ast & \ast & \ast \\ C_{1i}^T{K}_i^T{B}_i^T{P}_i& \ast & \ast & -C_{1i}^T{\Omega }_i{C}_{1i}& \ast & \ast & \ast \\ B_{wi}^T& 0& 0& 0& -{\gamma }^{2}I& \ast & \ast \\ {\displaystyle \frac{6}{{\eta }_M}}R& {\displaystyle \frac{6}{{\eta }_M}}R& 0& 0& 0& -{\displaystyle \frac{12}{{\eta }_M}}R& \ast \\ 0& {\displaystyle \frac{6}{{\eta }_M}}R& {\displaystyle \frac{6}{{\eta }_M}}R& 0& 0& 0& -{\displaystyle \frac{12}{{\eta }_M}}R\end{array}\right],$$

$${\widehat{\Phi }}_{11i}=A_i^T{P}_i+P_i{A}_i+Q+{\pi }_{ii}{P}_i-{\displaystyle \frac{4}{{\eta }_M}}R,$$

$${\widehat{\Phi }}_{21i}=C_{1i}^T{K}_i^T{B}_i^T{P}_i-{\displaystyle \frac{2}{{\eta }_M}}R,$$

$${\widehat{\Phi }}_{22i}={\sigma }_i{C}_{1i}^T{\Omega }_i{C}_{1i}-{\displaystyle \frac{8}{{\eta }_M}}R,$$

$${\widehat{\Xi }}_{21i}=[C_{2i},D_i{K}_i{C}_{1i},0,D_i{K}_i{C}_{1i},0,0,0],$$

$${\widehat{\Xi }}_{31i}=\sqrt{{\eta }_M}[P_i{A}_i,P_i{B}_i{K}_i{C}_{1i},0,P_i{B}_i{K}_i{C}_{1i},P_i{B}_{wi},0,0],$$

$$H_{Bi}=[B_i^T{P}_i,0,0,0,0,0,0,D_i^T,\sqrt{{\eta }_M}{B}_i^T{P}_i],$$

$$H_{fi}=[0,K_i{\Delta }_f{C}_{1i},0,K_i{\Delta }_f{C}_{1i},0,0,0,0,0],$$

$$H_I=[I,0,0,0,0,0,0,0,0].$$

For $\forall {\epsilon }_2>0$, Lemma (3) is used to deal with Inequality (24), and the following form can be obtained:

$${\widehat{\Xi }}_i+{\epsilon }_2{H}_{Bi}^T{H}_{Bi}+{\epsilon }_2^{-1}{\delta }_f{\tilde{H}}_{fi}{\tilde{H}}_{fi}+H_I^T\sum _{j=1,j\ne i}^r{\pi }_{ij}{P}_j{H}_I<0,$$

(25)

where ${\tilde{H}}_{fi}=[0,{\delta }_f{K}_i{C}_{1i},0,{\delta }_f{K}_i{C}_{1i},0,0,0,0,0]$.

Using the Schur complement to deal with inequality (25), we can get

$$\left[\begin{array}{cccc}{\widehat{\Xi }}_i& \ast & \ast & \ast \\ H_{Bi}& -{\epsilon }_2^{-1}I& \ast & \ast \\ {\tilde{H}}_{fi}& 0& -{\epsilon }_{2}I& \ast \\ H_I& 0& 0& {\widehat{\Lambda }}_i\end{array}\right]<0,$$

(26)

where ${\Lambda }_i=diag\{-P_1^{-1},\cdots ,-P_{i-1}^{-1},-P_{i+1}^{-1},\cdots ,-P_r^{-1}\}$.

By defining matrix variables in the following form $X_i=P_i^{-1}$, $\overline{R}=X_{i}R{X}_i$, $\overline{Q}=X_{i}Q{X}_i$, $\overline{{\Omega }_i}=X_i{C}_{1i}^T{\Omega }_i{C}_{1i}{X}_i$, ${\Psi }_i=diag\{X_i,X_i,X_i,X_i,I,X_i,X_i,I,X_i,I,I,\underset{\underset{r-1}{︸}}{I\cdots I}\}$ and multiplying left and right matrix inequalities (26) by ${\Psi }_i$ and ${\Psi }_i^T$, respectively, we can get

$$\left[\begin{array}{cccc}{\overline{\Xi }}_i& \ast & \ast & \ast \\ {\overline{\Sigma }}_{21i}& -{\epsilon }_2^{-1}I& \ast & \ast \\ {\overline{\Sigma }}_{31i}& 0& -{\epsilon }_{2}I& \ast \\ {\overline{\Sigma }}_{41i}& 0& 0& {\widehat{\Lambda }}_i\end{array}\right]<0,$$

(27)

where

$${\overline{\Xi }}_i=\left[\begin{array}{ccc}{\tilde{\Xi }}_{11i}& \ast & \ast \\ {\tilde{\Xi }}_{21i}& -I& \ast \\ {\tilde{\Xi }}_{31i}& 0& -2{\epsilon }_1{P}_i+{\epsilon }_1^{2}R\end{array}\right],$$

$${\tilde{\Xi }}_{11i}=\left[\begin{array}{ccccccc}{\tilde{\Phi }}_{11i}& \ast & \ast & \ast & \ast & \ast & \ast \\ {\tilde{\Phi }}_{21i}& {\tilde{\Phi }}_{22i}& \ast & \ast & \ast & \ast & \ast \\ 0& -{\displaystyle \frac{2}{{\eta }_M}}& -{\displaystyle \frac{4}{{\eta }_M}}\overline{R}& \ast & \ast & \ast & \ast \\ C_{1i}^T{K}_i^T{B}_i^T{P}_i& 0& 0& -C_{1i}^T{\Omega }_i{C}_{1i}& \ast & \ast & \ast \\ B_{wi}^T& 0& 0& 0& -{\gamma }^{2}I& \ast & \ast \\ {\displaystyle \frac{6}{{\eta }_M}}\overline{R}& {\displaystyle \frac{6}{{\eta }_M}}\overline{R}& 0& 0& 0& -{\displaystyle \frac{12}{{\eta }_M}}\overline{R}& \ast \\ 0& {\displaystyle \frac{6}{{\eta }_M}}\overline{R}& {\displaystyle \frac{6}{{\eta }_M}}\overline{R}& 0& 0& 0& -{\displaystyle \frac{12}{{\eta }_M}}\overline{R}\end{array}\right],$$

$${\tilde{\Phi }}_{11i}=X_i{A}_i^T+A_i{X}_i+\overline{Q}+{\pi }_{ij}{X}_i-{\displaystyle \frac{4}{{\eta }_M}}\overline{R},$$

$${\tilde{\Phi }}_{21i}=X_i{C}_{1i}^T{Z}_i^T{B}_i^T-{\displaystyle \frac{2}{{\eta }_M}}\overline{R},$$

$${\tilde{\Phi }}_{22i}={\sigma }_i{C}_{1i}^T{\Omega }_i{C}_{1i}-{\displaystyle \frac{8}{{\eta }_M}}\overline{R},$$

$${\tilde{\Phi }}_{41i}=X_i{C}_{1i}^T{Z}_i^T{B}_i^T,$$

$${\tilde{\Xi }}_{21i}=[C_{2i}{X}_i,D_i{K}_i{C}_{1i}{X}_i,0,D_i{K}_i{C}_{1i}{X}_i,0,0,0],$$

$${\tilde{\Xi }}_{31i}=\sqrt{{\eta }_M}[A_i{X}_i,B_i{K}_i{C}_{1i}{X}_i,0,B_i{K}_i{C}_{1i}{X}_i,B_{wi},0,0],$$

$${\overline{\Sigma }}_{21i}=[{\epsilon }_2{B}_i^T,0,0,0,0,0,0,{\epsilon }_2{D}_i^T,\epsilon \sqrt{{\eta }_M}{B}_i^T],$$

$${\overline{\Sigma }}_{31i}=[0,{\sigma }_f{K}_i{C}_{1i}{X}_i,0,{\sigma }_f{K}_i{C}_{1i}{X}_i,0,0,0,0,0],$$

$${\overline{\Sigma }}_{41i}=[{\Theta }_i,0,0,0,0,0,0,0,0],$$

$${\Theta }_i^T=[\sqrt{{\pi }_{i1}}{X}_i,\cdots ,\sqrt{{\pi }_{ii-1}}{X}_i,\sqrt{{\pi }_{ii+1}}{X}_i,\cdots ,\sqrt{{\pi }_{ir}}{X}_i].$$

We can see that there are coupling terms $B_i{K}_i{C}_{1i}{X}_i$, $D_i{K}_i{C}_{1i}{X}_i$, and $K_i{C}_{1i}{X}_i$ in Inequality (27), which cannot be solved using the LIM toolbox. Inspired by the studies [33,34], we define $K_i=Z_i{Y}_i^{-1}$ and $Y_i{C}_{1i}=C_{1i}{X}_i$ to decouple through a linear transformation. It can be seen that Formula (27) is equivalent to Formula (21).

For Equation $Y_i{C}_{1i}=C_{1i}{X}_i$, we introduce the value $s_i$ approaching zero from the right, and we obtain a suboptimal solution that satisfies the constraints of the equation by solving $s_i\to 0^{+},\left[\begin{array}{cc}{s}_{i}I& \ast \\ Y_i{C}_{1i}-C_{1i}{X}_i& I\end{array}\right]<0$, which is Formula (20).

Therefore, if the matrix Inequalities (21) and (22) hold, then the system (9) is asymptotically stable under the $H\infty$ performance index $\gamma$. □

Remark 7. 

In the process of deriving the main results, the difficulty encountered is how to deal with the non-linear terms $B_i{K}_i{C}_{1i}{X}_i$, $D_i{K}_i{C}_{1i}{X}_i$, and $K_i{C}_{1i}{X}_i$ in Equation (27). Inspired by [32,33], we find a method to solve this difficulty, and then we obtain the static output feedback controller design method of Markov-type networked control systems.

Remark 8. 

It is worth noting that the event-triggered parameter ${\sigma }_i$ changes with the modal changes of Markov-type network control systems. According to Theorem 2, the $H\infty$ control performance and telecommunication network resource utilization are related to the event-triggered parameters ${\sigma }_i$ and ${\Omega }_i$. It can be known from Theorem 2 that $H\infty$ control performance and communication network resource usage are concerned with the event-triggered parameters ${\sigma }_i$ and ${\Omega }_i$.

Remark 9. 

Although some studies can provide feasible results for dealing with static output feedback problems [33,34], they are difficult-to-handle situations, where quantization and event-triggered schemes exist in Markov-type network control systems. Theorem 2 presents a new method for designing event-triggered output feedback $H\infty$ controllers for Markov-type networked control systems.

Remark 10. 

In this paper, the transition probability matrix assumption of a Markovian jump system model is completely known, which is too ideal and not universal in theoretical analysis. When building the Markovian jump system model for the actual system, some information is imperfect due to the fact that the conditional probability information of individual subsystems cannot be detected or the detection cost is too high, which is the main reason for the existence of unknown elements in the Markovian jump system transition probability matrix, making the research results of this paper have certain limitations in practical applications.

4. Numerical Example and Case Study

In this section, we give three examples to illustrate the efficiency and advantage of the obtained results in this paper.

Example 1. 

Considering the following 2-mode Markov-type networked control system (9), the system matrix parameters are as follows:

$$A_1=\left[\begin{array}{cc}-0.2& 0.8\\ 0& -1\end{array}\right], B_1=\left[\begin{array}{c}-0.1\\ -0.1\end{array}\right], B_{w1}=\left[\begin{array}{c}1\\ -0.2\end{array}\right],$$

$$C_{11}=\left[\begin{array}{cc}1& 0.5\end{array}\right], C_{21}=\left[\begin{array}{cc}0.2& 0.15\\ 0.1& 0.3\end{array}\right], D_1=\left[\begin{array}{c}0.5\\ 0.2\end{array}\right],$$

$$A_2=\left[\begin{array}{cc}-1& -0.6\\ 0.8& -1.5\end{array}\right], B_2=\left[\begin{array}{c}-0.5\\ 0\end{array}\right], B_{w2}=\left[\begin{array}{c}0.3\\ 0.1\end{array}\right],$$

$$C_{12}=\left[\begin{array}{cc}1& -4\end{array}\right], C_{22}=\left[\begin{array}{cc}0.2& 0.1\\ 0.2& -0.3\end{array}\right], D_2=\left[\begin{array}{c}0.2\\ 0.1\end{array}\right].$$

It is assumed that the response Markov process transition rate matrix is

$$\Pi =\left[\begin{array}{cc}-0.5& 0.5\\ 0.3& -0.3\end{array}\right].$$

The initial state and external disturbance are given as $x={[-11]}^T$, and

$$\omega \left(t\right)=\left\{\begin{array}{cc}1,& 5\le t\le 10\hfill \\ -1,& 10<t\le 15\hfill \\ 0,& \mathrm{else}.\hfill \end{array}\right.$$

Case 1. 

Other parameter settings include ${\sigma }_1=0.1$, ${\sigma }_2=0.1$, $\gamma =2$, ${\epsilon }_1=1$, ${\epsilon }_2=1$, $s_1=0.001$, $s_2=0.001$, and ${\delta }_{fs}=0.5$. The comparison results of the upper bound of the maximum allowable time delay and the number of decision variables obtained by Theorem 2 in the literature [35] and Theorem 2 in this paper.

The comparison results are shown in

Table 1. The maximum allowable network delay and number of decision variables.

Method	${\mathit{\eta }}_{\mathit{M}}$	Number of Decision Variables
Theorem 2 ([35])	0.8302 s	$11.5n^2+5.5n$
Theorem 2	1.0200 s	$7.5n^2+4.5n$

, and the corresponding controller gain matrices are $K_1=-0.0981$ and $K_2=0.0014$. The simulation result for the responses of $x\left(t\right)$ is shown in Figure 3. Through a comparison in Figure 3, it can be found that by introducing an event-triggered scheme and signal quantization separately in the network control systems, compared with the four cases of simultaneously introducing an event-triggered scheme and signal quantization and not introducing an event-triggered scheme and signal quantization, this can indicate that the method adopted in this paper is reasonable and effective.

It can be seen from Table 1 that Theorem 2 in this paper gives a better result than Theorem 2 in [35]. On the one hand, because the combination of Wirtinger’s-based integral inequality and the reciprocally convex inequality is used to estimate the upper bound of the weak infinitesimal generator operator of the Lyapunov–Krasovskii function, while retaining some important information of delay as much as possible, the delay is avoided to be amplified and the network transmission delay is reduced, so the upper bound of the maximum allowable transmission network delay is obtained as 1.0200 s. On the other hand, compared with the free-weight matrix method to estimate the upper bound of the weak infinitesimal generating operator of the Lyapunov–Krasovskii function by using the combination of Wirtinger’s-based integral inequality and reciprocally convex inequality. Wirtinger’s-based integral inequality and reciprocally convex inequality can reduce the number of matrix variables; thus, this reduce the computational complexity. The computational complexity of the matrix variable obtained by this method is $7.5n^2+4.5n$.

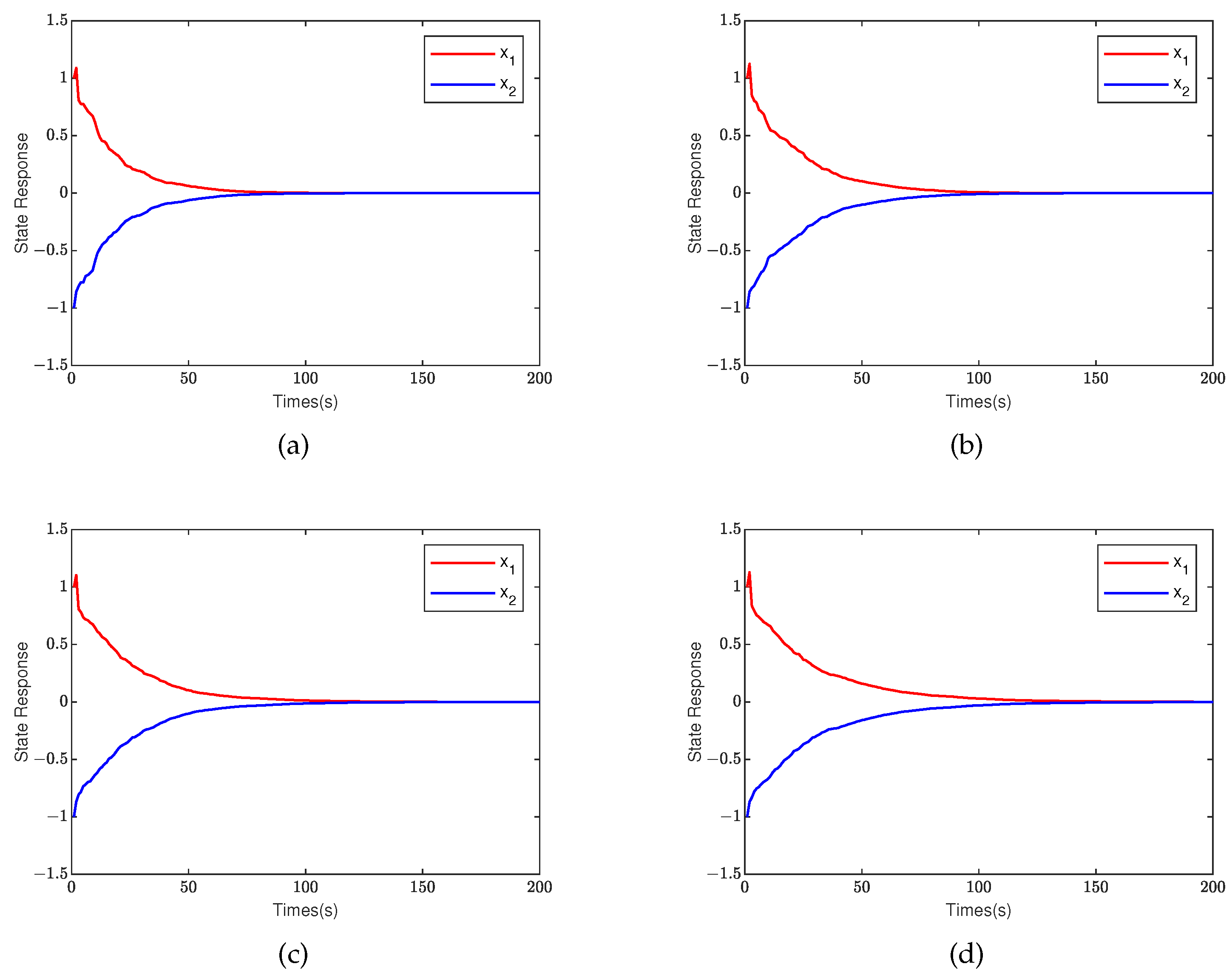
Case 2. 

Other parameter settings include $\gamma =2$, ${\epsilon }_1=1$, ${\epsilon }_2=1$, $s_1=0.001$, $s_2=0.001$, and ${\delta }_{fs}=0.5$. When ${\sigma }_1$ and ${\sigma }_2$ to different values, we observe the impact of different values on network delay.

From the results of

Table 2. The influence of ${\sigma }_1$ and ${\sigma }_2$ changes on network delay.

${\mathit{\sigma }}_1,{\mathit{\sigma }}_2$	${\mathit{\eta }}_{\mathit{M}}$	Controller Gain Matrices ${\mathit{K}}_{\mathit{i}}$
${\sigma }_1={\sigma }_2=0.0$	1.0240 s	$K_1=-0.1067$, $K_2=5.52\times {10}^{-4}$
${\sigma }_1={\sigma }_2=0.1$	1.0200 s	$K_1=-0.0981$, $K_2=1.40\times {10}^{-3}$
${\sigma }_1={\sigma }_2=0.2$	1.0180 s	$K_1=-0.0911$, $K_2=9.74\times {10}^{-4}$
${\sigma }_1={\sigma }_2=0.3$	1.0160 s	$K_1=-0.0855$, $K_2=8.60\times {10}^{-4}$
${\sigma }_1={\sigma }_2=0.4$	1.0140 s	$K_1=-0.0815$, $K_2=8.20\times {10}^{-4}$
${\sigma }_1={\sigma }_2=0.5$	1.0120 s	$K_1=-0.0780$, $K_2=7.05\times {10}^{-4}$
${\sigma }_1={\sigma }_2=0.6$	1.0110 s	$K_1=-0.0730$, $K_2=4.95\times {10}^{-4}$
${\sigma }_1={\sigma }_2=0.7$	1.0100 s	$K_1=-0.0670$, $K_2=3.87\times {10}^{-4}$

, we can see that by introducing the event-triggered scheme in networked control systems, when ${\sigma }_1={\sigma }_2=0$, the event-triggered weakening process is time-triggered, which is the largest delay in the network; when the value of ${\sigma }_1={\sigma }_2$ increases, the delay in networked control systems gradually decreases. The simulation result for the responses of $x\left(t\right)$ is shown in Figure 4. It can be seen from Figure 4a–d that the networked control system becomes larger with the value of the event-triggered parameters ${\sigma }_1$ and ${\sigma }_2$. Although the system is stable, there are some differences in the system state trajectories, indicating that the introduction of the event-triggered mechanism can reduce the delay problem in the networked control system.

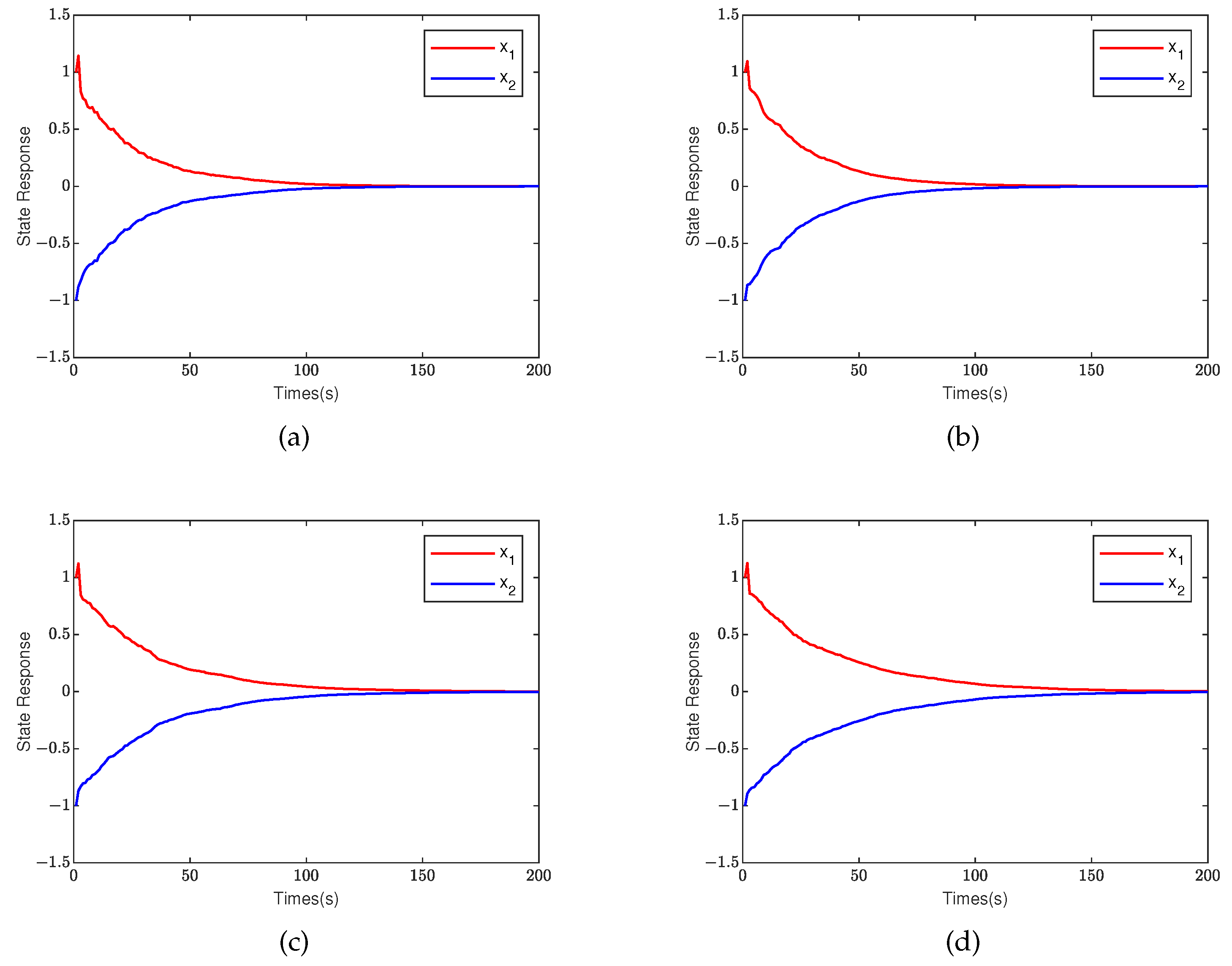
Case 3. 

Other parameter settings include ${\sigma }_1=0.1$, ${\sigma }_2=0.1$, $\gamma =2$, ${\epsilon }_1=1$, ${\epsilon }_2=1$, $s_1=0.001$, and $s_2=0.001$. When ${\delta }_{fs}$ to different values, we observe the impact of different values on network delay.

From the results of

Table 3. The influence of ${\delta }_{fs}$ changes on network delay.

${\mathit{\delta }}_{\mathit{f}\mathit{s}}$	${\mathit{\eta }}_{\mathit{M}}$	Controller Gain Matrices ${\mathit{K}}_{\mathit{i}}$
${\delta }_{fs}=0.1$	1.0340 s	$K_1=-0.1888$, $K_2=0.0270$
${\delta }_{fs}=0.2$	1.0310 s	$K_1=-0.1675$, $K_2=0.0019$
${\delta }_{fs}=0.3$	1.0270 s	$K_1=-0.1447$, $K_2=0.0021$
${\delta }_{fs}=0.4$	1.0230 s	$K_1=-0.1202$, $K_2=0.0018$
${\delta }_{fs}=0.5$	1.0200 s	$K_1=-0.0981$, $K_2=0.0014$
${\delta }_{fs}=0.6$	1.0190 s	$K_1=-0.0795$, $K_2=8.20\times {10}^{-4}$
${\delta }_{fs}=0.7$	1.0160 s	$K_1=-0.0657$, $K_2=7.08\times {10}^{-4}$
${\delta }_{fs}=0.8$	1.0140 s	$K_1=-0.0548$, $K_2=7.75\times {10}^{-4}$
${\delta }_{fs}=0.9$	1.0130 s	$K_1=-0.0458$, $K_2=5.17\times {10}^{-4}$

, we can see that when ${\delta }_{fs}=0$, the delay in the network is the largest by introducing the quantization scheme in the networked control system. When the value of ${\delta }_{fs}$ increases, the delay in the network gradually decreases. The simulation result for the responses of $x\left(t\right)$ is shown in Figure 5. From Figure 5a–d’s system state response curves, it can be seen that under the action of event-triggered mechanism, the state response time changes with the signal quantization parameter ${\delta }_{fs}$, and the response time of the system to the steady state also changes, indicating that the signal quantization can effectively reduce the delay problem in the network.

From the state response curves of Figure 3, Figure 4 and Figure 5, it can be seen that although there are some differences in the state trajectories, the system is stable and meets the expected performance index. From Table 1, Table 2 and Table 3, it can be seen that the introduction of an event-triggered scheme and quantization scheme can improve the utilization of network bandwidth resources and reduce network delay.

Example 2. 

Considering the following 4-mode Markov-type networked control systems (9), the system matrix parameters are as follows:

$$A_1=\left[\begin{array}{cc}-0.4& 0.8\\ 0& -1.2\end{array}\right], B_1=\left[\begin{array}{c}-0.1\\ -0.1\end{array}\right], B_{w1}=\left[\begin{array}{c}1\\ -0.2\end{array}\right],$$

$$C_{11}=\left[\begin{array}{cc}1& 0.5\end{array}\right], C_{21}=\left[\begin{array}{cc}0.2& 0.15\\ 0.1& 0.3\end{array}\right], D_1=\left[\begin{array}{c}0.5\\ 0.2\end{array}\right],$$

$$A_2=\left[\begin{array}{cc}-1.2& -0.6\\ 0.6& -1.5\end{array}\right], B_2=\left[\begin{array}{c}-0.5\\ 0\end{array}\right], B_{w2}=\left[\begin{array}{c}0.3\\ 0.1\end{array}\right],$$

$$C_{12}=\left[\begin{array}{cc}1& -4\end{array}\right], C_{22}=\left[\begin{array}{cc}0.2& 0.1\\ -0.2& 0.4\end{array}\right], D_2=\left[\begin{array}{c}0.2\\ 0.1\end{array}\right],$$

$$A_3=\left[\begin{array}{cc}-0.2& 0.8\\ 0& -1\end{array}\right], B_3=\left[\begin{array}{c}0.5\\ 1\end{array}\right], B_{w3}=\left[\begin{array}{c}0.1\\ 0.2\end{array}\right],$$

$$C_{13}=\left[\begin{array}{cc}1& 1\end{array}\right], C_{23}=\left[\begin{array}{cc}0.18& 0.2\\ 0.1& 0.24\end{array}\right], D_3=\left[\begin{array}{c}0.6\\ 0.1\end{array}\right],$$

$$A_4=\left[\begin{array}{cc}-1& -0.6\\ 0.8& -1.7\end{array}\right], B_4=\left[\begin{array}{c}0.5\\ 0.2\end{array}\right], B_{w4}=\left[\begin{array}{c}0.1\\ 0.1\end{array}\right],$$

$$C_{14}=\left[\begin{array}{cc}1& 0.6\end{array}\right], C_{24}=\left[\begin{array}{cc}0.2& 0.2\\ -0.4& -0.3\end{array}\right], D_4=\left[\begin{array}{c}0.4\\ 0.8\end{array}\right].$$

It is assumed that the response Markov process transition rate matrix is

$$\Pi =\left[\begin{array}{cccc}-0.5& 0.25& 0.15& 0.1\\ 0.1& -0.3& 0.1& 0.1\\ 0.1& 0.1& -0.5& 0.3\\ 0.1& 0.1& 0.1& -0.3\end{array}\right].$$

Considering the influence of time-delay on the stability of the Markov-type networked control systems (9) and applying Theorem 2, the upper bound of the maximum allowable time delay is 1.0947s, and the corresponding controller gain matrices are $K_1=-0.1186$, $K_2=0.0164$, $K_3=0.0781$, and $K_4=0.0094$. It can be seen from Figure 6 that the system can quickly reach the steady state.

Example 3. 

Considering the structure of the distributed photovoltaic power generation network control system shown in Figure 7, taking photovoltaic array 1 and photovoltaic array 5 as examples, analyze the distributed photovoltaic power generation network control system. This system considers the different operating conditions and control situations of photovoltaic power generation, power consumption, and energy storage between different photovoltaic arrays. The distributed photovoltaic power generation network control system transmits information through a communication network, and the controller sends control switch K1, K3 information through the network to determine the operation mode of the photovoltaic power generation system. $Z_2$, $Z_3$, $Z_4$, $Z_5$, $Z_6$, and $Z_7$ represent the impedance of the line, and $Z_1$ is the impedance of the load.

Based on the Markov switching strategy, establish a 4-mode Markov-type network control system for network communication between photovoltaic array 1 and photovoltaic array 5: $I_1$ represents the total current of photovoltaic generation in photovoltaic array 1; $I_2$ represents the total current of photovoltaic generation in photovoltaic array 5; $I_N$ represents the total demand current for power supply; and $I_G$ represents the current of the public power grid, where $I_2>I_1>I_G$. Among them, mode 1: when $I_N=I_1+I_2+I_G$, photovoltaic array 1 and photovoltaic array 5 simultaneously generate photovoltaic power; mode 2: when $I_N=I_1+I_G$, photovoltaic array 1 generates photovoltaic power, while photovoltaic array 5 generates photovoltaic power for energy storage; mode 2: when $I_N=I_2+I_G$, photovoltaic array 5 generates photovoltaic power, while photovoltaic array 1 generates photovoltaic power for energy storage; mode 2: when $I_N=I_G$, both photovoltaic array 1 and photovoltaic array 5 are storing energy for photovoltaic power generation.

The state vector $x\left(t\right)$ of the system is $x\left(t\right)={[x_1\left(t\right),x_2\left(t\right)]}^T$, which is the generation current, and $x_2\left(t\right)$ is the current flowing through the load in the circuit. $y\left(t\right)$ and $z\left(t\right)$, respectively, represent the measurable output power and control output power in the circuit. The matrix parameters of the distributed photovoltaic power generation network control system are as follows:

$$A_1=\left[\begin{array}{cc}-0.2& 0.8\\ 0& -1\end{array}\right], B_1=\left[\begin{array}{c}-0.1\\ -0.1\end{array}\right], B_{w1}=\left[\begin{array}{c}1\\ -0.2\end{array}\right],$$

$$C_{11}=\left[\begin{array}{cc}1& 0.5\end{array}\right], C_{21}=\left[\begin{array}{cc}0.2& 0.15\\ 0.1& 0.3\end{array}\right], D_1=\left[\begin{array}{c}0.5\\ 0.2\end{array}\right],$$

$$A_2=\left[\begin{array}{cc}-1& -0.6\\ 0.8& -1.5\end{array}\right], B_2=\left[\begin{array}{c}-0.5\\ 0\end{array}\right], B_{w2}=\left[\begin{array}{c}0.3\\ 0.1\end{array}\right],$$

$$C_{12}=\left[\begin{array}{cc}1& -4\end{array}\right], C_{22}=\left[\begin{array}{cc}0.2& 0.1\\ -0.2& 0.4\end{array}\right], D_2=\left[\begin{array}{c}0.2\\ 0.1\end{array}\right],$$

$$A_3=\left[\begin{array}{cc}-0.2& 0.8\\ 0& -1\end{array}\right], B_3=\left[\begin{array}{c}0.5\\ 1\end{array}\right], B_{w3}=\left[\begin{array}{c}0.1\\ 0.2\end{array}\right],$$

$$C_{13}=\left[\begin{array}{cc}1& 1\end{array}\right], C_{23}=\left[\begin{array}{cc}0.18& 0.2\\ 0.1& 0.24\end{array}\right], D_3=\left[\begin{array}{c}0.6\\ 0.1\end{array}\right],$$

$$A_4=\left[\begin{array}{cc}-1& -0.6\\ 0.8& -1.5\end{array}\right], B_4=\left[\begin{array}{c}0.5\\ 0.2\end{array}\right], B_{w4}=\left[\begin{array}{c}0.1\\ 0.1\end{array}\right],$$

$$C_{14}=\left[\begin{array}{cc}1& 0.6\end{array}\right], C_{24}=\left[\begin{array}{cc}0.2& 0.2\\ -0.4& -0.3\end{array}\right], D_4=\left[\begin{array}{c}0.4\\ 0.8\end{array}\right].$$

It is assumed that the response Markov process transition rate matrix is

$$\Pi =\left[\begin{array}{cccc}-0.5& 0.25& 0.15& 0.1\\ 0.1& -0.3& 0.1& 0.1\\ 0.1& 0.1& -0.5& 0.3\\ 0.1& 0.1& 0.1& -0.3\end{array}\right].$$

Considering the impact of network delay on the stability of distributed photovoltaic power generation network control systems, co-design is used to implement the design and implementation form of the controller. In the controller design process, the problem of variable coupling can be addressed through the idea of variable transformations, which can avoid matrix inversion and achieve variable decoupling. Provide a design method for the controller through analysis. For the delay problem in the distributed photovoltaic power generation network control system, using Theorem 2, the maximum allowable delay upper bound for ensuring the stable and smooth operation of the entire system is 1.1190 s, and the corresponding control gain matrix is $K_1=-0.0187$, $K_2=0.0040$, $K_3=0.0037$, and $K_4=0.0122$. The state response curve of the distributed photovoltaic power generation network control system is shown in Figure 8. It can be seen from Figure 8 that by applying Theorem 2, the distributed photovoltaic power generation network control system can quickly respond to reach a steady state.

5. Conclusions

Taking into account the characteristics of signal quantization and event-triggered schemes, this paper studies the output feedback $H\infty$ control problem of an event-triggered Markov-type network control system and proposes a stability criterion with low conservatism. Based on the stability analysis, a satisfying $H\infty$ disturbance attenuation level $\gamma$ output feedback controller design method is given. Numerical examples have verified the effectiveness of the proposed method. This article assumes that the transition rate matrix in the Markov jump system is known. In practical engineering applications, it is difficult to fully obtain the transition rate matrix, or the acquisition cost is very high. In future research, we will consider the case where the Markov transition rate matrix is partially unknown.