Superconvergence of Modified Nonconforming Cut Finite Element Method for Elliptic Problems
Abstract
1. Introduction
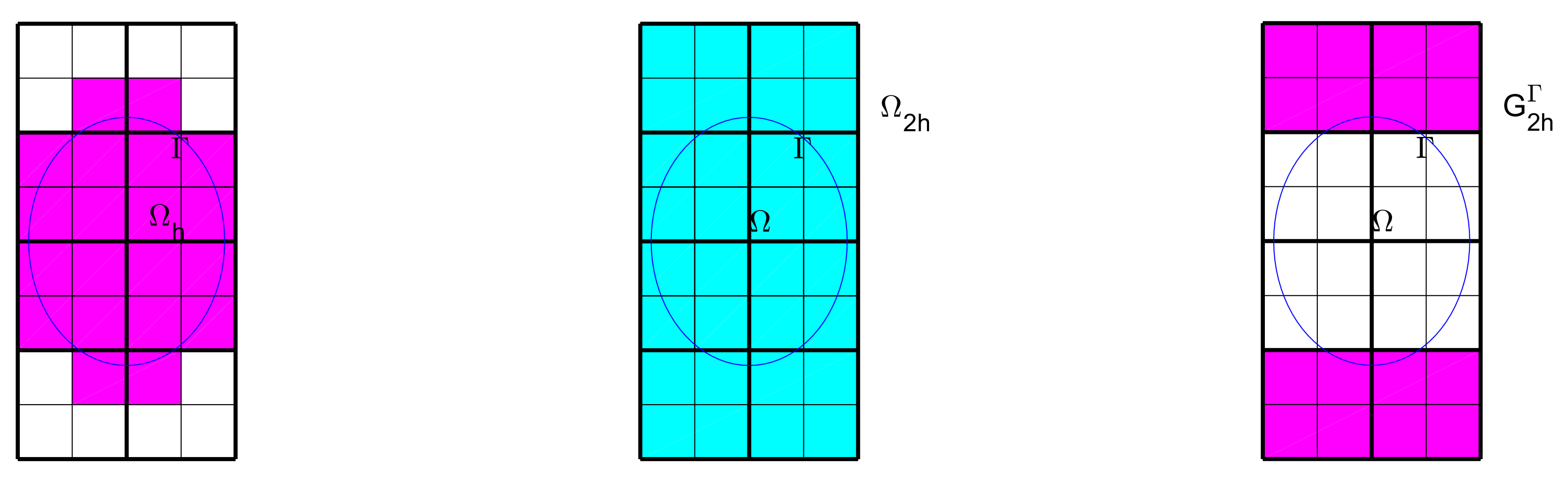
2. The Model Problem and the Finite Element Formulation
3. Supercloseness Analysis
4. Postprocessing and Superconvergence
5. Numerical Examples
5.1. Example 1
5.2. Example 2
5.3. Example 3
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hansbo, A.; Hansbo, P. An unfitted finite element method, based on Nitsche’s method, for elliptic interface problems. Comput. Methods Appl. Mech. Eng. 2002, 191, 5537–5552. [Google Scholar] [CrossRef]
- Burman, E.; Hansbo, P. Fictitious domain finite element methods using cut elements: I. A stabilized Lagrange multiplier method. Comput. Methods Appl. Mech. Eng. 2010, 199, 2680–2686. [Google Scholar] [CrossRef]
- Hansbo, P.; Larson, M.G.; Zahedi, S. A cut finite element method for a Stokes interface problem. Appl. Numer. Math. 2014, 85, 90–114. [Google Scholar] [CrossRef]
- Huang, P.; Wu, H.; Xiao, Y. An unfitted interface penalty finite element method for elliptic interface problems. Comput. Methods Appl. Mech. Eng. 2017, 323, 439–460. [Google Scholar] [CrossRef]
- Wang, N.; Chen, J. A nonconforming Nitsche’s extended finite element method for Stokes interface problems. J. Sci. Comput. 2019, 81, 342–374. [Google Scholar] [CrossRef]
- Massing, A.; Schott, B.; Wall, W.A. A stabilized Nitsche cut finite element method for the Oseen problem. Comput. Methods Appl. Mech. Eng. 2018, 328, 262–300. [Google Scholar] [CrossRef]
- Burman, E.; Claus, S.; Hansbo, P.; Larson, M.G.; Massing, A. CutFEM: Discretizing geometry and partial differential equations. Int. J. Numer. Methods Eng. 2015, 104, 472–501. [Google Scholar] [CrossRef]
- Lin, Q.; Yan, N. The Construction and Analysis of High Effciency Finite Element Methods; Hebei University Publishers: Baoding, China, 1996. (In Chinese) [Google Scholar]
- Zienkiewicz, O.C.; Zhu, J.Z. The superconvergent patch recovery and a posteriori error estimates. I. the recovery technique. Int. J. Numer. Methods Eng. 1992, 33, 1331–1364. [Google Scholar] [CrossRef]
- Naga, A.; Zhang, Z. A posteriori error estimates based on the polynomial preserving recovery. SIAM J. Numer. Anal. 2004, 42, 1780–1800. [Google Scholar] [CrossRef]
- Bank, R.E.; Xu, J.; Zheng, B. Superconvergent derivative recovery for lagrange triangular elements of degree p on unstructured grids. SIAM J. Numer. Anal. 2007, 45, 2032–2046. [Google Scholar] [CrossRef]
- Lin, Q.; Tobiska, L.; Zhou, A. Superconvergence and extrapolation of non-conforming low order finite elements applied to the poisson equation. IMA J. Numer. Anal. 2005, 25, 160–181. [Google Scholar] [CrossRef]
- Mao, S.; Chen, S.; Shi, D. Convergence and superconvergence of a nonconforming finite element on anisotropic mehses. Int. J. Numer. Anal. Model. 2007, 4, 16–38. [Google Scholar]
- Hu, J.; Ma, R. Superconvergence of both the Crouzeix-Raviart and morley elements. Numer. Math. 2016, 132, 491–509. [Google Scholar] [CrossRef]
- Li, Y. Superconvergent flux recovery of the Rannacher-Turek nonconforming element. J. Sci. Comput. 2021, 87, 32. [Google Scholar] [CrossRef]
- Ming, P.; Shi, Z. Superconvergence studies of quadrilateral nonconforming rotated Q1 elements. Int. J. Numer. Anal. Model. 2006, 3, 322–332. [Google Scholar]
- Stein, E. Singular Integrals and Differentiability Properties of Functions; Princeton University Press: Princeton, NJ, USA, 1970; Volume 30. [Google Scholar]
- Gilbarg, D.; Trudinger, N.S. Elliptic Partial Differential Equations of Second Order, 2nd ed.; Springer: Berlin, Germany, 1983; Volume 224. [Google Scholar]
- He, X.; Song, F.; Deng, W. A well-conditioned, nonconforming Nitsche’s extended finite element method for elliptic interface problems. Numer. Math. Theory Methods Appl. 2020, 13, 99–130. [Google Scholar]
- Arnold, D.; Boffi, D.; Falk, R. Approximation by quadrilateral elements. Math. Comput. 2002, 71, 909–922. [Google Scholar] [CrossRef]
- He, X.; Chen, Y.; Ji, H.; Wang, H. Superconvergence of unfitted rannacher-turek nonconforming element for elliptic interface problems. Appl. Numer. Math. 2024, 203, 32–51. [Google Scholar] [CrossRef]
| Rate | Rate | Rate | |||||
|---|---|---|---|---|---|---|---|
| 9.3335 | 8.6752 | 8.7346 | |||||
| 4.1432 | 1.1716 | 3.4709 | 1.3215 | 3.6997 | 1.2393 | ||
| 1.8062 | 1.1977 | 1.2783 | 1.4410 | 1.4339 | 1.3674 | ||
| 8.1059 | 1.1559 | 4.5268 | 1.4977 | 5.1910 | 1.4658 | ||
| 1.4864 | 1.4406 | 1.5410 | |||||
| 7.0590 | 1.0742 | 6.3422 | 1.1836 | 7.0242 | 1.1335 | ||
| 3.0848 | 1.1942 | 2.4337 | 1.3818 | 2.6597 | 1.4010 | ||
| 1.3424 | 1.2003 | 8.5553 | 1.5082 | 9.9456 | 1.4191 | ||
| 1.1737 | 1.1222 | 1.0300 | |||||
| 5.1093 | 1.1998 | 4.4336 | 1.3398 | 4.4650 | 1.2060 | ||
| 2.2139 | 1.2065 | 1.6559 | 1.4208 | 1.7724 | 1.3329 | ||
| 9.8307 | 1.1712 | 5.9211 | 1.4836 | 6.5601 | 1.4339 |
| Rate | Rate | Rate | |||||
|---|---|---|---|---|---|---|---|
| 9.0498 | 8.0253 | 8.7898 | |||||
| 3.8709 | 1.2252 | 2.9733 | 1.4324 | 3.3166 | 1.4061 | ||
| 1.7297 | 1.1621 | 1.0919 | 1.4452 | 1.2686 | 1.3864 | ||
| 7.8501 | 1.1397 | 3.7896 | 1.5267 | 4.3814 | 1.5337 | ||
| 1.4929 | 1.3847 | 1.4334 | |||||
| 6.3781 | 1.2269 | 5.2454 | 1.4004 | 5.1589 | 1.4743 | ||
| 2.7873 | 1.1942 | 1.9036 | 1.4622 | 2.0169 | 1.3548 | ||
| 1.2656 | 1.1390 | 6.7447 | 1.4969 | 7.4012 | 1.4463 | ||
| 1.5692 | 1.4741 | 1.7173 | |||||
| 7.0478 | 1.1547 | 6.0883 | 1.2757 | 7.0293 | 1.2887 | ||
| 2.9559 | 1.2535 | 2.1598 | 1.4951 | 2.6111 | 1.4287 | ||
| 1.3058 | 1.1786 | 7.5276 | 1.5206 | 9.2699 | 1.4940 |
| Rate | Rate | Rate | |||||
|---|---|---|---|---|---|---|---|
| 3.4465 | 3.1674 | 3.1945 | |||||
| 1.3095 | 1.3961 | 1.1489 | 1.4629 | 1.1297 | 1.4996 | ||
| 5.3084 | 1.3026 | 4.0167 | 1.5162 | 3.8787 | 1.5423 | ||
| 2.3179 | 1.1954 | 1.4131 | 1.5070 | 1.4416 | 1.4279 | ||
| 2.1853 | 1.9994 | 1.9957 | |||||
| 9.1487 | 1.2561 | 7.4441 | 1.4254 | 7.2634 | 1.4582 | ||
| 3.9748 | 1.2026 | 2.8217 | 1.3995 | 2.9227 | 1.3133 | ||
| 1.7577 | 1.1771 | 9.6124 | 1.5536 | 1.0655 | 1.4557 | ||
| 2.5133 | 2.2064 | 2.7792 | |||||
| 9.8588 | 1.3500 | 8.0975 | 1.4461 | 8.5858 | 1.6946 | ||
| 4.2002 | 1.2309 | 2.9083 | 1.4772 | 3.2786 | 1.3888 | ||
| 1.9020 | 1.1429 | 1.0599 | 1.4561 | 1.1400 | 1.5239 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, X.; Song, F. Superconvergence of Modified Nonconforming Cut Finite Element Method for Elliptic Problems. Mathematics 2024, 12, 2595. https://doi.org/10.3390/math12162595
He X, Song F. Superconvergence of Modified Nonconforming Cut Finite Element Method for Elliptic Problems. Mathematics. 2024; 12(16):2595. https://doi.org/10.3390/math12162595
Chicago/Turabian StyleHe, Xiaoxiao, and Fei Song. 2024. "Superconvergence of Modified Nonconforming Cut Finite Element Method for Elliptic Problems" Mathematics 12, no. 16: 2595. https://doi.org/10.3390/math12162595
APA StyleHe, X., & Song, F. (2024). Superconvergence of Modified Nonconforming Cut Finite Element Method for Elliptic Problems. Mathematics, 12(16), 2595. https://doi.org/10.3390/math12162595





