A COMSOL-Based Numerical Simulation of Heat Transfer in a Hybrid Nanofluid Flow at the Stagnant Point across a Stretching/Shrinking Sheet: Implementation for Understanding and Improving Solar Systems
Abstract
1. Introduction
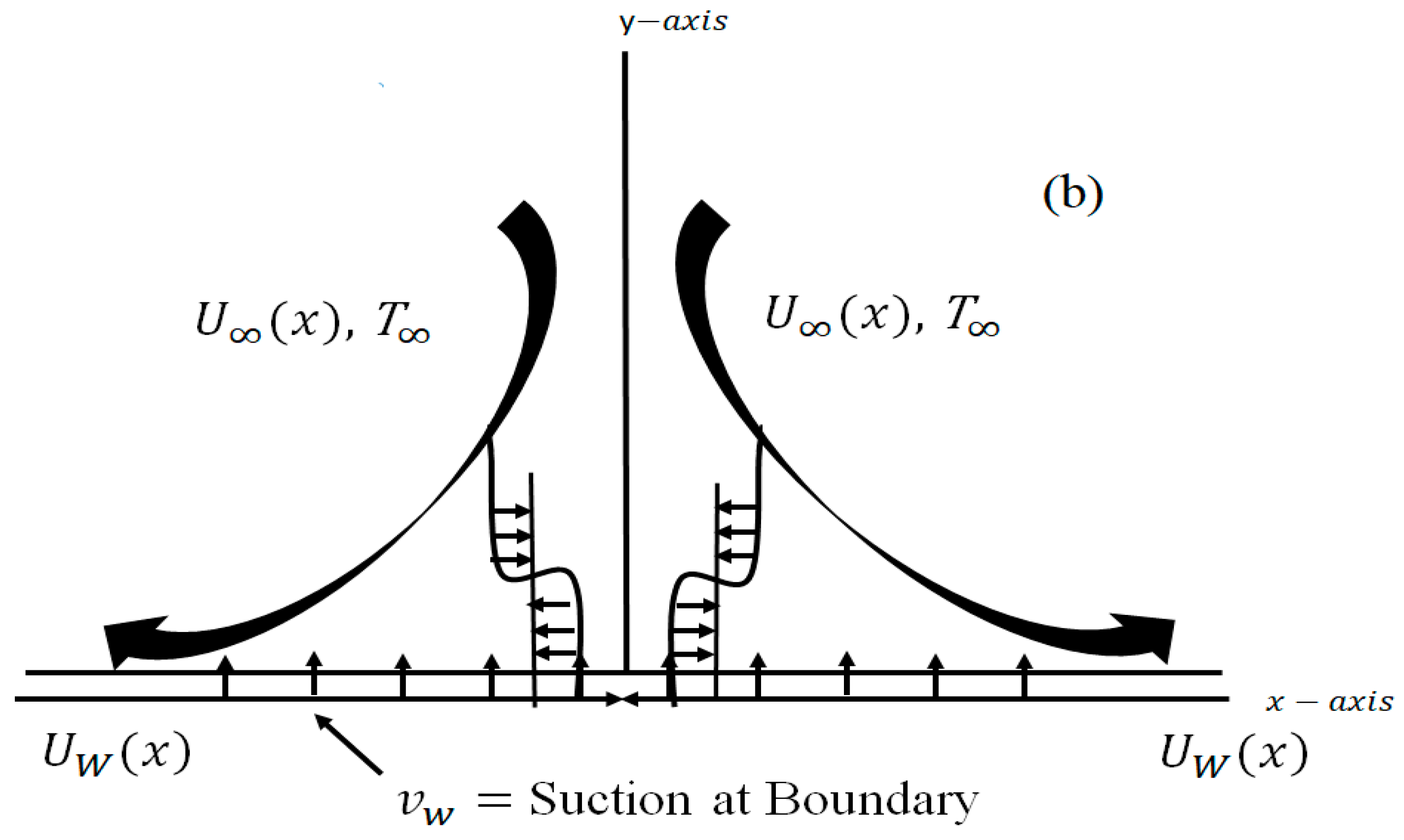
2. Problem Formulation
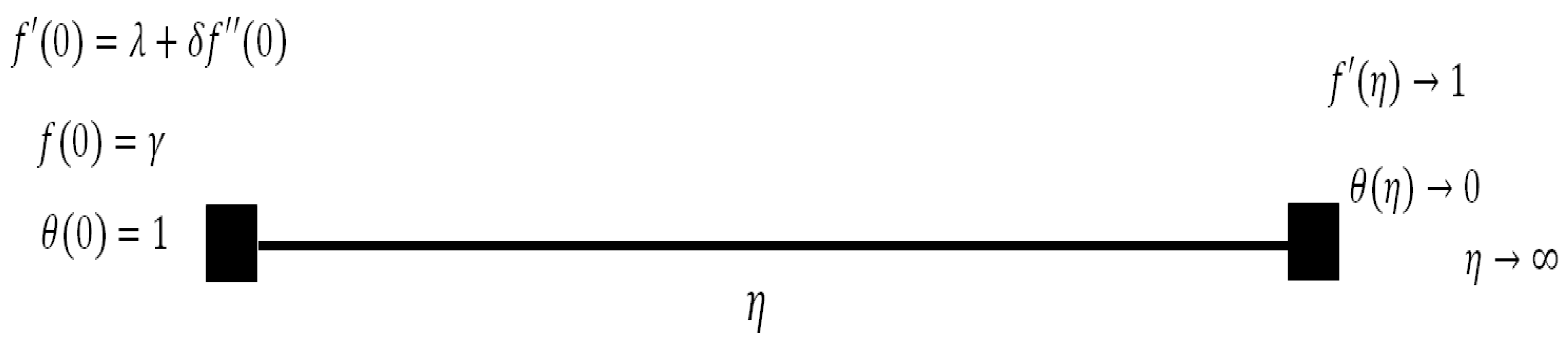
2.1. Governing PDEs and Boundary Conditions
2.2. Similarity Approach
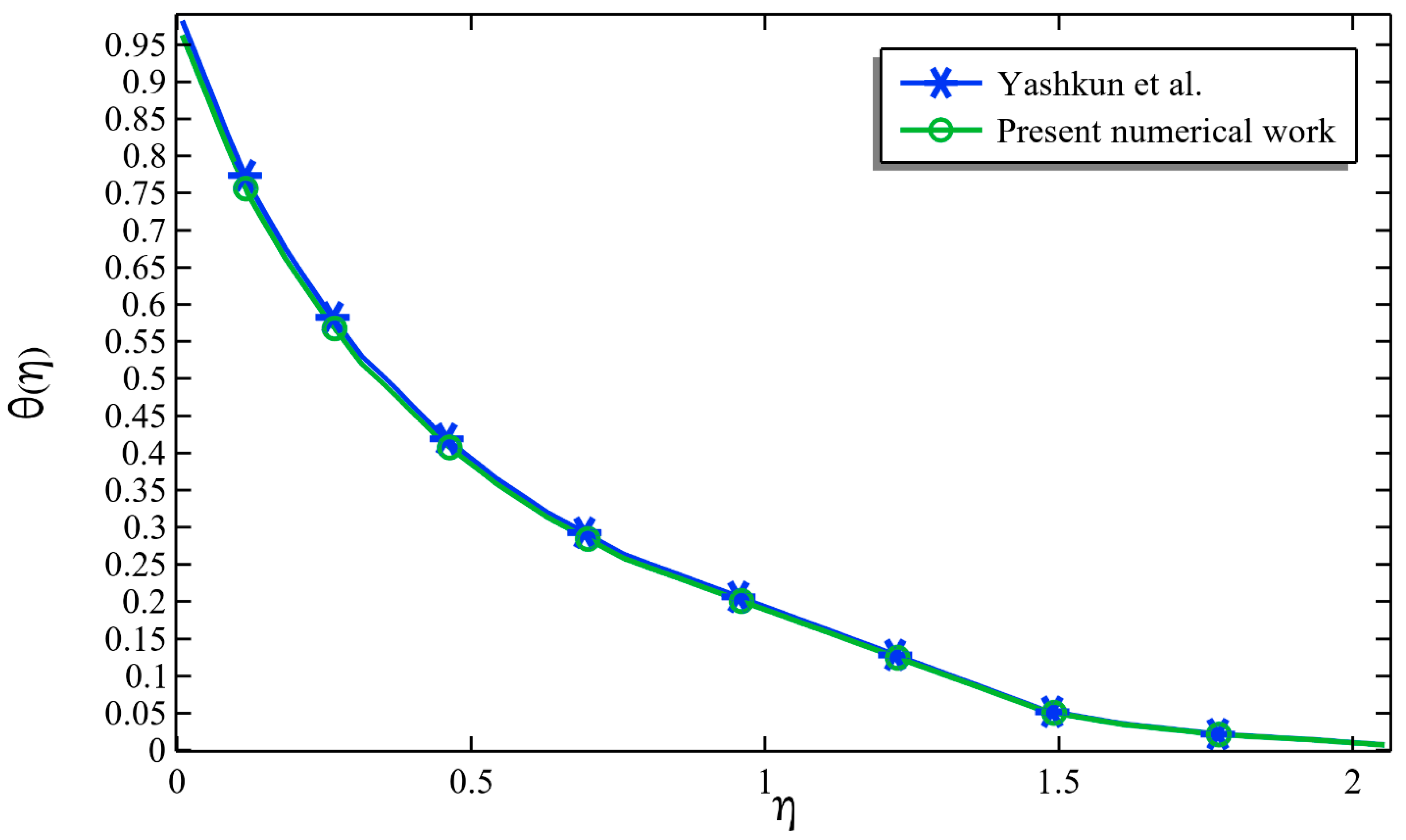
3. Mesh Independence Study and Verification
4. Discussion of Results
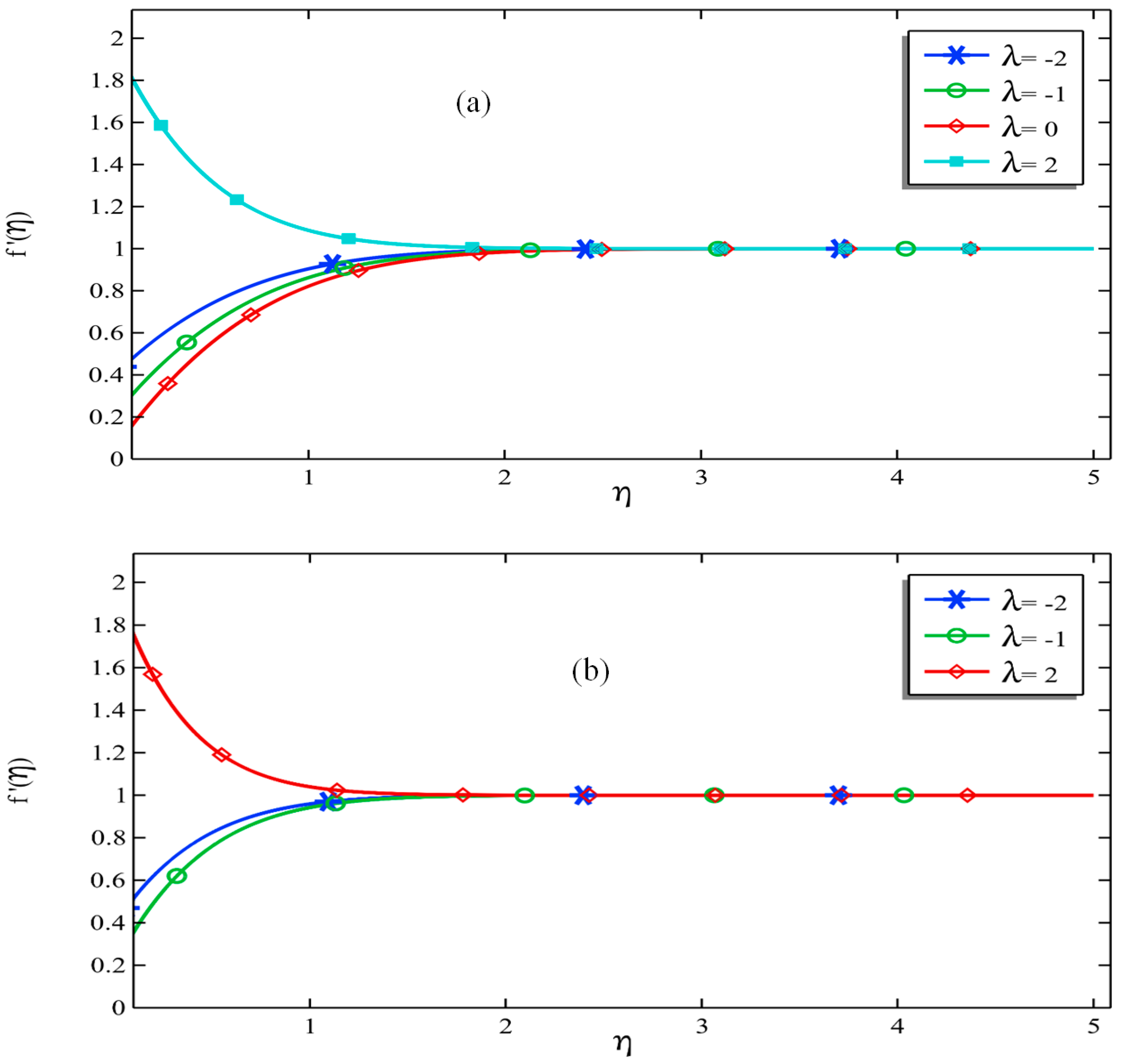
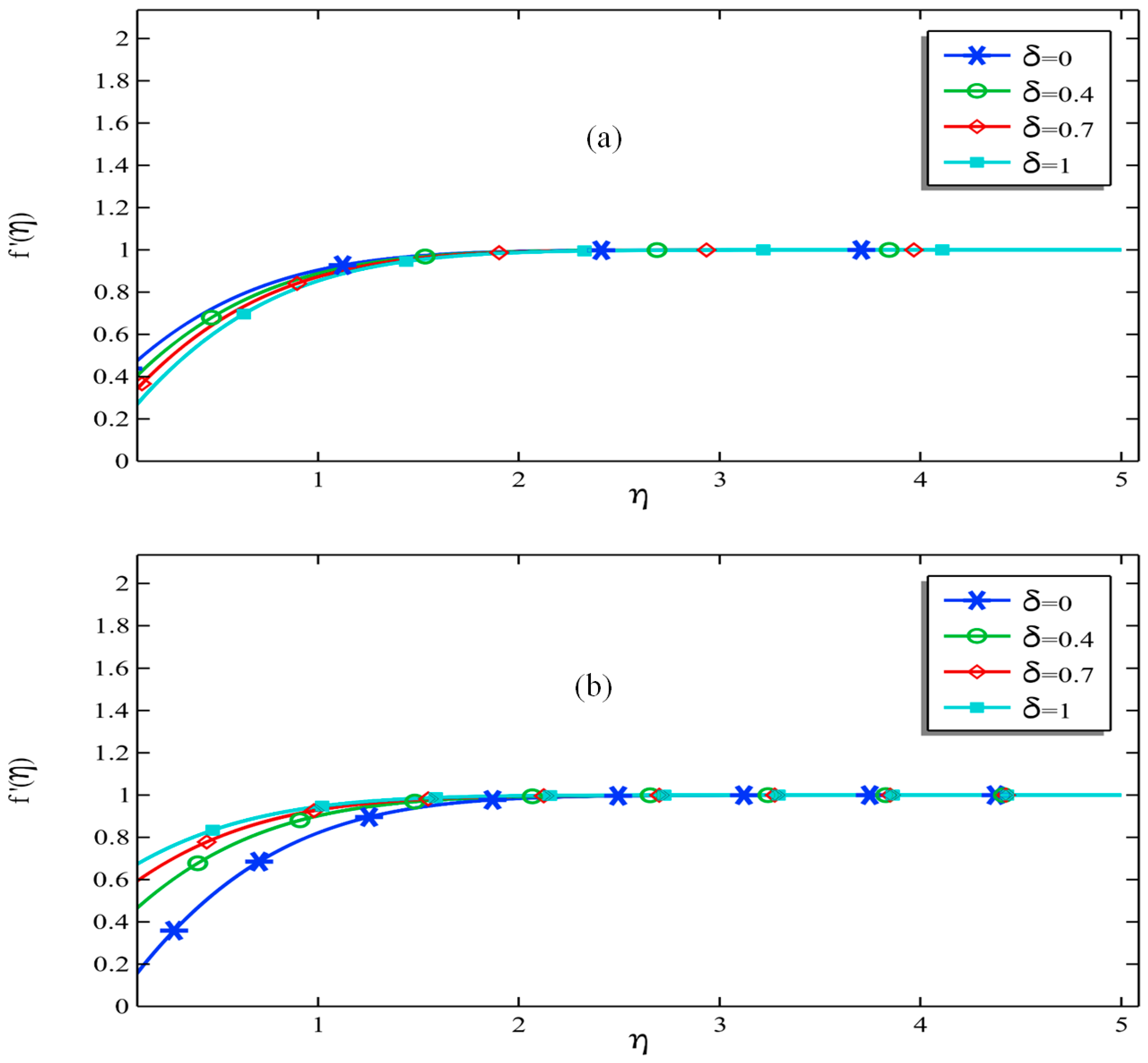
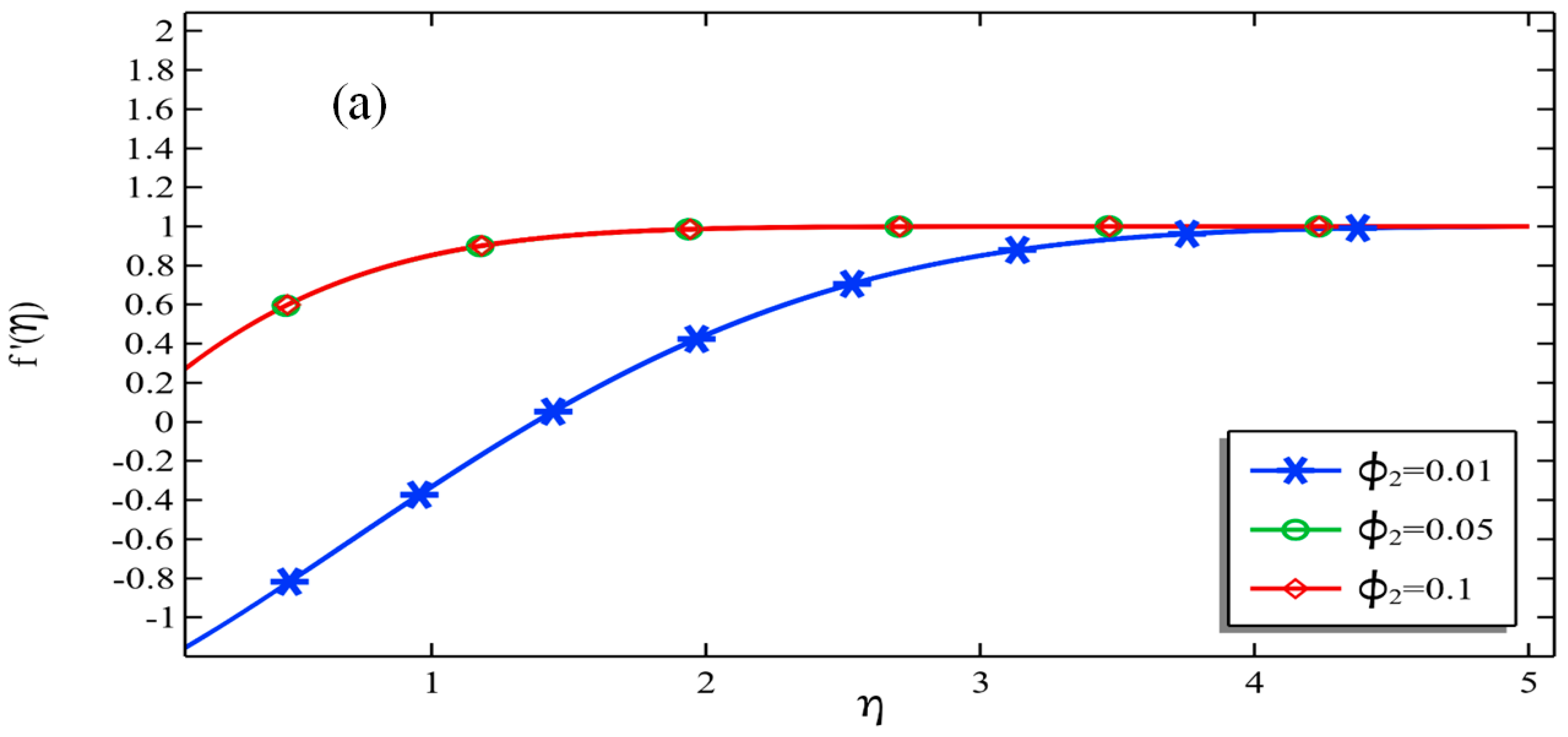
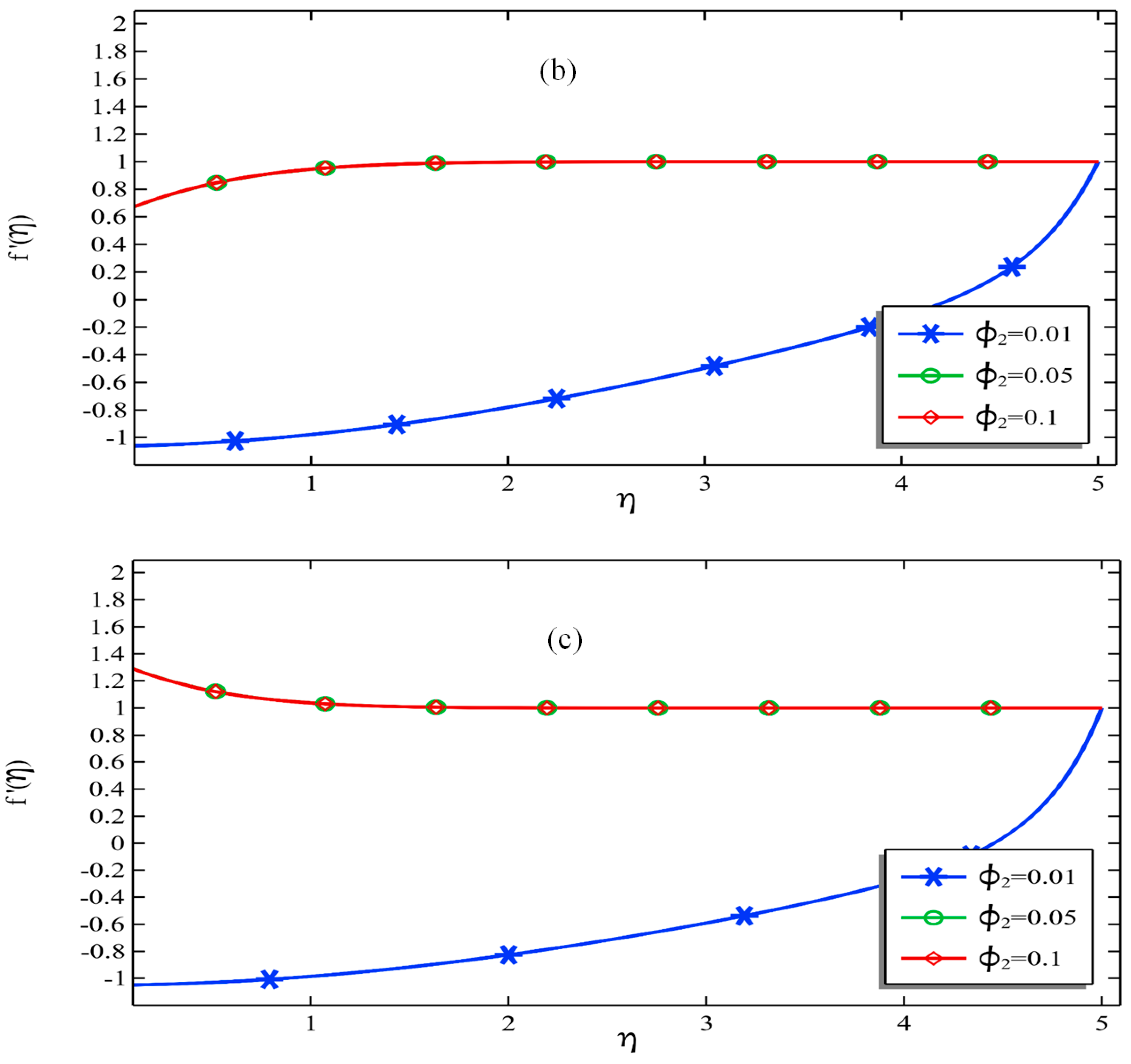
4.1. Velocity Outline
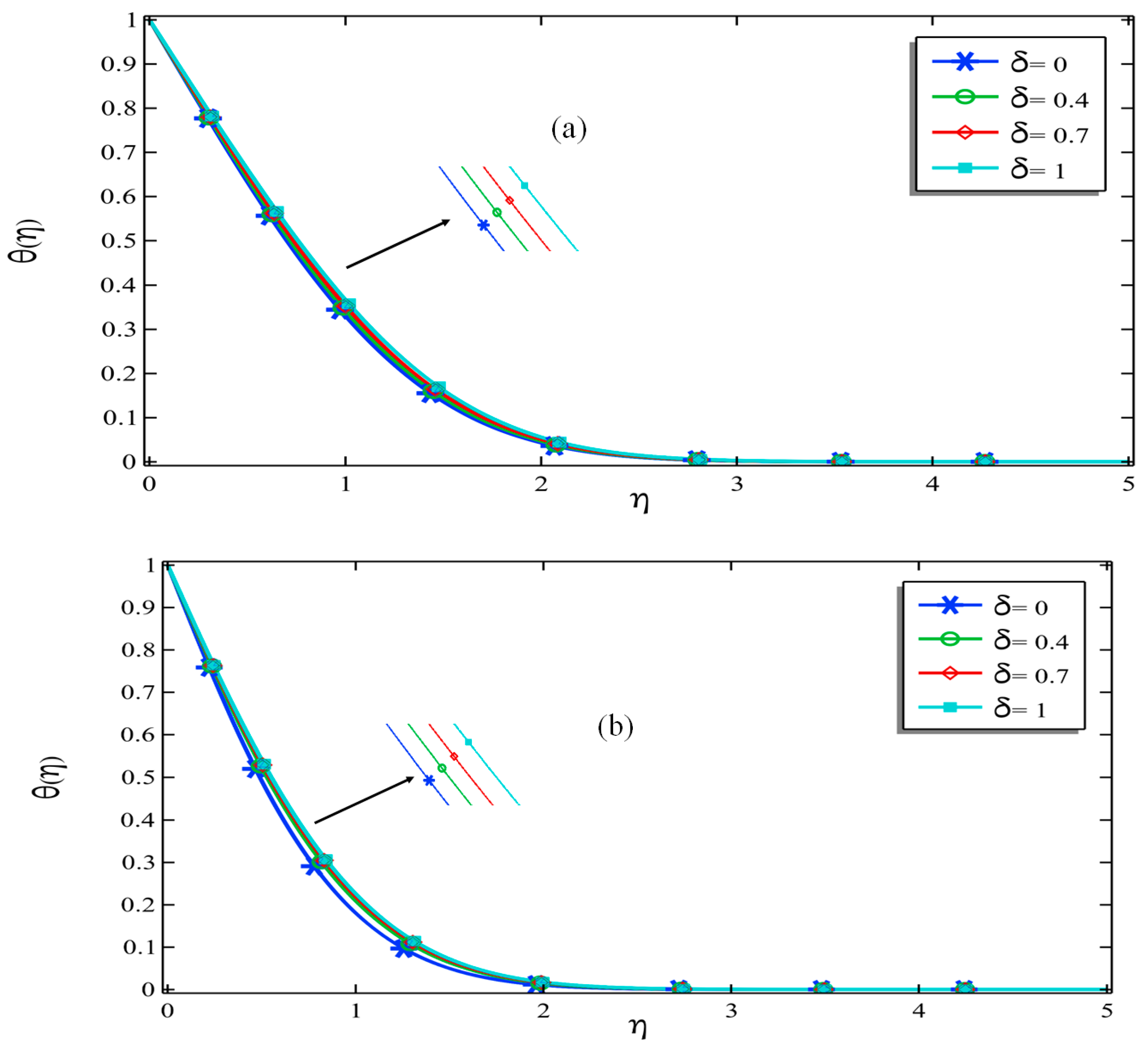
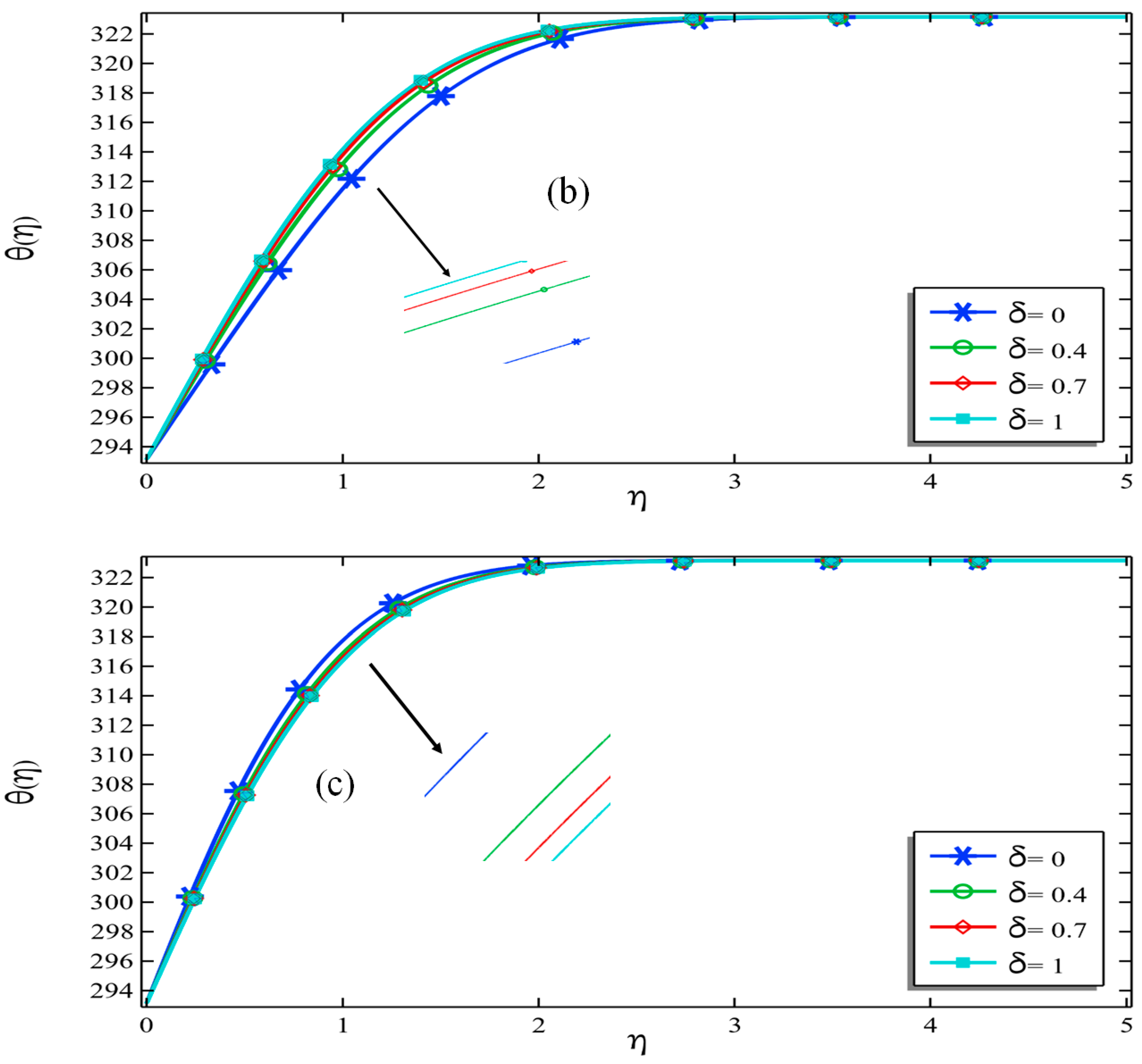
4.2. Temperature Outline
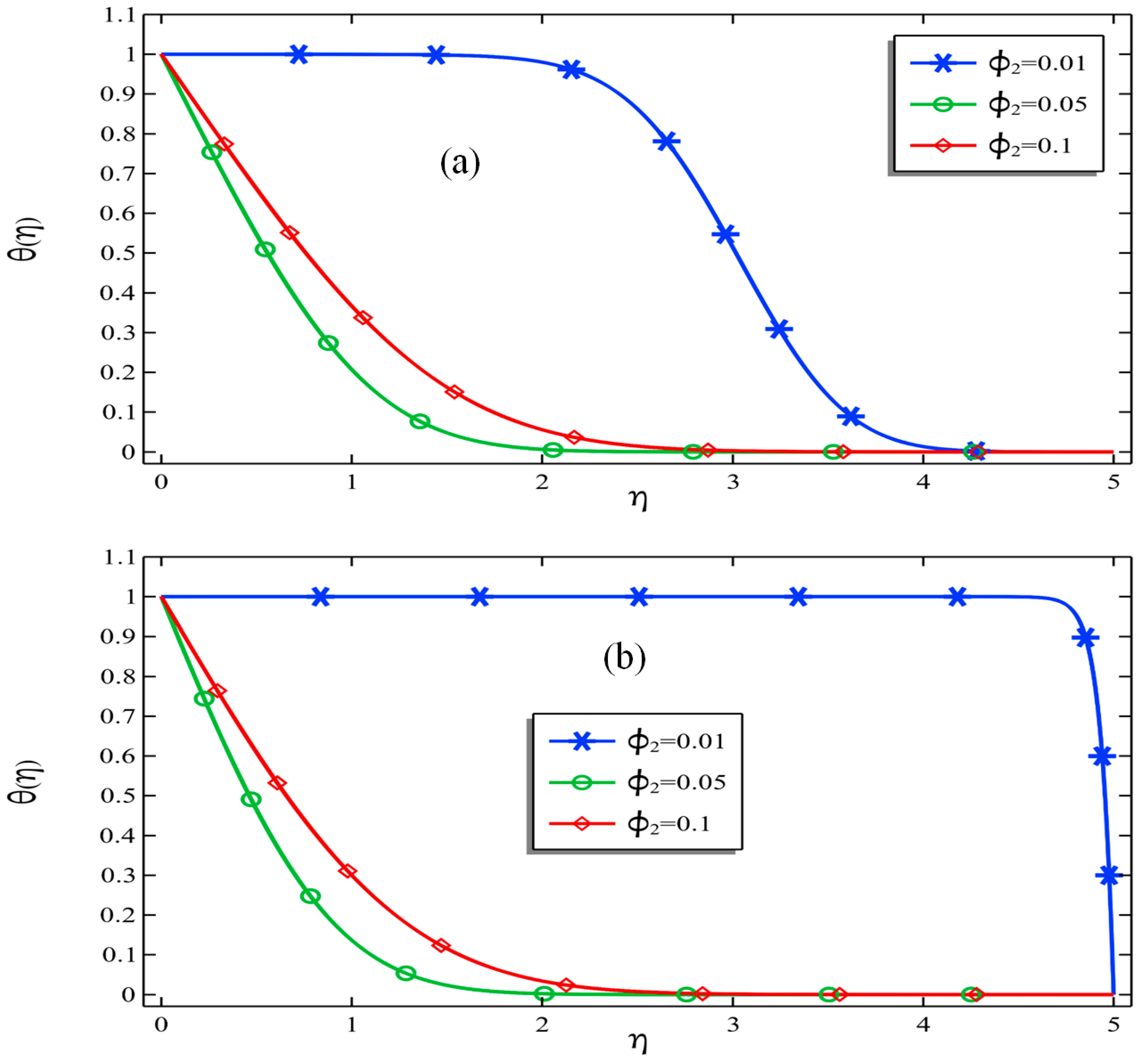
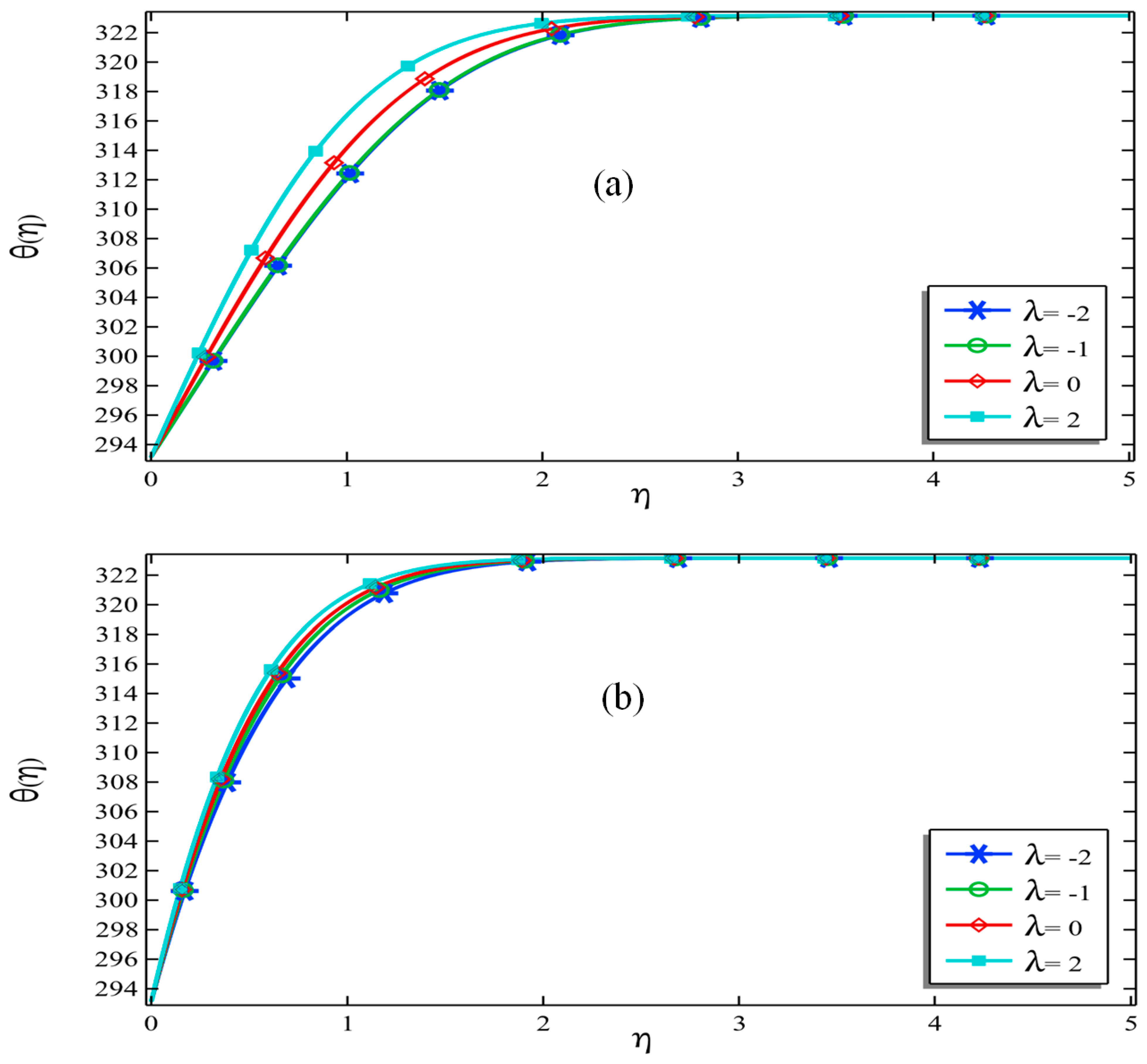
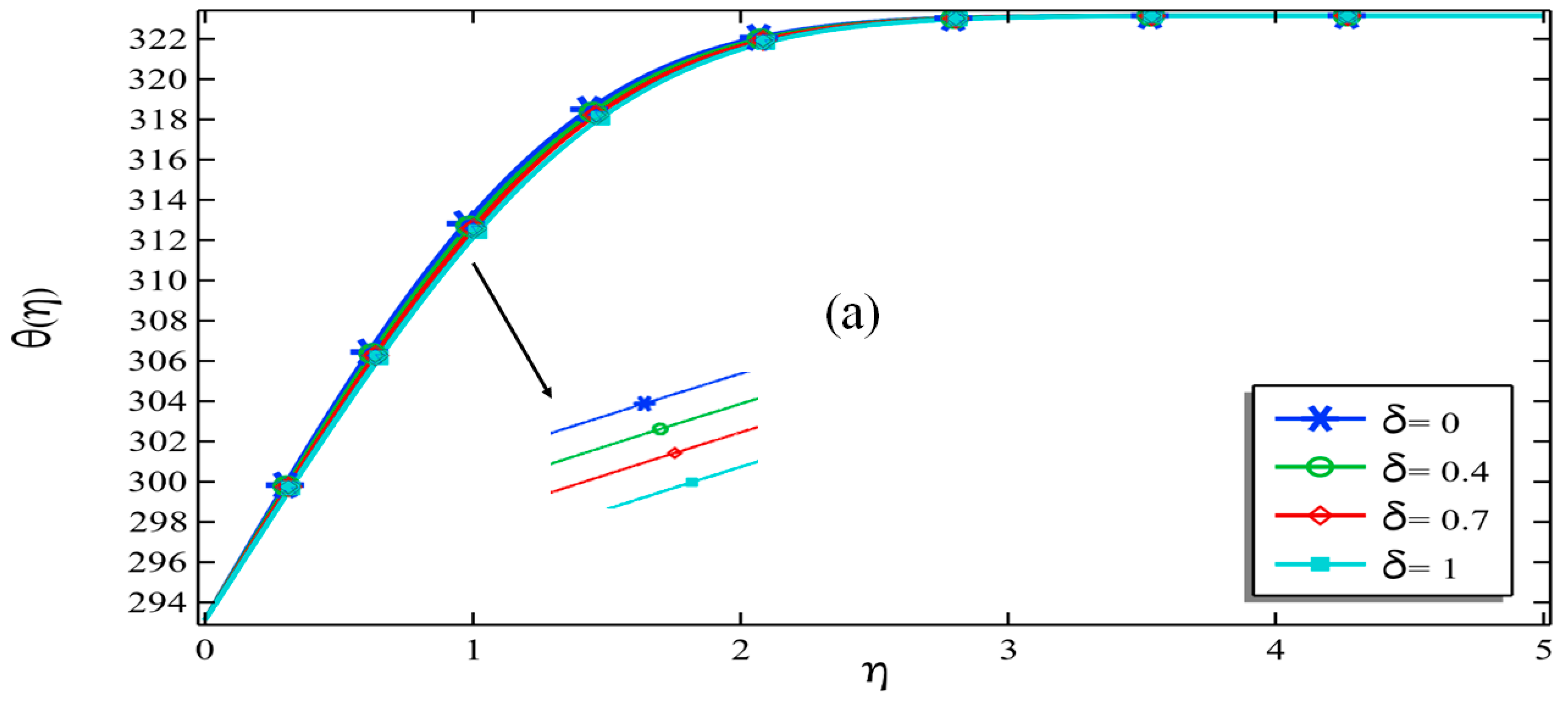
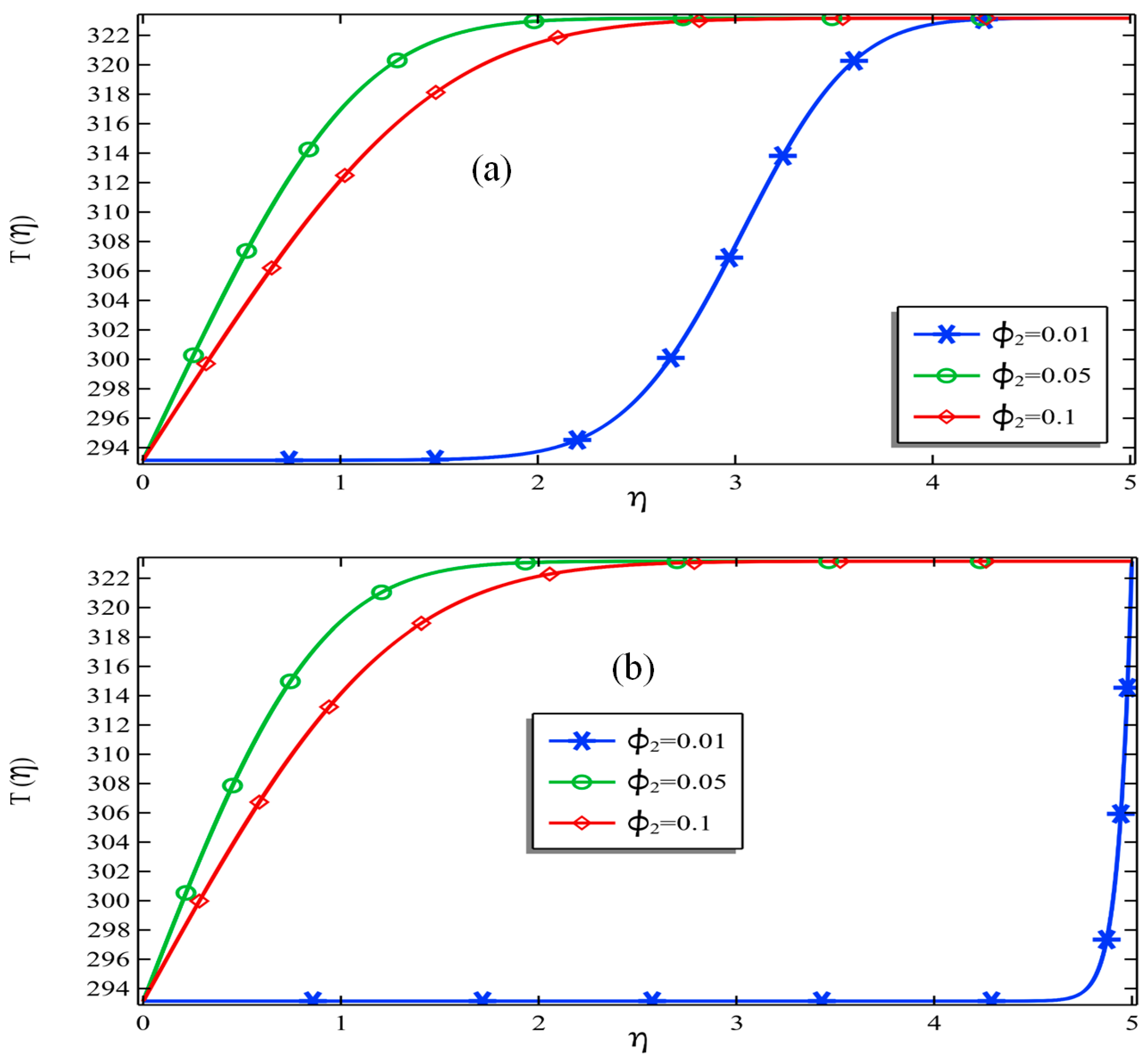
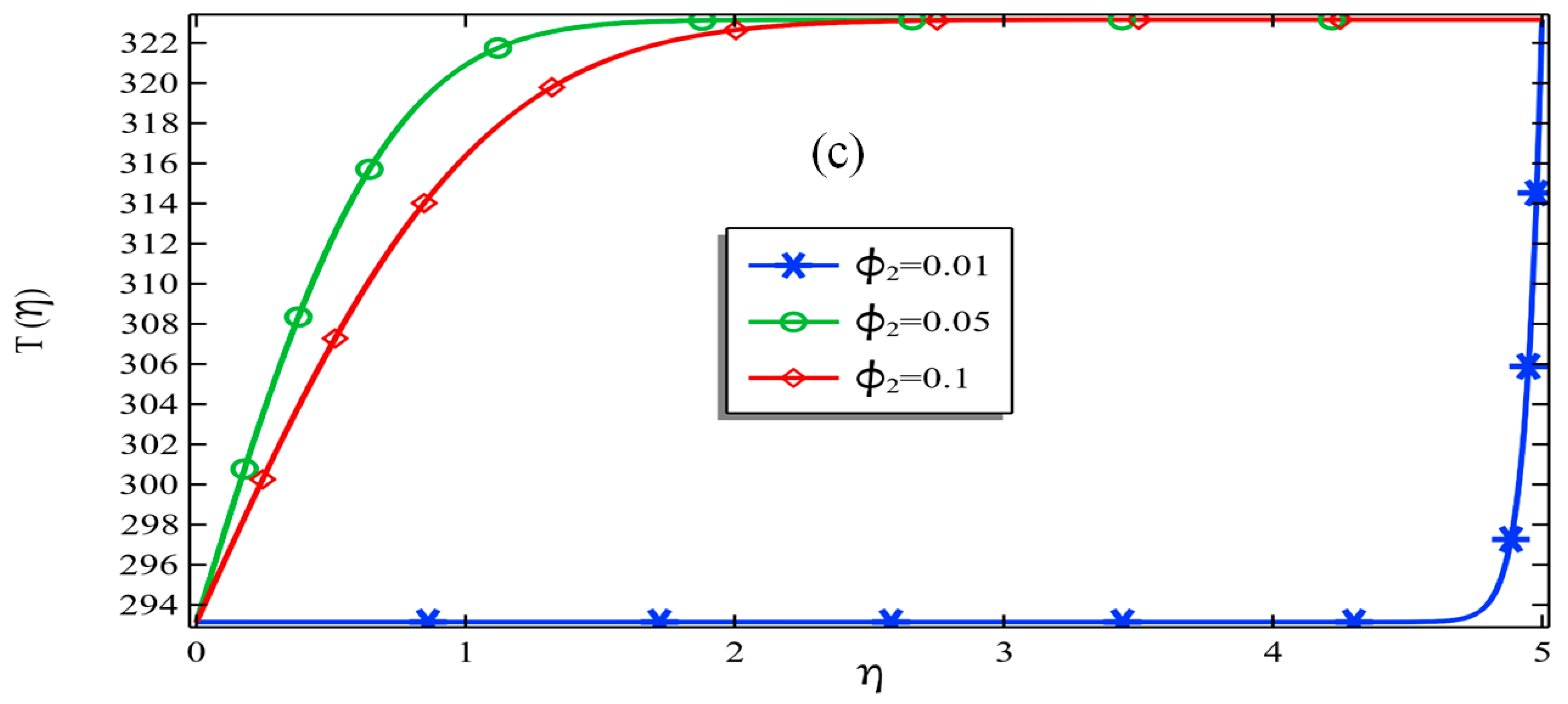
4.3. Temperature Distribution
4.4. Nusselt Number and Skin Friction Coefficient
4.5. An Application of the Results in PV/T
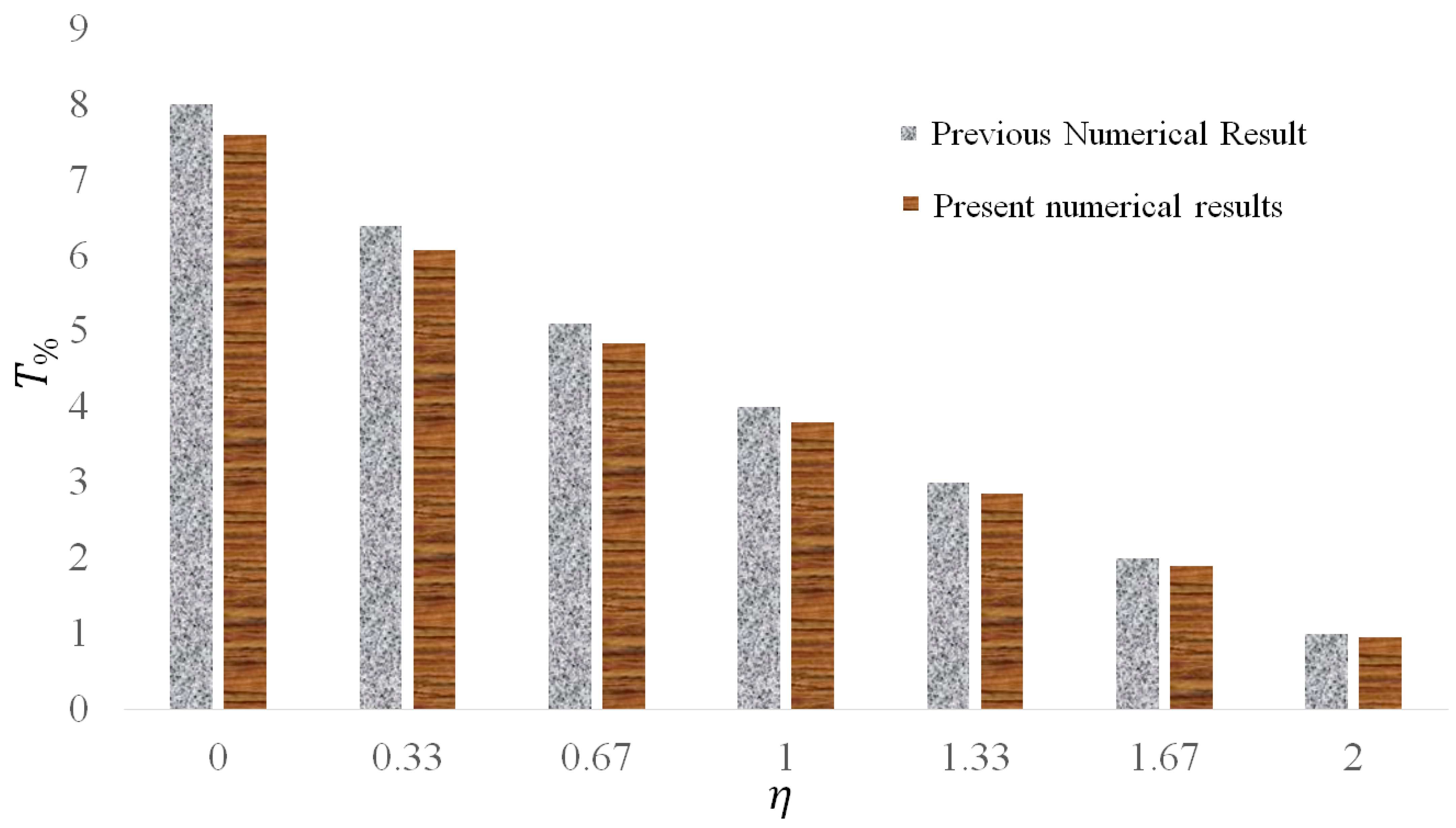
Validation of the Work
5. Conclusions
- ➢
- The velocity outline escalated as the normalized distance from the stagnant point escalated. It escalated in the shrinking case and decayed in the stretching case, while increasing the suction parameter always led to a decay in the velocity outline.
- ➢
- The temperature outline was negatively related to , and increasing the suction parameter significantly decayed the temperature outline. It was also concluded that in both the stretching and shrinking cases, projecting the estimations of the suction parameter led to a decay in the temperature outline.
- ➢
- When observing a specific case of temperature distribution from a cooling to a hot environment, it was observed that the temperature distribution was slightly slower in the shrinking case compared to the stretching case. Additionally, the temperature increment could be better controlled by increasing the slip flow parameter. The temperature expansion along the sheet was directly proportional to estimations but could be better controlled by increasing or decreasing the suction parameter.
- ➢
- An escalation in the stretching/shrinking parameter from −2 to 2 estimations enhanced the convective process, resulting in an escalated Nusselt number. Especially in the shrinking case, an escalation in the volume fraction of copper stabilized the convection in the domain, leading to an escalated Nusselt number.
- ➢
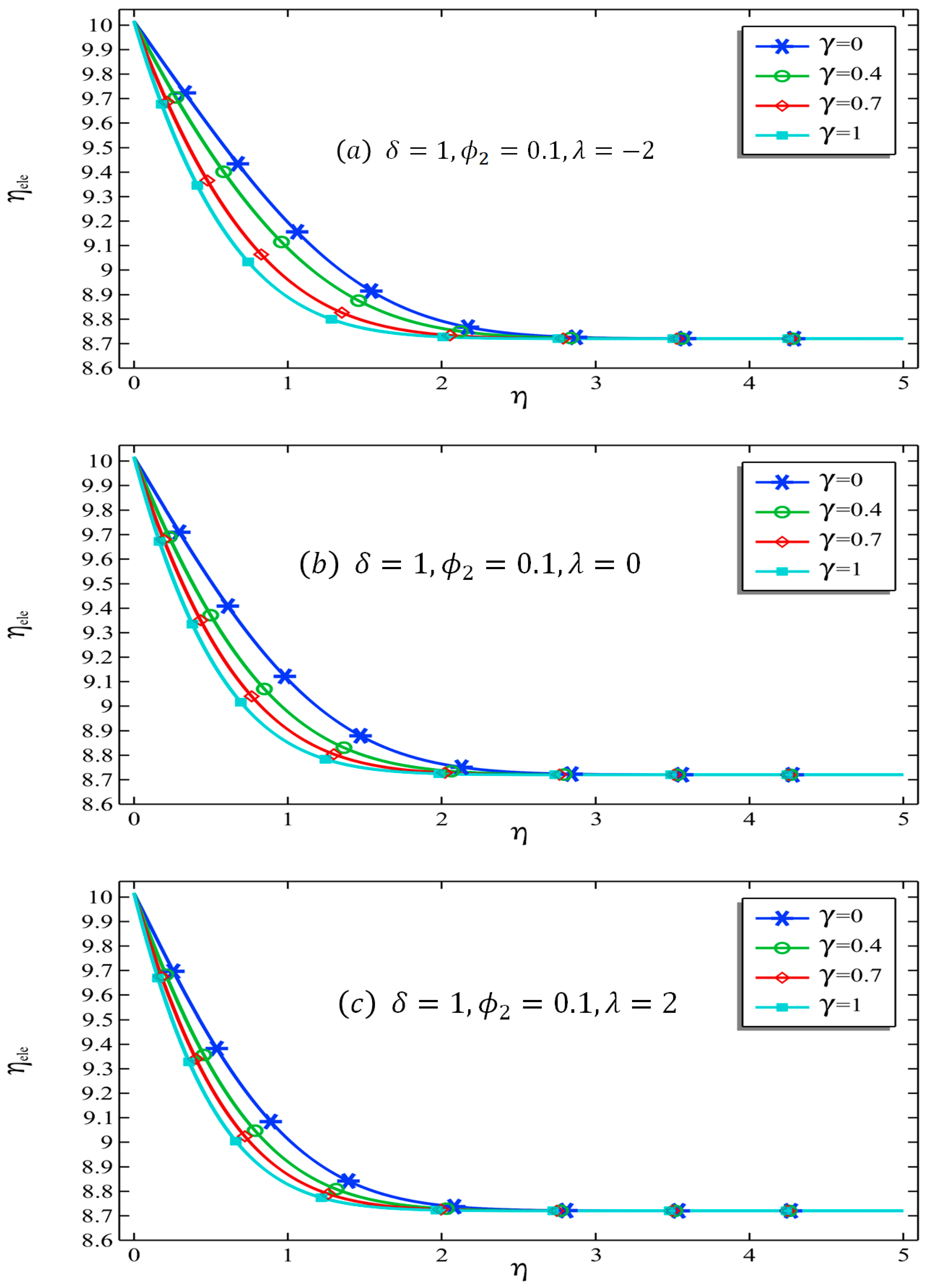
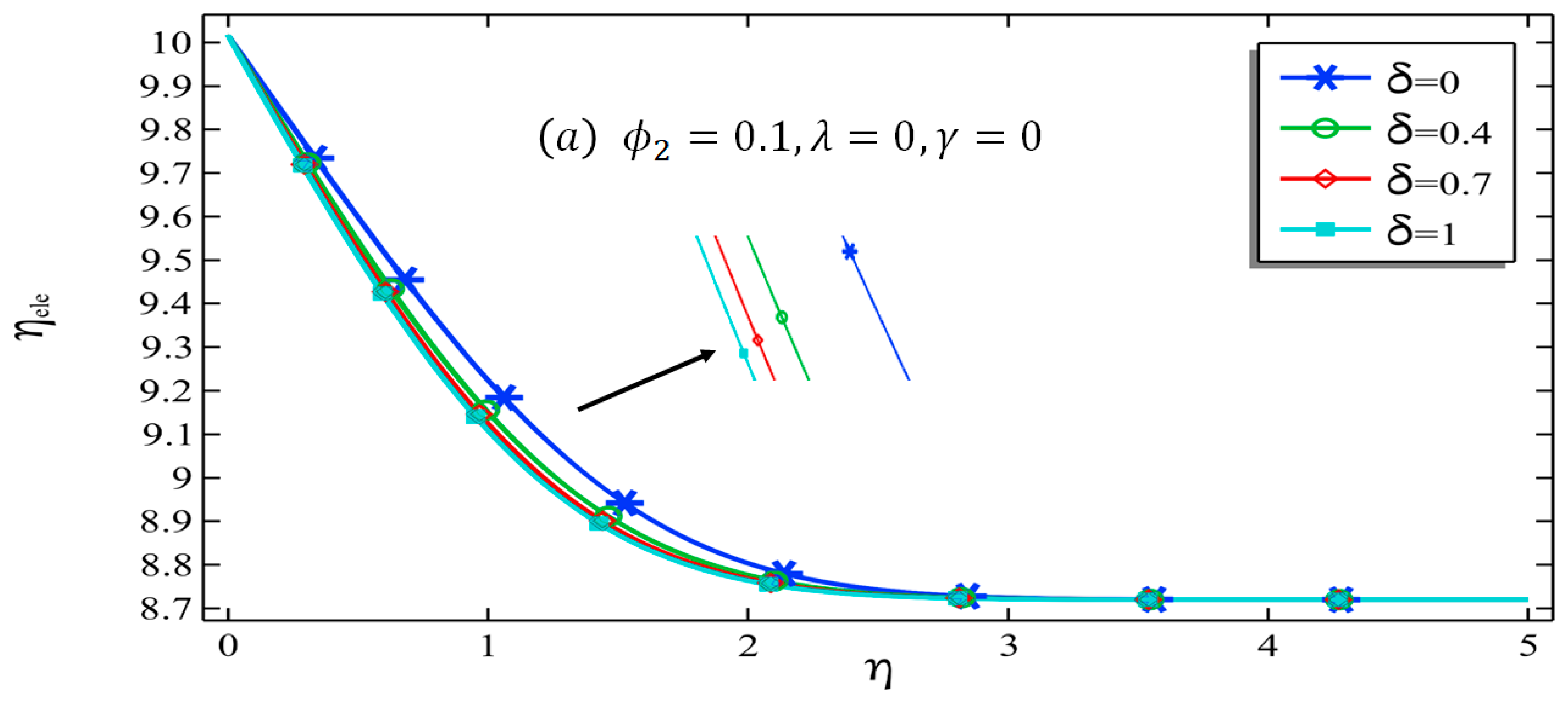
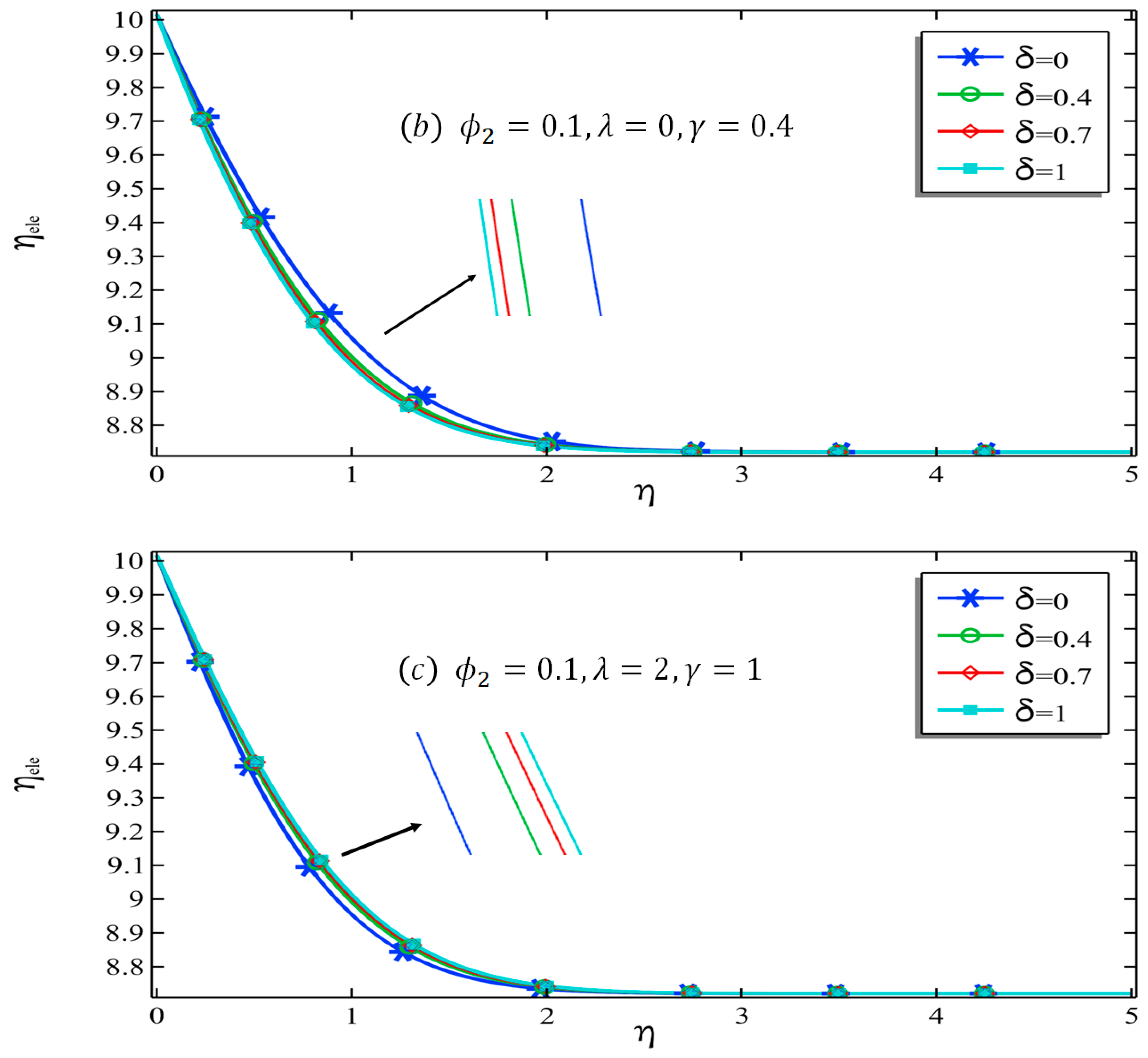
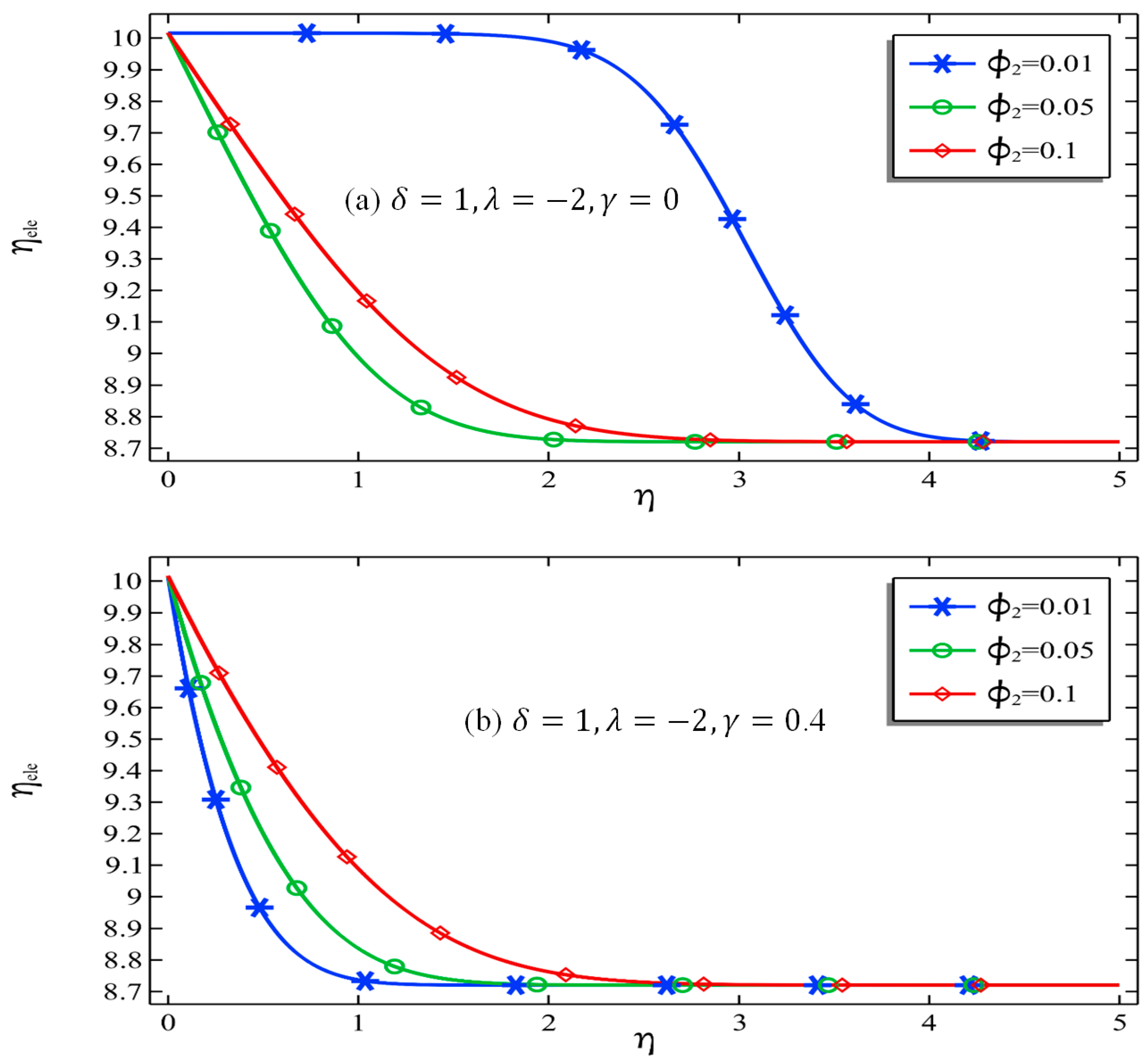
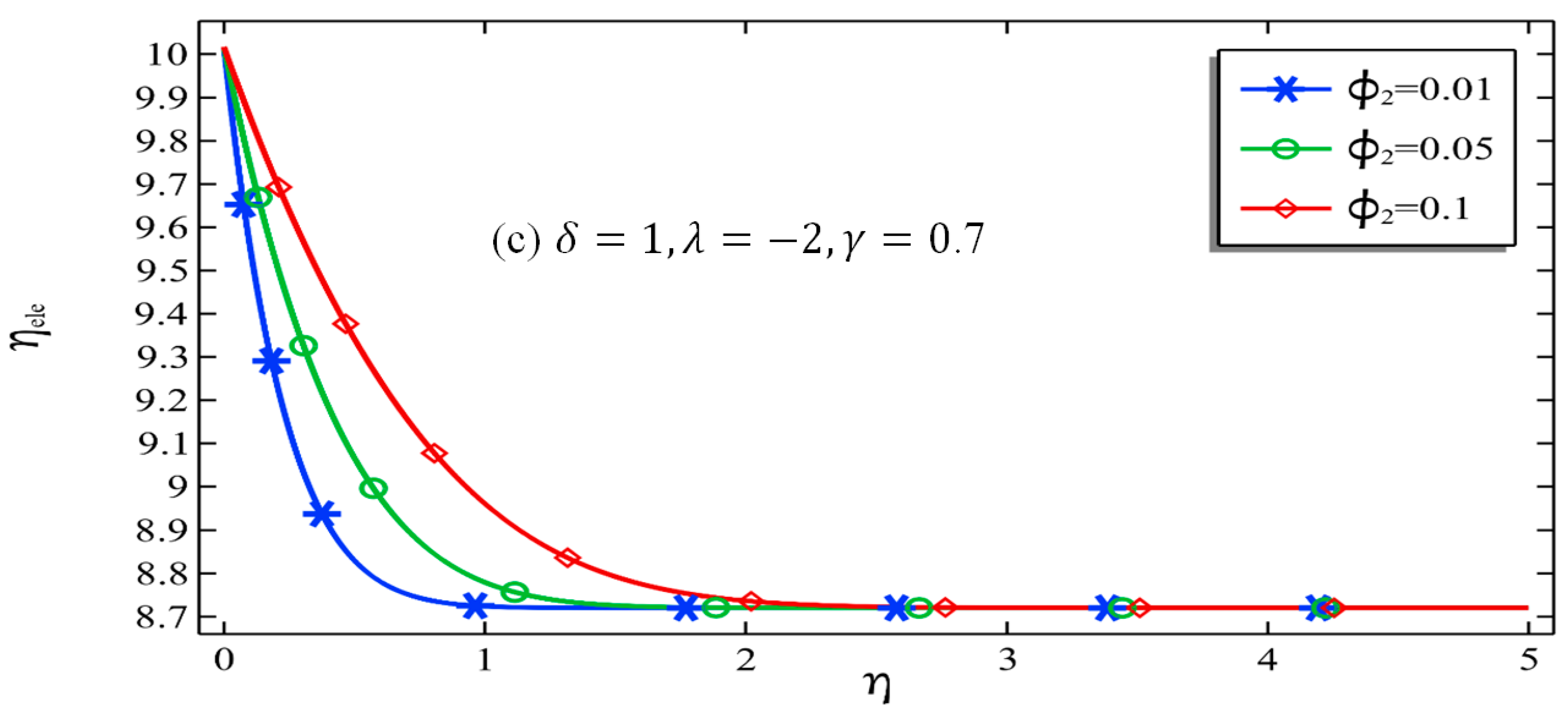
- Utilizing the application of stagnant point flow to enhance the electrical efficiency of a photovoltaic thermal system, it was observed that electrical efficiency declined with increasing estimations. Additionally, employing a sheet with stretching impact was noted as more effective for optimizing electrical production. Furthermore, it was observed that increasing the suction impact resulted in a decline in the electrical performance of the PV/T system.
- ➢
- In the presence of only a suction effect at the lower end of the sheet, the electrical efficiency of the PV/T system could be optimized by increasing the volume fraction of copper in the base fluid. The maximum average efficiency was achieved when = 0.4, = 2, = 0.7, and = 0.01, which was about 10%.
Future Directions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Area of the panel | |
| Stretching/shrinking sheet parameter | |
| Suction and injection parameter | |
| Specific heat of water | |
| Specific heat of nanofluids | |
| Specific heat of nanoparticles | |
| Specific heat of alumina | |
| Specific heat of copper | |
| Solar irradiance | |
| Mass flow rate | |
| Horizontal velocity component | |
| Vertical velocity component | |
| Temperature | |
| Inlet temperature | |
| Outlet temperature | |
| Air temperature | |
| Wall velocity | |
| Ambient velocity | |
| Suction at boundary | |
| Total volume fraction | |
| Volume fraction of alumina | |
| Volume fraction of copper | |
| Slip flow parameter | |
| Suction parameter | |
| Similarity parameter | |
| Viscosity of water | |
| Viscosity of nanofluids | |
| Thermal conductivity of water | |
| Thermal conductivity of nanofluids | |
| Total thermal conductivity of nanoparticles | |
| Thermal conductivity of alumina | |
| Thermal conductivity of copper | |
| Stretching/shrinking parameter | |
| Kinematic viscosity | |
| Temperature profile | |
| Density of base fluid | |
| Density of hybrid nanofluids | |
| Total density of nanoparticles | |
| Density of alumina | |
| Density of copper | |
| Stream functions |
References
- Alghamdi, M.; Memon, A.A.; Muhammad, T.; Ali, M.R. A numerical investigation of a photovoltaic thermal system contained a trapezoidal channel with transport of silver and titanium oxide using the water as base fluids. Case Stud. Therm. Eng. 2023, 47, 103056. [Google Scholar] [CrossRef]
- Usman; Memon, A.A.; Alghamdi, M.; Muhammad, T. A forced convection of water aluminum oxide nanofluid flow and heat transfer study for a three dimensional annular with inner rotated cylinder. Sci. Rep. 2022, 12, 16735. [Google Scholar] [CrossRef]
- Memon, A.A.; Anwaar, H.; Muhammad, T.; Alharbi, A.A.; Alshomrani, A.S.; Aladwani, Y.R. A forced convection of water-aluminum oxide nanofluids in a square cavity containing a circular rotating disk of unit speed with high Reynolds number: A Comsol Multiphysics study. Case Stud. Therm. Eng. 2022, 39, 102370. [Google Scholar]
- Bhattacharyya, K.; Layek, G.C. Effects of suction/blowing on steady boundary layer stagnation-point flow and heat transfer towards a shrinking sheet with thermal radiation. Int. J. Heat Mass Transf. 2011, 54, 302–307. [Google Scholar] [CrossRef]
- Choi, S.U.; Eastman, J.A. Enhancing Thermal Conductivity of Fluids with Nanoparticles; No. ANL/MSD/CP-84938; CONF-951135-29; Argonne National Lab. (ANL): Argonne, IL, USA, 1995. [Google Scholar]
- Gislén, A.; Dacke, M.; Kröger, R.H.; Abrahamsson, M.; Nilsson, D.E.; Warrant, E.J. Superior underwater vision in a human population of sea gypsies. Curr. Biol. 2003, 13, 833–836. [Google Scholar] [CrossRef]
- Bachok, N.; Ishak, A.; Nazar, R.; Senu, N. Stagnation-point flow over a permeable stretching/shrinking sheet in a copper-water nanofluid. Bound. Value Probl. 2013, 2013, 39. [Google Scholar] [CrossRef]
- Deng, S.; Zhou, A.; Yue, D.; Hu, B.; Zhu, L. Distributed intrusion detection based on hybrid gene expression programming and cloud computing in cyber physical power system. IET Control. Theory Appl. 2017, 11, 1822–1829. [Google Scholar] [CrossRef]
- Buongiorno, J. Convective transport in nanofluids. J. Heat Transfer. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Daungthongsuk, W.; Wongwises, S. A critical review of convective heat transfer of nanofluids. Renew. Sustain. Energy Rev. 2007, 11, 797–817. [Google Scholar] [CrossRef]
- Trisaksri, V.; Wongwises, S. Critical review of heat transfer characteristics of nanofluids. Renew. Sustain. Energy Rev. 2007, 11, 512–523. [Google Scholar] [CrossRef]
- Wang, X.Q.; Mujumdar, A.S. A review on nanofluids-part I: Theoretical and numerical investigations. Braz. J. Chem. Eng. 2008, 25, 613–630. [Google Scholar] [CrossRef]
- Kakaç, S.; Pramuanjaroenkij, A. Review of convective heat transfer enhancement with nanofluids. Int. J. Heat Mass Transf. 2009, 52, 3187–3196. [Google Scholar] [CrossRef]
- Khan, W.A.; Pop, I. Boundary-layer flow of a nanofluid past a stretching sheet. Int. J. Heat Mass Transf. 2010, 53, 2477–2483. [Google Scholar] [CrossRef]
- Nield, D.A.; Kuznetsov, A.V. The Cheng–Minkowycz problem for natural convective boundary-layer flow in a porous medium saturated by a nanofluid. Int. J. Heat Mass Transf. 2009, 52, 5792–5795. [Google Scholar] [CrossRef]
- Nield, D.A.; Kuznetsov, A.V. The Cheng–Minkowycz problem for the double-diffusive natural convective boundary layer flow in a porous medium saturated by a nanofluid. Int. J. Heat Mass Transf. 2011, 54, 374–378. [Google Scholar] [CrossRef]
- Kuznetsov, A.V.; Nield, D.A. Natural convective boundary-layer flow of a nanofluid past a vertical plate. Int. J. Therm. Sci. 2010, 49, 243–247. [Google Scholar] [CrossRef]
- Kuznetsov, A.V.; Nield, D.A. Double-diffusive natural convective boundary-layer flow of a nanofluid past a vertical plate. Int. J. Therm. Sci. 2011, 50, 712–717. [Google Scholar] [CrossRef]
- Bachok, N.; Ishak, A.; Pop, I. Boundary-layer flow of nanofluids over a moving surface in a flowing fluid. Int. J. Therm. Sci. 2010, 49, 1663–1668. [Google Scholar] [CrossRef]
- Bachok, N.; Ishak, A.; Pop, I. Unsteady boundary-layer flow and heat transfer of a nanofluid over a permeable stretching/shrinking sheet. Int. J. Heat Mass Transf. 2012, 55, 2102–2109. [Google Scholar] [CrossRef]
- Khan, W.A.; Aziz, A. Natural convection flow of a nanofluid over a vertical plate with uniform surface heat flux. Int. J. Therm. Sci. 2011, 50, 1207–1214. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, M.I.; Waqas, M.; Alsaedi, A.; Khan, M.I. Radiative flow of micropolar nanofluid accounting thermophoresis and Brownian moment. Int. J. Hydrogen Energy 2017, 42, 16821–16833. [Google Scholar] [CrossRef]
- Khan, M.I.; Hayat, T.; Khan, M.I.; Alsaedi, A. Activation energy impact in nonlinear radiative stagnation point flow of Cross nanofluid. Int. Commun. Heat Mass Transf. 2018, 91, 216–224. [Google Scholar] [CrossRef]
- Hayat, T.; Muhammad, T.; Alsaedi, A.; Alhuthali, M.S. Magnetohydrodynamic three-dimensional flow of viscoelastic nanofluid in the presence of nonlinear thermal radiation. J. Magn. Magn. Mater. 2015, 385, 222–229. [Google Scholar] [CrossRef]
- Hayat, T.; Aziz, A.; Muhammad, T.; Alsaedi, A. On magnetohydrodynamic three-dimensional flow of nanofluid over a convectively heated nonlinear stretching surface. Int. J. Heat Mass Transf. 2016, 100, 566–572. [Google Scholar] [CrossRef]
- Hayat, T.; Muhammad, T.; Shehzad, S.A.; Alsaedi, A. An analytical solution for magnetohydrodynamic Oldroyd-B nanofluid flow induced by a stretching sheet with heat generation/absorption. Int. J. Therm. Sci. 2017, 111, 274–288. [Google Scholar] [CrossRef]
- Muhammad, T.; Alsaedi, A.; Hayat, T.; Shehzad, S.A. A revised model for Darcy-Forchheimer three-dimensional flow of nanofluid subject to convective boundary condition. Results Phys. 2017, 7, 2791–2797. [Google Scholar] [CrossRef]
- Muhammad, T.; Alsaedi, A.; Shehzad, S.A.; Hayat, T. A revised model for Darcy-Forchheimer flow of Maxwell nanofluid subject to convective boundary condition. Chin. J. Phys. 2017, 55, 963–976. [Google Scholar] [CrossRef]
- Abu-Nada, E. Application of nanofluids for heat transfer enhancement of separated flows encountered in a backward facing step. Int. J. Heat Fluid Flow 2008, 29, 242–249. [Google Scholar] [CrossRef]
- Ahmad, S.; Rohni, A.M.; Pop, I. Blasius and Sakiadis problems in nanofluids. Acta Mech. 2011, 218, 195–204. [Google Scholar] [CrossRef]
- Bachok, N.; Ishak, A.; Pop, I. Flow and heat transfer over a rotating porous disk in a nanofluid. Phys. B Condens. Matter 2011, 406, 1767–1772. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, M.I.; Waqas, M.; Alsaedi, A.; Farooq, M. Numerical simulation for melting heat transfer and radiation effects in stagnation point flow of carbon–water nanofluid. Comput. Methods Appl. Mech. Eng. 2017, 315, 1011–1024. [Google Scholar] [CrossRef]
- Hayat, T.; Khan, M.I.; Farooq, M.; Alsaedi, A.; Yasmeen, T. Impact of Marangoni convection in the flow of carbon–water nanofluid with thermal radiation. Int. J. Heat Mass Transf. 2017, 106, 810–815. [Google Scholar] [CrossRef]
- Miklavčič, M.; Wang, C. Viscous flow due to a shrinking sheet. Q. Appl. Math. 2006, 64, 283–290. [Google Scholar] [CrossRef]
- Yousefi, K.; Saleh, R. Three-dimensional suction flow control and suction jet length optimization of NACA 0012 wing. Meccanica 2015, 50, 1481–1494. [Google Scholar] [CrossRef]
- Zhang, W.; Jiang, Y.; Li, L.; Chen, G. Effects of wall suction/blowing on two-dimensional flow past a confined square cylinder. SpringerPlus 2016, 5, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Saeed, F.; Selig, M.S. Multipoint inverse airfoil design method for slot-suction airfoils. J. Aircr. 1996, 33, 708–715. [Google Scholar] [CrossRef][Green Version]
- Sheikholeslami, M. Effect of uniform suction on nanofluid flow and heat transfer over a cylinder. J. Braz. Soc. Mech. Sci. Eng. 2015, 37, 1623–1633. [Google Scholar] [CrossRef]
- Mahian, O.; Kolsi, L.; Amani, M.; Estellé, P.; Ahmadi, G.; Kleinstreuer, C.; Marshall, J.S.; Siavashi, M.; Taylor, R.A.; Niazmand, H.; et al. Recent advances in modeling and simulation of nanofluid flows-Part I: Fundamentals and theory. Phys. Rep. 2019, 790, 1–48. [Google Scholar] [CrossRef]
- Memon, A.A.; Memon, M.A.; Fenta, A. A laminar forced convection via transport of water–copper–aluminum hybrid nanofluid through heated deep and shallow cavity with Corcione model. Sci. Rep. 2023, 13, 4915. [Google Scholar] [CrossRef] [PubMed]
- Akkurt, N.; Shedd, T.; Memon, A.A.; Ali, M.R.; Bouye, M. Analysis of the forced convection via the turbulence transport of the hybrid mixture in three-dimensional L-shaped channel. Case Stud. Therm. Eng. 2023, 41, 102558. [Google Scholar] [CrossRef]
- Alqarni, M.S.; Memon, A.A.; Anwaar, H.; Usman and Muhammad, T. The forced convection analysis of water alumina nanofluid flow through a 3D annulus with rotating cylinders via κ−ε turbulence model. Energies 2022, 15, 6730. [Google Scholar] [CrossRef]
- Elhag, S.H.; Memon, A.A.; Memon, M.A.; Bhatti, K.; Jacob, K.; Alzahrani, S.; Seidu, J. Analysis of forced convection with hybrid Cu-Al2O3 nanofluids injected in a three-dimensional rectangular channel containing three perpendicular rotating blocks with turbulent modeling. J. Nanomater. 2022, 2022, 2446972. [Google Scholar] [CrossRef]
- Memon, A.A.; Khan, W.A.; Muhammad, T. Numerical investigation of photovoltaic thermal energy efficiency improvement using the backward step containing Cu-Al2O3 hybrid nanofluid. Alex. Eng. J. 2023, 75, 391–406. [Google Scholar] [CrossRef]
- Yashkun, U.; Zaimi, K.; Bakar, N.A.A.; Ferdows, M. Nanofluid stagnation-point flow using Tiwari and Das model over a stretching/shrinking sheet with suction and slip effects. J. Adv. Res. Fluid Mech. Therm. Sci. 2020, 70, 62–76. [Google Scholar] [CrossRef]
- Alharbi, A.A. Thermal analysis of heat transport in a slip flow of ternary hybrid nanofluid with suction upon a stretching/shrinking sheet. Case Stud. Therm. Eng. 2024, 54, 103965. [Google Scholar] [CrossRef]
- Shaikh, G.M.; Memon, A.A.; Memon, M.A.; Yashkun, U.; Obalalu, A.M.; Koten, H. Numerical study of flow behavior and heat transfer of ternary water-based nanofluids in the presence of suction/injection, stretching/shrinking sheet. J. Therm. Eng. 2024, 10, 1021–1043. [Google Scholar] [CrossRef]
- Joshi, T.; Parkash, O.; Gallegos, R.K.B.; Krishan, G. Parametric investigation of slurry transport: Computational insight into the impact of particle composition and Prandtl numbers. Phys. Fluids 2024, 36, 023308. [Google Scholar] [CrossRef]
- Allehiany, F.M.; Memon, A.A.; Memon, M.A.; Fenta, A. Maximizing electrical output and reducing heat-related losses in photovoltaic thermal systems with a thorough examination of flow channel integration and nanofluid cooling. Sci. Rep. 2023, 13, 16961. [Google Scholar] [CrossRef]
- Akram, M.; Memon, A.A.; Memon, M.A.; Obalalu, A.M.; Khan, U. Investigation of a two-dimensional photovoltaic thermal system using hybrid nanofluids and a rotating cylinder. Nanoscale Adv. 2023, 5, 5529–5542. [Google Scholar] [CrossRef]
- Khanjari, Y.; Pourfayaz, F.; Kasaeian, A.B. Numerical investigation on using of nanofluid in a water-cooled photovoltaic thermal system. Energy Convers. Manag. 2016, 122, 263–278. [Google Scholar] [CrossRef]
- Bhattarai, S.; Oh, J.H.; Euh, S.H.; Kafle, G.K.; Kim, D.H. Simulation and model validation of sheet and tube type photovoltaic thermal solar system and conventional solar collecting system in transient states. Sol. Energy Mater. Sol. Cells 2012, 103, 184–193. [Google Scholar] [CrossRef]







| Symbol | Expression/Range of Estimations | Description |
|---|---|---|
| Stretching and shrinking parameter | ||
| Kinematic viscosity of nanofluid | ||
| Suction parameter | ||
| Slip flow parameter | ||
| (2nd derivative of f) | ||
| (2nd derivative of θ) |
| Symbol | Related Expression/Estimations | Description |
|---|---|---|
| Volume fraction of alumina | ||
| 0.01 | Volume fraction of copper | |
| 3880 [kg/m3] | Density of alumina | |
| 8954 [kg/m3] | Density of copper | |
| Total density of nanoparticles | ||
| 765 [J/(kg K)] | Specific heat of alumina | |
| 383.1 [J/(kg K)] | Specific heat of copper | |
| Specific heat of particles | ||
| Total volume fraction of nanoparticles | ||
| 40 [W/(m K)] | Thermal conductivity of alumina | |
| 386 [W/(m K)] | Thermal conductivity of copper | |
| Total thermal conductivity of nanofluid | ||
| 998 [kg/m3] | Density of base fluid | |
| Density of hybrid nanofluid | ||
| 4182 [J/(kg K)] | Specific heat of base fluid | |
| Specific heat capacity of nanofluid | ||
| 0.597 [W/(m K)] | Thermal conductivity of the base fluid | |
| Thermal conductivity of the nanofluid | ||
| 0.000998 [Pa s] | Viscosity of the base fluid | |
| Viscosity of nanofluid | ||
| 298.15 [K] | Cool temperature/wall temperature | |
| 318.15 [K] | Hot temperature/ambient |
| 0 | −2 | 0 | 0.05 | −1.63 × 10−7 | −1.47 × 10−8 |
| 0 | −2 | 0 | 0.1 | −1.22 × 10−6 | −1.00 × 10−7 |
| 0 | −2 | 1 | 0.01 | 5.26 × 10−6 | 7.69 × 10−6 |
| 0 | −2 | 1 | 0.05 | −3.33 × 10−7 | −2.95 × 10−8 |
| 0 | −2 | 1 | 0.1 | −5.91 × 10−6 | −5.07 × 10−7 |
| 0 | −1 | 0 | 0.01 | 7.23 × 10−5 | 0.066168 |
| 0 | −1 | 0 | 0.05 | −4.46 × 10−7 | −4.10 × 10−8 |
| 0 | −1 | 0 | 0.1 | −9.69 × 10−8 | 2.86 × 10−8 |
| 0 | −1 | 1 | 0.01 | 6.01 × 10−6 | 0.085804 |
| 0 | −1 | 1 | 0.05 | −7.52 × 10−9 | −7.60 × 10−10 |
| 0 | −1 | 1 | 0.1 | −1.96 × 10−7 | 2.90 × 10−8 |
| 0 | 0 | 0 | 0.01 | −2.45 × 10−6 | 0.2831 |
| 0 | 0 | 0 | 0.05 | −7.58 × 10−9 | −1.04 × 10−9 |
| 0 | 0 | 0 | 0.1 | 6.93 × 10−14 | 2.27 × 10−8 |
| 0 | 0 | 1 | 0.01 | −2.64 × 10−6 | 0.2856 |
| 0 | 0 | 1 | 0.05 | −2.00 × 10−8 | −7.20 × 10−10 |
| 0 | 0 | 1 | 0.1 | −5.22 × 10−8 | 3.49 × 10−9 |
| 0 | 1 | 0 | 0.01 | −4.42 × 10−6 | 0.37308 |
| 0 | 1 | 1 | 0.01 | 8.57 × 10−6 | 0.37384 |
| 0 | 2 | 0 | 0.01 | −5.21 × 10−6 | 0.40512 |
| 0 | 2 | 0 | 0.05 | −4.51 × 10−9 | −3.30 × 10−10 |
| 0 | 2 | 0 | 0.1 | −1.02 × 10−9 | 2.18 × 10−10 |
| 0 | 2 | 1 | 0.01 | 5.50 × 10−7 | 0.40521 |
| 0 | 2 | 1 | 0.05 | −8.30 × 10−8 | −6.99 × 10−9 |
| 0 | 2 | 1 | 0.1 | −6.75 × 10−10 | 6.90 × 10−10 |
| 0 | −2 | 0.05 | 1.3963 | 1.3457 | 1.2997 | 1.2454 |
| 0 | −2 | 0.1 | 1.3098 | 1.2722 | 1.238 | 1.1977 |
| 0 | −1 | 0.01 | 7.72 × 10−11 | 7.72 × 10−11 | 7.72 × 10−11 | 2.78 × 10−10 |
| 0 | −1 | 0.05 | 1.2701 | 1.2043 | 1.1527 | 1.2568 |
| 0 | −1 | 0.1 | 1.2164 | 1.1678 | 1.1297 | 1.2077 |
| 0 | 0 | 0.01 | 1.08 × 10−10 | 1.65 × 10−9 | 1.6325 | 1.61 × 10−9 |
| 0 | 0 | 0.05 | 1.1578 | 1.3886 | 1.4794 | 1.5348 |
| 0 | 0 | 0.1 | 1.1337 | 1.3045 | 1.3718 | 1.4129 |
| 0 | 1 | 0.01 | 1.08 × 10−10 | 2.41 × 10−8 | 6.28 × 10−9 | |
| 0 | 2 | 0.01 | 4.63 × 10−11 | 3.00 × 10−8 | 2.3164 | 1.77 × 10−8 |
| 0 | 2 | 0.05 | 2.2336 | 2.0354 | 1.9713 | 1.9313 |
| 0 | 2 | 0.1 | 1.9386 | 1.7878 | 1.7393 | 1.7092 |
| 1 | −2 | 0.01 | 0.06575 | 0.017388 | 0.007362 | |
| 1 | −2 | 0.05 | 4.2389 | 4.171 | 4.0994 | 4.0461 |
| 1 | −2 | 0.1 | 2.9869 | 2.9338 | 2.8778 | 2.8394 |
| 1 | −1 | 0.01 | 7.87 × 10−10 | 1.64 × 10−9 | 1.80 × 10−5 | 4.7810−9 |
| 1 | −1 | 0.05 | 4.135 | 4.0399 | 4.1454 | 4.2389 |
| 1 | −1 | 0.1 | 2.9062 | 2.8319 | 2.9153 | 2.9878 |
| 1 | 0 | 0.01 | 2.96 × 10−6 | 6.5366 | 9.88 × 10−10 | 1.00 × 10−9 |
| 1 | 0 | 0.05 | 4.2855 | 4.3573 | 4.3974 | |
| 1 | 0 | 0.1 | 3.0238 | 3.0797 | 3.111 | |
| 1 | 2 | 0.01 | 1.50 × 10−6 | 4.08 × 10−8 | 4.58 × 10−8 | 5.03 × 10−8 |
| 1 | 2 | 0.05 | 4.9559 | 4.7496 | 4.6937 | 4.6612 |
| 1 | 2 | 0.1 | 3.5506 | 3.3871 | 3.343 | 3.3175 |
| 0.4 | 2 | 0.7 | 0.01 | 10.001 |
| 0.4 | 2 | 1 | 0.01 | 10.001 |
| 0.7 | 1 | 0 | 0.01 | 10.001 |
| 0.7 | 1 | 0.4 | 0.01 | 10.001 |
| 0.7 | 1 | 0.7 | 0.01 | 10.001 |
| 0.7 | 1 | 1 | 0.01 | 10.001 |
| 0.7 | 2 | 0 | 0.01 | 10.001 |
| 0.7 | 2 | 0.7 | 0.01 | 10.001 |
| 0.7 | 2 | 1 | 0.01 | 10.001 |
| 1 | 1 | 0 | 0.01 | 10.001 |
| 1 | 1 | 0.4 | 0.01 | 10.001 |
| 1 | 1 | 0.7 | 0.01 | 10.001 |
| 1 | 0 | 0 | 0.01 | 9.9998 |
| 0.7 | 0 | 0 | 0.01 | 9.9997 |
| 0.7 | 0 | 0.4 | 0.01 | 9.9996 |
| 0.7 | 0 | 0.7 | 0.01 | 9.9996 |
| 1 | 0 | 0.7 | 0.01 | 9.9996 |
| 1 | 0 | 1 | 0.01 | 9.9996 |
| 0.4 | 0 | 0.4 | 0.01 | 9.9995 |
| 0.4 | 0 | 0.7 | 0.01 | 9.9995 |
| 0.4 | 0 | 1 | 0.01 | 9.9995 |
| 0.7 | 0 | 1 | 0.01 | 9.9995 |
| 0 | 0 | 0 | 0.01 | 9.9992 |
| 0 | 0 | 0.4 | 0.01 | 9.9992 |
| 0 | 0 | 1 | 0.01 | 9.9992 |
| 0 | −1 | 1 | 0.01 | 9.9921 |
| 0 | −1 | 0.7 | 0.01 | 9.9917 |
| 0 | −1 | 0.4 | 0.01 | 9.9912 |
| 0.7 | −1 | 0 | 0.01 | 9.991 |
| 0.4 | −1 | 0.4 | 0.01 | 9.9908 |
| 0.4 | −1 | 0.7 | 0.01 | 9.9905 |
| 0 | −1 | 0 | 0.01 | 9.9904 |
| 1 | −1 | 0 | 0.01 | 9.9894 |
| 0.7 | −1 | 0.4 | 0.01 | 9.989 |
| 0.7 | −1 | 1 | 0.01 | 9.9837 |
| 1 | −1 | 0.4 | 0.01 | 9.9819 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alharbi, A.A.; Alzahrani, A.R.R. A COMSOL-Based Numerical Simulation of Heat Transfer in a Hybrid Nanofluid Flow at the Stagnant Point across a Stretching/Shrinking Sheet: Implementation for Understanding and Improving Solar Systems. Mathematics 2024, 12, 2493. https://doi.org/10.3390/math12162493
Alharbi AA, Alzahrani ARR. A COMSOL-Based Numerical Simulation of Heat Transfer in a Hybrid Nanofluid Flow at the Stagnant Point across a Stretching/Shrinking Sheet: Implementation for Understanding and Improving Solar Systems. Mathematics. 2024; 12(16):2493. https://doi.org/10.3390/math12162493
Chicago/Turabian StyleAlharbi, Ahmad Ayyad, and Ali Rashash R. Alzahrani. 2024. "A COMSOL-Based Numerical Simulation of Heat Transfer in a Hybrid Nanofluid Flow at the Stagnant Point across a Stretching/Shrinking Sheet: Implementation for Understanding and Improving Solar Systems" Mathematics 12, no. 16: 2493. https://doi.org/10.3390/math12162493
APA StyleAlharbi, A. A., & Alzahrani, A. R. R. (2024). A COMSOL-Based Numerical Simulation of Heat Transfer in a Hybrid Nanofluid Flow at the Stagnant Point across a Stretching/Shrinking Sheet: Implementation for Understanding and Improving Solar Systems. Mathematics, 12(16), 2493. https://doi.org/10.3390/math12162493






