Cost Evaluation for Capacity Planning Based on Patients’ Pathways via Semi-Markov Reward Modelling
Abstract
1. Introduction
2. Methods
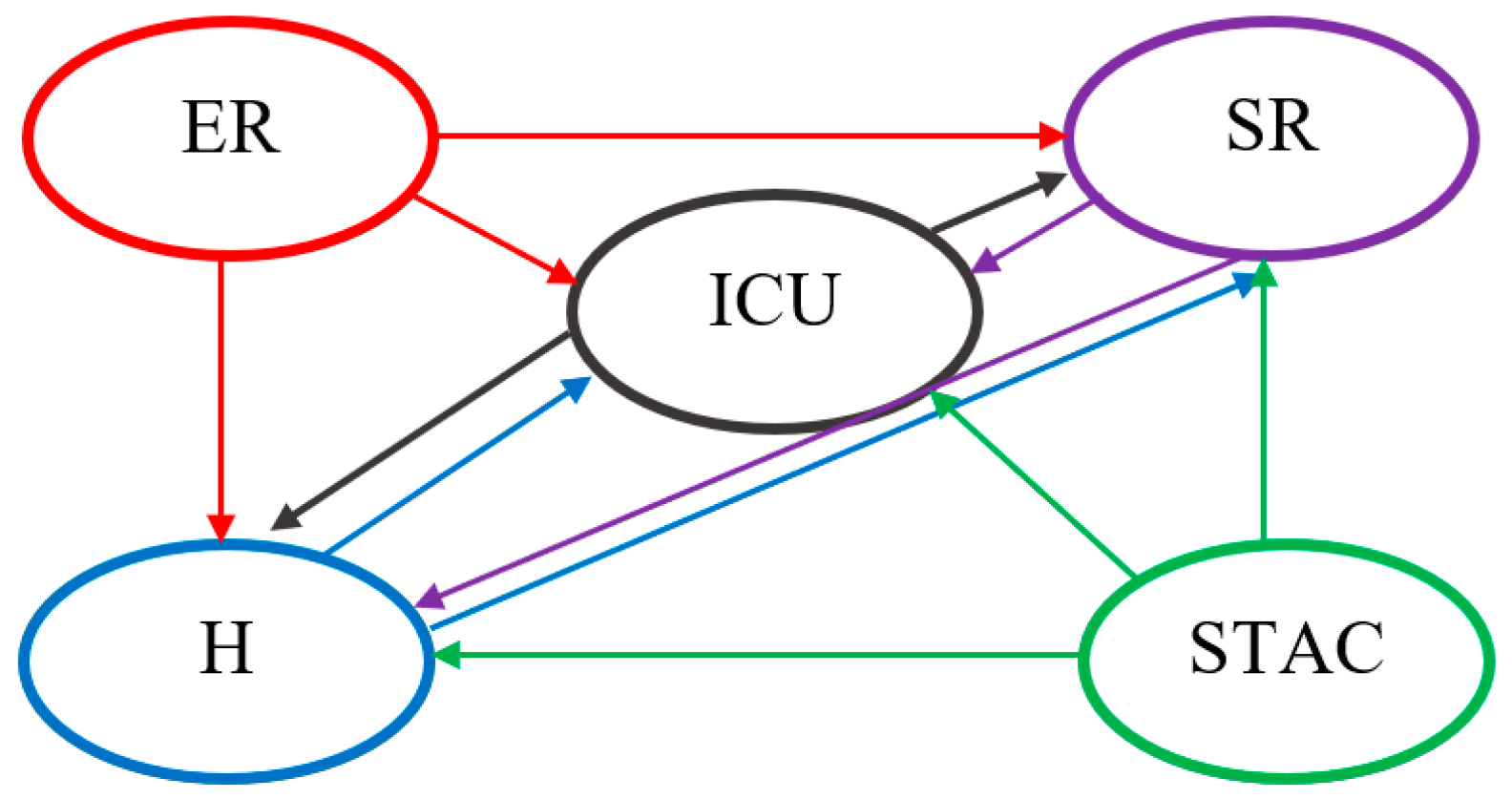
2.1. Population Structure
2.2. States’ Inflows
2.3. States’ Current Availability
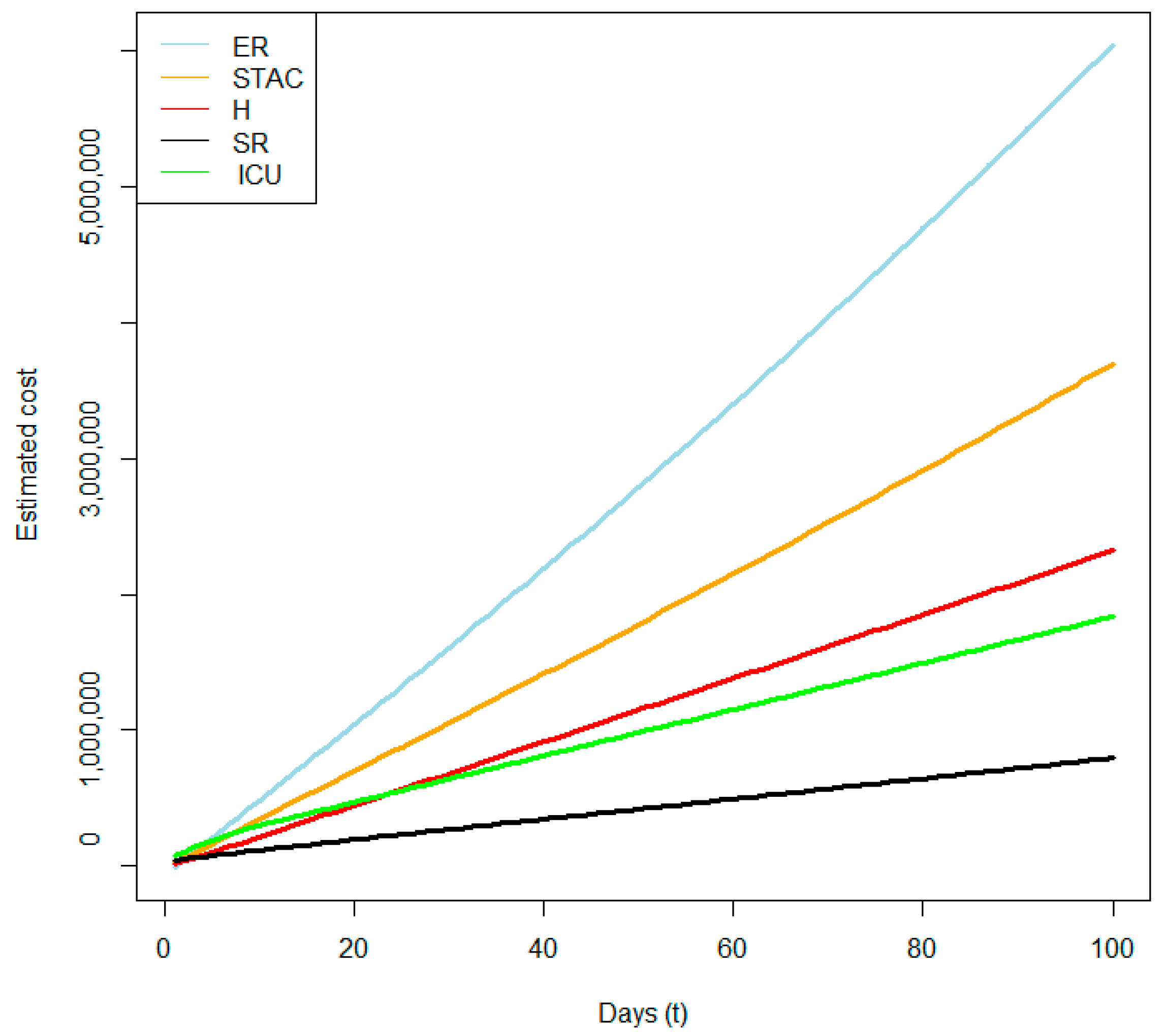
2.4. Attachment of Costs
3. Illustration
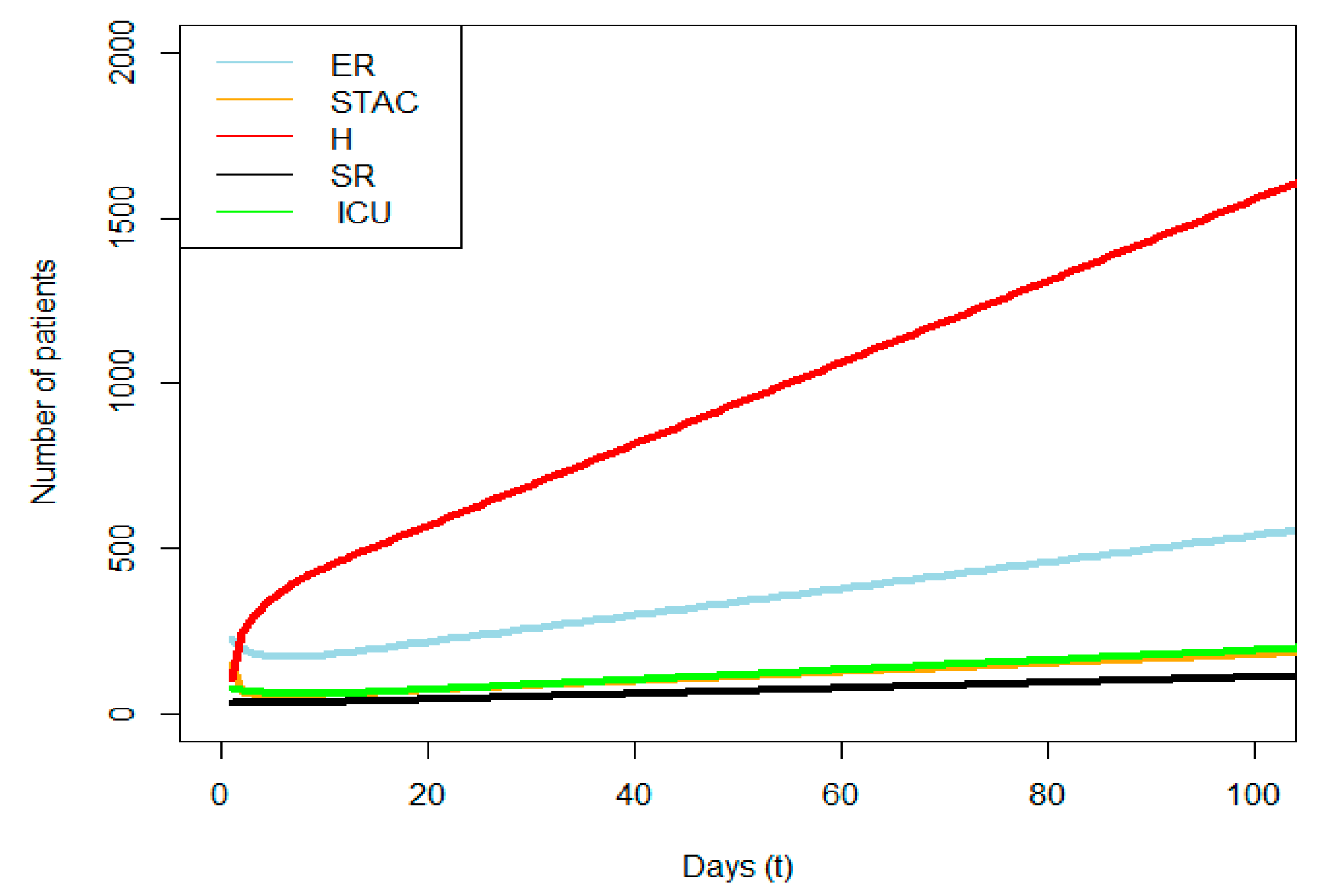
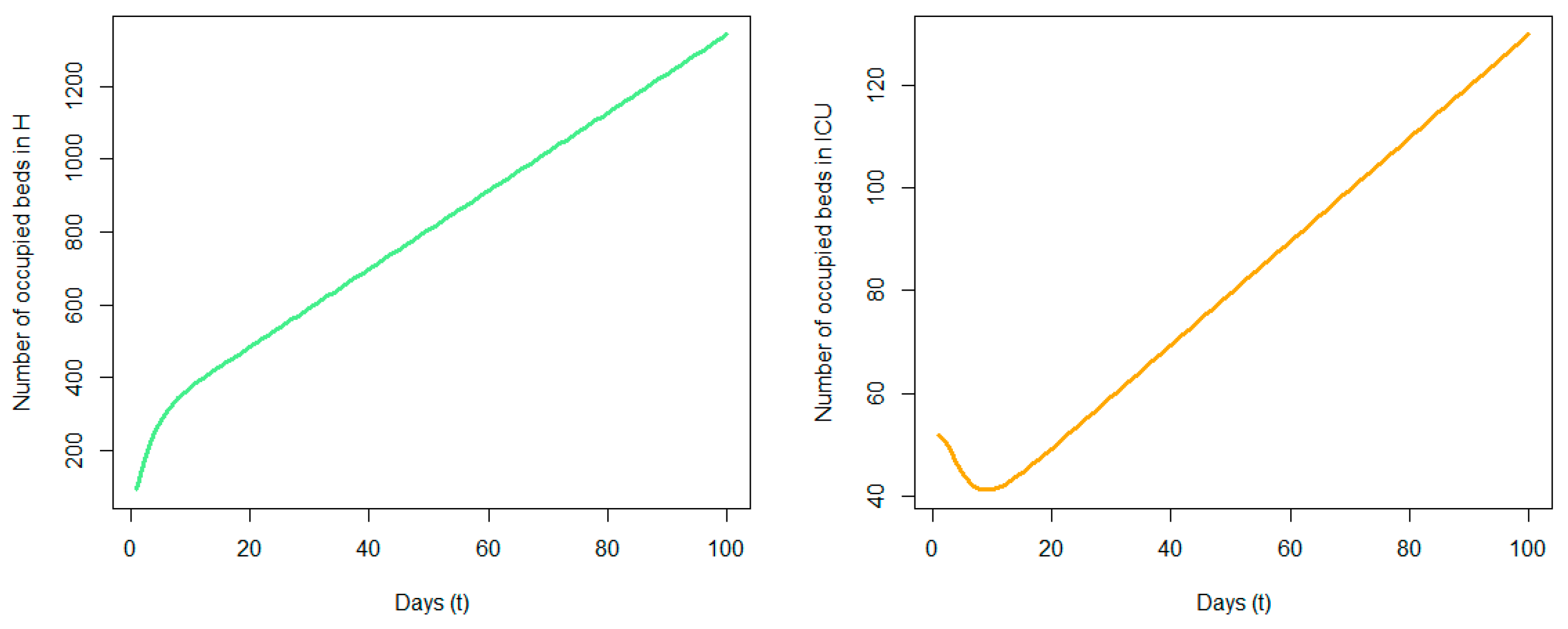
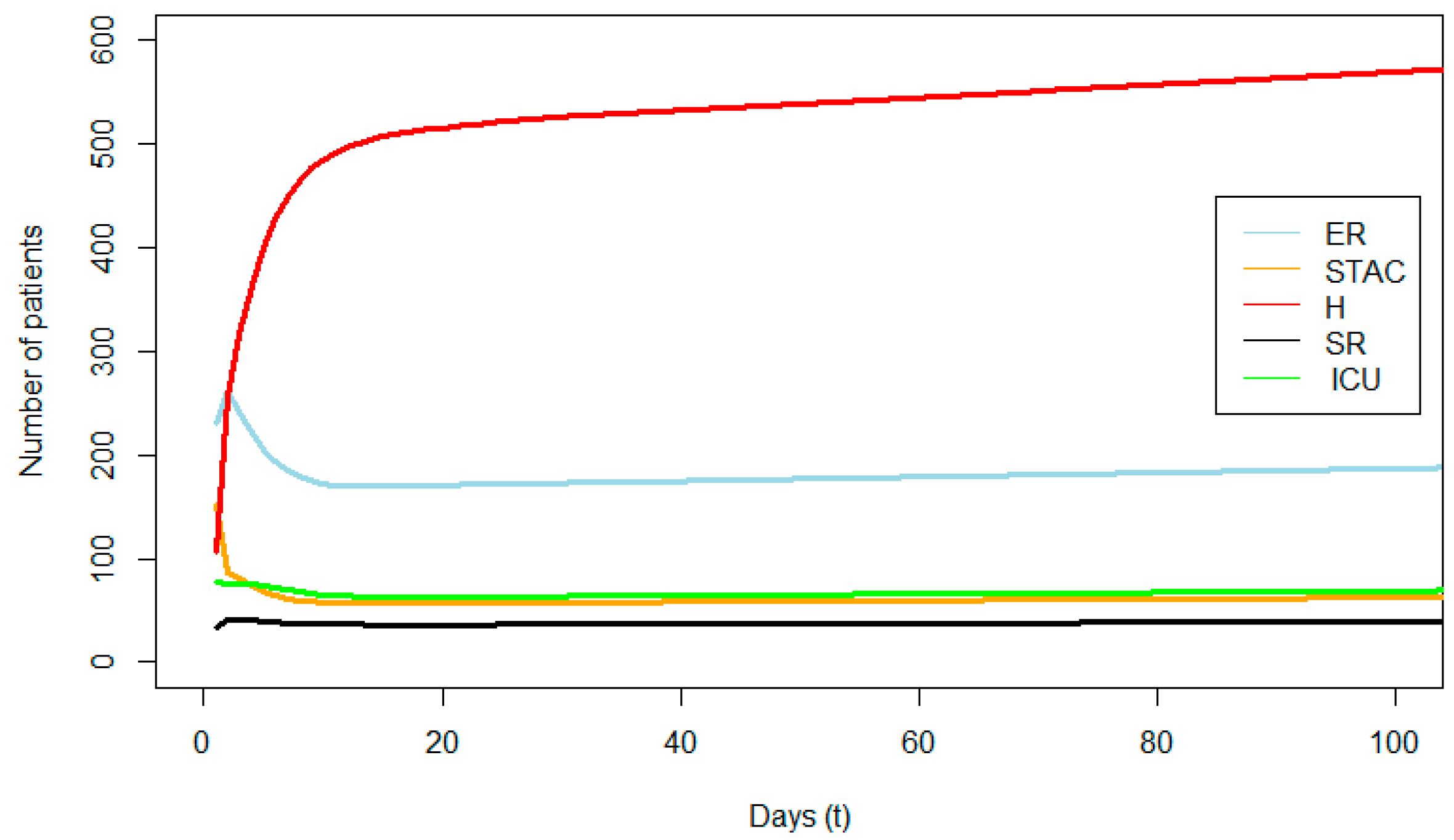
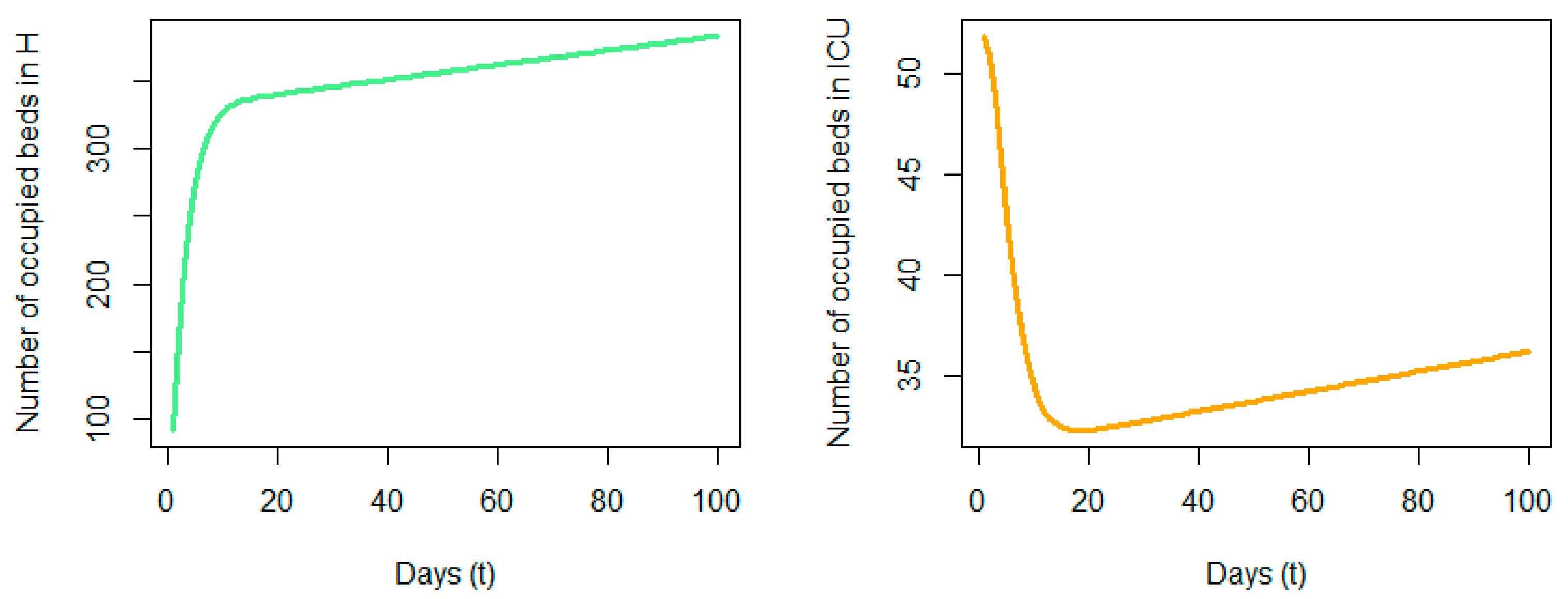
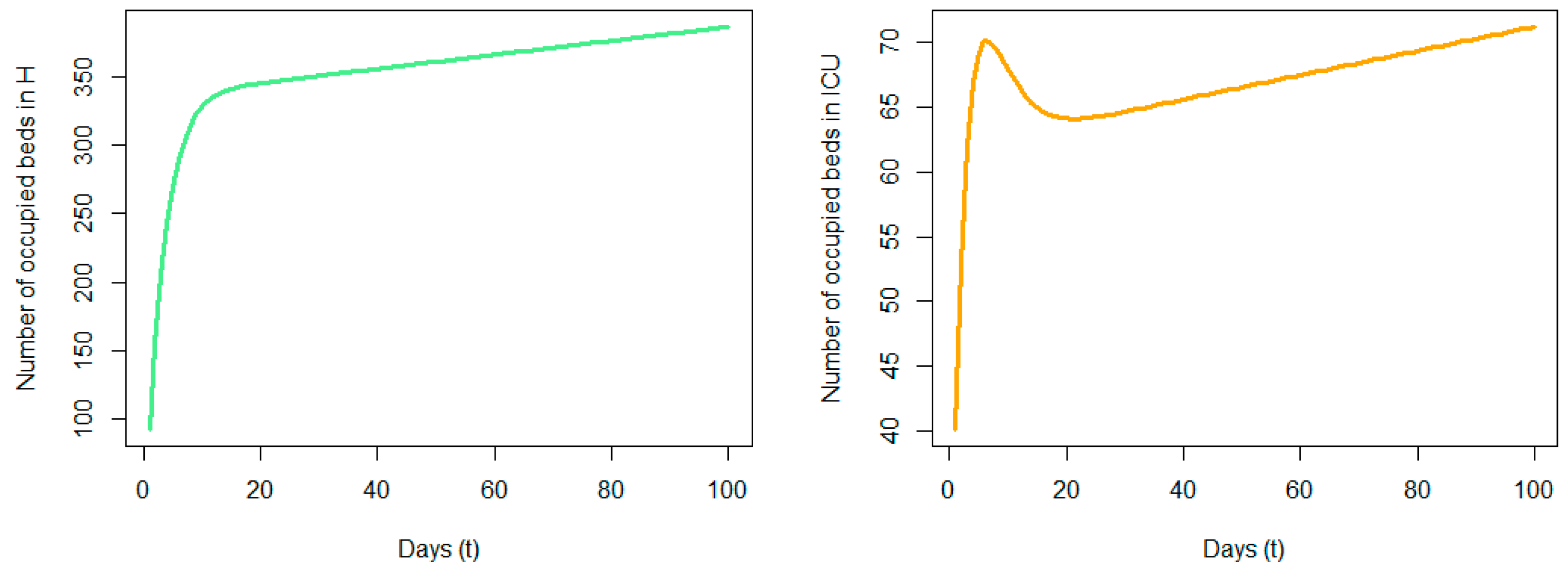
3.1. Scenario 1
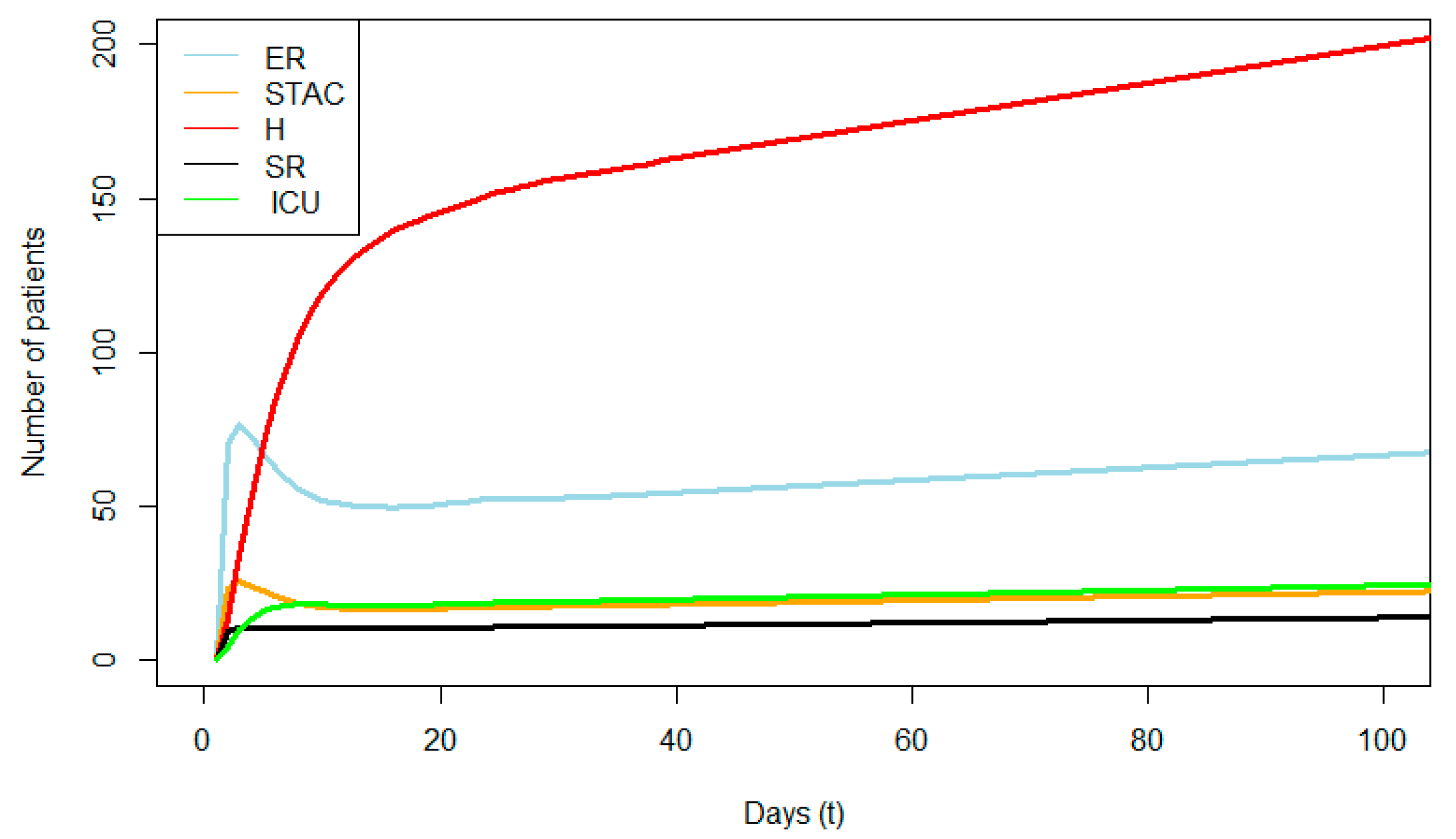
3.2. Scenario 2
3.3. Scenario 3
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Howard, R.A. Dynamic Probabilistic Systems: Semi-Markov and Decision Processes; Dover Publications: Mineola, NY, USA, 2007; Volume 2. [Google Scholar]
- Vassiliou, P.-C.G.; Papadopoulou, A. Non-homogeneous Semi-Markov Systems and Maintainability of the State Sizes. J. Appl. Probab. 1992, 29, 519–534. [Google Scholar] [CrossRef]
- Bartholomew, D.J.; Andrew, F.F.; Sally, I.M. Statistical Techniques for Manpower Planning; Wiley: Hoboken, NJ, USA, 1979. [Google Scholar]
- Papadopoulou, A.A. Economic Rewards in Non-Homogeneous Semi-Markov Systems. Commun. Stat. Theory Methods 2004, 33, 681696. [Google Scholar] [CrossRef]
- Vassiliou, P.-C.G. Non-Homogeneous Markov Chains and Systems Theory and Applications; Taylor and Francis Group: Milton Park, UK, 2022. [Google Scholar]
- McClean, S.I. A Semi-Markov Model for a Multigrade Population with Poisson Recruitment. J. Appl. Probab. 1980, 17, 846–852. [Google Scholar] [CrossRef]
- McClean, S.I. Semi-Markov Models for Manpower Planning. In Semi-Markov Models; Springer: Berlin/Heidelberg, Germany, 1986; pp. 283–300. [Google Scholar]
- D’Amico, G.; Di Biase, G.; Janssen, J.; Manca, R. Semi-Markov Migration Models for Credit Risk; Wiley Online Library: Hoboken, NJ, USA, 2017. [Google Scholar]
- Vassiliou, P.-C.G. Non-Homogeneous Semi-Markov and Markov Renewal Processes and Change of Measure in Credit Risk. Mathematics 2021, 9, 55. [Google Scholar] [CrossRef]
- Janssen, J.; Manca, R. Applied Semi-Markov Processes; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Janssen, J. Semi-Markov Models: Theory and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Schbath, S.; Prum, B.; de Turckheim, E. Exceptional Motifs in Different Markov Chain Models for a Statistical Analysis of DNA sequences. J. Comput. Biol. 1995, 2, 417–437. [Google Scholar] [CrossRef] [PubMed]
- De Dominicis, R.; Manca, R. Some New Results on the Transient Behaviour of Semi-Markov Reward Processes. Methods Oper. Res. 1986, 53, 387–397. [Google Scholar]
- Vasileiou, A.; Vassiliou, P.-C.G. An Inhomogeneous Semi-Markov Model for the Term Structure of Credit Risk Spreads. Adv. Appl. Probab. 2006, 38, 171–198. [Google Scholar] [CrossRef]
- Vassiliou, P.-C.G.; Vasileiou, A. Asymptotic Behaviour of the Survival Probabilities in an Inhomogeneous Semi-Markov Model for the Migration Process in Credit Risk. Linear Algebra Appl. 2013, 438, 2880–2903. [Google Scholar] [CrossRef]
- Vassiliou, P.-C.G. Semi-Markov Migration Process in a Stochastic Market in Credit Risk. Linear Algebra Appl. 2014, 450, 13–43. [Google Scholar] [CrossRef]
- Gillespie, J.; McClean, S.; Scotney, B.; Garg, L.; Barton, M.; Fullerton, K. Costing Hospital Resources for Stroke Patients Using Phase-Type Models. Health Care Manag. Sci. 2011, 14, 279–291. [Google Scholar] [CrossRef]
- Katsaliaki, K.; Mustafee, N. Applications of Simulation within the Healthcare Context. J. Oper. Res. Soc. 2011, 62, 1431–1451. [Google Scholar] [CrossRef] [PubMed]
- McClean, S.; Barton, M.; Garg, L.; Fullerton, K. A Modeling Framework that Combines Markov Models and Discrete-Event Simulation for Stroke Patient Care. ACM Trans. Model. Comput. Simul. 2011, 21, 1–26. [Google Scholar] [CrossRef]
- Cooper, K.; Brailsford, S.C.; Davies, R. Choice of Modelling Technique for Evaluating Health Care Interventions. J. Oper. Res. Soc. 2007, 58, 168–176. [Google Scholar] [CrossRef]
- Marshall, A.H.; McClean, S.I. Conditional Phase-Type Distributions for Modelling Patient Length of Stay in Hospital. Int. Trans. Oper. Res. 2003, 10, 565–576. [Google Scholar] [CrossRef]
- McClean, S.; Garg, L.; Fullerton, K. Costing Mixed Coxian Phase-Type Systems with Poisson Arrivals. Commun. Stat. Theory Methods 2014, 43, 1437–1452. [Google Scholar] [CrossRef]
- Garg, L.; McClean, S.; Meenan, B.; Millard, P. Non-homogeneous Markov Models for Sequential Pattern Mining of Healthcare Data. IMA J. Manag. Math. 2008, 20, 327–344. [Google Scholar] [CrossRef]
- Papadopoulou, A.A.; Tsaklidis, G.; McClean, S.; Garg, L. On the Moments and the Distribution of the Cost of a Semi Markov Model for Healthcare Systems. Methodol. Comput. Appl. Probab. 2012, 14, 717–737. [Google Scholar] [CrossRef]
- McClean, S.; Millard, P. Using Markov Models to Manage High Occupancy Hospital Care. In Proceedings of the 3th International IEEE Conference Intelligent Systems, London, UK, 4–6 September 2006. [Google Scholar]
- McClean, S.; Garg, L.; Meeman, B.; Millard, P. Using Markov Models to Find Interesting Patient Pathways. In Proceedings of the Computer Based Medical Systems Annual IEEE Symposium, Maribor, Slovenia, 20–22 June 2007; pp. 713–718. [Google Scholar]
- McClean, S.I.; McAlea, B.; Millard, P.H. Using a Markov Reward model to Estimate Spend-Down Costs for a Geriatric Department. J. Oper. Res. Soc. 1998, 49, 1021–1025. [Google Scholar] [CrossRef]
- Taylor, G.I.; McClean, S.I.; Millard, P.H. Stochastic Models of Geriatric Patient Bed Occupancy Behavior. J. R Statist. Soc. A 1999, 163, 39–48. [Google Scholar] [CrossRef]
- Marshall, A.H.; McClean, S.I.; Shapcott, C.M.; Millard, P.M. Modelling Patient Duration of Stay to Facilitate Resource Management of Geriatric Hospitals. Health Care Manag. Sci. 2002, 5, 313–319. [Google Scholar] [CrossRef]
- McClean, S.; Gillespie, T.; Scotney, B.; Fullerton, K. Using Phase Type Models to Cost a Cohort of Stroke Patients. Eur. J. Oper. Res. 2012, 236, 190–199. [Google Scholar] [CrossRef]
- Faddy, M.J.; McClean, S.I. Analyzing Data on Lengths of Stay of Hospital Patients Using Phase-type Distributions. Appl. Stoch. Models Bus. Ind. 1999, 15, 311–317. [Google Scholar] [CrossRef]
- Bartholomew, D.J. Stochastic Models for Social Processes, 3rd ed.; Wiley: Chichester, UK, 1982. [Google Scholar]
- Papadopoulou, A.A. Counting Transitions-Entrance Probabilities in Non-Homogeneous Semi-Markov Systems. Appl. Stochastic Models Data Anal. 1998, 13, 199–206. [Google Scholar] [CrossRef]


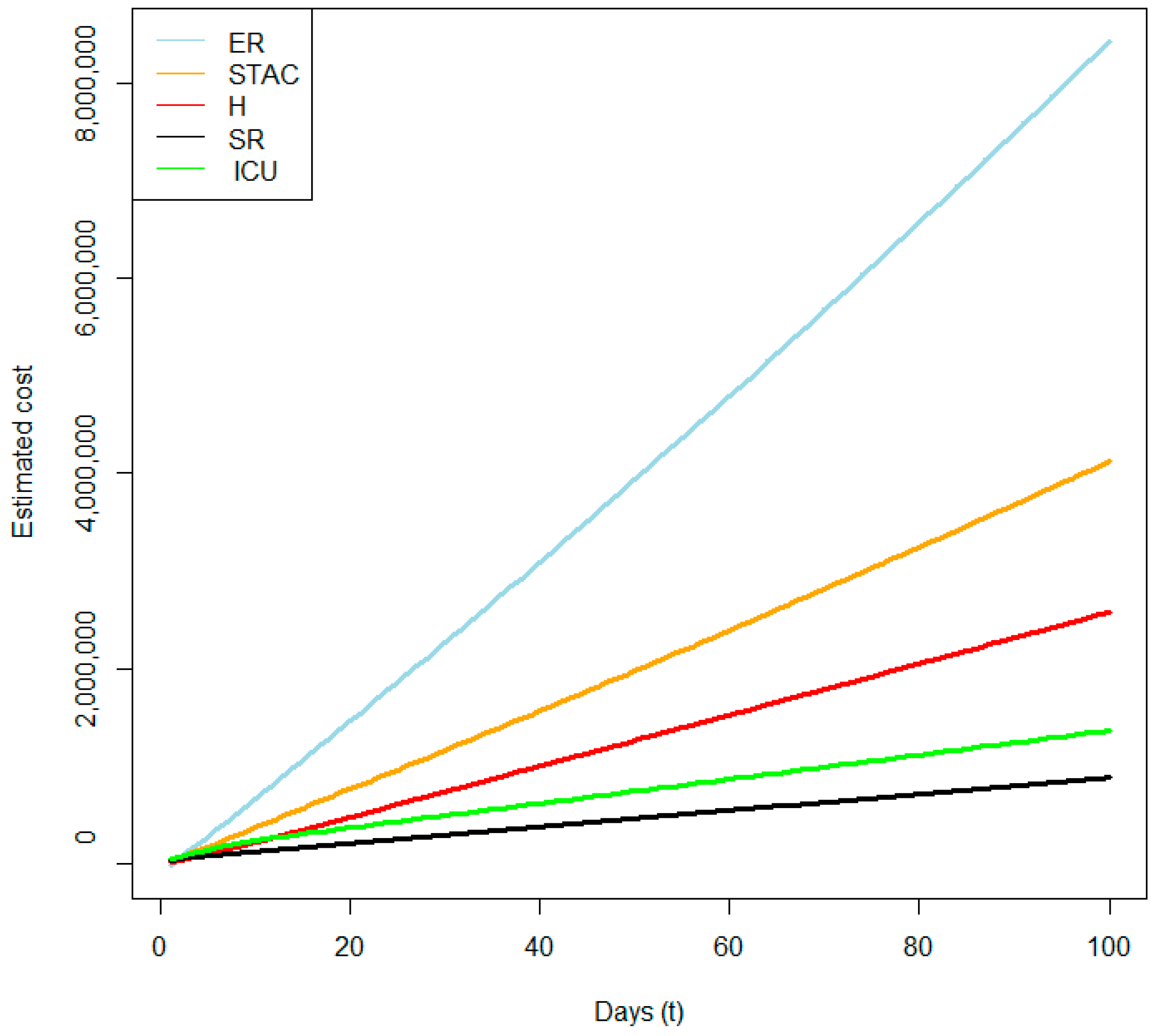
| Intercept | β (Slope) | |
|---|---|---|
| Emergency | 143.275 | 3.976 |
| Short-term acute care | 49.262 | 1.314 |
| Hospitalization | 312.6 | 12.4 |
| Surgery room | 27.6764 | 0.8401 |
| Intensive Care Unit | 47.142 | 1.465 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chatzimichail, C.; Kolias, P.; Papadopoulou, A. Cost Evaluation for Capacity Planning Based on Patients’ Pathways via Semi-Markov Reward Modelling. Mathematics 2024, 12, 1430. https://doi.org/10.3390/math12101430
Chatzimichail C, Kolias P, Papadopoulou A. Cost Evaluation for Capacity Planning Based on Patients’ Pathways via Semi-Markov Reward Modelling. Mathematics. 2024; 12(10):1430. https://doi.org/10.3390/math12101430
Chicago/Turabian StyleChatzimichail, Christina, Pavlos Kolias, and Alexandra Papadopoulou. 2024. "Cost Evaluation for Capacity Planning Based on Patients’ Pathways via Semi-Markov Reward Modelling" Mathematics 12, no. 10: 1430. https://doi.org/10.3390/math12101430
APA StyleChatzimichail, C., Kolias, P., & Papadopoulou, A. (2024). Cost Evaluation for Capacity Planning Based on Patients’ Pathways via Semi-Markov Reward Modelling. Mathematics, 12(10), 1430. https://doi.org/10.3390/math12101430







