On Bending of Piezoelectrically Layered Perforated Nanobeams Embedded in an Elastic Foundation with Flexoelectricity
Abstract
1. Introduction
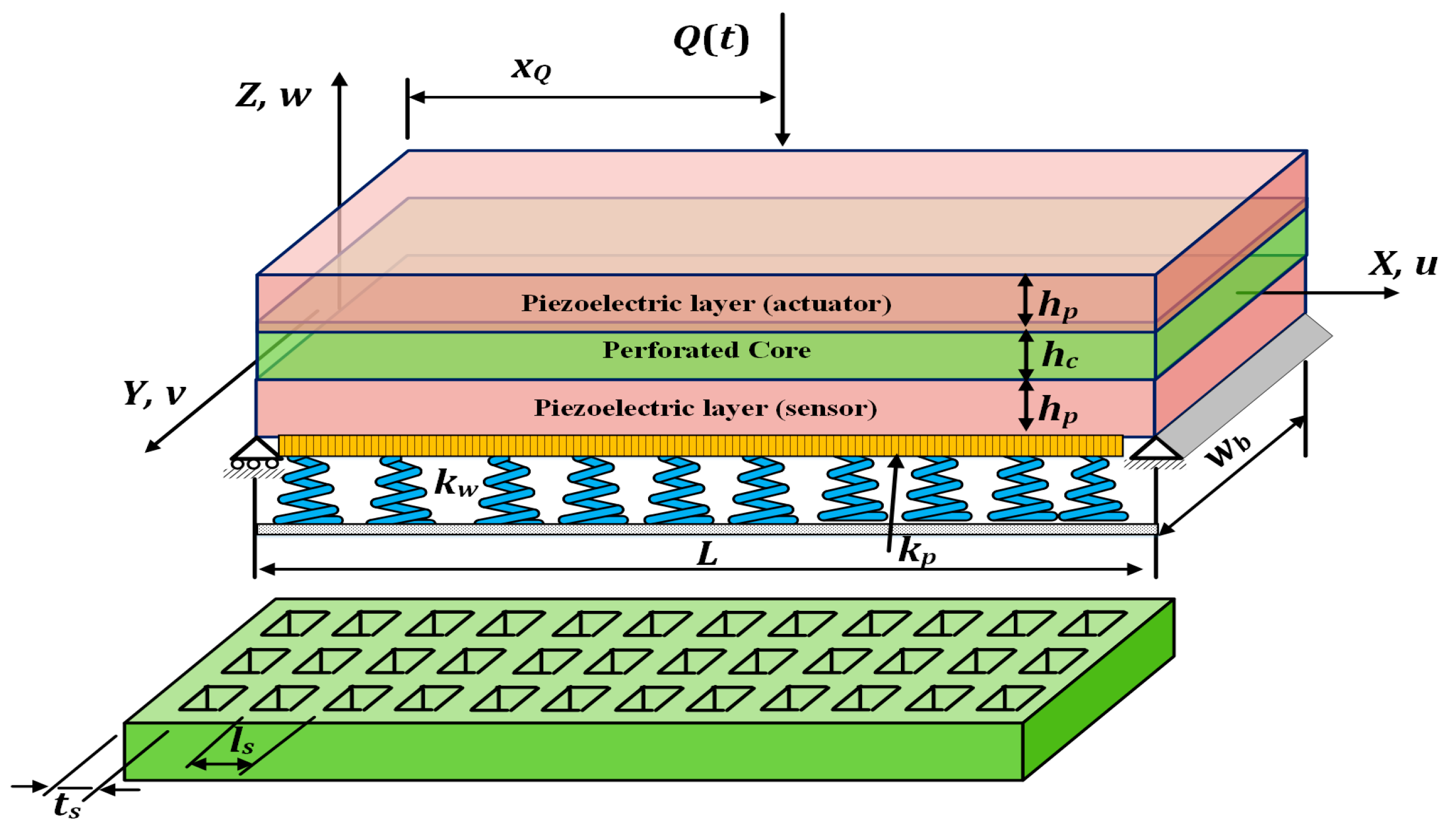
2. Mathematical Model
2.1. Equivalent Geometrical Parameters
2.2. Displacement and Basic Mechanical Relations
2.3. Electric Field and the Electromechanical Relations
2.4. The Modified Nonlocal Strain Gradient Theory with Flexoelectricity Effect
2.5. Energy Formulation and the Governing Equation
3. Analytical Solution Methodology
4. Numerical Results and Discussion
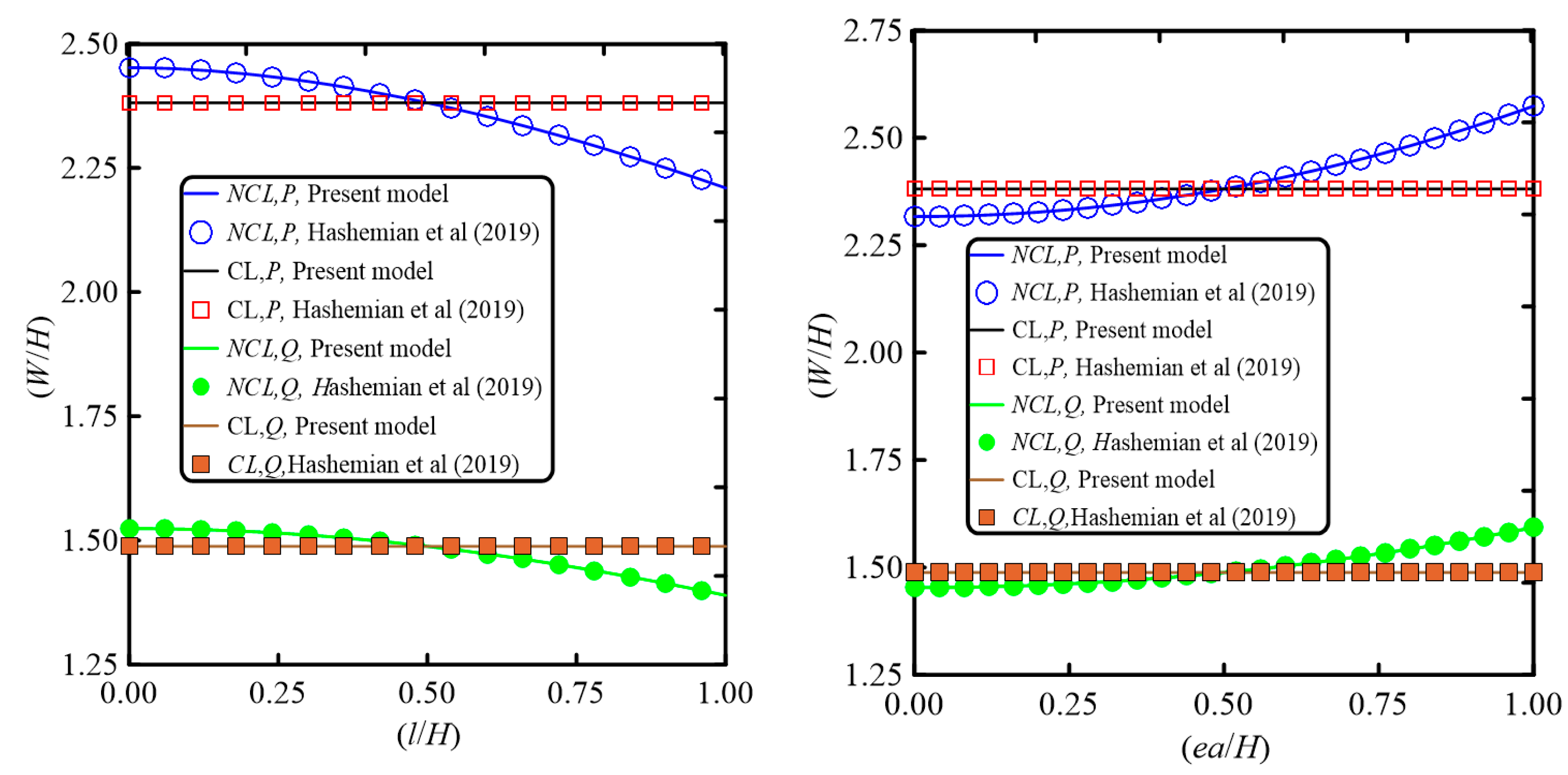
4.1. Verification of the Developed Methodology
4.2. Numerical Experiments and Discussion
5. Conclusions
- ✓
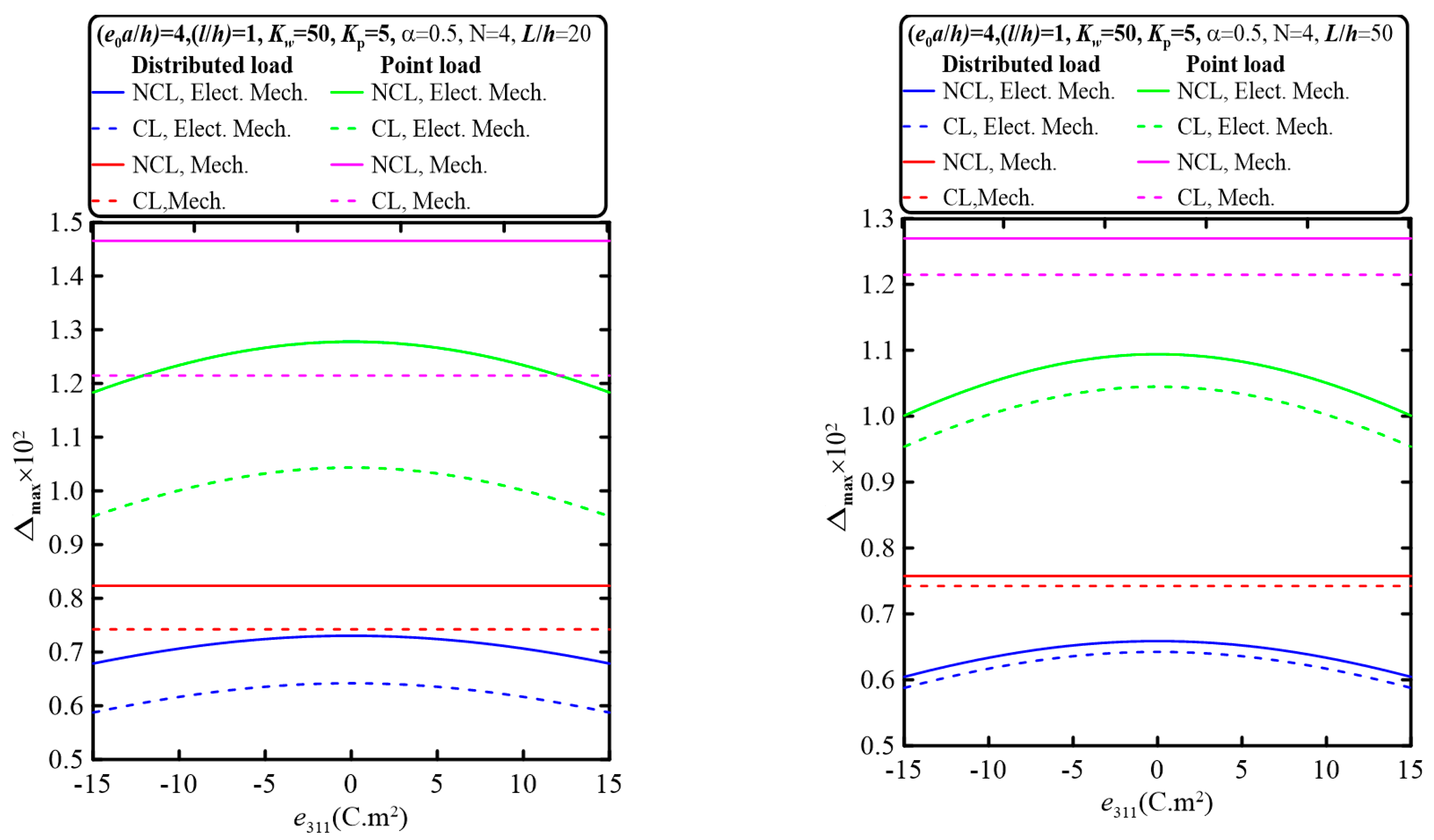
- The electromechanical bending is affected by the piezoelectric coefficient, e311. It decreases with the increase in the absolute value of the piezoelectric coefficient. Due to increasing the absolute value of the piezoelectric coefficient from 0 to 15, the relative percentage difference of the nondimensional maximum transverse deflection reaches about 8.7% for a point load and about 8.5% for a uniformly distributed load at a beam slenderness ratio of 50.
- ✓
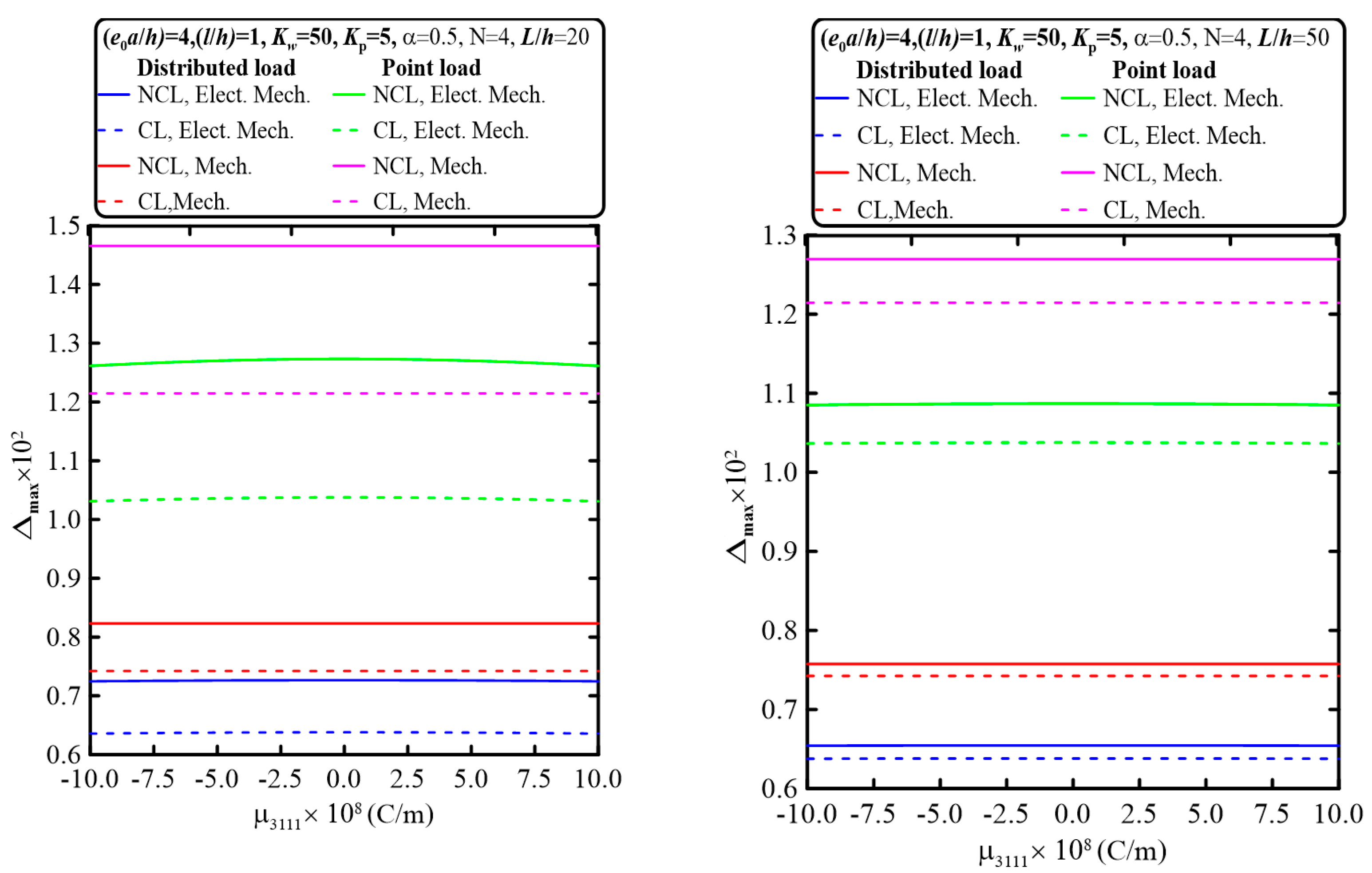
- On the contrary, the electromechanical bending behavior is slightly affected by the electric-field–strain-gradient coupling coefficient, μ3111; almost constant electromechanical bending behavior is obtained with increasing μ3111. With the increase in the absolute value of the electric-field–strain-gradient coupling coefficient, μ3111, from 0 to 10, the relative percentage difference of the nondimensional maximum transverse deflection reaches about 0.9274% for a point load and about 0.3654% for a uniformly distributed load.
- ✓
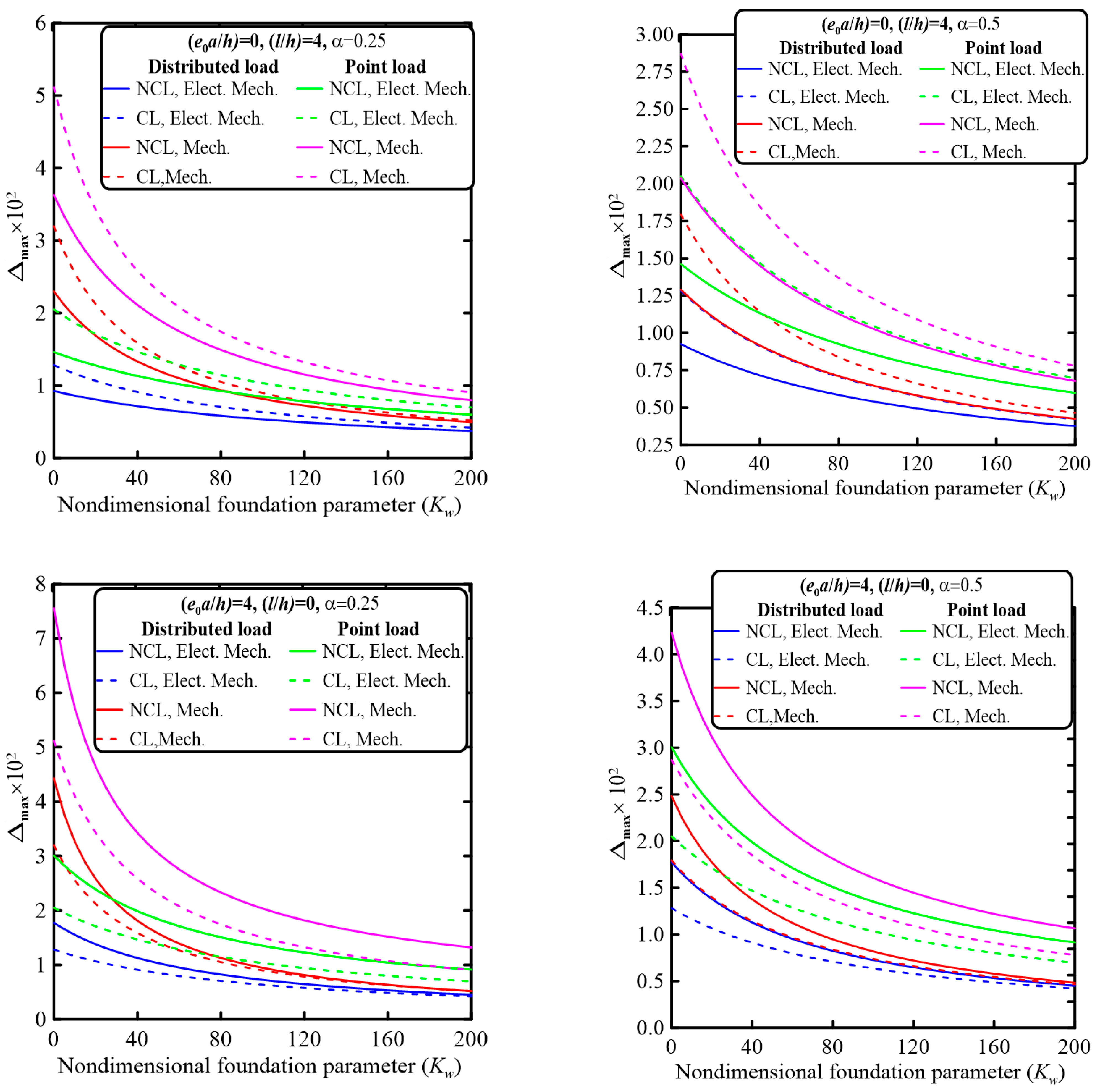
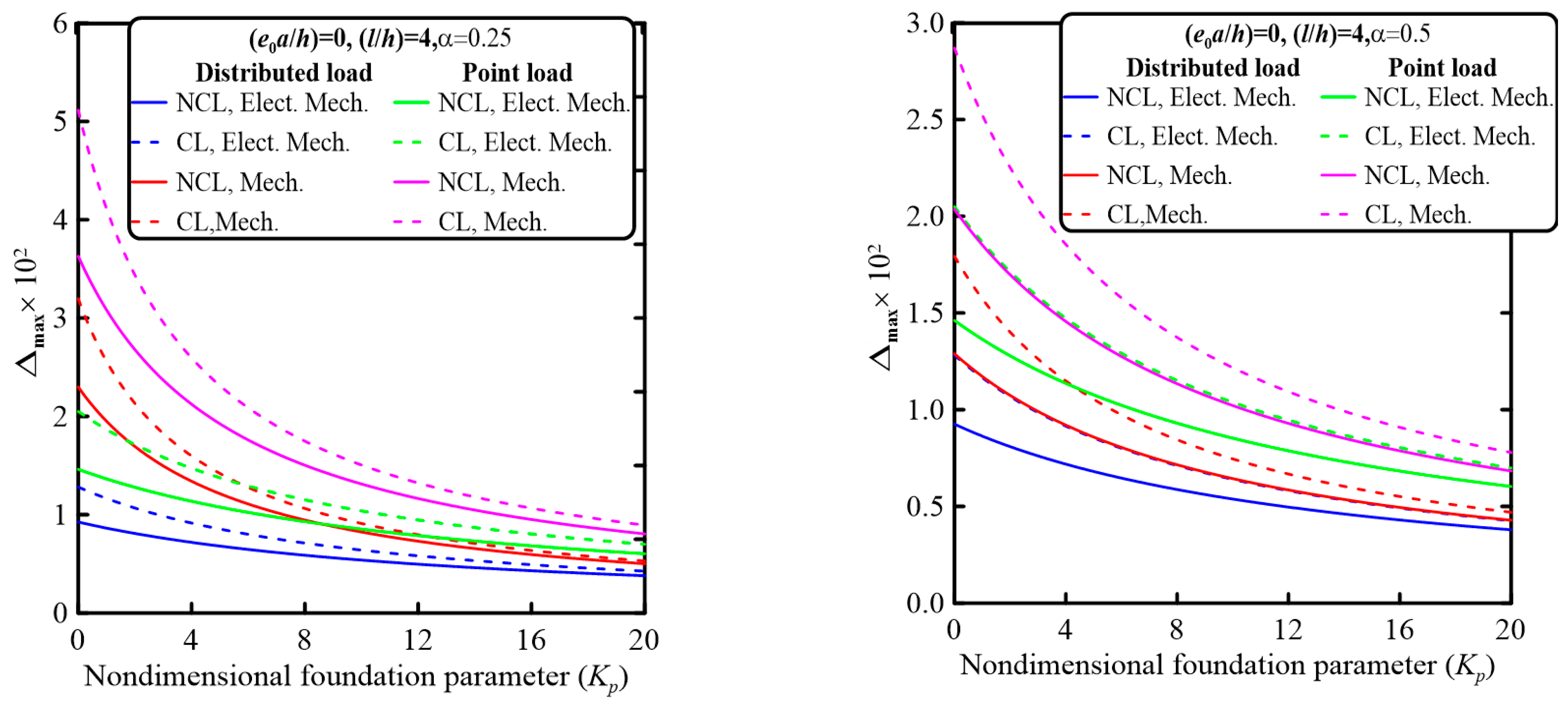
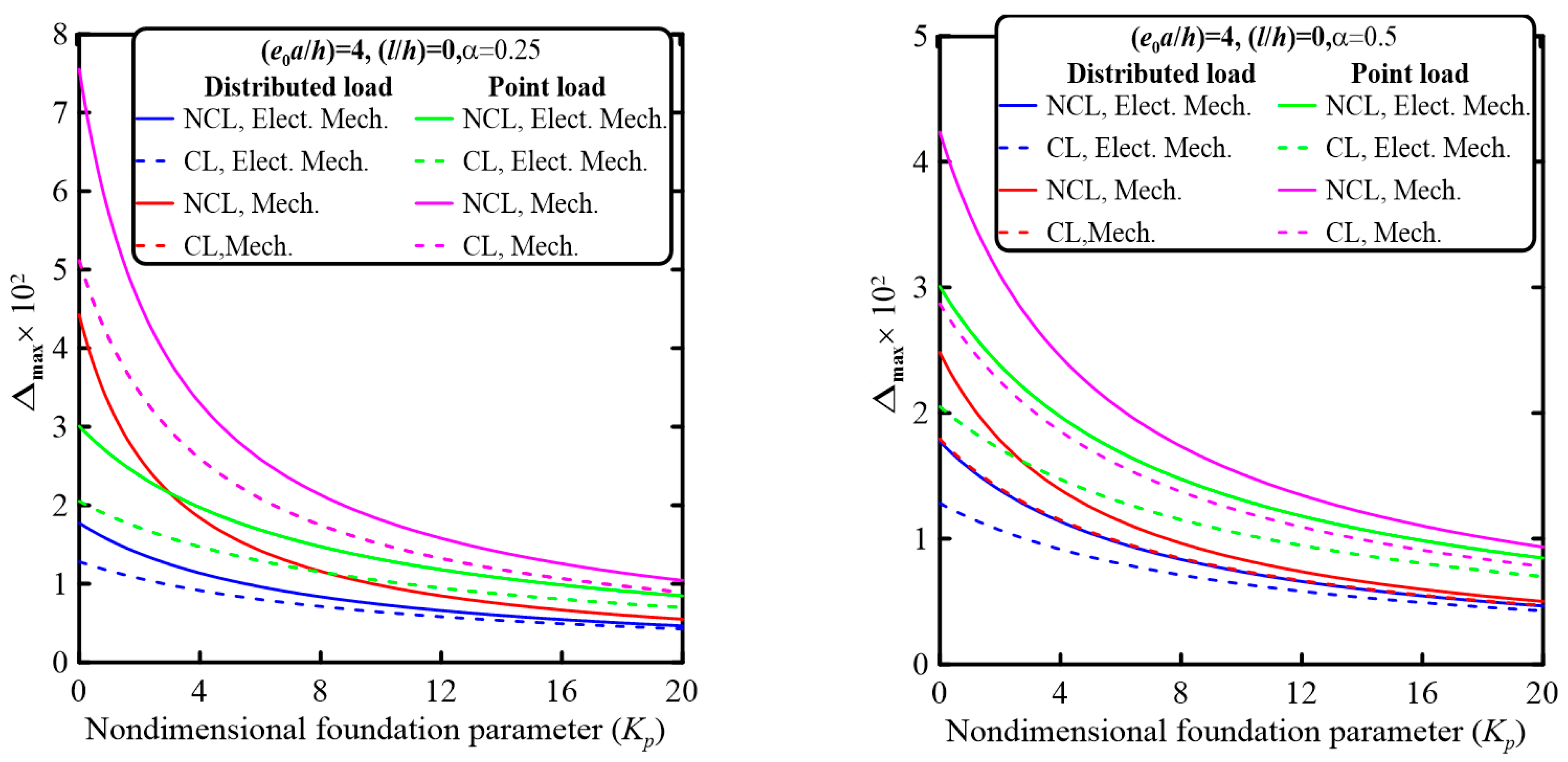
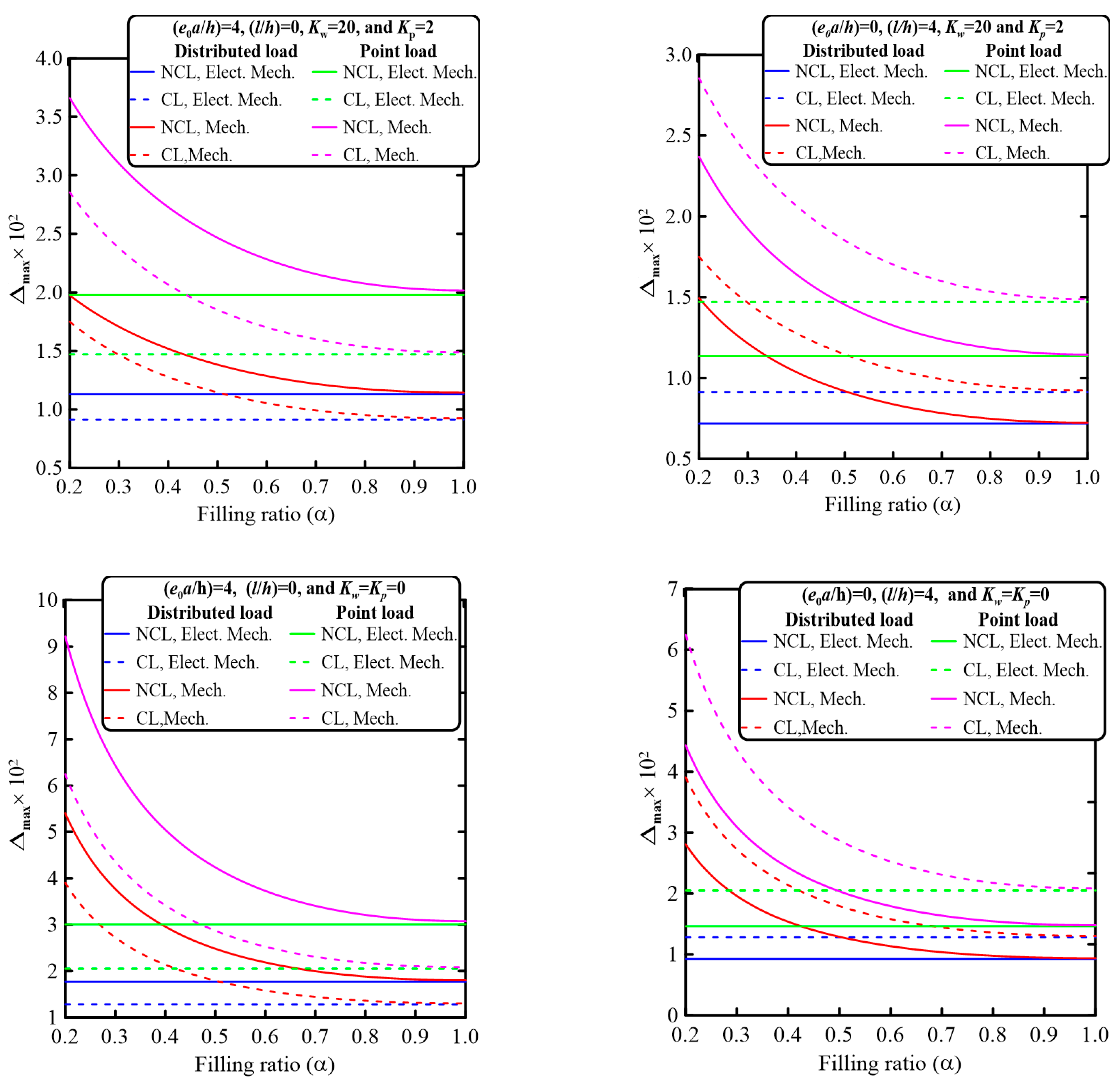
- The elastic foundation parameters have a significant effect on the electromechanical and mechanical bending behavior. The bending deflection is nonlinearly dependent on the elastic foundation parameters, Kw and Kp. The elastic foundation introduces a stiffening effect and, thus, smaller values of the bending deflection are produced with increasing these parameters. Increasing the nondimensional foundation parameter, Kw, from 0 to 200 produces a relative percentage difference in the maximum transverse deflection of 74.54% for the electromechanical behavior and 88.29% for the mechanical behavior at a perforation filling ratio of 0.25. Furthermore, increasing the nondimensional foundation parameter Kp from 0 to 20 results in a relative percentage difference of the maximum transverse deflection of 73.8254% for the electromechanical behavior and 87.584% for the mechanical behavior.
- ✓
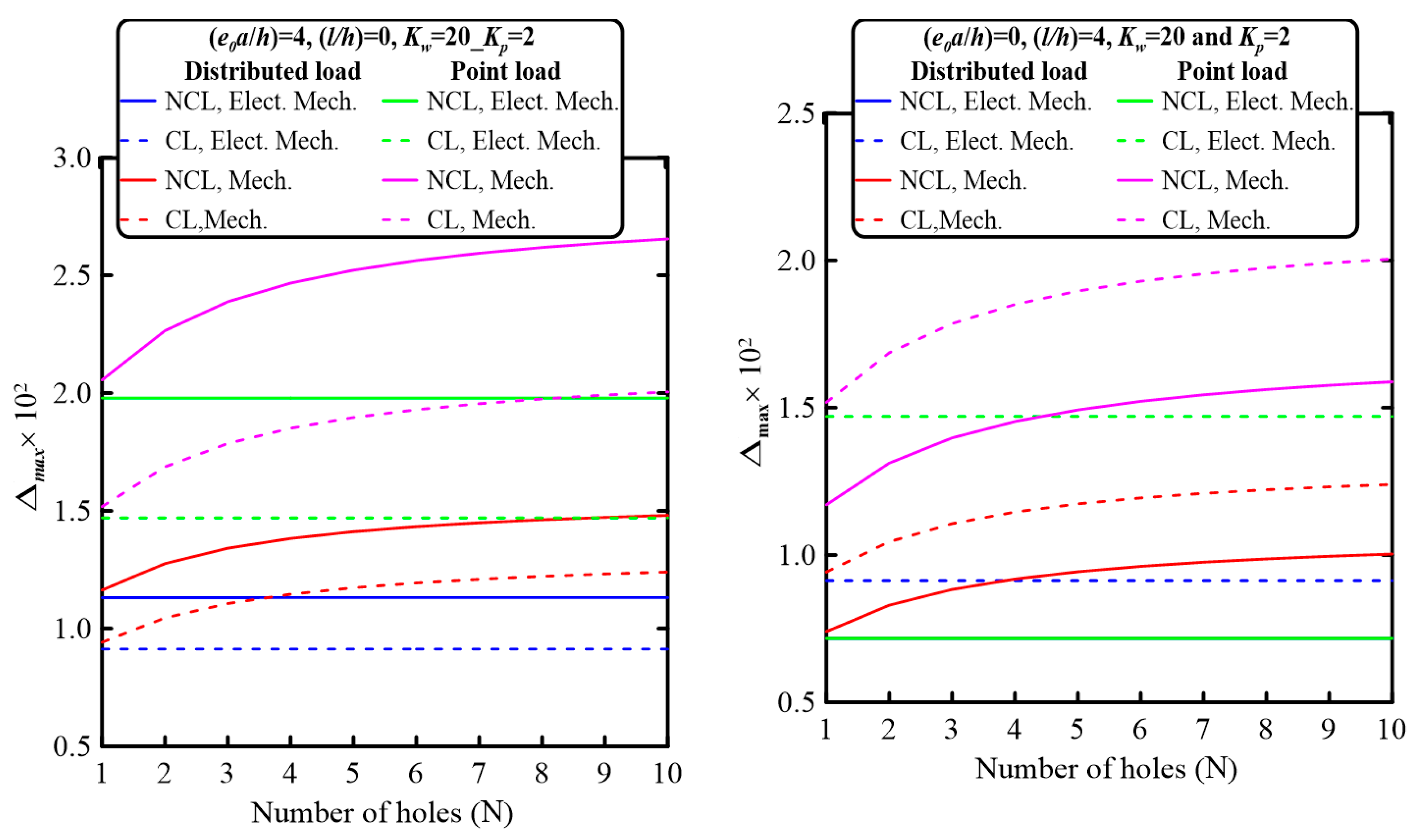
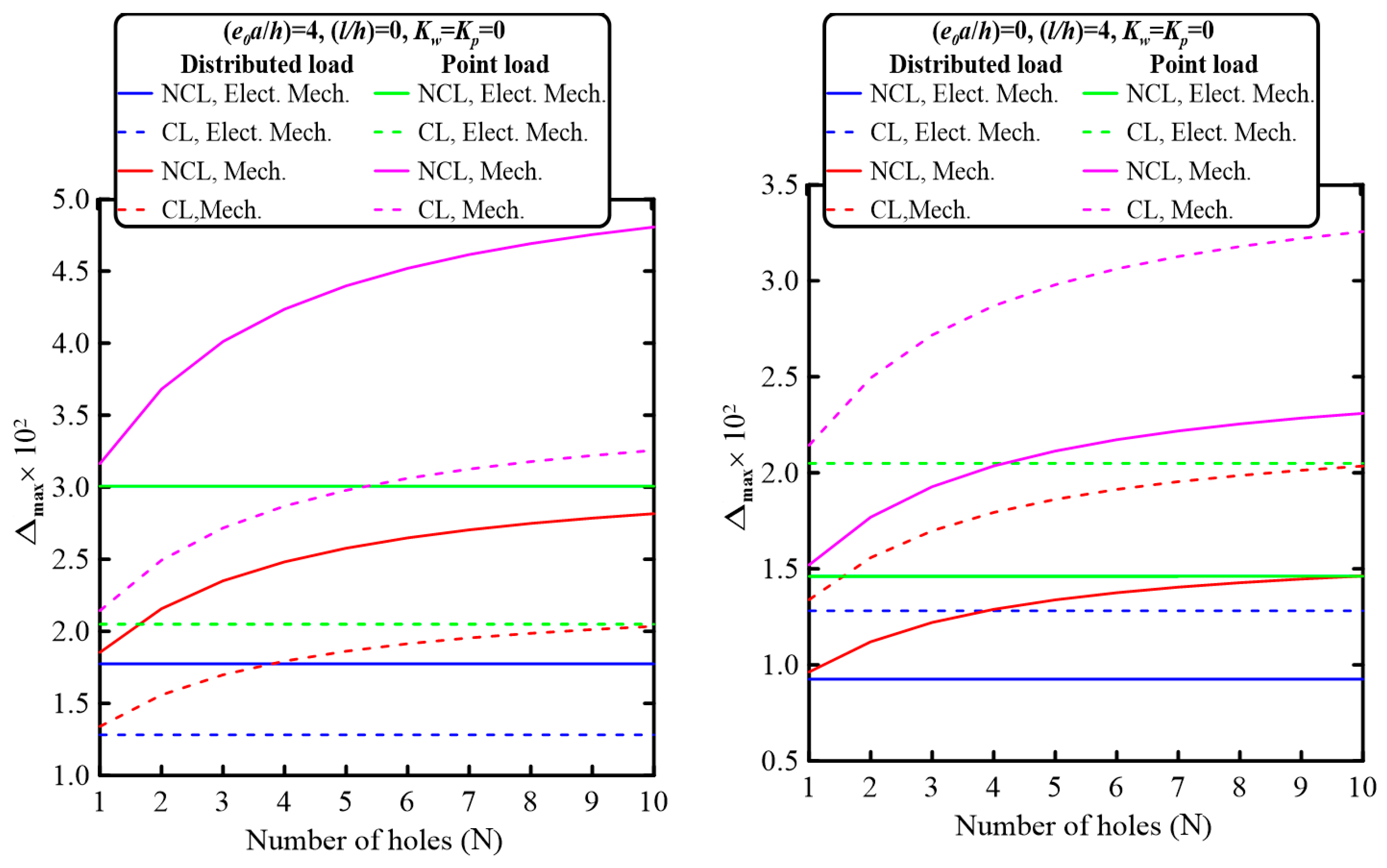
- The perforation configuration parameters greatly affect the mechanical bending behavior. Increasing the perforation filling ratio at a constant number of holes results in a decrease in the mechanical bending deflection. The relative percentage difference in the maximum mechanical transverse deflection reaches about 199.86% due to increasing the filling ratio from 0.2 to 1 without the elastic foundation, while this percentage difference is decreased to 106.94% for the nonclassical mechanical behavior and 91.83% for the classical mechanical behavior under the point load with elastic foundation parameters Kw = 20 and Kp = 2. Furthermore, increasing the number of holes increases the mechanical bending deflection. The relative percentage difference of the maximum transverse deflection reaches 34.1643% without the elastic foundation, while this difference is decreased to 24.28% with elastic foundation constants Kw = 20 and Kp = 2.
- ✓
- Utilizing the modified nonlocal strain gradient provides system flexibility to control stiffening and softening effects. Incorporating the nonlocal effect produces a softening effect, thus increasing the system flexibility. On the other hand, introduction of the strain gradient effect results in a stiffening effect, which leads to smaller values of the bending deflection. Due to increasing the nondimensional strain gradient parameter (l/h) from 1 to 4, the relative percentage difference of the maximum transverse deflection reaches 41.132% for the electromechanical behavior and 37.6% for the mechanical behavior at a beam aspect ratio of 10. Further increasing the nondimensional nonlocality parameter (ea/h) from 1 to 4 produces a relative percentage difference of 53.97% for the electromechanical behavior and 46.17% for the mechanical behavior.
- ✓
- The applied loading pattern significantly affects the bending performance; larger values of the transverse bending deflection are detected with the point load compared with that obtained by the corresponding uniformly distributed loading patterns.
6. Future Work Recommendations
- ✓
- The proposed methodology could be extended to include more nonclassical phenomena such as surface stress effects.
- ✓
- To increase the flexibility of the developed procedure and improve the mechanical performance, a viscoelastic effect could be included in the bulk material as well as the elastic foundation.
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, W.; Li, M.; Jin, F.; He, T.; Ma, Y. Nonlinear magnetic-mechanical-thermo-electric coupling characteristic analysis on the coupled extensional and flexural vibration of flexoelectric energy nanoharvester with surface effect. Compos. Struct. 2023, 308, 116687. [Google Scholar] [CrossRef]
- Shi, Y.; Li, N.; Wang, Y.; Ye, J. An analytical model for nonlinear magnetoelectric effect in laminated composites. Compos. Struct. 2021, 263, 113652. [Google Scholar] [CrossRef]
- Deng, F.; Yu, W.; Liang, X.; Shen, S. A Mixed Finite Element Method for Large Deformation of Flexoelectric Materials. Appl. Math. Model. 2023, 118, 303–321. [Google Scholar] [CrossRef]
- Baroudi, S.; Najar, F.; Jemai, A. Static and dynamic analytical coupled field analysis of piezoelectric flexoelectric nanobeams: A strain gradient theory approach. Int. J. Solids Struct. 2018, 135, 110–124. [Google Scholar] [CrossRef]
- Sedighi, H.M. Size-dependent dynamic pull-in instability of vibrating electrically actuated microbeams based on the strain gradient elasticity theory. Acta Astronaut. 2014, 95, 111–123. [Google Scholar] [CrossRef]
- Kheibari, F.; Beni, Y.T. Size dependent electro-mechanical vibration of single-walled piezoelectric nanotubes using thin shell model. Mater. Des. 2017, 114, 572–583. [Google Scholar] [CrossRef]
- Kaghazian, A.; Hajnayeb, A.; Foruzande, H. Free vibration analysis of a piezoelectric nanobeam using nonlocal elasticity theory. Struct. Eng. Mech. 2017, 61, 617–624. [Google Scholar] [CrossRef]
- Moory-Shirbani, M.; Sedighi, H.M.; Ouakad, H.M.; Najar, F. Experimental and mathematical analysis of a piezoelectrically actuated multilayered imperfect microbeam subjected to applied electric potential. Compos. Struct. 2018, 184, 950–960. [Google Scholar] [CrossRef]
- Chen, Q.; Zheng, S.; Li, Z.; Zeng, C. Size-dependent free vibration analysis of functionally graded porous piezoelectric sandwich nanobeam reinforced with graphene platelets with consideration of flexoelectric effect. Smart Mater. Struct. 2021, 30, 035008. [Google Scholar] [CrossRef]
- Gupta, M.; Meguid, S.A.; Kundalwal, S.I. Synergistic effect of surface-flexoelectricity on electromechanical response of BN-based nanobeam. Int. J. Mech. Mater. Des. 2022, 18, 3–19. [Google Scholar] [CrossRef]
- Van Minh, P.; Van Ke, T. A Comprehensive Study on Mechanical Responses of Non-uniform Thickness Piezoelectric Nanoplates Taking into Account the Flexoelectric Effect. Arab. J. Sci. Eng. 2022, 1–26. [Google Scholar] [CrossRef]
- Qian, D.; Wang, J. Studies of a new-style resonator to control electro-mechanical coupling bandgap of a locally resonant piezoelectric/elastic phononic crystal double-layer nonlocal nanobeam. Appl. Math. Model. 2022, 102, 786–796. [Google Scholar] [CrossRef]
- Zhao, X.; Zheng, S.; Li, Z. Effects of porosity and flexoelectricity on static bending and free vibration of AFG piezoelectric nanobeams. Thin-Walled Struct. 2020, 151, 106754. [Google Scholar] [CrossRef]
- Malikan, M.; Eremeyev, V.A. On nonlinear bending study of a piezo-flexomagnetic nanobeam based on an analytical-numerical solution. Nanomaterials 2020, 10, 1762. [Google Scholar] [CrossRef] [PubMed]
- Malikan, M.; Eremeyev, V.A. On the dynamics of a visco–piezo–flexoelectric nanobeam. Symmetry 2020, 12, 643. [Google Scholar] [CrossRef]
- Tocci Monaco, G.; Fantuzzi, N.; Fabbrocino, F.; Luciano, R. Trigonometric solution for the bending analysis of magneto-electro-elastic strain gradient nonlocal nanoplates in hygro-thermal environment. Mathematics 2021, 9, 567. [Google Scholar] [CrossRef]
- Naderi, A.; Fakher, M.; Hosseini-Hashemi, S. On the local/nonlocal piezoelectric nanobeams: Vibration, buckling, and energy harvesting. Mech. Syst. Signal Process. 2021, 151, 107432. [Google Scholar] [CrossRef]
- Li, H.; Li, C.; Shen, J.; Yao, L. Vibration analysis of rotating functionally graded piezoelectric nanobeams based on the nonlocal elasticity theory. J. Vib. Eng. Technol. 2021, 9, 1155–1173. [Google Scholar] [CrossRef]
- Ren, Y.M.; Schiavone, P.; Qing, H. On well-posed integral nonlocal gradient piezoelectric models for static bending of functionally graded piezoelectric nanobeam. Eur. J. Mech.-A/Solids 2022, 96, 104735. [Google Scholar] [CrossRef]
- Li, L.; Ren, Y.; Jin, Q. Electro-mechanical vibration and stress field of piezoelectric nanobeam with symmetrical FGM core under the low-velocity impact. Eur. Phys. J. Plus 2022, 137, 751. [Google Scholar] [CrossRef]
- Ansari, R.; Faraji Oskouie, M.; Nesarhosseini, S.; Rouhi, H. Vibrations of piezoelectric nanobeams considering flexoelectricity influence: A numerical approach based on strain-driven nonlocal differential/integral models. J. Braz. Soc. Mech. Sci. Eng. 2022, 44, 57. [Google Scholar] [CrossRef]
- Alam, M.; Mishra, S.K. A boundary layer solution for the post-critical thermo-electro-mechanical stability of nonlocal-strain gradient Functionally Graded Piezoelectric cylindrical shells. Eur. J. Mech. A/Solids 2023, 97, 104836. [Google Scholar] [CrossRef]
- Boyina, K.; Piska, R. Wave propagation analysis in viscoelastic Timoshenko nanobeams under surface and magnetic field effects based on nonlocal strain gradient theory. Appl. Math. Comput. 2023, 439, 127580. [Google Scholar] [CrossRef]
- Lu, L.; Guo, X.; Zhao, J. Size-dependent vibration analysis of nanobeams based on the nonlocal strain gradient theory. Int. J. Eng. Sci. 2017, 116, 12–24. [Google Scholar] [CrossRef]
- She, G.L.; Yan, K.M.; Zhang, Y.L.; Liu, H.B.; Ren, Y.R. Wave propagation of functionally graded porous nanobeams based on non-local strain gradient theory. Eur. Phys. J. Plus 2018, 133, 368. [Google Scholar] [CrossRef]
- Hashemian, M.; Foroutan, S.; Toghraie, D. Comprehensive beam models for buckling and bending behavior of simple nanobeam based on nonlocal strain gradient theory and surface effects. Mech. Mater. 2019, 139, 103209. [Google Scholar] [CrossRef]
- Karami, B.; Janghorban, M.; Rabczuk, T. Dynamics of two-dimensional functionally graded tapered Timoshenko nanobeam in thermal environment using nonlocal strain gradient theory. Compos. Part B Eng. 2020, 182, 107622. [Google Scholar] [CrossRef]
- Ducottet, S.; El Baroudi, A. Small-scale effects on the radial vibration of an elastic nanosphere based on nonlocal strain gradient theory. Nanotechnology 2023, 34, 115704. [Google Scholar] [CrossRef]
- Hai, T.; Al-Masoudy, M.M.; Alsulamy, S.; El Ouni, M.H.; Ayvazyan, A.; Kumar, A. Size-dependent free vibration analysis of honeycomb sandwich microplates integrated with piezoelectric actuators based on the modified strain gradient theory. Compos. Struct. 2023, 305, 116555. [Google Scholar] [CrossRef]
- Vahidi-Moghaddam, A.; Rajaei, A.; Azadi Yazdi, E.; Eghtesad, M.; Necsulescu, D.S. Nonlinear forced vibrations of nonlocal strain gradient microbeams. Mech. Based Des. Struct. Mach. 2023, 51, 1035–1053. [Google Scholar] [CrossRef]
- Moradi-Dastjerdi, R.; Behdinan, K. Stress waves in a lightweight substrate plate actuated with piezoelectric layers under sinusoidal time-dependent pressures. Aerosp. Sci. Technol. 2023, 132, 108057. [Google Scholar] [CrossRef]
- Eghbali, M.; Hosseini, S.A.; Pourseifi, M. Free transverse vibrations analysis of size-dependent cracked piezoelectric nano-beam based on the strain gradient theory under mechanic-electro forces. Eng. Anal. Bound. Elem. 2022, 143, 606–612. [Google Scholar] [CrossRef]
- Yang, W.; Wang, S.; Kang, W.; Yu, T.; Li, Y. A unified high-order model for size-dependent vibration of nanobeam based on nonlocal strain/stress gradient elasticity with surface effect. Int. J. Eng. Sci. 2023, 182, 103785. [Google Scholar] [CrossRef]
- Gui, Y.; Wu, R. Buckling analysis of embedded thermo-magneto-electro-elastic nano cylindrical shell subjected to axial load with nonlocal strain gradient theory. Mech. Res. Commun. 2023, 128, 104043. [Google Scholar] [CrossRef]
- Zhang, F.; Bai, C.; Wang, J. Study on dynamic stability of magneto-electro-thermo-elastic cylindrical nanoshells resting on Winkler–Pasternak elastic foundations using nonlocal strain gradient theory. J. Braz. Soc. Mech. Sci. Eng. 2023, 45, 23. [Google Scholar] [CrossRef]
- Luschi, L.; Pieri, F. An analytical model for the determination of resonance frequencies of perforated beams. J. Micromechanics Microengineering 2014, 24, 055004. [Google Scholar] [CrossRef]
- Eltaher, M.A.; Abdraboh, A.M.; Almitani, K.H. Resonance frequencies of size dependent perforated nonlocal nanobeam. Microsyst. Technol. 2018, 24, 3925–3937. [Google Scholar] [CrossRef]
- Eltaher, M.A.; Kabeel, A.M.; Almitani, K.H.; Abdraboh, A.M. Static bending and buckling of perforated nonlocal size-dependent nanobeams. Microsyst. Technol. 2018, 24, 4881–4893. [Google Scholar] [CrossRef]
- Abdelrahman, A.A.; Eltaher, M.A. On bending and buckling responses of perforated nanobeams including surface energy for different beams theories. Eng. Comput. 2022, 38, 2385–2411. [Google Scholar] [CrossRef]
- Abdelrahman, A.A.; Eltaher, M.A.; Kabeel, A.M.; Abdraboh, A.M.; Hendi, A.A. Free and forced analysis of perforated beams. Steel Compos. Struct. 2019, 31, 489–502. [Google Scholar] [CrossRef]
- Alazwari, M.A.; Abdelrahman, A.A.; Wagih, A.; Eltaher, M.A.; Abd-El-Mottaleb, H.E. Static analysis of cutout microstructures incorporating the microstructure and surface effects. Steel Compos. Struct. 2021, 38, 583–597. [Google Scholar] [CrossRef]
- Eltaher, M.A.; Shanab, R.A.; Mohamed, N.A. Analytical solution of free vibration of viscoelastic perforated nanobeam. Arch. Appl. Mech. 2023, 93, 221–243. [Google Scholar] [CrossRef]
- Nabawy, A.E.; Abdelhaleem AM, M.; Alieldin, S.S.; Abdelrahman, A.A. Study of the dynamic behavior of porous functionally graded suspension structural systems using finite elements methods. Steel Compos. Struct. 2022, 45, 697–713. [Google Scholar] [CrossRef]
- Abdelrahman, A.A.; Esen, I.; Daikh, A.A.; Eltaher, M.A. Dynamic analysis of FG nanobeam reinforced by carbon nanotubes and resting on elastic foundation under moving load. Mech. Based Des. Struct. Mach. 2021, 1–24. [Google Scholar] [CrossRef]
- Amendola, A.; Zampoli, V.; Luciano, R. Damped waves under nonlocal Euler–Bernoulli and extended Green–Naghdi II theories in radiating thermoelastic nanobeams. Acta Mech. 2023, 1–9. [Google Scholar] [CrossRef]
- Abdelrahman, A.A.; Mohamed, N.A.; Eltaher, M.A. Static bending of perforated nanobeams including surface energy and microstructure effects. Eng. Comput. 2022, 38, 415–435. [Google Scholar] [CrossRef]
- Zeng, S.; Wang, K.; Wang, B.; Wu, J. Vibration analysis of piezoelectric sandwich nanobeam with flexoelectricity based on nonlocal strain gradient theory. Appl. Math. Mech. 2020, 41, 859–880. [Google Scholar] [CrossRef]
- Krommer, M. On the correction of the Bernoulli-Euler beam theory for smart piezoelectric beams. Smart Mater. Struct. 2001, 10, 668. [Google Scholar] [CrossRef]
- Eftekhari, S.A.; Toghraie, D. Vibration and dynamic analysis of a cantilever sandwich microbeam integrated with piezoelectric layers based on strain gradient theory and surface effects. Appl. Math. Comput. 2022, 419, 126867. [Google Scholar] [CrossRef]
- Liang, X.; Hu, S.L.; Shen, S.P. Effects of surface and flexoelectricity on a piezoelectric nanobeam. Smart Mater. Struct. 2014, 23, 035020. [Google Scholar] [CrossRef]
- Ansari, R.; Nesarhosseini, S.; Faraji Oskouie, M.; Rouhi, H. Size-dependent buckling analysis of piezoelectric nanobeams resting on elastic foundation considering flexoelectricity effect using the stress-driven nonlocal model. Eur. Phys. J. Plus 2021, 136, 876. [Google Scholar] [CrossRef]
- Mehralian, F.; Beni, Y.T. Vibration analysis of size-dependent bimorph functionally graded piezoelectric cylindrical shell based on nonlocal strain gradient theory. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 27. [Google Scholar] [CrossRef]
- Wang, Y.; Ma, Z.; Lei, W.; Zou, Y.; Lu, C. Double effect of electrochemical reaction and substrateon hardness in electrodes of lithium-ion batteries. Acta Mech. 2016, 227, 2505–2510. [Google Scholar] [CrossRef]
- Assie, A.; Akbas, S.D.; Kabeel, A.M.; Abdelrahman, A.A.; Eltaher, M.A. Dynamic analysis of porous functionally graded layered deep beams with viscoelastic core. Steel Compos. Struct. 2022, 43, 79–90. [Google Scholar] [CrossRef]
- Şimşek, M. Some closed-form solutions for static, buckling, free and forced vibration of functionally graded (FG) nanobeams using nonlocal strain gradient theory. Compos. Struct. 2019, 224, 111041. [Google Scholar] [CrossRef]
- Chen, W.Q.; Lü, C.F.; Bian, Z.G. A mixed method for bending and free vibration of beams resting on a Pasternak elastic foundation. Appl. Math. Model. 2004, 28, 877–890. [Google Scholar] [CrossRef]
- De Rosa, M.A.; Maurizi, M.J. The influence of concentrated masses and Pasternak soil on the free vibrations of Euler beams—Exact solution. J. Sound Vib. 1998, 212, 573–581. [Google Scholar] [CrossRef]



| Foundation Parameters | ||||||
|---|---|---|---|---|---|---|
| Kw | Present | Ref. [56] | Analytical Ref. [56] | Ref. [57] | % Error | |
| 0 | 0 | 1.302083 | 1.302290 | 1.302290 | 1.3033 | 0.093378347 |
| 10 | 0.644771 | 0.644827 | 0.644827 | 0.6457 | 0.143874864 | |
| 25 | 0.366091 | 0.366111 | 0.366111 | 0.3671 | 0.274856987 | |
| 10 | 0 | 1.180396 | 1.180567 | 1.180567 | 1.1814 | 0.084983917 |
| 10 | 0.613275 | 0.613325 | 0.613326 | 0.6141 | 0.134342941 | |
| 25 | 0.355649 | 0.355668 | 0.355668 | 0.3566 | 0.266685362 | |
| 102 | 0 | 0.64002 | 0.640074 | 0.640074 | 0.6403 | 0.043729502 |
| 10 | 0.425557 | 0.425582 | 0.425582 | 0.4261 | 0.127434874 | |
| 25 | 0.282834 | 0.282846 | 0.282846 | 0.2836 | 0.270098731 | |
| Parameters | Thickness, h (nm) | Length, L (nm) | Width, wb(nm) | Young’s Modulus E (GPa) | Mass Density ρ (Kg/m3) | Poisson’s Ratio, ν | e311 (C/m2) | μ3111 (C/m) | a33 N/(m2.K) | l |
|---|---|---|---|---|---|---|---|---|---|---|
| Elastic core | 3 | 100 | 5 | 0.130 | 1380 | 0.24 | ---- | ---- | ------ | -- |
| Piezoelectric Layer | 1 | 100 | 5 | 132 | 7500 | 0.27 | −4.1 | 5 × 10−8 | 7.124 × 10−9 | -- |
| %DΔmax | ||||||||
|---|---|---|---|---|---|---|---|---|
| Point Load | Distributed Load | Point Load | Distributed Load | |||||
| α = 0.25 | α = 0.5 | α = 0.25 | α = 0.5 | α = 0.25 | α = 0.5 | α = 0.25 | α = 0.5 | |
| NCL_Elect | 69.60735 | 69.60589 | 74.53625 | 74.53472 | 59.03702 | 59.03509 | 59.34576 | 59.34389 |
| CL_Elect | 65.98284 | 65.98116 | 67.14135 | 67.13964 | 65.98284 | 65.98116 | 67.14135 | 67.13964 |
| NCL_Mec | 82.49071 | 74.88125 | 88.2882 | 80.53019 | 78.0187 | 66.70369 | 78.4219 | 67.05033 |
| CL_Mech | 82.31208 | 72.84932 | 83.74434 | 74.14721 | 82.31208 | 72.84932 | 83.74434 | 74.14721 |
| %DΔmax | ||||||||
|---|---|---|---|---|---|---|---|---|
| Point Load | Distributed Load | Point Load | Distributed Load | |||||
| α = 0.25 | α = 0.5 | α = 0.25 | α = 0.5 | α = 0.25 | α = 0.5 | α = 0.25 | α = 0.5 | |
| NCL_Elect | 71.8572 | 71.8557 | 73.8245 | 73.8230 | 58.7368 | 58.7349 | 59.0230 | 59.0211 |
| CL_Elect | 65.8932 | 65.8915 | 66.7878 | 66.7861 | 65.8932 | 65.8915 | 66.7878 | 66.7861 |
| NCL_Mec | 86.1856 | 77.9658 | 87.5840 | 79.8012 | 77.8320 | 66.4352 | 78.1861 | 66.7520 |
| CL_Mech | 82.5244 | 72.8639 | 83.4433 | 73.8111 | 82.5244 | 72.8639 | 83.4433 | 73.8111 |
| %DΔmax | ||||||||
|---|---|---|---|---|---|---|---|---|
| Point Load | Distributed Load | Point Load | Distributed Load | |||||
| Kw = Kp = 0 | Kw = 20, Kp = 2 | Kw = Kp = 0 | Kw = 20, Kp = 2 | Kw = Kp = 0 | Kw = 20, Kp = 2 | Kw = Kp = 0 | Kw = 20, Kp = 2 | |
| NCL_Elect | 0.0162 | 0.0107 | 0.0163 | 0.0103 | 0.0164 | 0.0127 | 0.0163 | 0.0127 |
| CL_Elect | 0.0163 | 0.0117 | 0.0162 | 0.0116 | 0.0163 | 0.0117 | 0.0162 | 0.0116 |
| NCL_Mec | 199.8589 | 81.4321 | 199.8589 | 72.6340 | 199.8589 | 106.9395 | 199.8589 | 106.3326 |
| CL_Mech | 199.8588 | 91.8268 | 199.8590 | 89.3870 | 199.8588 | 91.8268 | 199.8590 | 89.3870 |
| %DΔmax | ||||||||
|---|---|---|---|---|---|---|---|---|
| Point Load | Distributed Load | Point Load | Distributed Load | |||||
| Kw = Kp = 0 | Kw = 20, Kp = 2 | Kw = Kp = 0 | Kw = 20, Kp = 2 | Kw = Kp = 0 | Kw = 20, Kp = 2 | Kw = Kp = 0 | Kw = 20, Kp = 2 | |
| NCL_Elect | 0.0081 | 0.0053 | 0.0081 | 0.0051 | 0.0081 | 0.0063 | 0.0082 | 0.0063 |
| CL_Elect | 0.0081 | 0.0058 | 0.0080 | 0.0057 | 0.0081 | 0.0058 | 0.0080 | 0.0057 |
| NCL_Mec | 34.1643 | 22.5597 | 34.1643 | 21.3813 | 34.1643 | 26.3062 | 34.1643 | 26.24783 |
| CL_Mech | 34.1643 | 24.2761 | 34.1643 | 24.0080 | 34.1643 | 24.2761 | 34.1643 | 24.0080 |
| %DΔmax | ||||||||
|---|---|---|---|---|---|---|---|---|
| Point Load | Distributed Load | Point Load | Distributed Load | |||||
| L/h = 10 | L/h = 20 | L/h = 10 | L/h = 20 | L/h = 10 | L/h = 20 | L/h = 10 | L/h = 20 | |
| NCL_Elect | 41.13188 | 16.64304 | 39.5017 | 14.6687 | 53.9718 | 22.5435 | 37.1580 | 13.8975 |
| NCL_Mec | 37.550 | 14.85231 | 35.4802 | 12.4886 | 46.1735 | 20.4469 | 28.285 | 10.7989 |
| %DΔmax | ||||||||
|---|---|---|---|---|---|---|---|---|
| Point Load | Distributed Load | Point Load | Distributed Load | |||||
| L/h = 20 | L/h = 50 | L/h = 20 | L/h = 50 | L/h = 20 | L/h = 50 | L/h = 20 | L/h = 50 | |
| NCL_Elect | 7.3857 | 8.5263 | 7.0698 | 8.2201 | 0.9274 | 0.1629 | 0.2627 | 0.0547 |
| CL_Elect | 8.6976 | 8.7156 | 8.4846 | 8.4896 | 0.6451 | 0.1120 | 0.3654 | 0.0585 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdelrahman, A.A.; Saleem, H.A.; Abdelhaffez, G.S.; Eltaher, M.A. On Bending of Piezoelectrically Layered Perforated Nanobeams Embedded in an Elastic Foundation with Flexoelectricity. Mathematics 2023, 11, 1162. https://doi.org/10.3390/math11051162
Abdelrahman AA, Saleem HA, Abdelhaffez GS, Eltaher MA. On Bending of Piezoelectrically Layered Perforated Nanobeams Embedded in an Elastic Foundation with Flexoelectricity. Mathematics. 2023; 11(5):1162. https://doi.org/10.3390/math11051162
Chicago/Turabian StyleAbdelrahman, Alaa A., Hussein A. Saleem, Gamal S. Abdelhaffez, and Mohamed A. Eltaher. 2023. "On Bending of Piezoelectrically Layered Perforated Nanobeams Embedded in an Elastic Foundation with Flexoelectricity" Mathematics 11, no. 5: 1162. https://doi.org/10.3390/math11051162
APA StyleAbdelrahman, A. A., Saleem, H. A., Abdelhaffez, G. S., & Eltaher, M. A. (2023). On Bending of Piezoelectrically Layered Perforated Nanobeams Embedded in an Elastic Foundation with Flexoelectricity. Mathematics, 11(5), 1162. https://doi.org/10.3390/math11051162






