Categorical Join and Generating Families in Diffeological Spaces

Abstract

We prove that a diffeological space is diffeomorphic to the categorical join of any generating family of plots.

1. Introduction

Diffeological spaces were introduced by Souriau [1] as a generalization of differentiable manifolds. This setting includes not only finite-dimensional manifolds, but also manifolds with boundary, infinite-dimensional manifolds, leaf spaces of foliations, or spaces of differentiable maps. The main reference is Iglesias-Zemmour’s book [2]. A recent survey is [3]. Many fundamental results are also exposed in nLab [4].

A diffeological structure on a set X is given by declaring which maps from open subsets of Euclidean spaces into X are considered to be smooth. These maps are called the plots of the diffeology (Section 2). They can be seen as n-dimensional curves, $n\ge 0$, on X. This contravariant idea differs from the covariant classical one of declaring which real maps from a manifold X are smooth. On the other hand, the idea of an atlas on a manifold is generalized by considering a generating family of plots (Section 4).

Our main result (Theorem 2) will be to give a categorical interpretation of a generating family, namely by proving that the diffeological space is the join of the family, that is, the push-out of the pull-back. This establishes a connection between two seemingly unrelated ideas.

Most examples will be related to finite-dimensional manifolds.

2. Diffeological Spaces

A diffeology on the set X is a family $\mathcal{D}$ of set maps $\alpha :U\to X$ called plots such that:

• The domain of each plot $\alpha$ is an open subset $U\subset {\mathbb{R}}^n$ of some Euclidean space ${\mathbb{R}}^n$, $n\ge 0$;
• Any constant map $\alpha :U\subset {\mathbb{R}}^n\to X$ belongs to the family $\mathcal{D}$;
• If $\alpha :U\subset {\mathbb{R}}^n\to X$ belongs to $\mathcal{D}$ and if $h:V\subset {\mathbb{R}}^m\to U\subset {\mathbb{R}}^n$ is a $C^{\infty }$-map, then the composition $\alpha \circ h$ belongs to $\mathcal{D}$;
• The map $\alpha :U\to X$ belongs to $\mathcal{D}$ if and only if it locally belongs to $\mathcal{D}$, that is, for each $p\in U$, there exists some open subset $p\in V\subset U$ such that ${\alpha }_{\mid V}$ belongs to $\mathcal{D}$.

Note that the domain U and the Euclidean space ${\mathbb{R}}^n$, $n\ge 0$, depend on the plot $\alpha$.

A diffeological space $(X,\mathcal{D})$ is a set X endowed with a diffeology $\mathcal{D}$.

Remark 1.

Diffeological spaces are considered to be of class ${\mathcal{C}}^{\infty }$. By changing ${\mathcal{C}}^{\infty }$ by ${\mathcal{C}}^r$ in axiom (3) for some $0\le r\le \omega$, we could obtain a theory for the diffeological spaces of class ${\mathcal{C}}^r$.

Example 1.

Let M be a finite-dimensional manifold. The manifold diffeology ${\mathcal{D}}_M$ is the collection of all ${\mathcal{C}}^{\infty }$-maps $U\subset {\mathbb{R}}^m\to M$ defined on open subsets of Euclidean spaces with values in M.

Example 2.

Let R be any equivalence relation on the manifold M and let $\pi :M\to M/R$ be the quotient map. We endow the quotient set $M/R$ with the quotient diffeology ${\mathcal{D}}_M/R$ where the map $\alpha :U\to M/R$ belongs to ${\mathcal{D}}_M/R$ if it locally factors in some plot of the manifold diffeology ${\mathcal{D}}_M$ on M.

Example 3.

Let $N\subset M$ be any subset of the manifold M. We endow N with the subspace diffeology formed by the plots $\alpha :U\to M$ in the manifold diffeology ${\mathcal{D}}_M$ such that $\alpha \left(U\right)\subset N$.

The last two examples show that diffeology is a much more flexible setting than the classical one.

3. Smooth Maps

Definition 1.

Let $(X,{\mathcal{D}}_X)$, $(Y,{\mathcal{D}}_Y)$ be two diffeological spaces. A set map $f:X\to Y$ is smooth when $\alpha \in {\mathcal{D}}_X$ implies $f\circ \alpha \in {\mathcal{D}}_Y$.

Example 4.

Let $M,N$ be ${\mathcal{C}}^{\infty }$-manifolds endowed with the manifold diffeologies ${\mathcal{D}}_M$, ${\mathcal{D}}_N$, respectively. Then, the smooth maps between M and N as diffeological spaces are the ${\mathcal{C}}^{\infty }$-maps as differentiable manifolds.

Proposition 1.

The composition of two smooth maps is a smooth map.

Proposition 2.

The quotient map $\pi :M\to M/R$ of Example 2 is smooth. Moreover, a map $f:M/R\to N$ is smooth if and only if the composition $f\circ \pi :M\to N$ is smooth.

Corollary 1.

A quotient map $\alpha :U\to X$ is a diffeomorphism if and only if it is bijective.

Proof. 

Let ${\alpha }^{-1}:X\to U$ be the inverse map. Since ${\alpha }^{-1}\circ \alpha ={\mathrm{id}}_X$ is smooth, ${\alpha }^{-1}$ is smooth. □

Example 5.

If $N\subset M$ is a subset endowed with the subspace diffeology of Example 3, then the inclusion map $i_N:N\subset M$ is smooth. Moreover, a map $f:P\to N$ is smooth if and only if $i_N\circ f:P\to M$ is smooth.

Example 6.

By Axiom 3 of diffeology, the plots $\alpha :U\to X$ of a diffeology are smooth maps.

Definition 2.

A diffeomorphism is a smooth map with a smooth inverse.

4. Generating Families

Definition 3.

It is easy to check that the intersection of diffeologies on a set X is a diffeology on X. Then, if $\mathcal{F}$ is any family of set maps ${\alpha }_i:U_i\subset {\mathbb{R}}^{n_i}\to X$ we can consider the smallest diffeology $\mathcal{D}=⟨\mathcal{F}⟩$ containing $\mathcal{F}$. We will say that $\mathcal{F}$ is a generating family for the diffeology $\mathcal{D}=⟨\mathcal{F}⟩$.

We will assume that the collection $\mathcal{F}$ contains all the constant plots.

Example 7.

Any atlas on a manifold M is a generating family of the manifold diffeology.

Example 8.

Let $(X,{\mathcal{D}}_X)$ be a diffeological space and let $f:X\to Y$ be a set map. The diffeology on Y generated by the maps $f\circ \alpha$, with $\alpha \in {\mathcal{D}}_X$, is called the final diffeology. It is formed by the maps that are locally of the form $f\circ \alpha$ for some $\alpha \in {\mathcal{D}}_X$.

Example 9.

Let $(Y,{\mathcal{D}}_Y)$ be a diffeological space and let $f:X\to Y$ be a set map. The initial diffeology on X is the diffeology generated by the set maps $\alpha :U\subset {\mathbb{R}}^n\to X$ such that $f\circ \alpha \in {\mathcal{D}}_Y$.

Example 10.

Let $(X,{\mathcal{D}}_X)$, $(Y,{\mathcal{D}}_Y)$ be two diffeological spaces. The product diffeology on $X\times Y$ is the intersection of the initial diffeologies for the projections $p_X:X\times Y\to X$ and $p_Y:X\times Y\to Y$.

Example 11.

The coproduct diffeology on the disjoint union $X\bigsqcup Y$ is generated by the final diffeologies for the inclusions $i_X:X\hookrightarrow X\bigsqcup Y$ and $i_Y:Y\hookrightarrow X\bigsqcup Y$.

It is easy to check that the product and coproduct diffeologies verify the usual universal properties.

The following criterion of generation is very useful.

Theorem 1

([2] Art. 1.68). Let the diffeology $\mathcal{D}=⟨\mathcal{F}⟩$ be generated by the family $\mathcal{F}$. A set map $\alpha :U\subset {\mathbb{R}}^n\to X$ belongs to $\mathcal{D}$ if and only if it locally factors through some element of $\mathcal{F}$ (we assume that constant plots are all contained in $\mathcal{F}$).

Moreover, a map $f:(X,{\mathcal{D}}_X)\to (Y,{\mathcal{D}}_Y)$ is smooth if and only if $f\circ \alpha \in {\mathcal{D}}_Y$ for any $\alpha \in \mathcal{F}$ in a generating family $\mathcal{F}$ of ${\mathcal{D}}_X$.

5. Categorical Constructions

The category of diffeological spaces and smooth maps has limits and colimits. Here, we will show how to construct the pull-back, the push-out, and the join of the two smooth maps. Most of time, these maps will be plots of some diffeology on X. We refer to the recent paper [5] for other categorical constructions.

5.1. Pull-Back

Let $\alpha :U\to X$, $\beta :V\to X$ be two smooth maps with the same codomain. The pull-back of $\alpha$ and $\beta$ is the set

$$P=\left\{\right(u,v)\in U\times V:\alpha (u)=\beta (v\left)\right\}.$$

We endow it with the subspace diffeology of the product diffeology on $U\times V$.

The projections $p_1:U\times V\to U$ and $p_2:U\times V\to V$ induce smooth maps

$$p_U:P\to U,p_V:P\to V,$$

which verify $\alpha \circ p_U=\beta \circ p_V$ and the universal property of a pull-back:

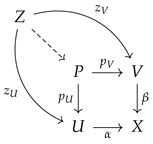5.2. Push-Out

Let $p_U:P\to U$, $p_V:P\to V$ be two smooth maps with the same domain. The push-out of $p_U$ and $p_V$ is the quotient $J=(U\bigsqcup V)/R$ of the coproduct $U\bigsqcup V$ by the equivalence relation R generated by

$$u\in U,v\in V,uRv\mathrm{iff}\exists p\in P:p_U\left(p\right)=u,p_V\left(p\right)=v.$$

The maps $j_U=\pi \circ i_U$ and $j_V=\pi \circ i_V$ verify $j_U\circ p_U=j_V\circ p_V$ and the universal property of a push-out:

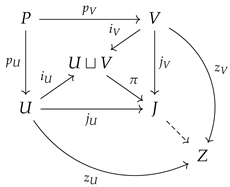5.3. Join

Given two smooth maps $\alpha :U\to X$ and $\beta :V\to X$, it is an exercise to check that the push-out of the pull-back of $\alpha$ and $\beta$ is diffeomorphic to the join $U\ast V$ of $\alpha$ and $\beta$ [6], defined as follows: there is a well-defined smooth map $\alpha \ast \beta :U\ast V\to X$ and maps $j_U:U\to U\ast V$ and $j_V:V\to U\ast V$ such that

• $(\alpha \ast \beta )\circ j_U=\alpha$,  $(\alpha \ast \beta )\circ j_V=\beta$,
• They verify the universal property

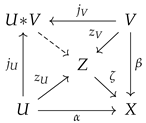
Analogously, given k maps ${\alpha }_i:U_i\to X$, the join $U=U_1\ast \cdots \ast U_k$ and the universal map $\alpha ={\alpha }_1\ast \cdots \ast {\alpha }_k:U=U_1\ast \cdots \ast U_k\to X$ are defined by induction.

Notice that, in the definition of the join, the maps $\alpha$ and $\beta$ are arbitrary smooth maps, so for three plots ${\alpha }_1,{\alpha }_2,{\alpha }_3$, one can consider the join of the maps ${\alpha }_1\ast {\alpha }_2$ and ${\alpha }_3$.

Remark 2.

It is possible to define the join of an infinite number of plots, but we will not develop this idea for the sake of simplicity.

Lemma 1.

Let $\mathcal{F}=\{{\alpha }_i:U_i\to X\}$ is a generating family. Then, the universal map $\alpha ={\ast }_i{\alpha }_i:U={\ast }_i{U}_i\to X$ of the join is a quotient map.

Proof. 

Let $\gamma :W\to X$ be a plot on X. By Theorem 1, for each $p\in W$, there exists a neighborhood $W_p\subset W$ such that $\gamma$ factors through some ${\alpha }_i$. Hence, it factors through the disjoint union and consequently through the join. □

6. Main Result

Next, theorem is our main result.

Theorem 2.

Let $(X,\mathcal{D})$ be a diffeological space. A family $\mathcal{F}=\{{\alpha }_i:U_i\to X\}$ of plots is a generating family for $\mathcal{D}$ if and only if there is a diffeomorphism $\alpha ={\alpha }_1\ast \cdots \ast {\alpha }_n:U=U_1\ast \cdots \ast U_n\to X$ commuting with the maps ${\alpha }_i$ and the natural maps $j_i:U_i\to U$, that is, $\alpha \circ j_i={\alpha }_i$ for all i.

Proof. 

Let $\mathcal{F}$ be a generating family. The map $\alpha$ is surjective because generating families include all constant plots.

It is injective because $\alpha \left(\left[u_i\right]\right)=\alpha \left(\left[u_j\right]\right)$ means that either $u_i=u_j$ or $u_i\in U_i$, $u_j\in U_j$ and ${\alpha }_i\left(u_i\right)={\alpha }_j\left(u_j\right)$, that is, $\left[u_i\right]=\left[u_j\right]$. Then, $\alpha$ is bijective and Corollary 1 applies. Hence, $\alpha$ is a diffeomorphism.

The converse statement follows from Lemma 1. □

Example 12.

Let X be the disjoint union of a line $X_1=\mathbb{R}$ and a point $X_0=\left\{0\right\}$, endowed with the diffeology generated by the constant plot ${\alpha }_0:{\mathbb{R}}^0\to X$, ${\alpha }_0\left(0\right)=0$, and the identity plot ${\alpha }_1:{\mathbb{R}}^1\to X_1\subset X$, ${\alpha }_1\left(t\right)=t$, respectively. Clearly, $X\cong U_0\ast U_1=U_0\bigsqcup U_1$.

Example 13.

Let X be the set

$$X=\{(x,y)\in {\mathbb{R}}^2:xy=0\}.$$

We consider the diffeology on X generated by the two plots

$$\begin{array}{cc}\hfill {\alpha }_1& :U_1=\mathbb{R}\to X,{\alpha }_1\left(t\right)=(t,0),\hfill \\ \hfill {\alpha }_2& :U_2=\mathbb{R}\to X,{\alpha }_2\left(t\right)=(0,t).\hfill \end{array}$$

Then, $X\cong U_1\ast U_2$.

As we pointed out in [7], this is not the cross diffeology on X induced by the manifold diffeology of ${\mathbb{R}}^2$.

Example 14.

The manifold diffeology on a manifold M is the join of any atlas. More precisely, let $\{{\alpha }_i\subset {\mathbb{R}}^m:U_i\to M\}$ be an atlas on the manifold $M^m$. Then

$$\bigcup _i{\alpha }_i\left(U_i\right)=M\cong {\ast }_i{U}_i,$$

where the open subsets $U_i\cong \alpha \left(U_i\right)\subset M$ are glued by inclusions.

7. Conclusions

The category of diffeological spaces and smooth maps contains smooth manifolds as a full subcategory but has better properties, such as having all limits and colimits. One basic idea, that of generating families, seems to have not been fully exploited, as we show in our main Theorem 2. This kind of result has applications in Cartan calculus (see [7]).