“It Is Like a Feeling”: Theorizing Emotion in Mathematics through Complex Embodiment

Abstract

Conversations of educational equity in mathematics necessitate a more deliberate, nuanced look at the mathematical processes of learning for students of color from historically marginalized communities. This paper describes the theoretical work of a research collaborative that seeks to develop understanding of the experiences around mathematical identity of Latinas labeled with Learning Disabilities in mathematics classrooms. Expanding the theory of Complex Embodiment from Disability Studies, we explore new interdisciplinary theoretical and methodological tools to analyze the emotional, embodied experience of learning mathematics in the social worlds of mathematics classrooms, using emotional discourse. We take up theoretical and methodological practices around intersectionality through analysis of how power and positioning operate in mathematics identity development. We find that the young woman whose narratives we explore in this paper is positioned through deficit discourses around disability and multilingual learners, yet she understands herself through a positive mathematical affinity she shares with her mother. Over time, we see her narratives shift emotionally away from mathematics, as well as away from this connection with her mother. Her narratives help us develop a theoretical perspective that understands emotion in mathematics learning as both embodied and socially constructed.

1. Introduction

During an interview in her seventh-grade year, a Latina named Rita was explaining how she learned differently from teachers and peers. She said, “Well it is like a feeling. If the teacher explains, I will understand it.” In our work, we have heard students make this connection again and again between emotions and mathematics learning. This particular comment also connected to Rita’s strong relationship with her mathematics teacher, who like her, was Latina. Mathematics learning is mediated by relationships, emotions, culture, and intersectionality. All things, including mathematical things, are known in and through emotion, bodies, and cultural constructions [1]. We do not learn mathematics in school in an environment separate from our emotions and identities. On the contrary, learning mathematics is a particularly emotional experience as it is the only subject in school with its own associated anxiety in the Diagnostic and Statistical Manual of Mental Disorders (DSM) [2]. While research in mathematics education has explored embodiment, such research has separated emotion from embodiment as if the body is only the senses, rather than how we process experience. We experience the world, and mathematics, through bodies that are marked and positioned in particular ways by cultural practices. In this paper, we posit the importance of understanding student experience and embodiment (including emotion) in mathematics classrooms, both from an experiential point of view and in how students and their experiences are framed through cultural practices [3].

Educational equity warrants a closer look at the mathematical learning taking place for students from historically marginalized communities. For far too long, mathematics education has not done enough to fully capture the mathematical experiences of students navigating multiple axes of power and identity, such as students of color, students with disabilities (Respecting language preferences that vary in the disability community, we use both identity-first and person-first language in this paper. We also use dis/Ability as well as disability, to call attention to the construction of ability through disability), and girls. The dearth of research on students of color with disabilities demands a much-needed examination of students’ experiential learning amidst their intersectional identities [4]. We seek to add to scholarship on mathematics identity and develop additional interdisciplinary theoretical and methodological tools around emotion, embodiment, and intersectionality. Our focus is Latinas with Learning Disabilities, and with this focus, we engage in emotion, embodiment, experience, relationships, and intersectionality we believe are critical to understanding the holistic and nuanced experiences of this particular group of students (and all students). Complex embodiment understands disability as simultaneously embodied and a social construction, and the two as co-constructing each other. Bringing the theory of complex embodiment from Disability Studies into mathematics education [5,6], we map embodied differences, as well as social construction and feeling-meaning making [7] around those differences exploring a new theorization of Complex Embodiment in Mathematics.

In this paper, we analyze the mathematical identity processes of Rita, a Latina who was assigned official labels of both English Language Learner and a Learning Disability. To better understand intersectionality, we map circulating narratives and the ways in which she is culturally positioned in terms of race, disability, and gender. Narratives are used in identity work in mathematics scholarship, but there is a disconnect between embodiment in mathematics (found in observations) and identity in mathematics (found mostly in interviews). We use data from ethnographic fieldwork, interview transcripts, and videos, finding and analyzing bodymind narratives.

1.1. Theoretical Framework

Our conceptual framework includes previous research on mathematical identities of Latinx and disabled students, as well as Disability Studies (DS). We take up the theory of Complex Embodiment from DS [6] and use it to better understand the complexities of identification processes in mathematics for multiply-marginalized youth [5,8]. We do this by examining the mathematical experiences and identity processes for Latinas with Learning Disabilities through the lens of mathematics classrooms as figured worlds and exploring the ways race and language intersect with identity development.

1.2. Identity Development in Mathematics

This research uses identity as a theoretical and methodological lens. Identity is a critical tool to understand how individual students, positioned in particular ways in mathematics classrooms, take up identity positions. A practice theory of identity [9] is used to comprehend the processes that occur as individual students come to identify, or not, with mathematics. We come to understand mathematics classrooms as figured worlds, or activity systems where meaning is created through the shared discourses and cultural practices of its participants. Within these figured worlds, participants are continually arranging self-understandings around mathematics, internalizing some circulating discourses while rejecting others. Consequentially, multiple, contradictory discourses can birth spaces for change. Examples of circulating discourses include majoritarian narratives [10] regarding race, gender, and disability, including ability and disability in mathematics [8,11,12]. The learning of mathematics, thus, often accompanies processes of racialization [3].

Zavala (2014) [13] argues that we need additional research about the intersection of race and language for Latina/o youth and their mathematics identity development. Sociocultural research on identity processes for Latina/o students in mathematics embraces testimonios [13,14]. Testimonios, or student voices, are a methodological approach that intentionally center marginalized experiences [15]; by engaging in this testimonial approach, students may become empowered to reclaim their belongingness within school spaces such as mathematics classrooms.

Latinx students must negotiate both racial stereotypes and linguistic positioning in mathematics classrooms [13,16]. Undergraduate Latinas negotiated a complex set of discourses to be successful in mathematics, some of which were specific to Latina experiences such as pressure to be a mother [14]. Research behind Latinx identity processes must address how narratives of achievement are culturally embedded and understood by these students [16]. Listening to the stories and messaging that young Latinas are receiving and internalizing from others is therefore critical to our understanding of how they come to shape their mathematics identity.

The literature in mathematics education has routinely failed to include students with disabilities. Additionally, the field of special education consistently and almost exclusively produces quantitative research theorizing disability through a “deficit” lens [17]. Consequently, research on mathematics identity for students with Learning Disabilities is scarce. Learning Disabilities (LD) are a special education label in the United States used to designate students with difficulties processing language and/or mathematics, who show strengths in some areas and have sustained challenges in others [18,19]. Current definitions of the term in the US include dyslexia and dyscalculia. In investigating the development of mathematics identity for Latinx students with LD, Lambert [5,11,12] has found teachers’ understanding of disability to be contextual, shifting with mathematical pedagogy. For instance, new potential for mathematics success was imagined when Latinx students with LD reframed majoritarian narratives of “slow” students [11,12]. Our collaborative research is thus focused on investigating the mathematical experiences and identity processes taking place for Latinas with LD. We pay particular attention to narratives of growth towards and away from mathematics.

1.3. Latinas Navigating School and Mathematics Classrooms

Our focus on relationships as mediating mathematical learning is supported by research on how Latinas are influenced and impacted by both peers and family members in their relationships with school [20,21]. Latina elementary school girls showed that more communication with their mom was associated with higher grades in mathematics and liking mathematics more [20]. Relationships are powerful levers for emotion. In recognizing the critical role that relationships amongst teachers and peers play in learning mathematics [22], we attempt to understand how relationships with peers within the mathematics classroom and relationships with mothers impact the mathematical identity of Latinas with LD. While peer support is important for both genders, we know that given adolescent girls’ focus on and interest in peer relationships, these relationships may be especially poignant when it comes to their engagement with science, technology, engineering, and mathematics (STEM) subjects [23]. Perceived peer support for adolescent girls has been linked to their increased social and cognitive engagement with subjects such as mathematics and science. Several studies have revealed that this peer support is also associated with increased self-efficacy and promising emotionality towards mathematics and science. For example, Riegle-Crumb et al.’s study [24] found that high school girls who reported having female friends that excelled in science and mathematics were also more likely to enroll in these advanced subjects. Peers of adolescent girls can therefore have an impact on the development of future identities. This link between peer support and adolescent girls’ relationship with mathematics and science may therefore be critical for the study of Latinas who may feel their teachers hold lower expectations for their academic achievement [25].

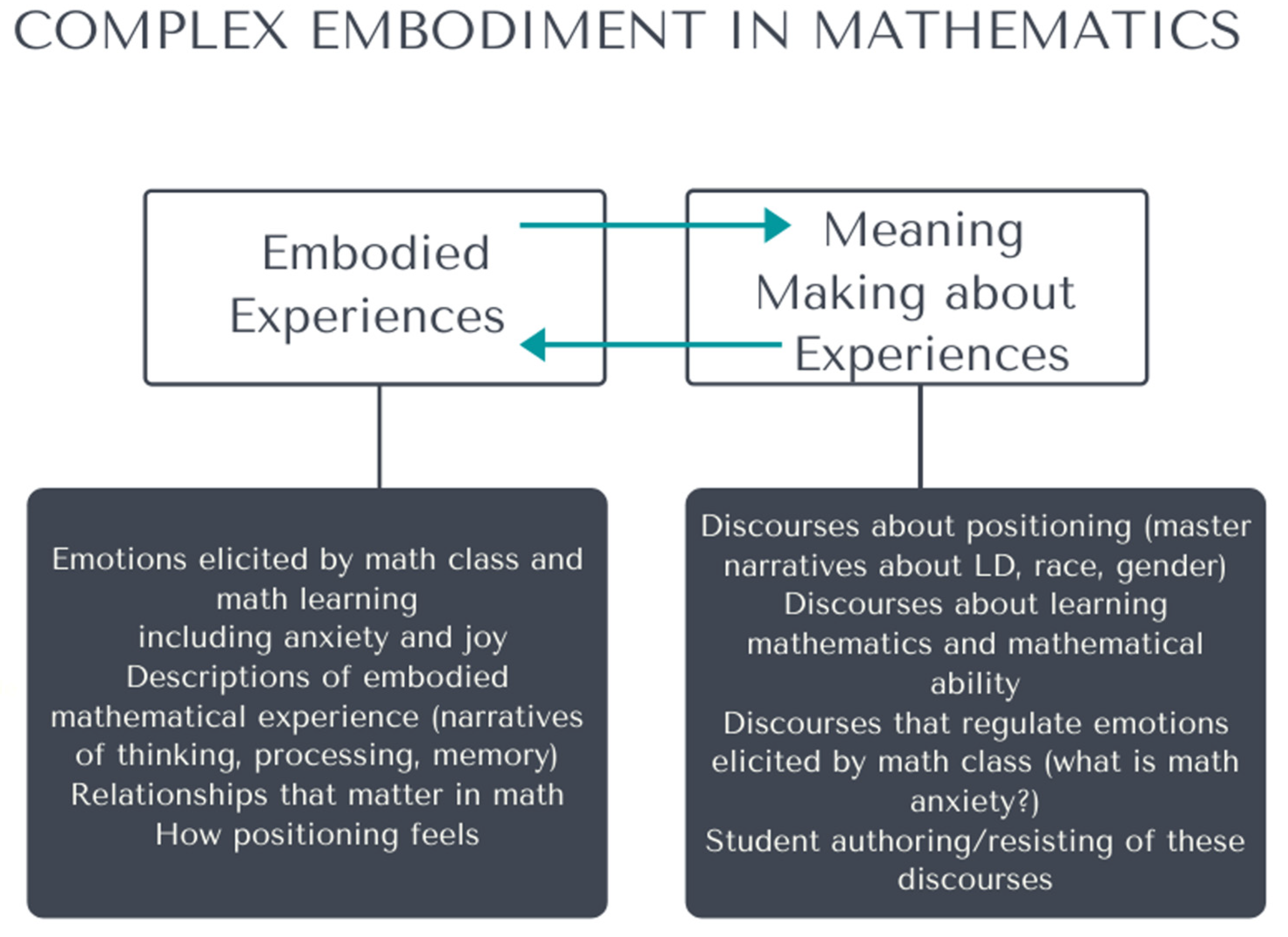
1.4. Disability Studies and Complex Embodiment

We take a critical approach to the field of special education in the United States, which developed from multiple discourses such as experimental psychology, clinical medicine, and behaviorism, all of which tend to view disability as a condition of the individual. Reid and Valle [26] describe how these multiple circulating discourses construct individual children as having a “Learning Disability.” They suggest that this focus on finding individual deficits in children who are “underperforming” creates educational contexts that focus on finding and then fixing these “deficits,” rather than investigating how pedagogical contexts construct disability—both ability and disability at the intersections of language, ethnicity, and gender. When we analyze mathematics education through a Disability Studies (DS) lens, we notice how disability and intelligence are constructed through processes as varied as mathematical activity, pedagogy, research methods, and the borderlands between institutions and feelings, affect, and emotionality [27]. DS analyzes power and positioning in disability, and how disability operates within intersectionality [28].

In his theory of complex embodiment, disabled scholar Siebers [6] rejects both the purely social model of disability, for erasing embodiment, and the medical model, which defines disability as individual and requiring medicalized “interventions.” Disability is not solely within the body, nor purely social, but both. Embodied knowledge is produced through cultural processes; language to describe our bodies does not spring from a neutral source. Thus, when we describe a body, we use concepts formed in social worlds, which in turn shape our bodies. Siebers argues attention to the body can never be an afterthought when interrogating disability, because the body is the site of political resistance. Bringing this theory to mathematics education, we extend complex embodiment by foregrounding intersectionality, relationships, and emotion.

1.5. Emotion as Embodiment

Embodiment has been theorized as critical to the invention of mathematics itself; mathematics is not separate from the body but created through human actions in space and time [1]. Research on gesture in mathematics has explored how learners use gesture to communicate and construct mathematical ideas [29]. Research on embodiment in mathematics education has explored embodied differences of disability [30]. Embodiment is most often used to understand mathematical cognition, excluding emotion as part of embodied experience. We argue embodiment should reflect bodyminds [31] without unnecessary separation between the feelings of the mind and the body. We seek to include bodymind narratives, narratives of emotion as well as descriptions of how cognitive processes feel, such as thinking, learning, listening, and remembering in mathematics.

Historically, emotion, feelings, and affects in education have been framed from a medical-psychological model of emotionality in education [32,33,34]. What counts as emotion in education is something inside students’ minds and bodies solely, and in turn students’ minds and bodies become the site for “identification”, “remediation”, and “intervention.” This is similar to the medical model of disability in schools and society from the medical model centering the neurology, mind, and body as the site of disability. For example, the fields of special education and LD have lacked a more robust and pluralistic articulation about what counts as emotion, feelings, and affects in the lives of those labeled with special education labels such as LD.

Within the field of education, Kenway and Youdell [34] call for socio-cultural-spatial analyses of education and emotion. Based on the researcher’s (participant) observations, thick descriptions are generated as data, not only of the educational phenomenon in question but also how emotion is being experienced by participants—including the researcher themselves. In other words, from a socio-cultural-spatial perspective on emotion and education, emotion is not only within the neurology of children or humans, but emotion is social, relational, and indexed within the materiality of spaces and places. Borrowing from both feminist theorists and specifically from Ahmed [35], Benesch [36] conceptualizes emotions as:

Embodied (sensations and feelings); they are shifting (we are moved), not static or monolithic; and they are socially constructed (our interpretation of them might depend on others’ prior interpretations), not private, internal, or cognitive. In addition, these feminist scholars include emotions in critical theory not to reject post-structuralism, but rather to incorporate this missing dimension. Power and emotion go hand in hand, from their perspective, and the study of both, heightens the potential for critical praxis.

([36], p. 44)

Here we see Benesch explicate the feminist definition of emotion in such a way that defines emotions as shifting, dynamic, and socially situated since they are open to interpretations of the individual experiencing them and others within contexts that are filled with “emotional affinities” ([36], p. 52). Benesch’s attention to the relationship between power and emotions foregrounds what she later explicates as critical praxis’ potential for subtle moment-to-moment social transformation. That is, also in the analysis or reflections about the actions within practice in specific contexts. Therefore, Benesch adheres to a theory of emotion that includes not only a theory of power but of agency and change.

1.6. Intersectionality

Our analysis of emotion on embodiment must take into account how discrimination based on marginalized identities such as race, ethnicity, disability, and gender operate in personal, interpersonal, structural, and political dimensions of society [37,38,39]. Intersectionality necessitates that we examine power and position, including the shifting of power across multiple contexts [39]. Our strategies for centering intersectionality extend to the composition of a diverse research team, including scholars that ethnically identify as Latinx, White, Middle Eastern, and those that identify as LD, and those that do not. Additionally, we include theoretical tools taken from DS as such tools were created for and by historically marginalized people [4,40]. We examine the narratives and emotion discourses that unfold in the classroom, tracking how students resist and/or reframe these circulating narratives and dominant emotional discourses. Therefore, the methodology behind addressing intersectionality should include an analysis of power and positioning at the individual and institutional level with the inclusion of discursive, emotive, and material levels of talk [33]. Our methodological analysis is further described in Figure 1.

Our research question integrates the analysis of mathematical identity with emotion. We ask, “How does emotion matter in the mathematical identity processes of a Latina with a learning disability?”

2. Materials and Methods

The data from this paper come from a larger longitudinal ethnographic study, which analyzes the identification processes of Latino/a focal students and teachers as they participate in a seventh-grade mathematics classroom. Additional data on methodology are available [5,11,12].

2.1. Participants

This research was situated in a middle school in New York City. Most students in the school identified as Dominican American, often with multiple generations in the United States. The school was successful based on standardized test scores, scoring in the top 25% of middle schools in the city based on mathematics test scores. The school had a comprehensive creative arts program and other enrichment opportunities. Students with Learning Disabilities were educated in general education classrooms that were co-taught by a general educator and a special educator. The school was chosen by the first author so that the research would be situated in a school that offered positive experiences to students of color, rather than the deficit-based research that attends only to negative experiences [41].

The participants in the original study included nine focus students and their teachers in both sixth and seventh grade. Our focus student, Rita, identified herself as a girl, as Latina, and as “always good at mathematics” when the first author spoke to her in sixth grade. Rita was labeled as a long-term English Language Learner and received special education services for a Specific Learning Disability. The sixth-grade special education teacher, Ms. Emerson, was white and in her third year of teaching. Her co-teacher, Mr. Pierce, was also white and in his third year of classroom teaching. Her seventh-grade mathematics teacher was Ms. Marquez, who was Latina.

2.2. Data Sources and Analysis

This paper includes data from an interview with Rita’s teachers in sixth grade, ethnographic field notes of her engagement in sixth and seventh grade, and two interviews with Rita in seventh grade. The first author collected data about the cultural practices of the mathematics classroom through a two-year ethnography of the students’ mathematics classrooms, including field notes, teacher interviews, and video recordings. In addition, to capture the students’ experience, the first author also conducted multiple interviews with the students. The research was designed to provide multiple data sources, both of the classroom and individuals. At the classroom level, data were collected through participant observation, video recordings, and classroom artifacts. Interviews were the primary source of data at the individual level. The first author developed analytic memos of each focus student. Trustworthiness was established through triangulation of these multiple data sources, concurrent data analysis, and member checks.

Our collective analysis began with the creation of an interdisciplinary team that included expertise in mathematics and disability, Latina youth development, Critical Disability Studies, and intersectionality. As a collective, we read transcripts and field notes and analyzed video. Our collective analysis sessions focused on identifying the emotions that these narratives elicited in us and in Rita. We also engaged in larger discussions, particularly around the counter-narratives that Rita was responding to. This led us to larger discussions about the theory and methodologies used in understanding mathematics identity at her intersections and through her emotionality. In this paper, we utilize an emotional discourse analysis to present our findings [42,43,44,45]. Emotion discourse is contextualized within social practices and found within the responses of students’ interview data, including reactions, opinions, etc. [44]. Following the process outlined in the work of Hernández-Saca [43], we identified the content of their responses- the “WHATs”- and the “HOWs”- the manner in which the participants marked emotionality through intensifiers [46] and through emotion implicative WHATs [45]. Any responses or topics from a speaker or narrator that socially and culturally bring up feelings, emotions, and/or affects such as “taking a test,” however, that the speaker does not explicitly name or label with any feelings, emotions and/or affects are considered emotion implicative WHATs [45]. In turn, emotion implicative WHATs are topics or responses that imply emotionality about the topic (the WHAT) the speaker is talking about. Intensifiers are the indices of social and emotional expression within the linguistic responses and statements of speakers [44]. All data were coded by the second author within Google Documents based on the following emotion-laden talk coding key we developed for this study (See

Table 1. Emotion-Laden Talk Coding Key.

Emotion Discourse Code	Emotion Discourse Code Meaning
Bolded and underlined	Repetitive Word
Italicized	Intensifier [positive, negative or in-between]
Bold	Emotion Implicative WHAT
Bolded, italicized and underlined	emotion, feeling, and/or affect lexicon
Single Asterisk (*) and underlined	* metaphors
Double Asterisk (**) and bold	** identity [indicates that the talk was about the speaker’s identities, such as their race, ethnicity, gender, or dis/Ability, or both or more than one academic, social and emotional identity (e.g., Latina; mathematics learner, etc.]
Placed the Paralinguistic Label or Code within comments ○ Paralinguistics are the aspects of spoken communication that do not involve words. These may add emphasis or shades of meaning to what people say. Examples: Body language, gestures, facial expressions, tone and pitch of voice are all examples of paralinguistic features.
Placed the Embodiment Label or Code within comments ○ We also coded for instances of embodiment, given that we were interested in how students experienced their dis/Ability, emotionality, and intersectionality about mathematics as complex embodiment. So one of our a priori codes was “Embodiment”

). We then discussed the coded narratives as a collective.

3. Results

Our re-analysis of findings from the original study will focus on three data sources. First, we will analyze narratives from Rita’s sixth-grade teachers from an interview. This data will be interwoven with ethnographic observations. Second, we will present field notes from Rita in her seventh-grade mathematics class. Finally, we will present bodymind narratives from Rita’s interviews in seventh grade, again, in the context of the larger ethnographic project.

3.1. Narratives of Transformation into a “Pretty Good Student”: Rita’s Sixth Grade Teachers

Observations of our study participant, Rita, commenced half-way through her sixth-grade mathematics class. Rita identified as “Latin” when asked in an interview; she had light olive skin, straight, long dark hair, and donned stylish eyeglasses with black frames. Rita was regularly found sitting and working with a group of almost all girls, all of whom were hard-working and serious-seeming. Within that group, Rita was often spotted sitting next to Shaundra, a Latina. Shaundra had excellent grades and no Individualized Education Program (IEP) or English Language Learner (ELL) status. Similarly, Rita received high grades in her mathematics class, finishing the year with an average above 100%. In field notes, it was noted that Rita was typically quiet and serious, rarely confused in class, and was often used as a resource by others. During participant observation, Rita was not observed using Spanish, including in social talk, while others in the class did move back and forth between Spanish and English.

The sixth-grade teachers were interviewed at the end of that academic year. Based on these observations, the first author was both surprised and dismayed when Ms. Emerson described Rita’s entry into sixth grade:

Ms. Emerson: [Rita] came in as like, hmmm, for a lack of another way of putting it, the *uber special ed kid, cried on her way to school, really was always upset, very, seemingly struggled a lot, she also seemed like she used to [switch] a lot more between Spanish and English, and I don’t know whether it was like… fear of speaking in English.

We notice first the highly emotional language used in this narrative of Rita, indicated by the cluster of coding of emotional talk. Referred to as the “uber special ed kid”, Rita was said to have “cried on her way to school”. Additionally, Ms. Emerson shared that it was “fear” that prevented Rita from speaking English, readily connecting fear to Rita’s status as an emergent bilingual. We coded the language terms, Spanish and English as emotional implicative whats because one’s language, such as Spanish and/or English, has an emotional association within U.S. culture. For example, culturally and socially, oftentimes English is seen as the default language of school and is thought of as being “emotionless” and “rational,” ironically carrying a valence of zero. However, for many Latinx students or the Latino community, such experiences with English and corresponding English-Only laws are connected to more strict dispositions in the lived experiences of English Language Learners with and without dis/Abilities who happen to speak Spanish. Spanish, culturally and socially, seems to be more about a language of the heart and love [47].

Further, when Mr. Pierce spoke, he described Rita as possessing “the raw materials” and referred to her as “very smart but not a lot of basic skills”, suggesting that ability is an innate quality. These descriptions of Rita were difficult for the first author to understand: “crying”, “struggled a lot”, “not a lot of basic skills.” Academic struggles are emotional struggles, at least in this narrative.

This negative portrayal and highly emotionalized characterization of Rita should not be considered in isolation, however. Both teachers immediately noted Rita’s metamorphosis into the confident student seen in the second half of the year. Mr. Pierce narrated:

Mr. Pierce: [Rita] took on the **persona almost of Shaundra, people just grouped them together [puts hands out, hands come together], oh there is Shaundra and Rita they are both smart, and Rita kind of grew into **being [hands together come up] a pretty **good student, when at the beginning of the year she seemed not so good.

Mr. Pierce communicates Rita’s transformation using a gesture, showing how they “grew into being a pretty good student” with two hands that started apart, then moved upwards together. The teachers’ narrated Rita’s transformation as relational and embodied, based on her relationship with Shaundra. This allows us to see the figured world of the classroom, and how being a “good student” is connected to moving away from bilingualism and disability. Furthermore, Mr. Pierce’s emotion discourse underscored the fact that Rita’s transformation was based on an identity that was relational and embodied since her friendship with Shaundra was the factor that mediated such a transformation. This points to the fact that students’ social and emotional lives are part and parcel of the academic identification processes that they and their interlocutors navigate in their figured worlds. In other words, our emotion discourse analysis indicated the salience of identity as recounted by Mr. Pierce.

3.2. Narratives of Transformation of Mathematics: Rita in Seventh Grade

Rita brought this new set of behaviors, a new role as a serious, smart student into seventh grade, and was easily recognizable as a serious student. Rita’s engagement was consistent in the fall of seventh grade. More than once, Rita was the only one in a large group of students who did extra work when she finished. She was the only student to get the right answer to a difficult integer word problem. Rita was able to tackle conceptual problems as well as learning procedures. She was able to engage in problem solving without being told exactly what to do. Her grades remained high throughout the year.

In the spring, however, the pedagogy in the class shifted dramatically [11]. In the fall, Ms. Marquez had engaged students in inquiry-based mathematics, as well as a more procedural mathematics approach focused on a textbook. In the spring, feeling pressure from standardized tests, Ms. Marquez shifted towards a procedural-only curriculum, despite her personal beliefs that students needed to engage in inquiry-based problem solving. Ms. Marquez was extremely conflicted about this shift away from meaning making but felt the pressure to deliver high test scores. The class also shifted from heterogenous groupings in the fall to fixed groups based on the teacher’s perspective of students’ mathematical ability. Rita was placed in what was called “the middle group,” which neither catered to the highest achieving group nor the group dominated by other students with IEPs.

In the spring, with this shift to procedural mathematics, Rita seemed less happy in the classroom. She seemed to be somewhat disgruntled during the spring, as she communicated a strong dislike of fractions, telling the first author “I hate fractions.” At another moment, she got annoyed at being told to solve a problem a particular way, saying “multiply this and this and this” in a very frustrated voice. The following excerpt from field notes describes a particular class on algebra and Rita’s response. The first author walked into the classroom as they were discussing simplifying the expression −8x − (−11x). The field notes read (and are coded):

I could feel the intensively negative energy in the air. The teachers seemed frustrated. Kids seemed mostly lethargic, and some actively annoyed. Despite many weeks of work in the fall on adding and subtracting negative numbers, none of the students seemed able to remember the rules, let alone apply them to a situation that included variables. I heard a child call out that they were “confused.” Ms. Marquez became frustrated at this point, telling the kids, “I don’t know why you are confused about integers.” I found a spot near the back, next to the wall of windows, right behind a table with Luis, Carmen and Rita. I could see that Rita was the only student studiously taking notes. I heard Carmen say to no one in particular, “**I am bad at algebra so **I am *lost, super *lost in the world” and a few minutes later, “**I am lost *like a little child.” Ms. Marquez told the kids to “forget about the variable,” and rewrote the problem as −8 − (−11). Up until this point, Rita had been the **only student at her table taking notes. When Ms. Marquez wrote this, however, Rita sat up onto her knees on her chair and said angrily, without being called on, “the letters are important, if you forget them, then you wouldn’t get it right.” Ms. Marquez either did not hear what Rita said, or ignored it. Rita looked upset, but sat back down onto her seat. Carmen continued with her monologue, “the letters confuse **me.”

Such an outburst was very unusual for Rita in her mathematics classrooms. This moment became a critical incident, which the first author discussed with Rita at her final interview at the end of seventh grade, several months later. Author 1 begins the field note by stating— “I could feel the intensively negative energy in the air”—therefore, sensing the affective atmosphere in the air of the classroom. Author 1 goes on to use a total of 39 emotion discourses to describe Rita’s mathematics classroom and Rita’s relationships with her peers. Across the above field note Author 1 used 9 intensifiers (i.e., intensively; seemed (3); mostly; actively; many; only; studiously), and 11 emotion, feelings, and/or affect lexicons (e.g., feel; frustrated (2); annoyed; lethargic; confused (3); bad; angrily; upset). Identifying the emotion implicative whats of these emotions helps us identify what is making the students (and researcher) feel so intensively about mathematics. (i.e., negative energy; weeks of work; adding and subtracting negative numbers; remember the rules; taking notes; forget about the variable; the letters are important, if you forget them). There were also indicators of identity in this mathematical moment (I am bad at algebra; I am; I am lost; me; only student at her table taking notes).

Given the first author’s emotions, feelings, and affective field notes about Rita’s mathematics classroom, we see how emotionality matters in mathematics, and school-based identity processes and contexts for Rita and her relationships. In other words, Rita’s mathematics context involved an affective atmosphere that was felt by the researcher [48]. According to Andersen [48] (2009), affective atmospheres involve:

A material imagination based on the movement and lightness of air, we learn from the former about the turbulence of atmospheres and their indeterminate quality. From the latter, we learn that atmospheres are singular affective qualities that emanate from but exceed the assembling of bodies. As such, to attend to affective atmospheres is to learn to be affected by the ambiguities of affect/emotion, by that which is determinate and indeterminate, present and absent, singular and vague.

(p. 77)

Rita seemed to remember the incident immediately when she was interviewed:

Dr. Lambert: I have this other memory of you this year where you said, Ms. Marquez was talking about algebra and variables, and integers, and she was almost getting a little frustrated, not with anybody in particular, but she was like, why did you guys all forget integers, just forget about the numb-the letters and just do the integers, and you were sitting in your seat, do you remember this?

Rita: Yeah

Dr. Lambert Do you remember what you said?

Rita: Why should we forget about the letter? The letter is always going to be there!

Dr. Lambert: …I have never seen you say something in the middle of the class any other time, but it is like you got mad about it because of the idea…

Rita: Cause like, you are always going to have the letter because the letter always equals something, and then if you forget about it, at the end of the whole thing, it is going to be like, {oh what does the letter mean} equals nothing apparently.

We notice that not only is this an emotional response to a pedagogical choice made by the teacher but that Rita ends with humor, that the variable will “equal nothing apparently.” Rita demonstrates a strong mathematical understanding of variables here—they have values, and the whole point of algebra is understanding what the variable means. The letter in the mathematical equation here served as an emotion implicative what and can be understood through the concept of a sticky object [35]—a thing, person, or place that involves emotional significance due to the political, historical, cultural, and economic interaction between the thing, person, or place. Elsewhere, we have argued that learning disabilities are ideological and sticky objects [43] by exploring the idea of LD and being labeled with LD. Rita here also further shows the stickiness of learning, in this case, mathematics, as it relates to specific mathematics-domain information that is salient to the solving of mathematical calculations. We see the results of such stickiness for Rita in her use of the term, “forget” as it relates to the letter in the mathematical equation, as another emotion implicative what, since the sociocultural experience of forgetting is emotion-laden. Rita ends with communicating the salience of the letter, which forgotten, would be detrimental to solving the equation and in turn, produce either a positive emotional experience afterward or a negative one due to the fact that not knowing the letter leads to, as Rita stated, “nothing apparently.”

3.3. Narratives of Transformation: Rita “I Just Don’t Like It”

In her first interview in seventh grade, Rita begins by establishing her competence in mathematics, just as she had done with the first author several times during class, telling the first author that “for me, I have always been good in math.” She attributed this to her mother, saying, “I think I got it from my mom, because my mom is good in math.” The story begins in certainty, what is really known. Rita understands herself as “always good at math.” She is confident about this, strongly stating this in interviews and in impromptu moments during class. She confidently placed herself at one pole of an important dualism in how kids make sense of mathematics: being good or bad at it.

She then describes a shift in her feelings towards mathematics: “But now I am just, I don’t know, I just stopped liking math.” Rita’s narrative then transitions and becomes filled with uncertainty, manifested in the repetition of “I don’t know,” which forms a contrast with the certainty that opens the narrative. It is critical when analyzing the development of narrative to pay attention to moments like “I don’t know.” These phrases mark disjunction, confusion, and emergent meaning making. It can help us find the moments of transition, of shifting beliefs. Rita does not say that she is no longer “good at math,” but that her emotional connection to the subject has changed. It is a statement focused on her role in this story, filled with her agency alone.

In the final interview with Rita in June, the first author asked her how her family saw her as a mathematics learner and heard a similar, but slightly different narrative.

Dr. Lambert: What about your family, what kind of a **math learner do they think you are?

Rita: They think **I’m good [nods]. **I got it from my mom.

Dr. Lambert: Your mom enjoys doing math?

Rita: She’s really good at math, and I’m just like, she helps me sometimes, like [unclear] and [unclear] so that’s how I became really good in math, because of my mom, and I used to love math, and now I tell her that I hate it, and she is like [Why] She gets upset.

Dr. Lambert: What does she do when you say that? She gets upset?

Rita: Yeah she does, like “I’m really good at it, and you just don’t like it?” She’s like What happened to you I’m like I don’t like it.

There is tension in this narrative between binaries that too often creep into children’s narratives about mathematics: loving and hating mathematics, being good at mathematics, and the usually unnamed opposite. Rita’s narratives are squarely focused on processes of identification, on emotionally charged words about mathematics: “I hate it, I used to love it, she gets upset”. Here, in the second narrative, there are no more refrains of “I don’t know.” Now Rita is clear: “I don’t like it.”

The last stanzas of the narrative, where Rita performed the dialogue between herself and her mother about this shift, is full of voices, unresolved conflict, and speaks to the unfinished nature of identities, the critical role of relationships, mediated by language and emotions. The question of whether or not Rita liked mathematics is a critical one at home and at school. How Rita defined herself in relation to mathematics matters. Her mother has made an identity claim in mathematics that her daughter was rejecting, even as Rita seemed to agree, “I got it from my mom.” Rita provides an example of how her understanding of self as a mathematics learner is refracted through her relationships with others.

We also notice that the strongest words—“I hate it”—are reserved for interactions with her mother. Our histories as teenagers enter into the analysis—the first author remembers saying “I hate math” to her own mother in fifth grade, something she would have never said to another adult, certainly never to a teacher. For the first author, and we suspect for Rita, this dialogue with her mother is a critical site of identity development around mathematics.

Rita followed this story about her mother with an exploration of what gets harder, as the first author continued pressing for her to explain this shift to her. Rita again emphasized that “I don’t know how to explain it,” but then finds this small moment in her class to narrate.

Dr. Lambert: But you know, she has the same question that I have for you. We really wanna know. Right?

Rita: She …I don’t know how to explain it. It’s just getting harder, like I could take it, but then somethings I am just oh God, like Ms. P. heard me saying to Mack that he was getting a panic attack about it, Sometimes I’m like oh my god what is she talking about, especially when it is like a whole big line the integers thing, from here to here, it is like really long, I’m just like [shocked face] like I forget the rules or I don’t use them so I’m like freaked out.

Rita’s talk here is emotional, layered with her feelings, as indicated by the coding of her emotional talk. It is not as if Rita has no feelings any more about mathematics, she has strong negative feelings: “panic,” and “freaked out.” This is not a narrative about mathematics being boring— it is intensely experienced. The fact that her seventh-grade mathematics class is harder seems assumed. Rita assured the first author that “I could take it” —she can endure what must be endured, not enjoyed. What interferes with her ability to “take it” are moments that make her (and her peers) panic: “a whole big line the integers thing from here to here.” Rita makes several points here about “harder.”

Harder was closely connected, here and at other points, with a mathematics of procedures: remembering rules and difficult procedures. When Rita sees the long line of integers to add or subtract on the board, she narrates an immediate response, panic about which rules she might have to use, and whether or not she is going to be able to remember them. Panic and forgetting are closely related in the experience of the kids in mathematics. For Rita, this shift to “not liking mathematics” was outside herself. It was a change in her mathematics class, not a change in herself. It got harder. Rita very clearly narrates what this “harder” is. It is a long string of integers to compute, the “panic attack” kids feel when faced with this, “I’m freaked out.” The change in pedagogy, from discussion-based mathematics to procedural mathematics is what makes mathematics “harder.” We see this in the description of integers as connected to the critical incident in the fieldnotes. Mathematics is getting harder because her desire for meaning is being ignored.

In the narratives she presents, Rita is not turning off to mathematics because of a perception about girls and mathematics, or Latinas and mathematics; in fact, her own mother is a role model for positive identification with mathematics. Rita turns off to mathematics because of how it is now done in her classroom—individualistic memory performances. Rita’s mom wants to know why Rita does not like mathematics, as does the first author. When Rita’s mom asks, “what happened to you?” she is not asking because Rita’s grades have fallen; in fact, she still has As in her mathematics class. She is asking what is happening to her daughter’s identification with mathematics. Rita’s response, analyzing the narratives as a whole, is how mathematics class feels. For Rita, the absolute worst moment, this one, the line of integers and her resultant panic attack is absolutely related to the performance of memory that she must do to continue to be a viable serious student. This moment is terrifying. The turn from discussion-based to procedural mathematics means not only a turn from one kind of thinking to another kind of thinking, all done alone in the mind of an individual kid, it means a turn from collaborative meaning-making to individualist memory performances: a new figured world not hinged on relationship building and meaning-making.

4. Conclusions

Embodiment in mathematics has long theorized mathematical cognition without the inclusion of emotion as part of that embodied experience. Feelings of the mind and the body exist in tandem, requiring exploration, not separation. Complex Embodiment [6] reminds us that social positioning occurs both outside and inside our bodies. Here we develop our theory of how this matters in mathematics classrooms. Our research question asked: How does emotion matter in the mathematical identity processes of a Latina with a learning disability? We found three ways in which emotion played a role in her identity processes. First, Rita is positioned not only through deficit language around her labels of multilingual learner and student with a disability but in a particularly emotional way. Her struggle is narrated as an emotional struggle. Second, there are two narratives of transformation in the data, and both are emotional. We have particularly strong data from Rita’s perspective about how the emotional process of disidentifying with mathematics is related to disidentifying with her mother. Finally, we hear in her narratives a desire for mathematical meaning, and an emotional response to mathematics as a subject she needs to endure rather than enjoy. We will describe each in turn.

We view emotion as critical to how Rita is positioned by her sixth-grade teachers. She is described as an “uber special-ed kid” who “cried on her way to school” and was “always upset.” Her bilingualism is described emotionally, that Rita felt afraid to speak in English. We have no way of knowing how accurate this description is of Rita’s emotional state and behavior. We can, however, see how these two teachers saw a struggling student as emotional, rather than simply lacking skills. Application of negative affectivity has historically served as a tool of white patriarchy to disparagingly label and displace individuals from marginalized communities, including women, especially Black women [49,50,51]. Evidence suggests that framings such as these are disproportionately applied to students of color, including multilingual learners [52]. Emotion is therefore important in capturing how adults position students. An alternative framing is that her teachers see her emotional state as the most important factor in her engagement in school. These teachers certainly did not focus on her cognitive skills, but on her emotional state.

Rita’s first transformation in the data, narrated by her sixth-grade teachers, is from the emotional “uber SPED kid” to a strong, confident student. According to her teachers, this was a highly relational and embodied shift, in which Rita took up the ways of being of her friend. Mr. Pierce narrates that the two girls become one, rising together. In doing so, Rita became a good student and is now “smart” by association. This was not the only example in the data that displayed students learning mathematics in close relation to their friends. Rita’s friends, Shaundra and Carmen, in sixth and seventh grade, respectively, are at the forefront of her learning mathematics and her relationship with mathematics. Additionally, her relationship with her Latina seventh grade teacher is seen mediating her learning and relationship with mathematics. We see that Rita’s construction of her mathematical identity is influenced by female relationships.

The second transformation in the data is Rita’s gradual shift away from identifying with mathematics. While Rita’s teachers accredit her mathematical success to her relational growth alongside Shaundra, Rita’s mother has positioned Rita’s mathematical identity alongside her own. Through Rita’s re-enactment of their dialogue, we know that her mother is in the words of Rita, “good at mathematics” and because of her, “that’s how I became really good in mathematics”. Additionally, Rita discloses that she used to love mathematics because of her mom. Rita’s experiences in her sixth-grade classroom are reinforced by the strong mathematical identity she has adopted from her mother, when she “used to love mathematics”. However, as she enters seventh grade and shares with her mom that she hates mathematics, her mother gets upset. Rita’s experience in the classroom is repositioning their relationship regarding their shared love for mathematics. While we do not know how they move forward, it is certain that the emotional levers between mom, mathematics, and her own identity are closely tied to one another. How she understands herself as a learner is intimately related to her relationship with her mother. Relationships matter both in how she is positioned and in transformations of who she is as a student (from the teachers’ perspective). This pull away from mathematics is experienced as a pull away from her mother, and leaves them both “upset.”

What prompted this move away from mathematics? Rita returns multiple times to the theme of “it gets harder” in terms of her mathematics class. She connects this “harder” not necessarily to the material just being more challenging, but her relationship to the meaning of the material shifting. The narrative is not that mathematics stayed the same, but was more challenging. Instead, the narrative is that the “harder” was a mathematics increasingly disconnected to meaning-making. This kind of mathematics, without meaning, provokes “panic” in Rita. She assures the interviewer that she “can take it,” but why? This shift is emotional, experienced as strong emotions by Rita. As found by other researchers in how women make a place for themselves in mathematics [53], Rita must make sense of conflicting discourses about her place in the world of mathematics. Other studies of gender in mathematics have found high school girls moving away from mathematics as it became less about connected knowledges [54].

Finally, intersectionality matters in her mathematical identity development as well. In this study, we bring attention to Rita’s positioning as broken and non-normative through both her ELL and LD classifications. While bilingualism is generally framed as an asset with white students [55], falling “more between English and Spanish” is shorthand for a problem, an immediate deficit for Latinx students. In the story told by the teacher, as Rita becomes more like Shaundra, a student not labeled as ELL or having an IEP, she becomes better. They see Rita as transcending these deficit-laden markers, having moved beyond her disability and language use. We question this framing of becoming a “good student” as she leaves behind her bilingualism and disability. Bilingualism is complex and dynamic [56], where emergent bilinguals engage in translanguaging practices, moving between languages. By centering Rita’s voice, or testimonio, as researchers we are offered a glimpse into a complex and shifting figured world of mathematical identity. By traditional measures, Rita can be described as having a strong mathematical identity; she receives high grades and is often used as a resource by her own peers. Prior to this transformation (in the eyes of her teachers) she is a Latina with a learning disability and emotional irregularities. Her testimonios illustrate a far more nuanced counter-narrative, one easily overshadowed by traditional majoritarian narratives. Therefore the shift in power is marked by our own shift in methodology—the intersectional lens.

A note on methodology. Narratives are not novel when it comes to identity work in mathematics scholarship, but often overlooked is the nuance of emotion and where it surfaces between embodiment in mathematics (found in observations) and identity in mathematics (found in interviews). Our use of mathematics narratives to make connections between how mathematics is embodied and how identity in mathematics is expressed contributed to a more complex understanding of Rita’s mathematical identity processes. Through observations and interviews we were able to see how Rita was positioned in terms of her race, disability, and gender, and the way social relationships emerged.

Finally, how does this analysis further our theorizing of complex embodiment in mathematics? We have outlined how the experience of mathematics learning and identification are embodied for Rita. We see how relationships and emotions are central to her mathematical experience. We can note how teachers position Rita through embodied discourses that include labels, and also a narrative of the struggling student as emotional. And, particularly through her narratives of disidentification, Rita authors herself as moving away from mathematics because of the experience, the feeling, of mathematics no longer being about sense-making. This is the dialogical, iterative process of complex embodiment. We experience mathematics, and then we make sense of it using the cultural practices that circulate.

5. Implications

This study has implications for research on ways of using mathematics emotion narratives to make connections between how mathematics is embodied and how identity in mathematics is understood through intersectionality. Teachers’ explicit and/or implicit perceptions of students’ emotional state surrounding mathematical identity and performance have an influence on how students perceive and embody mathematics. This highlights the importance of understanding how emotions are embodied and has particular implications for training secondary teachers to be cognizant and sensitive of the impact of pedagogical practices on their students’ academic, social, and emotional identity development. More research is needed on how families influence the academic, social, and emotional identity development process and embodiment of mathematics for Latina adolescents with and without dis/Abilities. However, foregrounding an explicit Disability Studies approach to the academic, social, and emotional development of students of color with and without mathematics-based dis/Abilities is generative of paradigmatic shifts in praxis, methodology, and theory for guaranteeing equal educational opportunities to learn for students. This approach not only accounts for the technical dimensions of academic, social, and emotional human development contexts but also the contextual (e.g., people, herstories, backgrounds, local, national, and global histories) and critical (e.g., identities, difference, intersectionality, power, emotionality, etc.) aspects. A Disability Studies approach would provide the field of mathematics a rich praxical, methodological, and theoretical framework from which to generate new ways of feeling and thinking for mathematics teacher educators and in-service and pre-service mathematics teachers to develop the skills, knowledge, and dispositions necessary for reframing pedagogical practices that center student voices [57], but more importantly emotionality, relationality, and intersectionality. Such a reframing has the potential to revise what the mathematics classroom feels, sounds, and looks like for affective intersectional dis/Ability justice [43,58] for ALL at their intersections of power and identities in education contexts.