How Many Fractional Derivatives Are There?
Abstract
:1. Introduction
- We work on .
- We use the two-sided Laplace transform (LT):where is any function defined on and is its transform, provided that it has a non empty region of convergence (ROC).
- The Fourier transform (FT), , is obtained from the LT through the substitution with
2. What Is a Fractional Derivative?
- P1
- LinearityThe operator is linear.
- P2
- IdentityThe zero order derivative of a function returns the function itself.
- P3
- Backward compatibilityWhen the order is integer, FD gives the same result as the ordinary derivative.
- P4
- The index lawholds for and .
- P5
- The generalized Leibniz ruleholds. As is clear, when we obtain the classical Leibniz rule.
- P’4
- The generalized index lawholds for any and .
- The anti-derivative is unique.
- The anti-derivative is a left and right inverse, while any primitive is only right inverse.
3. Unified Fractional Derivative
- Fourier transformation
- EigenfunctionsLet Then,meaning that the sisoids are the eigenfunctions of the UFD with eigenvalue
- Periodicity inThe UFD is periodic in with period 4as we observe from (2).
- Additivity and commutativity of the orders
- Existence of inverse derivativeFrom (13), the anti-derivative exists when and . Therefore,
- Identity operator
- forward derivative,
- backward derivative,
- Riesz derivative and potential,
- Feller derivative and potential,
- Hilbert transform, ,
- choosing particular values of the parameters and ,
- restricting the domain of the function at hand.
4. One-Sided Derivatives
4.1. The GL Derivatives
4.2. The Impulse Response
4.3. Liouville’s Derivatives
- 1.
- These derivative definitions are equivalent for functions with LT or FT.
- 2.
- For some particular classes of functions this may not be correct, e.g., the L derivatives are better than the LC. Possible causes for this are:
- The convolution makes the functions smoother;
- The derivative may introduce roughness or spikes.
- 3.
- These 2 + 2 formulations lead to most derivatives described in [7,8]. We must reinforce something very important: all the above defined derivatives are valid for functions defined in any interval of . This means that we do not need to change the definitions to accommodate them to the domain of the function at hand. One thing is the definition, another one is the computation of the derivative. It is a situation similar to the one we find in the LT or FT. We do not need to change the definitions to agree with the domain of the function. Therefore, most derivative definitions in [7,8] have no reason to be considered as autonomous derivatives.
- 4.
- These derivatives do not introduce any initial conditions.
- 5.
- For a given derivative, there is always an anti-derivative.
4.4. RL and C Derivatives
- Riemann–Liouville (RL) derivativesand
- (Dzherbashian-)Caputo (C) derivativesand
4.5. Multistep Derivatives
4.6. Second Generation Operators
5. Pseudo-Fractional-Derivatives
5.1. Some Comments
5.2. “Derivatives” That Are High-Pass Filters
5.3. “Disguised” Order 1 Derivatives
6. Which Derivatives?
- In space problems, we can use the above formulæor the corresponding right-side versions, if there is any privileged direction. If this does not happen, we must use a two-sided derivative, preferably (2).
Author Contributions
Funding
Conflicts of Interest
References
- Dugowson, S. Les Différentielles Métaphysiques (Histoire et Philosophie de la Généralisation de l’ordre de Dérivation). Ph.D. Thesis, Université Paris Nord, Paris, France, 1994. [Google Scholar]
- Liouville, J. Memóire sur le calcul des différentielles à indices quelconques. J. L’École Polytech. Paris 1832, 13, 71–162. [Google Scholar]
- Liouville, J. Memóire sur quelques questions de Géométrie et de Méchanique, et sur un nouveau genre de calcul pour résoudre ces questions. J. L’École Polytech. Paris 1832, 13, 1–69. [Google Scholar]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives; Gordon and Breach: Yverdon, Switzerland, 1993. [Google Scholar]
- Podlubny, I. Fractional Differential Equations: An Introduction to Fractional Derivatives, Fractional Differential Equations, to Methods of Their Solution and Some of Their Applications; Academic Press: San Diego, CA, USA, 1999; pp. 1–3. [Google Scholar]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; Elsevier: Amsterdam, The Netherlands, 2006. [Google Scholar]
- De Oliveira, E.C.; Tenreiro Machado, J.A. A review of definitions for fractional derivatives and integral. Math. Probl. Eng. 2014, 2014, 238459. [Google Scholar] [CrossRef] [Green Version]
- Teodoro, G.S.; Machado, J.T.; De Oliveira, E.C. A review of definitions of fractional derivatives and other operators. J. Comput. Phys. 2019, 388, 195–208. [Google Scholar] [CrossRef]
- Ortigueira, M.D.; Machado, J.T. What is a fractional derivative? J. Comput. Phys. 2015, 293, 4–13. [Google Scholar] [CrossRef]
- Ortigueira, M.D.; Machado, J.A.T. Which Derivative? Fractal Fract. 2017, 1, 3. [Google Scholar] [CrossRef]
- Ortigueira, M.D.; Machado, J.A.T. Fractional Derivatives: The Perspective of System Theory. Mathematics 2019, 7, 150. [Google Scholar] [CrossRef] [Green Version]
- Ortigueira, M.D. Two-sided and regularised Riesz-Feller derivatives. Math. Methods Appl. Sci. 2021, 44, 8057–8069. [Google Scholar] [CrossRef]
- Ortigueira, M.D.; Bengochea, G.; Machado, J.A.T. Substantial, tempered, and shifted fractional derivatives: Three faces of a tetrahedron. Math. Methods Appl. Sci. 2021, 44, 9191–9209. [Google Scholar] [CrossRef]
- Ortigueira, M.D.; Bengochea, G. Bilateral Tempered Fractional Derivatives. Symmetry 2021, 13, 823. [Google Scholar] [CrossRef]
- Ortigueira, M.D. Fractional Calculus for Scientists and Engineers; Lecture Notes in Electrical Engineering; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Bohner, M.; Peterson, A. Dynamic Equations on Time Scales: An Introduction with Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2001. [Google Scholar]
- Ortigueira, M.D.; Valério, D. Fractional Signals and Systems; De Gruyter: Berlin, Germany, 2020. [Google Scholar]
- Ortigueira, M.D.; Machado, J.A.T. New discrete-time fractional derivatives based on the bilinear transformation: Definitions and properties. Recent Advances in the Fractional-Order Circuits and Systems: Theory, Design and Applications. J. Adv. Res. 2020, 25, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Liouville, J. Note sur une formule pour les différentielles à indices quelconques à l’occasion d’un mémoire de M. Tortolini. J. Math. Pures Appl. 1855, 20, 115–120. [Google Scholar]
- Herrmann, R. Fractional Calculus, 3rd ed.; World Scientific: Singapore, 2018. [Google Scholar]
- Grünwald, A.K. Ueber “begrentz” Derivationen und deren Anwendung. Z. Math. Phys. 1867, 12, 441–480. [Google Scholar]
- Letnikov, A. Note relative à l’explication des principes fondamentaux de la théorie de la différentiation à indice quelconque (A propos d’un mémoire). Mat. Sb. 1873, 6, 413–445. [Google Scholar]
- Riemann, B. Versuch einer allgemeinen Auffassung der Integration und Differentiation. In Gesammelte Werke; Cambridge University Press: Cambridge, UK, 1876; Volume 62. [Google Scholar]
- Rudolf, H. Applications of Fractional Calculus in Physics; World Scientific: Singapore, 2000. [Google Scholar]
- Ortigueira, M.D.; Machado, J.T. Revisiting the 1D and 2D Laplace transforms. Mathematics 2020, 8, 1330. [Google Scholar] [CrossRef]
- Wang, J.L.; Li, H.F. Surpassing the fractional derivative: Concept of the memory-dependent derivative. Comput. Math. Appl. 2011, 62, 1562–1567. [Google Scholar] [CrossRef] [Green Version]
- Oppenheim, A.V.; Willsky, A.S.; Hamid, S. Signals and Systems, 2nd ed.; Prentice-Hall: Upper Saddle River, NJ, USA, 1997. [Google Scholar]
- Kolwankar, K.M.; Gangal, A.D. Local fractional calculus: A calculus for fractal space-time. In Fractals; Springer: Berlin/Heidelberg, Germany, 1999; pp. 171–181. [Google Scholar]
- Tarasov, V.E. No nonlocality. No fractional derivative. Commun. Nonlinear Sci. Numer. Simul. 2018, 62, 157–163. [Google Scholar] [CrossRef] [Green Version]
- Khalil, R.; Al Horani, M.; Yousef, A.; Sababheh, M. A new definition of fractional derivative. J. Comput. Appl. Math. 2014, 264, 65–70. [Google Scholar] [CrossRef]
- Chen, W. Time–space fabric underlying anomalous diffusion. Chaos Solitons Fractals 2006, 28, 923–929. [Google Scholar] [CrossRef] [Green Version]

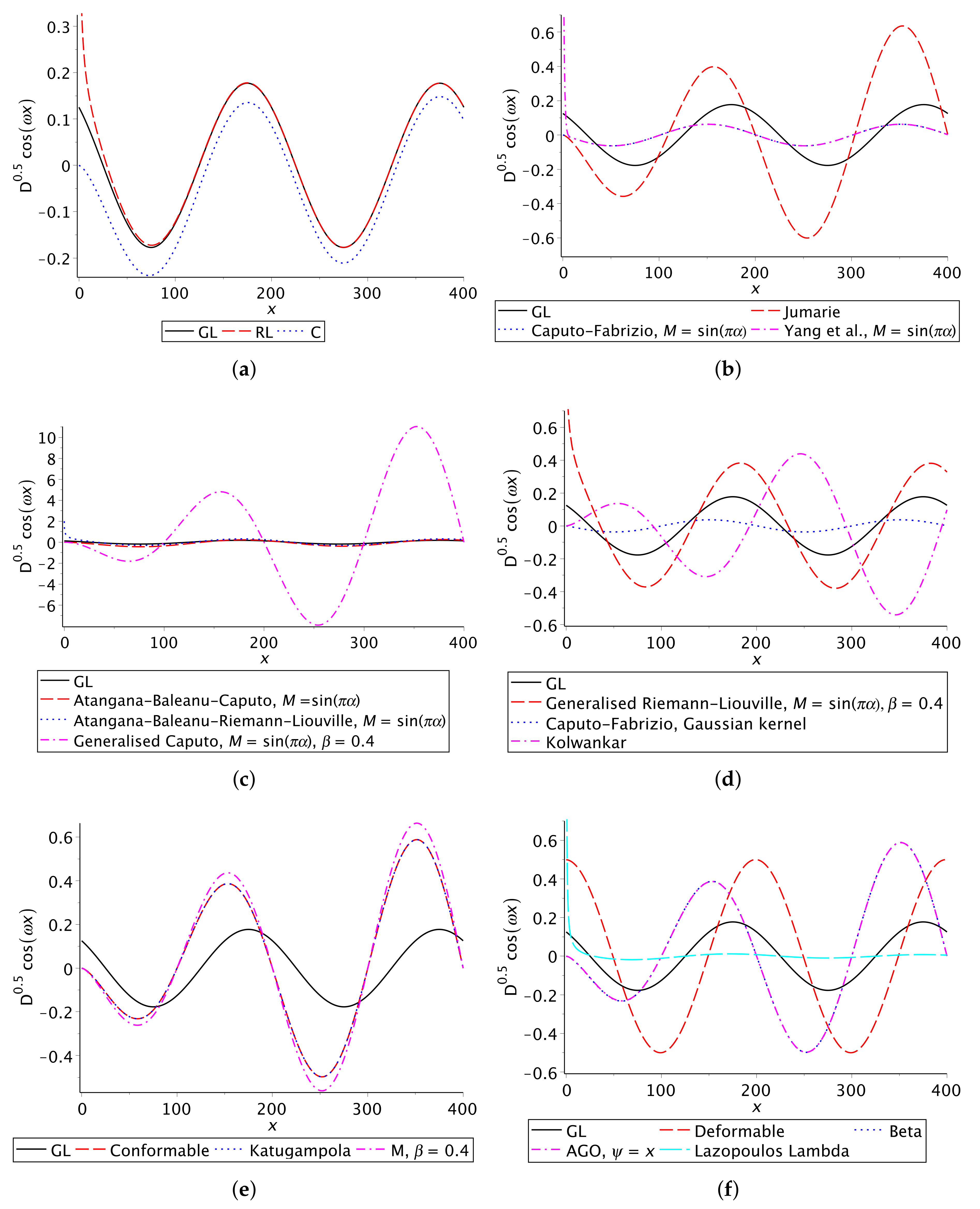

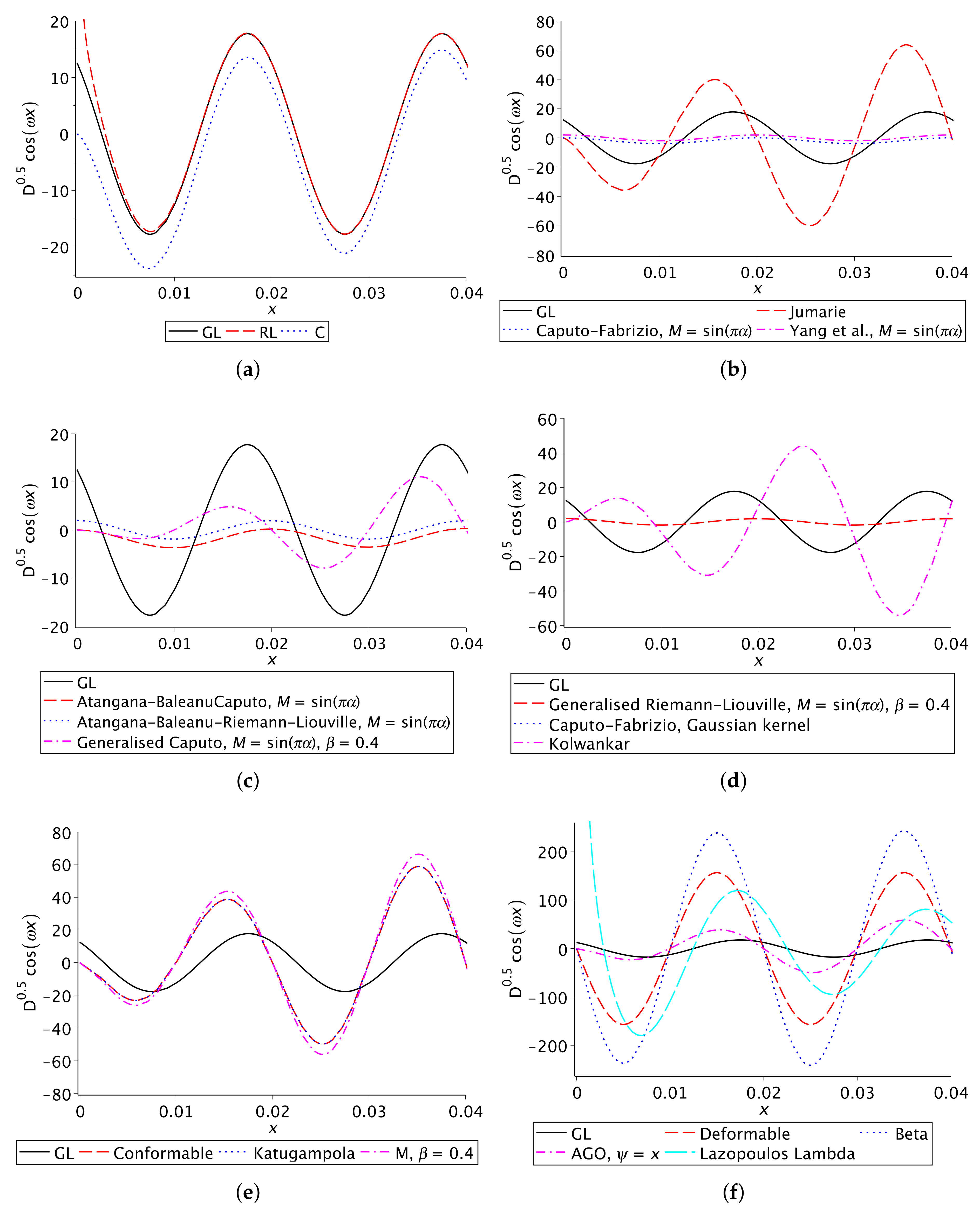
| Name | Definition | Parameters | Domain | |
|---|---|---|---|---|
| L | ||||
| LC | ||||
| GL | ||||
| Name | Definition | Parameters | Domain | |
|---|---|---|---|---|
| RL | ||||
| C | ||||
| GL | ||||
| Name | Definition | Domain |
|---|---|---|
| Hadamard | ||
| Quantum | ||
| Marchaud | ||
| Jumarie | ||
| Erdélyi-Kober | ||
| k-Hilfer | ||
| Hilfer-Katugampola | ||
| -Hilfer, with | ||
| Name | Definition | Domain |
|---|---|---|
| Caputo-Fabrizio | ||
| Yang et al. | ||
| Atangana-Baleanu-Caputo | ||
| Atangana-Baleanu-Riemann–Liouville | ||
| Generalized Caputo | ||
| Generalized Riemann–Liouville | ||
| Caputo-Fabrizio, Gaussian kernel | ||
| Sun-Hao-Zhang-Baleanu |
| Name | Definition | Domain |
|---|---|---|
| Kolwankar | where is the RL derivative | |
| Chen | ||
| Conformable | ||
| Katugampola | ||
| M | ||
| Deformable | ||
| Beta | ||
| AGO | ||
| Generalized | ||
| General conformable | ||
| Lazopoulos’s Lambda |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Valério, D.; Ortigueira, M.D.; Lopes, A.M. How Many Fractional Derivatives Are There? Mathematics 2022, 10, 737. https://doi.org/10.3390/math10050737
Valério D, Ortigueira MD, Lopes AM. How Many Fractional Derivatives Are There? Mathematics. 2022; 10(5):737. https://doi.org/10.3390/math10050737
Chicago/Turabian StyleValério, Duarte, Manuel D. Ortigueira, and António M. Lopes. 2022. "How Many Fractional Derivatives Are There?" Mathematics 10, no. 5: 737. https://doi.org/10.3390/math10050737
APA StyleValério, D., Ortigueira, M. D., & Lopes, A. M. (2022). How Many Fractional Derivatives Are There? Mathematics, 10(5), 737. https://doi.org/10.3390/math10050737








