Evaluation and Mathematical Analysis of a Four-Dimensional Lotka–Volterra-like Equation Designed to Describe the Batch Nisin Production System
Abstract
:1. Introduction
2. Results and Discussion
2.1. Design of the Four-Dimensional Equation
- (i)
- The culture pH (pH(t)) decline could be described as the difference between the buffer capacity of the medium [19] and the decrease in pH due to lactic acid production by the biomass as follows:
- (ii)
- (iii)
- Biomass (X(t)) could be considered as one predator that grows logistically competing with nisin for the nitrogen source and depending on the culture pH and the TN source concentration [5], as follows:
- (iv)
- Nisin (BT(t)) could be considered as the second predator that is produced by the biomass but competes with it for the nitrogen source and depends on the culture pH and the TN source concentration [5], as follows:
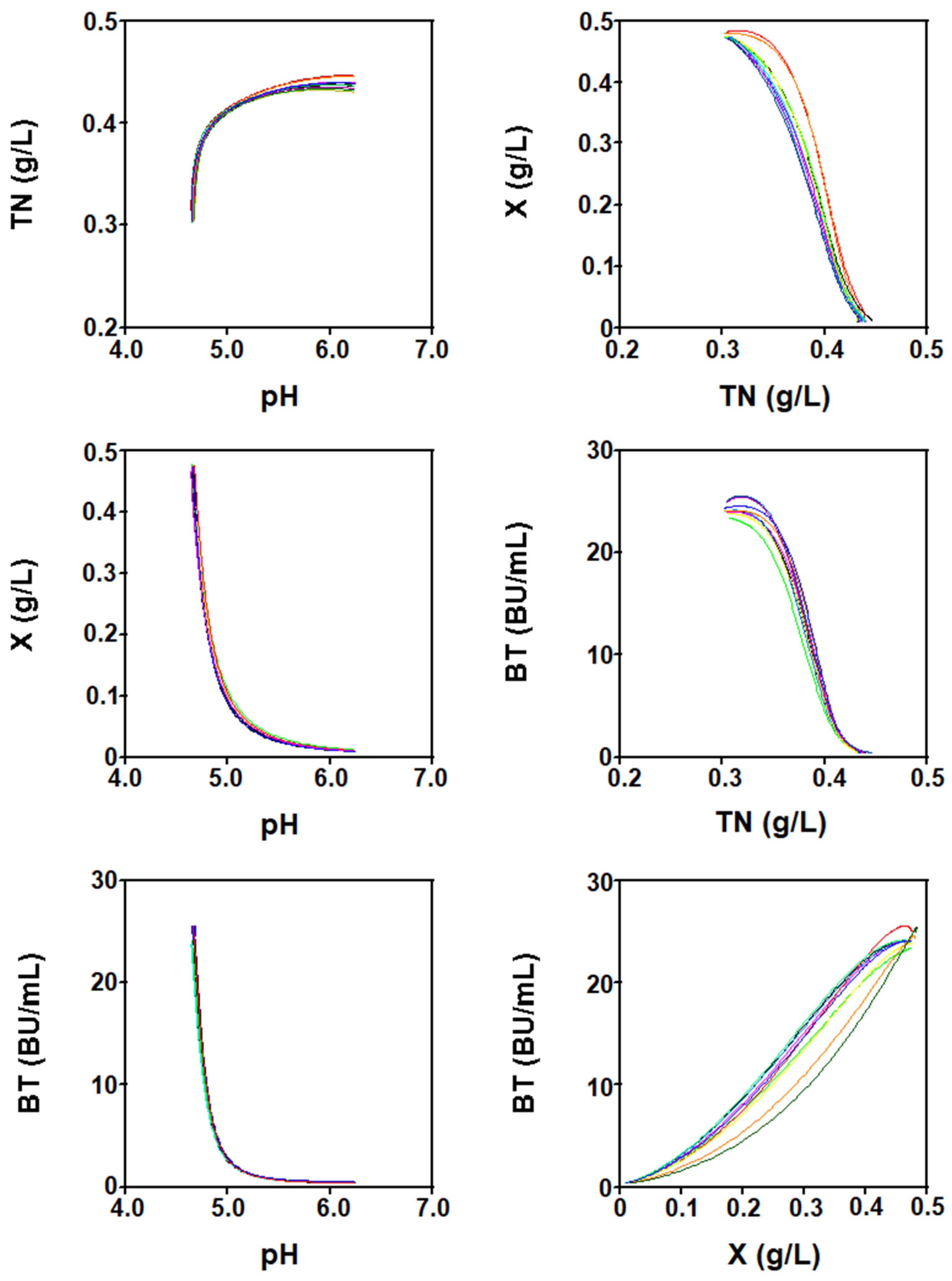
2.2. Modeling the Batch Nisin Production System in Different Series of Fermentations in DW Media Using a Global Set of Model Parameters
2.3. Modeling the Batch Nisin Production System in Individual Cultures Corresponding to Each Series of Fermentations
2.3.1. Series of Fermentation DW-G
2.3.2. Series of Fermentation DW-TS-TP
2.3.3. Series of Fermentation DW-MRS
2.4. Mathematical Analysis of the Four-Dimensional Lotka–Volterra Equation
2.4.1. Generalized Four-Dimensional Lotka–Volterra Equation
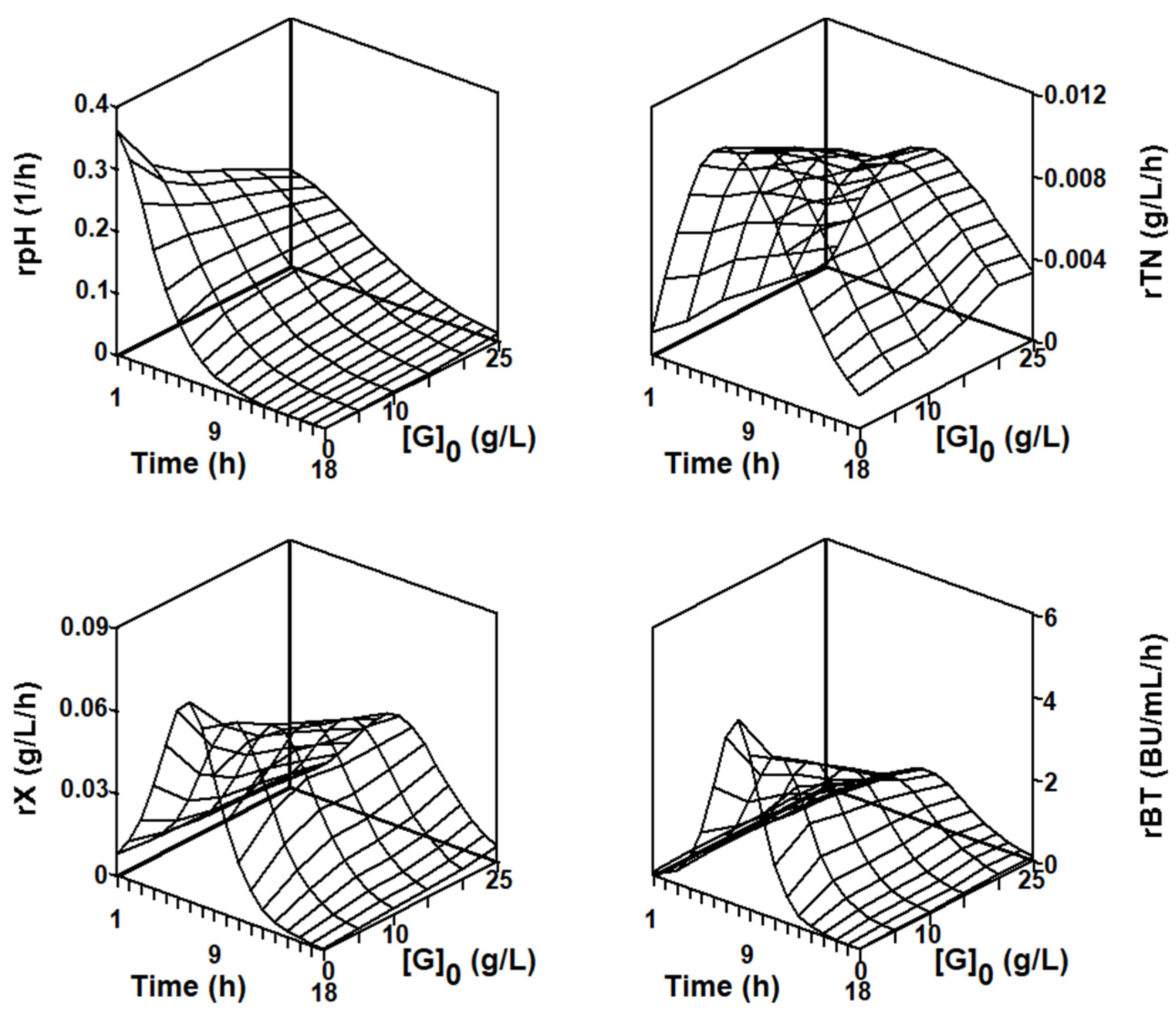
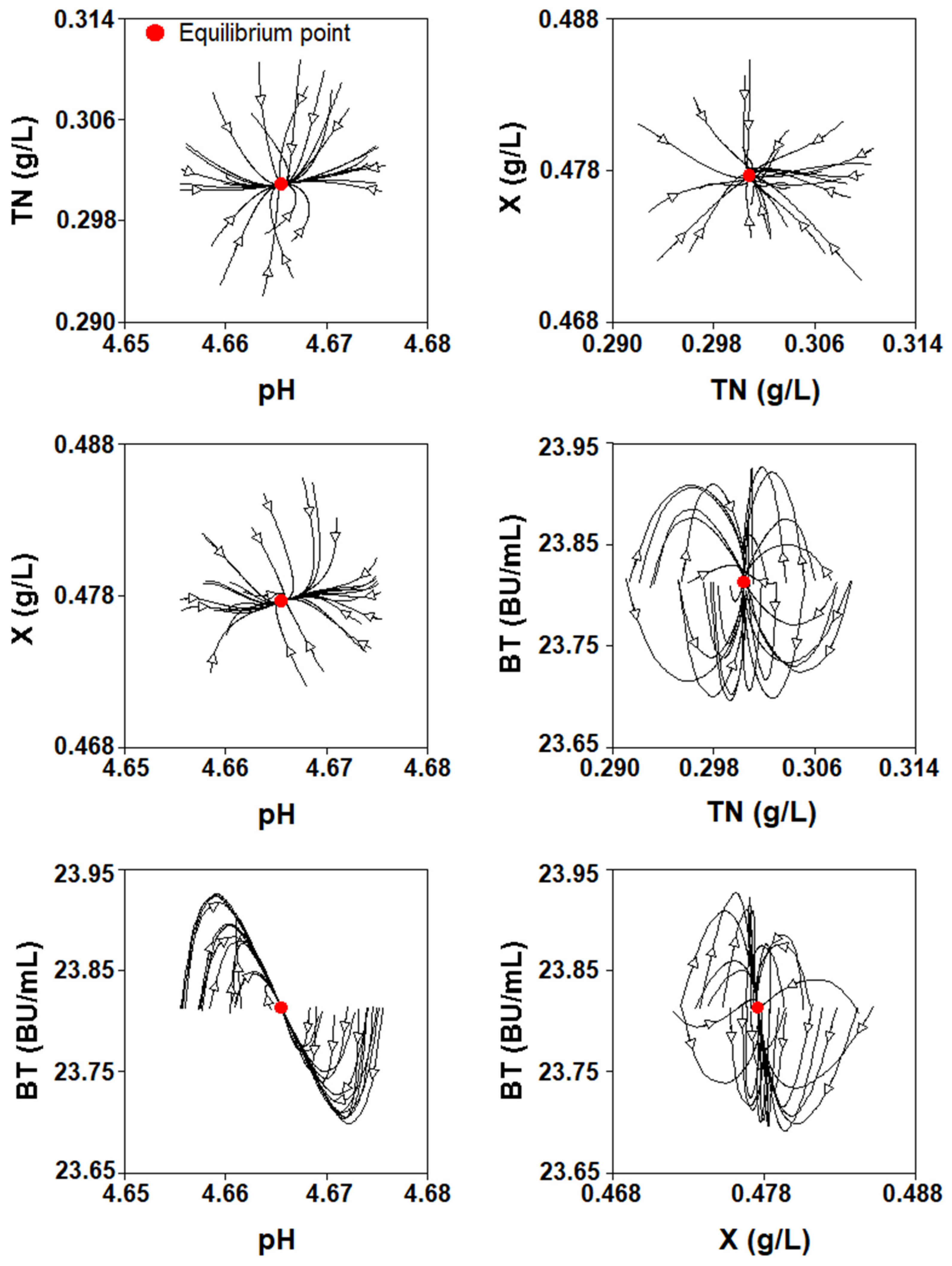
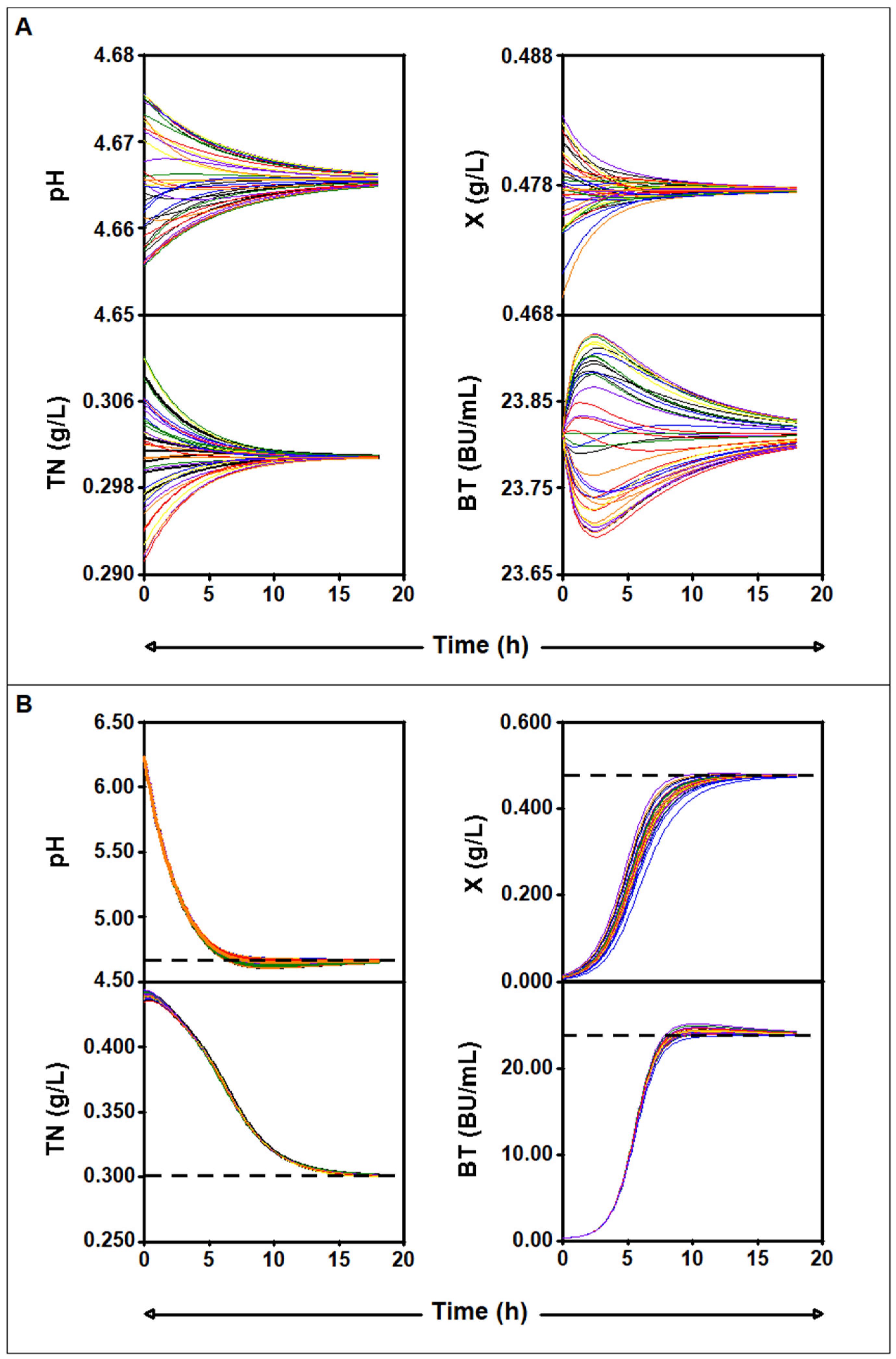
2.4.2. Numerical Analysis
2.4.3. Study of the Parameter Values for Which There Is an Asymptotically Stable Solution
3. Materials and Methods
3.1. Microorganisms, Culture Media, and Inoculum Preparation
3.2. Batch Cultures
3.3. Analytical Methods
3.4. Statistical Significance of the Parameters and Equation
3.5. Mathematical Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| t | Time (h) |
| pH(t) | Culture pH value over the time |
| pH0 | Initial culture pH value |
| pHf | Final culture pH |
| a | Intrinsic pH drop rate (h−1) |
| b | Quotient between the intrinsic pH drop rate and the theoretical minimum pH value for growth (h-1) |
| c | Constant that represents the effect of biomass production on pH time course (L/g/h) |
| TN(t) | Total nitrogen concentration (g/L) over the time |
| TN0 | Initial total nitrogen concentration (g/L) |
| TNf | Final total nitrogen concentration (g/L) |
| d | Intrinsic TN consumption rate (h−1) |
| e | Quotient between the intrinsic TN consumption rate and the theoretical maximum TN concentration that biomass can consume (L/g/h) |
| f | Intrinsic TN consumption rate (L/g/h) for biomass production |
| g | Intrinsic TN consumption rate (mL/BU/h) for nisin production |
| h | Constant that represents the effect of pH time course on TN consumption (h−1) |
| X(t) | Biomass concentration (g/L) over the time |
| X0 | Initial biomass concentration (g/L) |
| Xmax | Maximum biomass concentration (g/L) |
| i | Intrinsic growth rate (h−1) |
| j | Quotient between the intrinsic growth rate and the theoretical maximum biomass concentration that system can support (L/g/h) |
| k | Efficiency of TN utilization (mL/BU/h) to be channeled into cells of L. lactis rather than nisin (competition coefficient) |
| l | Constant that represents the effect of pH time course on the growth (h−1) |
| m | Constant that represents the effect of TN time course on the growth (L/g/h) |
| BT(t) | Nisin concentration (BU/mL) over the time |
| BTmax | Maximum nisin concentration (BU/mL) |
| n | Intrinsic nisin production rate (h−1) |
| o | Quotient between the intrinsic nisin production rate and the theoretical maximum nisin concentration that biomass can produce (h−1) |
| p | Efficiency of TN utilization (mL/BU/h) to be channeled into nisin rather than cells of L. lactis (competition coefficient) |
| q | Constant that represents the effect of pH time course on nisin synthesis (h−1) |
| r | Constant that represents the effect of TN time course on nisin synthesis (L/g/h) |
| A, B, C0, C1, C2 | Constants in Equation (5) |
| D, E, F | Constants in Equation (6) |
| G, H, I | Constants in Equation (7) |
| [G]0 | Initial glucose concentration (g/L) |
| [Nut]0 | Initial concentration of MRS broth nutrients (g/L) |
| RPDM | Mean relative percentage deviation modulus |
| Bf | Bias factor |
| Af | Accuracy factor |
| s | Number of experimental data |
| Yexpi | Experimental values of culture pH and concentration of total nitrogen, biomass, and nisin |
| Ypredi | Predicted values by the corresponding equation for culture pH and concentration of total nitrogen, biomass, and nisin. |
| R2 | Correlation coefficient |
References
- Delves-Broughton, J. Nisin. In Encyclopedia of Food Microbiology, 2nd ed.; Robinson, R., Batt, C.A., Tortorello, M.L., Eds.; Academic Press: London, UK, 2014; pp. 187–193. [Google Scholar]
- Leroy, F.; De Vuyst, L. Growth of the bacteriocin-producing Lactobacillus sakei strain CTC 494 in MRS broth is strongly reduced due to nutrient exhaustion: A nutrient depletion model for the growth of lactic acid bacteria. Appl. Environ. Microbiol. 2001, 67, 4407–4413. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- van Impe, J.F.; Poschet, F.; Geeraerd, A.H.; Vereecken, K.M. Towards a novel class of predictive microbial growth models. Int. J. Food Microbiol. 2005, 100, 97–105. [Google Scholar] [CrossRef] [PubMed]
- Wachenheim, D.E.; Patterson, J.A.; Ladisch, M.R. Analysis of the logistic function model: Derivation and applications specific to batch cultured microorganisms. Bioresour. Technol. 2003, 86, 157–164. [Google Scholar] [CrossRef]
- Guerra, N.P.; Torrado, A.; López, C.; Fajardo, P.; Pastrana, L. Dynamic mathematical models to describe the growth and nisin production by Lactococcus lactis subsp. lactis CECT 539 in both batch and re-alkalized fed-batch cultures. J. Food Eng. 2007, 82, 103–113. [Google Scholar]
- Vázquez, J.A.; Murado, M.A. Unstructured mathematical model for biomass, lactic acid and bacteriocin production by lactic acid bacteria in batch fermentation. J. Chem. Technol. Biotechnol. 2008, 83, 91–96. [Google Scholar] [CrossRef] [Green Version]
- Shirsat, N.; Mohd, A.; Whelan, J.; English, N.J.; Glennon, B.; Al-Rubeai, M. Revisiting Verhulst and Monod models: Analysis of batch and fed-batch cultures. Cytotechnology 2015, 67, 515–530. [Google Scholar] [CrossRef] [Green Version]
- Guerra, N.P. Modeling the batch bacteriocin production system by lactic acid bacteria by using modified three-dimensional Lotka–Volterra equations. Biochem. Eng. J. 2014, 88, 115–130. [Google Scholar] [CrossRef]
- Callewaert, R.; De Vuyst, L. Bacteriocin production with Lactobacillus amylovorus DCE 471 is improved and stabilized by fed-batch fermentation. Appl. Environ. Microbiol. 2000, 66, 606–613. [Google Scholar] [CrossRef] [Green Version]
- Wick, L.M.; Weilenmann, H.; Egli, T. The apparent clock-like evolution of Escherichia coli in glucose-limited chemostats is reproducible at large but not at small population sizes and can be explained with Monod kinetics. Microbiology 2002, 148, 2889–2902. [Google Scholar] [CrossRef] [Green Version]
- Luedeking, R.; Piret, E.L. A kinetic study of the lactic acid fermentation. Batch process at controlled pH. J. Biochem. Microbiol. Technol. Eng. 1959, 1, 393–412. [Google Scholar] [CrossRef]
- Cabo, M.L.; Murado, M.A.; González, M.P.; Pastoriza, L. Effects of aeration and pH gradient on nisin production: A mathematical model. Enzym. Microb. Technol. 2001, 29, 264–273. [Google Scholar] [CrossRef]
- Constandinides, K.; Damianou, P.A. Lotka–Volterra equations in three dimensions satisfying the Kowalevski-Painlevé property. Regul. Chaotic Dyn. 2011, 16, 311–328. [Google Scholar] [CrossRef]
- Yang, R.; Ray, B. Factors influencing production of bacteriocins by lactic acid bacteria. Food Microbiol. 1994, 11, 281–291. [Google Scholar] [CrossRef]
- Wang, R.; Xiao, D. Bifurcations and chaotic dynamics in a 4-dimensional competitive Lotka–Volterra system. Nonlinear Dyn. 2010, 59, 411–422. [Google Scholar] [CrossRef]
- Costas, M.; Alonso, E.; Guerra, N.P. Nisin production in realkalized fed-batch cultures in whey with feeding with lactose- or glucose-containing substrates. Appl. Microbiol. Biotechnol. 2016, 100, 7899–7908. [Google Scholar] [CrossRef]
- Costas, M.; Alonso, E.; Outeiriño, D.; Guerra, N.P. Production of a highly concentrated probiotic culture of Lactococcus lactis CECT 539 containing high amounts of nisin. 3-Biotech 2018, 8, 292. [Google Scholar]
- Costas, M.; Alonso, E.; Bazán, D.L.; Bendaña, R.J.; Guerra, N.P. Batch and fed-batch production of probiotic biomass and nisin in nutrient-supplemented whey media. Braz. J. Microbiol. 2019, 50, 915–925. [Google Scholar]
- Urbansky, E.T.; Schock, M.R. Understanding, deriving, and computing buffer capacity. J. Chem. Educ. 2000, 77, 1640–1644. [Google Scholar] [CrossRef]
- Poolman, B.; Konings, W.N. Relation of growth of Streptococcus lactis and Streptococcus cremoris to amino acid transport. J. Bacteriol. 1988, 170, 700–707. [Google Scholar] [CrossRef] [Green Version]
- Abbasiliasi, S.; Tan, J.S.; Ibrahim, T.A.T.; Bashokouh, F.; Ramakrishnan, N.R.; Mustafa, S.; Arif, A.B. Fermentation factors influencing the production of bacteriocins by lactic acid bacteria: A review. RSC Adv. 2017, 7, 29395–29420. [Google Scholar] [CrossRef]
- Salaün, F.; Mietton, B.; Gaucheron, F. Buffering capacity of dairy products. Int. Dairy J. 2005, 15, 95–109. [Google Scholar] [CrossRef]
- Parente, E.; Ricciardi, A.; Addario, G. Influence of pH on growth and bacteriocin production by Lactococcus lactis subsp. lactis 14ONWC during batch fermentation. Appl. Microbiol. Biotechnol. 1994, 41, 388–394. [Google Scholar] [CrossRef]
- van Niel, E.W.J.; Hahn-Hägerdal, B. Nutrient requirements of lactococci in defined growth media. Appl. Microbiol. Biotechnol. 1999, 52, 617–627. [Google Scholar] [CrossRef] [Green Version]
- Moret-Tatay, C.; Gamermann, D.; Navarro-Pardo, E.; Fernández de Córdoba, P. ExGUtils: A python package for statistical analysis with the ex-gaussian probability density. Front. Psychol. 2018, 9, 612. [Google Scholar] [CrossRef] [Green Version]
- Hsu, S.-B.; Ruan, S.; Yang, T.-H. Analysis of three species Lotka–Volterra food web models with omnivory. J. Math. Anal. Appl. 2015, 426, 659–687. [Google Scholar] [CrossRef]
- Adamu, H.A. Mathematical analysis of predator-prey model with two preys and one predator. Int. J. Eng. Appl. Sci. 2018, 5, 17–23. [Google Scholar]
- Castro-Palacio, J.C.; Isidro, J.M.; Navarro-Pardo, E.; Velázquez-Abad, L.; Fernández-de-Córdoba, P. Monte Carlo Simulation of a modified Chi distribution with unequal variances in the generating gaussians. A Discrete Methodology to Study Collective Response Times. Mathematics 2021, 9, 77. [Google Scholar] [CrossRef]
- Ortigosa, N.; Orellana-Panchame, M.; Castro-Palacio, J.C.; Fernández de Córdoba, P.; Isidro, J.M. Monte Carlo simulation of a modified Chi distribution considering asymmetry in the generating functions: Application to the Study of Health-Related Variables. Symmetry 2021, 13, 924. [Google Scholar] [CrossRef]
- Goudar, C.T.; Joeris, K.; Konstantinov, K.B.; Piret, J.M. Logistic equations effectively model mammalian cell batch and fed-batch kinetics by logically constraining the fit. Biotechnol. Prog. 2005, 21, 1109–1118. [Google Scholar] [CrossRef] [PubMed]
- Tomás Miguel, J.M.; Meléndez Moral, J.C.; Navarro Pardo, E. Factorial confirmatory models of Ryff’s scales in a sample of elderly people. Psicothema 2008, 20, 304–310. [Google Scholar] [PubMed]
- Kanzow, C.; Yamashita, N.; Fukushima, M. Levenberg–Marquardt methods with strong local convergence properties for solving nonlinear equations with convex constraints. J. Comput. Appl. Math. 2004, 172, 375–397. [Google Scholar] [CrossRef] [Green Version]
- Behling, R.; Gonçalves, D.S.; Santos, S.A. Local convergence analysis of the Levenberg-Marquardt framework for nonzero-residue nonlinear least-squares problems under an error bound condition. J. Optim. Theory Appl. 2019, 183, 1099–1122. [Google Scholar] [CrossRef]
- Vazquez, J.A.; Lorenzo, J.M.; Fuciños, P.; Franco, D. Evaluation of non-linear equations to model different animal growths with mono and bisigmoid profiles. J. Theor. Biol. 2012, 314, 95–105. [Google Scholar] [CrossRef] [PubMed]
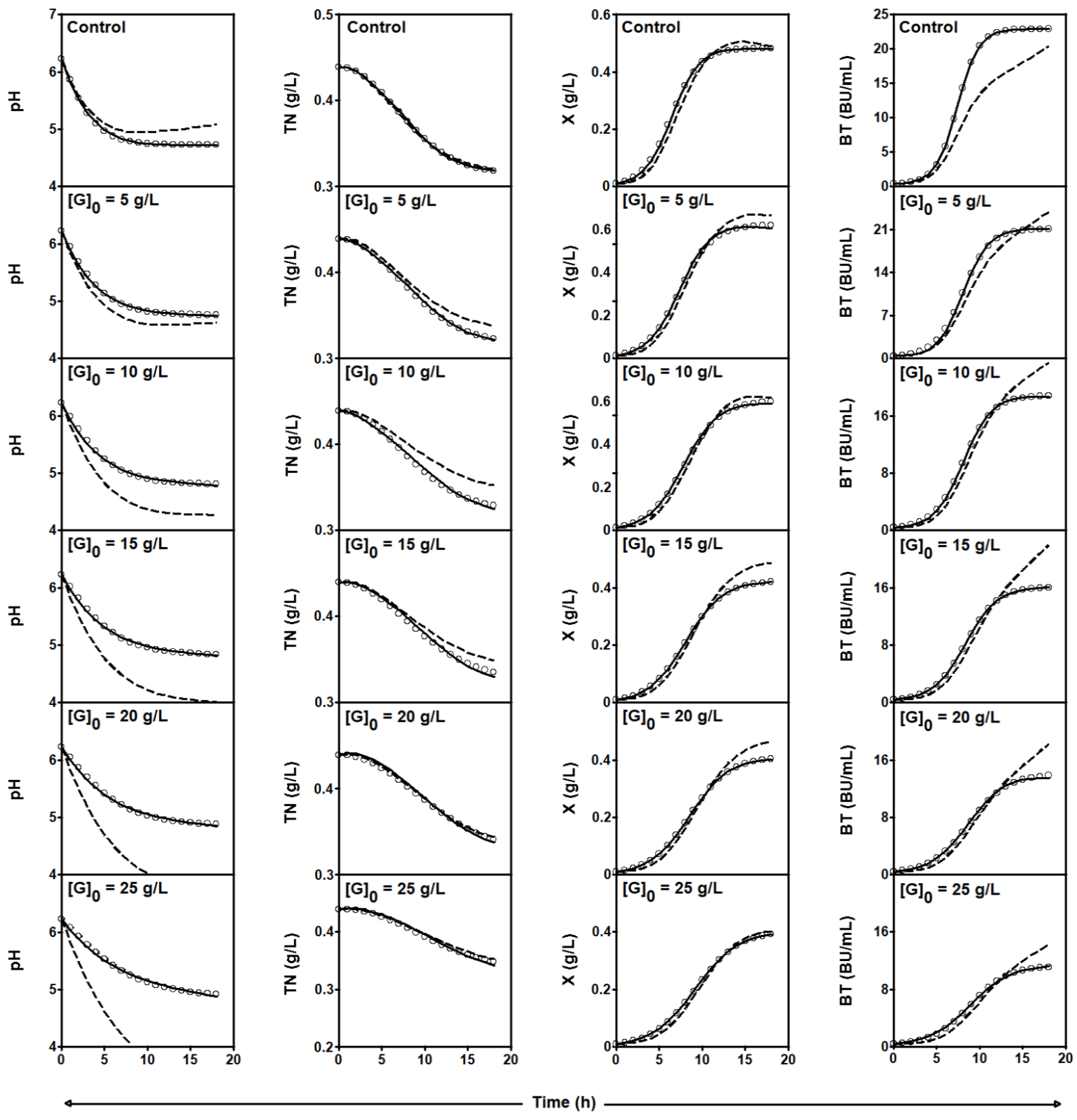
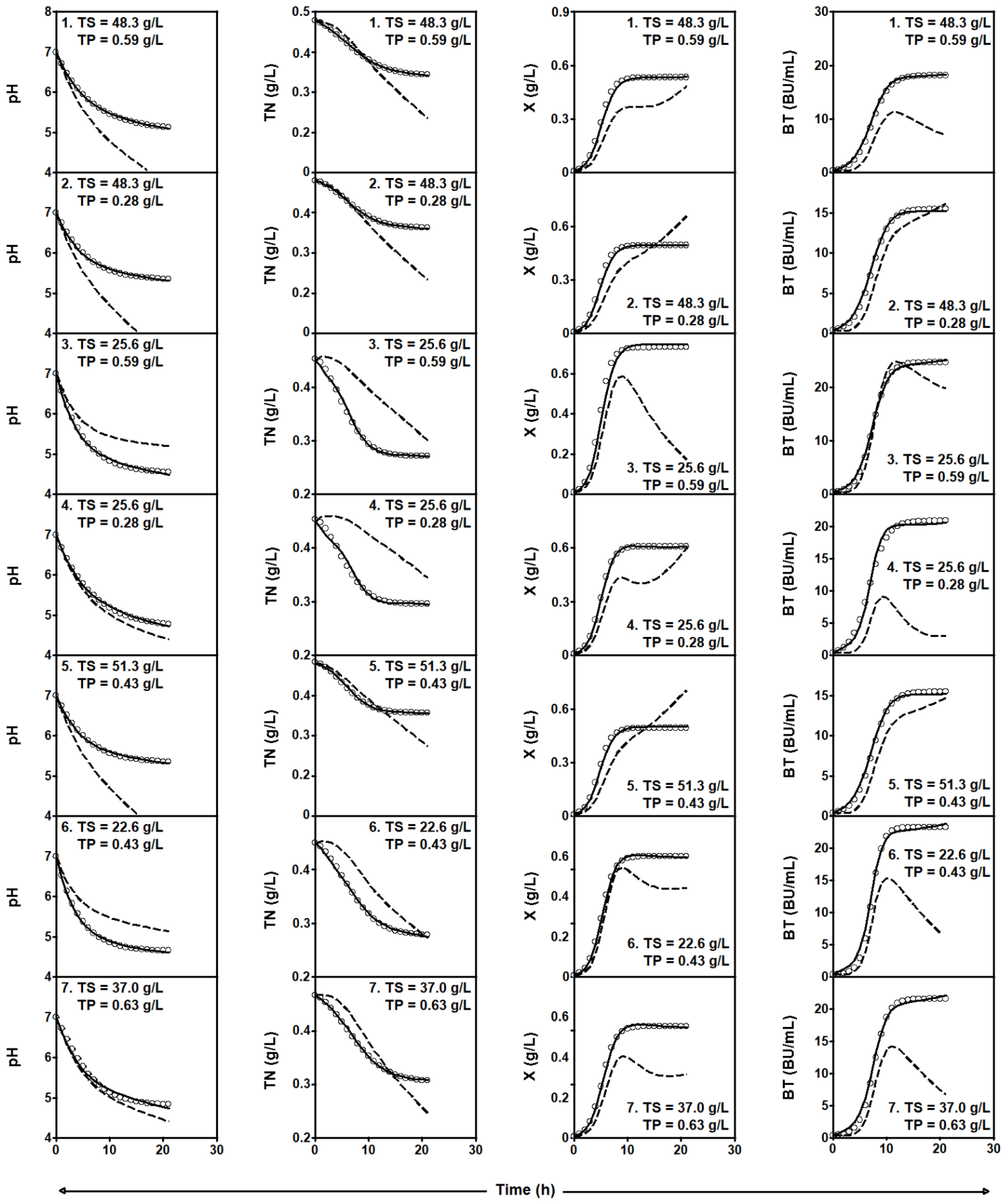

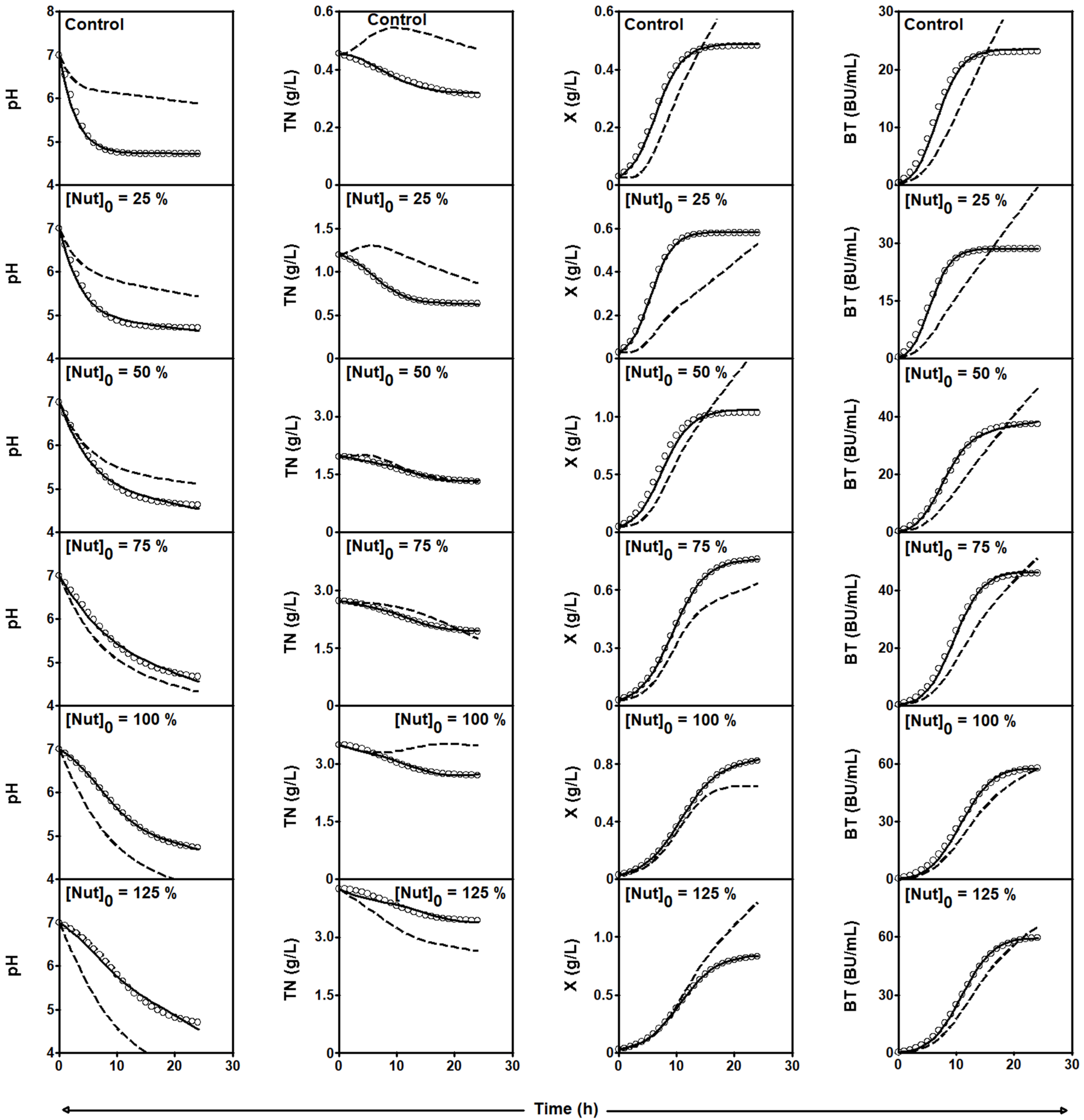



| Medium | TS (g/L) | TN (g/L) | TP (g/L) | Pr (g/L) |
|---|---|---|---|---|
| DW | 20.54 ± 0.514 | 0.45 ± 0.014 | 0.25 ± 0.021 | 2.04 ± 0.083 |
| DW25 | 20.96 ± 0.021 | 1.20 ± 0.006 | 0.30 ± 0.001 | 5.39 ± 0.025 |
| DW50 | 21.93 ± 0.014 | 1.96 ± 0.013 | 0.36 ± 0.05 | 8.72 ± 0.034 |
| DW75 | 22.89 ± 0.016 | 2.72 ± 0.002 | 0.43 ± 0.07 | 12.05 ± 0.019 |
| DW100 | 23.85 ± 0.010 | 3.49 ± 0.016 | 0.50 ± 0.017 | 15.38 ± 0.031 |
| DW125 | 24.81 ± 0.023 | 4.25 ± 0.004 | 0.57 ± 0.013 | 18.71 ± 0.026 |
| CMPW | 101.33 ± 1.314 | 0.54 ± 0.024 | 0.06 ± 0.009 | 3.47 ± 0.046 |
| Points | Experiment | TS (g/L) | TP (g/L) | TN (g/L) | Pr (g/L) |
|---|---|---|---|---|---|
| Factorial | 1 | 48.321 ± 0.001 | 0.589 ± 0.001 | 0.479 ± 0.002 | 2.531 ± 0.018 |
| 2 | 48.321 ± 0.001 | 0.281 ± 0.001 | 0.479 ± 0.002 | 2.531 ± 0.018 | |
| 3 | 25.639 ± 0.001 | 0.589 ± 0.001 | 0.453 ± 0.003 | 2.122 ± 0.028 | |
| 4 | 25.639 ± 0.001 | 0.281 ± 0.001 | 0.453 ± 0.003 | 2.122 ± 0.028 | |
| Axial | 5 | 51.352 ± 0.003 | 0.435 ± 0.002 | 0.483 ± 0.001 | 2.583 ± 0.047 |
| 6 | 22.611 ± 0.001 | 0.435 ± 0.002 | 0.450 ± 0.001 | 2.068 ± 0.012 | |
| 7 | 36.984 ± 0.002 | 0.631 ± 0.003 | 0.466 ± 0.002 | 2.318 ± 0.026 | |
| 8 | 36.984 ± 0.002 | 0.240 ± 0.001 | 0.466 ± 0.002 | 2.318 ± 0.026 | |
| Center (five replicates) | 9–13 | 36.984 ± 0.002 | 0.435 ± 0.001 | 0.466 ± 0.002 | 2.318 ± 0.026 |
| Parameter | DW-G Series | DW-TS-TP Series | DW-MRS Series |
|---|---|---|---|
| a | −0.155 ± 0.039 (p = 0.0001) | −0.083 ± 0.022 (p = 0.0003) | −0.105 ± 0.022 (p < 0.0001) |
| b | −0.036 ± 0.007 (p < 0.0001) | −0.019 ± 0.003 (p < 0.0001) | −0.0229 ± 0.003 (p = 0.0001) |
| c | 0.040 ± 0.018 (p = 0.0254) | 0.006 ± 0.009 (p = 0.5015) | −0.018 ± 0.010 (p = 0.0023) |
| RpH2 | 0.5382 | 0.8603 | 0.6108 |
| RPDM | 10.9930 | 10.1454 | 13.8907 |
| Bf | 0.8972 | 0.9516 | 0.9999 |
| Af | 1.1285 | 1.1001 | 1.1483 |
| F-ratio | 21.41 | 414.42 | 41.94 |
| p-value | <0.0001 | <0.0001 | <0.0001 |
| d | −0.087 ± 0.022 (p = 0.0002) | −0.034 ± 0.029 (p = 0.2427) | −0.163 ± 0.060 (p = 0.0079) |
| e | −0.636 ± 0.055 (p < 0.0001) | −0.512 ± 0.056 (p < 0.0001) | −0.012 ± 0.002 (p < 0.0001) |
| f | −0.081 ± 0.010 (p < 0.0001) | −0.123 ± 0.014 (p < 0.0001) | −0.346 ± 0.052 (p < 0.0001) |
| g | −0.0001 ± 0.000 (p = 0.4338) | 0.002 ± 0.000 (p < 0.0001) | 0.004 ± 0.0005 (p < 0.0001) |
| h | 0.032 ± 0.001 (p < 0.0001) | 0.029 ± 0.006 (p < 0.0001) | −0.018 ± 0.010 (p = 0.0602) |
| RTN2 | 0.9650 | 0.6909 | 0.2184 |
| RPDM | 1.4121 | 12.4855 | 20.6241 |
| Bf | 0.9745 | 1.0389 | 1.1310 |
| Af | 1.0143 | 1.1077 | 1.1957 |
| F-ratio | 349.16 | 66.00 | 22.58 |
| p-value | < 0.0001 | < 0.0001 | 1.0000 |
| i | 1.800 ± 0.327 (p < 0.0001) | 2.381 ± 0.150 (p < 0.0001) | 1.415 ± 0.221 (p < 0.0001) |
| j | 1.376 ± 0.098 (p < 0.0001) | 0.736 ± 0.067 (p < 0.0001) | 0.366 ± 0.078 (p = 0.0001) |
| k | −0.007 ± 0.001 (p = 0.0016) | 0.021 ± 0.001 (p < 0.0001) | 0.006 ± 0.002 (p = 0.0023) |
| l | 0.404 ± 0.035 (p = 0.0016) | 0.262 ± 0.0290 (p < 0.0001) | 0.227 ± 0.040 (p < 0.0001) |
| m | −1.880 ± 0.432 (p < 0.0001) | 0.636 ± 0.166 (p = 0.0002) | −0.104 ± 0.013 (p < 0.0001) |
| RX2 | 0.9669 | 0.8686 | 0.5670 |
| RPDM | 13.3796 | 25.9596 | 29.5432 |
| Bf | 0.9079 | 0.7488 | 0.8132 |
| Af | 1.1626 | 1.4377 | 1.3899 |
| F-ratio | 369.40 | 222.04 | 16.46 |
| p-value | < 0.0001 | < 0.0001 | < 0.0001 |
| n | 3.730 ± 0.335 (p < 0.0001) | 1.406 ± 0.170 (p < 0.0001) | 0.232 ± 0.141 (p = 0.0998) |
| o | 2.058 × 10−5 ± 0.004 (p = 0.9955) | 0.051 ± 0.001 (p < 0.0001) | 0.002 ± 0.001 (p = 0.0089) |
| p | 1.788 ± 0.114 (p < 0.0001) | −1.148 ± 0.073 (p < 0.0001) | 0.136 ± 74.506 (p = 0.0020) |
| q | 0.532 ± 0.041 (p < 0.0001) | 0.260 ± 0.030 (p < 0.0001) | 0.008 ± 0.025 (p = 0.7806) |
| r | 0.984 ± 1.295 (p = 0.4489) | −0.723 ± 0.140 (p < 0.0001) | −0.051 ± 0.008 (p < 0.0001) |
| RBT2 | 0.9001 | 0.9208 | 0.8890 |
| RPDM | 18.6672 | 34.7477 | 32.1298 |
| Bf | 0.8735 | 0.6200 | 0.7513 |
| Af | 1.2311 | 1.9114 | 1.4540 |
| F-ratio | 109.89 | 402.53 | 130.96 |
| p-value | <0.0001 | <0.0001 | <0.0001 |
| Initial Glucose Concentrations (g/L) in the DW Medium | ||||||
|---|---|---|---|---|---|---|
| Parameter | 0 | 5 | 10 | 15 | 20 | 25 |
| a | −0.154 ± 0.003 (p < 0.0001) | −0.147 ± 0.001 (p < 0.0001) | −0.137 ± 0.002 (p < 0.0001) | −0.122 ± 0.001 (p < 0.0001) | −0.108 ± 0.004 (p < 0.0001) | −0.090 ± 0.003 (p < 0.0001) |
| b | −0.037 ± 0.001 (p < 0.0001) | −0.033 ± 0.001 (p < 0.0001) | −0.030 ± 0.001 (p < 0.0001) | −0.027 ± 0.001 (p < 0.0001) | −0.024 ± 0.001 (p < 0.0001) | −0.020 ± 0.002 (p < 0.0001) |
| c | 0.039 ± 0.001 (p < 0.0001) | 0.021 ± 0.001 (p < 0.0001) | 0.014 ± 0.001 (p < 0.0001) | 0.013 ± 0.001 (p < 0.0001) | 0.009 ± 0.001 (p < 0.0001) | 0.002 ± 0.000 (p < 0.0001) |
| RpH2 | 0.9988 | 0.9980 | 0.9973 | 0.9973 | 0.9973 | 0.9968 |
| RPDM | 0.2083 | 0.3362 | 0.4013 | 0.4057 | 0.4107 | 0.4180 |
| Bf | 0.9997 | 0.9990 | 0.9988 | 0.9989 | 0.9988 | 0.9993 |
| Af | 1.0021 | 1.0034 | 1.0040 | 1.0041 | 1.0041 | 1.0042 |
| F-ratio | 369.17 | 322.20 | 313.95 | 313.38 | 310.46 | 305.77 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| d | −0.194 ± 0.003 (p < 0.0001) | −0.190 ± 0.001 (p < 0.0001) | −0.184 ± 0.001 (p < 0.0001) | −0.171 ± 0.001 (p < 0.0001) | −0.168 ± 0.002 (p < 0.0001) | −0.164 ± 0.001 (p < 0.0001) |
| e | −0.909 ± 0.001 (p < 0.0001) | −0.899 ± 0.001 (p < 0.0001) | −0.884 ± 0.004 (p < 0.0001) | −0.851 ± 0.002 (p < 0.0001) | −0.846 ± 0.001 (p < 0.0001) | −0.836 ± 0.003 (p < 0.0001) |
| f | −0.066 ± 0.002 (p < 0.0001) | −0.067 ± 0.005 (p < 0.0001) | −0.067 ± 0.003 (p < 0.0001) | −0.067 ± 0.002 (p < 0.0001) | −0.067 ± 0.001 (p < 0.0001) | −0.066 ± 0.002 (p < 0.0001) |
| g | −0.002 ± 0.000 (p < 0.0001) | −0.002 ± 0.000 (p < 0.0001) | −0.002 ± 0.000 (p < 0.0001) | −0.002 ± 0.000 (p < 0.0001) | −0.002 ± 0.000 (p < 0.0001) | −0.002 ± 0.000 (p < 0.0001) |
| h | 0.034 ± 0.001 (p < 0.0001) | 0.034 ± 0.001 (p < 0.0001) | 0.033 ± 0.001 (p < 0.0001) | 0.034 ± 0.001 (p < 0.0001) | 0.034 ± 0.001 (p < 0.0001) | 0.034 ± 0.002 (p < 0.0001) |
| RTN2 | 0.9995 | 0.9993 | 0.9987 | 0.9997 | 0.9988 | 0.9998 |
| RPDM | 0.2355 | 0.2260 | 0.3286 | 0.1284 | 0.2986 | 0.0979 |
| Bf | 1.0001 | 0.9996 | 0.9994 | 0.9999 | 1.0006 | 1.0002 |
| Af | 1.0024 | 1.0023 | 1.0033 | 1.0013 | 1.0030 | 1.0010 |
| F-ratio | 198.79 | 156.66 | 179.35 | 159.14 | 167.55 | 366.64 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| i | 0.843 ± 0.001 (p < 0.0001) | 0.810 ± 0.004 (p < 0.0001) | 0.740 ± 0.001 (p < 0.0001) | 0.626 ± 0.003 (p < 0.0001) | 0.531 ± 0.003 (p < 0.0001) | 0.513 ± 0.000 (p < 0.0001) |
| j | 0.787 ± 0.016 (p < 0.0001) | 0.787 ± 0.009 (p < 0.0001) | 0.787 ± 0.002 (p < 0.0001) | 0.787 ± 0.008 (p < 0.0001) | 0.787 ± 0.008 (p < 0.0001) | 0.787 ± 0.001 (p < 0.0001) |
| k | 0.008 ± 0.001 (p < 0.0001) | 0.008 ± 0.000 (p < 0.0001) | 0.008 ± 0.001 (p < 0.0001) | 0.008 ± 0.001 (p < 0.0001) | 0.008 ± 0.001 (p < 0.0001) | 0.008 ± 0.001 (p < 0.0001) |
| l | 0.066 ± 0.003 (p < 0.0001) | 0.065 ± 0.001 (p < 0.0001) | 0.056 ± 0.002 (p < 0.0001) | 0.040 ± 0.001 (p < 0.0001) | 0.026 ± 0.000 (p < 0.0001) | 0.026 ± 0.001 (p < 0.0001) |
| m | −0.104 ± 0.012 (p < 0.0001) | −0.103 ± 0.007 (p < 0.0001) | −0.102 ± 0.012 (p < 0.0001) | −0.101 ± 0.001 (p < 0.0001) | −0.092 ± 0.001 (p < 0.0001) | −0.079 ± 0.001 (p < 0.0001) |
| RX2 | 0.9997 | 0.9990 | 0.9991 | 1.0000 | 1.0000 | 1.0000 |
| RPDM | 2.6773 | 2.1414 | 1.6724 | 0.9334 | 0.6215 | 0.4064 |
| Bf | 0.9764 | 0.9952 | 1.0056 | 0.9937 | 0.9962 | 1.0005 |
| Af | 1.0282 | 1.0218 | 1.0168 | 1.0095 | 1.0063 | 1.0041 |
| F-ratio | 163893.47 | 158803.25 | 165456.52 | 169736.76 | 179962.55 | 18968.82 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| n | 3.727 ± 0.015 (p < 0.0001) | 3.664 ± 0.016 (p < 0.0001) | 2.672 ± 0.033 (p < 0.0001) | 1.939 ± 0.022 (p < 0.0001) | 1.496 ± 0.016 (p < 0.0001) | 0.922 ± 0.005 (p < 0.0001) |
| o | 0.036 ± 0.002 (p < 0.0001) | 0.036 ± 0.001 (p < 0.0001) | 0.036 ± 0.004 (p < 0.0001) | 0.036 ± 0.003 (p < 0.0001) | 0.036 ± 0.003 (p < 0.0001) | 0.036 ± 0.002 (p < 0.0001) |
| p | 0.137 ± 0.006 (p < 0.0001) | 0.137 ± 0.004 (p < 0.0001) | 0.137 ± 0.022 (p < 0.0001) | 0.137 ± 0.011 (p < 0.0001) | 0.137 ± 0.010 (p < 0.0001) | 0.137 ± 0.002 (p < 0.0001) |
| q | 0.607 ± 0.013 (p < 0.0001) | 0.605 ± 0.009 (p < 0.0001) | 0.409 ± 0.003 (p < 0.0001) | 0.276 ± 0.002 (p < 0.0001) | 0.201 ± 0.021 (p < 0.0001) | 0.100 ± 0.001 (p < 0.0001) |
| r | −0.092 ± 0.003 (p < 0.0001) | −0.092 ± 0.002 (p < 0.0001) | −0.092 ± 0.002 (p < 0.0001) | −0.092 ± 0.001 (p < 0.0001) | −0.092 ± 0.004 (p < 0.0001) | −0.092 ± 0.001 (p < 0.0001) |
| RBT2 | 1.0000 | 0.9997 | 0.9998 | 1.0000 | 0.9992 | 0.9997 |
| RPDM | 0.7956 | 7.5772 | 2.8486 | 0.5489 | 1.2762 | 4.3632 |
| Bf | 0.9953 | 0.9232 | 0.9748 | 1.0042 | 0.9997 | 1.0365 |
| Af | 1.0082 | 1.0894 | 1.0300 | 1.0054 | 1.0124 | 1.0423 |
| F-ratio | 16623.57 | 14125.66 | 14185.31 | 16845.44 | 9845.37 | 14054.20 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| Factorial Points | Axial Points | |||||||
|---|---|---|---|---|---|---|---|---|
| Parameter | TS = 48.3 g/L TP = 0.59 g/L | TS = 48.3 g/L TP = 0.28 g/L | TS = 25.6 g/L TP = 0.59 g/L | TS = 25.6 g/L TP = 0.28 g/L | TS = 51.3 g/L TP = 0.43 g/L | TS = 22.6 g/L TP = 0.43 g/L | TS = 37.0 g/L TP = 0.63 g/L | TS = 37.0 g/L TP = 0.24 g/L |
| a | −0.009 ± 0.001 (p < 0.0001) | −0.008 ± 0.011 (p < 0.0001) | −0.015 ± 0.002 (p < 0.0001) | −0.013 ± 0.001 (p < 0.0001) | −0.008 ± 0.001 (p < 0.0001) | −0.014 ± 0.001 (p < 0.0001) | −0.012 ± 0.001 (p < 0.0001) | −0.011 ± 0.002 (p < 0.0001) |
| b | −0.007 ± 0.002 (p < 0.0001) | −0.007 ± 0.002 (p < 0.0001) | −0.012 ± 0.003 (p < 0.0001) | −0.009 ± 0.001 (p < 0.0001) | −0.007 ± 0.001 (p < 0.0001) | −0.012 ± 0.001 (p < 0.0001) | −0.009 ± 0.002 (p < 0.0001) | −0.008 ± 0.002 (p < 0.0001) |
| c | 0.041 ± 0.003 (p < 0.0001) | 0.050 ± 0.011 (p < 0.0001) | 0.045 ± 0.005 (p < 0.0001) | 0.034 ± 0.000 (p < 0.0001) | 0.050 ± 0.000 (p < 0.0001) | 0.057 ± 0.004 (p < 0.0001) | 0.036 ± 0.001 (p < 0.0001) | 0.043 ± 0.002 (p < 0.0001) |
| RpH2 | 0.9961 | 0.9918 | 0.9941 | 0.9952 | 0.9918 | 0.9971 | 0.9897 | 0.9903 |
| RPDM | 0.5322 | 0.6954 | 0.9820 | 0.7802 | 0.6954 | 0.6045 | 1.1740 | 0.9703 |
| Bf | 0.9997 | 0.9995 | 0.9994 | 0.9994 | 0.9995 | 0.9999 | 0.9988 | 0.9990 |
| Af | 1.0053 | 1.0070 | 1.0099 | 1.0078 | 1.0070 | 1.0061 | 1.0118 | 1.0098 |
| F-ratio | 1225.62 | 1502.17 | 1414.37 | 1203.42 | 1203.42 | 588.82 | 1308.83 | 698.32 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| d | −0.188 ± 0.023 (p < 0.0001) | −0.209 ± 0.016 (p < 0.0001) | −0.595 ± 0.029 (p < 0.0001) | −0.741 ± 0.026 (p < 0.0001) | −0.453 ± 0.023 (p < 0.0001) | −0.154 ± 0.002 (p < 0.0001) | −0.196 ± 0.007 (p < 0.0001) | −0.170 ± 0.008 (p < 0.0001) |
| e | −0.914 ± 0.037 (p < 0.0001) | −0.940 ± 0.022 (p < 0.0001) | −1.897 ± 0.088 (p < 0.0001) | −2.224 ± 0.101 (p = 0.0103) | −1.447 ± 0.066 (p < 0.0001) | −0.903 ± 0.016 (p < 0.0001) | −0.955 ± 0.021 (p < 0.0001) | −0.885 ± 0.013 (p < 0.0001) |
| f | −0.058 ± 0.002 (p < 0.0001) | −0.073 ± 0.006 (p < 0.0001) | −0.288 ± 0.013 (p < 0.0001) | −0.368 ± 0.019 (p < 0.0001) | −0.206 ± 0.018 (p < 0.0001) | −0.058 ± 0.002 (p < 0.0001) | −0.073 ± 0.001 (p < 0.0001) | −0.073 ± 0.003 (p < 0.0001) |
| g | −0.001 ± 0.000 (p = 0.0012) | −0.001 ± 0.000 (p = 0.0025) | −0.001 ± 0.000 (p < 0.0001) | −0.001 ± 0.000 (p = 0.0024) | −0.001 ± 0.000 (p < 0.0001) | −0.001 ± 0.000 (p < 0.0001) | −0.001 ± 0.000 (p < 0.0001) | −0.001 ± 0.000 (p < 0.0001) |
| h | 0.034 ± 0.002 (p < 0.0001) | 0.034 ± 0.007 (p < 0.0001) | 0.034 ± 0.004 (p < 0.0001) | 0.034 ± 0.003 (p < 0.0001) | 0.034 ± 0.002 (p < 0.0001) | 0.034 ± 0.004 (p < 0.0001) | 0.034 ± 0.003 (p < 0.0001) | 0.034 ± 0.002 (p < 0.0001) |
| RTN2 | 0.9998 | 0.9996 | 0.9974 | 0.9947 | 0.9987 | 0.9987 | 0.9995 | 0.9973 |
| RPDM | 0.1460 | 0.1608 | 0.5997 | 0.7355 | 0.2837 | 0.6074 | 0.2451 | 0.7085 |
| Bf | 1.0000 | 1.0002 | 0.9992 | 0.9995 | 1.0002 | 0.9992 | 0.9998 | 0.9989 |
| Af | 1.0015 | 1.0016 | 1.0060 | 1.0074 | 1.0028 | 1.0061 | 1.0025 | 1.0071 |
| F-ratio | 111.24 | 136.45 | 128.63 | 145.52 | 809.54 | 101.89 | 99.51 | 221.34 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| i | 0.805 ± 0.027 (p < 0.0001) | 0.781 ± 0.011 (p < 0.0001) | 0.923 ± 0.026 (p < 0.0001) | 0.940 ± 0.005 (p < 0.0001) | 0.845 ± 0.019 (p < 0.0001) | 0.978 ± 0.027 (p < 0.0001) | 0.860 ± 0.027 (p < 0.0001) | 0.823 ± 0.016 (p < 0.0001) |
| j | 1.172 ± 0.084 (p < 0.0001) | 1.319 ± 0.025 (p < 0.0001) | 0.884 ± 0.010 (p < 0.0001) | 1.127 ± 0.014 (p < 0.0001) | 1.349 ± 0.103 (p < 0.0001) | 0.967 ± 0.010 (p < 0.0001) | 0.973 ± 0.025 (p < 0.0001) | 1.098 ± 0.103 (p < 0.0001) |
| k | 0.005 ± 0.001 (p < 0.0001) | 0.005 ± 0.000 (p < 0.0001) | 0.005 ± 0.000 (p < 0.0001) | 0.005 ± 0.000 (p = 0.0012) | 0.005 ± 0.000 (p < 0.0001) | 0.005 ± 0.000 (p < 0.0001) | 0.005 ± 0.000 (p = 0.0012) | 0.005 ± 0.000 (p < 0.0001) |
| l | 0.017 ± 0.001 (p < 0.0001) | 0.009 ± 0.001 (p < 0.0001) | 0.033 ± 0.002 (p < 0.0001) | 0.030 ± 0.002 (p < 0.0001) | 0.018 ± 0.001 (p < 0.0001) | 0.044 ± 0.003 (p < 0.0001) | 0.029 ± 0.001 (p < 0.0001) | 0.024 ± 0.002 (p < 0.0001) |
| m | −0.002 ± 0.000 (p < 0.0001) | −0.002 ± 0.001 (p < 0.0001) | −0.002 ± 0.000 (p < 0.0001) | −0.002 ± 0.000 (p < 0.0001) | −0.002 ± 0.001 (p < 0.0001) | −0.002 ± 0.002 (p < 0.0001) | −0.002 ± 0.001 (p < 0.0001) | −0.002 ± 0.001 (p < 0.0001) |
| RX2 | 0.9973 | 0.9958 | 0.9939 | 0.9989 | 0.9965 | 0.9992 | 0.9983 | 0.9984 |
| RPDM | 3.2062 | 3.5977 | 4.8630 | 2.0830 | 3.3137 | 2.4733 | 3.0536 | 2.8520 |
| Bf | 0.9797 | 0.9788 | 0.9680 | 0.9802 | 0.9796 | 0.9816 | 0.9778 | 0.9794 |
| Af | 1.0336 | 1.0378 | 1.0525 | 1.0215 | 1.0347 | 1.0259 | 1.0321 | 1.0299 |
| F-ratio | 2655.11 | 2222.57 | 2246.34 | 18756.55 | 11253.26 | 36008.93 | 5001.64 | 4953.73 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| n | 1.068 ± 0.018 (p < 0.0001) | 1.066 ± 0.009 (p < 0.0001) | 1.314 ± 0.015 (p < 0.0001) | 1.136 ± 0.086 (p < 0.0001) | 1.067 ± 0.043 (p < 0.0001) | 1.226 ± 0.111 (p < 0.0001) | 1.136 ± 0.044 (p < 0.0001) | 1.071 ± 0.003 (p < 0.0001) |
| o | 0.035 ± 0.003 (p < 0.0001) | 0.040 ± 0.003 (p < 0.0001) | 0.030 ± 0.001 (p < 0.0001) | 0.033 ± 0.002 (p < 0.0001) | 0.040 ± 0.005 (p < 0.0001) | 0.036 ± 0.001 (p < 0.0001) | 0.036 ± 0.001 (p < 0.0001) | 0.037 ± 0.002 (p < 0.0001) |
| p | 0.098 ± 0.010 (p < 0.0001) | 0.098 ± 0.006 (p < 0.0001) | 0.098 ± 0.002 (p < 0.0001) | 0.098 ± 0.006 (p < 0.0001) | 0.098 ± 0.004 (p < 0.0001) | 0.098 ± 0.005 (p < 0.0001) | 0.098 ± 0.006 (p < 0.0001) | 0.098 ± 0.001 (p < 0.0001) |
| q | 0.080 ± 0.005 (p < 0.0001) | 0.081 ± 0.002 (p < 0.0001) | 0.117 ± 0.001 (p < 0.0001) | 0.086 ± 0.002 (p < 0.0001) | 0.081 ± 0.002 (p < 0.0001) | 0.073 ± 0.004 (p < 0.0001) | 0.073 ± 0.002 (p < 0.0001) | 0.073 ± 0.006 (p < 0.0001) |
| r | −0.103 ± 0.008 (p < 0.0001) | −0.103 ± 0.003 (p < 0.0001) | −0.309 ± 0.018 (p < 0.0001) | −0.103 ± 0.008 (p < 0.0001) | −0.103 ± 0.003 (p < 0.0001) | −0.103 ± 0.008 (p < 0.0001) | −0.103 ± 0.003 (p < 0.0001) | −0.103 ± 0.009 (p < 0.0001) |
| RBT2 | 0.9988 | 0.9983 | 0.9988 | 0.9971 | 0.9987 | 0.9982 | 0.9983 | 0.9983 |
| RPDM | 6.2540 | 7.5243 | 7.4690 | 4.1038 | 7.3319 | 21.2434 | 16.3565 | 14.6312 |
| Bf | 1.0562 | 1.0597 | 1.0602 | 1.0275 | 1.0653 | 1.1581 | 1.1369 | 1.1155 |
| Af | 1.0596 | 1.0714 | 1.0702 | 1.0406 | 1.0691 | 1.1700 | 1.1398 | 1.1272 |
| F-ratio | 15333.43 | 15698.19 | 6008.51 | 9253.62 | 57045.82 | 11115.87 | 12489.56 | 13001.43 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| Center Points | Optimum Conditions | |||||
|---|---|---|---|---|---|---|
| Parameter | TS = 37.0 g/L TP = 0.43 g/L | TS = 37.0 g/L TP = 0.43 g/L | TS = 37.0 g/L TP = 0.43 g/L | TS = 37.0 g/L TP = 0.43 g/L | TS = 37.0 g/L TP = 0.43 g/L | TS = 22.6 g/L TP = 0.46 g/L |
| a | −0.012 ± 0.002 (p < 0.0001) | −0.012 ± 0.001 (p < 0.0001) | −0.012 ± 0.001 (p < 0.0001) | −0.012 ± 0.001 (p < 0.0001) | −0.012 ± 0.002 (p < 0.0001) | −0.016 ± 0.001 (p < 0.0001) |
| b | −0.008 ± 0.001 (p < 0.0001) | −0.008 ± 0.003 (p < 0.0001) | −0.009 ± 0.001 (p < 0.0001) | −0.008 ± 0.002 (p < 0.0001) | −0.008 ± 0.001 (p < 0.0001) | −0.013 ± 0.001 (p < 0.0001) |
| c | 0.033 ± 0.004 (p < 0.0001) | 0.033 ± 0.002 (p < 0.0001) | 0.035 ± 0.002 (p < 0.0001) | 0.035 ± 0.002 (p < 0.0001) | 0.035 ± 0.002 (p < 0.0001) | 0.048 ± 0.000 (p < 0.0001) |
| RpH2 | 0.9903 | 0.9905 | 0.9779 | 0.9805 | 0.9794 | 0.9937 |
| RPDM | 1.1229 | 1.1156 | 1.7516 | 1.6095 | 1.6444 | 1.0972 |
| Bf | 0.9988 | 0.9988 | 0.9979 | 0.9980 | 0.9979 | 0.9989 |
| Af | 1.0113 | 1.0112 | 1.0177 | 1.0163 | 1.0166 | 1.0110 |
| F-ratio | 1442.53 | 1286.83 | 1399.58 | 1306.41 | 1119.82 | 2610.26 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| d | −0.168 ± 0.008 (p < 0.0001) | −0.174 ± 0.010 (p < 0.0001) | −0.196 ± 0.014 (p < 0.0001) | −0.169 ± 0.008 (p < 0.0001) | −0.168 ± 0.016 (p < 0.0001) | −0.472 ± 0.024 (p < 0.0001) |
| e | −0.893 ± 0.013 (p < 0.0001) | −0.913 ± 0.004 (p < 0.0001) | −0.955 ± 0.010 (p < 0.0001) | −0.893 ± 0.016 (p < 0.0001) | −0.893 ± 0.055 (p < 0.0001) | −1.605 ± 0.102 (p < 0.0001) |
| f | −0.087 ± 0.005 (p < 0.0001) | −0.087 ± 0.002 (p < 0.0001) | −0.073 ± 0.002 (p < 0.0001) | −0.073 ± 0.001 (p < 0.0001) | −0.087 ± 0.004 (p < 0.0001) | −0.367 ± 0.008 (p < 0.0001) |
| g | −0.001 ± 0.000 (p < 0.0001) | −0.001 ± 0.000 (p < 0.0001) | −0.001 ± 0.000 (p < 0.0001) | −0.001 ± 0.000 (p < 0.0001) | −0.001 ± 0.000 (p < 0.0001) | −0.001 ± 0.000 (p < 0.0001) |
| h | 0.034 ± 0.001 (p < 0.0001) | 0.034 ± 0.002 (p < 0.0001) | 0.034 ± 0.001 (p < 0.0001) | 0.034 ± 0.002 (p < 0.0001) | 0.034 ± 0.001 (p < 0.0001) | 0.036 ± 0.003 (p < 0.0001) |
| RTN2 | 0.9947 | 0.9933 | 0.9941 | 0.9883 | 0.9941 | 0.9983 |
| RPDM | 1.3103 | 1.2626 | 2.0280 | 1.6987 | 1.6361 | 1.0880 |
| Bf | 0.9968 | 0.9982 | 1.0042 | 0.9970 | 0.9835 | 1.0000 |
| Af | 1.0132 | 1.0127 | 1.0205 | 1.0172 | 1.0168 | 1.0109 |
| F-ratio | 256.84 | 231.13 | 512.73 | 488.79 | 385.82 | 419.77 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| i | 0.933 ± 0.017 (p < 0.0001) | 0.932 ± 0.011 (p < 0.0001) | 0.937 ± 0.010 (p < 0.0001) | 0.933 ± 0.006 (p < 0.0001) | 0.936 ± 0.008 (p < 0.0001) | 0.995 ± 0.019 (p < 0.0001) |
| j | 1.052 ± 0.010 (p < 0.0001) | 1.034 ± 0.00034 (p < 0.0001) | 1.036 ± 0.022 (p < 0.0001) | 1.048 ± 0.013 (p < 0.0001) | 1.057 ± 0.016 (p < 0.0001) | 0.851 ± 0.008 (p < 0.0001) |
| k | 0.005 ± 0.001 (p < 0.0001) | 0.005 ± 0.000 (p < 0.0001) | 0.005 ± 0.001 (p < 0.0001) | 0.005 ± 0.001 (p < 0.0001) | 0.005 ± 0.001 (p < 0.0001) | 0.005 ± 0.001 (p < 0.0001) |
| l | 0.037 ± 0.001 (p < 0.0001) | 0.039 ± 0.001 (p < 0.0001) | 0.038 ± 0.001 (p < 0.0001) | 0.038 ± 0.001 (p < 0.0001) | 0.037 ± 0.001 (p < 0.0001) | 0.055 ± 0.001 (p < 0.0001) |
| m | −0.002 ± 0.000 (p < 0.0001) | −0.002 ± 0.001 (p < 0.0001) | −0.002 ± 0.001 (p < 0.0001) | −0.002 ± 0.000 (p < 0.0001) | −0.002 ± 0.001 (p < 0.0001) | −0.002 ± 0.001 (p < 0.0001) |
| RX2 | 0.9990 | 0.9992 | 0.9993 | 0.9984 | 0.9996 | 0.9993 |
| RPDM | 3.3758 | 2.6198 | 2.1861 | 3.9710 | 3.7304 | 2.5775 |
| Bf | 1.0056 | 0.9919 | 0.9786 | 1.0054 | 1.0251 | 0.9767 |
| Af | 1.0344 | 1.0270 | 1.0229 | 1.0406 | 1.0374 | 1.0272 |
| F-ratio | 7954.52 | 11136.19 | 24025.14 | 8212.25 | 33154.51 | 27521.83 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| n | 1.136 ± 0.013 (p < 0.0001) | 1.136 ± 0.009 (p < 0.0001) | 1.136 ± 0.010 (p < 0.0001) | 1.136 ± 0.005 (p < 0.0001) | 1.136 ± 0.011 (p < 0.0001) | 1.322 ± 0.007 (p < 0.0001) |
| o | 0.036 ± 0.001 (p < 0.0001) | 0.036 ± 0.003 (p < 0.0001) | 0.036 ± 0.001 (p < 0.0001) | 0.037 ± 0.002 (p < 0.0001) | 0.035 ± 0.002 (p < 0.0001) | 0.032 ± 0.001 (p < 0.0001) |
| p | 0.098 ± 0.001 (p < 0.0001) | 0.098 ± 0.002 (p < 0.0001) | 0.098 ± 0.001 (p < 0.0001) | 0.098 ± 0.002 (p < 0.0001) | 0.098 ± 0.001 (p < 0.0001) | 0.098 ± 0.002 (p < 0.0001) |
| q | 0.073 ± 0.003 (p < 0.0001) | 0.073 ± 0.001 (p < 0.0001) | 0.073 ± 0.004 (p < 0.0001) | 0.073 ± 0.003 (p < 0.0001) | 0.037 ± 0.006 (p < 0.0001) | 0.107 ± 0.001 (p < 0.0001) |
| r | −0.103 ± 0.011 (p < 0.0001) | −0.103 ± 0.009 (p < 0.0001) | −0.103 ± 0.015 (p < 0.0001) | −0.103 ± 0.021 (p < 0.0001) | −0.103 ± 0.008 (p < 0.0001) | −0.103 ± 0.013 (p < 0.0001) |
| RBT2 | 0.9975 | 0.9974 | 0.9985 | 0.9934 | 0.9971 | 0.9988 |
| RPDM | 18.0670 | 17.3313 | 16.4117 | 20.0055 | 17.1747 | 15.0306 |
| Bf | 1.1513 | 1.1488 | 1.1279 | 1.1670 | 1.1209 | 1.1187 |
| Af | 1.1542 | 1.1493 | 1.1388 | 1.1758 | 1.1459 | 1.1286 |
| F-ratio | 7764.25 | 9873.12 | 8895.19 | 7983.49 | 10895.37 | 3211.61 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| Initial Nutrient Concentrations (%, w/v) in the DW Medium | ||||||
|---|---|---|---|---|---|---|
| Parameter | 0 | 25 | 50 | 75 | 100 | 125 |
| a | −0.032 ± 0.001 (p < 0.0001) | −0.037 ± 0.001 (p < 0.0001) | −0.043 ± 0.002 (p < 0.0001) | 0.177 ± 0.002 (p < 0.0001) | 0.290 ± 0.015 (p < 0.0001) | 0.282 ± 0.004 (p < 0.0001) |
| b | −0.017 ± 0.001 (p < 0.0001) | −0.016 ± 0.003 (p < 0.0001) | −0.014 ± 0.001 (p < 0.0001) | 0.022 ± 0.000 (p < 0.0001) | 0.039 ± 0.002 (p < 0.0001) | 0.038 ± 0.001 (p < 0.0001) |
| c | 0.103 ± 0.002 (p < 0.0001) | 0.057 ± 0.001 (p < 0.0001) | 0.022 ± 0.001 (p < 0.0001) | 0.086 ± 0.001 (p < 0.0001) | 0.116 ± 0.010 (p < 0.0001) | 0.115 ± 0.008 (p < 0.0001) |
| RpH2 | 0.9964 | 0.9950 | 0.9941 | 0.9987 | 0.9994 | 0.9991 |
| RPDM | 0.5787 | 0.8501 | 1.0040 | 0.4249 | 0.3100 | 0.3525 |
| Bf | 0.9997 | 0.9989 | 0.9988 | 1.0001 | 1.0001 | 1.0003 |
| Af | 1.0058 | 1.0086 | 1.0101 | 1.0043 | 1.0031 | 1.0035 |
| F-ratio | 378.83 | 375.38 | 371.42 | 396.38 | 422.44 | 409.56 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| d | −0.068 ± 0.001 (p < 0.0001) | −0.189 ± 0.002 (p < 0.0001) | −0.355 ± 0.015 (p < 0.0001) | −0.379 ± 0.016 (p < 0.0001) | 0.364 ± 0.005 (p < 0.0001) | 0.416 ± 0.002 (p < 0.0001) |
| e | −0.415 ± 0.004 (p < 0.0001) | −0.430 ± 0.006 (p < 0.0001) | −0.382 ± 0.003 (p < 0.0001) | −0.297 ± 0.011 (p < 0.0001) | 0.066 ± 0.001 (p < 0.0001) | 0.068 ± 0.003 (p < 0.0001) |
| f | 0.292 ± 0.011 (p < 0.0001) | 0.338 ± 0.007 (p < 0.0001) | 0.392 ± 0.004 (p < 0.0001) | 0.395 ± 0.020 (p < 0.0001) | 0.230 ± 0.016 (p < 0.0001) | 0.148 ± 0.011 (p < 0.0001) |
| g | −0.007 ± 0.001 (p < 0.0001) | −0.012 ± 0.002 (p < 0.0001) | −0.010 ± 0.001 (p < 0.0001) | −0.008 ± 0.000 (p < 0.0001) | −0.001 ± 0.000 (p < 0.0001) | −0.001 ± 0.000 (p < 0.0001) |
| h | 0.016 ± 0.001 (p < 0.0001) | 0.045 ± 0.001 (p < 0.0001) | 0.057 ± 0.001 (p < 0.0001) | 0.061 ± 0.002 (p < 0.0001) | 0.018 ± 0.001 (p < 0.0001) | 0.018 ± 0.001 (p < 0.0001) |
| RTN2 | 0.9871 | 0.9982 | 0.9978 | 0.9850 | 0.9992 | 0.9962 |
| RPDM | 1.2891 | 0.9744 | 0.5372 | 1.7542 | 0.2335 | 0.2001 |
| Bf | 1.0013 | 1.0004 | 0.9996 | 0.9975 | 0.9998 | 0.9997 |
| Af | 1.0129 | 1.0098 | 1.0054 | 1.0178 | 1.0023 | 1.0020 |
| F-ratio | 1212.37 | 1311.19 | 1309.21 | 1125.41 | 1321.28 | 1285.43 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| i | 0.928 ± 0.013 (p < 0.0001) | 1.103 ± 0.016 (p < 0.0001) | 1.106 ± 0.008 (p < 0.0001) | 1.110 ± 0.014 (p < 0.0001) | 1.118 ± 0.024 (p < 0.0001) | 1.243 ± 0.011 (p < 0.0001) |
| j | 0.731 ± 0.010 (p < 0.0001) | 0.830 ± 0.019 (p < 0.0001) | 0.542 ± 0.025 (p < 0.0001) | 0.289 ± 0.005 (p < 0.0001) | 0.139 ± 0.003 (p < 0.0001) | 0.021 ± 0.002 (p < 0.0001) |
| k | 0.007 ± 0.001 (p < 0.0001) | 0.007 ± 0.001 (p < 0.0001) | 0.007 ± 0.001 (p < 0.0001) | 0.007 ± 0.000 (p < 0.0001) | 0.007 ± 0.000 (p < 0.0001) | 0.007 ± 0.001 (p < 0.0001) |
| l | 0.087 ± 0.004 (p < 0.0001) | 0.087 ± 0.003 (p < 0.0001) | 0.087 ± 0.004 (p < 0.0001) | 0.086 ± 0.002 (p < 0.0001) | 0.086 ± 0.001 (p < 0.0001) | 0.091 ± 0.001 (p < 0.0001) |
| m | 0.019 ± 0.002 (p < 0.0001) | 0.019 ± 0.001 (p < 0.0001) | 0.080 ± 0.002 (p < 0.0001) | 0.080 ± 0.004 (p < 0.0001) | 0.076 ± 0.003 (p < 0.0001) | 0.090 ± 0.002 (p < 0.0001) |
| RX2 | 0.9994 | 0.9999 | 0.9994 | 0.9987 | 0.9998 | 0.9991 |
| RPDM | 2.6384 | 0.7688 | 2.6386 | 2.0128 | 1.8566 | 4.4775 |
| Bf | 0.9785 | 0.9946 | 0.9787 | 0.9964 | 0.9862 | 0.9671 |
| Af | 1.0279 | 1.0078 | 1.0277 | 1.0204 | 1.0191 | 1.0480 |
| F-ratio | 6014.11 | 7051.64 | 6001.32 | 4318.51 | 6886.49 | 4806.29 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
| n | 0.546 ± 0.024 (p < 0.0001) | 0.899 ± 0.013 (p < 0.0001) | 0.967 ± 0.019 (p < 0.0001) | 1.134 ± 0.010 (p < 0.0001) | 1.186 ± 0.008 (p < 0.0001) | 1.225 ± 0.015 (p < 0.0001) |
| o | 0.015 ± 0.001 (p < 0.0001) | 0.015 ± 0.001 (p < 0.0001) | 0.009 ± 0.001 (p < 0.0001) | 0.007 ± 0.001 (p < 0.0001) | 0.005 ± 0.001 (p < 0.0001) | 0.004 ± 0.001 (p < 0.0001) |
| p | 0.385 ± 0.010 (p < 0.0001) | 0.385 ± 0.007 (p < 0.0001) | 0.385 ± 0.013 (p < 0.0001) | 0.385 ± 0.014 (p < 0.0001) | 0.385 ± 0.004 (p < 0.0001) | 0.385 ± 0.018 (p < 0.0001) |
| q | 0.003 ± 0.000 (p < 0.0001) | 0.049 ± 0.003 (p < 0.0001) | 0.077 ± 0.002 (p < 0.0001) | 0.106 ± 0.008 (p < 0.0001) | 0.120 ± 0.004 (p < 0.0001) | 0.127 ± 0.0015 (p < 0.0001) |
| r | 0.013 ± 0.002 (p < 0.0001) | 0.013 ± 0.003 (p < 0.0001) | 0.013 ± 0.001 (p < 0.0001) | 0.013 ± 0.001 (p < 0.0001) | 0.013 ± 0.001 (p < 0.0001) | 0.013 ± 0.003 (p < 0.0001) |
| RBT2 | 0.9991 | 0.9984 | 0.9994 | 0.9994 | 0.9986 | 0.9987 |
| RPDM | 4.8680 | 5.8719 | 6.4048 | 7.4056 | 11.5425 | 11.6937 |
| Bf | 0.9541 | 0.9405 | 0.9330 | 0.9237 | 0.8769 | 0.8765 |
| Af | 1.0556 | 1.0719 | 1.0781 | 1.0890 | 1.1505 | 1.1503 |
| F-ratio | 892.54 | 808.19 | 801.22 | 2315.09 | 1366.93 | 2404.16 |
| p-value | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 | <0.0001 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Giménez-Palomares, F.; Fernández de Córdoba, P.; Mejuto, J.C.; Bendaña-Jácome, R.J.; Pérez-Guerra, N. Evaluation and Mathematical Analysis of a Four-Dimensional Lotka–Volterra-like Equation Designed to Describe the Batch Nisin Production System. Mathematics 2022, 10, 677. https://doi.org/10.3390/math10050677
Giménez-Palomares F, Fernández de Córdoba P, Mejuto JC, Bendaña-Jácome RJ, Pérez-Guerra N. Evaluation and Mathematical Analysis of a Four-Dimensional Lotka–Volterra-like Equation Designed to Describe the Batch Nisin Production System. Mathematics. 2022; 10(5):677. https://doi.org/10.3390/math10050677
Chicago/Turabian StyleGiménez-Palomares, Fernando, Pedro Fernández de Córdoba, Juan C. Mejuto, Ricardo J. Bendaña-Jácome, and Nelson Pérez-Guerra. 2022. "Evaluation and Mathematical Analysis of a Four-Dimensional Lotka–Volterra-like Equation Designed to Describe the Batch Nisin Production System" Mathematics 10, no. 5: 677. https://doi.org/10.3390/math10050677
APA StyleGiménez-Palomares, F., Fernández de Córdoba, P., Mejuto, J. C., Bendaña-Jácome, R. J., & Pérez-Guerra, N. (2022). Evaluation and Mathematical Analysis of a Four-Dimensional Lotka–Volterra-like Equation Designed to Describe the Batch Nisin Production System. Mathematics, 10(5), 677. https://doi.org/10.3390/math10050677









