Analysis of Solutions, Asymptotic and Exact Profiles to an Eyring–Powell Fluid Modell
Abstract
:1. Introduction
2. Mathematical Model
3. Preliminaries
4. Existence and Uniqueness Analysis
5. Travelling Waves’ Existence and Regularity
5.1. Geometric Perturbation Theory
5.2. Travelling Waves’ Profiles
6. Numerical Validation Assessments
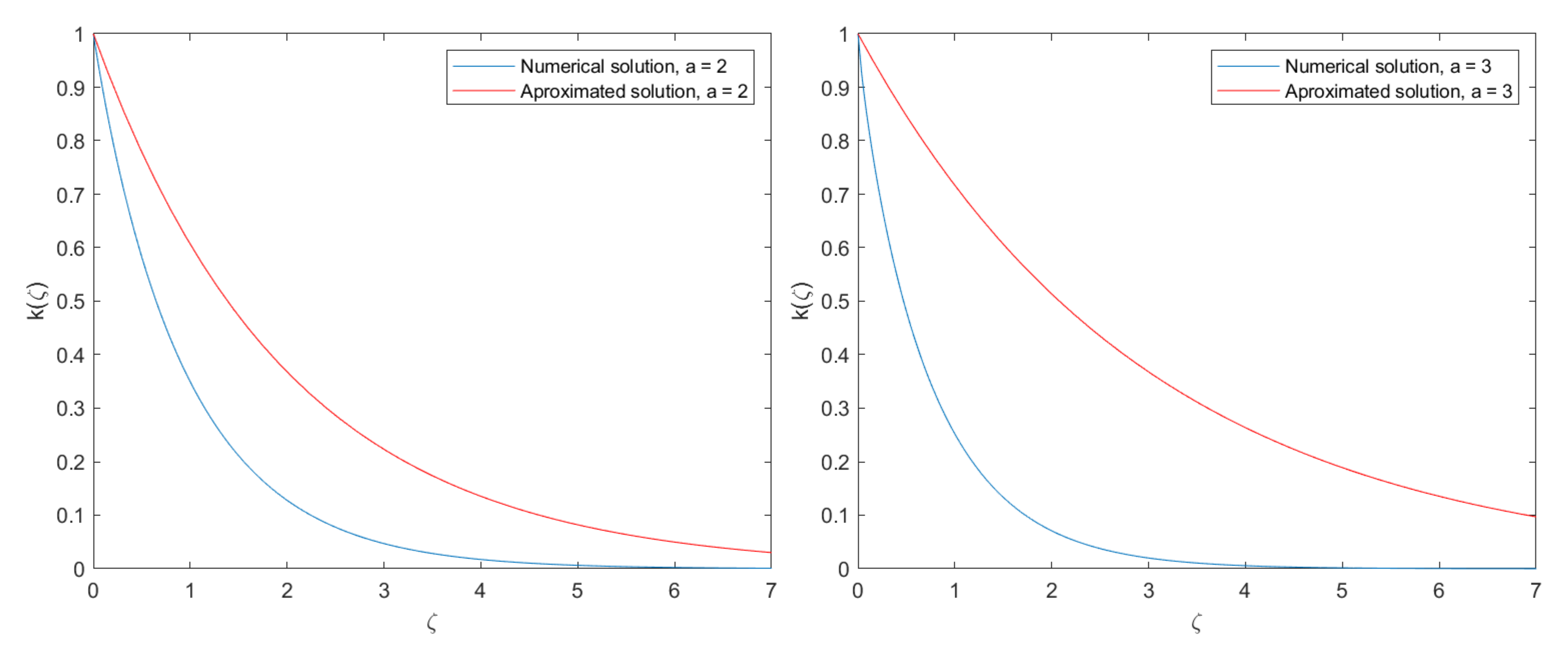
- The solver bvp4c in MATLAB was employed. This solver is based on a Runge–Kutta implicit approach with interpolant extensions [31]. The bvp4c collocation method requires specifying pseudo-boundary conditions. In this case, the left boundary is considered positive, , and the right boundary is given by the null critical state, . As the intention was to determine the exact coincidence along the profiles for which the exponential tail is given, the solutions were translated into the zero state by the standard vertical translation;
- The integration domain was assumed as , sufficiently large so as to hinder any potential effect of the pseudo-boundary conditions imposed by the collocation method involved in the bvp4c solver;
- The domain was split into 100,000 nodes with an absolute error of during the computation;
- An absolute error criterion was considered to stop the exploration criteria. The travelling wave speed for which both solutions, the numerically exact one and the analytical approach, were sufficiently close with an absolute error of , named as the critical . For this particular speed, The analytical solution in (39) can be regarded as a valid solution to the problem (34);
- The associated fluid constants in (34) were as one. The travelling wave speed a was the parameter used in the search for an analytical profile matching the error tolerance. In addition and with no loss of generality, . Note that this particular selection of constant values did not impact the ending conclusions, i.e., on the existence of an analytical exponential profile matching the exact solution for a certain value in the travelling wave speed.
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Metzner, A.; Otto, R. Agitation of non-Newtonian fluids. AIChE J. 1957, 3, 3–10. [Google Scholar] [CrossRef]
- Rajagopal, K. A note on unsteady unidirectional flows of a non-Newtonian fluid. Int. J. Non Linear Mech. 1982, 17, 369–373. [Google Scholar] [CrossRef]
- Rajagopal, K.; Gupta, A. An exact solution for the flow of a nonNewtonian fluid past an infinite porous plate. Meccanica 1984, 19, 158–160. [Google Scholar] [CrossRef]
- Eldabe, N.; Hassan, A.; Mohamed, M.A. Effect of couple stresses on the MHD of a non-Newtonian unsteady flow between two parallel porous plates. Z. Naturforschung A 2003, 58, 204–210. [Google Scholar] [CrossRef] [Green Version]
- Shao, S.; Lo, E.Y. Incompressible SPH method for simulating Newtonian and non-Newtonian flows with a free surface. Adv. Water Resour. 2003, 26, 787–800. [Google Scholar] [CrossRef]
- Fetecau, C. Analytical solutions for non-Newtonian fluid flows in pipe-like domains. Int. J. Non-Linear Mech. 2004, 39, 225–231. [Google Scholar] [CrossRef]
- Akbar, N.S.; Ebaid, A.; Khan, Z. Numerical analysis of magnetic field effects on Eyring–Powell fluid flow towards a stretching sheet. J. Magn. Magn. Mater. 2015, 382, 355–358. [Google Scholar] [CrossRef]
- Hina, S. MHD peristaltic transport of Eyring–Powell fluid with heat/mass transfer, wall properties and slip conditions. J. Magnetism. Magn. Mater. 2016, 404, 148–158. [Google Scholar] [CrossRef]
- Bhatti, M.; Abbas, T.; Rashidi, M.; Ali, M.; Yang, Z. Entropy generation on MHD Eyring–Powell nanofluid through a permeable stretching surface. Entropy 2016, 18, 224. [Google Scholar] [CrossRef] [Green Version]
- Ara, A.; Khan, N.A.; Khan, H.; Sultan, F. Radiation effect on boundary layer flow of an Eyring–Powell fluid over an exponentially shrinking sheet. Ain Shams Eng. J. 2004, 5, 1337–1342. [Google Scholar] [CrossRef] [Green Version]
- Hayat, T.; Iqbal, Z.; Qasim, M.; Obaidat, S. Steady flow of an Eyring–Powell fluid over a moving surface with convective boundary conditions. Int. J. Heat Mass Transfer. 2012, 55, 1817–1822. [Google Scholar] [CrossRef]
- Hayat, T.; Awais, M.; Asghar, S. Radiactive effects in a three dimensional flow of MHD Eyring–Powell fluid. J. Egypt Math. Soc. 2013, 21, 379–384. [Google Scholar] [CrossRef] [Green Version]
- Jalil, M.; Asghar, S.; Imran, S.M. Self similar solutions for the flow and heat transfer of Powell-Eyring fluid over a moving surface in parallel free stream. Int. J. Heat Mass Transf. 2013, 65, 73–79. [Google Scholar] [CrossRef]
- Khan, J.A.; Mustafa, M.; Hayat, T.; Farooq, M.A.; Alsaedi, A.; Liao, S.J. On model for three-dimensional flow of nanofluid: An application to solar energy. J. Mol. Liq. 2014, 194, 41–47. [Google Scholar] [CrossRef]
- Riaz, A.; Ellahi, R.; Sait, S.M. Role of hybrid nanoparticles in thermal performance of peristaltic flow of Eyring–Powell fluid model. J. Therm. Anal. Calorim. 2021, 143, 1021–1035. [Google Scholar] [CrossRef]
- Gholinia, M.; Hosseinzadeh, K.; Mehrzadi, H.; Ganji, D.D.; Ranjbar, A.A. Investigation of MHD Eyring–Powell fluid flow over a rotating disk under effect of homogeneous–heterogeneous reactions. Case Stud. Therm. Eng. 2019, 13, 100356. [Google Scholar] [CrossRef]
- Umar, M.; Akhtar, R.; Sabir, Z.; Wahab, H.A.; Zhiyu, Z.; Imran, A.; Shoaib, M.; Raja, M.A.Z. Numerical treatment for the three-dimensional Eyring–Powell fluid flow over a stretching sheet with velocity slip and activation energy. Adv. Math. Phys. 2019, 2019, 9860471. [Google Scholar] [CrossRef] [Green Version]
- Nazeer, M.; Ahmad, F.; Saeed, M.; Saleem, A.; Naveed, S.; Akram, Z. Numerical solution for flow of a Eyring–Powell fluid in a pipe with prescribed surface temperature. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 1–10. [Google Scholar] [CrossRef]
- Talebizadeh, P.; Moghimi, M.A.; Kimiaeifar, A.; Ameri, M. Numerical and analytical solutions for natural convection flow with thermal radiation and mass transfer past a moving vertical porous plate by DQM and HAM. Int. J. Comput. Methods 2011, 8, 611–631. [Google Scholar] [CrossRef]
- Noghrehabadi, A.; Ghalambaz, M.; Ghanbarzadeh, A. A new approach to the electrostatic pull-in instability of nanocantilever actuators using the ADM–Padé technique. Comput. Math. Appl. 2012, 64, 2806–2815. [Google Scholar] [CrossRef] [Green Version]
- Noghrehabadi, A.; Mirzaei, R.; Ghalambaz, M.; Chamkha, A.; Ghanbarzadeh, A. Boundary layer flow heat and mass transfer study of Sakiadis flow of viscoelastic nanofluids using hybrid neural network-particle swarm optimization (HNNPSO). Therm. Sci. Eng. Prog. 2017, 4, 150–159. [Google Scholar] [CrossRef]
- Hayat, T.; Tanveer, A.; Yasmin, H.; Alsaadi, F. Simultaneous effects of Hall current and thermal deposition in peristaltic transport of Eyring–Powell fluid. Int. J. Biomath. 2015, 8, 1550024. [Google Scholar] [CrossRef]
- Kolmogorov, A.N.; Petrovskii, I.G.; Piskunov, N.S. Study of the diffusion equation with growth of the quantity of matter and its application to a biological problem. Byull. Moskov. Gos. Univ. 1937, 1, 1–25. [Google Scholar]
- Fisher, R.A. The advance of advantageous genes. Ann. Eugen. 1937, 7, 355–369. [Google Scholar] [CrossRef] [Green Version]
- Bilal, M.; Ashbar, S. Flow and heat transfer analysis of Eyring–Powell fluid over stratified sheet with mixed convection. J. Egypt. Math. Soc. 2020, 28, 40. [Google Scholar] [CrossRef]
- Ramzan, M.; Bilal, M.; Kanwal, S.; Chung, J.D. Effects of variable thermal conductivity and nonlinear thermal radiation past an Eyring–Powell nanofluid flow with chemical reaction. Commun. Theor. Phys. 2017, 67, 723–731. [Google Scholar] [CrossRef]
- Pablo, A.D. Estudio de una Ecuación de Reacción—Difusión. Ph.D. Thesis, Universidad Autónoma de Madrid, Madrid, Spain, 1989. [Google Scholar]
- Pablo, A.D.; Vázquez, J.L. Travelling waves and finite propagation in a reaction–diffusion Equation. J Differ. Equ. 1991, 93, 19–61. [Google Scholar] [CrossRef] [Green Version]
- Fenichel, N. Persistence and smoothness of invariant manifolds for flows. Indiana Univ. Math. J. 1971, 21, 193–226. [Google Scholar] [CrossRef]
- Jones, C.K.R. Geometric Singular Perturbation Theory in Dynamical Systems; Springer: Berlin/Heidelberg, Germany, 1995. [Google Scholar]
- Enright, H.; Muir, P.H. A Runge-Kutta Type Boundary Value ODE Solver with Defect Control; Teh. Rep. 267/93; University of Toronto, Dept. of Computer Sciences: Toronto, ON, Canada, 1993. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Díaz, J.L.; Rahman, S.U.; Sánchez Rodríguez, J.C.; Simón Rodríguez, M.A.; Filippone Capllonch, G.; Herrero Hernández, A. Analysis of Solutions, Asymptotic and Exact Profiles to an Eyring–Powell Fluid Modell. Mathematics 2022, 10, 660. https://doi.org/10.3390/math10040660
Díaz JL, Rahman SU, Sánchez Rodríguez JC, Simón Rodríguez MA, Filippone Capllonch G, Herrero Hernández A. Analysis of Solutions, Asymptotic and Exact Profiles to an Eyring–Powell Fluid Modell. Mathematics. 2022; 10(4):660. https://doi.org/10.3390/math10040660
Chicago/Turabian StyleDíaz, José Luis, Saeed Ur Rahman, Juan Carlos Sánchez Rodríguez, María Antonia Simón Rodríguez, Guillermo Filippone Capllonch, and Antonio Herrero Hernández. 2022. "Analysis of Solutions, Asymptotic and Exact Profiles to an Eyring–Powell Fluid Modell" Mathematics 10, no. 4: 660. https://doi.org/10.3390/math10040660
APA StyleDíaz, J. L., Rahman, S. U., Sánchez Rodríguez, J. C., Simón Rodríguez, M. A., Filippone Capllonch, G., & Herrero Hernández, A. (2022). Analysis of Solutions, Asymptotic and Exact Profiles to an Eyring–Powell Fluid Modell. Mathematics, 10(4), 660. https://doi.org/10.3390/math10040660





