Three-Stage-Impulse Control of Memristor-Based Chen Hyper-Chaotic System
Abstract
1. Introduction
- (i)
- The memrisive hyper-chaotic system is successfully constructed, and the results of numerical simulation and circuit simulation are basically consistent;
- (ii)
- The modular design of the system circuit is simple, using relatively few components, and the circuit parameters can be determined directly;
- (iii)
- A new control scheme is proposed that can be extended to other nonlinear systems.
2. Formulation of a New Memristor-Based Hyper-Chaotic System
3. Model Description and Some Initial Conditions
4. Theoretical Analysis and Main Results
5. Numerical Simulation Example
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lorenz, E.N. Deterministic nonperiodic flow. J. Atmos. Sci. 1963, 20, 130–141. [Google Scholar] [CrossRef]
- Ueta, T.; Chen, G. Bifurcation analysis of Chen’s equation. Int. J. Bifurc. Chaos 2000, 10, 1917–1931. [Google Scholar] [CrossRef]
- Wu, C.; Zhang, Y.; Yang, N. Analysis of a novel four-wing hyperchaotic system from pseudo to real and circuit experimental research. In Proceedings of the 2014 International Conference on Information Science, Electronics and Electrical Engineering (ISEEE), Harbin, China, 17–18 May 2014; pp. 1138–1142. [Google Scholar] [CrossRef]
- Bao, B.; Liu, Z.; Xu, J. New chaotic system and its hyperchaos generation. J. Syst. Eng. Electron. 2009, 20, 1179–1187. [Google Scholar]
- Zhang, S.; Zeng, Y.; Li, Z. A novel four-dimensional no-equilibrium hyper-chaotic system with grid multiwing hyper-chaotic hidden attractors. J. Comput. Nonlinear Dyn. 2018, 13, 090908. [Google Scholar] [CrossRef]
- Laarem, G. A new 4-D hyper chaotic system generated from the 3-D Rösslor chaotic system, dynamical analysis, chaos stabilization via an optimized linear feedback control, it’s fractional order model and chaos synchronization using optimized fractional order sliding mode control. Chaos Soliton Fractals 2021, 152, 111437. [Google Scholar] [CrossRef]
- Chua, L.O. Memristor-the missing circuit element. IEEE Trans. Circuit Theory 1971, 18, 507–519. [Google Scholar] [CrossRef]
- Strukov, D.B.; Snider, G.S.; Stewart, D.R.; Williams, R.S. The missing memristor found. Nature 2008, 453, 80–83. [Google Scholar] [CrossRef]
- Sahin, M.; Taskiran, Z.C.; Guler, H.; Hamamci, S. Simulation and implementation of memristive chaotic system and its application for communication systems. Sens. Actuators A-Phys. 2019, 290, 107–118. [Google Scholar] [CrossRef]
- Itoh, M.; Chua, L.O. Memristor oscillators. Int. J. Bifurc. Chaos 2008, 18, 3183–3206. [Google Scholar] [CrossRef]
- Bao, B.; Bao, H.; Wang, N.; Xu, Q. Hidden extreme multistability in memristive hyperchaotic system. Chaos Soliton Fractals 2017, 94, 102–111. [Google Scholar] [CrossRef]
- Xi, H.; Li, Y.; H, X. Generation and nonlinear dynamical analyses of fractional-order memristor-based Lorenz systems. Entropy 2014, 16, 6240–6253. [Google Scholar] [CrossRef]
- Wang, X.; Min, X.; Zhou, P.; Yu, D. Hyperchaotic circuit based on memristor feedback with multistability and symmetries. Complexity 2020, 2020, 2620375. [Google Scholar] [CrossRef]
- Wang, M.; Deng, Y.; Liao, X.; Li, Z.; Ma, M.; Zeng, Y. Dynamics and circuit implementation of a four-wing memristive chaotic system with attractor rotation. Int. J. Nonlinear Mech. 2019, 111, 149–159. [Google Scholar] [CrossRef]
- Tu, Z.; Wang, D.; Yang, X.; Cao, J. Lagrange stability of memristive quaternion-valued neural networks with neutral items. Neurocomputing 2020, 339, 380–389. [Google Scholar] [CrossRef]
- Chen, M.; Sun, M.; Bao, B.; Wu, H.; Xu, Q.; Wang, J. Controlling extreme multistability of memristor emulator-based dynamical circuit in flux–charge domain. Nonlinear Dyn. 2018, 91, 1395–1412. [Google Scholar] [CrossRef]
- Li, H.; Yang, Y.; Li, W.; He, S.; Li, C. Extremely rich dynamics in a memristor-based chaotic system. Eur. Phys. J. Plus 2020, 135, 579. [Google Scholar] [CrossRef]
- Lai, Q.; Wan, Z.; Kuate, P.D.; Fotsin, H. Coexisting attractors, circuit implementation and synchronization control of a new chaotic system evolved from the simplest memristor chaotic circuit. Commun. Nonlinear Sci. 2020, 89, 105341. [Google Scholar] [CrossRef]
- Huang, L.; Wang, Y.; Jiang, Y.; Lei, T. A Novel Memristor Chaotic System with a Hidden Attractor and Multistability and Its Implementation in a Circuit. Math. Probl. Eng. 2021, 2021, 7457220. [Google Scholar] [CrossRef]
- Akif, A.; Karthikeyan, R.; Ali, D.; Muhammed, A.P.; Mustafa, Z.Y. A simple fractional-order chaotic system based on memristor and memcapacitor and its synchronization application. Chaos Soliton Fractals 2021, 152, 111306. [Google Scholar] [CrossRef]
- Wang, Z.; Qi, G. Modeling and analysis of a three-terminal-memristor-based conservative chaotic system. Entropy 2021, 23, 71. [Google Scholar] [CrossRef]
- Wang, X.; Gao, M.; Lu, H.C.; Wang, C. Tri-valued memristor-based hyper-chaotic system with hidden and coexistent attractors. Chaos Soliton Fractals 2022, 159, 112177. [Google Scholar] [CrossRef]
- Khan, A.; Tyagi, B. Analysis and hyper-chaos control of a new 4-D hyper-chaotic system by using optimal and adaptive control design. Int. J. Dyn. Control 2017, 5, 1147–1155. [Google Scholar] [CrossRef]
- Vaidyanathan, S.; Abba, O.A.; Gambo, B.; Alidou, M. A new three-dimensional chaotic system: Its adaptive control and circuit design. Int. J. Autom. Control 2019, 13, 101–121. [Google Scholar] [CrossRef]
- Li, D.; Lu, S.; Liu, L. Adaptive NN cross backstepping control for nonlinear systems with partial time-varying state constraints and its applications to hyper-chaotic systems. IEEE. Trans. Syst. Man. Cybern. Syst. 2021, 51, 2821–2832. [Google Scholar] [CrossRef]
- Tu, Z.; Ding, N.; Li, L.; Feng, Y.; Zou, L.; Zhang, W. Adaptive synchronization of memristive neural networks with time-varying delays and reaction-diffusion term. Appl. Math. Comput. 2017, 311, 118–128. [Google Scholar] [CrossRef]
- Hosseinabadi, P.A.; Abadi, A.S.S.; Mekhilef, S. Adaptive terminal sliding mode control of hyper-chaotic uncertain 4-order system with one control input. In Proceedings of the 2018 IEEE Conference on Systems, Process and Control (ICSPC), Melaka, Malaysia, 31 August–14 December 2018; pp. 94–99. [Google Scholar] [CrossRef]
- Mofid, O.; Momeni, M.; Mobayen, S.; Fekih, A. A Disturbance-Observer-Based Sliding Mode Control for the Robust Synchronization of Uncertain Delayed Chaotic Systems: Application to Data Security. IEEE Access 2021, 9, 16546–16555. [Google Scholar] [CrossRef]
- Lee, S.; Park, M.; Kwon, O.; Selvaraj, P. Improved Synchronization Criteria for Chaotic Neural Networks with Sampled-data Control Subject to Actuator Saturation. Int. J. Control Autom. 2019, 17, 2430–2440. [Google Scholar] [CrossRef]
- Wu, H.; Li, C.; He, Z.; Wang, Y.; He, Y. Lag synchronization of nonlinear dynamical systems via asymmetric saturated impulsive control. Chaos Soliton Fractals 2021, 152, 111290. [Google Scholar] [CrossRef]
- Sabaghian, A.; Balochian, S. Parameter estimation and synchronization of hyper chaotic Lu system with disturbance input and uncertainty using two under-actuated control signals. Trans. Inst. Meas. Control 2019, 41, 1729–1739. [Google Scholar] [CrossRef]
- Sabaghian, A.; Balochian, S.; Yaghoobi, M. Synchronisation of 6D hyper-chaotic system with unknown parameters in the presence of disturbance and parametric uncertainty with unknown bounds. Connect. Sci. 2020, 32, 362–383. [Google Scholar] [CrossRef]
- Yang, X.; Liu, Y.; Cao, J.; Leszek, R. Synchronization of coupled time-delay neural networks with mode-dependent average dwell time switching. IEEE Trans. Neural Netw. Learn. Syst. 2020, 31, 5483–5496. [Google Scholar] [CrossRef]
- Yang, X.; Wan, X.; Cheng, Z.; Cao, J.; Liu, Y.; Leszek, R. Synchronization of switched discrete-time neural networks via quantized output control with actuator fault. IEEE Trans. Neural Netw. Learn. Syst. 2020, 32, 4191–4201. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Ke, T.; Xia, Q.; Xie, C.; Xu, Y. Finite-Time Impulsive Control of Financial Risk Dynamic System with Chaotic Characteristics. Connect. Sci. 2021, 2021, 5207154. [Google Scholar] [CrossRef]
- Li, X.; Li, P. Stability of time-delay systems with impulsive control involving stabilizing delays. Automatica 2021, 124, 109336. [Google Scholar] [CrossRef]
- Li, C.; Wu, S.; Feng, G.; Liao, X. Stabilizing effects of impulses in discrete-time delayed neural networks. IEEE Trans. Neural Netw. 2011, 22, 323–329. [Google Scholar] [CrossRef] [PubMed]
- Liao, C.; Tu, D.; Feng, Y.; Zhang, W.; Wang, Z.; Onasanya, B.O. A Sandwich Control System with Dual Stochastic Impulses. IEEE/CAA J. Autom. Sin. 2022, 9, 741–744. [Google Scholar] [CrossRef]
- Li, C.; Feng, G.; Huang, T. On hybrid impulsive and switching neural networks. IEEE Trans. Syst. Man Cybern. 2008, 38, 1549–1560. [Google Scholar] [CrossRef]
- Bao, B.; Xu, J.; Liu, Z. Initial state dependent dynamical behaviors in a memristor based chaotic circuit. Chaos Soliton Fractals 2010, 27, 070504. [Google Scholar] [CrossRef]
- Wang, W.; Zeng, Y.; Sun, R. Research on a six-order chaotic circuit with three memristors. Acta Phys. Sin. 2017, 66. [Google Scholar] [CrossRef]
- Wolf, A.; Swift, J.B.; Swinney, H.L.; Vastano, J.A. Determining Lyapunov exponents from a time series. Physica D 1985, 16, 285–317. [Google Scholar] [CrossRef]
- Leutcho, G.; Kengne, J.; Kengne, L.K. Dynamical analysis of a novel autonomous 4-D hyperjerk circuit with hyperbolic sine nonlinearity: Chaos, antimonotonicity and a plethora of coexisting attractors. Chaos Soliton Fractals 2018, 107, 67–87. [Google Scholar] [CrossRef]
- Frederickson, P.; Kaplan, J.L.; Yorke, E.D.; Yorke, J.A. The Liapunov dimension of strange attractors. J. Differ. Equ. 1983, 49, 185–207. [Google Scholar] [CrossRef]
- Gabelli, J.; Fve, G.; Berroir, B.; Etienne, B.; Glattli, D. Violation of Kirchhoff’s Laws for a Coherent RC Circuit. Science 2006, 313, 499–502. [Google Scholar] [CrossRef] [PubMed]
- Feng, Y.; Li, C.; Huang, T. Sandwich control systems. In Proceedings of the 2015 Sixth International Conference on Intelligent Control and Information Processing (ICICIP), Wuhan, China, 26–28 November 2015; pp. 1–5. [Google Scholar] [CrossRef]
- Sanchez, E.N.; Perez, J.P. Input-to-state stability (ISS) analysis for dynamic neural networks. Chaos Soliton Fractals 1999, 46, 1395–1398. [Google Scholar] [CrossRef]
- Boyd, S.; Ghaoui, L.E.; Feron, E. Linear Matrix Inequalities in System and Control Theory. Chaos Soliton Fractals 1994, 15, 157–193. [Google Scholar] [CrossRef]



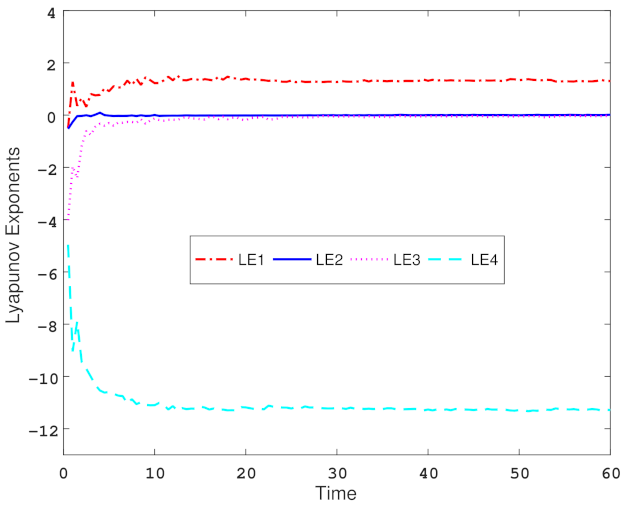
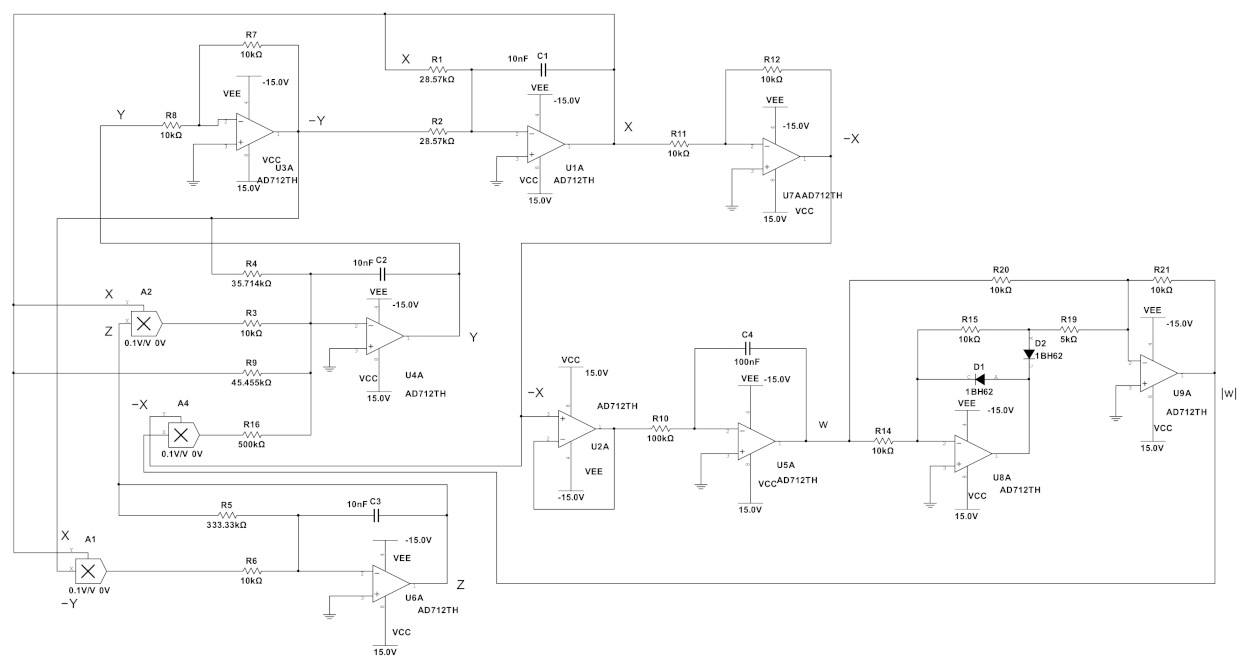
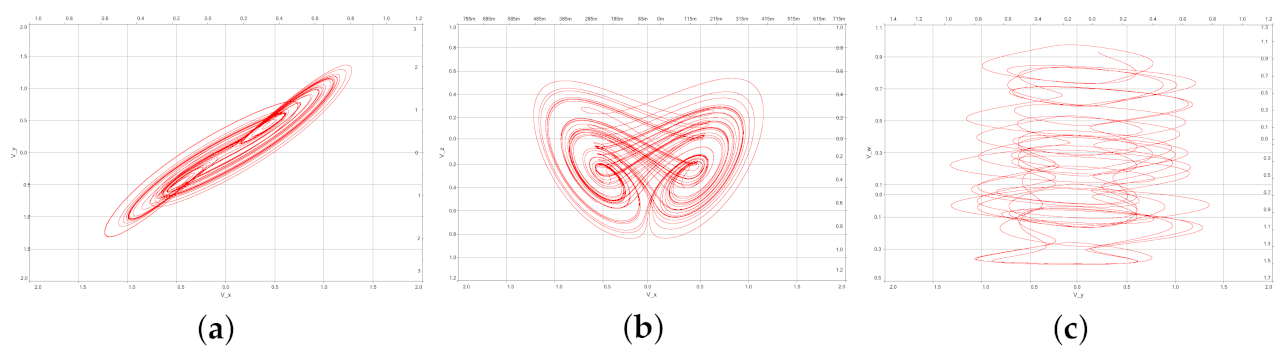

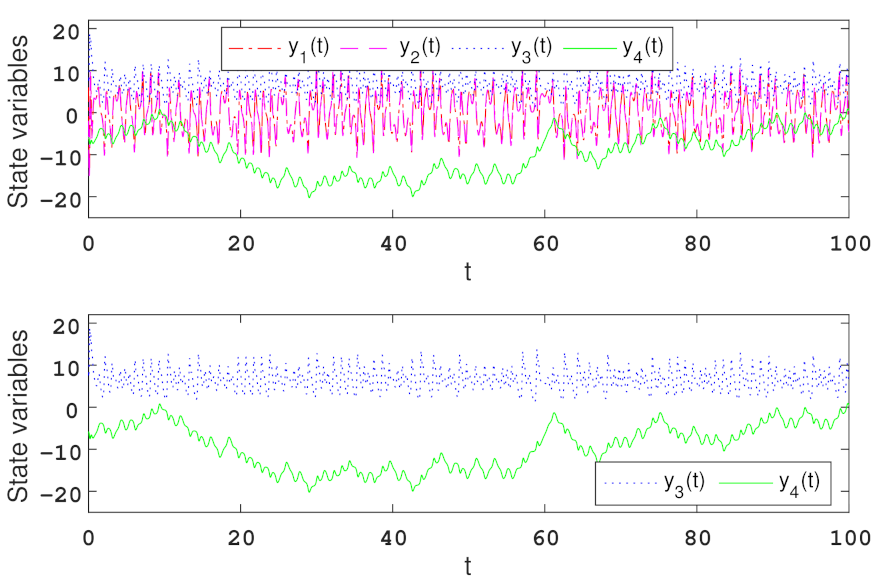
| Time | ||||
|---|---|---|---|---|
| ⋮ | ⋮ | ⋮ | ⋮ | ⋮ |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, X.; Wen, S.; Feng, Y.; Onasanya, B.O. Three-Stage-Impulse Control of Memristor-Based Chen Hyper-Chaotic System. Mathematics 2022, 10, 4560. https://doi.org/10.3390/math10234560
Xie X, Wen S, Feng Y, Onasanya BO. Three-Stage-Impulse Control of Memristor-Based Chen Hyper-Chaotic System. Mathematics. 2022; 10(23):4560. https://doi.org/10.3390/math10234560
Chicago/Turabian StyleXie, Xianyang, Shiping Wen, Yuming Feng, and Babatunde Oluwaseun Onasanya. 2022. "Three-Stage-Impulse Control of Memristor-Based Chen Hyper-Chaotic System" Mathematics 10, no. 23: 4560. https://doi.org/10.3390/math10234560
APA StyleXie, X., Wen, S., Feng, Y., & Onasanya, B. O. (2022). Three-Stage-Impulse Control of Memristor-Based Chen Hyper-Chaotic System. Mathematics, 10(23), 4560. https://doi.org/10.3390/math10234560







