Several Types of q-Differential Equations of Higher Order and Properties of Their Solutions
Abstract
1. Preliminaries and Introduction
2. Various q-Differential Equations of Higher Order Forms Related to q-Sigmoid Polynomials
3. Properties and Structures of Approximation Roots of q-Sigmoid Polynomials
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jackson, H.F. q-Difference equations. Am. J. Math 1910, 32, 305–314. [Google Scholar] [CrossRef]
- Kac, V.; Cheung, P. Quantum Calculus; Springer: Berlin/Heidelberg, Germany, 2002; ISBN 0-387-95341-8. [Google Scholar]
- Bangerezako, G. An Introduction to q-Difference Equations; University of Burundi: Bujumbura, Burundi, 2008; preprint. [Google Scholar]
- Comtet, L. Advanced Combinatorics; Reidel: Dordrecht, The Netherlands, 1974. [Google Scholar]
- Carmichael, R.D. The general theory of linear q-qifference equations. Am. J. Math. 1912, 34, 147–168. [Google Scholar] [CrossRef]
- Jackson, H.F. On q-functions and a certain difference operator. Trans. R. Soc. Edinb. 1950, 46, 253–281. [Google Scholar] [CrossRef]
- Konvalina, J. A unified interpretation of the binomial coefficients, the Stirling numbers, and the Gaussian coefficients. Amer. Math. Mon. 2000, 107, 10. [Google Scholar] [CrossRef]
- Mason, T.E. On properties of the solution of linear q-difference equations with entire function coefficients. Am. J. Math. 1915, 37, 439–444. [Google Scholar] [CrossRef]
- Rodrigues, P.S.; Wachs-Lopes, G.; Santos, R.M.; Coltri, E.; Giraldi, G.A. A q-extension of sigmoid functions and the application for enhancement of ultrasound images. Entropy 2019, 21, 430. [Google Scholar] [CrossRef] [PubMed]
- Trjitzinsky, W.J. Analytic theory of linear q-difference equations. Acta Math. 1933, 61, 1–38. [Google Scholar] [CrossRef]
- Silindir, B.; Yantir, A. Generalized quantum exponential function and its applications. Filomat 2019, 33, 15. [Google Scholar] [CrossRef]
- Zil, D.G. A First Course in Differential Equations: With Modeling Applications, 9th ed.; Brooks/Cole; Cengage Learning, Inc.: Boston, MA, USA, 2009; ISBN -13: 978-04951082452009. [Google Scholar]
- Han, J.; Wilson, R.S.; Leurgans, S.E. Sigmoidal mixed models for longitudinal data. Stat. Methods Med. Res. 2018, 27, 863–875. [Google Scholar] [CrossRef]
- Ito, Y. Representation of functions by superpositions of a step or sigmoid function and their applications to neural network theory. Neural Netw. 1991, 4, 385–394. [Google Scholar] [CrossRef]
- Kim, M.; Song, Y.; Wang, S.; Xia, Y.; Jiang, X. Secure Logistic Regression Based on Homomorphic Encryption: Design and Evaluation. JMIR Med. Inform. 2018, 6, e19. [Google Scholar] [CrossRef] [PubMed]
- Kang, J.Y. Some relationships between sigmoid polynomials and other polynomials. J. Appl. Pure Math. 2019, 1, 1–2. [Google Scholar]
- Han, J.; Moraga, C. The influence of the sigmoid function parameters on the speed of backpropagation learning. In Proceedings of the International Workshop on Artificial Neural Networks, Malaga-Torremolinos, Spain, 7–9 June 1995. [Google Scholar] [CrossRef]
- Kang, J.Y. Some properties and distribution of the zeros of the q-sigmoid polynomials. Discret. Dyn. Nat. Soc. 2020, 2020, 4169840. [Google Scholar] [CrossRef]
- Kwan, H.K. Simple sigmoid-like activation function suitable for digital hardware implementation. Electron. Lett. 1992, 28, 1379–1380. [Google Scholar] [CrossRef]
- Ryoo, C.S.; Kang, J.Y. Phenomenon of scattering of zeros of the (p,q)-cosine sigmoid polynomials and (p,q)-sine sigmoid polynomials. Fractal Fract. 2021, 5, 245. [Google Scholar] [CrossRef]
- Andrews, G.E.; Askey, R.; Roy, R. Special Functions; Cambridge Press: Cambridge, UK, 1999. [Google Scholar]
- Gi-Sang, C. A note on the Bernoulli and Euler polynomials. Appl. Math. Lett. 2003, 16, 3. [Google Scholar]
- Endre, S.; David, M. An Introduction to Numerical Analysis; Cambridge University Press: Cambridge, UK, 2003; ISBN 0-521-00794-1. [Google Scholar]
- Ryoo, C.S. Some identities involving the generalized polynomials of derangements arising from differential equation. J. Appl. Math. Inform. 2020, 38, 159–173. [Google Scholar]

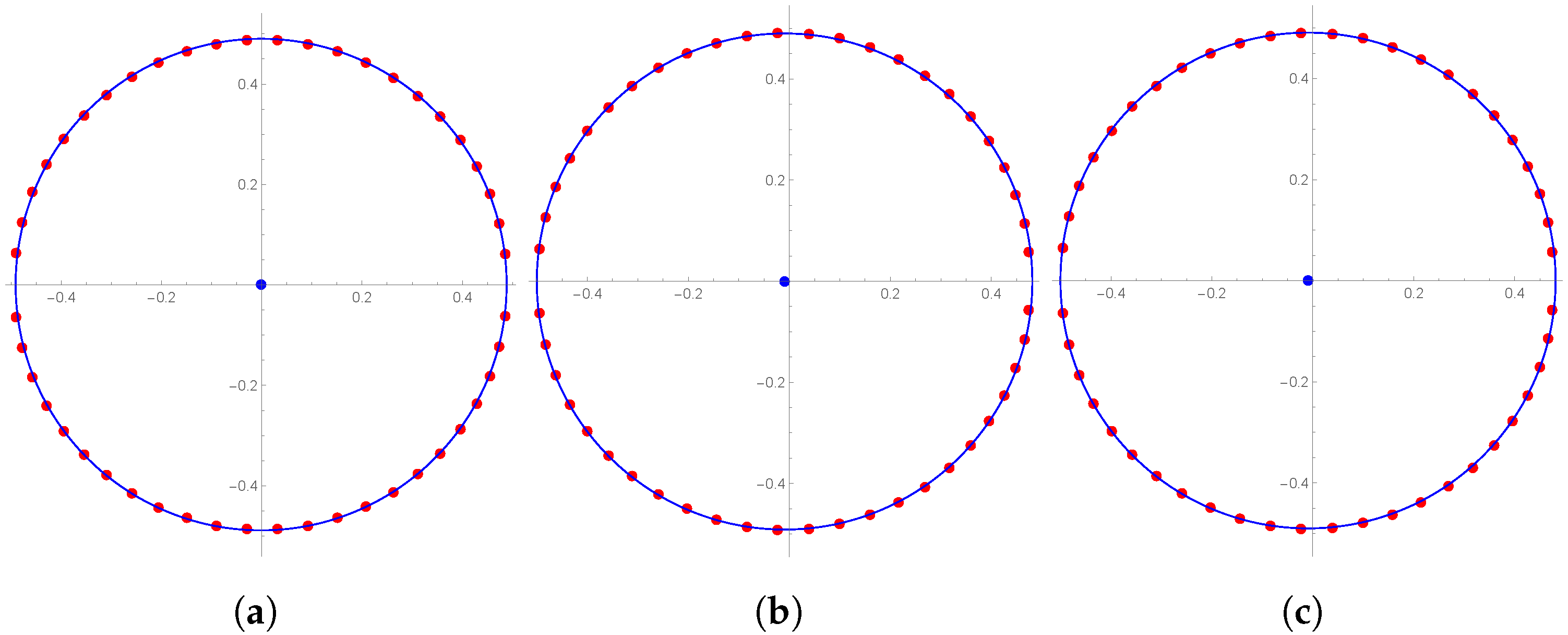
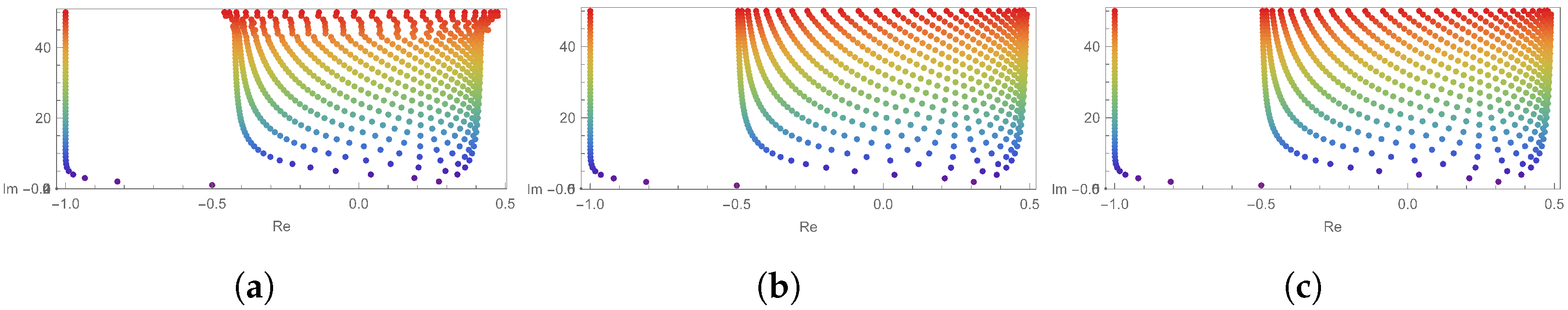
| The Center (x, y) | |||
|---|---|---|---|
| q | 0.01 | 0.001 | 0.0001 | |
|---|---|---|---|---|
| n | ||||
| ⋮ | ⋮ | ⋮ | ⋮ | |
| 5 | ||||
| 6 | , | , | , | |
| 7 | ||||
| 8 | , | , | , | |
| 9 | ||||
| 10 | , | , | , | |
| ⋮ | ⋮ | ⋮ | ⋮ | |
| 19 | ||||
| 20 | , | , | , | |
| ⋮ | ⋮ | ⋮ | ⋮ | |
| 29 | ||||
| 30 | , | , | , | |
| ⋮ | ⋮ | ⋮ | ⋮ | |
| 39 | ||||
| 40 | , | , | , | |
| ⋮ | ⋮ | ⋮ | ⋮ | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ryoo, C.-S.; Kang, J.-Y. Several Types of q-Differential Equations of Higher Order and Properties of Their Solutions. Mathematics 2022, 10, 4469. https://doi.org/10.3390/math10234469
Ryoo C-S, Kang J-Y. Several Types of q-Differential Equations of Higher Order and Properties of Their Solutions. Mathematics. 2022; 10(23):4469. https://doi.org/10.3390/math10234469
Chicago/Turabian StyleRyoo, Cheon-Seoung, and Jung-Yoog Kang. 2022. "Several Types of q-Differential Equations of Higher Order and Properties of Their Solutions" Mathematics 10, no. 23: 4469. https://doi.org/10.3390/math10234469
APA StyleRyoo, C.-S., & Kang, J.-Y. (2022). Several Types of q-Differential Equations of Higher Order and Properties of Their Solutions. Mathematics, 10(23), 4469. https://doi.org/10.3390/math10234469






