A Novel Cam-Based Variable Stiffness Actuator: Pitch Curve Synthetic Approach for Reconfiguration Design
Abstract
:1. Introduction
2. Reconfiguration Method of the Cam-Based VSA
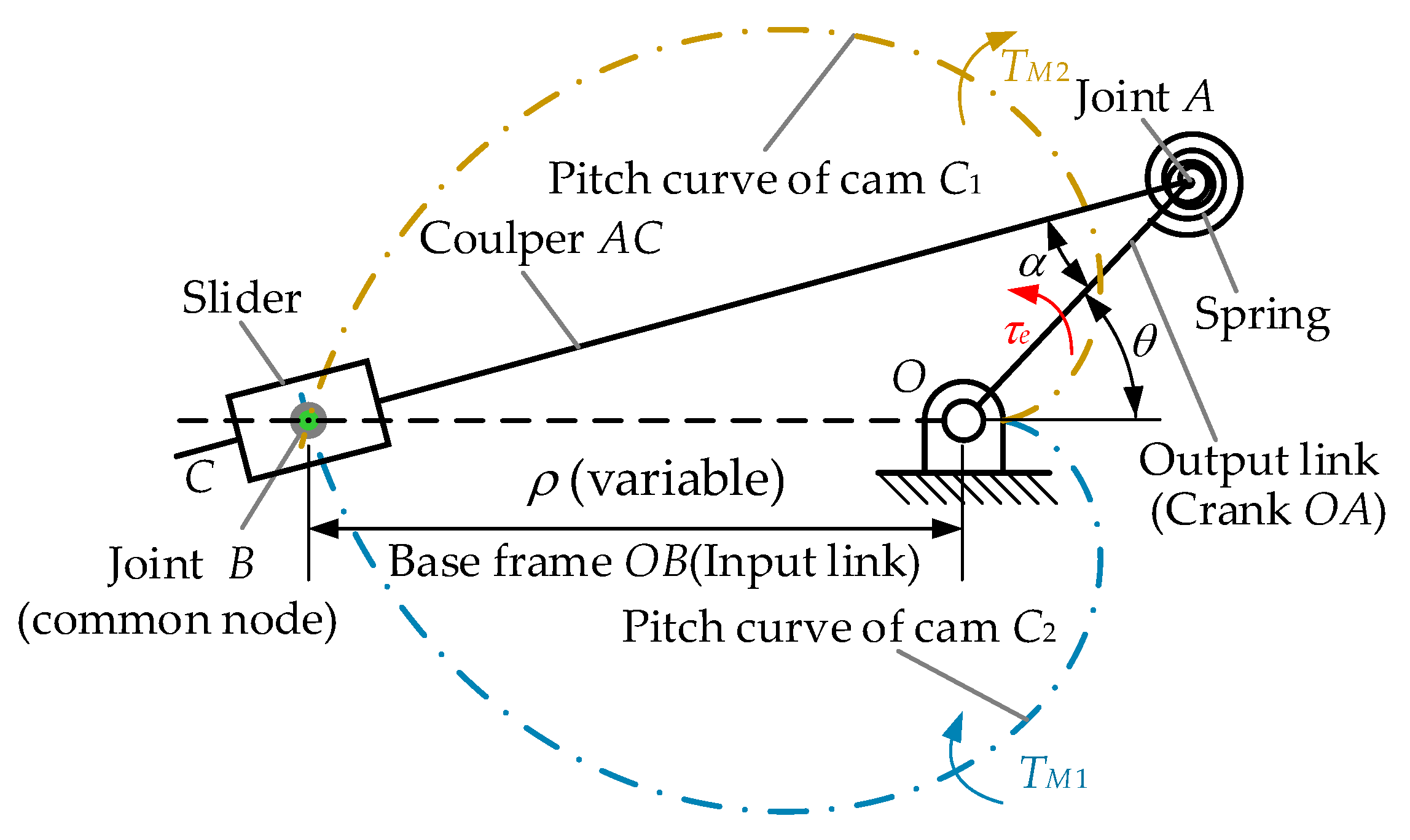
2.1. Problem Description
2.2. Reconfiguration Method of the Cam-Based VSA for High Versatility
3. The Cam Pitch Curve Synthetic Approach for Reconfiguration Design
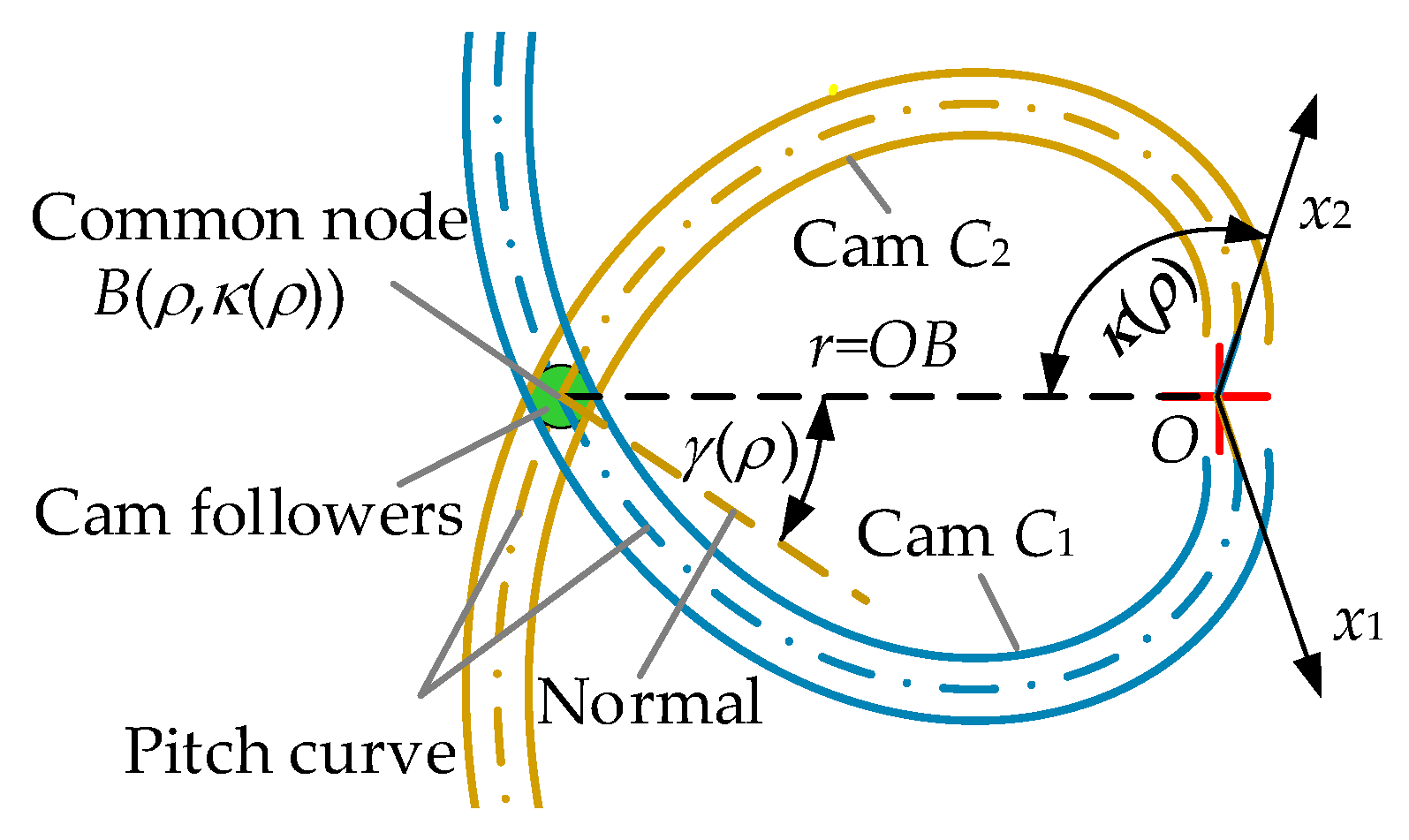
3.1. Cam Pitch Curve Modeling
3.2. Feasible Solution Space of Cam Reconfiguration under the Basic Constraints
3.3. Cam Reconfiguration Synthetic Based on Different Performance Index of the Variable Stiffness Actuator
3.4. Three Cam Reconfiguration Results Based on Three Special Cases
4. Experiment Verification and Analysis of the Optimal Load Design
4.1. Load Distribuation Erification by Adams Simulation
- (1)
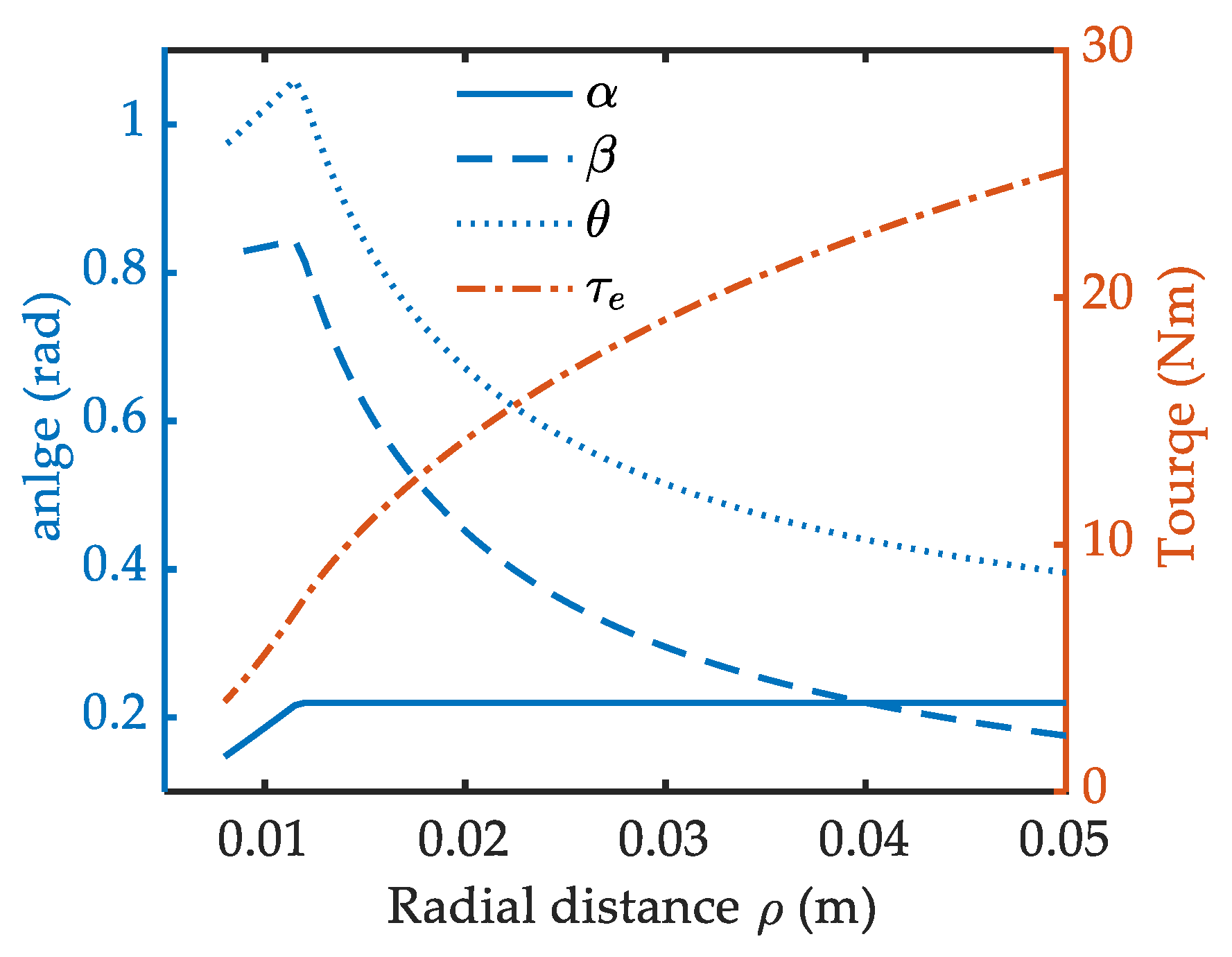
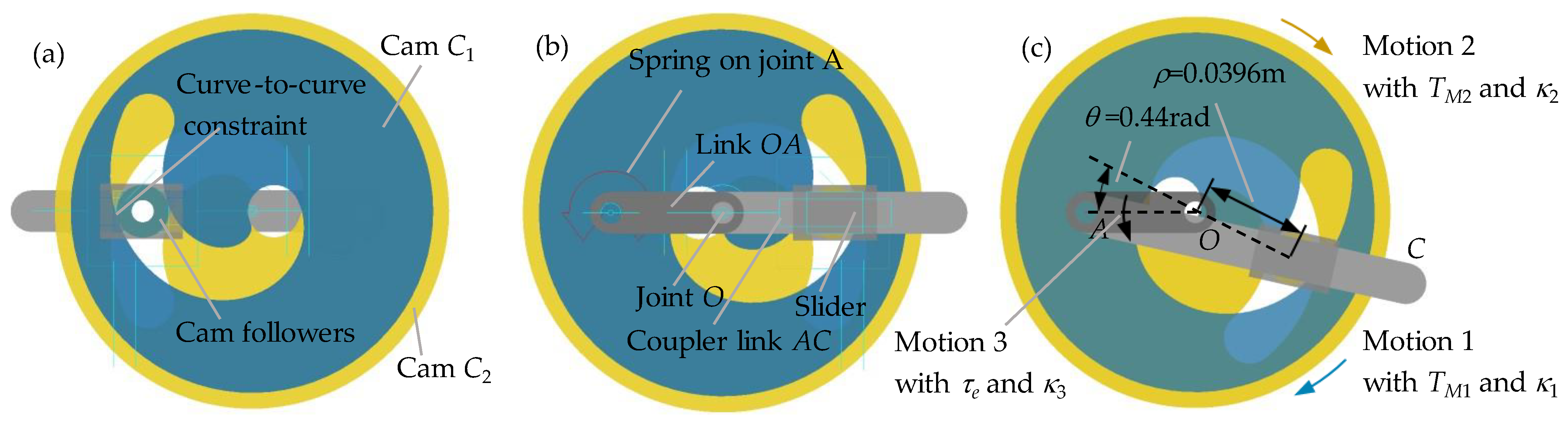
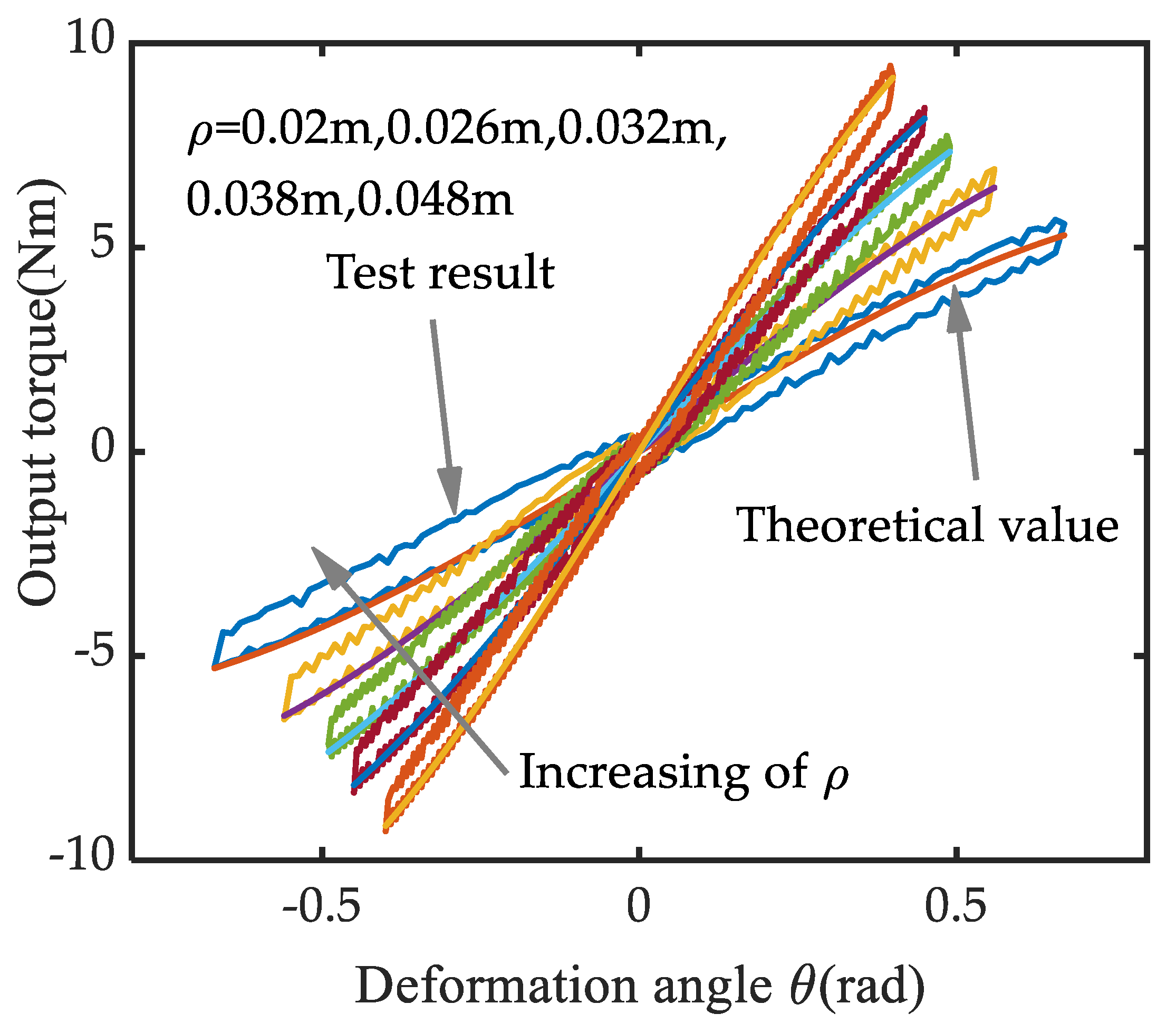
- First, adjust the value of ρ with no deformation by the reverse motion of cams C1 and C2. The measurement range varies from ρ = 0.008 m to ρ = 0.05 m, with a step of 0.002 m. The motion angle κ1 and κ2 of cams C1 and C2 are decided by
- (2)
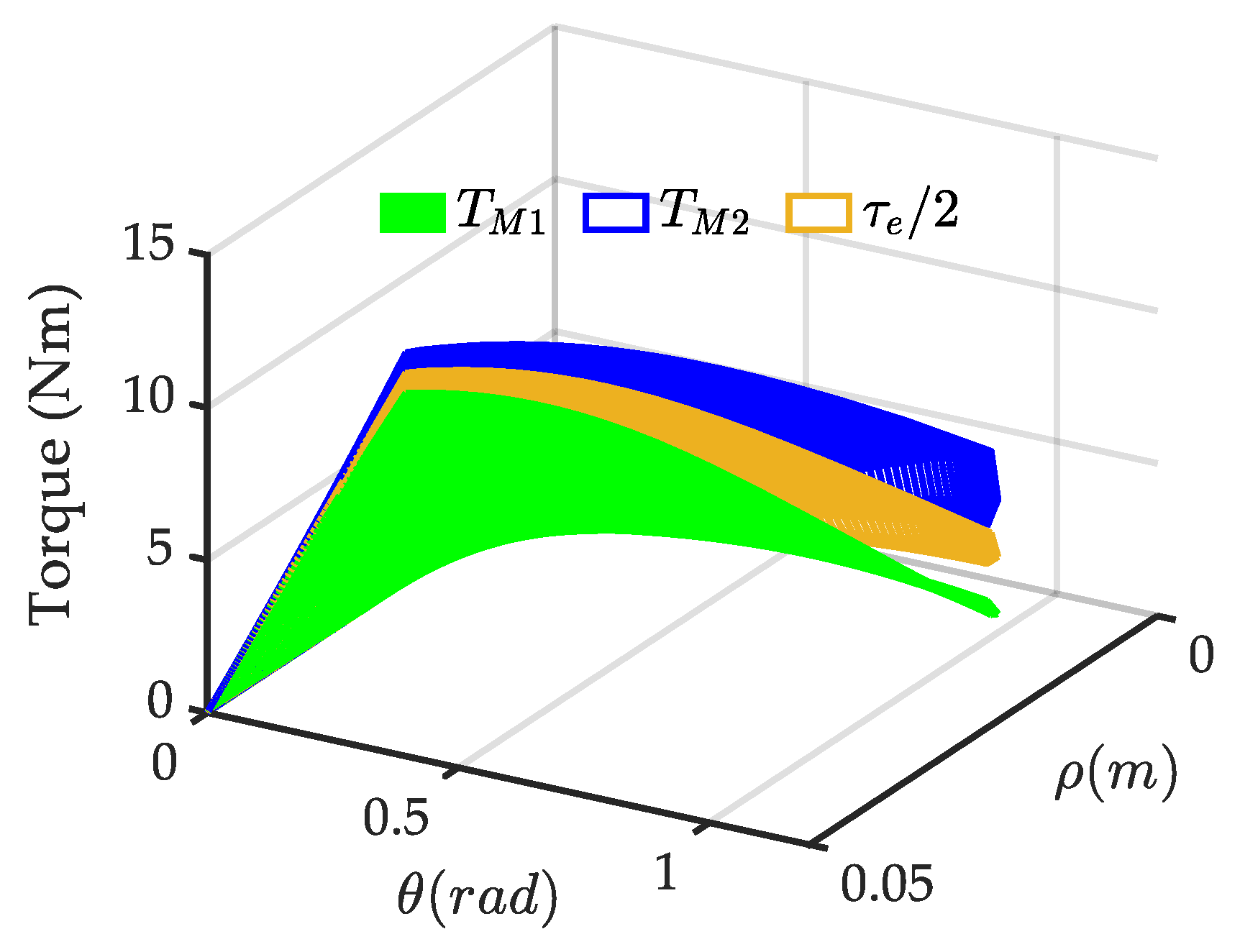
- Secondly, deform the variable stiffness module with an angle θ by synchronized motion. The maximum θ corresponding to each ρ is shown in Figure 4. It is mainly constrained by the allowable spring angle. Now the motion angle exerted on the cams C1 and C2 become
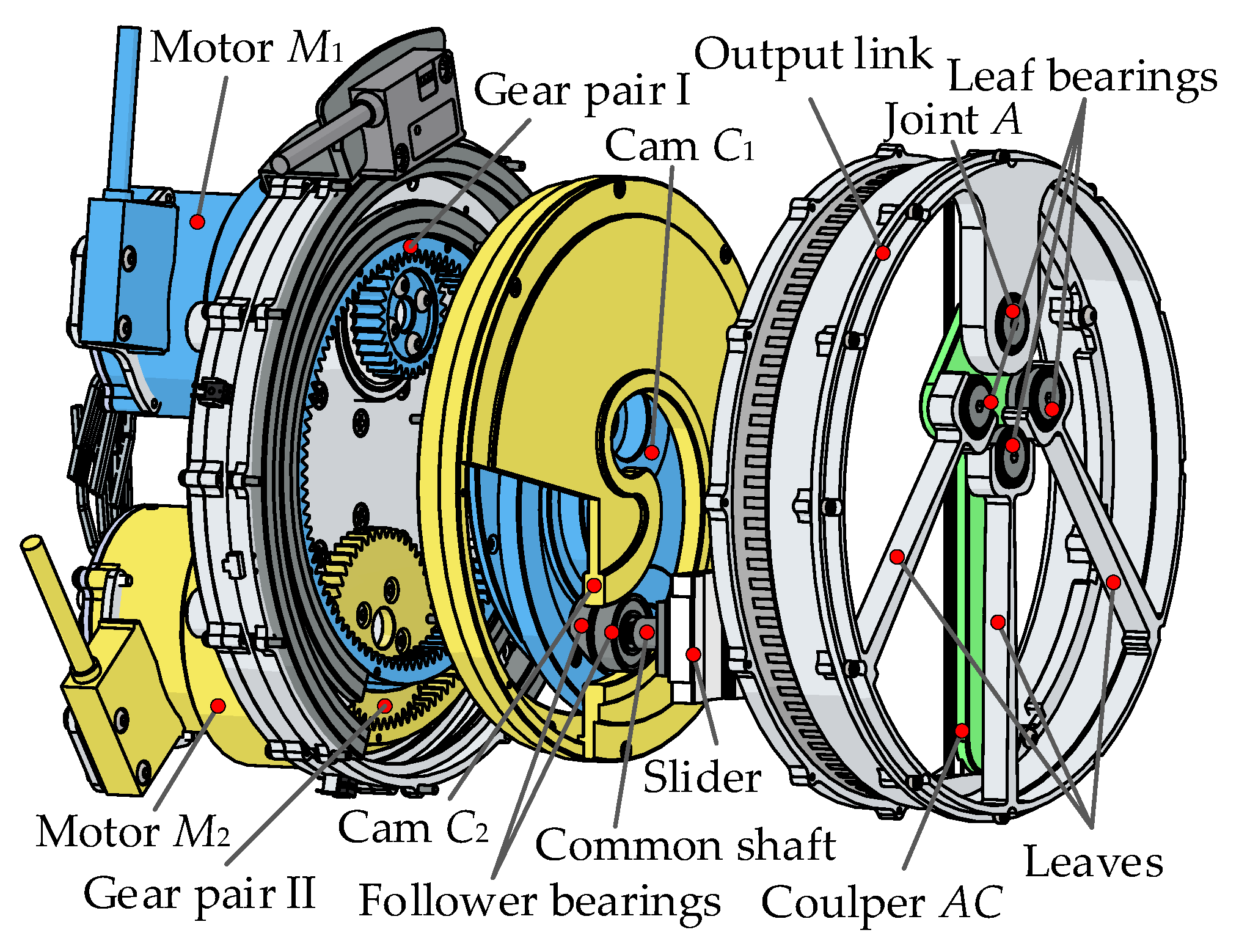
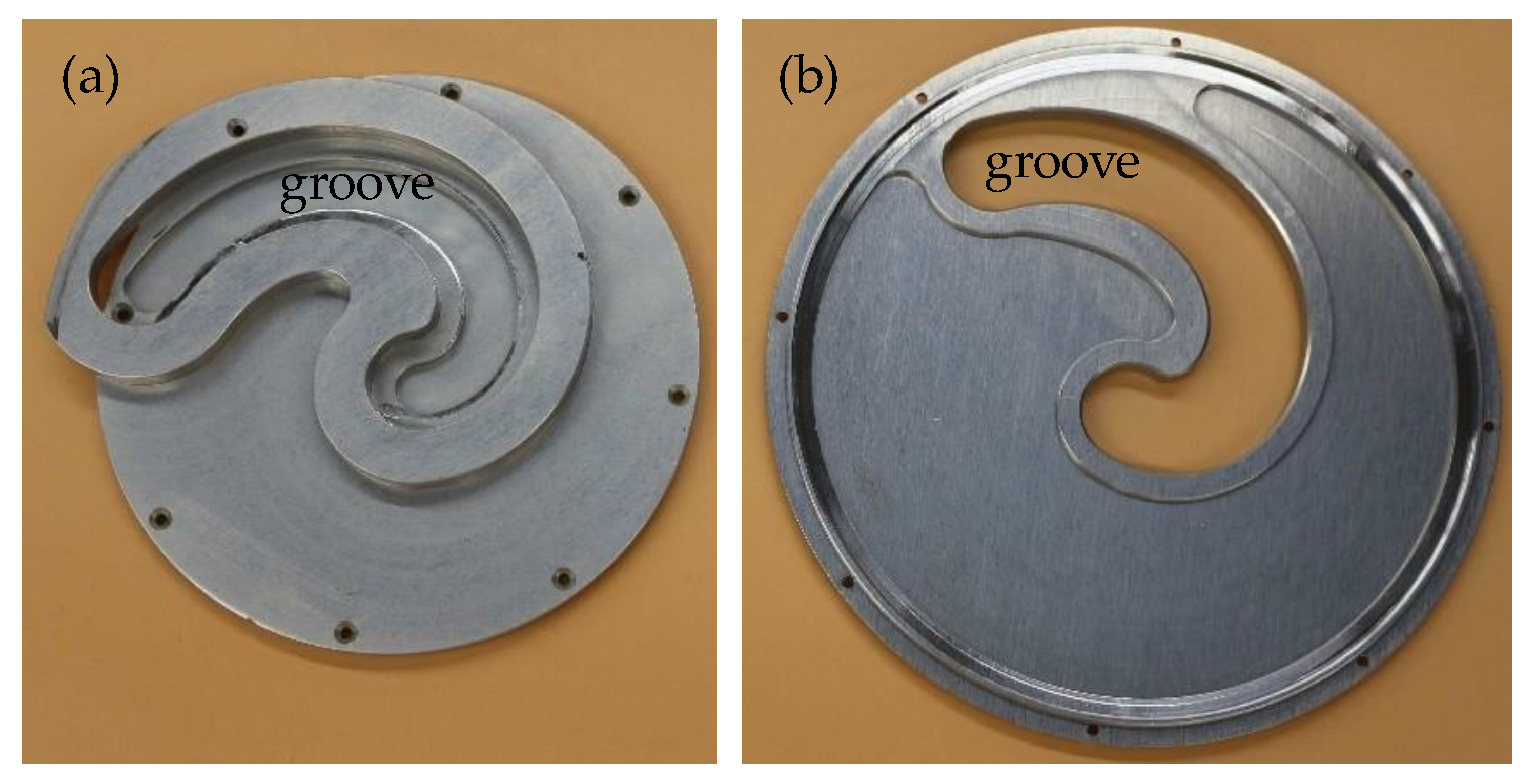
4.2. Performance Validation by Physical Prototype
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hong, C.; Tang, D.; Quan, Q.; Cao, Z.; Deng, Z. A combined series-elastic actuator & parallel-elastic leg no-latch bio-inspired jumping robot. Mech. Mach. Theory 2020, 149, 103814. [Google Scholar]
- Liu, L.; Misgeld, B.J.E.; Pomprapa, A.; Leonhardt, S. A Testable Robust Stability Framework for the Variable Impedance Control of 1-DOF Exoskeleton with Variable Stiffness Actuator. IEEE Trans. Control Syst. Technol. 2021, 29, 2728–2737. [Google Scholar] [CrossRef]
- Shao, Y.; Zhang, W.; Su, Y.; Ding, X. Design and optimisation of load-adaptive actuator with variable stiffness for compact ankle exoskeleton. Mech. Mach. Theory 2021, 161, 104323. [Google Scholar] [CrossRef]
- Moltedo, M.; Cavallo, G.; Baček, T.; Lataire, J.; Vanderborght, B.; Lefeber, D.; Rodriguez-Guerrero, C. Variable stiffness ankle actuator for use in robotic-assisted walking: Control strategy and experimental characterization. Mech. Mach. Theory 2019, 134, 604–624. [Google Scholar] [CrossRef]
- Gomez-Vargas, D.; Casas-Bocanegra, D.; Múnera, M.; Roberti, F.; Carelli, R.; Cifuentes, C.A. Variable Stiffness Actuators for Wearable Applications in Gait Rehabilitation. In Interfacing Humans and Robots for Gait Assistance and Rehabilitation; Springer: Cham, Switzerland, 2022; pp. 193–212. [Google Scholar]
- Li, Z.; Li, W.; Chen, W.H.; Zhang, J.; Wang, J.; Fang, Z.; Yang, G. Mechatronics design and testing of a cable-driven upper limb rehabilitation exoskeleton with variable stiffness. Rev. Sci. Instrum. 2021, 92, 024101. [Google Scholar] [CrossRef] [PubMed]
- Robinson, D.W.; Pratt, J.E.; Paluska, D.J.; Pratt, G.A. Series elastic actuator development for a biomimetic walking robot. In Proceedings of the 1999 IEEE/ASME International Conference on Advanced Intelligent Mechatronics (Cat. No. 99TH8399), Atlanta, GA, USA, 19–23 September 1999; pp. 561–568. [Google Scholar]
- de Gaitani, F.H.M.; dos Santos, W.M.; Siqueira, A.A.G. Design and Performance Analysis of a Compact Series Elastic Actuator for Exoskeletons. J. Control Autom. Electr. Syst. 2022, 33, 1012–1021. [Google Scholar] [CrossRef]
- Yang, Z.; Li, X.; Xu, J.; Chen, R.; Yang, H. A new low-energy nonlinear variable stiffness actuator for the knee joint. Mech. Based Des. Struct. Mach. 2022, 1–15. [Google Scholar] [CrossRef]
- Christie, M.D.; Sun, S.; Ning, D.H.; Du, H.; Zhang, S.W.; Li, W.H. A highly stiffness-adjustable robot leg for enhancing locomotive performance. Mech. Syst. Signal Process. 2019, 126, 458–468. [Google Scholar] [CrossRef]
- Beckerle, P.; Stuhlenmiller, F.; Rinderknecht, S. Stiffness Control of Variable Serial Elastic Actuators: Energy Efficiency through Exploitation of Natural Dynamics. Actuators 2017, 6, 28. [Google Scholar] [CrossRef] [Green Version]
- Liu, H.; Zhu, D.; Xiao, J. Conceptual design and parameter optimization of a variable stiffness mechanism for producing constant output forces. Mech. Mach. Theory 2020, 154, 104033. [Google Scholar] [CrossRef]
- Albu-Schaffer, A.; Eiberger, O.; Grebenstein, M.; Haddadin, S.; Ott, C.; Wimbock, T.; Wolf, S.; Hirzinger, G. Soft robotics. IEEE Robot. Autom. Mag. 2008, 15, 20–30. [Google Scholar] [CrossRef]
- Li, Z.; Bai, S.; Madsen, O.; Chen, W.; Zhang, J. Design, modeling, and testing of a compact variable stiffness mechanism for exoskeletons. Mech. Mach. Theory 2020, 151, 103905. [Google Scholar] [CrossRef]
- Wolf, S.; Grioli, G.; Eiberger, O.; Friedl, W.; Grebenstein, M.; Höppner, H.; Burdet, E.; Caldwell, D.G.; Carloni, P.; Catalano, M.G.; et al. Variable stiffness actuators: Review on design and components. IEEE/ASME Trans. Mechatron. 2015, 21, 2418–2430. [Google Scholar] [CrossRef]
- Lee, J.H.; Wahrmund, C.; Jafari, A. A Novel Mechanically Overdamped Actuator with Adjustable Stiffness (MOD-AwAS) for Safe Interaction and Accurate Positioning. Actuators 2017, 6, 22. [Google Scholar] [CrossRef]
- Vanderborght, B.; Albu-Schäffer, A.; Bicchi, A.; Burdet, E.; Caldwell, D.G.; Carloni, R.; Catalano, M.; Eiberger, O.; Friedl, W.; Ganesh, G.; et al. Variable impedance actuators: A review. Robot. Auton. Syst. 2013, 61, 1601–1614. [Google Scholar] [CrossRef] [Green Version]
- Li, Z.; Bai, S.; Chen, W.; Zhang, J. Nonlinear Stiffness Analysis of Spring-Loaded Inverted Slider Crank Mechanisms With a Unified Model. J. Mech. Robot. 2019, 12, 031011. [Google Scholar] [CrossRef]
- Moore, R.; Schimmels, J.M. Design of a Quadratic, Antagonistic, Cable-Driven, Variable Stiffness Actuator. J. Mech. Robot. 2021, 13, 031001. [Google Scholar] [CrossRef]
- Li, Z.; Chen, W.; Zhang, J.; Li, Q.; Wang, J.; Fang, Z.; Yang, G. A novel cable-driven antagonistic joint designed with variable stiffness mechanisms. Mech. Mach. Theory 2022, 171, 104716. [Google Scholar] [CrossRef]
- Zhang, M.; Ma, P.; Sun, F.; Sun, X.; Xu, F.; Jin, J.; Fang, L. Dynamic Modeling and Control of Antagonistic Variable Stiffness Joint Actuator. Actuators 2021, 10, 116. [Google Scholar] [CrossRef]
- Govindan, N.; Ramesh, S.; Thondiyath, A. Design of a Variable Stiffness Joint Module to Quickly Change the Stiffness and to Reduce the Power Consumption. IEEE Access 2020, 8, 138318–138330. [Google Scholar] [CrossRef]
- Sun, J.; Guo, Z.; Sun, D.; He, S.; Xiao, X. Design, modeling and control of a novel compact, energy-efficient, and rotational serial variable stiffness actuator (SVSA-II). Mech. Mach. Theory 2018, 130, 123–136. [Google Scholar] [CrossRef]
- Shao, Y.; Zhang, W.; Ding, X. Configuration synthesis of variable stiffness mechanisms based on guide-bar mechanisms with length-adjustable links. Mech. Mach. Theory 2021, 156, 104153. [Google Scholar] [CrossRef]
- Bi, S.S.; Liu, C.; Zhao, H.Z.; Wang, Y.L. Design and analysis of a novel variable stiffness actuator based on parallel-assembled-folded serial leaf springs. Adv. Robot. 2017, 31, 990–1001. [Google Scholar] [CrossRef]
- Li, X.; Zhu, H.; Lin, W.; Chen, W.; Low, K.H. Structure-Controlled Variable Stiffness Robotic Joint Based on Multiple Rotary Flexure Hinges. IEEE Trans. Ind. Electron. 2020, 68, 12452–12461. [Google Scholar] [CrossRef]
- Choi, J.; Hong, S.; Lee, W.; Kang, S.; Kim, M. A Robot Joint with Variable Stiffness Using Leaf Springs. IEEE Trans. Robot. 2011, 27, 229–238. [Google Scholar] [CrossRef]
- Zhu, Y.; Wu, Q.; Chen, B.; Xu, D.; Shao, Z. Design and Evaluation of a Novel Torque-Controllable Variable Stiffness Actuator with Reconfigurability. IEEE/ASME Trans. Mechatron. 2021, 27, 292–303. [Google Scholar] [CrossRef]
- Li, Z.; Bai, S. A novel revolute joint of variable stiffness with reconfigurability. Mech. Mach. Theory 2019, 133, 720–736. [Google Scholar] [CrossRef]
- Mengacci, R.; Garabini, M.; Grioli, G.; Catalano, M.G.; Bicchi, A. Overcoming the torque/stiffness range tradeoff in antagonistic variable stiffness actuators. IEEE/ASME Trans. Mechatron. 2021, 26, 3186–3197. [Google Scholar] [CrossRef]
- Sun, L.; Zhao, W.; Wang, B.; Huang, S.; Li, J. Mechanical design and analysis of a reconfigurable variable stiffness mechanism based on permanent magnetic springs and swing guide mechanism. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2022, 09544062221130205. [Google Scholar] [CrossRef]
- Xu, Y.; Guo, K.; Sun, J.; Li, J. Design, modeling and control of a reconfigurable variable stiffness actuator. Mech. Syst. Signal Process. 2021, 160, 107883. [Google Scholar] [CrossRef]
- Qian, Y.; Han, S.; Aguirre-Ollinger, G.; Fu, C.; Yu, H. Design, Modelling, and Control of a Reconfigurable Rotary Series Elastic Actuator with Nonlinear Stiffness for Assistive Robots. arXiv 2022, arXiv:2205.14412. [Google Scholar]
- Mei, F.; Bi, S.; Liu, C.; Chang, Q. Optimal Design of Cam Curve Dedicated to Improving Load Uniformity of Bidirectional Antagonistic VSA. In Proceedings of the International Conference on Intelligent Robotics and Applications, Yantai, China, 22–25 October 2021; Springer: Cham, Switzerland; pp. 3–13. [Google Scholar]
- Mei, F.; Bi, S.; Chen, L.; Gao, H. A novel design of planar high-compliance joint in variable stiffness module with multiple uniform stress leaf branches on rigid-flexible integral linkage. Mech. Mach. Theory 2022, 174, 104889. [Google Scholar] [CrossRef]



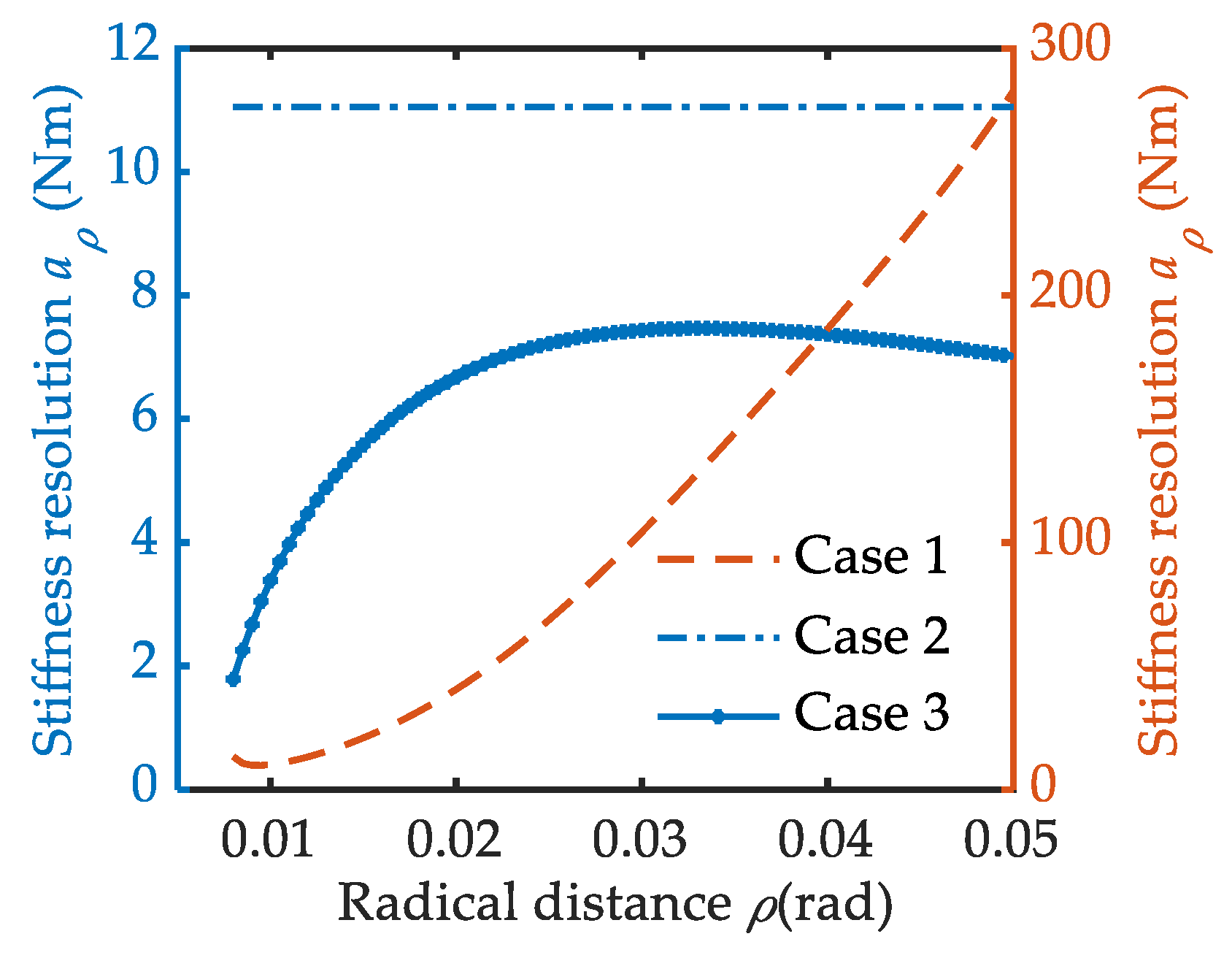

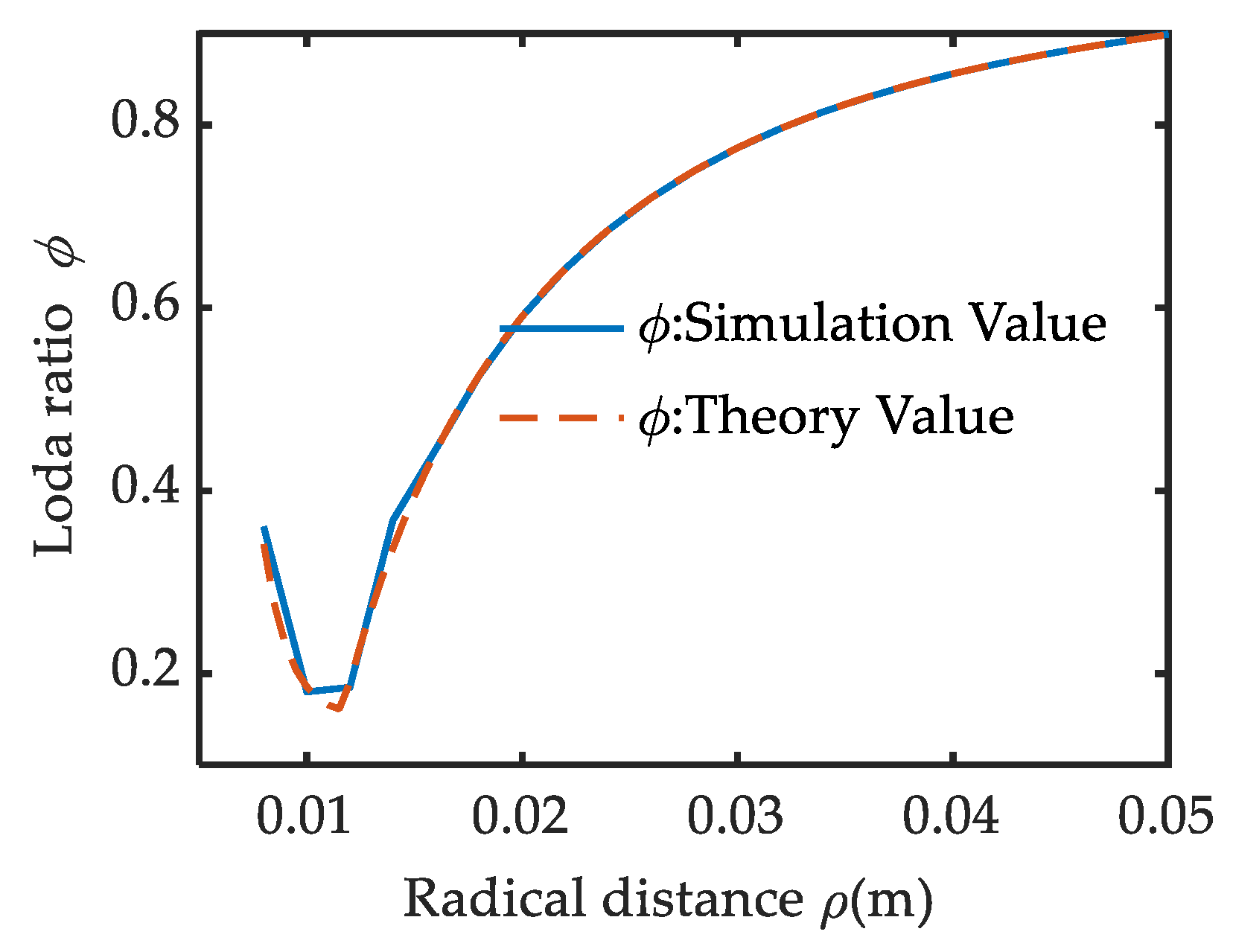

| Case 1 | Case 2 | Case 3 | |
|---|---|---|---|
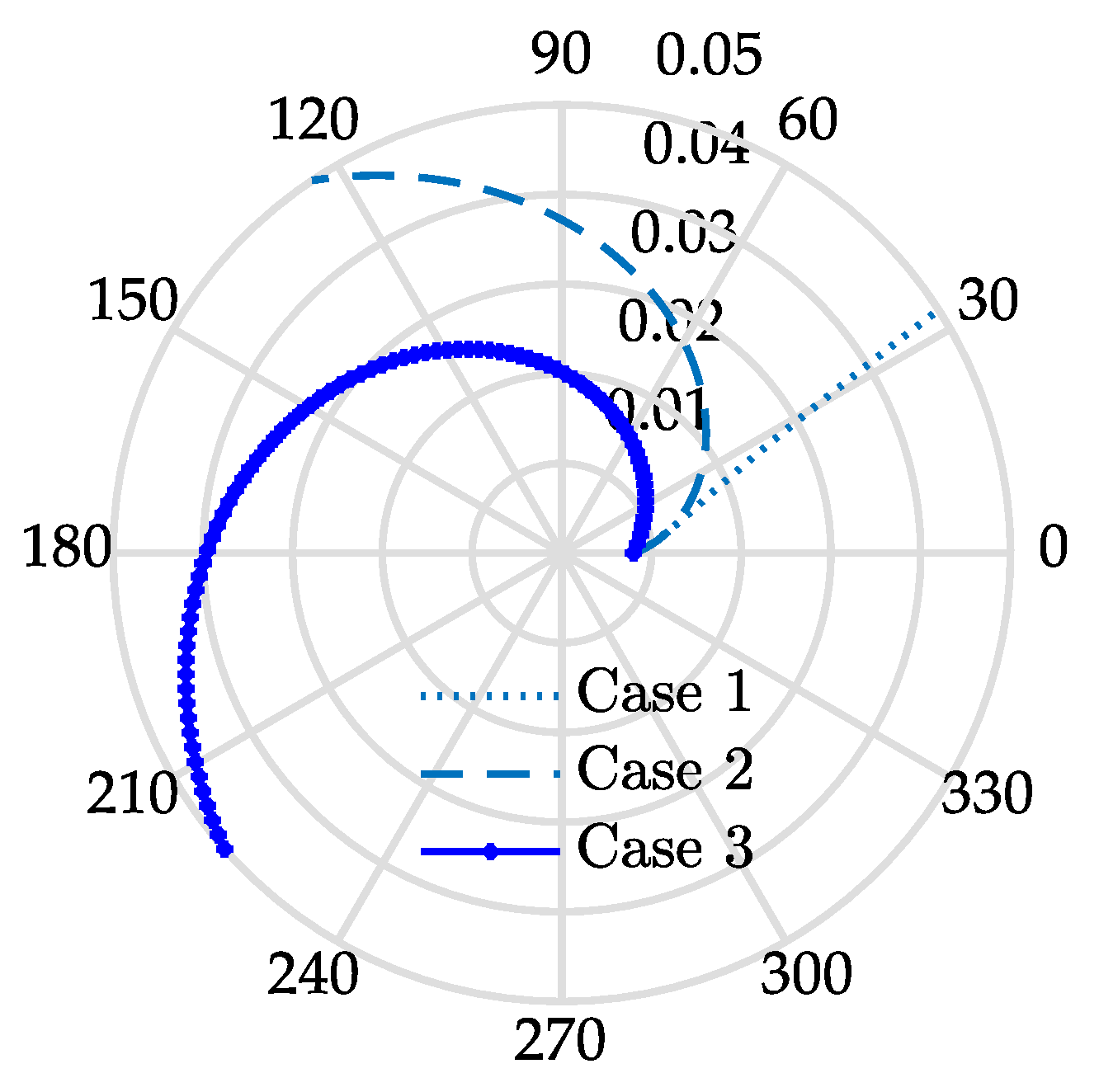
| Stroke of polar angle κ | κ = 0.57 rad | κ = 2.16 rad | κ = 3.86 rad |
| Stiffness resolution aρ | 10 Nm < aρ <282 Nm | aρ = 11 Nm | 1.78 Nm < aρ < 7.5 Nm |
| Load ratio ϕ | ϕ < −0.2 in the whole ρ range | ϕ < −0.2 with ρ < 0.014 m; ϕ > 0.6 with ρ > 0.026 m | ϕ > 0.6 with 0.02 < ρ < 0.032 m; ϕ > 0.8 with ρ > 0.032 m. |
| Pressure angle γ(ρ) | Maximum γ(ρ) close to the upper solution boundary | γ(ρ) close to case 1 in low ρ range; γ(ρ) close to case 2 in high ρ range. | Minimum γ(ρ) close to the lower solution boundary |
| Performance (Unit) | Value |
|---|---|
| Power (W) | 60 |
| Radial dimension (mm) | 82 |
| Axial dimension (mm) | 127 |
| Weight (kg) | 2.5 |
| Range of output angle (rad) | −2π~2π |
| Stiffness adjustment range (Nm/rad) | 2.51~26.23 |
| Maximum output torque (Nm) | 9.5 |
| Deformability (rad/s) | 0.38~1.785 |
| Maximum stiffness adjustment time (s) | 1.57 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mei, F.; Bi, S.; Li, B. A Novel Cam-Based Variable Stiffness Actuator: Pitch Curve Synthetic Approach for Reconfiguration Design. Mathematics 2022, 10, 4088. https://doi.org/10.3390/math10214088
Mei F, Bi S, Li B. A Novel Cam-Based Variable Stiffness Actuator: Pitch Curve Synthetic Approach for Reconfiguration Design. Mathematics. 2022; 10(21):4088. https://doi.org/10.3390/math10214088
Chicago/Turabian StyleMei, Fanghua, Shusheng Bi, and Bianhong Li. 2022. "A Novel Cam-Based Variable Stiffness Actuator: Pitch Curve Synthetic Approach for Reconfiguration Design" Mathematics 10, no. 21: 4088. https://doi.org/10.3390/math10214088
APA StyleMei, F., Bi, S., & Li, B. (2022). A Novel Cam-Based Variable Stiffness Actuator: Pitch Curve Synthetic Approach for Reconfiguration Design. Mathematics, 10(21), 4088. https://doi.org/10.3390/math10214088





