A Meshfree Approach for Solving Fractional Galilei Invariant Advection–Diffusion Equation through Weighted–Shifted Grünwald Operator
Abstract
1. Introduction
2. The Time Discretization Approximation
3. Description of the BSM Method
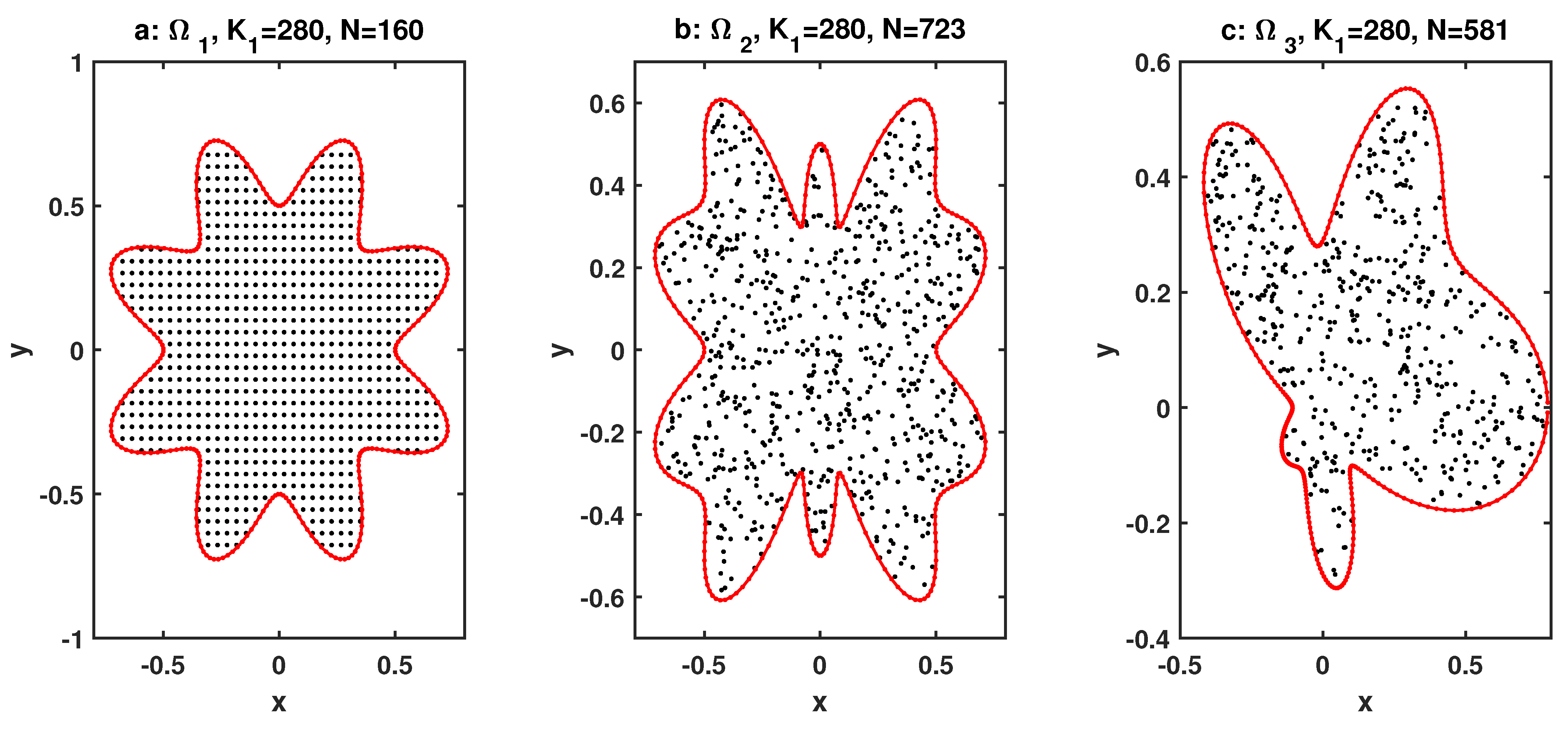
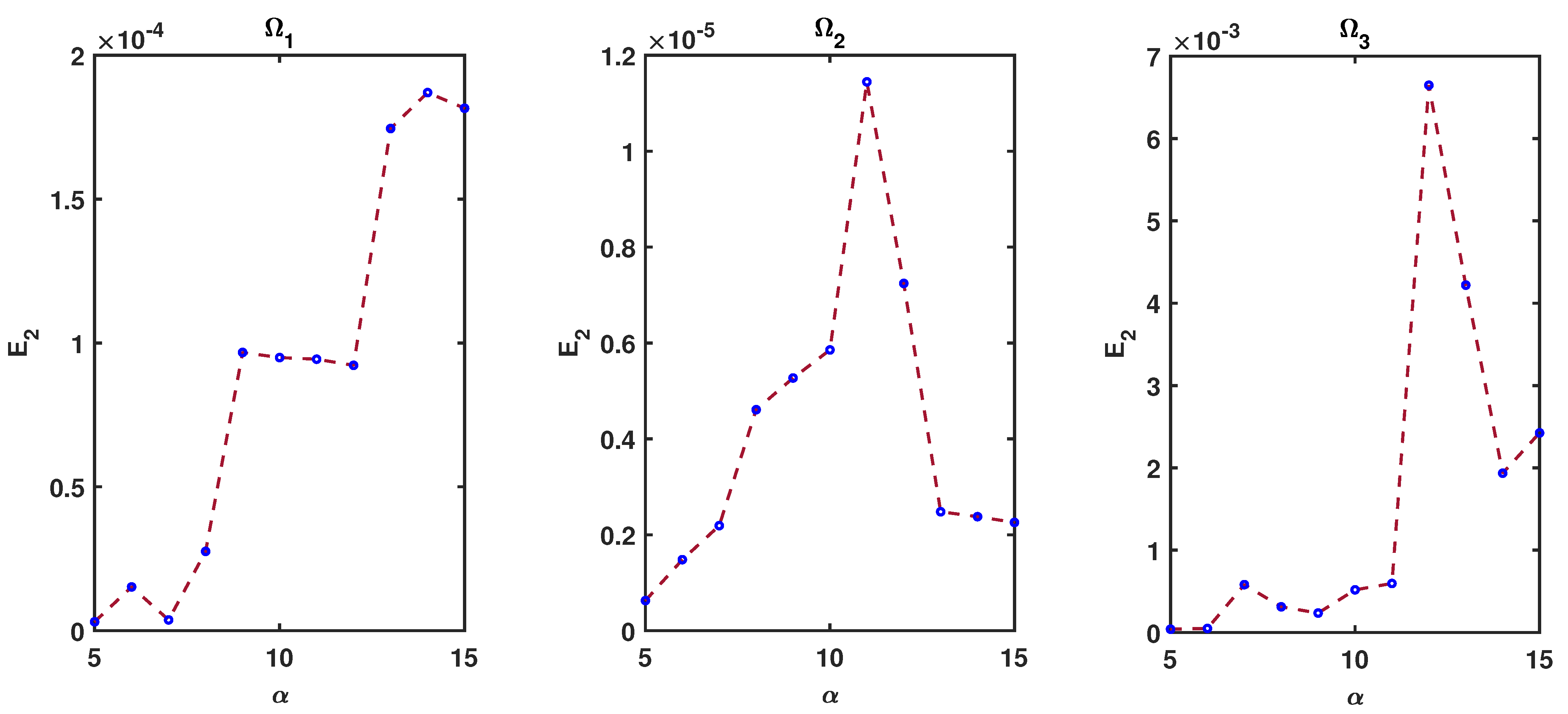
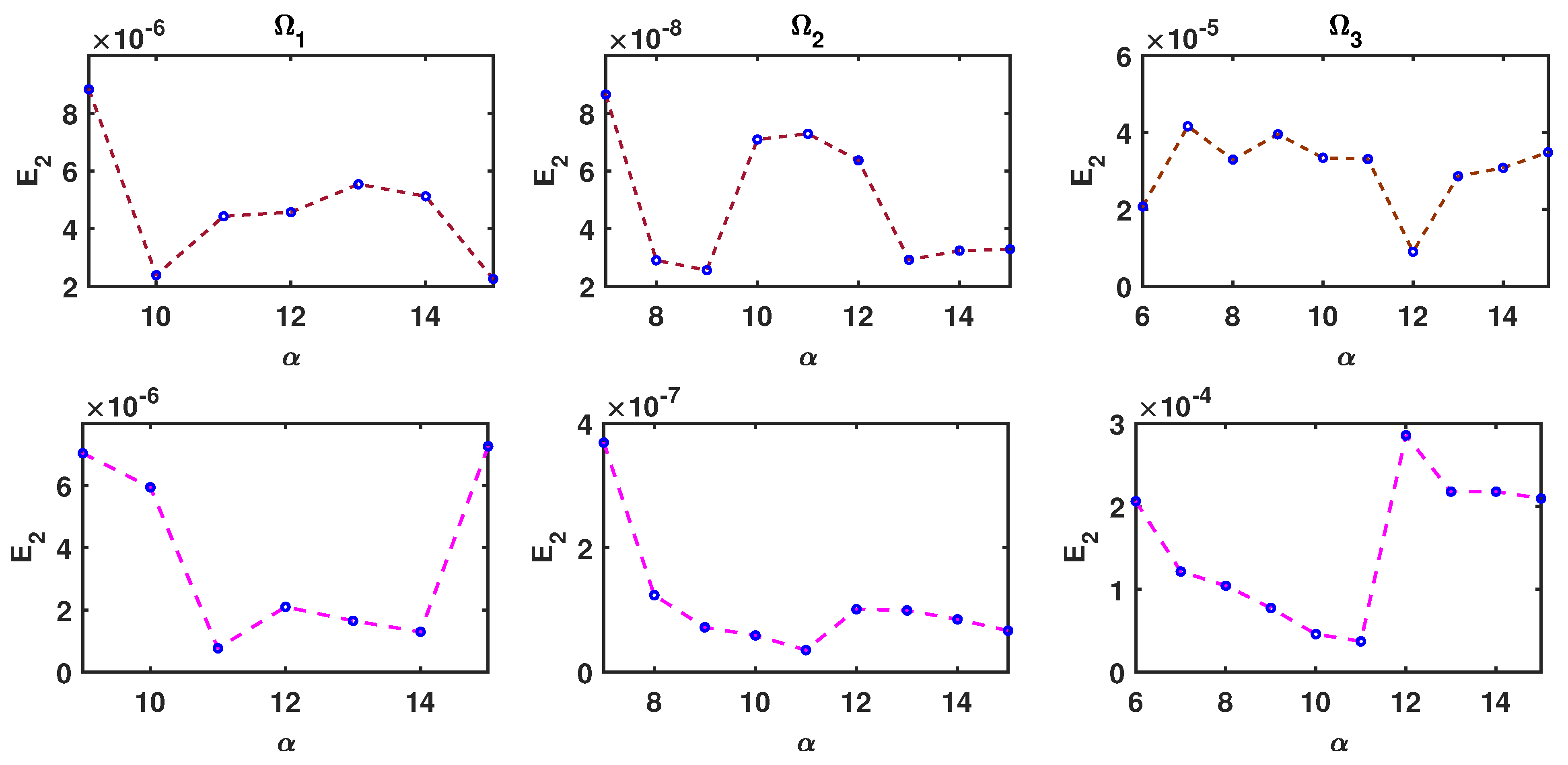
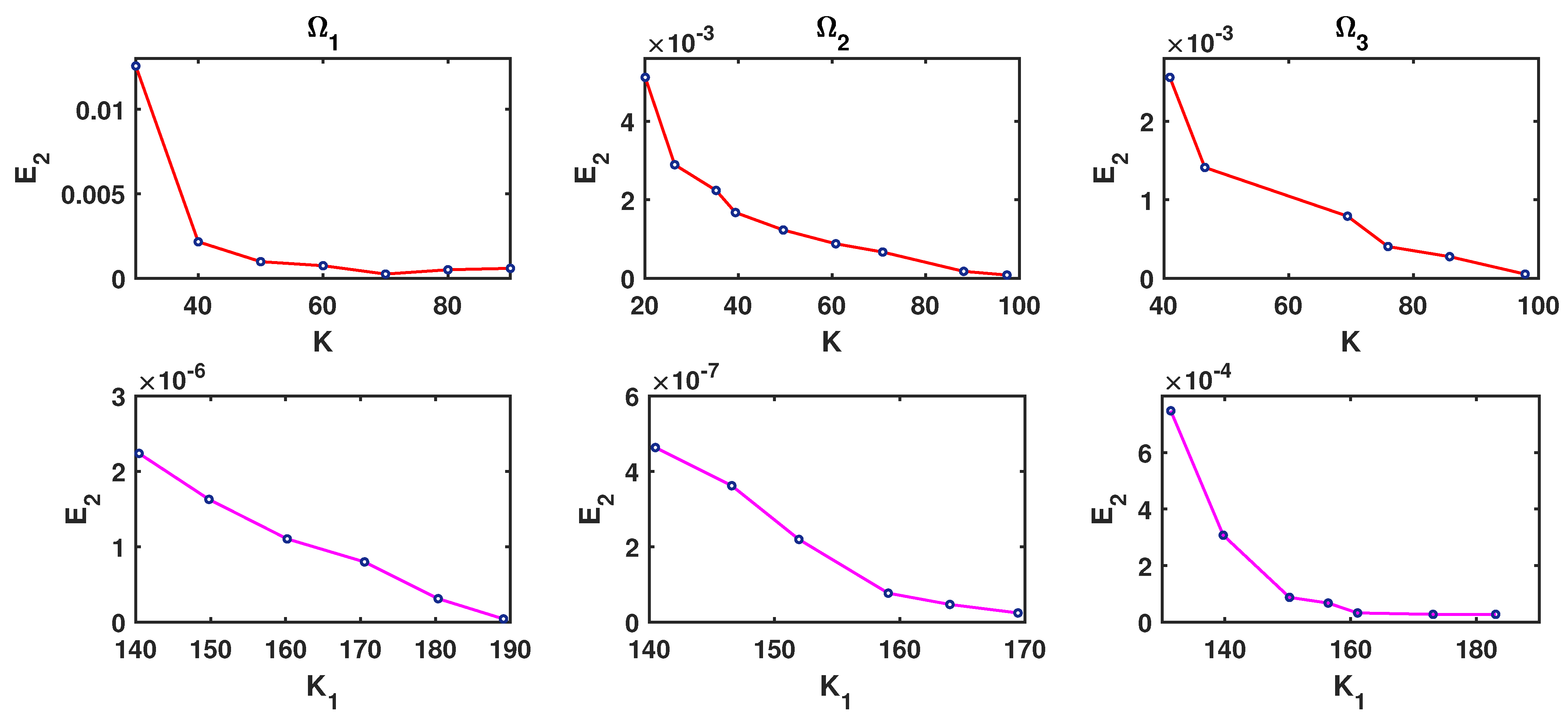
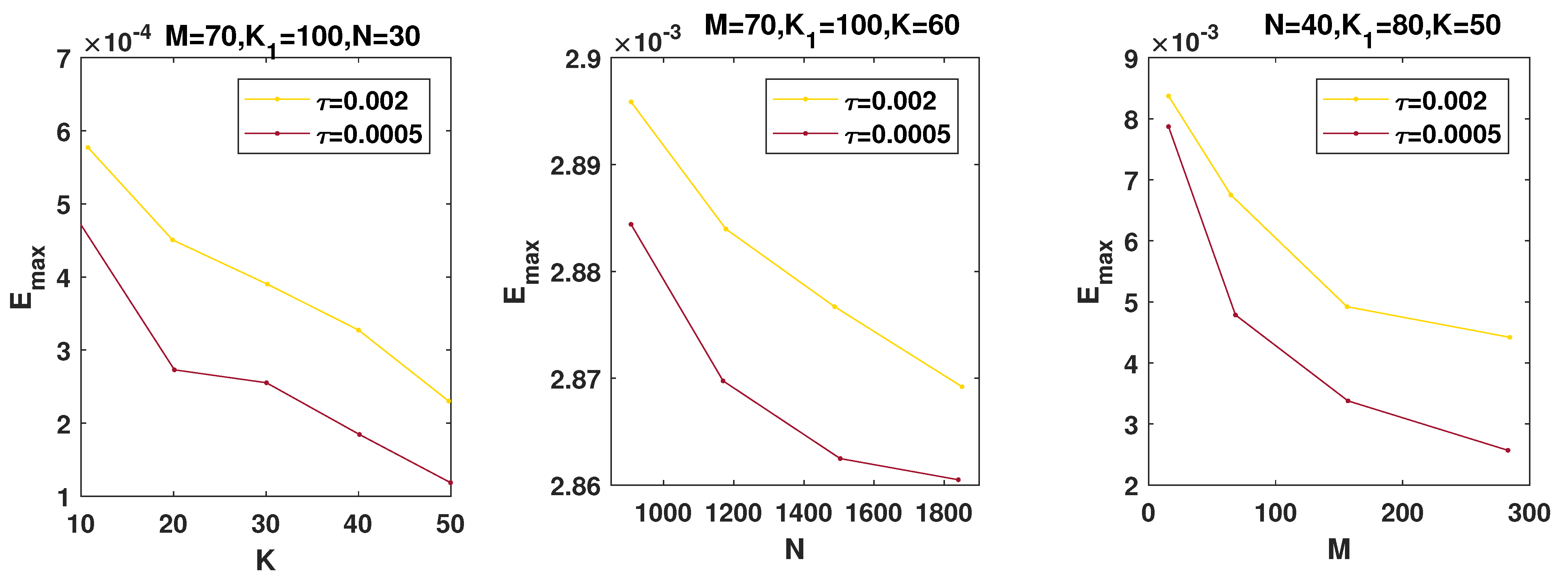
4. Numerical Results in Two Space Dimensions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Safari, F.; Chen, W. Coupling of the improved singular boundary method and dual reciprocity method for multi-term time-fractional mixed diffusion-wave equations. Comput. Math. Appl. 2019, 78, 1594–1607. [Google Scholar] [CrossRef]
- Shojaei, A.; Hermann, A.; Cyron, C.J.; Seleson, P.; Silling, S.A. A hybrid meshfree discretization to improve the numerical performance of peridynamic models. Comput. Methods Appl. Mech. Eng. 2022, 391, 114544. [Google Scholar] [CrossRef]
- Seleson, P.; Gunzburger, M.; Parks, M.L. Interface problems in nonlocal diffusion and sharp transitions between local and nonlocal domains. Comput. Methods Appl. Mech. Eng. 2013, 266, 185–204. [Google Scholar] [CrossRef]
- Shojaei, A.; Hermann, A.; Seleson, P.; Cyron, C.J. Dirichlet absorbing boundary conditions for classical and peridynamic diffusion-type models. Comput. Mech. 2020, 66, 773–793. [Google Scholar] [CrossRef]
- Zhao, J.; Chen, Z.; Mehrmashhadi, J.; Bobaru, F. Construction of a peridynamic model for transient advection-diffusion problems. Int. J. Heat Mass Transf. 2018, 126, 1253–1266. [Google Scholar] [CrossRef]
- Aleroev, T.; Orlov, V. On One Approximate Method of a Boundary Value Problem for a One-Dimensional Advection–Diffusion Equation. Axioms 2022, 11, 541. [Google Scholar] [CrossRef]
- Mahmoud, E.I.; Aleroev, T.S. Boundary Value Problem of Space-Time Fractional Advection Diffusion Equation. Mathematics 2022, 10, 3160. [Google Scholar] [CrossRef]
- Ndou, N.; Dlamini, P.; Jacobs, B.A. Enhanced Unconditionally Positive Finite Difference Method for Advection–Diffusion–Reaction Equations. Mathematics 2022, 10, 2639. [Google Scholar] [CrossRef]
- Abbaszadeh, M.; Dehghan, M. The Crank-Nicolson/interpolating stabilized element-free Galerkin method to investigate the fractional Galilei invariant advection-diffusion equation. Math. Methods Appl. Sci. 2021, 44, 2752–2768. [Google Scholar] [CrossRef]
- Kumar, S.; Pandey, P.; Das, S. Gegenbauer wavelet operational matrix method for solving variable-order non-linear reaction–diffusion and Galilei invariant advection–diffusion equations. Comput. Appl. Math. 2019, 38, 162. [Google Scholar] [CrossRef]
- Chen, C.; Liu, F.; Anh, V.; Turner, I. Numerical simulation for the variable-order Galilei invariant advection diffusion equation with a nonlinear source term. Appl. Math. Comput. 2011, 217, 5729–5742. [Google Scholar] [CrossRef]
- Zaky, M.A. Existence, uniqueness and numerical analysis of solutions of tempered fractional boundary value problems. Appl. Numer. Math. 2019, 145, 429–457. [Google Scholar] [CrossRef]
- Zaky, M.A. Recovery of high order accuracy in Jacobi spectral collocation methods for fractional terminal value problems with non-smooth solutions. J. Comput. Appl. Math. 2019, 357, 103–122. [Google Scholar] [CrossRef]
- Le Vot, F.; Abad, E.; Metzler, R.; Yuste, S.B. Continuous time random walk in a velocity field: Role of domain growth, Galilei-invariant advection-diffusion, and kinetics of particle mixing. New J. Phys. 2020, 22, 73048. [Google Scholar] [CrossRef]
- Zaky, M.A.; Baleanu, D.; Alzaidy, J.F.; Hashemizadeh, E. Operational matrix approach for solving the variable-order nonlinear Galilei invariant advection–diffusion equation. Adv. Differ. Equ. 2018, 2018, 102. [Google Scholar] [CrossRef]
- Meerschaert, M.M.; Tadjeran, C. Finite difference approximations for fractional advection–dispersion flow equations. J. Comput. Appl. Math. 2004, 172, 65–77. [Google Scholar] [CrossRef]
- Baeumer, B.; Kovács, M.; Sankaranarayanan, H. Higher order Grünwald approximations of fractional derivatives and fractional powers of operators. Trans. Am. Math. Soc. 2015, 367, 813–834. [Google Scholar] [CrossRef]
- Tian, W.; Zhou, H.; Deng, W. A class of second order difference approximations for solving space fractional diffusion equations. Math. Comput. 2015, 84, 1703–1727. [Google Scholar] [CrossRef]
- Zhou, H.; Tian, W.; Deng, W. Quasi-compact finite difference schemes for space fractional diffusion equations. J. Sci. Comput. 2013, 56, 45–66. [Google Scholar] [CrossRef]
- Wang, P.; Huang, C. An implicit midpoint difference scheme for the fractional Ginzburg–Landau equation. J. Comput. Phys. 2016, 312, 31–49. [Google Scholar] [CrossRef]
- Tadjeran, C.; Meerschaert, M.M. A second-order accurate numerical method for the two-dimensional fractional diffusion equation. J. Comput. Phys. 2007, 220, 813–823. [Google Scholar] [CrossRef]
- Meerschaert, M.M.; Tadjeran, C. Finite difference approximations for two-sided space-fractional partial differential equations. Appl. Numer. Math. 2006, 56, 80–90. [Google Scholar] [CrossRef]
- Kazem, S.; Dehghan, M. Semi-analytical solution for time-fractional diffusion equation based on finite difference method of lines (MOL). Eng. Comput. 2019, 35, 229–241. [Google Scholar] [CrossRef]
- Dehghan, M.; Abbaszadeh, M. The solution of nonlinear Green–Naghdi equation arising in water sciences via a meshless method which combines moving kriging interpolation shape functions with the weighted essentially non–oscillatory method. Commun. Nonlinear Sci. Numer. Simul. 2019, 68, 220–239. [Google Scholar] [CrossRef]
- Karamali, G.; Dehghan, M.; Abbaszadeh, M. Numerical solution of a time-fractional PDE in the electroanalytical chemistry by a local meshless method. Eng. Comput. 2019, 35, 87–100. [Google Scholar] [CrossRef]
- Jafari, H.; Sadeghi, J.; Safari, F.; Kubeka, A. Factorization method for fractional Schrödinger equation in D-dimensional fractional space and homogeneous manifold SL(2,C)/GL(1,C). Comput. Methods Differ. Equ. 2019, 7, 199–205. [Google Scholar]
- Safari, F. The solution of the Schrödinger equation for Makarov potential and homogeneous manifold SL(2,C)/GL(1,C). Pramana 2020, 94, 59. [Google Scholar] [CrossRef]
- Lin, J.; Xu, Y.; Zhang, Y. Simulation of linear and nonlinear advection–diffusion–reaction problems by a novel localized scheme. Appl. Math. Lett. 2020, 99, 106005. [Google Scholar] [CrossRef]
- Lin, J.; Feng, W.; Reutskiy, S.; Xu, H.; He, Y. A new semi-analytical method for solving a class of time fractional partial differential equations with variable coefficients. Appl. Math. Lett. 2021, 112, 106712. [Google Scholar] [CrossRef]
- Reutskiy, S.Y. A new semi-analytical collocation method for solving multi-term fractional partial differential equations with time variable coefficients. Appl. Math. Model. 2017, 45, 238–254. [Google Scholar]
- Reutskiy, S.Y.; Lin, J. A semi-analytic collocation method for space fractional parabolic PDE. Int. J. Comput. Math. 2018, 95, 1326–1339. [Google Scholar] [CrossRef]
- Safari, F.; Azarsa, P. Backward substitution method based on Müntz polynomials for solving the nonlinear space fractional partial differential equations. Math. Methods Appl. Sci. 2020, 43, 847–864. [Google Scholar] [CrossRef]
- Lin, J.; Reutskiy, S. A cubic B-spline semi-analytical algorithm for simulation of 3D steady-state convection-diffusion-reaction problems. Appl. Math. Comput. 2020, 371, 124944. [Google Scholar] [CrossRef]
- Hong, Y.; Lin, J.; Chen, W. A typical backward substitution method for the simulation of Helmholtz problems in arbitrary 2D domains. Eng. Anal. Bound. Elem. 2018, 93, 167–176. [Google Scholar] [CrossRef]
- Safari, F.; Sun, H. Improved singular boundary method and dual reciprocity method for fractional derivative Rayleigh–Stokes problem. Eng. Comput. 2020, 37, 3151–3166. [Google Scholar] [CrossRef]
- Reutskiy, S.; Lin, J. A semi-analytic collocation technique for steady-state strongly nonlinear advection-diffusion-reaction equations with variable coefficients. Int. J. Numer. Methods Eng. 2017, 112, 2004–2024. [Google Scholar] [CrossRef]
- Safari, F.; Chen, W. Numerical approximations for space–time fractional Burgers’ equations via a new semi-analytical method. Comput. Math. Appl. 2021, 96, 55–66. [Google Scholar] [CrossRef]
- Zhuang, P.; Liu, F.; Anh, V.; Turner, I. Numerical methods for the variable-order fractional advection-diffusion equation with a nonlinear source term. SIAM J. Numer. Anal. 2009, 47, 1760–1781. [Google Scholar] [CrossRef]
- Wang, Z.; Vong, S. Compact difference schemes for the modified anomalous fractional sub-diffusion equation and the fractional diffusion-wave equation. J. Comput. Phys. 2014, 277, 1–15. [Google Scholar] [CrossRef]
- Lin, J.; Reutskiy, S.Y.; Lu, J. A novel meshless method for fully nonlinear advection–diffusion-reaction problems to model transfer in anisotropic media. Appl. Math. Comput. 2018, 339, 459–476. [Google Scholar] [CrossRef]
- Abbaszadeh, M.; Dehghan, M. Numerical and analytical investigations for neutral delay fractional damped diffusion–wave equation based on the stabilized interpolating element free Galerkin (IEFG) method. Appl. Numer. Math. 2019, 145, 488–506. [Google Scholar] [CrossRef]
- Zhang, T.; Li, X. Variational multiscale interpolating element-free Galerkin method for the nonlinear Darcy–Forchheimer model. Comput. Math. Appl. 2020, 79, 363–377. [Google Scholar] [CrossRef]






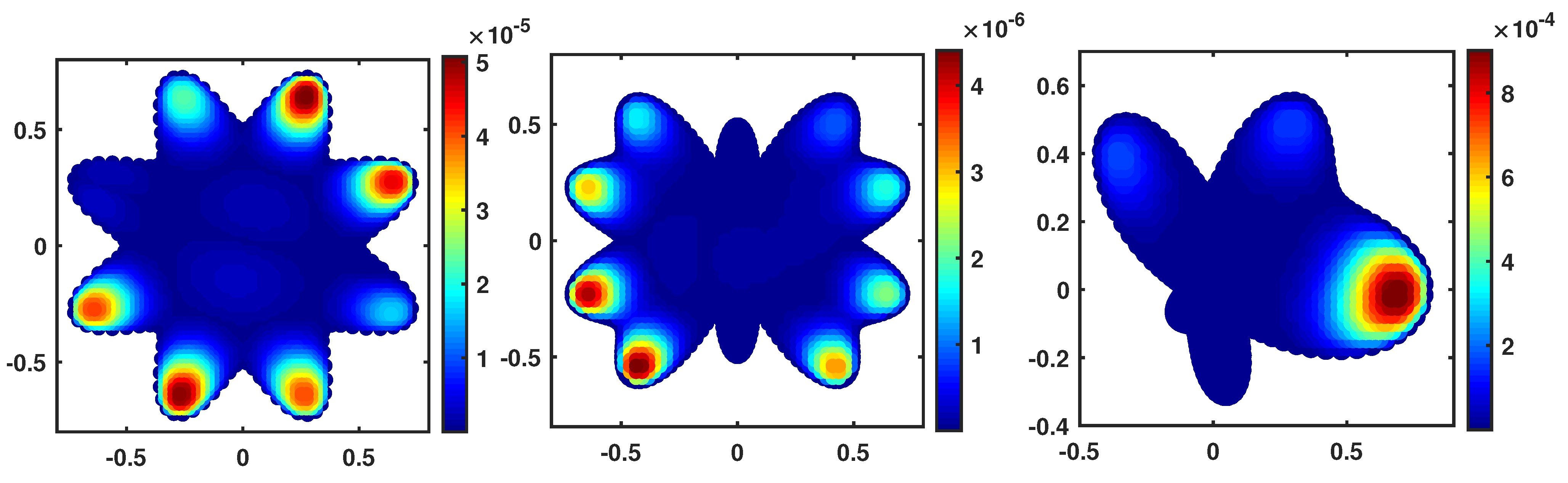
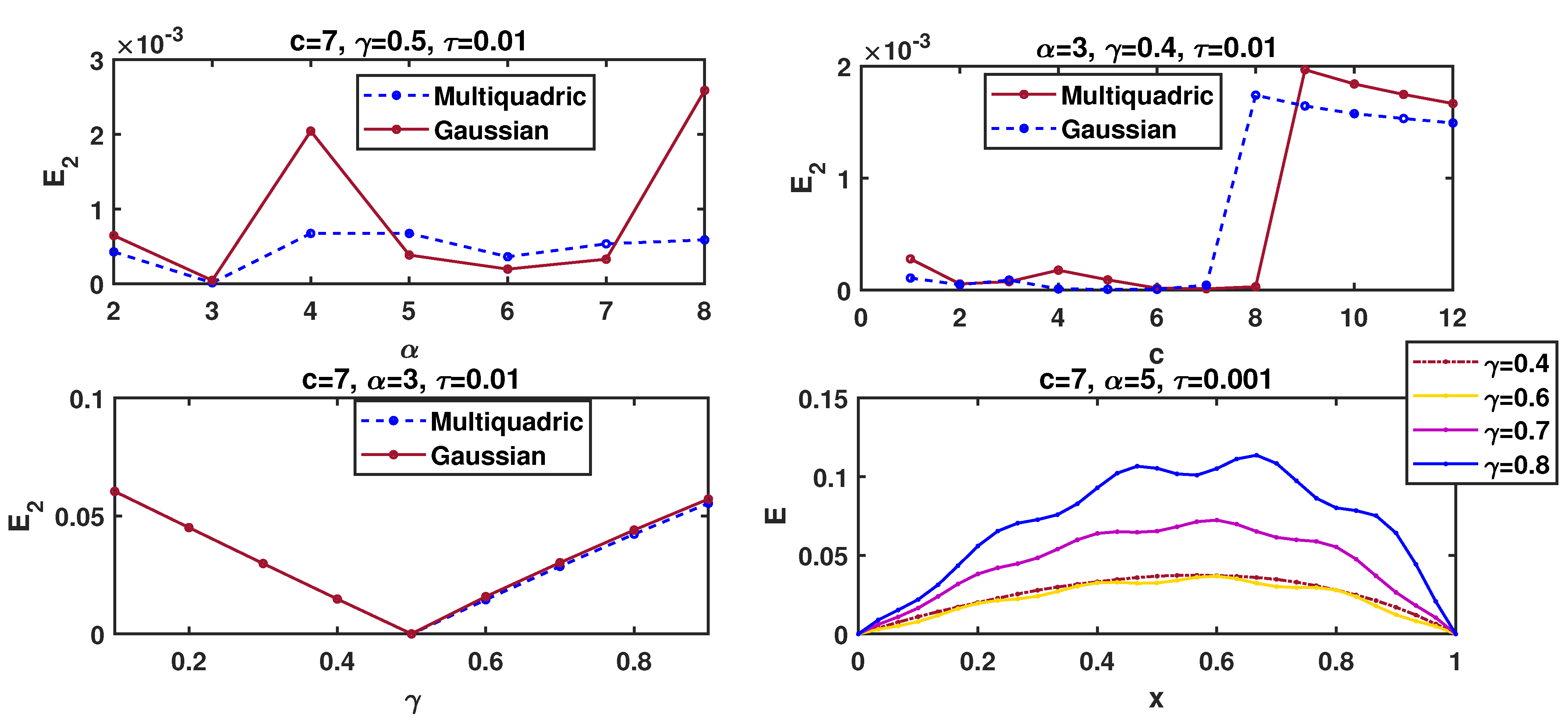
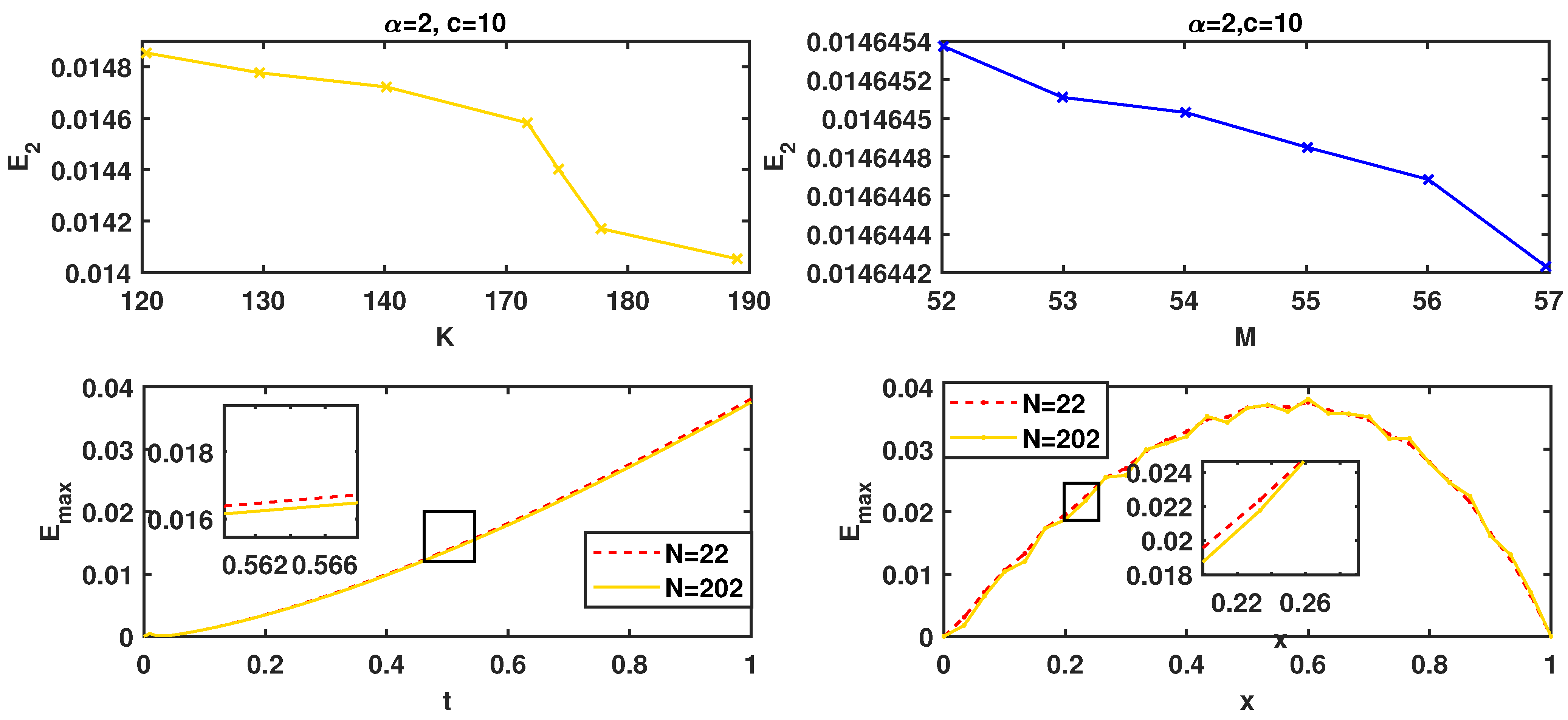

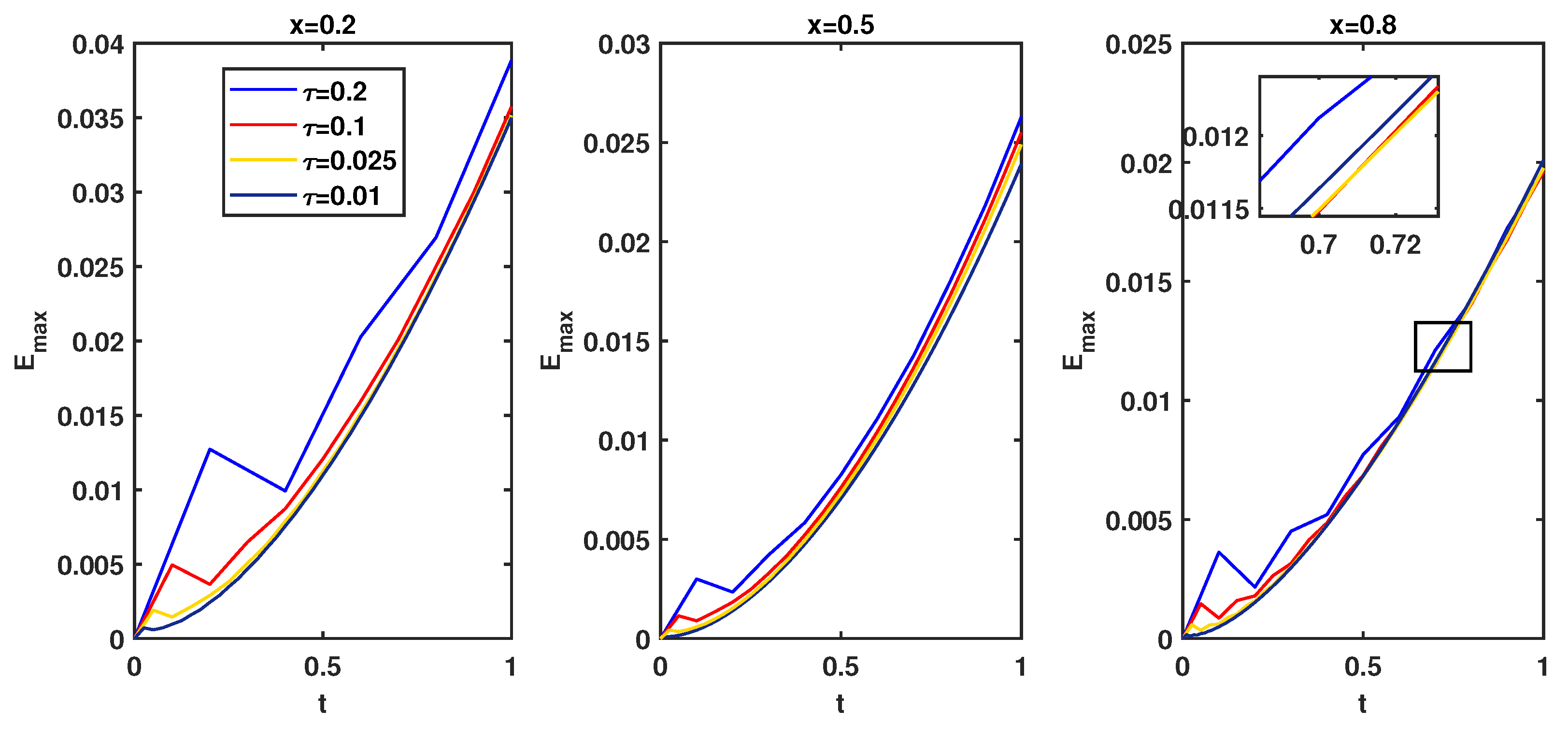

| Basis Functions | ||||||
|---|---|---|---|---|---|---|
| Multiquadric | 3.155 | 2.136 | 2.219 | 6.854 | 3.205 | 1.411 |
| Gaussian | 2.059 | 1.135 | 2.101 | 6.829 | 3.263 | 1.512 |
| T | , | , | , | |||
|---|---|---|---|---|---|---|
| 1 | 5.358 | 2.638 | 1.205 | 3.792 | 3.292 | 2.274 |
| 1.085 | 2.638 | 2.441 | 3.792 | 6.668 | 2.274 | |
| ISEFG Method [9] | Present Method | |||||||
|---|---|---|---|---|---|---|---|---|
| CPU Time | Rate | , | , | , | CPU Time | Rate | ||
| 3.058 | 12 | - | 3.954 | 4.114 | 1.044 | 18.65 | - | |
| 7.649 | 31 | 1.99 | 9.905 | 9.129 | 9.140 | 33.12 | 1.99 | |
| 1.912 | 79 | 1.99 | 2.0588 | 2.811 | 2.100 | 85.16 | 1.93 | |
| Chen [11] | ISEFG [9] | BSM | Chen [11] | ISEFG [9] | BSM | Chen [11] | ISEFG [9] | BSM | |
|---|---|---|---|---|---|---|---|---|---|
| 9.7 | 5.7 | 3.78 | 1.3 | 4.8 | 7.82 | 1.6 | 4.3 | 4.15 | |
| 1.4 | 1.2 | 3.75 | 9.0 | 6.7 | 4.54 | 1.9 | 4.4 | 3.84 | |
| x | t | ||
|---|---|---|---|
| 0.125 | 8.0815 | 3.4780 | 5.9259 |
| 0.250 | 1.6325 | 7.0311 | 1.1986 |
| 0.375 | 2.5009 | 1.0790 | 1.8427 |
| 0.500 | 3.3724 | 1.4520 | 2.4803 |
| 0.625 | 4.2862 | 1.8428 | 3.1485 |
| 0.750 | 5.3120 | 2.2887 | 3.9157 |
| 0.875 | 6.4214 | 2.7739 | 4.7506 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Safari, F.; Tong, Q.; Tang, Z.; Lu, J. A Meshfree Approach for Solving Fractional Galilei Invariant Advection–Diffusion Equation through Weighted–Shifted Grünwald Operator. Mathematics 2022, 10, 4008. https://doi.org/10.3390/math10214008
Safari F, Tong Q, Tang Z, Lu J. A Meshfree Approach for Solving Fractional Galilei Invariant Advection–Diffusion Equation through Weighted–Shifted Grünwald Operator. Mathematics. 2022; 10(21):4008. https://doi.org/10.3390/math10214008
Chicago/Turabian StyleSafari, Farzaneh, Qingshan Tong, Zhen Tang, and Jun Lu. 2022. "A Meshfree Approach for Solving Fractional Galilei Invariant Advection–Diffusion Equation through Weighted–Shifted Grünwald Operator" Mathematics 10, no. 21: 4008. https://doi.org/10.3390/math10214008
APA StyleSafari, F., Tong, Q., Tang, Z., & Lu, J. (2022). A Meshfree Approach for Solving Fractional Galilei Invariant Advection–Diffusion Equation through Weighted–Shifted Grünwald Operator. Mathematics, 10(21), 4008. https://doi.org/10.3390/math10214008







