Transport Phenomena in Excitable Systems: Existence of Bounded Solutions and Absorbing Sets
Abstract
:1. Introduction
2. Mathematical Considerations
3. Conditions for Bounded Solutions
4. Existence of Absorbing Sets
5. Results and Implications
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Keener, J.P.; Sneyd, J. Mathematical Physiology; Springer: New York, NY, USA, 1998. [Google Scholar]
- Murray, J.D. Mathematical Biology I; Springer: New York,, NY, USA, 2002. [Google Scholar]
- Izhikevich, E.M. Dynamical Systems in Neuroscience: The Geometry of Excitability and Bursting; The MIT Press: London, UK, 2007. [Google Scholar]
- Izhikovich, E.M. Neural excitability, spiking and bursting. Int. J. Bifurc. Chaos 2000, 10, 1171–1266. [Google Scholar] [CrossRef]
- Nagumo, J.; Arimoto, S.; Yoshizawa, S. An Active Pulse Transmission Line Simulating Nerve Axon. Proc. IRE 1962, 50, 2061–2070. [Google Scholar] [CrossRef]
- Hodgin, A.I.; Huxley, A. Quantitative description of membrane currents and its applications to conduction and excitation in Nerve. J. Physiol. 1952, 112, 500–544. [Google Scholar] [CrossRef]
- De Angelis, M.; Renno, P. Asymptotic effects of boundary perturbations in excitable systems. Discret. Contin. Dyn. Syst. Ser. D 2014, 19, 2039–2045. [Google Scholar] [CrossRef]
- Kudryashov, N.A.; Rybka, K.R.; Sboev, A. Analytical properties of the perturbed FitzHugh-Nagumo model. Appl. Math. Lett. 2018, 76, 142–147. [Google Scholar] [CrossRef]
- De Angelis, M. A priori estimates for excitable models. Meccanica 2013, 48, 2491–2496. [Google Scholar] [CrossRef]
- Torcicollo, I. On the non-linear stability of a continuous duopoly model with constant conjectural variation. Int. J. Non-Linear Mech. 2016, 81, 268–273. [Google Scholar] [CrossRef]
- De Angelis, M. A wave equation perturbed by viscous terms: Fast and slow times diffusion effects in a Neumann problem. Ric. Mat. 2019, 68, 237–252. [Google Scholar] [CrossRef]
- Gambino, G.; Lombardo, M.C.; Giunta, V.; Rubino, G. Cross-diffusion effects on stationary pattern formation in the FitzHugh-Nagumo model. Discret. Contin. Dyn. Syst. -Ser. B 2022. [Google Scholar] [CrossRef]
- De Angelis, M.; Fiore, G. Diffusion effects in a superconductive model. Commun. Pure Appl. Anal. 2014, 13, 217–223. [Google Scholar] [CrossRef]
- Scott, A.C. The Nonlinear Universe Chaos, Emergence, Life; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- De Angelis, M.; Renno, P. On Asymptotic Effects of Boundary Perturbations in Exponentially Shaped Josephson Junctions. Acta Appl. Math. 2014, 132, 251–259. [Google Scholar] [CrossRef]
- Scott, A.C. Neuroscience: A Mathematical Primer; Springer: New York, NY, USA, 2002. [Google Scholar]
- De Angelis, M. On the transition from parabolicity to hyperbolicity for a nonlinear equation under Neumann boundary conditions. Meccanica 2018, 53, 3651–3659. [Google Scholar] [CrossRef]
- Lin, V.; Liu, W.B.; Bao, H.; Shen, Q. Bifurcation mechanism of periodic bursting in a simple three-element-based memristive circuit with fast-slow effect. Chaos Solitons Fractals 2020, 131, 109524. [Google Scholar] [CrossRef]
- Juzekaeva, E.; Nasretdinov, A.; Battistoni, S.; Berzina, T.; Iannotta, S.; Khazipov, R.; Erokhin, V.; Mukhtarov, M. Coupling Cortical Neurons through Electronic Memristive Synapse. Adv. Mater. Technol. 2019, 4, 1800350. [Google Scholar] [CrossRef]
- Bertram, R.; Manish, T.; Butte, J.; Kiemel, T.; Sherman, A. Topological and phenomenological classification of bursting oscillations. Bull. Math. Biol. 1995, 57, 413. [Google Scholar] [CrossRef]
- Quarteroni, A.; Manzoni, A.; Vergara, C. The cardiovascular system:mathematical modelling, numerical algorithms and clinical applications. Acta Numer. 2017, 26, 365–590. [Google Scholar] [CrossRef]
- Carillo, S.; Schiebold, C. Construction of Soliton Solutions of the Matrix Modified Korteweg–de Vries Equation. In Advances in Nonlinear Dynamics; NODYCON Conference Proceedings Series; Lacarbonara, W., Balachandran, B., Leamy, M.J., Ma, J., Tenreiro Machado, J.A., Stepan, G., Eds.; Springer: Cham, Switzerland, 2022; pp. 481–495. [Google Scholar]
- De Angelis, M. A Note on Explicit Solutions of FitzHugh-Rinzel System. Nonlinear Dyn. Syst. Theory 2021, 21, 360–366. [Google Scholar] [CrossRef]
- Li, H.; Guoa, Y. New exact solutions to the Fitzhugh Nagumo equation. Appl. Math. Comput. 2006, 180, 524–528. [Google Scholar] [CrossRef]
- Zemlyanukhin, A.I.; Bochkarev, A.V. Analytical Properties and Solutions of the FitzHugh–Rinzel Model. Rus. J. Nonlin. Dyn. 2019, 15, 3–12. [Google Scholar] [CrossRef]
- De Angelis, F.; De Angelis, M. On solutions to a FitzHugh–Rinzel type model. Ric. Mat. 2021, 70, 51–65. [Google Scholar] [CrossRef]
- Smoller, J. Shock Waves and Reaction-Diffusion Equations, 2nd ed.; Springer: New York, NY, USA, 1994. [Google Scholar]
- Capone, F.; Carfora, M.F.; De Luca, R.; Torcicollo, I. Turing patterns in a reaction–diffusion system modeling hunting cooperation. Math. Comput. Simul. 2019, 165, 172–180. [Google Scholar] [CrossRef]
- De Angelis, M. A priori estimates for solutions of FitzHugh–Rinzel system. Meccanica 2022, 57, 1035–1045. [Google Scholar] [CrossRef]
- Rionero, S. Longtime behaviour and bursting frequency, via a simple formula, of FitzHugh–Rinzel neurons. Rend. Lincei. Sci. Fis. e Nat. 2021, 32, 857–867. [Google Scholar] [CrossRef]
- Xie, W.; Xu, J.; Cai, L.; Jin, Y. Dynamics and geometric desingularization of the multiple time scale Fitzhugh Nagumo Rinzel model with fold singularity. Commun. Nonlinear Sci. Numer. Simul. 2018, 63, 322–338. [Google Scholar] [CrossRef]
- Gutman, M.; Aviram, I.; Rabinovitch, A. Abnormal frequency locking and the function of the cardiac pacemaker. Phys. Rev. E 2004, 70, 037202. [Google Scholar] [CrossRef] [PubMed]
- Dikansky, A. Fitzhugh-Nagumo equations in a nonhomogeneous medium. Discret. Contin. Dyn. Syst. 2005, 2005, 216–224. [Google Scholar]
- Faghih, R.T.; Savla, K.; Dahleh, M.A.; Brown, E.N. Broad Range of Neural Dynamics From a Time-Varying FitzHugh–Nagumo Model and its Spiking Threshold Estimation. IEEE Trans. Biomed. Eng. 2012, 59, 816–823. [Google Scholar] [CrossRef]
- Rinzel, J. A Formal Classification of Bursting Mechanisms in Excitable Systems. In Mathematical Topics in Population Biology, Morphogenesis and Neurosciences; Lecture Notes in Biomathematics; Teramoto, E., Yumaguti, M., Eds.; Springer: Berlin/Heidelberg, Germany, 1987; Volume 71, pp. 8–26. [Google Scholar]
- Rocsoreanu, C.; Georgescu, A.; Giurgiteanu, N. The FitzHugh-Nagumo Model: Bifurcation and Dynamics; Springer Science Business Media: Berlin/Heidelberg, Germany, 2012; Volume 10. [Google Scholar]
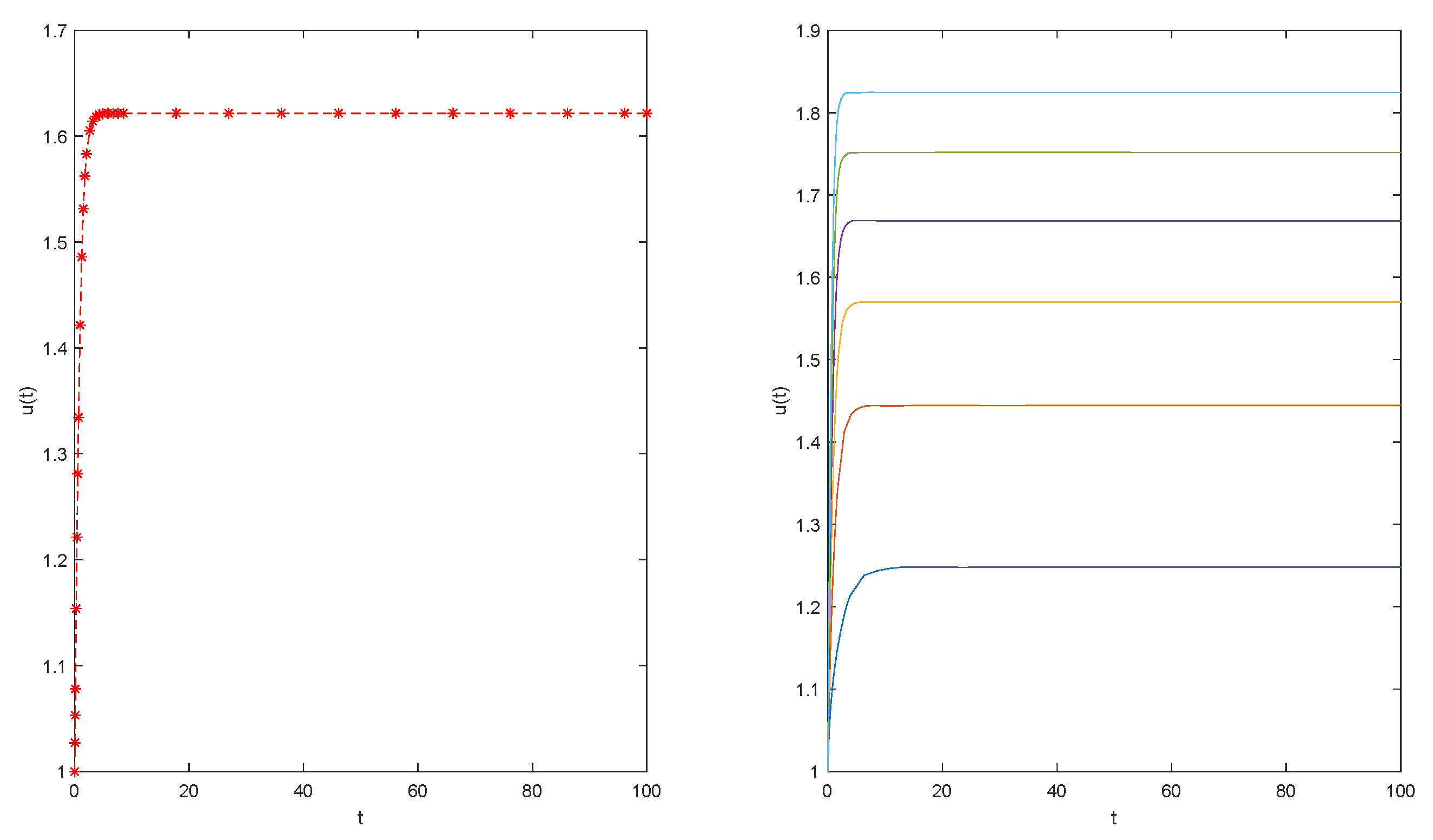
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
De Angelis, M. Transport Phenomena in Excitable Systems: Existence of Bounded Solutions and Absorbing Sets. Mathematics 2022, 10, 2041. https://doi.org/10.3390/math10122041
De Angelis M. Transport Phenomena in Excitable Systems: Existence of Bounded Solutions and Absorbing Sets. Mathematics. 2022; 10(12):2041. https://doi.org/10.3390/math10122041
Chicago/Turabian StyleDe Angelis, Monica. 2022. "Transport Phenomena in Excitable Systems: Existence of Bounded Solutions and Absorbing Sets" Mathematics 10, no. 12: 2041. https://doi.org/10.3390/math10122041
APA StyleDe Angelis, M. (2022). Transport Phenomena in Excitable Systems: Existence of Bounded Solutions and Absorbing Sets. Mathematics, 10(12), 2041. https://doi.org/10.3390/math10122041





