A New Kantorovich-Type Rational Operator and Inequalities for Its Approximation
Abstract
1. Introduction
2. Definition of Kantorovich-Type Operator
3. A Korovkin-Type Approximation Result
4. Rates of Convergence
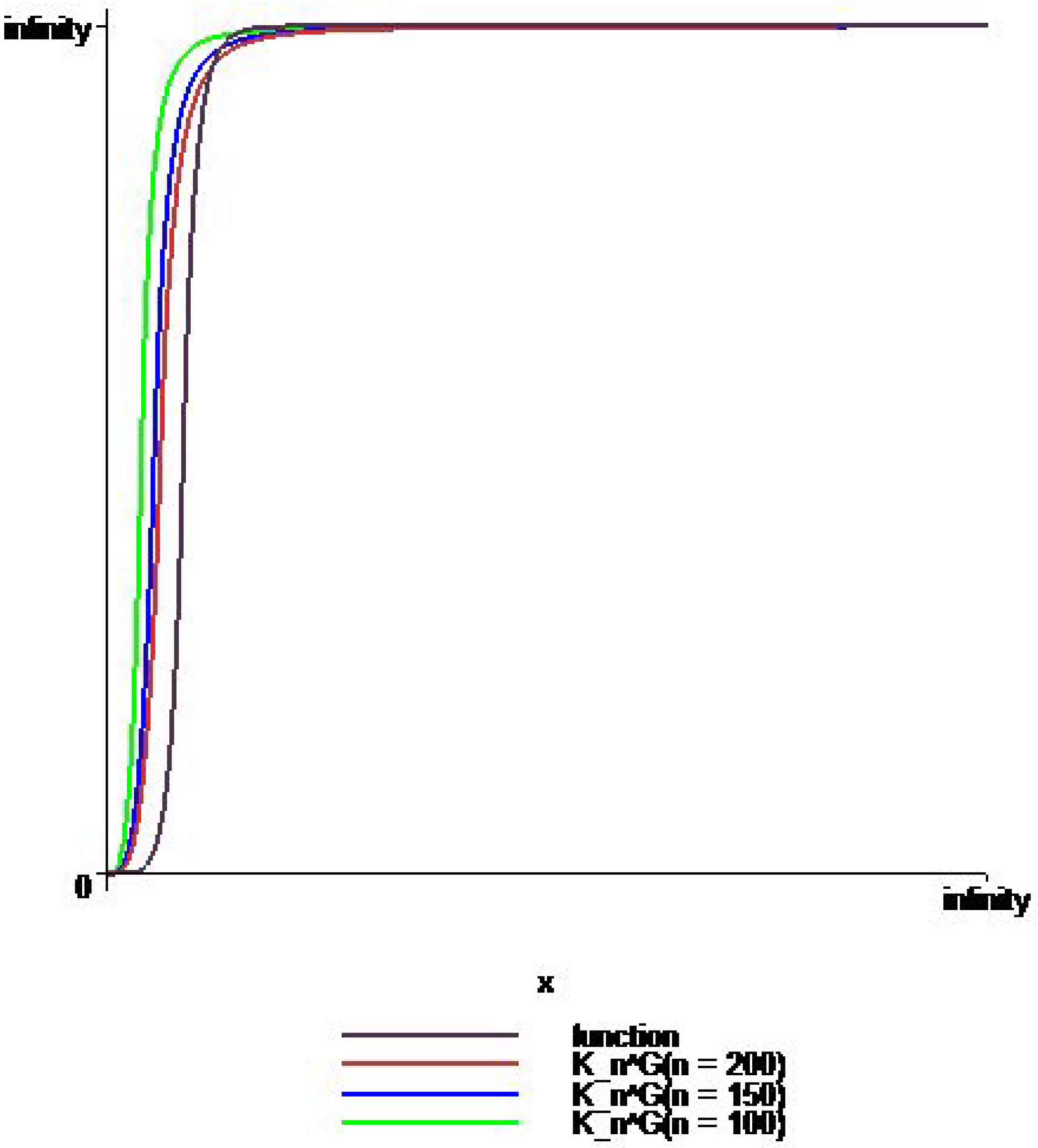
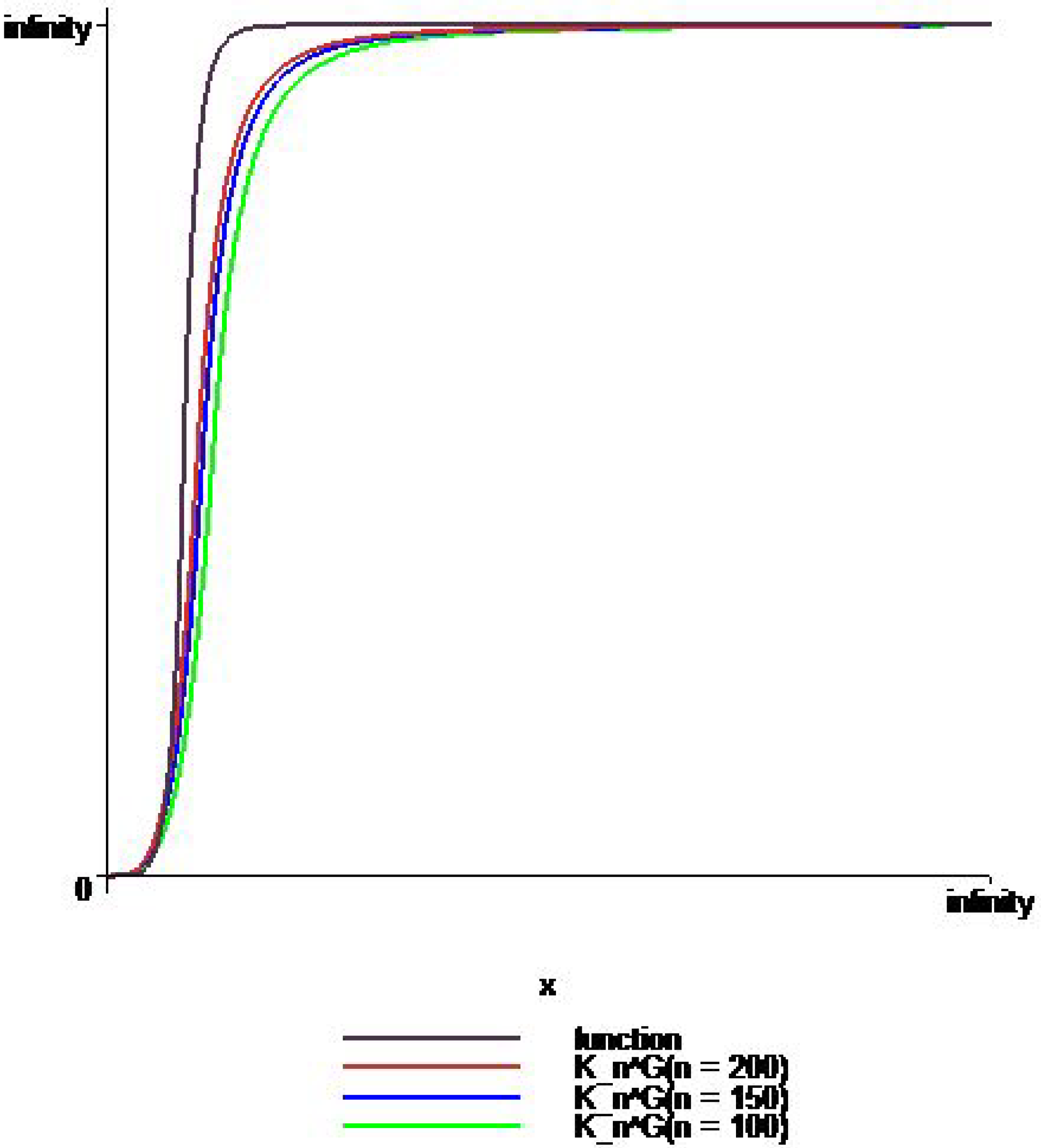
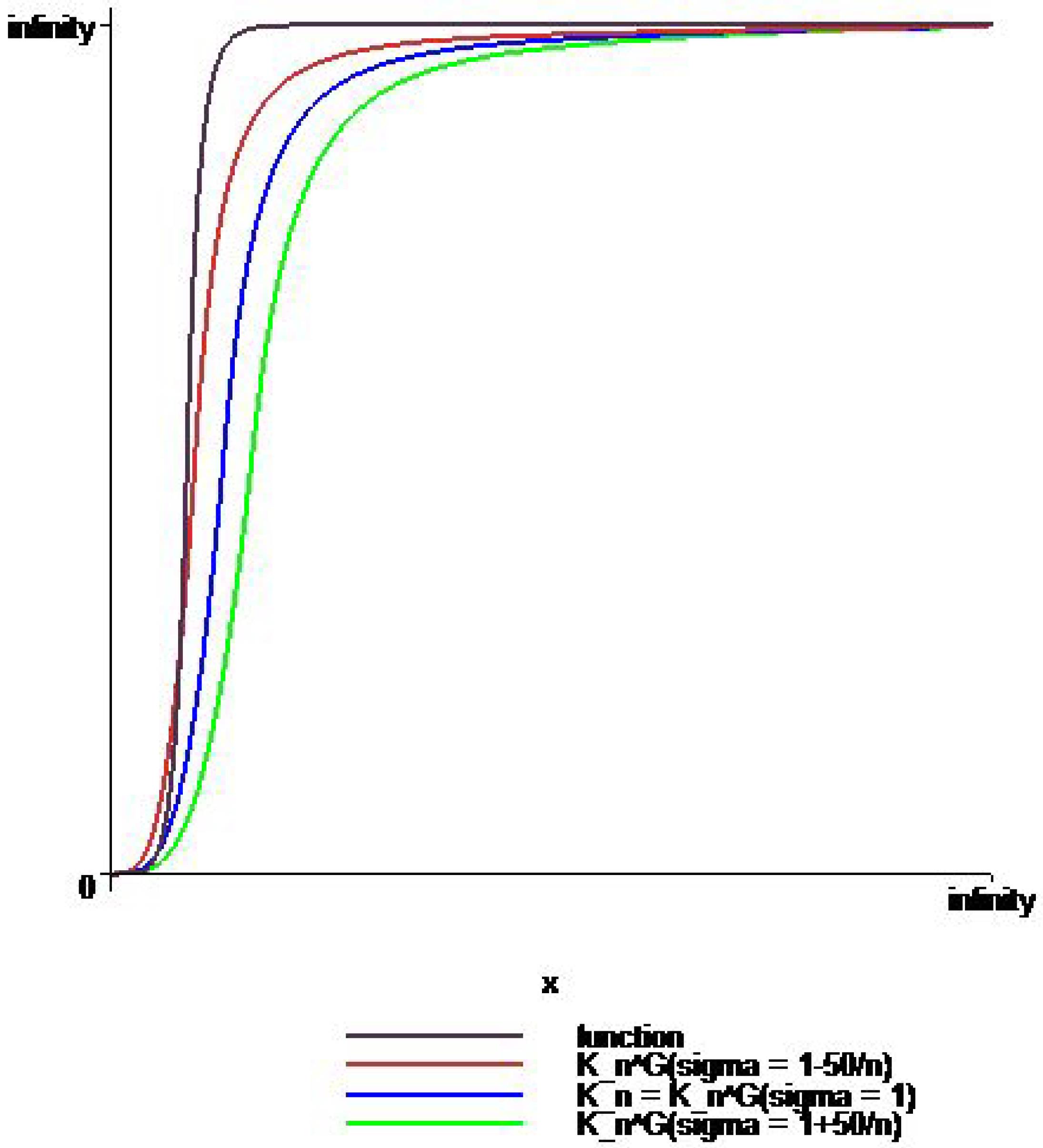
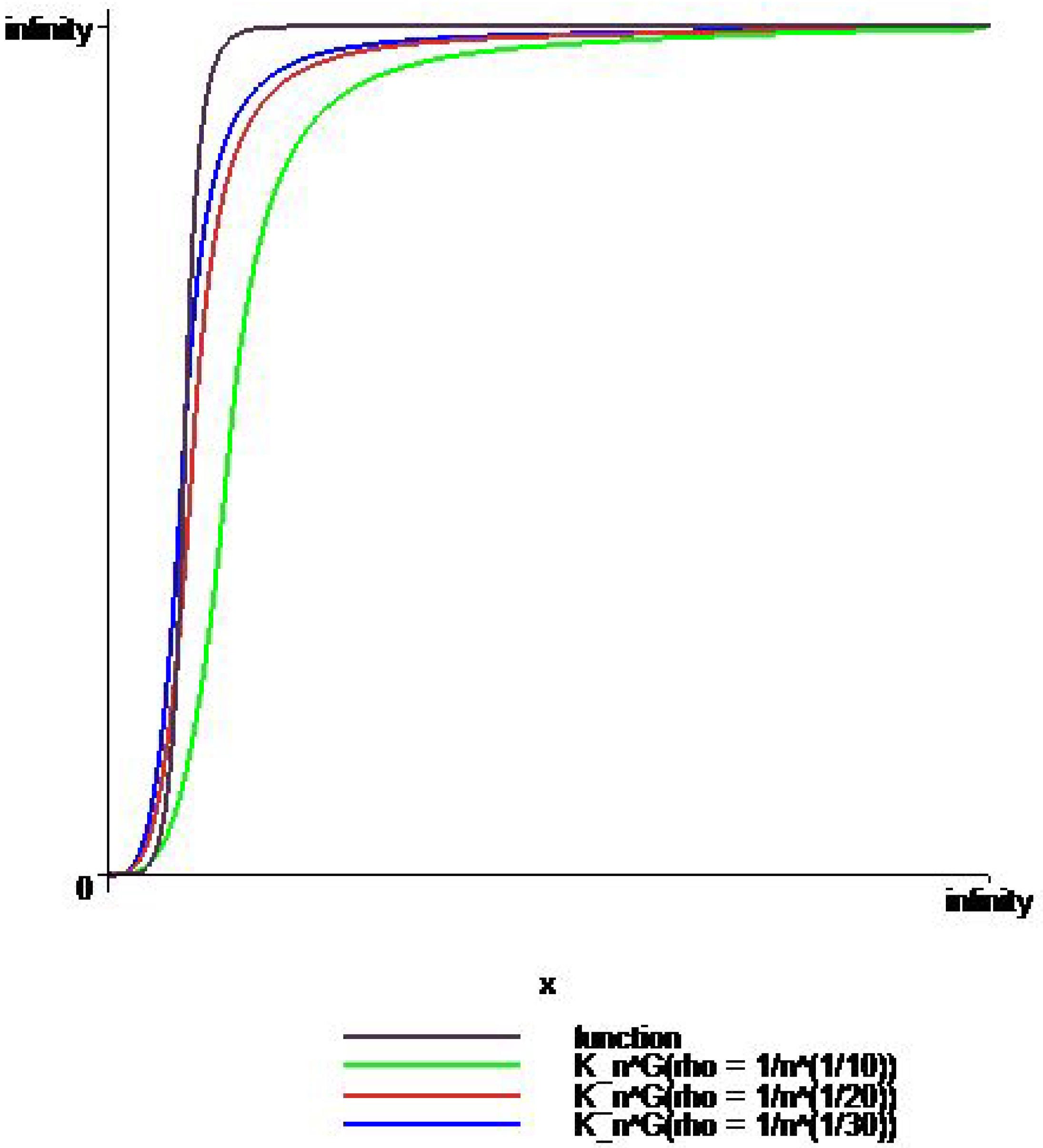
5. Some Comparisons
6. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Balázs, K. Approximation by Bernstein type rational functions. Acta Math. Hung. 1975, 26, 123–134. [Google Scholar] [CrossRef]
- Balázs, K.; Szabados, J. Approximation by Bernstein type rational functions II. Acta Math. Hung. 1982, 40, 331–337. [Google Scholar] [CrossRef]
- Balázs, K. Approximation by Bernstein type rational functions on the real axis. Acta Math. Hung. 1985, 46, 195–204. [Google Scholar] [CrossRef]
- Totik, V. Saturation for Bernstein type rational functions. Acta Math. Hung. 1984, 43, 219–250. [Google Scholar] [CrossRef]
- Abel, U.; Vecchia, B.D. Asymptotic approximation by the operators of K. Balázs and Szabados. Acta Sci. Math. (Szeged) 2000, 66, 137–145. [Google Scholar]
- Agratini, O. On a class of Bernstein-type rational functions. Numer. Funct. Anal. Optim. 2020, 41, 483–494. [Google Scholar] [CrossRef]
- Agratini, O. Approximation process on Kantorovich type. Miskolc Math. Notes 2001, 2, 3–10. [Google Scholar] [CrossRef]
- Agratini, O. On approximation properties Balázs-Szabados operators and their Kantorovich extension. Korean J. Comput. Appl. Math. 2002, 9, 361–372. [Google Scholar] [CrossRef]
- Gupta, V.; İspir, N. On Bézier variant of generalized Kantorovich type Balázs operators. Appl. Math. Lett. 2005, 18, 1053–1061. [Google Scholar] [CrossRef][Green Version]
- Gupta, V.; Lupaş, A. On the rate of approximation for the Bézier variant of Kantorovich-Balázs operators. Gen. Math. 2004, 1, 3–18. [Google Scholar] [CrossRef]
- Gupta, V.; Zeng, X.M. Rate of approximation for the Bézier variant of Balázs Kantorovich operators. Math. Slovaca 2007, 57, 349–358. [Google Scholar] [CrossRef]
- Özkan, E.Y. Approximation properties of Kantorovich type q-Balázs-Szabados operators. Demonstr. Math. 2019, 52, 10–19. [Google Scholar] [CrossRef]
- Hamal, H.; Sabancıgil, P. Some approximation properties of new Kantorovich type q-analogue of Balázs-Szabados operators. J. Inequal. Appl. 2020, 2020, 159. [Google Scholar] [CrossRef]
- Özkan, E.Y.; Aksoy, G. On a new generalization of Bernstein-type rational functions and its approximation. Mathematics 2022, 10, 973. [Google Scholar] [CrossRef]
- Korovkin, P.P. On convergence of linear positive operators in the space of continuous functions. Dokl. Akad. Nauk. SSSR 1953, 90, 961–964. [Google Scholar]
- Devore, R.A.; Lorentz, G.G. Constructive Approximation: Polynomials and Splines Approximation; Springer: Berlin/Heidelberg, Germany, 1993. [Google Scholar]
- Butzer, P.L.; Berens, H. Semi-Groups of Operators and Approximation; Springer: New York, NY, USA, 1967. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Özkan, E.Y. A New Kantorovich-Type Rational Operator and Inequalities for Its Approximation. Mathematics 2022, 10, 1982. https://doi.org/10.3390/math10121982
Özkan EY. A New Kantorovich-Type Rational Operator and Inequalities for Its Approximation. Mathematics. 2022; 10(12):1982. https://doi.org/10.3390/math10121982
Chicago/Turabian StyleÖzkan, Esma Yıldız. 2022. "A New Kantorovich-Type Rational Operator and Inequalities for Its Approximation" Mathematics 10, no. 12: 1982. https://doi.org/10.3390/math10121982
APA StyleÖzkan, E. Y. (2022). A New Kantorovich-Type Rational Operator and Inequalities for Its Approximation. Mathematics, 10(12), 1982. https://doi.org/10.3390/math10121982





