ρ — Adic Analogues of Ramanujan Type Formulas for 1/π

Abstract

Following Ramanujan’s work on modular equations and approximations of π, there are formulas for $1/\pi$ of the form

$$\sum _{k=0}^{\infty }\frac{{\left(\frac{1}{2}\right)}_k{\left(\frac{1}{d}\right)}_k{\left(\frac{d-1}{d}\right)}_k}{k{!}^3}(ak+1){\left({\lambda }_d\right)}^k=\frac{\delta }{\pi }$$

for $d=2,3,4,6,$ where ${\lambda }_d$ are singular values that correspond to elliptic curves with complex multiplication, and $a,\delta$ are explicit algebraic numbers. In this paper we prove a $p-$adic version of this formula in terms of the so-called Ramanujan type congruence. In addition, we obtain a new supercongruence result for elliptic curves with complex multiplication.

1. Introduction

Ramanujan [1] gave a list of infinite series identities of the form

$$\sum _{k=0}^{\infty }\frac{{\left(\frac{1}{2}\right)}_k{\left(\frac{1}{d}\right)}_k{\left(\frac{d-1}{d}\right)}_k}{k{!}^3}(ak+1){\left({\lambda }_d\right)}^k=\frac{\delta }{\pi }$$

for $d=2,3,4,6,$ where ${\lambda }_d$ are singular values that correspond to elliptic curves with complex multiplication, and $a,\delta$ are explicit algebraic numbers. Below is an example of one identity from Ramanujan’s list,

$$\sum _{k=0}^{\infty }\frac{{\left(\frac{1}{2}\right)}_k^3}{k{!}^3}(6k+1)\frac{1}{4^k}=\frac{4}{\pi }$$

Here, ${\left(a\right)}_k$ denotes the rising factorial ${\left(a\right)}_k=a(a+1)\cdots (a+k-1).$ In fact, the following similar identity was given earlier by Bauer [2],

$$\sum _{k=0}^{\infty }\frac{{\left(\frac{1}{2}\right)}_k^3}{k{!}^3}(4k+1){(-1)}^k=\frac{2}{\pi }$$

Proofs of these formulas were first given by J. Borwein and P. Borwein [3] and D. Chudnovsky and G. Chudnovsky [4]. Both approaches rely on the arithmetic of elliptic integrals of the first and second kind, including the Legendre relation at singular values. Finding new formulas for $1/{\pi }^k$ using various techniques has been an active research area. We refer interested readers to two survey papers, one by Baruah, Berndt, and Chan [5] and another by Zudilin [6], as well as a list of conjectures due to Z.-W. Sun [7]. One of the motivations for studying Ramanujan formulas for $1/\pi$ is to efficiently compute the decimal digits of $\pi .$

In 1997, van Hamme discovered several surprising $p-$adic analogues of Ramanujan formulas for $1/\pi$ [8]. For each prime $p>3$ he conjectured

$$\begin{array}{ccc}\hfill \sum _{k=0}^{p-1}\frac{{\left(\frac{1}{2}\right)}_k^3}{k{!}^3}(4k+1){(-1)}^k& \equiv & \left(\frac{-1}{p}\right)p(mod{p}^3)\hfill \end{array}$$

(1.1)

$$\begin{array}{ccc}\hfill \sum _{k=0}^{\frac{p-1}{2}}\frac{{\left(\frac{1}{2}\right)}_k^3}{k{!}^3}(6k+1)\frac{1}{4^k}& \equiv & \left(\frac{-1}{p}\right)p(mod{p}^4)\hfill \end{array}$$

(1.2)

where $\left(\frac{·}{p}\right)$ is the Legendre symbol.

The congruence (1.1) was first proved by Mortenson using hypergeometric evaluation identities [9]. This method is dependent upon the availability of such corresponding identities. Later, Equation (1.1) was also established by Zudilin using the techniques of Wilf and Zeilberger [10], which, unfortunately, are not easy to use in general. Motivated by work of Mortenson [9] as well as by McCarthy and Osburn [11], the third author proved congruence (1.2) [12]. Recently, Z.-W. Sun has given a refinement of congruence (1.1) modulo $p^4$ by adding a factor involving Euler numbers [13].

It is interesting to note that both Equations (1.1) and (1.2) hold modulo $p^3$ with either $p-1$ or $(p-1)/2$ for the limit of summation. However, this is not true modulo $p^4$ for either Equation (1.2) or Sun’s refinement of Equation (1.1).

In this paper, we prove a general result on Ramanujan type congruences modulo $p^2$ under further assumptions. In Section 2, we introduce necessary notation and state our main result. Our method relies on the arithmetic and geometry of elliptic curves, which includes Picard–Fuchs equations, formal expansions of the invariant differentials of elliptic curves, the Chowla–Selberg formula for periods of elliptic curves with complex multiplication, as well as Atkin and Swinnerton–Dyer congruences and results due to Katz. In Section 3, we review a method to prove Ramanujan type formulas for $1/\pi$ utilizing Picard–Fuchs equations associated to families of elliptic curves, originating in the work of Chowla and Selberg [14]. In Section 4 we discuss arithmetic of certain families of elliptic curves. We conclude in Section 5 with the proof of our result.

2. Statement of Results

For r a nonnegative integer and ${\alpha }_i,{\beta }_i\in \mathbb{C}$, the hypergeometric series ${}_{r+1}{F}_r$ is defined by

$${}_{r+1}{F}_r\left[\begin{array}{ccc}{\alpha }_1& \dots & {\alpha }_{r+1}\\ {\beta }_1& \dots & {\beta }_r\end{array};x\right]=\sum _{k=0}^{\infty }\frac{{\left({\alpha }_1\right)}_k{\left({\alpha }_2\right)}_k\dots {\left({\alpha }_{r+1}\right)}_k}{{\left({\beta }_1\right)}_k\dots {\left({\beta }_r\right)}_k}·\frac{x^k}{k!}$$

which converges for $\left|x\right|<1$. We write

$${}_{r+1}{F}_r{\left[\begin{array}{ccc}{\alpha }_1& \dots & {\alpha }_{r+1}\\ {\beta }_1& \dots & {\beta }_r\end{array};x\right]}_n=\sum _{k=0}^n\frac{{\left({\alpha }_1\right)}_k{\left({\alpha }_2\right)}_k\dots {\left({\alpha }_{r+1}\right)}_k}{{\left({\beta }_1\right)}_k\dots {\left({\beta }_r\right)}_k}·\frac{x^k}{k!}$$

to denote the truncation of the series after the $x^n$ term.

For $d\in \{2,3,4,6\}$ let ${\tilde{E}}_d\left(t\right)$ denote the following families of elliptic curves parameterized by t,

$$\begin{array}{cc}\hfill {\tilde{E}}_2\left(t\right):& y^2=x(x-1)(x-t)\hfill \\ \hfill {\tilde{E}}_3\left(t\right):& y^2+xy+\frac{t}{27}y=x^3\hfill \\ \hfill {\tilde{E}}_4\left(t\right):& y^2=x(x^2+x+\frac{t}{4})\hfill \\ \hfill {\tilde{E}}_6\left(t\right):& y^2+xy=x^3-\frac{t}{432}\hfill \end{array}$$

There are in fact many ways to choose such models. Recall that an elliptic curve over a number field is said to have complex multiplication (CM) if its endomorphism ring over $\overline{\mathbb{Q}}$ is an order of an imaginary quadratic field.

For t such that ${\tilde{E}}_d\left(t\right)$ has CM, let ${\lambda }_d=-4t(t-1)$, and write $E_d\left({\lambda }_d\right)={\tilde{E}}_d\left(\frac{1-\sqrt{1-{\lambda }_d}}{2}\right)$. We note that $t=\frac{1-\sqrt{1-{\lambda }_d}}{2}$ is determined up to a choice of square root, but we will see this does not affect our conclusion. Assume $|{\lambda }_d|<1$ for any embedding. Then there is a Ramanujan type formula

$$\sum _{k=0}^{\infty }\frac{{\left(\frac{1}{2}\right)}_k{\left(\frac{1}{d}\right)}_k{\left(\frac{d-1}{d}\right)}_k}{k{!}^3}{\left({\lambda }_d\right)}^k(ak+1)=\frac{\delta }{\pi }$$

(2.1)

for some unique algebraic numbers $a,\delta$ depending on d and ${\lambda }_d$. To be more explicit, a can be computed from a so-called singular value function [3]. Specific choices of CM values of ${\lambda }_d$ as well as the corresponding constants $a,\delta$ can be derived from various data given by the Borweins [3].

Theorem 1. For $d\in \{2,3,4,6\}$, let ${\lambda }_d\in \overline{\mathbb{Q}}$ such that $\mathbb{Q}\left({\lambda }_d\right)$ is totally real, the elliptic curve $E_d\left({\lambda }_d\right)$ has complex multiplication, and $|{\lambda }_d|<1$ for an embedding of ${\lambda }_d$ to $\mathbb{C}$. For each prime p that is unramified in $\mathbb{Q}\left(\sqrt{1-{\lambda }_d}\right)$ and coprime to the discriminant of $E_d\left({\lambda }_d\right)$ such that $a,{\lambda }_d$ can be embedded in ${\mathbb{Z}}_p^{*}$ (and we fix such embeddings), then

$$\sum _{k=0}^{p-1}\frac{{\left(\frac{1}{2}\right)}_k{\left(\frac{1}{d}\right)}_k{\left(\frac{d-1}{d}\right)}_k}{k{!}^3}{\left({\lambda }_d\right)}^k(ak+1)\equiv \text{sgn}·\left(\frac{1-{\lambda }_d}{p}\right)·p(mod{p}^2)$$

where $\left(\frac{1-{\lambda }_d}{p}\right)$ is the Legendre symbol, and $\text{sgn}=\pm 1$, equaling 1 if and only if $E_d\left({\lambda }_d\right)$ is ordinary modulo p.

Remark 1. In fact, Zudilin conjectured the above to be true modulo $p^3$ when $d=2,3,4,6$.

Remark 2. Our conclusion in Theorem 1 also holds for totally real singular moduli with $|{\lambda }_d|\ge 1$. In either case, the values of a are predicted by the Chowla–Selberg formula [14]. See work of Guillera and Zudilin for existing “divergent” Ramanujan type supercongruences [15].

Remark 3. When $d=2$, there is an underlying $K3$ surface $X_{\lambda }$ described by the equation

$$X_{\lambda }:z^2=x(x-1)y(y-1)(x-\lambda y)$$

When $\lambda \ne -1$, this manifold is related to the one-parameter family of elliptic curves of the form

$$E_{\lambda }:y^2=(x-1)\left(x^2-\frac{1}{1-\lambda }\right)$$

(2.2)

via the so-called Shioda–Inose structure [16]. The arithmetic relation between $X_{\lambda }$ and $E_{\lambda }$ is obtained by Ahlgren, Ono, and Penniston [17]. The $j-$invariant of $E_{\lambda }$ is

$$j\left(E_{\lambda }\right)=64\frac{{(4-\lambda )}^3}{{\lambda }^2}$$

In fact, $E_{\lambda }$ is isomorphic to $E_2\left(\lambda \right)$ as above. In the next section, we will use the curve $E_{\lambda }$ to demonstrate some basic ingredients.

Moreover, we obtain the following supercongruence. The case for $d=2$ was proved by Kibelbek, Long, Moss, Sheller and Yuan [18].

Theorem 2. With notation and assumptions as in Theorem 1,

$$\sum _{k=0}^{p-1}\frac{{\left(\frac{1}{2}\right)}_k{\left(\frac{1}{d}\right)}_k{\left(\frac{d-1}{d}\right)}_k}{k{!}^3}{\left({\lambda }_d\right)}^k\equiv L(mod{p}^2)$$

Here $L=0$ when $E_d\left({\lambda }_d\right)$ has supersingular reduction at p, and otherwise $L=\left(\frac{1-{\lambda }_d}{p}\right){\alpha }_p^2$ where ${\alpha }_p$ is the unit root of the geometric Frobenius at p acting on the first cohomology of the elliptic curve $E_d\left({\lambda }_d\right)$.

3. Ramanujan Type Formula for $1/\pi$

In this section we discuss one method for obtaining Ramanujan type formulas for expansions of $1/\pi$. These formulas were even known prior to Ramanujan by mathematicians including Bauer [2]. Systematic ways to obtain such formulas have been studied both by the Borwein brothers [3] and the Chudnovsky brothers [4]. Formulas of the form Equation (2.1) are obtained through either the classical Legendre relation between periods and quasi-periods at singular values or the Wronskian of the Picard–Fuchs equation associated to the corresponding families of elliptic curves. In the next section we demonstrate an algebraic perspective that dates back to Chowla and Selberg [14] and has recently been recast by Zudilin [6]. The family of elliptic curves we will analyze is $E_{\lambda }$ given by Equation (2.2) above.

3.1. Hypergeometric Formulas

Here, we collect some well-known hypergeometric series identities that are useful for our discussion. The first two formulas have been described by Andrews, Askey, and Roy [19]. For parameters $a,b,c$ and value of x such that both sides are well-defined,

$$\begin{array}{ccc}\hfill {}_2{F}_1\left[\begin{array}{cc}a& b\\ & c\end{array};x\right]& =& {(1-x)}^{-a}{}_2{F}_1\left[\begin{array}{cc}a& c-b\\ & c\end{array};\frac{x}{x-1}\right]\hfill \end{array}$$

(3.1)

$$\begin{array}{ccc}\hfill {}_2{F}_1\left[\begin{array}{cc}a& b\\ & a-b+1\end{array};x\right]& =& {(1-x)}^{-a}{}_2{F}_1\left[\begin{array}{cc}\frac{a}{2}& \frac{1+a}{2}-b\\ & a-b+1\end{array};\frac{-4x}{{(1-x)}^2}\right]\hfill \end{array}$$

(3.2)

where we note that Equation (3.1) is due to Pfaff. It follows that

$${}_2{F}_1\left[\begin{array}{cc}1-a& a\\ & 1\end{array};x\right]={}_2{F}_1\left[\begin{array}{cc}\frac{1-a}{2}& \frac{a}{2}\\ & 1\end{array};-4x(x-1)\right]$$

(3.3)

as

$$\begin{array}{ccc}\hfill {}_2{F}_1\left[\begin{array}{cc}1-a& a\\ & 1\end{array};x\right]& =& {(1-x)}^{a-1}{}_2{F}_1\left[\begin{array}{cc}1-a& 1-a\\ & 1\end{array};\frac{x}{x-1}\right]\text{by}\text{Equation}\left(3.1\right)\hfill \\ & =& {}_2{F}_1\left[\begin{array}{cc}\frac{1-a}{2}& \frac{a}{2}\\ & 1\end{array};-4x(x-1)\right]\text{by}\text{Equation}\left(3.2\right).\hfill \end{array}$$

Thus, we state the following lemma.

Lemma 3. For x such that both hand sides converge,

$${}_2{F}_1\left[\begin{array}{cc}1-a& a\\ & 1\end{array};\frac{1\pm \sqrt{1-x}}{2}\right]={}_2{F}_1\left[\begin{array}{cc}\frac{1-a}{2}& \frac{a}{2}\\ & 1\end{array};x\right]$$

            ☐

Clausen’s formula [19] states that

$${}_2{F}_1{\left[\begin{array}{cc}a& b\\ & a+b+\frac{1}{2}\end{array};x\right]}^2={}_3{F}_2\left[\begin{array}{ccc}2a& 2b& a+b\\ & a+b+\frac{1}{2}& 2a+2b\end{array};x\right]$$

(3.4)

Combining Clausen’s formula with Lemma 3 yields the following

$${}_2{F}_1{\left[\begin{array}{cc}1-a& a\\ & 1\end{array};\frac{1\pm \sqrt{1-x}}{2}\right]}^2={}_2{F}_1{\left[\begin{array}{cc}\frac{1-a}{2}& \frac{a}{2}\\ & 1\end{array};x\right]}^2={}_3{F}_2\left[\begin{array}{ccc}\frac{1}{2}& 1-a& a\\ & 1& 1\end{array};x\right]$$

(3.5)

In the following section, these identities will be used to formulate solutions to the Picard–Fuchs equations of $E_d\left({\lambda }_d\right)$.

3.2. Picard–Fuchs Equation

We now compute the Picard–Fuchs equation satisfied by the periods of $E_{\lambda }:y^2=(x-1)(x^2-\frac{1}{1-\lambda })$. The differential of the first kind, ${\omega }_{\lambda }$, up to a scalar is given by

$${\omega }_{\lambda }=\frac{dx}{y}=\frac{dx}{\sqrt{(x-1)(x^2-\frac{1}{1-\lambda })}}$$

while a differential of the second kind, ${\eta }_{\lambda },$ is given by

$${\eta }_{\lambda }=(x-1)\frac{dx}{y}=\frac{(x-1)dx}{\sqrt{(x-1)(x^2-\frac{1}{1-\lambda })}}=\sqrt{\frac{x-1}{x^2-\frac{1}{1-\lambda }}}dx$$

Note that $y={(x-1)}^{\frac{1}{2}}{(x^2-(1/(1-\lambda )))}^{\frac{1}{2}}$ and, to ease notation, let

$$\begin{array}{ccc}q\hfill & :=\hfill & 3/8x^4-x^3/2-(2-\lambda )/\left(8(1-\lambda )\right)x^2+(2-\lambda )/\left(4(1-\lambda )\right)x-1/\left(8(1-\lambda )\right)\hfill \\ g_{\lambda }\hfill & :=\hfill & q/\left(y^3\right)\hfill \end{array}$$

We may view ${\omega }_{\lambda }=:\omega$ as a function of the parameter λ. Denote ${\omega }^{\prime }:=\frac{d}{d\lambda }\omega$, and ${\omega }^{\prime \prime }:=\frac{d^2}{d{\lambda }^2}\omega$. One may compute that

$$d{g}_{\lambda }=\lambda {(1-\lambda )}^2{\omega }^{\prime \prime }+{(1-\lambda )}^2{\omega }^{\prime }-3/16\omega$$

As $d{g}_{\lambda }$ is an exact form, the integral of $d{g}_{\lambda }$ is 0 and we obtain that the periods of the elliptic curve $E_{\lambda }$ satisfy the Picard–Fuchs equation

$$P{F}_{\lambda }:\frac{d^2}{d{\lambda }^2}f+\frac{1}{\lambda }\frac{d}{d\lambda }f+\frac{3}{16\lambda {(1-\lambda )}^2}f=0$$

(3.6)

Lemma 4. Let $f\left(\lambda \right)$ be defined by

$$f\left(\lambda \right):={(1-\lambda )}^{1/4}{}_2{F}_1\left[\begin{array}{cc}\frac{1}{4}& \frac{1}{4}\\ & 1\end{array};\lambda \right]={}_2{F}_1\left[\begin{array}{cc}\frac{1}{4}& \frac{3}{4}\\ & 1\end{array};\frac{\lambda }{\lambda -1}\right]byEquation\left(3.1\right).$$

Then up to constant multiples, $f\left(\lambda \right)$ is the unique solution of $P{F}_{\lambda }$ that is holomorphic near $\lambda =0$.

Proof. Write

$$a\left(\lambda \right):={}_2{F}_1\left[\begin{array}{cc}\frac{1}{4}& \frac{1}{4}\\ & 1\end{array};\lambda \right]=\sum _{k=0}^{\infty }\frac{{\left(\frac{1}{4}\right)}_k^2}{k{!}^2}{\lambda }^k=\sum _{k=0}^{\infty }{a}_k{\lambda }^k$$

so that $f\left(\lambda \right)={(1-\lambda )}^{1/4}a\left(\lambda \right)$. Then the coefficients $a_k$ satisfy

$$a_{k+1}{(k+1)}^2=a_k{(k+1/4)}^2$$

Using the product rule, we see that

$$\begin{array}{c}\lambda {(1-\lambda )}^2\frac{d^2}{d{\lambda }^2}f+{(1-\lambda )}^2\frac{d}{d\lambda }f+\frac{3}{16}f=\\ ={(1-\lambda )}^{5/4}\left(\frac{-1}{16}a+\left(1-\frac{3}{2}\lambda \right)a^{\prime }+(\lambda -{\lambda }^2)a^{\prime \prime }\right)\hfill \\ ={(1-\lambda )}^{5/4}\sum _{k=0}^{\infty }\left(-\frac{1}{16}{a}_k+(k+1)a_{k+1}-\frac{3}{2}k{a}_k-k(k-1)a_k+k(k+1)a_{k+1}\right){\lambda }^k\hfill \\ ={(1-\lambda )}^{5/4}\sum _{k=0}^{\infty }\left({(k+1)}^2{a}_{k+1}-{(k+1/4)}^2{a}_k\right){\lambda }^k\hfill \\ =0\hfill \end{array}$$

              ☐

It is well-known that Picard–Fuchs equations arising from algebraic varieties are Fuchsian equations with only regular singularities. Also, attached to each differential equation with isolated singularities is the so-called monodromy representation of the fundamental group of the base curve with singularities removed [20]. The image of this representation, well-defined up to conjugation, is called the monodromy group of the differential equation. Shortly, we shall elaborate upon the monodromy group for $P{F}_{\lambda }$.

3.3. Modular Forms and Singular Values of Modular Forms

We gather some results regarding modular forms. Modular forms are functions, F, defined on the Poincaré upper half plane, $\mathcal{H}$, that satisfy the weight k modular invariance condition

$$F\left(\frac{az+b}{cz+d}\right)={(cz+d)}^{k}F\left(z\right)$$

for all $z\in \mathcal{H}$ and $\left(\begin{array}{cc}a& b\\ c& d\end{array}\right)$ in some subgroup $\Gamma \subseteq S{L}_2\left(\mathbb{Z}\right)$ of finite index. As well, they have moderate growth conditions at the cusps of Γ, which are equivalence classes of $\mathbb{Q}\cup \left\{\infty \right\}$ under the action of Γ. Here, for simplicity, we assume that k is an integer. Holomorphic integral weight modular forms for Γ form a finitely generated $\mathbb{C}-$algebra

$$M(\Gamma )=⨁M_k(\Gamma )$$

graded by the weight k. A modular form is said to be weakly holomorphic if it is holomorphic on $\mathcal{H}$ and is either holomorphic or meromorphic at the cusps. Two of the most well-known weakly holomorphic weight 0 modular forms are the modular $j-$function, for $\Gamma =S{L}_2\left(\mathbb{Z}\right)$, and the modular lambda function for the principal level$-2$ congruence subgroup $\Gamma \left(2\right)$, defined here by

$$L\left(z\right)=16\frac{\eta {\left(2z\right)}^4\eta {(z/2)}^2}{\eta {\left(z\right)}^6}$$

(3.7)

The function $\eta \left(z\right)$ is the classical eta-function

$$\eta \left(z\right)=q^{1/24}\prod _{n\ge 1}(1-q^n),q=e^{2\pi iz}$$

The modular $j-$function and lambda function are related by the identity

$$j=2^8\frac{{(L^2-L+1)}^3}{L^2{(L-1)}^2}=64\frac{{(2L(2L-2)+4)}^3}{{\left(2L(2L-2)\right)}^2}$$

(3.8)

Every meromorphic modular form F has a $q-$expansion at infinity

$$F\left(z\right)=\sum _{n=-n_0}^{\infty }{a}_n{q}^{n/\mu }$$

where again $q=e^{2\pi iz}$ and $\mu \in \mathbb{N}$ is called the cusp width of Γ at infinity. Recall the weight 2 quasi-modular Eisenstein series

$${\mathsf{E}}_2\left(z\right)=1-24\sum _{n\ge 1}\frac{n{q}^n}{1-q^n}$$

Then

$${\mathsf{E}}_2^{*}\left(z\right)={\mathsf{E}}_2\left(z\right)-\frac{3}{\pi \text{Im}\left(z\right)}$$

is a weight 2 non-holomorphic modular form. We state an essential fact which can be found in work of Zagier [21].

Proposition 5. For each imaginary quadratic field K, there is a number ${\Omega }_K\in {\mathbb{C}}^{*}$ such that for all meromorphic modular forms F of weight k with algebraic coefficients, and all $\tau \in K\cap \mathcal{H}$,

$$F\left(\tau \right)·{\Omega }_K^{-k}\in \overline{\mathbb{Q}}$$

Moveover, ${\mathsf{E}}_2^{*}\left(\tau \right){\Omega }_K^{-2}\in \overline{\mathbb{Q}}$

In a manner similar to Zagier, we define the derivatives ${\vartheta }_k$ and ${\partial }_k$ [21]. For a weight k modular form F with algebraic coefficients, let

$${\vartheta }_{k}F\left(z\right):=F^{\prime }\left(z\right)-\frac{k}{12}{\mathsf{E}}_2\left(z\right)F\left(z\right),{\partial }_{k}F\left(z\right):=F^{\prime }\left(z\right)-\frac{k}{4\pi \text{Im}\left(z\right)}F\left(z\right)$$

interpreting $F^{\prime }\left(z\right)$ as $\frac{dF}{dq}=\frac{1}{2\pi i}\frac{\partial F}{\partial z}$. Then ${\vartheta }_{k}F$ is weight $k+2$ modular form with algebraic coefficients and

$${\vartheta }_{k}F\left(z\right)={\partial }_{k}F\left(z\right)-\frac{k}{12}{\mathsf{E}}_2^{*}\left(z\right)F\left(z\right)$$

By Proposition 5, the values

$${\vartheta }_{k}F\left(\tau \right){\Omega }_K^{-k-2}\in \overline{\mathbb{Q}},\text{ and }{\partial }_{k}F\left(\tau \right){\Omega }_K^{-k-2}\in \overline{\mathbb{Q}}$$

for all $\tau \in K\cap \mathcal{H}.$ For future reference we state this as a lemma.

Lemma 6. Let K be an imaginary quadratic field and F a weight k meromorphic modular form with algebraic coefficients. Then there is a number ${\Omega }_K\in {\mathbb{C}}^{*}$, depending on K, such that for any $\tau \in K\cap \mathcal{H}$, $F\left(\tau \right){\Omega }_K^{-k}$ and ${\partial }_{k}F\left(\tau \right){\Omega }_K^{-k-2}$ are algebraic.

Remark 4. In our setting, we assume $E_{\lambda }$ has complex multiplication. Then R, the endomorphism ring of $E_{\lambda }$, is an order of an imaginary quadratic field K. In this case, there are two cycles $C_1,C_2$ in $H_1(E_{\lambda },\mathbb{Z})$ such that

$$\frac{{\int }_{C_1}{\omega }_{\lambda }}{{\int }_{C_2}{\omega }_{\lambda }}=\tau \in K\cap \mathcal{H}$$

It is known that hypergeometric series can be related to theta series [3]; for instance

$${}_2{F}_1\left[\begin{array}{cc}\frac{1}{2}& \frac{1}{2}\\ & 1\end{array};L\left(z\right)\right]={\theta }_3^2\left(z\right)$$

(3.9)

where ${\theta }_3\left(z\right)={\sum }_{n\in \mathbb{Z}}{q}^{n^2/2}$ is a weight $1/2$ modular form, and the modular Lambda function $L\left(z\right)$ is as in Equation (3.7). In fact this is a special case of a general result of Stiller [22] regarding solutions of suitable degree 2 ordinary Fuchsian differential equations, which are weight 1 modular forms of the corresponding monodromy groups.

Lemma 7. The monodromy group Γ for the Picard–Fuchs equation $P{F}_{\lambda }$ from Equation (3.6) is isomorphic to a level 2 subgroup of $S{L}_2\left(\mathbb{Z}\right)$.

Proof. Recall that the $j-$invariant of the elliptic curve $E_{\lambda }$ is

$$j\left(E_{\lambda }\right)=64\frac{{(4-\lambda )}^3}{{\lambda }^2}$$

By Equation (3.8), we see that λ can be viewed as a degree 2 polynomial in L, i.e.,

$$\lambda \left(z\right)=-2L\left(z\right)(2L\left(z\right)-2)=-4L{\left(z\right)}^2+4L\left(z\right)$$

Hence $\lambda \left(z\right)$ is a modular function over $\Gamma \left(2\right)$ with algebraic coefficients.

Inserting $\lambda :=-2L(2L-2)$ as a function of $q=e^{2\pi iz}$ and using Equations (3.3) and (3.9), we can make the following statement.

Lemma 8. Both

$${}_2{F}_1\left[\begin{array}{cc}\frac{1}{4}& \frac{1}{4}\\ & 1\end{array};\lambda \left(z\right)\right]={\theta }_3^2\left(z\right)and{(1-\lambda \left(z\right))}^{1/4}{}_2{F}_1\left[\begin{array}{cc}\frac{1}{4}& \frac{1}{4}\\ & 1\end{array};\lambda \left(z\right)\right]$$

are weight 1 modular forms with algebraic Fourier coefficients.

3.4. Formulas for $1/\pi$ in Terms of Periods

We now return to the method for obtaining Ramanujan type formulas for expansions of $1/\pi$. Let E be an elliptic curve defined over $\overline{\mathbb{Q}}$ with complex multiplication, R the endomorphism ring of E over $\mathbb{C}$ which is assumed to be an order of the imaginary quadratic field $K=\mathbb{Q}\left(\sqrt{-d}\right)$ of discriminant $-d$, and ω its invariant differential defined over $\overline{\mathbb{Q}}$. Then Chowla and Selberg [14] (see also the work of Gross [23]) proved that for any $C\in H_1(E,\mathbb{Z})$, the transcendental part of the period integral ${\int }_C\omega$ is as follows:

$$b_K:=\sqrt{\pi }\prod _{0<a<d}\Gamma {(a/d)}^{n\epsilon \left(a\right)/4h}$$

where n is the order of unit group in $\mathbb{Q}\left(\sqrt{-d}\right)$, ε is a primitive Dirichlet character modulo d for the quadratic field $\mathbb{Q}\left(\sqrt{-d}\right)$, and h is the class number of $\mathbb{Q}\left(\sqrt{-d}\right)$. The well-known Gross–Koblitz formula is the $p-$adic analogue of the Chowla–Selberg formula. Moreover, consider ω as an element of $H_{DR}^1(E,\mathbb{C})$, the dual of $H_1(E,\mathbb{Z}){\otimes }_{\mathbb{Z}}\mathbb{C}$. The $2-$dimensional space $H_{DR}^1(E,\mathbb{C})$ is endowed with the action of the endomorphism ring R. In particular, ω is an eigenfunction of R. There is another eigenfunction ν of R in $H_{DR}^1\left(E\right)$ that is not parallel to ω. Then ${\int }_C\nu$ is an algebraic multiple of $2\pi i/b_K$. Putting this together, one obtains the relation

$${\int }_C\omega ·{\int }_C\nu \sim \pi$$

(3.10)

where ∼ denotes equality up to multiplication by an algebraic number.

We will now to return to our discussion of $E_{\lambda }$ by relating the corresponding hypergeometric series to the Chowla–Selberg formulas. Consider $E_{\lambda }$ now as an elliptic curve defined over $\mathbb{C}\left(\lambda \right)$. Recall that

$${\omega }_{\lambda }=\frac{dx}{y}=\frac{dx}{\sqrt{(x-1)(x^2-\frac{1}{1-\lambda })}}$$

is the holomorphic differential $1-$form on $E_{\lambda }$, which is unique up to scalars. Let $C_{\lambda }$ be a family of $1-$cycles on $E_{\lambda }$ that moves complex analytically as λ varies. Then

$$p\left(\lambda \right):={\int }_{C_{\lambda }}{\omega }_{\lambda }$$

describes the variation of the periods. Since applying the Picard–Fuchs differential equation to ${\omega }_{\lambda }$ yields an exact form $d{g}_{\lambda }$,

$$P{F}_{\lambda }\circ p\left(\lambda \right)={\int }_{C_{\lambda }}d{g}_{\lambda }=0$$

by Green’s theorem. As $p\left(\lambda \right)$ is a holomorphic solution to $P{F}_{\lambda }$ near 0,

$$p\left(\lambda \right)=A·f\left(\lambda \right)$$

(3.11)

for some constant term A, and f as defined in Lemma 4,

$$f_{\lambda }\left(z\right):=f\left(\lambda \left(z\right)\right)={(1-\lambda )}^{1/4}{}_2{F}_1\left[\begin{array}{cc}\frac{1}{4}& \frac{1}{4}\\ & 1\end{array};\lambda \left(z\right)\right]$$

Furthermore, f can be viewed as a weight 1 modular form with respect to the variable $z\in \mathcal{H}$ by Lemma 8. Note that since λ has algebraic coefficients with respect to $q^{1/2}=e^{\pi iz}$, as remarked in the proof of Lemma 7, so does $f_{\lambda }\left(z\right)$. To write down the corresponding ${\nu }_{\lambda }$, which is also an eigenfunction of R, we use the fact that $H_{DR}^1(E_{\lambda }/\mathbb{C}\left[\left[\lambda \right]\right])$ admits the Gauss–Manin connection, so that

$${\partial }_{\lambda }{\omega }_{\lambda }\in H_{DR}^1(E_{\lambda }/\mathbb{C}\left[\left[\lambda \right]\right])$$

Thus ${\nu }_{\lambda }$ can be written as the form $\frac{d{f}_{\lambda }}{d\lambda }-c{f}_{\lambda }$ for some constant c. In terms of modular forms, Lemma 6 ensures that both

$$\mu :={\partial }_1\left(f_{\lambda }\right)\left(\tau \right){\Omega }_K^{-3}\in \overline{\mathbb{Q}},\text{ and }w:=f_{\lambda }\left(\tau \right){\Omega }_K^{-1}\in \overline{\mathbb{Q}}$$

Lemma 9. Let $\tau \in K$ be such that $w:=f_{\lambda }\left(\tau \right){\Omega }_K^{-1}\ne 0$. Then there is a unique algebraic number $c^{\prime }$ such that

$$f_{\lambda }\left(\tau \right)\left(\frac{d{f}_{\lambda }}{d\lambda }-c^{\prime }{f}_{\lambda }\right)\left(\tau \right)\sim 1/\pi$$

Proof. We have that

$$\frac{d{f}_{\lambda }}{d\lambda }=\frac{d{f}_{\lambda }}{dq}\frac{dq}{d\lambda }\left(\tau \right)=\left({\partial }_1{f}_{\lambda }\left(\tau \right)+\frac{1}{4\pi \text{Im}\left(\tau \right)}{f}_{\lambda }\left(\tau \right)\right)\frac{dq}{d\lambda }$$

and $\frac{dq}{d\lambda }={\left({\partial }_0\lambda \right)}^{-1}$ is a modular form of weight $-2$. By Proposition 5 there is some $\xi \in \overline{\mathbb{Q}}$ such that $\frac{dq}{d\lambda }\left(\tau \right){\Omega }_K^2=\xi$. Put $c^{\prime }:=\mu \xi /w$; then

$$f_{\lambda }\left(\tau \right)\left(\frac{d{f}_{\lambda }}{d\lambda }-c^{\prime }{f}_{\lambda }\right)\left(\tau \right)={\Omega }_{K}w\left({\Omega }_K\mu \xi +\frac{1}{4\pi \text{Im}\left(\tau \right)}{\Omega }_K^{-1}w\xi -\frac{\mu \xi }{w}{\Omega }_{K}w\right)=\frac{w^2\xi }{4\pi \text{Im}\left(\tau \right)}\sim 1/\pi$$

(3.12)

              ☐

Combining Equations (3.11) and (3.12) with the results of Chowla–Selberg, we obtain the following corollary.

Corollary 10. The function $p\left(\lambda \right)$ describing the variation of the period integrals is equal to the product of π with the solution to the Picard–Fuchs equation, up to multiplication by an algebraic number;

$$p\left(\lambda \right)\sim \pi f_{\lambda }$$

Remark 5. Recall, ${\nu }_{\lambda }$ can be written as the form $\frac{d{f}_{\lambda }}{d\lambda }-c{f}_{\lambda }$ for some constant c. In Corollary 10, $c=\mu \xi /w$, with $\mu ,\xi ,w$ as in Lemma 9.

Remark 6. If τ is an imaginary quadratic number, then $\lambda \left(\tau \right)$ is algebraic, so is ${(1-\lambda \left(\tau \right))}^{1/4}$. That is, one can draw a similar conclusion for the series ${\tilde{f}}_{\lambda }\left(\tau \right)={}_2{F}_1\left[\begin{array}{cc}1/4& 1/4\\ & 1\end{array};\lambda \left(\tau \right)\right]$. Hence, there is an algebraic constant C such that

$${\tilde{f}}_{\lambda }\left(\tau \right)·\left(\frac{d{\tilde{f}}_{\lambda }}{d\lambda }-C{\tilde{f}}_{\lambda }\left(\tau \right)\right)\sim \frac{1}{\pi }$$

Proposition 11 (Borweins and Chudnovskys [3,4]) There exists $a\in \overline{\mathbb{Q}}$ such that

$$\sum _{k=0}^{\infty }\frac{{\left(\frac{1}{2}\right)}_k^3}{k{!}^3}(ak+1){\left(\lambda \right)}^k\sim \frac{1}{\pi }$$

As in Remark 6, one can take $a=-1/2C$. In fact, it follows from Clausen’s formula (3.4) and a normalization that the left hand side has constant term 1.

Remark 7. Using the same technique, one can obtain Ramanujan type formulas for $1/\pi$ corresponding to other series with $d=3,4,6$ as mentioned in the introduction. In fact, the Picard–Fuchs equation of each family of elliptic curves ${\tilde{E}}_d\left(t\right)$ has local solutions ${}_2{F}_1\left[\begin{array}{cc}1/d& (d-1)/d\\ & 1\end{array};t\right]$ near $t=0$ with $d=2,3,4,6.$ The case of $d=2$ is well-known. The other cases can be verified by using the results of Stienstra and Beukers [24]. In the literature, one can find families of elliptic curves ${\tilde{E}}_d\left(t\right)$ in weighted projective space whose Picard–Fuchs equation has ${}_2{F}_1\left[\begin{array}{cc}1/d& (d-1)/d\\ & 1\end{array};t\right]$ as local holomorphic solutions at 0 [25]. (Note that these equations are in terms of $t^{1/d}$ and not t for $d=2,3,4,6.$) The corresponding monodromy groups of the Picard–Fuchs equations are four genus zero hyperbolic triangular groups:

$${\Gamma }_0^{*}\left(4\right)\cong \Gamma \left(2\right),{\Gamma }_0^{*}\left(3\right),{\Gamma }_0^{*}\left(2\right),S{L}_2\left(\mathbb{Z}\right)$$

respectively [26]. Here ${\Gamma }_0^{*}\left(n\right)$ is the group generated by the congruence subgroup ${\Gamma }_0\left(n\right)$ consisting of elements in $S{L}_2\left(\mathbb{Z}\right)$ that are upper triangular modulo n, and the Fricke involution $W_n=\left(\begin{array}{cc}0& -1\\ n& 0\end{array}\right)$. Similar to Lemma 8, inserting t with suitable modular functions of the corresponding genus zero groups, one obtains explicit weight 1 modular forms.

4. The Arithmetic of the Elliptic Curves

4.1. Expansions of the Invariant Differentials at Infinity

Motivated by the Hypergeometric Transformation Formula Stated in Lemma 3, we fix a choice of square root $\sqrt{1-{\lambda }_d}$ for $d=2,3,4,6$ and let $E_d\left({\lambda }_d\right)={\tilde{E}}_d\left(\frac{1-\sqrt{1-{\lambda }_d}}{2}\right),$ as in Section 2. We will show that the choice of square root does not matter in this setting.

Lemma 12. Let $p\ge 5$ be a prime. For each family of curves ${\tilde{E}}_d\left(t\right):y^2+Ay=B,$ listed in the introduction, with $A=a_{1}x+a_3$, $B=x^3+a_2{x}^2+a_{4}x+a_6$, let $z=\frac{-x}{y}$ be the local uniformizer. Expand

$$\frac{dx}{2y+A}=H\left(z\right)dz=\sum _{n\ge 1}{H}_{d,n-1}\left(t\right)z^{n-1}dz$$

where $H_{d,n-1}\left(t\right)$ denotes the corresponding coefficient of $z^{n-1}$ as a function of t. Then

$$H_{d,p-1}\left(t\right)\equiv {}_2{F}_1{\left[\begin{array}{cc}\frac{1}{d}& \frac{d-1}{d}\\ & 1\end{array};t\right]}_{p-1}(modp)$$

Proof. By Equation (1.2) in the work of Ditters [27], $H_{d,n-1}\left(t\right)$ is the coefficient of $x^{n-1}$ in the polynomial

$${\displaystyle \sum _{\begin{array}{c}s+j=n-1\\ j\le s\end{array}}\left(\genfrac{}{}{0pt}{}{s}{j}\right)B^j{A}^{s-j}}$$

• For $d=2$: $A=0$, $B=x(x-1)(x-t)$.

  $$\begin{array}{ccc}\hfill H_{2,p-1}\left(t\right)& =& {}_2{F}_1\left[\begin{array}{cc}\frac{1-p}{2}& \frac{1-p}{2}\\ & 1\end{array};t\right]\hfill \\ & \equiv & {}_2{F}_1{\left[\begin{array}{cc}\frac{1}{2}& \frac{1}{2}\\ & 1\end{array};t\right]}_{(p-1)}(modp)\hfill \end{array}$$
• For $d=3$: $A=x+\frac{t}{27}$, $B=x^3$. $H_{3,p-1}\left(t\right)$ is the coefficient of $x^{p-1}$ in

  $${\displaystyle \sum _{\begin{array}{c}s+j=p-1\\ j\le s\end{array}}\left(\genfrac{}{}{0pt}{}{s}{j}\right)B^j{A}^{s-j}}$$
  By a similar argument, we obtain

  $$\begin{array}{ccc}\hfill H_{3,p-1}\left(t\right)& =& \sum _{j=0}^{\frac{p-1}{3}}\left(\genfrac{}{}{0pt}{}{p-1-j}{j}\right)\left(\genfrac{}{}{0pt}{}{p-1-2j}{p-1-3j}\right){\left(\frac{t}{27}\right)}^j\hfill \\ & =& {}_3{F}_2\left[\begin{array}{ccc}\frac{1-p}{3}& \frac{2-p}{3}& \frac{3-p}{3}\\ & 1& 1-p\end{array};t\right]\hfill \\ & \equiv & {}_2{F}_1{\left[\begin{array}{cc}\frac{1}{3}& \frac{2}{3}\\ & 1\end{array};t\right]}_{(p-1)}(modp)\hfill \end{array}$$
• For $d=4$: $A=0$, $B=x(x^2+x+\frac{t}{4})$.

  $$\begin{array}{ccc}\hfill H_{4,p-1}& =& \sum _{i=0}^{\frac{p-1}{4}}\left(\genfrac{}{}{0pt}{}{\frac{p-1}{2}}{j,j,\frac{p-1}{2}-2j}\right){\left(\frac{t}{4}\right)}^j\hfill \\ & =& {}_2{F}_1{\left[\begin{array}{cc}\frac{1-p}{4}& \frac{3-p}{4}\\ & 1\end{array};t\right]}_{(p-1)}(modp)\hfill \end{array}$$
• For $d=6$: $A=x$, $B=x^3-\frac{t}{432}$.

  $$\begin{array}{ccc}\hfill H_{6,p-1}\left(t\right)& \equiv & \sum _{j=0}^{\frac{p-1}{6}}\left(\genfrac{}{}{0pt}{}{p-1-3j}{3j}\right)\left(\genfrac{}{}{0pt}{}{3j}{j}\right){\left(\frac{-t}{432}\right)}^j\hfill \\ & \equiv & {}_6{F}_5\left[\begin{array}{cccccc}\frac{1-p}{6}& \frac{2-p}{6}& \frac{3-p}{6}& \frac{4-p}{6}& \frac{5-p}{6}& \frac{6-p}{6}\\ & \frac{1-p}{3}& \frac{2-p}{3}& \frac{3-p}{3}& 1& \frac{1}{2}\end{array};t\right]\hfill \\ & \equiv & {}_2{F}_1{\left[\begin{array}{cc}\frac{1}{6}& \frac{5}{6}\\ & 1\end{array};t\right]}_{(p-1)}(modp)\hfill \end{array}$$

              ☐

We observe that the congruences above hold for any $t\in {\mathbb{F}}_p$, and for the four values of d in consideration and for $t=\frac{1-\sqrt{1-{\lambda }_d}}{2},$ the coefficient $H_{d,p-1}\left(t\right)$ can be stated explicitly.

Lemma 13. For $d=2,3,4,6$, and $p\ge 5$ prime,

$$\begin{array}{c}{H}_{d,p-1}\left(\frac{1-\sqrt{1-{\lambda }_d}}{2}\right)\hfill \\ \hfill \equiv \left\{\begin{array}{cc}{}_2{F}_1{\left[\begin{array}{c}\frac{1}{2d}\frac{1}{2}-\frac{1}{2d}\\ 1\end{array};{\lambda }_d\right]}_{p-1}(modp),\hfill & ifp\equiv 1,d-1(mod2d),\hfill \\ {(1-{\lambda }_d)}^{1/2}{}_2{F}_1{\left[\begin{array}{c}\frac{1}{2}+\frac{1}{2d}1-\frac{1}{2d}\\ 1\end{array};{\lambda }_d\right]}_{p-1}(modp),\hfill & otherwise.\hfill \end{array}\right.\end{array}$$

Proof. Let $P_n\left(x\right):={}_2{F}_1\left[\begin{array}{cc}-n& n+1\\ & 1\end{array};\frac{1-x}{2}\right]$ be the nth Legendre polynomial, following the notation given by Andrews, Askey, and Roy [19]. $P_n\left(x\right)$ is an odd (resp. even) function of x if n is odd (resp. even). By Lemma 12,

$$H_{d,p-1}\left(\frac{1-\sqrt{1-{\lambda }_d}}{2}\right)\equiv P_n\left(\sqrt{1-{\lambda }_d}\right)(modp)$$

where $n=\frac{p-1}{d}$ if $p\equiv 1(modd)$, and $n=\frac{p+1-d}{d}$ if $p\equiv -1(modd)$. If n is even, i.e., when $p\equiv 1$, or $p\equiv d-1(mod2d)$, $P_n\left(\sqrt{1-{\lambda }_d}\right)$ is a polynomial in $(1-{\lambda }_d)$, which is congruent modulo p to ${}_2{F}_1{\left[\begin{array}{cc}1/2d& 1/2-1/2d\\ & 1\end{array};{\lambda }_d\right]}_{p-1}$ from Lemma 3. When n is odd, $P_n\left(\sqrt{1-{\lambda }_d}\right)$ is $\sqrt{1-{\lambda }_d}$ times a polynomial in $(1-{\lambda }_d)$. Then the claim follows from Lemma 3 and the following transformation of Euler [19],

$${}_2{F}_1{\left[\begin{array}{cc}\frac{1}{2}-\frac{a}{2}& \frac{a}{2}\\ & 1\end{array};{\lambda }_d\right]}_{p-1}={(1-{\lambda }_d)}^{1/2}{}_2{F}_1{\left[\begin{array}{cc}\frac{1}{2}+\frac{a}{2}& 1-\frac{a}{2}\\ & 1\end{array};{\lambda }_d\right]}_{p-1}$$

              ☐

Similarly, squares of $H_{d,p-1}\left(t\right),$ in this setting, can be described precisely using Equation (3.5).

Corollary 14. For $d=2,3,4,6$, and $p\ge 5$ prime,

$$H_{d,p-1}{\left(\frac{1-\sqrt{1-{\lambda }_d}}{2}\right)}^2\equiv {}_3{F}_2{\left[\begin{array}{ccc}\frac{1}{2}& \frac{1}{d}& \frac{d-1}{d}\\ & 1& 1\end{array};{\lambda }_d\right]}_{p-1}(modp)$$

4.2. Geometric Interpretation of Lemma 13

For $d=2$, it is straightforward to check that the $j-$invariant of $E_2\left({\lambda }_2\right)$ is in $\mathbb{Q}\left({\lambda }_2\right)$. Consequently, $E_2\left({\lambda }_2\right)$ has a model defined over $\mathbb{Q}\left({\lambda }_2\right)$. To be more explicit, rewrite $E_2\left({\lambda }_2\right)$ in the form

$$y^2=x^3-27(16-{\lambda }_2)x-432({\lambda }_2+8)\sqrt{1-{\lambda }_2}$$

Applying the quartic twist $(x,y)\mapsto (\sqrt{1-{\lambda }_2}X,\sqrt[4]{1-{\lambda }_2}Y)$, gives the equation

$${\widehat{E}}_2\left({\lambda }_2\right):Y^2=(1-{\lambda }_2)X^3-27(16-{\lambda }_2)X-432({\lambda }_2+8)$$

defined over $\mathbb{Q}\left({\lambda }_2\right)$, which is isomorphic to $E_{{\lambda }_2}:Y^2=(X-1)(X^2-\frac{1}{1-{\lambda }_2})$ over $\mathbb{Q}\left({\lambda }_2\right)$.

Similarly for $d=6$, $E_6\left({\lambda }_6\right)$ is isomorphic over $\mathbb{Q}\left(\sqrt{1-{\lambda }_6}\right)$ to

$$y^2=x^3-27x+54\sqrt{1-{\lambda }_6}$$

Applying $(x,y)\mapsto (\sqrt{1-{\lambda }_4}X,\sqrt[4]{1-{\lambda }_4}Y)$, yields the twisted curve

$${\widehat{E}}_6\left({\lambda }_6\right):Y^2=(1-{\lambda }_6)X^3-27X+54$$

In the cases $d=2,6$,

$$\frac{-x}{y}=\frac{-X}{Y}\sqrt[4]{1-{\lambda }_d}$$

When $d=3$, $E_d\left({\lambda }_d\right)$ has no model over $\mathbb{Q}\left({\lambda }_d\right)$. However, rewrite $E_3\left({\lambda }_3\right)$ as

$$y^2=x^3+{\left(\frac{x}{2}+\frac{1-\sqrt{1-{\lambda }_3}}{108}\right)}^2$$

and apply a quartic twist $(x,y)\mapsto ({\alpha }^{2}X,{\alpha }^{3}Y)$ to $E_3\left({\lambda }_3\right)$ with $\alpha =\sqrt[4]{1-{\lambda }_3}$ to get

$$Y^2=X^3+\frac{1}{4{\alpha }^2}{\left(X+\frac{1-\sqrt{1-{\lambda }_3}}{54{\alpha }^2}\right)}^2$$

The curve is $3-$isogenous over $\mathbb{Q}(\sqrt{1-{\lambda }_3},\sqrt{3})$ to

$${\widehat{E}}_3\left({\lambda }_3\right):Y^2=X^3-\frac{27}{4c^2}{\left(X+\frac{-1-\sqrt{1-{\lambda }_3}}{2{\alpha }^2}\right)}^2$$

(see work of Top [28]), which is isomorphic to its conjugate under a different embedding of $\sqrt{1-{\lambda }_3}$.

Similarly, when $d=4$, $E_d\left({\lambda }_d\right)$ is $2-$isogenous to $E_d^{\prime }\left({\lambda }_d\right)={\tilde{E}}_d\left(\frac{1+\sqrt{1-{\lambda }_d}}{2}\right)$. To see this, we apply a similar quartic twist to get

$${\widehat{E}}_4\left({\lambda }_4\right):Y^2=X\left(X^2+\frac{1}{{\alpha }^2}X+\frac{1-\sqrt{1-{\lambda }_4}}{8{\alpha }^4}\right)$$

which is $2-$isogenous over $\mathbb{Q}(\sqrt{1-{\lambda }_4},\sqrt{2})$ to

$${\widehat{E}}_4^{\prime }\left({\lambda }_4\right):Y^2=X\left(X^2-\frac{2}{{\alpha }^2}X+\frac{1+\sqrt{1-{\lambda }_4}}{2{\alpha }^4}\right)$$

The curve is isomorphic over $\mathbb{Q}(\sqrt{1-{\lambda }_4},\sqrt{2})$ to ${\widehat{E}}_4\left({\lambda }_4\right)$ under a different embedding of $\sqrt{1-{\lambda }_4}$.

In the cases $d=3,4$,

$$\frac{-x}{y}=\frac{-X}{Y}\frac{1}{\sqrt[4]{1-{\lambda }_d}}$$

4.3. Interpretation in Terms of Galois Representations

For any number field F, we use $G_F$ to denote $\text{Gal}(\overline{F}/F)$. Let ℓ be an arbitrary prime number and ${\widehat{\rho }}_{d,\ell }$ the Galois representations of $G_K$ attached to Tate modules of ${\widehat{E}}_d\left({\lambda }_d\right)$. We show that for each $d=2,3,4,6$ there is an associated Galois representation $\tilde{\rho }$ that is odd.

When $d=2,6$, $K=\mathbb{Q}\left({\lambda }_d\right)$, which is assumed to be totally real. Recall that for any conjugacy class c of complex conjugation in $G_{\mathbb{Q}\left({\lambda }_d\right)}$, ${\widehat{\rho }}_{d,\ell }\left(c\right)$ is not a scalar matrix, as ${\widehat{E}}_d\left({\lambda }_d\right)$ has CM, and the Galois representation ${\widehat{\rho }}_{d,\ell }$ is odd. In this setting, we write $\tilde{\rho }={\widehat{\rho }}_{d,\ell }$.

When $d=3,4$, $K=\mathbb{Q}\left(\sqrt{1-{\lambda }_d}\right)$. If $K=\mathbb{Q}\left({\lambda }_d\right)$, as in the setting above, we consider $\tilde{\rho }={\widehat{\rho }}_{d,\ell }$. Suppose $K\ne \mathbb{Q}\left({\lambda }_d\right)$. Let σ be any element in $G_K$ which sends $\sqrt{1-{\lambda }_d}$ to $-\sqrt{1-{\lambda }_d}$. The above discussion implies that

$$\psi :={\widehat{\rho }}_{d,\ell }{|}_{G_{\mathbb{Q}(\sqrt{1-{\lambda }_d},\sqrt{s_d})}}$$

is isomorphic to ${\psi }^{\sigma }$, its conjugation by σ, where $s_3=3,s_4=2$. As ψ is obtained as a restriction, ψ is indeed isomorphic to its conjugate by any element in $\text{Gal}(\mathbb{Q}(\sqrt{1-{\lambda }_d},\sqrt{s_d})/\mathbb{Q}\left({\lambda }_d\right))$. This implies that if ψ is absolutely irreducible, then it can be lifted to a $2-$dimensional odd representation $\phi$ of $G_{\mathbb{Q}\left({\lambda }_d\right)}$, which is well-defined up to a character of $\text{Gal}(\mathbb{Q}(\sqrt{1-{\lambda }_d},\sqrt{s_d})/\mathbb{Q}\left({\lambda }_d\right))$. Otherwise, $\psi ={\chi }_1\oplus {\chi }_2$ for some $1-$dimensional characters ${\chi }_i$ of $G_{\mathbb{Q}(\sqrt{1-{\lambda }_d},\sqrt{s_d})}$ for $i=1,2$. As ψ is isomorphic to ${\psi }^{\sigma }$, either ${\chi }_1^{\sigma }\cong {\chi }_1$, which implies ${\chi }_1$ can be lifted to a $1-$dimensional character of $G_{\mathbb{Q}({\lambda }_d,\sqrt{1-{\lambda }_d})}$, contradicting the fact that ${\chi }_1$ originated from a CM elliptic curve, or ${\chi }_2\cong {\chi }_1^{\sigma }$, which implies that

$$\phi ={\text{Ind}}_{G_{\mathbb{Q}(\sqrt{1-{\lambda }_d},\sqrt{s_d})}}^{G_{\mathbb{Q}({\lambda }_d,\sqrt{s_d})}}{\chi }_1$$

is absolutely irreducible. The induced character $\phi$ can be extended to a $2-$dimensional Galois representation $\tilde{\rho }$ of $G_{\mathbb{Q}\left({\lambda }_d\right)}$, well-defined up to a finite character of $\text{Gal}(\mathbb{Q}(\sqrt{1-{\lambda }_d},\sqrt{s_d})/\mathbb{Q}\left({\lambda }_d\right))$.

In either case, when $K\ne \mathbb{Q}\left({\lambda }_d\right)$ or $K=\mathbb{Q}\left({\lambda }_d\right)$, the representation $\tilde{\rho }$ is odd, which can be seen from the fact that it corresponds to an automorphic representation of $G{L}_2\left(\mathbb{Q}\left({\lambda }_d\right)\right)$ due to our CM assumption. For any prime p such that ${\lambda }_d$ embeds in ${\mathbb{Q}}_p$, fixing an embedding of ${\lambda }_d$ into ${\mathbb{Q}}_p$ corresponds to fixing a place v of ${\mathcal{O}}_{\mathbb{Q}\left({\lambda }_d\right)}$ above p with relative degree 1. Let ${\text{Fr}}_v$ be the corresponding geometric Frobenius conjugacy class in $G_{\mathbb{Q}\left({\lambda }_d\right)}$. Then by the oddness of $\tilde{\rho }$, we have the following.

Lemma 15. For $\tilde{\rho }$ defined above, the determinant of $\tilde{\rho }\left({\mathrm{Fr}}_v\right)$ is p and its trace is determined up to a ± sign.

5. Proof of Theorems 1 and 2

Results of Atkin, Swinnerton-Dyer and Katz 

Atkin and Swinnerton-Dyer [29] proved the following. Let $E:y^2=x^3-Bx-C$ be a nonsingular elliptic curve over a totally real field. Let $p>3$ be any prime such that $B,C$ can be embedded in ${\mathbb{Z}}_p$ and E has good reduction modulo $p.$ Let ξ be any local uniformizer of E at infinity over ${\mathbb{Z}}_p$ that is a formal power series of $\frac{-x}{y}$ with coefficients in ${\mathbb{Z}}_p$ that starts with $\frac{-x}{y}$. Then the holomorphic differential

$$-\frac{dx}{2y}=(1+{\sum }_{n\ge 1}a\left(n\right){\xi }^n)d\xi$$

has coefficients in ${\mathbb{Z}}_p.$ Moreover, for the coefficient $a\left(n\right)$ of the differential, the following congruence holds for all $n\ge 1$,

$$a(np-1)-[p+1-\#(E/{\mathbb{F}}_p)]·a(n-1)+p·a(n/p-1)\equiv 0(mod{p}^{1+{\mathrm{o}rd}_{p}n})$$

where we define $a\left(n\right)=0$ when n is not a positive integer. Note that the assumption of E being defined over a totally real field is necessary to conclude that the last coefficient is p. Otherwise, it is only determined up to a ± sign. Atkin and Swinnerton-Dyer’s result inspired the work of Cartier [30] and Katz [31]. Here we shall use Katz’s approach with a slight generalization using the approach outlined by Stienstra and Beukers [24] so that the base ring is not restricted to ${\mathbb{Z}}_p$.

Throughout this section, we assume that E is an elliptic curve defined over a number field $\mathbb{Q}\left(\sqrt{1-{\lambda }_d}\right)$ by a cubic equation in terms of x and y with identity $\underline{O}$. We further assume that E has CM by an order of an imaginary quadratic field $\mathbb{Q}\left(\tau \right)$. The endomorphism ring of $E/\mathbb{C}$ is denoted by R.

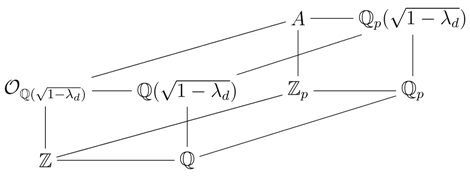
Choose some prime p such that ${\lambda }_d$ can be embedded into ${\mathbb{Z}}_p$ and such that p is unramified in $\mathbb{Q}\left(\sqrt{1-{\lambda }_d}\right)$. We extend the $p-$adic valuation of ${\mathbb{Q}}_p$ to ${\mathbb{Q}}_p\left(\sqrt{1-{\lambda }_d}\right)$ so that $\left|p\right|=1/p$ and let $A=\{x\in {\mathbb{Q}}_p\left(\sqrt{1-{\lambda }_d}\right):|x|\le 1\}$. The ring A is a local ring with maximal ideal $\left(p\right)=pA$. This information can be organized in the following diagram.

Note that A is the ring of Witt vectors of $k={\mathbb{F}}_p\left(\sqrt{1-{\lambda }_d}\right)$. Let σ be the nontrivial automorphism on $A=W\left({\mathbb{F}}_p\left(\sqrt{1-{\lambda }_d}\right)\right)$ lifted from the Frobenius automorphism $z\mapsto z^p$ on ${\mathbb{F}}_p$. It satisfies the relation $\sigma \left(a\right)\equiv a^p(modpA)$ for all $a\in A$. If $A={\mathbb{Z}}_p$, σ is simply the identity map on ${\mathbb{Z}}_p$. Otherwise, σ is conjugation, mapping $\sqrt{1-{\lambda }_d}$ to $-\sqrt{1-{\lambda }_d}$. We further assume that E has a model over A and has good reduction modulo $pA$. From Katz we know the de Rham cohomology space $H_{DR}^1(E/A)$ is isomorphic to

$$\frac{\{f\in {\mathbb{Q}}_p\left[\sqrt{1-\lambda }\right]\left[[x,y]\right]:f\left(\underline{O}\right)=0,df\mathrm{ integral in }A\}}{\{f\in A\left[[x,y]\right]:f\left(\underline{O}\right)=0\}}$$

Further details are given by Katz [31]. Both eigenfunctions ω and ν of the endomorphism ring R, as discussed earlier, in $H_{DR}^1(E,\mathbb{C})$ can be embedded in $H_{DR}^1(E/A)$ under our assumption. We choose a local uniformizer ξ of E over A near $\underline{O}$ that is a formal power series of $-\frac{x}{y}$ starting with $-\frac{x}{y}$. Using expansion at infinity with ξ to get $H_{DR}^1(\widehat{E}/A)$, consisting of formal differentials of E of the form $\sum c\left(n\right){\xi }^{n}d\xi$, where $c\left(n\right)\in A$. By formal integration, these differentials will be represented as ${\sum }_{n\ge 1}\frac{c(n-1)}{n}{\xi }^n$ in $H_{DR}^1(\widehat{E}/A)$. Below, we assume

$$\omega =\sum _{n\ge 1}\frac{a(n-1)}{n}{\xi }^{n}d\xi ,\text{and}\nu =\sum _{n\ge 1}\frac{b(n-1)}{n}{\xi }^{n}d\xi$$

with coefficients in A. Under this notation, any degree–p Frobenius lifting Φ of $\xi \mapsto {\xi }^p$ from ${\mathbb{F}}_p$ to A acts semi-linearly, which sends the class represented by

$$\sum _{n\ge 1}\frac{c\left(n\right)}{n}{\xi }^n$$

to the class represented by

$$\sum _{n\ge 1}\frac{c{\left(n\right)}^{\sigma }}{n}\Phi {\left(\xi \right)}^n$$

Let $H_{DR}^1(\widehat{E}/A,\left(p\right))$ be the cohomology group defined from a subcomplex of the de Rham complex of E with respect to the divided power ideal $\left(p\right)$. To be more precise, $H_{DR}^1(\widehat{E}/A,\left(p\right))$ is isomorphic to

$$\frac{\{f\in {\mathbb{Q}}_p\left[\sqrt{1-\lambda }\right]\left[\left[\xi \right]\right]:f\left(\underline{O}\right)=0,df\text{ intergral in }A\}}{\{f\in pA\left[\left[\xi \right]\right]:f\left(\underline{O}\right)=0\}}$$

In this space, the class representing ω is annihilated by ${\Phi }^2-b_1\Phi +b_2$ where $b_1,b_2\in A$. By comparison between étale and crystalline cohomologies, $T^2-b_{1}T+b_2$ is related to the local Hasse–Weil zeta function of E over K and, consequently, $b_2^2=\pm p^2$. It follows that

$$a(n{p}^r-1)-b_{1}a{(n{p}^{r-1}-1)}^{\sigma }+b_{2}a{(n{p}^{r-2}-1)}^{{\sigma }^2}\equiv 0(mod{p}^{r}A)$$

(5.1)

For simplicity, we assume that $a\left(0\right)$ and $b\left(0\right)$ are both 1 after normalization.

Proposition 16. With notation and assumptions as above, if under the choice of the local uniformizer there exists a degree p (resp. $p^2$) Frobenius lifting Φ that commutes with the induced action of R on $H_{DR}^1(\widehat{E}/A,\left(p\right))$ in the ordinary (resp. supersingular case) then

$$a(p-1)b(p-1)=\text{sgn}·b_2(mod{p}^{2}A)$$

where $\text{sgn}=\pm 1$ and it is 1 if and only if $b_1\not\equiv 0(modpA)$, i.e., p is ordinary.

Proof. Recall that $b_2^2=\pm p^2$. When p is ordinary, i.e., $a(p-1)\not\equiv 0(modpA)$, and Φ commutes with R, then ω and ν are eigenfunctions of Φ with eigenvalues π and $\overline{\pi }$, which are the two roots of $T^2-b_{1}T+b_2=0$. In particular, $\overline{\pi }$ is the unit root in A and $\pi =b_2/\overline{\pi }.$ (See work of Katz [32] for further details.) From comparing the pth coefficients of $\sum \frac{a{\left(n\right)}^{\sigma }}{n}\Phi \left(n\right)=\pi ·\frac{a\left(n\right)}{n}{t}^n$, we conclude that

$$1\equiv \pi \frac{a(p-1)}{p}(mod\pi A)$$

Similarly, we have

$$1\equiv \overline{\pi }\frac{b(p-1)}{p}(mod\pi A)$$

and thus

$$1\equiv \frac{\pi \overline{\pi }a(p-1)b(p-1)}{p^2}=\frac{b_2}{p}·\frac{a(p-1)b(p-1)}{p}(modpA)$$

Multiplication by p on both sides gives $a(p-1)b(p-1)\equiv b_2(mod{p}^{2}A)$.

In the supersingular case, $b_1=0$. We first assume that there is a lifting $\varphi$ of $t\mapsto t^p$ which stabilizes the A–submodule of $H_{DR}^1(E/A,\left(p\right))$ generated by ω and ν. Assume that the matrix of Φ under the basis consisting of ω and ν is $M=\left(\begin{array}{cc}u& v\\ w& -u\end{array}\right)$. In particular, $-u^2-vw=b_2$. We now determine M modulo $pA$. Assume that $u\not\equiv 0(modpA)$, then $v,w$ are nonzero modulo $pA$ as well. Otherwise, the determinant cannot be p. As both u and w are units, one can choose a different basis such that the matrix becomes diagonal modulo $\pi A.$ With determinant p, one of the eigenvalues is a unit, contradicting the fact that the trace is 0 modulo $pA$. Thus in fact $u\equiv 0(modpA)$ and $uz-vw\equiv -vw\equiv b_2(mod{p}^{2}A)$. In this case,

$$1\equiv v\frac{b(p-1)}{p}(modpA)\text{and}1\equiv w\frac{a(p-1)}{p}(modpA)$$

This implies w is a unit and $v\equiv 0(modpA)$ and the desired result follows, namely

$$a(p-1)b(p-1)\equiv \frac{p^2}{vw}\equiv -b_2(mod{p}^{2}A)$$

In fact, such a lift $\varphi$ exists by adding any p-torsion of the elliptic curve to ${\mathbb{Q}}_p\left(\sqrt{1-{\lambda }_d}\right)$, which allows us to solve ${\varphi }^2=\Phi$. Here Φ is the degree–$p^2$ Frobenius lifting that commutes with R under our assumption. Both ω and ν are eigenfunctions of Φ with the same eigenvalue $-b_2$. The corresponding field extension is ramified. However, using the corresponding maximal ideal, the above argument shows that

$$\frac{a(p-1)b(p-1)-b_2}{p}$$

lies in the maximal ideal. Hence, our claim follows.

              ☐

The following lemma is an immediate consequence of the fact that the Euler numbers $\phi \left(d\right)\le 2$, for $d\in \{2,3,4,6\}$.

Lemma 17. Let $d=\{2,3,4,6\}$. Then for any odd prime p coprime to d,

$$\frac{{\left(\frac{1}{d}\right)}_k{\left(\frac{d-1}{d}\right)}_k}{k{!}^2}\equiv 0(modp)$$

when $\frac{p-1}{d}<k\le p-1$ and

$$\frac{{\left(\frac{1}{d}\right)}_k{\left(\frac{d-1}{d}\right)}_k}{k{!}^2}\equiv 0(mod{p}^2)$$

when $p-\frac{p+1}{d}<k\le p-1$

Consequently, we have the following truncated version of Clausen’s formula.

Lemma 18. Let $d\in \{2,3,4,6\}$, p be an odd prime coprime to d, and $t\in {\mathbb{Z}}_p$. Then

$${}_2{F}_1{\left[\begin{array}{cc}\frac{1}{d}& \frac{d-1}{d}\\ & 1\end{array};t\right]}_{p-1}^2\equiv {}_3{F}_2{\left[\begin{array}{ccc}\frac{1}{2}& \frac{1}{d}& \frac{d-1}{d}\\ & 1& 1\end{array};-4t(t-1)\right]}_{p-1}(mod{p}^2)$$

Proof. The Clausen formula can be also stated as

$${}_2{F}_1{\left[\begin{array}{cc}\frac{1}{d}& \frac{d-1}{d}\\ & 1\end{array};t\right]}^2={}_3{F}_2\left[\begin{array}{ccc}\frac{1}{2}& \frac{1}{d}& \frac{d-1}{d}\\ & 1& 1\end{array};-4t(t-1)\right]$$

Note that both hand sides are polynomials of degree no larger than $2p-2$. In fact, modulo $p^2$, the polynomial on the right has only degree $p-1$. It suffices to prove the kth coefficient on the left hand side is also 0 modulo $p^2$ when $k\ge p$, which follows from Lemma 17.   ☐

We now prove Theorems 1 and 2.

Proof of Theorems 1 and 2. Let $\xi =\frac{-x}{y}$ be the local uniformizer of the elliptic curve. As discussed in Section 3.4, we assume $\nu =c{\partial }_{{\lambda }_d}\omega +\omega$ for some constant $c\in A$ under our assumptions. So $a(p-1)=H_{d,p-1}\left(t\right)$ with $t=\frac{1-\sqrt{1-{\lambda }_d}}{2}$ and $b(p-1)$ can be computed accordingly. Note that the leading coefficients of both ω and ν are 1.

Let $d\in \{2,4\}$. Then $\frac{-x}{y}$, as a function on the elliptic curve, has its zeros and poles supported at the 2-torsion points of the elliptic curve. Consequently, in the ordinary case the multiplication by π map, $\left[\pi \right]$, is a degree-p rational function of t, where π is the non-unit root of $T^2-b_{1}T+b_2$ as discussed in Proposition 16. As π is in the ring of integers of the CM quadratic field that contains the endomorphism ring R, π commutes with R. The formal power series $\sum \frac{a\left(n\right)}{n}{\xi }^n$ is the formal logarithm of the formal group arising from the elliptic curve, which is of height 1 when modulo $pA$ due to the ordinary assumption. (The unfamiliar reader is encouraged to consult work of Stienstra and Beukers [24] for a reference on this terminology.) Therefore,

$$\left[\pi \right]\left(\xi \right)={\Delta }_d^{(p-1)/4}{\xi }^p(modpA)$$

for some ${\Delta }_d$ that can be computed explicitly. For further details, consult papers of Kibelbek et al. [18] and Costeret al. [33]. If we replace ξ by $\tilde{\xi }:={\Delta }_d^{-1/4}\xi$, then $\left[\pi \right]\left(\tilde{\xi }\right)\equiv {\tilde{t}}^p(modpA)$, which allows us to apply Proposition 16. As computed by Kibelbeket al., this change of variable corresponds to the quartic twists for both cases that we discussed in 4.3. Recall that in Section 4.2, after applying the corresponding quartic twist, we find that the constant term is p from the oddness conclusion stated as Lemma 15. Similarly, for $d=3$, the zeros and poles of $\frac{-x}{y}$ locate at the 3-torsion points of the elliptic curve. From the theory of elliptic functions, one can show that the multiplication by π map on t, likewise a degree–p rational map of t, after a similar quartic twisting, can be used as the Frobenius map in Proposition 16. For $d=6$, it follows from $S{L}_2\left(\mathbb{Z}\right)$, the monodromy group for $E_6\left(t\right)$ is an index-6 supergroup of the monodromy group for $E_2\left(t\right)$, which is $\Gamma \left(2\right)$ (see Remark 7). In each case, we need to replace ξ by $\tilde{\xi }:={\Delta }_d^{-1/4}\xi$ to use Proposition 16 and ${\Delta }_d^{(p-1)/2}\equiv \left(\frac{1-{\lambda }_d}{p}\right)(mod\pi A)$. In conclusion, we have

$$a(p-1)b(p-1){\Delta }_d^{(p-1)/2}\equiv p(mod{p}^{2}A)$$

for the ordinary case. Now we relate $a(p-1)b(p-1){\Delta }_d^{(p-1)/2}$ to our theorems.

We first prove Theorem 2. The case of $d=2$ was proved by Kibelbek et al. [18]; we handle the cases $d=3,4,6$ here. When p is supersingular, the claim follows from Lemma 18. Let p be ordinary. Recall that $a\left(n\right)=H_{d,n-1}\left(t\right)$ as in Lemma 12. One can use the same formula to compute $H_{d,(d-1)p-1}\left(t\right)$. In particular, $H_{d,(d-1)-1}\left(t\right)=1$ for $d=3,4,6$. Let $k=1$ or $d-1$. By comparing the ${\tilde{\xi }}^{kp}$th coefficients of

$$\sum \frac{{\left(a\left(n\right){\Delta }_d^{n/4}\right)}^{\sigma }}{n}\Phi {\left(\tilde{\xi }\right)}^n=\pi \sum \frac{a\left(n\right){\Delta }_d^{n/4}}{n}{\tilde{\xi }}^n$$

we have

$$1\equiv \frac{\pi }{p}·H_{d,kp-1}\left(t\right){\Delta }_d^{(kp-k)/4}(mod{\pi }^{2}A)$$

for some $\gamma \in A$, where ${\Delta }_d^{(kp-k)/4}=\epsilon (1-k\gamma p)(mod{\pi }^{2}A)$ with ε a forth root of unity and ${\epsilon }^2=\left(\frac{1-{\lambda }_d}{p}\right)$. Meanwhile, for both $k=1$ and $k=d-1$, $H_{d,kp-1}\left(t\right)$ are hypergeometric series in t that terminate before the pth term. By the formula given in Lemma 12, the previous two lemmas, and the p-adic analysis given by the third author [12],

$$H_{p,kp-1}\left(t\right)={}_2{F}_1{\left[\begin{array}{cc}\frac{1}{d}& \frac{d-1}{d}\\ & 1\end{array};t\right]}_{(p-1)}+k\delta p$$

(5.2)

for some $\delta \in A$ when $k=1,d-1$. Thus $\gamma \equiv \delta (mod\pi A)$, which implies

$${}_2{F}_1{\left[\begin{array}{cc}\frac{1}{d}& \frac{d-1}{d}\\ & 1\end{array};\frac{1-\sqrt{1-{\lambda }_d}}{2}\right]}_{(p-1)}=\frac{p}{\pi \epsilon }(mod{\pi }^{2}A)$$

The claim of Theorem 2 follows from Lemma 18. The ordinary case of Theorem 1 also follows.

To conclude the supersingular case for Theorem 1, we note that the multiplication by $-p$ map on $t=\frac{-x}{y}$ results in a degree–$p^2$ rational map on t, which can be used for Proposition 16 after the same quartic twist as the ordinary case.         ☐