Next Article in Journal
Optimising Data Analytics to Enhance Postgraduate Student Academic Achievement: A Systematic Review
Previous Article in Journal
The Early Career Framework: Why Context Matters for Teacher Professional Development
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Education Sciences
Volume 14
Issue 11
10.3390/educsci14111262
education-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

An Investigation of High School Preservice Teachers’ Self-Efficacy in Teaching Mathematics

by
Winston Hendricks
1,
Babawande Emmanuel Olawale
1,* and
Khalid Saddiq
2
1
School of Further and Continuing Education, Faculty of Education, University of Fort Hare, Alice 5700, South Africa
2
Department of Mathematics Education, University of Tasmania, Launceston, TAS 7248, Australia
*
Author to whom correspondence should be addressed.
Educ. Sci. 2024, 14(11), 1262; https://doi.org/10.3390/educsci14111262
Submission received: 17 October 2024 / Revised: 15 November 2024 / Accepted: 18 November 2024 / Published: 19 November 2024
Browse Figures
Versions Notes

Abstract

:
The influential theory proposed by Bandura suggests that teachers with high self-efficacy—those who strongly believe in their abilities—are more likely to effectively enhance learners’ performance. Numerous studies have consistently provided substantial evidence supporting this claim. Therefore, it is essential to investigate preservice teachers’ self-efficacy in teaching mathematics and their expectations for outcomes. This study aims to ascertain the self-efficacy beliefs of preservice high school teachers regarding mathematics instruction. The Mathematics Teaching Efficacy Beliefs Instrument (MTEBI) survey was completed by 63 participants from a rural institution. This survey was administered after the completion of a Mathematics Methods course but before the onset of the school experience exercise (practicum). The MTEBI consists of 21 items and uses a five-point, forced-choice Likert-like scale to assess participants’ perceptions of their effectiveness in teaching mathematics. The survey evaluates two subscales: Personal Knowledge of Mathematics Teaching Efficacy (PMTE) and Expectancy of Mathematics Teaching Outcomes (MTOE). The results on the PMTE subscale showed a mean score of 43.1 and a standard deviation of 6.1, indicating that respondents possess good personal mathematics teaching efficacy. On the MTOE subscale, the participants had a mean score of 31.5 and a standard deviation of 3.72, reflecting a positive anticipation of learners’ mathematics learning outcomes. The research also found no statistically significant differences in self-efficacy in teaching mathematics or expectations between the two genders. However, female preservice mathematics teachers reported a notably higher average in teaching self-efficacy and teaching outcome expectancy compared to their male colleagues. Consequently, this study concludes that although female preservice teachers may be more effective than their male counterparts, there is a general need to enhance self-efficacy among all preservice teachers by providing various opportunities, such as increased coursework, field experiences, and peer mentoring.

1. Introduction

The concept of mathematics teaching efficacy refers to the importance of having teachers who possess confidence in their ability to instruct all students in mathematics effectively. Many job vacancies in recent years now require advanced mathematical expertise [1,2]. Educators’ beliefs and attitudes about the effectiveness of their teaching methods in bringing about changes in their classroom practices influence their actual teaching methods [3]. Several studies, such as those by [2,4,5,6], have shown that mathematics teachers who possess a strong sense of self-efficacy in the classroom are more inclined to use student-centered teaching models. Conversely, research conducted by [7,8] revealed that teachers who were dissatisfied or had low levels of mathematical efficacy were more likely to avoid the planning and teaching of mathematics. Research has also demonstrated that teaching self-efficacy, which pertains to teachers’ beliefs about their competence in carrying out professional duties, plays an important role in shaping teachers’ teaching approaches and student achievement [9,10,11]. Extensive studies have confirmed that individuals’ self-perceptions of their abilities significantly affect their behavior, motivation, and overall success or failure [11,12,13,14]. The direct and lasting positive impact of teaching self-efficacy in mathematics on student performance has made self-efficacy an increasingly important topic in teacher education programs. Thus, while it is necessary to investigate high school preservice teachers’ self-efficacy in teaching mathematics, the novelty of this study lies in the integration of self-efficacy theory within the framework of social cognitive theory, which adds depth to the understanding of how perceived self-efficacy influences teaching behaviors. Furthermore, this study’s emphasis on the impact of teacher self-efficacy on student outcomes contributes to the existing literature by highlighting the importance of teacher beliefs in educational effectiveness, particularly in the context of mathematics education.

1.1. Conceptualizing Teacher Self-Efficacy

“Teacher efficacy” describes how a teacher feels about their teaching effectiveness and capacity to facilitate favorable student learning outcomes [2,5,11]. Self-efficacy is the belief in one’s ability to perform assigned activities effectively [15]. In education, where teachers face challenges that require originality, perseverance, and determination, self-efficacy holds great significance. According to Bandura’s social cognitive theory, self-efficacy beliefs stem from the confidence that one can successfully plan and execute the necessary actions to achieve specific goals [15]. Thus, [16] delineated four origins of self-efficacy: physiological conditions, verbal influence, indirect experiences, and achievements in performance. He posited that personal achievements, derived from mastery experiences, are the primary determinant of an individual’s self-efficacy. Therefore, successfully completing challenging assignments with little assistance from others fosters a sense of competence, thereby enhancing one’s self-efficacy [15,16]. Another determinant that can impact an individual’s self-efficacy is vicarious experiences. This phenomenon occurs when an individual observes another person performing a challenging activity and develops a sense of assurance in their own ability to achieve the same outcome [15,16]. Other factors influencing individual self-efficacy include their physiological state, which encompasses anxiety and stress related to a task, and verbal persuasion regarding their competence in managing difficult situations or tasks, particularly from a reliable and trustworthy source [16]. Consequently, Tschannen-Moran, etc. [17] investigated the formation of teachers’ beliefs about their teaching abilities and discovered that all four of [16]’s suggested sources—including mastery experiences—must be incorporated into sources of teaching efficacy expectations. Therefore, it becomes pertinent that teacher preparation programs adopt measures to enhance preservice teachers’ readiness and beliefs to teach mathematics in high school settings. To achieve this, teacher preparation programs can support preservice teachers’ self-efficacy by ascertaining the self-efficacy of high school preservice teachers, thoroughly analyzing their self-efficacy in mathematics, and investigating specific strategies to improve their self-efficacy in teaching the subject. Hence, the former serves as motivation for the present study, as it seeks to examine the self-efficacy beliefs of preservice high school teachers regarding their mathematics teaching.

1.2. Preparation of Preservice Mathematics Teachers

In the preparation of preservice mathematics teachers, Avery, etc. [18] contends that it is essential to create a working atmosphere that is both demanding and supportive to bolster the self-confidence of prospective teachers. Therefore, McLaughlin [19] argues that method courses should be included to provide preservice teachers with opportunities to actively participate in scientific activities, contributing to the acquisition of the knowledge, skills, and mindset needed to become competent and self-assured professionals. Furthermore, courses in mathematical techniques should enhance confidence in one’s ability to excel in mathematics by employing specific teaching methods and learning activities [8]. Nevertheless, adequately preparing mathematics teachers is becoming increasingly significant in teacher training programs [20,21,22]. Providing educators with the essential skills to teach mathematics effectively is an urgent concern for those seeking to improve students’ knowledge acquisition [23,24]. Regrettably, it cannot be assumed that preservice mathematics teachers enrolled in South African universities will possess proficiency in mathematics. While this study aimed to examine high school preservice teachers’ self-efficacy in teaching mathematics, the concept of learning to teach allowed for an exploration of the literature on pedagogical content knowledge.
Extensive studies have been conducted regarding the correlation between mathematics teachers’ subject matter expertise and their teaching effectiveness. Numerous academic studies have shown compelling evidence for a positive relationship between these two factors [24,25,26,27,28,29,30]. Research indicates that teachers should acquire pedagogical content knowledge (PCK), which combines subject knowledge and pedagogical abilities [24,25]. The term “pedagogical content knowledge” refers to understanding various methods of presenting mathematical material to enhance students’ comprehension [24,26]. According to [26], the process of conveying information is complex and multidimensional. He posits that teachers should be fundamentally familiar with several areas, including general curriculum knowledge; content knowledge; pedagogical content knowledge; knowledge of educational contexts; knowledge of learners and their characteristics; and knowledge of educational ends, purposes, and values. Additionally, Olawale [21] asserts that exceptional teachers must be able to engage with students effectively and possess a thorough understanding of their subject matter. Successful teachers must also possess certain dispositions, according to [31], which include collegiality, self-reflection, collaborative and interactive skills, and the ability to adapt their personal and professional practices through reflection.
Although preservice teachers often hold strong beliefs about teaching and learning upon entering teacher education programs, their individual educational experiences significantly influence their understanding of the curriculum and their teaching approaches [22]. Consequently, they tend to teach in the same manner in which they were instructed [32,33,34]. Since most preservice teachers have received their mathematics instruction through traditional methods, this presents a significant challenge for secondary mathematics education [32,35,36]. As a result, preservice teachers often choose to teach courses that emphasize the instructor and demonstrate limited awareness of other pedagogical approaches. This issue is compounded by the fact that some teacher education programs and field experiences tend to reinforce pre-existing beliefs in preservice teachers rather than challenge their current knowledge [37,38]. Therefore, it becomes imperative to examine the self-efficacy of high school preservice teachers in teaching mathematics.

1.3. Framework: The Self-Efficacy Theory (SET)

The theoretical basis for this study is the self-efficacy theory (SET), a component of [16]’s social cognitive theory. According to this theory, two crucial factors influence behavior: perceived self-efficacy and outcome expectations [16,39]. Outcome expectations refer to the perceived benefits and drawbacks of engaging in a particular behavior [39]. The SET posits that individuals choose to engage in activities they believe they can excel in, while avoiding those they perceive as challenging. However, even when an activity appears difficult, individuals with a strong sense of self-efficacy are confident in their ability to succeed [13]. As a result, they view challenges as obstacles to overcome rather than threats to avoid. In contrast, individuals who doubt their ability to successfully tackle difficult tasks perceive these challenges as potential threats. Consequently, they tend to avoid such tasks due to their perceived inadequacies or the barriers that hinder their path to achievement [13]. These individuals often give up when faced with difficulties, leading to a loss of faith in their capabilities [13,40]. The SET theory suggests that the sense of efficacy is shaped by four factors—physical and emotional state, verbal persuasion, vicarious experience, and mastery experience [13,40,41]—as illustrated in Figure 1 below:
As shown in Figure 1 above, Bandura outlined four main sources of self-efficacy: mastery experiences, vicarious experiences, verbal persuasion, and physiological conditions [13,16,40]. Mastery experiences are highly significant, as they enhance individuals’ confidence in their abilities when they successfully complete a task. Conversely, failures can reduce self-efficacy, especially if they occur prematurely in the learning process [40]. Observing others succeed through vicarious experiences can bolster one’s confidence in their own abilities, particularly if the observer identifies with the model. Verbal persuasion refers to the impact of others’ support and comments on one’s self-efficacy; however, firsthand experiences are typically less influential [13]. Physiological states include individuals’ external and internal reactions when faced with challenging circumstances [15]. Positive emotional states can enhance self-efficacy, while negative emotions can diminish it [16,40]. Bandura’s self-efficacy theory has significant implications for high school preservice teachers. The theory suggests that educators and teacher preparation programs should prioritize the cultivation of self-efficacy by implementing targeted interventions, such as mentorship opportunities, practical teaching experiences, and reflective processes. By fostering a strong sense of self-efficacy among preservice teachers, educational institutions can enhance their readiness to navigate the complexities of teaching mathematics, ultimately leading to improved educational outcomes for their future students.

2. Materials and Methods

2.1. Research Paradigm, Approach, and Design

The paradigm used in this study was positivist. As stated by [42], the hypothetico-deductive approach is employed by positivism to test a priori hypotheses, which are typically expressed in quantitative terms. According to [42], this paradigm allows for the identification of functional relationships between outcomes (dependent variables) and causal and explanatory factors (independent variables). The selection of the positivist paradigm for this study was based on its emphasis on the systematic and objective application of scientific procedures to collect and analyze information about high school preservice teachers. In this research, a quantitative methodology was delineated by [43] as a method for evaluating objective theories by investigating the correlation between variables. This methodology was deemed appropriate since it facilitates the use of standardized assessment instruments to consistently and reliably measure degrees of self-efficacy. This study utilized a post test-only control group design. Thus, some scholars [8,44,45] defined a post test-only study design as an experimental model in which participants are administered a post test only after exposure to the independent variable. The selection of this design was based on its ability to allow researchers to assess the impact of the intervention on the outcome (self-efficacy) without any potential distortion or influence from pretesting.

2.2. Population, Sample, and Sampling Technique

According to [46], a population is a group of individuals or organizations that possess unique characteristics distinguishing them from other entities. This study population was a cohort of 1326 preservice teachers registered at the Faculty of Education at a rural institution in the Eastern Cape Province of South Africa during the 2023 academic term. The research utilized a stratified sampling method described by [45,47], which involves selecting a subset from a population by considering their classification and employing random selection. This approach is suitable as it ensures a more representative and dependable sample, thereby enhancing the validity of the study’s findings. This study’s target demographic consisted of high school preservice teachers currently in their final year of study and enrolled in “Mathematics Method” courses. The strata were established based on domain specialization, specifically mathematics, in this instance. A total of 63 high school preservice mathematics instructors from the selected university were included in the sample for this quantitative investigation. The descriptive statistics of the sample for this study are tabulated in Table 1 below.

2.3. Data Collection Instruments, Analysis, and Ethics

Questionnaires were used in this study to gather data from pre-selected samples of primary school teachers who had taken PPG and those who had not. Using a 5-point Likert scale, the questionnaire had 21 items. The scale range consisted of strongly disagree, disagree, slightly agree, agree, and strongly agree. Responses that expressed strong disagreement received the lowest points (1), and those that expressed strong agreement received the highest points (5). The Mathematics Teaching Efficiency Beliefs Instrument (MTEBI) scale, created by Enochs, Smith, and Huinker (2000), was used to collect research data. The MTEBI comprises two subscales: the Mathematics Teaching Outcome Expectancy (MTOE) and the Personal Mathematics Teaching Efficacy (PMTE). Question items 2, 3, 5, 6, 8, 11, 15, 16, 17, 18, 19, 20, and 21 are among the 13 items in the PMTE. In contrast, there are eight items in the MTOE: items 1, 4, 7, 9, 10, 12, 13, and 14. The PMTE scale had scores between 13 and 65, whereas the MTOE had values between 8 and 40. According to [1], the Personal Mathematics Teaching Efficacy (PMTE) and Mathematics Teaching Outcome Expectancy (MTOE) are valid and reliable instruments that constitute the Mathematics Teaching Efficiency Beliefs Instrument (MTEBI) scale. For the PMTE scale, the Cronbach alpha coefficient of internal consistency was 0.88, while for the MTOE scale, it was 0.77 [1]. The instrument’s construct validity was established by employing a Confirmatory Factor Analysis, which revealed that the two subscales were not reliant on each other [1]. For this study, question items 3, 6, 8, 15, 17, 18, 19, and 21 had to be reversed before the data were examined to obtain consistent values between the positive and negative assertions. A response that indicates “strongly disagree” will result in a score of 1. The score must then be changed back to the greatest possible number, which is 5. A score of 3 remains unchanged, while a score of 4 is flipped to 2, a score of 5 is flipped to 1, and a score of 2 is flipped to 4. After the data were acquired, the degree of participants’ efficacy in teaching mathematics, as indicated by the dataset, was arranged and summarized using quantitative descriptive statistics. The mean of the individual items and the subscales (PMTE and MTOE) was computed to determine the central tendency. The range and standard deviation were calculated to gauge variability.

3. Results

The participants’ overall PMTE subscale scores (M = 43.1, SD = 6.1) show that they have good personal efficacy in teaching mathematics, meaning that they believe they have the knowledge and skills needed to do the job well. As indicated in Table 2, the two PMTE items with the highest scores were 2, “I will continually find better ways to teach mathematics” (M = 4.6, SD = 0.7), and 11, “I understand mathematics concepts well enough to be effective in teaching high school mathematics” (M = 4.4, SD = 0.4). The PMTE subscales’ Cronbach’s alpha (0.729) confirmed that the items are sufficiently consistent to suggest that the measure is reliable.
In Figure 2 above, The PMTE survey results indicate a dichotomy in high school preservice teachers’ perceptions: while many express confidence in their understanding and ability to teach mathematics, there are notable concerns about their overall effectiveness and capability to engage students in learning. However, three major quotes emphasize the importance of self-efficacy in mathematics teaching and the potential benefits of professional development to bolster teachers’ confidence and effectiveness in the classroom.
Q2: “I will continually find better ways to teach mathematics”—This statement reflects a proactive attitude towards teaching improvement, which is essential for effective pedagogy.
Q17: “I wonder if I have the necessary skills to teach mathematics”—This quote underscores the self-doubt that can hinder teachers’ effectiveness, indicating a gap that professional development could address.
Q19: “When a student has difficulty understanding mathematics concepts, I will usually be at a loss as to how to help the student understand it better”—This highlights a critical area where teachers may need additional support and resources to enhance their instructional strategies.
The survey results reveal a complex picture of teachers’ self-efficacy in mathematics instruction. High mean scores for items related to finding better teaching methods and understanding mathematical concepts indicate a strong belief in their capabilities. However, the presence of lower scores on questions addressing overall effectiveness and student engagement suggests that many high school preservice teachers may struggle with feelings of inadequacy in their teaching practice. This duality highlights the need for targeted professional development that addresses both content knowledge and pedagogical strategies, fostering an environment where teachers feel empowered to enhance their teaching efficacy.
The MTOE subscale’s overall scores (M = 31.5, SD = 3.72) show that participants had a modest expectation that their instruction would benefit learners’ mathematical achievement. The highest-scoring items on the MTOE were 2, “When a student does better than usual in mathematics, it is often because the teacher exerted a little extra effort (M = 4.46, SD = 0.60)”; 9, “The inadequacy of a student’s mathematics background can be overcome by good teaching (M = 4.24, SD = 0.48)”; and 14, “If parents comment that their child is showing more interest in mathematics at school, it is probably due to the performance of the child’s teacher” (M = 4.16, SD = 0.38). Table 3 displays the results of the MTOE. The MTOE subscale’s Cronbach’s alpha of 0.727 confirmed that the items are sufficiently consistent to suggest the measure’s reliability.
In Figure 3 above, the highest-rated item (Q1) emphasizes the importance of teacher effort in student improvement, while the lowest-rated item (Q4) suggests that improvements in mathematics grades are often attributed to more effective teaching approaches. Other notable findings include the belief that good teaching can overcome students’ inadequate mathematics backgrounds (Q9) and that a teacher’s responsibility is closely linked to student achievement (Q12). Overall, the results highlight the significant role teachers play in shaping students’ mathematical success. For instance,
Q1: “When a student does better than usual in mathematics, it is often because the teacher exerted a little extra effort.”—This quote emphasizes the essential role of teacher effort in fostering student success, highlighting the dedication required from educators.
Q9: “The inadequacy of a student’s mathematics background can be overcome by good teaching.”—This statement reflects a hopeful perspective on the power of effective teaching to mitigate prior educational deficiencies.
Q12: “The teacher is generally responsible for the achievement of students in mathematics.”—This quote encapsulates the accountability educators feel regarding their students’ performance, suggesting a strong commitment to their teaching practices.
The survey MTOE subscale results underscore the critical role high school preservice teachers play in influencing students’ mathematical performance. The high mean scores for items emphasizing preservice teacher effort and effectiveness suggest that they believe their actions significantly impact student outcomes. Conversely, the lower score for attributing grade improvements to teaching approaches indicates a more nuanced view, recognizing that multiple factors contribute to student success. The findings reflect a strong belief in the potential of effective teaching to address students’ learning gaps, reinforcing the importance of teacher training, mentorship, and professional development programs.
Table 4 illustrates the disparities in self-efficacy and anticipation to teach mathematics among preservice teachers, categorized by gender. The table presents data on the average and variability of the Personal Mathematics Teaching Efficacy (PMTE) subscale and Mathematics Teaching Outcome Expectancy (MTOE) subscale scores, divided by the two primary genders of the study participants. This study found no statistically significant differences in self-efficacy in teaching mathematics or expectations between the two genders. However, female preservice mathematics teachers reported a notably higher average in teaching self-efficacy and teaching outcome expectancy compared to their male colleagues.

4. Discussion

The results of this study showed that preservice mathematics teachers expressed high self-efficacy in their ability to teach mathematics. As such, the findings contribute significantly to the existing body of literature surrounding teacher self-efficacy, particularly in the context of preservice mathematics education. Bandura’s theory of self-efficacy underscores the critical role of teachers’ beliefs in their capabilities, influencing their instructional practices and, consequently, student outcomes [13,16,40]. This study’s results corroborate Self-Efficacy Theory (SET), demonstrating that preservice teachers who possess high levels of self-efficacy are better equipped to foster positive learning environments and enhance student performance in mathematics [6,11,13,40]. Interestingly, the gender differences observed in self-efficacy beliefs are particularly noteworthy. The findings revealed that female participants were more efficacious in teaching mathematics at senior secondary schools. This can be attributed to their cognitive appraisal of skills and emotional reactions to teaching, which may vary between genders, as well as their intense involvement in reflective practices and the changing emphasis in teacher education programs towards fairness and inclusive practices. These factors may empower female preservice teachers, effectively strengthening their confidence in teaching mathematics [5,48]. Overall, the high mean score indicating that they will continue to seek better ways to teach mathematics clearly demonstrates a growth mindset and commitment to ongoing professional development. This proactive approach to improving their teaching skills suggests that preservice teachers believe in their ability to adapt and innovate in the classroom. These findings corroborate those of [8,49,50], who stated that highly effective teachers employ a variety of inclusive and innovative strategies, such as individualized learning goals and a strengths-based approach, along with the conviction that every learner can achieve.
Similarly, the findings revealed that the next mean with the highest frequency highlighted preservice student teachers’ confidence in their understanding of mathematical concepts, affirming their subject knowledge and expertise. This self-assurance can help them effectively communicate complex mathematical ideas to their learners and address misconceptions. These findings align with those of [51,52], who showed that preservice teachers with high self-efficacy throughout their training programs maintain increased confidence in their ability to teach in future classrooms. Thus, Arrington [48] argued that the development of preservice teachers’ self-efficacy for teaching is enhanced when they are provided with knowledge of what needs to be done, the skills necessary to do it, and sufficient practice using various strategies. Furthermore, by stating that they will typically be able to answer learners’ questions, preservice mathematics teachers demonstrate a belief in their ability to support students’ learning and provide effective guidance and instruction. This finding is consistent with other studies [8,49,53], which found that preservice teachers have a higher perception of their ability to teach mathematics compared to the average individual. Similarly, studies such as [54,55] found a positive correlation between higher levels of self-efficacy and the extent of teaching experience in mathematics.
Preservice teachers’ beliefs regarding the likely outcomes of their teaching practices, particularly in the context of student learning and performance, also differ by gender. In this study, female participants exhibited a stronger belief in their ability to succeed in a mathematics classroom and achieve specific learning outcomes than males. While this phenomenon can be attributed to factors such as a growth mindset, personal experiences, and varying feedback individuals may have received, it is crucial to recognize the significant variability in individual experiences. Therefore, it is important to avoid making sweeping generalizations that may not apply to all males or females. Efforts to provide a fair and nurturing learning environment can foster the development of robust self-confidence in all preservice teachers, regardless of their gender.

5. Conclusions

The present study examined the self-efficacy of high school preservice teachers in the domain of mathematics instruction, with a particular emphasis on gender differences. The findings revealed that preservice teachers demonstrated relatively high levels of self-efficacy in mathematics instruction. Specifically, in terms of overall mathematics teaching self-efficacy and anticipated teaching outcomes, female preservice teachers exhibited significantly higher self-efficacy levels compared to their male counterparts. These results underscore a critical need to enhance the self-efficacy of preservice teachers in mathematics instruction, particularly among male preservice teachers. Teacher educators should provide preservice teachers with opportunities to bolster their self-efficacy through academic coursework, practical field experiences, and mentorship from experienced educators. Furthermore, it is essential to address the gender disparity in mathematics self-efficacy by implementing activities designed to cultivate confidence in teaching mathematics. This research carries significant implications for the field of teacher education. Firstly, it emphasizes the necessity of prioritizing the enhancement of preservice teachers’ self-efficacy in mathematics instruction. Additionally, it highlights the urgent need to address the discrepancies in self-assurance in mathematics between genders. Further inquiry is warranted to analyze the factors contributing to preservice teachers’ confidence in mathematics instruction. Moreover, additional research is needed to develop and evaluate interventions aimed at improving preservice teachers’ self-efficacy.

6. Recommendation

Based on the results from the Personal Mathematics Teaching Efficacy (PMTE) and Mathematics Teaching Outcome Expectancy (MTOE) subscales, recommendations were made to enhance the self-efficacy of preservice teachers in mathematics education.
  • Professional Development: Teacher education programs should offer targeted professional development programs focused on enhancing mathematics teaching skills and self-efficacy. This could include workshops on effective teaching strategies, use of manipulatives, and addressing diverse student needs in mathematics.
  • Mentorship Programs: Teacher education programs should implement formal mentorship initiatives that connect preservice teachers with seasoned mathematics educators who can offer crucial support. Through this initiative, mentors will provide direction, impart good teaching practices, and assist preservice teachers in overcoming problems, augmenting their self-efficacy.
  • Foster a growth mindset culture within teacher education programs: Teacher education programs should continuously emphasize that teaching skills can be developed over time. Thus, highlighting success stories and providing mentorship opportunities can help alleviate self-doubt and encourage preservice teachers to embrace challenges in their teaching practice.
  • Self-Reflection Practices: Preservice teachers should be encouraged to engage in self-reflection regarding their teaching beliefs and practices. This can help them identify areas for improvement and build a growth mindset.
  • Provide access to resources and tools: This can help preservice teachers effectively engage students in mathematics. This includes training on the use of manipulatives, technology, and other instructional materials that can enhance understanding and interest in the subject.
Therefore, by implementing these recommendations, educational institutions can better prepare preservice mathematics teachers, ultimately leading to improved student outcomes in mathematics education.

Author Contributions

Conceptualization, B.E.O.; methodology, W.H., B.E.O. and K.S.; validation, W.H., B.E.O. and K.S.; formal analysis, W.H. and B.E.O.; investigation, B.E.O.; data curation, W.H., B.E.O. and K.S.; writing—original draft preparation, B.E.O.; writing—review and editing, W.H., B.E.O. and K.S.; supervision, W.H., B.E.O. and K.S. All authors have read and agreed to the published version of the manuscript.

Funding

This research received no funding.

Institutional Review Board Statement

Ethical clearance was sorted and obtained from INTER-FACULTY HUMAN RESEARCH ETHICS COMMITTEE (IFHREC) HEN001-24 (Project) 9 September 2024.

Informed Consent Statement

Informed consent was obtained from all subjects involved in the study.

Data Availability Statement

The data are contained within the article, and more are available upon request.

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Enochs, L.G.; Smith, P.L.; Huinker, D. Establishing factorial validity of the mathematics teaching efficacy beliefs instrument. Sch. Sci. Math. 2000, 100, 194–202. [Google Scholar] [CrossRef]
  2. Byrd, K.O.; Giles, R.M. Preservice Teachers’ Self-Efficacy for Teaching Mathematics. SRATE J. 2023, 32, 1–11. [Google Scholar]
  3. Alghamdi, A.A. Exploring early childhood teachers’ beliefs about STEAM education in Saudi Arabia. Early Child. Educ. J. 2023, 51, 247–256. [Google Scholar] [CrossRef]
  4. Swars, S.; Hart, L.C.; Smith, S.Z.; Smith, M.E.; Tolar, T. A longitudinal study of elementary pre-service teachers’ mathematics beliefs and content knowledge. Sch. Sci. Math. 2007, 107, 325–335. [Google Scholar] [CrossRef]
  5. Lee, C.W.; Walkowiak, T.A.; Nietfeld, J.L. Characterization of mathematics instructional practises for prospective elementary teachers with varying levels of self-efficacy in classroom management and mathematics teaching. Math. Educ. Res. J. 2017, 29, 45–72. [Google Scholar] [CrossRef]
  6. Olawale, B.E.; Hendricks, W. Mathematics teachers’ self-efficacy beliefs and its relationship with teaching practices. EURASIA J. Math. Sci. Technol. Educ. 2024, 20, em2392. [Google Scholar] [CrossRef]
  7. Trice, A.D.; Ogden, E.D. Correlates of mathematics anxiety in first-year elementary school teachers. Educ. Res. Q. 1986, 11, 3–4. [Google Scholar]
  8. Giles, R.; Byrd, K.; Bendolph, A. An investigation of elementary preservice teachers’ self-efficacy for teaching mathematics. Cogent Educ. 2016, 3, 1160523. [Google Scholar] [CrossRef]
  9. Zee, M.; Koomen, H.M.Y. Teacher Self-Efficacy and Its Effects on Classroom Processes, Student Academic Adjustment, and Teacher Well-Being: A Synthesis of 40 Years of Research. Rev. Educ. Res. 2016, 86, 981–1015. [Google Scholar] [CrossRef]
  10. Morris, D.; Usher, E.; Chen, J. Reconceptualizing the Sources of Teaching Self-Efficacy: A Critical Review of Emerging Literature. Educ. Psychol. Rev. 2017, 29, 795–833. [Google Scholar] [CrossRef]
  11. Olawale, B.E.; Hendricks, W. Self-efficacy and Academic Performance of Mathematics Students in a South African University during the COVID-19 Pandemic. Int. J. Math. Educ. Sci. Technol. 2022, 53, 573–581. [Google Scholar] [CrossRef]
  12. Korkmaz, F.; Ünsal, S. Developing the Scale of Teacher Self-Efficacy in Teaching Process. Eur. J. Educ. Res. 2016, 5, 73–83. [Google Scholar] [CrossRef]
  13. Bandura, A. Self-efficacy. In Encyclopedia of Human Behavior; Academic Press: New York, NY, USA, 1994; pp. 71–81. [Google Scholar]
  14. Kahyaoğlu, M.; Fire, S. Opinions of primary school teacher candidates on their professional self-efficacy. Kastamonu J. Educ. 2007, 15, 73–84. [Google Scholar]
  15. Bandura, A.; Adams, N. Analysis of Self-Efficacy Theory of behavior change. Cogn. Ther. Res. 1997, 1, 287–310. [Google Scholar] [CrossRef]
  16. Bandura, A. Social Foundations of Thought and Action: A Social Cognitive Theory; Prentice Hall: Englewood Cliffs, NJ, USA, 1986. [Google Scholar]
  17. Tschannen-Moran, M.; Hoy, A.W.; Hoy, W.K. Teacher efficacy: Its meaning and measure. Rev. Educ. Res. 1998, 68, 202–248. [Google Scholar] [CrossRef]
  18. Avery, L.M.; Meyer, D.Z. Teaching science as science is practiced: Opportunities and limits for enhancing preservice elementary teachers’ self-efficacy for science and science teaching. Sch. Sci. Math. 2012, 112, 395–409. [Google Scholar] [CrossRef]
  19. McLaughlin, D. Investigating preservice teachers’ self-efficacy through Saturday science. J. Coll. Sci. Teach. 2015, 45, 77–83. [Google Scholar] [CrossRef]
  20. Matthews, S.; Boden, M.; Visnovska, J. Engaging Pre-Service Non-Specialist Teachers in Teaching Mathematics Using Embodied Technology Tools. In Paper presented at the Annual Meeting of the Mathematics Education Research Group of Australasia (MERGA); ERIC: Auckland, New Zealand, 2018. [Google Scholar]
  21. Olawale, B. Teacher Quality and Learner Achievement in South African Schools. In Academic Performance—Students, Teachers and Institutions on the Stage; IntechOpen: London, UK, 2023; pp. 1–18. [Google Scholar]
  22. Olawale, B.E. Impact of Pre-Service Teacher Education Programme on Mathematics Student Teachers’ Teaching Practices during School Experiences. Educ. Sci. 2024, 14, 762. [Google Scholar] [CrossRef]
  23. Morris, A.; Hiebert, J.; Spitzer, S. Mathematical knowledge for teaching in planning and evaluating instruction: What can pre-service teachers learn? J. Res. Math. Educ. 2009, 40, 491–529. [Google Scholar] [CrossRef]
  24. Hine, G.S. Strengthening pre-service teachers’ mathematical content knowledge. J. Univ. Teach. Learn. Pract. 2015, 12, 1–16. [Google Scholar] [CrossRef]
  25. Shulman, L.S. Knowledge and teaching: Foundations of the new reform. Harv. Educ. Rev. 1987, 57, 1–22. [Google Scholar] [CrossRef]
  26. Shulman, L.S. Knowledge and teaching: Foundations of the new reform. In Learners and Pedagogy; SAGE: London, UK, 1999; pp. 61–77. [Google Scholar]
  27. Darling-Hammond, L. Doing What Matters Most: Investing in Quality Teaching; National Commission on Teaching & America’s Future, Kutztown Distribution Center: Kutztown, PA, USA, 1997. [Google Scholar]
  28. Ball, D.L.; Hill, H.; Bass, H. Knowing mathematics for teaching: Who knows mathematics well enough to teach third grade, and how can we decide? Am. Educ. 2005, 29, 14–46. [Google Scholar]
  29. Harris, K.L.; Jensz, F. The Preparation of Mathematics Teachers in Australia: Meeting the Demand for Suitably Qualified Mathematics Teachers in Secondary Schools; The University of Melbourne: Melbourne, Australia, 2006. [Google Scholar]
  30. Norton, S. How deeply and how well? How ready to teach mathematics after a one year program? Math. Educ. Spec. Educ. 2010, 12, 65–84. [Google Scholar]
  31. Miller, K.W.; Davidson, D.M. What makes a secondary school science and/or mathematics teacher “highly qualified”? Sci. Educ. 2006, 15, 56–59. [Google Scholar]
  32. Olawale, B.E. Democratic Citizenship in Mathematics Teachers’ Preparation in South African Universities, Alice. Ph.D. Thesis, University of Fort Hare, Dikeni, South Africa, 2021. [Google Scholar]
  33. Olawale, B.E. Democratic citizenship in mathematics teacher preparation in South African universities: Contradictions in theory and practice. Int. J. Pedagog. Teach. Educ. 2022, 6, 126–139. [Google Scholar] [CrossRef]
  34. Shulman, L.S. Those who understand: Knowledge growth in teaching. Educ. Res. 1986, 15, 4–14. [Google Scholar] [CrossRef]
  35. Ebby, C.B. Learning to teach mathematics differently: The interaction between coursework and fieldwork for preservice teachers. J. Math. Teach. Educ. 2000, 3, 69–97. [Google Scholar] [CrossRef]
  36. Shulman, L. Knowledge and Teaching: Foundations of the New Reform; Jossey Bass: San Francisco, CA, USA, 2004. [Google Scholar]
  37. Liston, D.P.; Zeichner, K.M. Reflective teaching and action research in preservice teacher education. J. Educ. Teach. 1990, 16, 235–254. [Google Scholar] [CrossRef]
  38. Zeichner, K. Rethinking connections between campus courses and field experiences in college- and university-based teacher education. J. Teach. Educ. 2010, 61, 89–99. [Google Scholar] [CrossRef]
  39. Schunk, D.H.; DiBenedetto, M.K. Self-Efficacy and Human Motivation; Elsevier: Amsterdam, The Netherlands, 2021; Volume 8, pp. 153–179. [Google Scholar] [CrossRef]
  40. Bandura, A. Self-Efficacy: The Exercise of Control; Freeman: New York, NY, USA, 1997. [Google Scholar]
  41. Pajares, F. Overview of Social Cognitive Theory and Self-Efficacy. 2002. Available online: http://people.wku.edu/richard.miller/banduratheory.pdf (accessed on 23 August 2024).
  42. Park, Y.S.; Konge, L.; Artino, A.R., Jr. The positivism paradigm of research. Acad. Med. 2020, 95, 690–694. [Google Scholar] [CrossRef]
  43. Creswell, J.W.; Creswell, J.D. Research Design: Qualitative, Quantitative, and Mixed Methods Approaches, 5th ed.; SAGE: Los Angeles, CA, USA, 2018. [Google Scholar]
  44. Croucher, S.M.; Cronn-Mills, D. Experimental Design. In Understanding Communication Research Methods; Routledge: London, UK, 2021; pp. 249–263. [Google Scholar] [CrossRef]
  45. Creswell, J.; Clark, V.P. Designing and Conducting Mixed Methods Research; Sage: New York, NY, USA, 2018. [Google Scholar]
  46. Hartas, D. Educational Research and Inquiry: Qualitative and Quantitative Approaches; Bloomsbury Publishing: London, UK, 2015. [Google Scholar]
  47. Iliyasu, R.; Etikan, I. Comparison of quota sampling and stratified random sampling. Biom. Biostat. Int. J. 2021, 10, 24–27. [Google Scholar] [CrossRef]
  48. Arrington, N.M. Enhancing preservice teachers’ self-efficacy for teaching diverse learners: Capturing young students’ attention through a read-a-loud and music. J. Scholarsh. Teach. Learn. 2023, 23, 76–95. [Google Scholar] [CrossRef]
  49. Özben; Kilicoglu, E. The development process of classroom teacher candidates for teaching mathematics: Self-efficacy, anxiety and professional belief. Particip. Educ. Res. 2021, 8, 129–146. [Google Scholar] [CrossRef]
  50. Woodcock, S.; Sharma, U.; Subban, P.; Hitches, E. Teacher self-efficacy and inclusive education practices: Rethinking teachers’ engagement with inclusive practices. Teach. Teach. Educ. 2022, 117, 103802. [Google Scholar] [CrossRef]
  51. Dogutas, A. Self-efficacy beliefs of teacher candidates on readiness to teaching profession at a university in Turkey. Igdir Univ. J. Soc. Sci. 2016, 9, 1–24. [Google Scholar]
  52. Frazier, R.; Bendixen, L.D.; Hoskins, W. Exploring the role of self-efficacy in prospective teachers’ career decision-making. J. Ethnogr. Qual. Res. 2019, 13, 261–271. [Google Scholar]
  53. Zuya, H.E.; Kwalat, S.K.; Attah, B.G. Pre-Service Teachers’ Mathematics Self-Efficacy and Mathematics Teaching Self-Efficacy. J. Educ. Pract. 2016, 7, 93–98. [Google Scholar]
  54. Ekmekci, A.; Corkin, D.; Papakonstantinou, A. The relationship between teacher related factors and mathematics teachers’ educational beliefs about mathematics. In Proceedings of the 42nd Annual Meeting of the Research Council on Mathematics Learning, Las Vegas, NV, USA, 26–28 February 2015. [Google Scholar]
  55. Johnson, A.L.; Lockee, B.B. Self-efficacy research in online learning. In Self-Efficacy in Instructional Technology Contexts; Springer Nature: New York, NY, USA, 2018; pp. 3–13. [Google Scholar] [CrossRef]
Education 14 01262 g001
Figure 1. Self-efficacy theory [13].
Figure 1. Self-efficacy theory [13].
Education 14 01262 g001
Education 14 01262 g002
Figure 2. Personal Mathematics Teaching Efficacy (PMTE) results.
Figure 2. Personal Mathematics Teaching Efficacy (PMTE) results.
Education 14 01262 g002
Education 14 01262 g003
Figure 3. Mathematics Teaching Outcome Expectancy (MTOE) results.
Figure 3. Mathematics Teaching Outcome Expectancy (MTOE) results.
Education 14 01262 g003
Table 1. Descriptive statistics of high school preservice mathematics teachers.
Table 1. Descriptive statistics of high school preservice mathematics teachers.
AttributeVariablesFreq (f)Percent (%)
GenderFemale4266.7
Male2133.3
Age19–20011.6
21–225282.5
23–240812.7
25 and above023.2
Subject CombinationMathematics + Physical Sciences3961.9
Mathematics + Life Sciences2438.1
Total 63100
Table 2. Personal Mathematics Teaching Efficacy (PMTE) subscale results.
Table 2. Personal Mathematics Teaching Efficacy (PMTE) subscale results.
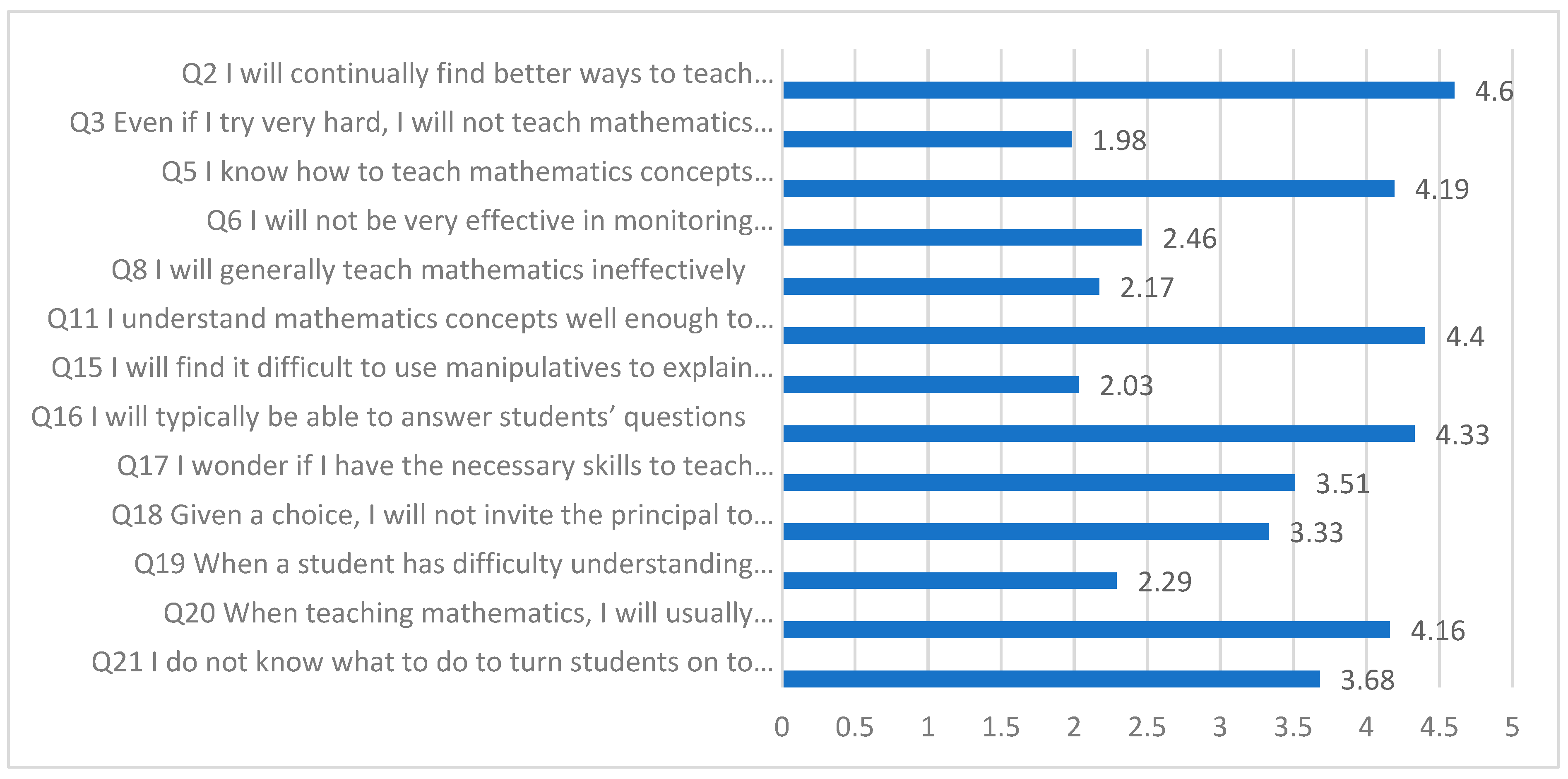
Question NumberItem Mean x ¯ Standard Deviation (SD)Rank
Q2“I will continually find better ways to teach mathematics” 4.600.7941
Q3 *“Even if I try very hard, I will not teach mathematics as well as I will do most subjects”1.980.31413
Q5“I know how to teach mathematics concepts effectively” 4.190.0604
Q6 *“I will not be very effective in monitoring mathematics activities”2.460.5339
Q8 *“I will generally teach mathematics ineffectively”2.170.66111
Q11“I understand mathematics concepts well enough to be effective in teaching high school mathematics”4.400.3702
Q15 *“I will find it difficult to use manipulatives to explain to students why mathematics works”2.030.60612
Q16“I will typically be able to answer students’ questions” 4.330.0473
Q17 *“I wonder if I have the necessary skills to teach mathematics” 3.510.4137
Q18 *“Given a choice, I will not invite the principal to evaluate my mathematics teaching”3.330.8678
Q19 *“When a student has difficulty understanding mathematics concepts, I will usually be at a loss as to how to help the student understand it better”2.290.63110
Q20“When teaching mathematics, I will usually welcome student questions”4.160.1445
Q21 *“I do not know what to do to turn students on to mathematics”3.680.6446
Note. Reverse-scored items are indicated with an asterisk (*).
Table 3. Mathematics Teaching Outcome Expectancy (MTOE) subscale results.
Table 3. Mathematics Teaching Outcome Expectancy (MTOE) subscale results.
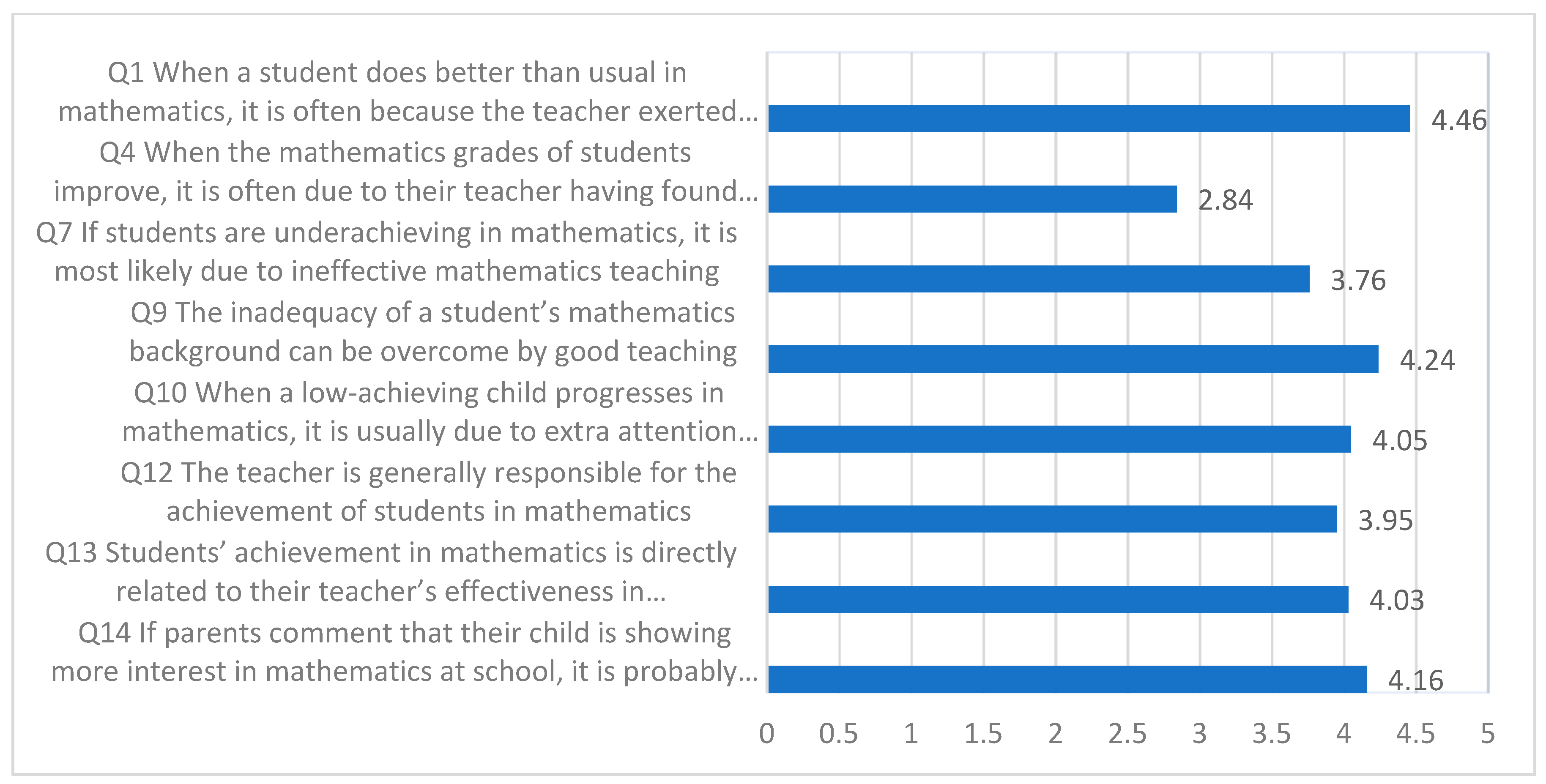
Question NumberItem Mean x ¯ Standard Deviation (SD)Rank
Q1“When a student does better than usual in mathematics, it is often because the teacher exerted a little extra effort”4.460.6001
Q4“When the mathematics grades of students improve, it is often due to their teacher having found a more effective teaching approach”2.840.8338
Q7“If students are underachieving in mathematics, it is most likely due to ineffective mathematics teaching”3.760.5427
Q9“The inadequacy of a student’s mathematics background can be overcome by good teaching”4.240.4892
Q10“When a low-achieving child progresses in mathematics, it is usually due to extra attention given by the teacher”4.050.2374
Q12“The teacher is generally responsible for the achievement of students in mathematics”3.950.6606
Q13“Students’ achievement in mathematics is directly related to their teacher’s effectiveness in mathematics teaching”4.030.3675
Q14“If parents comment that their child is showing more interest in mathematics at school, it is probably due to the performance of the child’s teacher”4.160.3823
Table 4. Differences in efficacy and expectancy of preservice mathematics teachers based on gender.
Table 4. Differences in efficacy and expectancy of preservice mathematics teachers based on gender.
Variable Female (n = 42)Male (n = 21)
x ¯ SD x ¯ SD
Preservice mathematics teaching self-efficacy in teaching mathematics 3.960.773.540.69
Preservice mathematics teaching outcome expectancy in teaching mathematics4.180.673.980.80
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Hendricks, W.; Olawale, B.E.; Saddiq, K. An Investigation of High School Preservice Teachers’ Self-Efficacy in Teaching Mathematics. Educ. Sci. 2024, 14, 1262. https://doi.org/10.3390/educsci14111262

AMA Style

Hendricks W, Olawale BE, Saddiq K. An Investigation of High School Preservice Teachers’ Self-Efficacy in Teaching Mathematics. Education Sciences. 2024; 14(11):1262. https://doi.org/10.3390/educsci14111262

Chicago/Turabian Style

Hendricks, Winston, Babawande Emmanuel Olawale, and Khalid Saddiq. 2024. "An Investigation of High School Preservice Teachers’ Self-Efficacy in Teaching Mathematics" Education Sciences 14, no. 11: 1262. https://doi.org/10.3390/educsci14111262

APA Style

Hendricks, W., Olawale, B. E., & Saddiq, K. (2024). An Investigation of High School Preservice Teachers’ Self-Efficacy in Teaching Mathematics. Education Sciences, 14(11), 1262. https://doi.org/10.3390/educsci14111262

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop