Analysis of Problem Posing Using Different Fractions Meanings
Abstract
:1. Introduction
- To determine the ability of pupils in posing problems related to fractions (RO1);
- To examine the ability that pupils have in solving problems when posing them (RO2);
- To analyze the predilection on the type of problem posed. That is, if pupils tend to formulate problems of a specific type or context (RO3);
- To study the effectiveness of the provided references. That is, if the type of given reference helps pupils to pose and solve a problem and if the given reference evokes the formulation of a specific type of fraction problem (RO4).
2. Theoretical Framework
2.1. Problem Posing
- Problem posing can increase the mathematical knowledge, since the task of creating or formulating a new problem allows the pupils to establish relationships between the different phases of problem solving [19]. In fact, thanks to problem posing, pupils reach complex reflection levels, reaching a reasoning stage that makes the construction of mathematical knowledge possible [20]. Moreover, problem posing allows avoidance or overcoming mathematical mistakes coming from the operative phase of problem resolution since pupils choose the proper information and data to operate later in the problem [21];
- Other studies establish a very positive and direct relationship between the task of problem posing and the creativity of pupils since fluency, flexibility and originality are proper features when formulating problems [18];
- Finally, problem posing is a great evaluation task for teachers, since it allows them to see pupils’ mathematical knowledge, to analyze their processes of reasoning and to assess their conceptual development [17].
- No reference: pupils should pose a problem freely, but including a fraction in the formulation;
- Visual reference: pupils are given an image or a graphic representation (as normally appear in primary schools books and resources) from which they should pose a related problem. These references are used with the objective of evoking a specific type of fractions problems;
- Written reference: pupils are given an operation with fractions and they should formulate a problem that could be solved using this operation.
2.2. Fractions Problems
- Part-whole with continuous unit (PW): This is the most basic and frequent fraction meaning. It refers to a whole divided into equal parts and indicates the relation that exists between a number of parts and the total.
- Part-whole with discrete unit or part-set meaning (PS): This meaning considers a unit formed by disjointed elements, that is, a set or group of items that is divided into subgroups with equal number of items in each of them.
- Quotient: This meaning is applied in contexts of distribution, when a whole or magnitude is equally distributed or divided into a number of parts of the same or different magnitude. In this way, two related but different submeanings can be established:
- Division of two numbers (D2N), when the whole and the parts have different units, that is, they are different magnitudes. Then, it is possible to consider the fraction as a non-exact division that is not necessarily done, but simply indicated [13].
- Rational number (RN), when the whole and the parts are referred to the same magnitude. Then, this meaning relates the idea of fraction with that of decimal numbers.
- Ratio: Other meaning is stated when two quantities, of the same or different type, are compared without giving an idea of number. This use of the fractions presents difficulties on many pupils, since this notion is normally shown as a calculation, corresponding to an algorithm, without being supported by graphic representations. Due to these difficulties, in this study this meaning is not considered, because it is not in the scope of 6th course of primary school where this study is set out.
- Operator: In this meaning, the fraction acts over a quantity through operations of division and multiplication, in order to transform this quantity in a new one. This meaning is associated with part-set one since the operation is always performed in a set. As occurs with the meaning of the fraction as a ratio, this meaning is many times seen as an algorithm without representing and understanding it.
- Measurement: Finally, this meaning arises from comparing two magnitudes, where one of them is the model taken to measure, and the other one is the one to be measured. Commonly, this meaning is confused with the idea of fraction as a rational number when using measurement units. For instance, 0.75 liters of water do not represent a fraction as a measurement, because liter is the capacity of the bottle, not a part from the whole, as it is a bottle whose capacity is 1 liter and is filled up its .
- Part-whole: This meaning is equivalent to the part-whole with continuous unit. An example of this type of problems could be “My brother has eaten and my father . Which fraction of pizza is remaining?”
- Part-set: This meaning is equivalent to the part-whole with discrete unit. An example of this type of problems could be “Mary has 25 sweets and she gives of sweets to her best friend, Kate. How many sweets does Kate have?”
- Division of two numbers: This meaning is equivalent to the quotient when numerator and denominator have different unit. An example of this type of problems could be “A teacher has 4 pieces of chocolate and he wants to divide them up his 12 pupils. How many pieces of chocolate will each pupil receive?”
- Rational number: This meaning is equivalent to the quotient when numerator and denominator share the same unit, that is they work as a magnitude and the fraction is related to a decimal number. An example of this type of problems could be “In a perfumery there are 30 liters of perfume and the workers have to distribute them into bottles of liters. How many bottles will be needed?”
3. Methodology
3.1. Participants
3.2. Instrument
4. Results and Discussion
- Pizza: Great invention, great part-whole evocation as expected.
- Set of points: Great invention, great part-set evocation as expected.
- Circular graph: Good invention, great part-whole evocation as expected.
- Rectangular graph: Good invention, good PW evocation as expected and also some part-set evocation.
- Set of candies: Medium level of invention, great part-set evocation as expected.
- Apples and boxes: Medium invention and evokes division of two number notion as expected but also part-set due the similarity of the reference.
- Number line: Difficult to invent but evokes rational number type as expected.
- Jar: Low level of invention but does not evoke rational number type as expected.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Castro, E. La invención de problemas y sus ámbitos de investigación. In Investigaciones en Pensamiento Numérico y Al-Gebraico e Historia de la Matemática y Educación Matemática; Universidad de Granada: Granada, Spain, 2011; pp. 1–15. [Google Scholar]
- Kilpatrick, J. Problem formulating: Where do good problems come from? In Cognitive Science and Mathematics Education; Psychology Press: Hove, UK, 1987; pp. 123–147. [Google Scholar]
- Polya, G. How to Solve It; Princeton University Press: Princeton, NJ, USA, 1957. [Google Scholar]
- Real Decreto 126/2014, de 28 de Febrero, por el que se Establece el Currículo Básico de la Educación Primaria; Ministerio de Educación: Madrid, Spain, 2014.
- Reyna-Galván, K. Un Acercamiento al Conocimiento de la Fracción en Estudiantes de Quinto Grado de Educación Primaria, Bajo los Significados de parte todo, Medida y Cociente. Ph.D. Thesis, Benemérita y Centenaria Escuela Normal del Estado de San Luis Potosí, San Luis, Mexico, 2020. [Google Scholar]
- Avila, A. Significados, representaciones y lenguaje: Las fracciones en tres generaciones de libros de texto para pri-maria. Educ. Mat. 2019, 31, 22–60. [Google Scholar] [CrossRef]
- Cruz, M.M. Enseñanza Del Concepto De Fracción: Prácticas Educativas Del Maestro De Matemáticas De Escuela Ele-mental; University of Puerto Rico, Rio Piedras (Puerto Rico): San Juan, Puerto Rico, 2018. [Google Scholar]
- Kieren, T.E. Personal knowledge of rational numbers: Its intuitive and formal development. In Number Concepts and Operations in the Middle Grades; National Council of Teachers of Mathematics, Inc.: Reston, VA, USA, 1988; pp. 162–181. [Google Scholar]
- Getenet, S.; Callingham, R. Teaching fractions for understanding: Addressing interrelated concepts. In Proceedings of the 40th Annual Conference of the Mathematics Education Research Group of Australasia, Melbourne, VIC, Australia, 2–6 July 2017; pp. 277–284. [Google Scholar]
- García, Y.J.R. Concepciones sobre las fracciones en docentes en formación en el área de matemática. Omnia 2011, 17, 11–33. [Google Scholar]
- Behr, M.J.; Lesh, R.; Post, T.; Silver, E.A. Rational number concepts. In Acquisition of Mathematics Concepts and Processes; Academic Press: New York, NY, USA; London, UK, 1983; pp. 91–126. [Google Scholar]
- Ni, Y. Semantic domains of rational numbers and the acquisition of fraction equivalence. Contemp. Educ. Psychol. 2001, 26, 400–417. [Google Scholar] [CrossRef] [PubMed]
- Da Silva, M.J.F. Investigando Saberes de Professores do Ensino Fundamental com Enfoque em Números Fracionários para a Quinta Série; Editora Edgard Blücher: Sao Paulo, Brazil, 2017. [Google Scholar]
- Lamon, S.J. Presenting and representing: From fractions to rational numbers. In The Roles of Representation in School Mathematics; National Council of Teachers of Mathematics: Reston, VA, USA, 2001; pp. 146–165. [Google Scholar]
- Perera, P.B.; Valdemoros, M.E. Propuesta didáctica para la enseñanza de las fracciones en cuarto grado de educación primaria. In Investigación en Educación Matemática XI; SEIEM: San Cristóbal de la Laguna, Tenerife, 2007; pp. 209–218. [Google Scholar]
- Ayllón, M.F.; Gómez, I.A. La invención de problemas como tarea escolar. Esc. Abierta 2014, 17, 29–40. [Google Scholar] [CrossRef]
- Ayllón, M.F. Invención de Problemas con Números Naturales, Enteros Negativos y Racionales: Tarea para Profesores de Educación Primaria en Formación; Trabajo de investigación tutelada, Universidad de Granada: Granada, Spain, 2005. [Google Scholar]
- Silver, E.A. On mathematical problem posing. Learn. Math. 1994, 14, 19–28. [Google Scholar]
- Davison, D.M.; Pearce, D.L. Using Writing Activities to Reinforce Mathematics Instruction. Arith. Teach. 1988, 35, 42–45. [Google Scholar] [CrossRef]
- Freudenthal, H. Mathematics as an Educational Task; Reidel Publ. Co.: Dordrecht, The Netherlands, 1973. [Google Scholar]
- Brown, S.I.; Walter, M.I. Problem Posing; Lawrence Erlbaum Associates: Hillsdale, NJ, USA, 1993. [Google Scholar]
- Christou, C.; Mousoulides, N.; Pittalis, M.; Pitta-Pantazi, D.; Sriraman, B. An empirical taxonomy of problem posing processes. ZDM 2005, 37, 149–158. [Google Scholar] [CrossRef]
- Stoyanova, E. Problem posing in Mathematics Classrooms. In Research in Mathematics Education: A Contemporary Perspective; McIntosh, A., Ellerton, N., Eds.; Edith Cowan University, MASTEC: Perth, WA, USA, 1998; pp. 164–185. [Google Scholar]
- Ball, D.L.; Lubienski, S.T.; Mewborn, D.S. Research on teaching mathematics: The unsolved problem of teachers’ mathematical knowledge. In Handbook of Research on Teaching; Richardson, V., Ed.; American Educational Research Association: Washington, DC, USA, 2001; pp. 433–456. [Google Scholar]
- Borko, H.; Eisenhart, M.; Brown, C.A.; Underhill, R.G.; Jones, D.; Agard, P.C. Learning to teach hard mathe-matics: Do novice teachers and their instructors give up too easily? J. Res. Math. Educ. 1992, 23, 194–222. [Google Scholar] [CrossRef]
- De Corte, E.; Greer, B.; Verschaffel, L. Mathematics teaching and learning. In Handbook of Educational Psychology; Berliner, D.C., Calfee, R.C., Eds.; Macmillan Library Reference; Prentice Hall International: New York, NY, USA, 1996; pp. 491–549. [Google Scholar]
- Tirosh, D. Enhancing prospective teachers’ knowledge of children’s conceptions: The case of division of fractions. J. Res. Math. Educ. 2000, 31, 5–25. [Google Scholar] [CrossRef]
- Barlow, A.T.; Cates, J.M. The impact of problem posing on elementary teachers’ beliefs about mathematics and mathematics teaching. Sch. Sci. Math. 2006, 106, 64–73. [Google Scholar] [CrossRef]
- Koichu, B.; Harel, G.; Manaster, A. Ways of thinking associated with mathematics teachers’ problem posing in the context of division of fractions. Instr. Sci. 2013, 41, 681–698. [Google Scholar] [CrossRef]
- Ma, L. Knowing and Teaching Elementary Mathematics: Teachers’ Knowledge of Fundamental Mathematics in China and the United States; Lawrence Erlbaum: Hillsdale, NJ, USA, 1999. [Google Scholar]
- Toluk-Ucar, Z. Developing pre-service teachers understanding of fractions through problem posing. Teach. Teach. Educ. 2009, 25, 166–175. [Google Scholar] [CrossRef]
- Kieren, T.E. On the mathematical, cognitive and instructional foundations of the rational numbers. In Number and Measurement: Papers from a Research Workshop; Lesh, R.A., Ed.; ERIC/SMEAC: Athens, GA, USA, 1976; pp. 101–144. [Google Scholar]
- Kieren, T.E. Rational and fractional numbers: From quotient fields to recursive understanding. In Rational Numbers: An Integration of Research; Carpenter, T.P., Fennema, E., Romberg, T.A., Eds.; Erlbaum: Hillsdale, NJ, USA, 1993; pp. 49–84. [Google Scholar]
- Mack, N. Confounding Whole-Number and Fraction Concepts When Building on Informal Knowledge. J. Res. Math. Educ. 1995, 26, 422–441. [Google Scholar] [CrossRef]
- Lamon, S. Teaching Fractions and Ratios for Understanding: Essential Content Knowledge and Instructional Strategies for Teachers; Lawrence Erlbaum: Mahwah, NJ, USA, 1999. [Google Scholar]
- Cázares, J. La invención de problemas en escolares de primaria. Un estudio evolutivo. In Memoria de Investigación de 3° Ciclo; Dpto. Didáctica de la Matemática, Universidad de Granada: Granada, Spain, 2002. [Google Scholar]
| Meaning | Statement | Graphic Representation |
|---|---|---|
| Part-whole (PW) | “Mary has eaten of the pizza” |  |
| Part-set (PS) | “ of the colors are blue” |  |
| Division of two number (D2N) | “Each child receives pieces of chocolate.” | 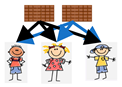 |
| Rational number (RN) | “A bottle of l of capacity.” | 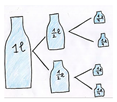 |
| Ratio | “Compare the quantity of cases and pencils” = = ” |  |
| Operator | “Take from 12 cakes” |  |
| Measurement | “The bottle is full up to of its total capacity” |  |
| Nr | Task | Reference Given | Type of Reference | Type of Problem Expected |
|---|---|---|---|---|
| 1 | Invent a problem that includes at least a fraction in its statement. Then, solve it. Explain or draw whatever you need to do the task. | None | Any meaning | |
| 2 | Invent a problem of fractions related to the following image. Then, solve it. Explain or draw whatever you need to do the task. |  | Visual reference: image | Part-whole |
| 3 | Invent a problem of fractions related to the following image. Then, solve it. Explain or draw whatever you need to do the task. |  | Visual reference: image | Part-set |
| 4 | Invent a problem of fractions related to the following image. Then, solve it. Explain or draw whatever you need to do the task. |  | Visual reference: image | Division of two numbers |
| 5 | Invent a problem of fractions related to the following image. Then, solve it. Explain or draw whatever you need to do the task. |  | Visual reference: image | Rational number |
| 6 | Invent a problem of fractions related to the following graphic representation. Then, solve it. Explain or draw whatever you need to do the task. |  | Visual reference: graphic representation | Part-whole |
| 7 | Invent a problem of fractions related to the following graphic representation. Then, solve it. Explain or draw whatever you need to do the task. |  | Visual reference: graphic representation | Part-whole |
| 8 | Invent a problem of fractions related to the following graphic representation. Then, solve it. Explain or draw whatever you need to do the task. |  | Visual reference: graphic representation | Part-set |
| 9 | Invent a problem of fractions related to the following graphic representation. Then, solve it. Explain or draw whatever you need to do the task. |  | Visual reference: graphic representation | Rational number |
| 10 | Invent a problem of fractions related to the following operation. Then, solve it. Explain or draw whatever you need to do the task | – | Written reference:Operation | Any meaning |
| Activity | Well Invented | Well Solved (*) | Wrongly Solved (*) | Wrongly Invented | Not Invented | Total |
|---|---|---|---|---|---|---|
| 1 No help | 67.3% | 66.7% | 33.3% | 32.7% | 0% | 49 |
2  | 66.7% | 78.6% | 21.4% | 33.3% | 0% | 21 |
3  | 42.2% | 73.7% | 26.3% | 55.6% | 2.2% | 45 |
4  | 52.2% | 70.8% | 29.2% | 43.5% | 4.3% | 46 |
5  | 36.9% | 52.9% | 47.1% | 60.9% | 2.2% | 46 |
6  | 56.5% | 80.8% | 19.2% | 39.2% | 4.3% | 46 |
7 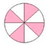 | 54.2% | 69.2% | 30.8% | 45.8% | 0% | 24 |
8  | 61.2% | 63.3% | 36.7% | 34.7% | 4.1% | 49 |
9 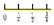 | 11.1% | 60.0% | 40.0% | 73.3% | 15.6% | 45 |
| 10 operation | 26.5% | 61.5% | 38.5% | 65.3% | 8.2% | 49 |
| TOTAL | 46.2% | 68.5% | 31.5% | 49.3% | 4.5% | 420 |
| Activity | Expected | PW | PS | D2N | RN | Others | TOTAL |
|---|---|---|---|---|---|---|---|
| 1 No help | FREE | 38.8% | 42.9% | 0% | 6.1% | 12.2% | 49 |
2  | PW | 85.7% | 4.8% | 0% | 0% | 9.5% | 21 |
3  | PS | 0% | 72.7% | 6.8% | 0% | 20.5% | 44 |
4  | D2N | 0% | 27.3% | 0% | 22.7% | 44 | |
5  | RN | 2.2% | 15.6% | 11.1% | 37.8% | 45 | |
6  | PW | 54.5% | 20.5% | 0% | 0% | 25.0% | 44 |
7  | PW | 75.0% | 4.2% | 0% | 0% | 20.8% | 24 |
8  | PS | 0% | 78.7% | 0% | 0% | 21.3% | 47 |
9  | RN | 2.6% | 13.2% | 0% | 15.8% | 38 | |
| 10 operation | FREE | 42.2% | 26.7% | 0% | 11.1% | 20.0% | 45 |
| TOTAL | 24.9% | 38.7% | 5.5% | 4.7% | 401 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Martinez, S.; Blanco, V. Analysis of Problem Posing Using Different Fractions Meanings. Educ. Sci. 2021, 11, 65. https://doi.org/10.3390/educsci11020065
Martinez S, Blanco V. Analysis of Problem Posing Using Different Fractions Meanings. Education Sciences. 2021; 11(2):65. https://doi.org/10.3390/educsci11020065
Chicago/Turabian StyleMartinez, Silvia, and Vanessa Blanco. 2021. "Analysis of Problem Posing Using Different Fractions Meanings" Education Sciences 11, no. 2: 65. https://doi.org/10.3390/educsci11020065
APA StyleMartinez, S., & Blanco, V. (2021). Analysis of Problem Posing Using Different Fractions Meanings. Education Sciences, 11(2), 65. https://doi.org/10.3390/educsci11020065






