Applying Quantum Mechanics for Extreme Value Prediction of VaR and ES in the ASEAN Stock Exchange †
Abstract
1. Introduction
2. Literature and Critical Thinking
2.1. The Origin of Quantum Distributions
2.2. Quantum Computing in Financial Econometrics
3. The Objective and Scope of Research
4. Methodology
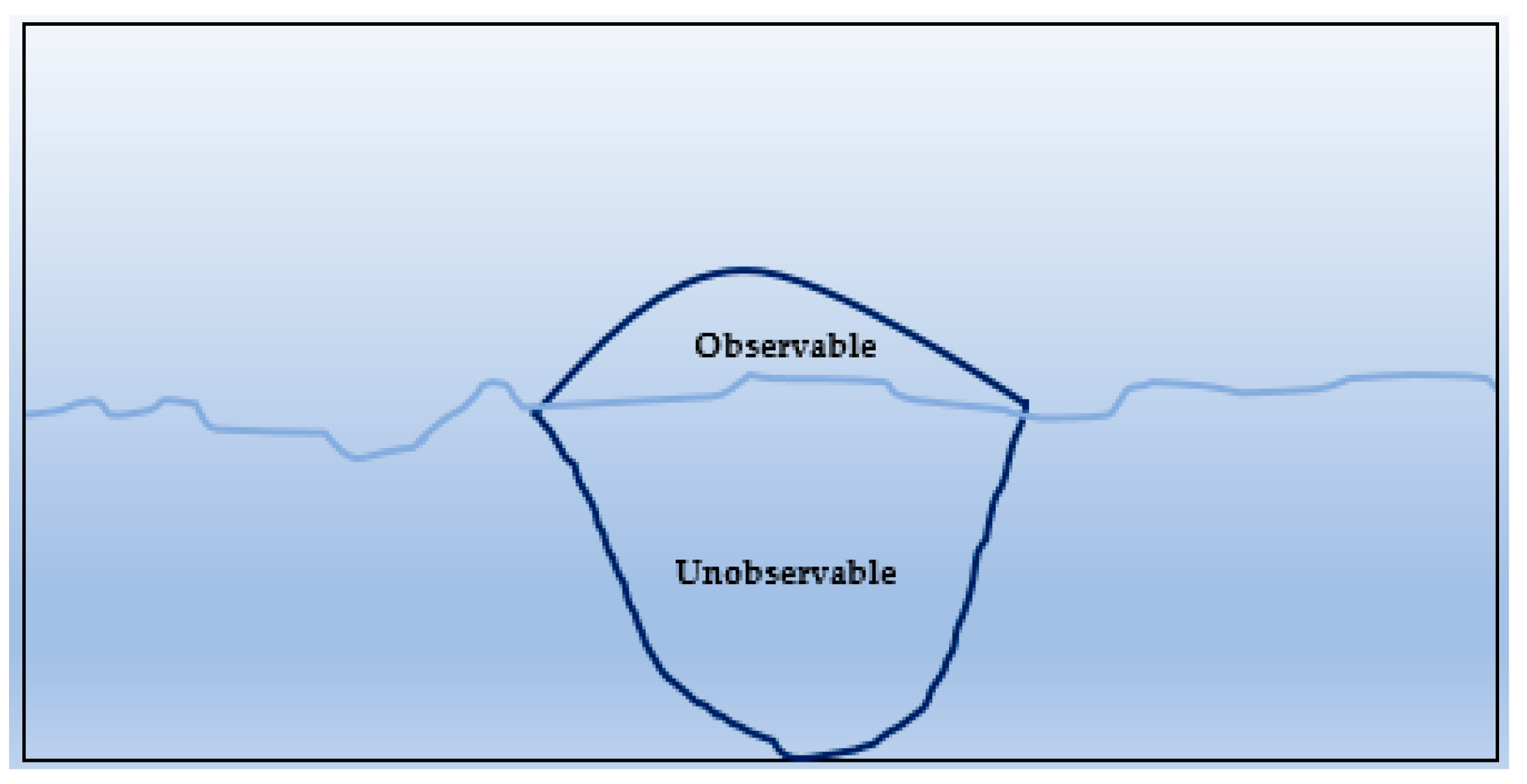
4.1. Quantum Mechanics and Wave Function for Time Series Movement
4.2. Extreme Value Analysis
4.3. Bayesian Inference and Simulations for Value at Risk (VaR) and Expected Shortfall (ES)
4.3.1. The Origin of the Prior Information
4.3.2. The Prior Density for Parameters at the Threshold
4.3.3. Posterior Density Estimations
4.3.4. Risk Measurement
5. Computational and Comparative Results
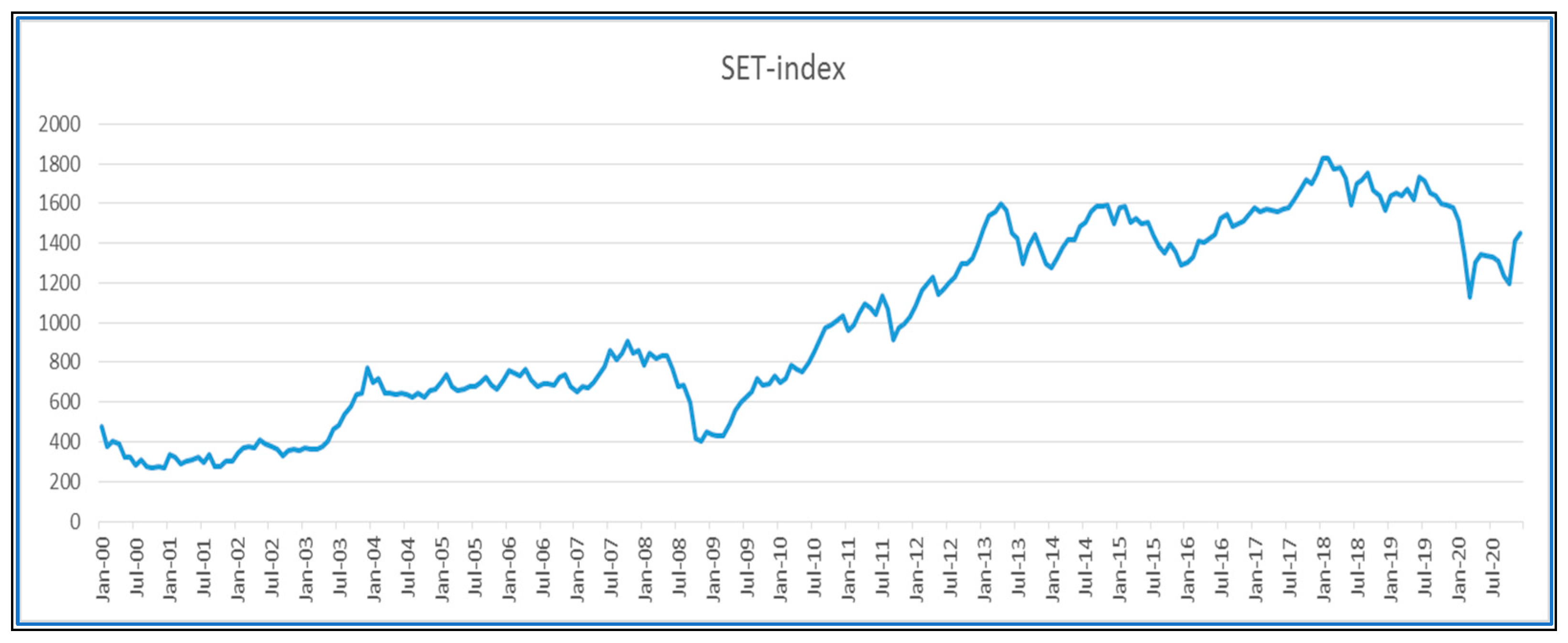
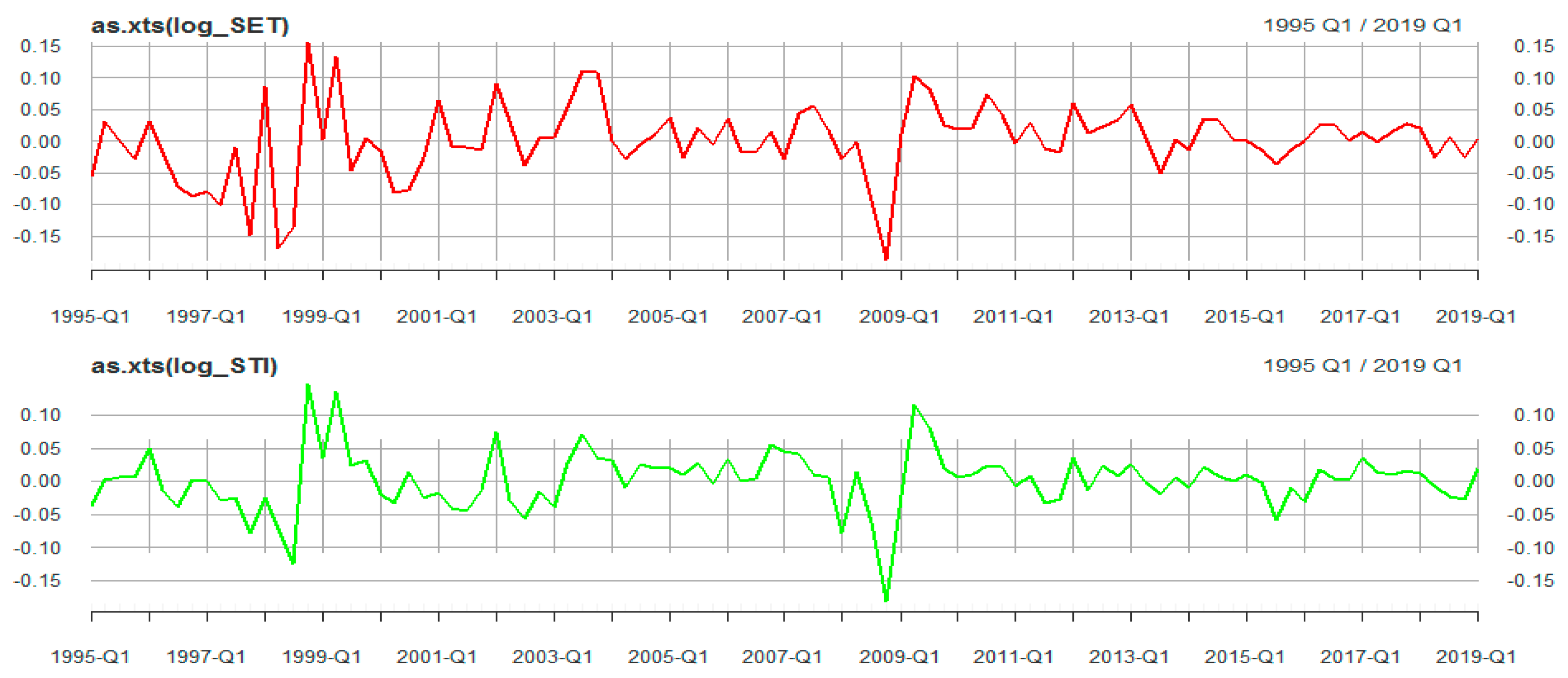
5.1. Data Visualization
5.2. The Distribution Outlook Comparison
5.3. Risk Measures by the Quantum Distribution
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ahn, Kwangwon, MooYoung Choi, Bingcun Dai, Sungbin Sohn, and Biao Yang. 2018. Modeling stock return distributions with a quantum harmonic oscillator. EPL (Europhysics Letters) 120: 38003. [Google Scholar] [CrossRef]
- Assaf, Ata. 2009. Extreme observations and risk assessment in the equity markets of MENA region: Tail measures and Value-at- Risk. International Review of Financial Analysis 18: 109–16. [Google Scholar] [CrossRef]
- Ataullah, Ali, Ian Davidson, and Mark Tippett. 2008. A wave function for stock market returns. Physica A 388: 455–61. [Google Scholar] [CrossRef]
- Ausloos, Marcel, Franck Jovanovic, and Christophe Schinckus. 2016. On the “usual” misunderstandings between econophysics and finance: Some clarifications on modelling approaches and efficient market hypothesis. International Review of Financial Analysis 47: 7–14. [Google Scholar] [CrossRef]
- Behrens, Cibele N. Behrens, Hedibert F. Lopes, and Dani Gamerman. 2004. Bayesian analysis of extreme events with threshold estimation. Statistical Modelling 4: 227–44. [Google Scholar] [CrossRef]
- Bowles, Samuel, and Wendy Carlin. 2020. What students learn in economics 101: Time for a change. Journal of Economic Literature 58: 176–214. [Google Scholar] [CrossRef]
- Bruß, Dagmar, and Norbert Lütkenhaus. 2000. Quantum key distribution: From principles to practicalities. Applicable Algebra in Engineering, Communication and Computing 10: 383–99. [Google Scholar] [CrossRef][Green Version]
- Chaiboonsri, Chukiat, and Satawat Wannapan. 2021. Multi-process analysis and portfolio optimization based on quantum mechanics (QM) under risk management in ASEAN Exchanges: A Case Study of Answering to the E-Commerce and E-Business Direction. In Handbook of Research on Innovation and Development of E-Commerce and E-Business in ASEAN. 2 vols. Hershey: IGI Global. 16p. [Google Scholar] [CrossRef]
- Choustova, Olga. 2007. Toward quantum-like modeling of financial processes. Journal of Physics: Conference Series 70: 012006. [Google Scholar] [CrossRef]
- Choustova, Olga. 2009. Quantum-like viewpoint on the complexity and randomness of the financial market. In Coping with the Complexity of Economics. New Economic Windows. Edited by Marisa Faggini and Thomas Lux. Milano: Springer. [Google Scholar] [CrossRef]
- de Zea Bermudez, P., M. A. Amaral Turkman, and K. F. Turkman. 2001. A predictive approach to tail probability estimation. Extremes 4: 295–314. [Google Scholar] [CrossRef]
- Ekert, Artur K., Bruno Huttner, G. Massimo Palma, and Asher Peres. 1994. Eavesdropping on quantum-cryptographical systems. Physical Review A 50: 1047–56. [Google Scholar] [CrossRef]
- Gençay, Ramazan, and Faruk Selçuk. 2004. Extreme value theory and Value-at-Risk: Relative performance in emerging markets. International Journal of Forecasting 20: 287–303. [Google Scholar] [CrossRef]
- Gonçalves, Carlos Pedro. 2013. Quantum financial economics—Risk and returns. Journal of Systems Science and Complexity 26: 187–200. [Google Scholar] [CrossRef]
- Gonçalves, Carlos Pedro. 2015. Financial market modeling with quantum neural networks. Review of Business and Economics Studies 3: 44–63. [Google Scholar]
- Gonçalves, Carlos Pedro dos Santos, and Carlos Gonçalves. 2008. An evolutionary quantum game model of financial market dynamics-theory and evidence. Capital Markets: Asset Pricing & Valuation 11. [Google Scholar] [CrossRef]
- Griffiths, David J. 1995. Introduction to Quantum Mechanics. Upper Saddle River: Prentice Hall. ISBN 0-13-124405-1. [Google Scholar]
- Hooker, Cliff. 2011. Philosophy of Complex Systems, 1st ed. Edited by Dov M. Gabbay, Paul Thagard and John Woods. Handbook of the Philosophy of Science. vol. 10, ISBN 9780080931227. Available online: https://www.sciencedirect.com/book/9780444520760/philosophy-of-complex-systems (accessed on 2 February 2021).
- Jung, Paul, and Greg Markowsky. 2013. Random walks at random times: Convergence to iterated Levy motion, fractional stable motions. The Annual of Probability 41: 2682–708. [Google Scholar] [CrossRef]
- Kac, Mark. 1947. Random walk and the theory of Brownian motion. The American Mathematical Monthly 54: 369–91. [Google Scholar] [CrossRef]
- Mantegna, Rosario N., and H. Eugene Stanley. 2000. An Introduction to Econophysics: Correlations and Complexity in Finance, 1st ed. Cambridge: Cambridge University Press. ISBN 0-521-62008-2. [Google Scholar]
- Metropolis, Nicholas, Arianna W. Rosenbluth, Marshall N. Rosenbluth, Augusta H. Teller, and Edward Teller. 1953. Equation of state calculations by fast computing machines. Journal of Chemical Physics 21: 1087–92. [Google Scholar] [CrossRef]
- Ogborn, Jon, and Edwin F. Taylor. 2005. Quantum physics explains Newton’s laws of motion. Physics Education 40: 26–34. [Google Scholar] [CrossRef]
- Pero, Siti Darwinda Mohamed, and Laila Suriya Ahmad Apandi. 2018. Malaysia’s leadership role in ASEAN An Assessment. Journal of International Studies 14: 65–79. [Google Scholar]
- Persky, Joseph. 1989. Adam Smith’s invisible hands. Journal of Economic Perspectives 3: 195–201. [Google Scholar] [CrossRef]
- Pickands, James. 1975. Statistical inference using extreme order statistics. The Annals of Statistics 3: 119–31. [Google Scholar]
- Piotrowski, Edward W., and Jan Sładkowski. 2001. Quantum-like approach to financial risk: Quantum anthropic principle. Acta Physica Polonica Series B 32: 3873–79. [Google Scholar]
- Piziak, Robert, and J. J. Mitchell. 2001. Hamiltonian formalism and the state of a physical System. Computers and Mathematics with Applications 42: 793–805. [Google Scholar] [CrossRef]
- Recherches sur la théorie des quanta (Researches on the quantum theory). 1925. Thesis, Paris. Available online: https://aflb.minesparis.psl.eu/LDB-oeuvres/De_Broglie_Kracklauer.pdf (accessed on 2 February 2021).
- Saghiri, Ali Mohammad, M. Daliri Khomami, and Mohammad Reza Meybodi. 2019. Random walk algorithms: Definitions, weaknesses, and learning automata-based approach. In Springer Briefs in Applied Sciences and Technology. Berlin/Heidelberg: Springer, pp. 1–7. [Google Scholar]
- Saperstein, Alvin M. 2010. Beyond uncertainty: Heisenberg, quantum physics, and the bomb. Physics Today 63. [Google Scholar] [CrossRef]
- Schrödinger, Erwin. 1926. An Undulatory Theory of the Mechanics of Atoms and Molecules. Physical Review 28: 1049–70. [Google Scholar] [CrossRef]
- Sijabat, Rosdiana. 2018. Understanding behavioral economics: A narrative perspective. Asian Development Policy Review 6: 77–87. [Google Scholar] [CrossRef]



| LOG_STI (Singapore) | LOG_SET (Thailand) | LOG_PSEI (Philippines) | LOG_PCI (Indonesia) | LOG_FTSE (Malaysia) | |
|---|---|---|---|---|---|
| Mean | 0.001480 | 0.000502 | 0.004399 | 0.010991 | 0.003793 |
| Median | 0.005334 | 0.001854 | 0.011447 | 0.016087 | 0.007192 |
| Maximum | 0.147158 | 0.154828 | 0.147340 | 0.161394 | 0.063923 |
| Minimum | −0.181316 | −0.188425 | −0.166256 | −0.213672 | −0.087201 |
| Std. Dev. | 0.044216 | 0.056605 | 0.048931 | 0.051288 | 0.025348 |
| Skewness | −0.228034 | −0.514831 | −0.455003 | −0.714628 | −1.066256 |
| Kurtosis | 6.995341 | 4.894663 | 4.245375 | 6.527976 | 5.221788 |
| Jarque-Bera | 67.37811 | 19.37479 | 9.912792 | 60.37246 | 39.51644 |
| Probability | 0.000000 | 0.000062 | 0.007038 | 0.000000 | 0.000000 |
| PP-test statistics | −7.761860 | −8.330687 | −8.993564 | −7.475574 | −7.891177 |
| Probability | 0.000000 | 0.000000 | 0.000000 | 0.000000 | 0.000000 |
| Data Distribution Based on Gaussian Random Walk | Data Distribution Based on the Wave Function | ||
|---|---|---|---|
| DIC | DIC | ||
| Thailand | (SET) | −909.6436 | −934.5987 * |
| Singapore | (STI) | −910.8061 | −1089.0160 * |
| Malaysia | (FTSE) | −1288.4080 | −1339.4820 * |
| Philippines | (PSEI) | −925.4325 | −1032.0220 * |
| Indonesia | (PCI) | −960.8347 | −1025.6590 * |
| 2.5% | Mean | 97.5% | ||
|---|---|---|---|---|
| Thailand | VaR at 99% confidence (0.01) | 0.1523 | 0.1940 | 0.2508 |
| Expected shortfall | (−0.1656) | (−0.2201) | (−0.3000) | |
| Singapore | VaR at 99% confidence (0.01) | 0.1287 | 0.1569 | 0.1982 |
| Expected shortfall | (−0.1432) | (−0.1831) | (−0.2472) | |
| Malaysia | VaR at 99% confidence (0.01) | 0.1080 | 0.1256 | 0.1496 |
| Expected shortfall | (−0.1137) | (−0.1361) | (−0.1678) | |
| Philippines | VaR at 99% confidence (0.01) | 0.1399 | 0.1691 | 0.2094 |
| Expected shortfall | (−0.1544) | (−0.1941) | (−0.2529) | |
| Indonesia | VaR at 99% confidence (0.01) | 0.1377 | 0.1702 | 0.2148 |
| Expected shortfall | (−0.1520) | (−0.1960) | (−0.2622) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chaiboonsri, C.; Wannapan, S. Applying Quantum Mechanics for Extreme Value Prediction of VaR and ES in the ASEAN Stock Exchange. Economies 2021, 9, 13. https://doi.org/10.3390/economies9010013
Chaiboonsri C, Wannapan S. Applying Quantum Mechanics for Extreme Value Prediction of VaR and ES in the ASEAN Stock Exchange. Economies. 2021; 9(1):13. https://doi.org/10.3390/economies9010013
Chicago/Turabian StyleChaiboonsri, Chukiat, and Satawat Wannapan. 2021. "Applying Quantum Mechanics for Extreme Value Prediction of VaR and ES in the ASEAN Stock Exchange" Economies 9, no. 1: 13. https://doi.org/10.3390/economies9010013
APA StyleChaiboonsri, C., & Wannapan, S. (2021). Applying Quantum Mechanics for Extreme Value Prediction of VaR and ES in the ASEAN Stock Exchange. Economies, 9(1), 13. https://doi.org/10.3390/economies9010013







