A Wavelet Investigation of Periodic Long Swings in the Economy: The Original Data of Kondratieff and Some Important Series of GDP per Capita
Abstract
1. Introduction
2. Data and Methodology
- -
- England—Index number of commodity prices 1780–1922 (to simplify correct identification, we marked them with a capital letter, and begin with A);
- -
- France—Index number of commodity prices 1858–1922 (B);
- -
- USA—Index number of commodity prices 1791–1922 (C);
- -
- England—Quotations of interest-bearing securities 1816–1922 (D);
- -
- France—Quotations of interest-bearing securities 1814–1922 (E);
- -
- England—Index of Weekly wages in agriculture 1789–1913 (F) and Cotton Textiles 1807–1913 (G);
- -
- France—Foreign trade 1827–1913 in per capita francs (H);
- -
- England—Coal production 1855–1917 in t/1000 inhabitant (I);
- -
- France—Coal consumption 1827–1913 in t/1000 inhabitant (J);
- -
- England—Pig iron production 1840–1914 in t/1000 inhabitant (K);
- -
- England—Lead production 1855–1920 in t/1000 inhabitant (L).
- -
- Brazil (1850–2018; Barro and Ursua 2008);
- -
- France (1280–2018; Ridolfi 2016);
- -
- Germany (1850–2018);
- -
- India (1884–2018);
- -
- Italy (1310–2018; Baffigi 2011; Malanima 2010);
- -
- Japan (1885–2018; Fukao et al. 2015);
- -
- Turkey (1913–2018);
- -
- UK (1252–2018; Broadberry et al. 2015);
- -
- USA (1800–2018; Sutch 2006);
- -
- Former USSR (1885–2018; Gregory 1982 and Markevich and Harrison 2011).
- -
- T = the last term of the discrete series;
- -
- e = Euler’s number (also known as Nepier’s constant equal to 2.71…);
- -
- i = is the conventional for imaginary part;
- -
- = is the radians representation of the frequency (fnt).
- -
- the functions ϕ and φ satisfy conditions (4) and (5).
- -
- j = 1, 2, …, J indexes the maximum scale sustainable with the data to process (each scale represents a fixed interval of frequencies);
- -
- k indexes the translation parameter;
- -
- are the trend smooth coefficients in the wavelet transform capturing the underlying behavior of the data at the coarsest scale;
- -
- are the detail wavelet coefficients representing deviations from the smooth behavior.
3. Results
- -
- e = Euler’s number (also known as Nepier’s constant equal to 2.71…);
- -
- i is the conventional for imaginary part;
- -
- ω is the angular frequency in radians per time unit (equivalent to 2).
- -
- * represent the complex conjugate;
- -
- τ is the parameter to localize the position of the particular daughter wavelet in the time domain by an equal increment of dt (in our case dt = 1);
- -
- s represents the scale value used in the FFT algorithms to evaluate (13) in an efficient way (Torrence and Compo 1998). The choice of the set of scales as fractional powers of 2 defines the wavelet coverage of the series in the frequency domain (Rösch and Schmidbauer 2018).
4. Conclusions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Adelman, Irma. 1965. Long cycles: Fact or artifact? American Economic Review 55: 444–63. [Google Scholar]
- Aftalion, Albert. 1909. La réalité des surproductions générales: Essai d’une théorie des crises générales et périodiques. Revue d’Économie Politique XXIII: 86–87. [Google Scholar]
- Aiyagari, Sudhakar. 1994. On the contribution of technology shocks to business cycles. Federal Reserve Bank of Minneapolis Quarterly Review 18: 22–34. [Google Scholar] [CrossRef]
- Almeshari, Ali, Mohamed Hisham Bin Dato Haji Yahya, Fakarudin Bin Kamarudin, Rosalan Ali, and Sha’ari Abd Hamid. 2023. Liquidity creation, oil term of trade shocks, and growth volatility in Middle Eastern and North African Countries (MENA). Economies 11: 147. [Google Scholar] [CrossRef]
- Anguiar-Conraria, Luis, and Maria Joana Soares. 2011. Business cycles synchronization and the Euro: A wavelet analysis. Journal of Macroeconomics 33: 477–89. [Google Scholar] [CrossRef]
- Aristizabal, Felipe, and Mladen. I. Glavinovic. 2003. Wavelet analysis of nonstationary fluctuations of Monte Carlo-Simulated excitatory postsynaptic currents. Biophysical Journal 85: 2170–85. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Baffigi, Alberto. 2011. Italian National Accounts, 1861–2011. Banca d’Italia Economic History Working Papers 18. Rome: Bank of Italy. [Google Scholar]
- Barro, Robert J., and José F. Ursua. 2008. Macroeconomic crises since 1870. Brooking Economic Papers on Economic Activity, Economic Studies Program 391: 255–350. [Google Scholar]
- Baxter, Marianne, and Robert King. 1999. Measuring business cycles: Approximate band-pass filters for economic time series. Review of Economic and Statistics 81: 575–93. [Google Scholar] [CrossRef]
- Beaudry, Paul, Dana Galizia, and Franck Portier. 2020. Putting the cycle back into business cycle analysis. American Economic Review 110: 1–47. [Google Scholar]
- Belkhir, Mohamed, Sami Ben Naceur, Bertrand Candelon, and Jean-Charles Wijnandts. 2022. Macroprudential policies, economic growth and banking crises. Emerging Markets Review 53: 100936. [Google Scholar] [CrossRef]
- Berry, Brian J. L. 2000. A pacemaker for the long wave. Technological Forecasting and Social Change 63: 1–23. [Google Scholar] [CrossRef]
- Berry, Brian J. L. 2006. Recurrent instabilities in K-wave macrohistory. In Kondratieff Waves, Warfare and World Security. Edited by T. C. Devezas. Amsterdam: IOS Press, pp. 22–29. [Google Scholar]
- Bieshaar, Hans, and Alfred Kleinknecht. 1984. Kondratieff long waves in aggregate output? An econometric test. Konjunkturpolitik 30: 279–303. [Google Scholar]
- Bloomfield, Peter. 2000. Fourier Analysis of Time Series—An Introduction. New York: John Wiley & Sons, Inc. [Google Scholar]
- Box, George P., Gwilym M. Jenkins, Gregory C. Reinsel, and Greta M. Ljung. 2016. Time Series Analysis-Forecasting and Control, 5th ed. Hoboken: John Wiley & Sons. [Google Scholar]
- Broadberry, Stephen, Bruce Campbell, Alexander Klein, Mark Overton, and Bas van Leewen. 2015. British Economic Growth 1278–1870. Cambridge: Cambridge University Press. [Google Scholar]
- Brock, William, Davis W. Dechert, and Blake Lebaron Jose Scheinkman. 1996. A test for independence based on the correlation dimension. Econometric Reviews 15: 197–235. [Google Scholar] [CrossRef]
- Bruce, Andrew, and HongYe Gao. 1996. Applied Wavelet Analysis with S-PLUS. New York: Springer. [Google Scholar]
- Cazelles, Bernard, Mario Chavez, Dominique Berteaux, Frédèric Ménard, Jon Olav Vik, Stéphanie Jenouvrier, and Nils C. Stenseth. 2008. Wavelet analysis of ecological time series. Oecologia 156: 287–304. [Google Scholar] [CrossRef]
- Chan, Kung-Sik, and Brian Ripley. 2022. TSA: Time Series Analysis. R Package Version 1.3.1. Available online: https://CRAN.R-project.org/package=TSA (accessed on 28 July 2023).
- Cheng, Han-Liang, and Nan-Kuang Chen. 2021. A study of financial cycles and the macroeconomy in Taiwan. Empirical Economics 61: 1749–78. [Google Scholar] [CrossRef]
- Christiano, Lawrence J., and Terry J. Fitzgerald. 2003. The band pass filter. International Economic Review 44: 435–65. [Google Scholar] [CrossRef]
- Crowley, Patrick M. 2007. A guide to wavelets for economists. Journal of Economic Surveys 21: 207–67. [Google Scholar] [CrossRef]
- Crowley, Patrick M., and Andrew H. Hallet. 2014. The great moderation under the microscope: Decomposition of macroeconomic cycles in US and UK aggregate demand. In Wavelet Applications in Economics and Finance. Edited by Marco Gallegati and Willi Semmler. Cham: Springer, pp. 47–71. [Google Scholar]
- Darné, Olivier, and Claude Diebolt. 2004. Unit roots and infrequent large shocks: New international evidence on output. Journal of Monetary Economics 51: 1449–65. [Google Scholar] [CrossRef]
- Daubechies, Ingrid. 1990. The wavelet transform time-frequency localization and signal analysis. IEEE Transactions on Information Theory 36: 961–1005. [Google Scholar] [CrossRef]
- Davidson, Paul. 2015. Post Keynesian Theory and Policy—A Realistic Analysis of the Market Oriented Capitalist Economy. Cheltenham: Edward Elgar. [Google Scholar]
- De Wolf, Salomon. 1924. Prosperitats und Depressionsperioden. In Der Lebendige Marxismus: Festgabe zum 70 Geburtstage von Karl Kautsky. Edited by Otto Jensen. Jena: Thuringer Verlagsanstalt, pp. 13–43. [Google Scholar]
- De Wolf, Salomon. 1929. Het Economisch Getij. Amsterdam: Emmering. [Google Scholar]
- Devezas, Tessaleno, and James Corredine. 2001. The biological determinants of long-wave behavior in socioeconomic growth and development. Technological Forecasting and Social Change 68: 1–57. [Google Scholar] [CrossRef]
- Diebolt, Claude. 2005. Long cycles revisited—An essay in econometric history. Economies et Sociétés 39: 23–47. [Google Scholar]
- Diebolt, Claude, and Cedric Doliger. 2006. Economic cycles under test: A spectral analysis. In Kondratieff Waves, Warfare and World Security. Edited by Tessaleno C. Devezas. Amsterdam: IOS Press, pp. 39–47. [Google Scholar]
- Focacci, Antonio. 2017. Controversial curves of the economy: An up-to-date investigation of long waves. Technological Forecasting and Social Change 116: 271–85. [Google Scholar] [CrossRef]
- Frisch, Ragnar. 1933. Propagation Problems and Impulse Problems in Dynamic Economics (in Economic Essays in Honour of Gustav Cassel, Allen & Unwin, London, pp. 171–3, 181–90, 197–203). In The Foundations of Econometric Analysis. 1995. Edited by David F. Hendry and Mary S. Morgan. Cambridge: Cambridge University Press, pp. 333–46. [Google Scholar]
- Fukao, Kyoji, Jean-Pascal Bassino, Tatsuji Makino, Ralph Paprzycki, Tokihiko Settsu, Masanori Takashima, and Joji Tokui. 2015. Regional Inequality and Industrial Structure in Japan: 1874–2008. Tokyo: Maruzen Publishing. [Google Scholar]
- Gali, Jordi. 1999. Technology, employment, and the business cycle: Do technology shocks explain aggregate fluctuations? American Economic Review 89: 249–77. [Google Scholar] [CrossRef]
- Gallegati, Marco, and Willi Semmler, eds. 2014. Wavelets Applications in Economics and Finance. Cham: Springer International Publishig. [Google Scholar]
- Gallegati, Marco, Mauro Gallegati, James B. Ramsey, and Willi Semmler. 2017. Long waves in prices: New evidence from wavelet analysis. Cliometrica 11: 127–51. [Google Scholar] [CrossRef]
- Garvy, George. 1943. Kondratieff’s theory of long cycles. The Review of Economics and Statistics 25: 203–20. [Google Scholar] [CrossRef]
- Gattei, Giorgio. 1981. I Cicli Economici Maggiori. Bologna: Nuova Casa Editrice Cappelli. [Google Scholar]
- Gençay, Ramazan, Fanuk Selçuk, and Brandon Whitcher. 2001. An Introduction to Wavelets and Other Filtering Methods in Finance and Economics. San Diego: Academic Press. [Google Scholar]
- Gerster, Hans J. 1992. Testing long waves in price and volume series from Sixteen Countries. In New Findings in Long Wave Research. Edited by Alfred Kleinknecht, Ernest Mandel and Immanuel Wallerstein. New York: St. Martin’s Press, pp. 120–47. [Google Scholar]
- Goldstein, Josha S. 1999. The existence, endogeneity and synchronization of long waves: Structural time series model estimates. Review of Radical Political Economics 31: 61–101. [Google Scholar] [CrossRef]
- Goldstein, Joshua S. 1988. Long Cycles: Prosperity and War in Modern Age. New Haven: Yale University Press. [Google Scholar]
- Gordon, Robert J. 1990. What is new-keynesian economics? Journal of Economic Literature 28: 1115–71. [Google Scholar]
- Granger, Clive W. J., and Michio Hatanaka. 1964. Spectral Analysis of Time Economic Time Series. Princeton: Princeton University Press. [Google Scholar]
- Gregory, Paul R. 1982. Russian National Income, 1885–1913. Cambridge: Cambridge University Press. [Google Scholar]
- Growiec, Jakub, Peter McAdam, and Jakub Mućk. 2018. Endogenous labor share cycles: Theory and evidence. Journal of Economic Dynamics and Control 87: 74–93. [Google Scholar] [CrossRef]
- Harkness, Jon P. 1968. A spectral-analytic test of the long-swing hypothesis in Canada. The Review of Economics and Statistics 50: 429–36. [Google Scholar] [CrossRef]
- Haustein, Heinz-Dieter, and Erich Neuwirth. 1982. Long waves in world industrial production, energy consumption, innovations, inventions, and patents and their identification by spectral analysis. Technological Forecasting and Social Change 22: 53–89. [Google Scholar] [CrossRef][Green Version]
- Hilmola, Olli-Pekka. 2007. Stock market performance and manufacturing capability of the fifth long-cycles industries. Futures 39: 393–407. [Google Scholar] [CrossRef]
- Huffaker, Rat, Marco Bittelli, and Rodolfo Rosa. 2017. Nonlinear Time Series Analysis with R. Oxford: Oxford University Press. [Google Scholar]
- Kaiser, Gerald. 2011. A Friendly Guide to Wavelets. New York: Springer. [Google Scholar]
- Kantor, Brian. 1979. Rational expectations and economic thought. Journal of Economic Literature 17: 1422–41. [Google Scholar]
- Keenan, Daniel M. 1985. A Tukey non-additivity-type test for time series nonlinearity. Biometrika 72: 39–44. [Google Scholar] [CrossRef]
- Klotz, Benjamin P., and Larry Neal. 1973. Spectral and Cross-Spectral Analysis of the Long-Swing Hypothesis. The Review of Economics and Statistics 55: 291–98. [Google Scholar] [CrossRef]
- Kondratieff, Nicolai. 1935. The Long Waves in Economic Life. The Review of Economic Statistics XVII: 105–15. [Google Scholar] [CrossRef]
- Kondratieff, Nicolai D. 1926. Die Langen Wellen der Konjunktur. Archiv für Sozialwissenschaft und Sozial Politik 56: 573–609. [Google Scholar]
- Korotayev, Andrey, and Seregy V. Tsirel. 2010. A spectral analysis of world GDP dynamics: Kondratieff waves, Kuznets swings, Juglar and Kitchin cycles in global economic development, and the 2008–2009 economic crisis. Structure and Dynamics 4: 3–57. [Google Scholar] [CrossRef]
- Li, Yuan, and Zhongjie Xie. 1999. The wavelet identification of thresholds and time delay of threshold autoregressive models. Statistica Sinica 9: 153–66. [Google Scholar]
- Lucas, Robert E., Jr. 1972. Expectations and the neutrality of money. Journal of Economic Theory 4: 103–24. [Google Scholar] [CrossRef]
- Lucas, Robert E., Jr. 1973. Some international evidence on output-inflation trade-offs. American Economic Review 63: 326–34. [Google Scholar]
- Lucas, Robert E., Jr. 1977. Understanding business cycles. In Stabilization of the Domestic and International Economy, Carnegie-Rochester Conferences on Public Policy, supplement. Edited by K. Brunner and A. H. Meltzer. Journal of Monetary Economics 5: S7–29. [Google Scholar]
- Malanima, Paolo. 2010. The long decline of a leading economy: GDP in central and northern Italy: 1300–1913. European Review of Economic History 15: 169–219. [Google Scholar] [CrossRef]
- Markevich, Andrei, and Mark Harrison. 2011. Great war: Civil war, and recovery Russia’s national income, 1913 to 1928. The Journal of Economic History 71: 672–703. [Google Scholar] [CrossRef]
- Martey Addo, Peter, Monica Billio, and Dominique Guégan. 2014. Non linear dynamics and wavelets for business cycles analysis. In Wavelets Applications in Economics and Finance. Edited by Marco Gallegati and Willi Semmler. Cham: Springer International Publishing. [Google Scholar]
- Metz, Rainer, and Winfried Stier. 1992. Modelling the long wave phenomena. Historical Social Research 17: 43–62. [Google Scholar]
- Metz, Rainer. 1992. A re-examination of long waves aggregate production series. In New Findings in Long Wave Research. Edited by Alfred Kleinknecht, Ernest Mandel and Immanuel Wallerstein. New York: St. Martin’s Press, pp. 80–119. [Google Scholar]
- Metz, Rainer. 2006. Empirical evidence and causation of Kondratieff cycles. In Kondratieff Waves, Warfare and World Security. Edited by Tessaleneo C. Devezas. Amsterdam: IOS Press, pp. 91–99. [Google Scholar]
- Metz, Rainer. 2011. Do Kondratieff waves exist? How time series techniques can help to solve the problem. Cliometrica 5: 205–38. [Google Scholar] [CrossRef]
- Minsky, Hyman P. 1992. The Financial Instability Hypothesis. Working Paper, 74. Annandale-on-Hudson: Levy Economics Institute of Bard College. [Google Scholar]
- MPD. 2020. Maddison Project Database. Available online: https://www.rug.nl/ggdc/historicaldevelopment/maddison/releases/maddison-project-database-2020?lang=en (accessed on 23 January 2023).
- Muth, Jean F. 1960. Optimal properties of exponentially weighted forecast. Journal of American Statistical Association 55: 299–306. [Google Scholar] [CrossRef]
- Muth, Jean F. 1961. Rational expectations and the theory of price movements. Econometrica 29: 315–35. [Google Scholar] [CrossRef]
- Nelson, Charles R., and Charles I. Plosser. 1982. Trends and random walks in macroeconomic time series. Journal of Monetary Economics 10: 139–62. [Google Scholar] [CrossRef]
- Percival, Donald, and Andrew Walden. 2000. Wavelets Methods for Time Series Analysis. Cambridge: Cambridge University Press. [Google Scholar]
- Petrakos, George, Konstantinos Rontos, Chiara Vavoura, and Ioannis Vavouras. 2023. The impact of recent economic crises on income inequality and the risk of poverty in Greece. Economies 11: 166. [Google Scholar] [CrossRef]
- Pigou, Arthur C. 1927. Industrial Fluctuations. London: Macmillan and Company Limited. [Google Scholar]
- Ramsey, James B. 2002. Wavelets in economics and finance: Past and future. Studies in Nonlinear Dynamics and Econometrics 6: 1–27. [Google Scholar] [CrossRef]
- Ramsey, James B., and Camille Lampard. 1998a. The decomposition of economic relationships by time scale using wavelets: Expenditure and income. Studies in Nonlinear Dynamics and Econometrics 3: 23–41. [Google Scholar] [CrossRef]
- Ramsey, James B., and Camille Lampard. 1998b. Decomposition of economic relationships by timescale using wavelets. Macroeconomic Dynamics 2: 49–71. [Google Scholar] [CrossRef]
- Rasmussen, Steel, Jan Holst, and Erik Mossekilde. 1989. Empirical investigation of economic long waves in aggregate production. European Journal of Operational Research 42: 279–93. [Google Scholar] [CrossRef]
- Reijnders, Johannes P. G. 1988. The Enigma of Long Waves. Utrecht: Drukkeru Elinkwijk BV. [Google Scholar]
- Reijnders, Johannes P. G. 1990. Long Waves in Economic Development. Aldershot: Edward Elgar. [Google Scholar]
- Reijnders, Johannes P. G. 2009. Trend movements and inverted Kondratieff waves in Dutch economy, 1800–1913. Structural Change and Economic Dynamics 20: 90–113. [Google Scholar] [CrossRef]
- Rhif, Manel, Ali Ben Abbes, Imed Riadh Farah, Beatriz Martinez, and Yanfang Sang. 2019. Wavelet transform application for/in non-stationary time series analysis: A review. Applied Sciences 9: 1345. [Google Scholar] [CrossRef]
- Ridolfi, Leonardo. 2016. The French Economy in the Long Durée. A Study on Real Wages, Working Days and Economic Performance from Louis IX to the Revolution (1250–1789). Dissertation IMT School for Advanced Studies, Lucca. Available online: http://e-theses.imtlucca.it/211/1/Ridolfi_phdthesis.pdf (accessed on 23 January 2023).
- Rösch, Angi, and Harald Schmidbauer. 2018. WaveletComp: Computational Wavelet Analysis. R Package Version 1.1. and “WaveletComp1.1: A Guided Tour through the R package” (Available in R Documentation). Available online: https://CRAN.R-project.org/package=WaveletComp (accessed on 23 January 2023).
- Schleicher, Christoph. 2002. An introduction to wavelets for economists. In Working Paper 2002–3. Ottawa: Bank of Canada. [Google Scholar]
- Schumpeter, Joseph. A. 1939. Business Cycles: A Theoretical, Historical and Statistical Analysis of the Capitalist Process. New York: McGraw-Hill Book Company. [Google Scholar]
- Silvestre, Joaquim. 1993. The market-power foundations of macroeconomic policy. Journal of Economic Literature 31: 105–41. [Google Scholar]
- Sleziak, Patrick, Kamila Hlačovă, and Jan Szolgay. 2015. Advantages of a time series analysis using wavelet transform as compared with a Fourier analysis. Slovak Journal of Civil Engineering 23: 30–36. [Google Scholar] [CrossRef]
- Slutsky, Eugen. 1927. The summation of random causes as the source of cyclic processes. Econometrica 5: 105–46. [Google Scholar] [CrossRef]
- Soper, John. C. 1975. Myth and Reality in Economic Time Series: The Long Swing Revisited. Southern Economic Journal 41: 570–79. [Google Scholar] [CrossRef]
- Sutch, Richard. 2006. National Income and Product Historical Statistics of the United States: Earliest Time to the Present. Edited by Susan B. Carter, Scott S. Gartner, Michael R. Haines, Alan Olmstead, Richard Sutch and Gavin Wright. New York: Cambridge University Press, pp. 23–25. [Google Scholar]
- Svabova, Lucia, Katarina Kramarova, and Dominika Chabadova. 2022. Impact of the COVID 19 pandemic on the business environment in Slovakia. Economies 10: 244. [Google Scholar] [CrossRef]
- Taylor, James B. 1988. Long waves in six nations: Results and speculations from a new methodology. Review IX: 373–92. [Google Scholar]
- Tong, Howell. 1978. On a threshold model. In Patterns Recognition and Signal Processing. NATO ASI Series E: Applied SC. No. 29. Edited by Chi-Hau Chen. Amsterdam: Sijthoff & Noordhoff, pp. 575–86. [Google Scholar]
- Torrence, Christofer, and Gilbert Compo. 1998. A practical guide to wavelet analysis. Bullettin of the American Meteorological Society 79: 61–78. [Google Scholar] [CrossRef]
- Trapletti, Adrian, and Kurt Hornik. 2023. tseries: Time Series Analysis and Computational Finance. R Package Version 0.10-54. Available online: https://CRAN.R-project.org/package=tseries (accessed on 1 June 2023).
- Tsoulfidis, Lefteris, and Aris Papageorgiou. 2019. The recurrence of long cycles: Theories, stylized facts and figures. World Review of Political Economy 10: 415–48. [Google Scholar] [CrossRef]
- Tsoulfidis, Lefteris, and Persefoni Tsaliki. 2022. The long recession and economic consequences of the covid-19 pandemic. Investigaciόn Econόmica 81: 3–29. [Google Scholar] [CrossRef]
- Tugan-Baranowsky, Michael. 1901. Studien zur Theorie und Geschichte der Handelskrisen in England. Jena: Scientia-Verlag. [Google Scholar]
- van Ewijk, Casper. 1982. A spectral analysis of the Kondratieff-cycle. Kyklos 35: 468–99. [Google Scholar] [CrossRef]
- van Gelderen, Jacob. 1913. Springvloed Besschouwingen over Industriéle Ontwikkeling en Prjisbeweging. Die Nieuwe Tijd 18: 254–77, 370–84, 446–64. [Google Scholar]
- Walker, James S. 2008. A Primer on Wavelets and Their Scientific Applications, 2nd ed. Boca Raton: Chapman & Hall. [Google Scholar]
- Warner, Rebecca. M. 1998. The Spectral Analysis of Time-Series Data. New York: The Guilford Press. [Google Scholar]
- Watson, Mark W. 1993. Measures of fit for calibrated models. Journal of Political Economy 101: 1011–41. [Google Scholar] [CrossRef][Green Version]
- Yule, George U. 1927. On a method of investigating periodicities in disturbed series with special reference to wolfer’s sunspot numbers. Philosophical Transactions of the Royal Society of London. Series A 226: 267–98. [Google Scholar]


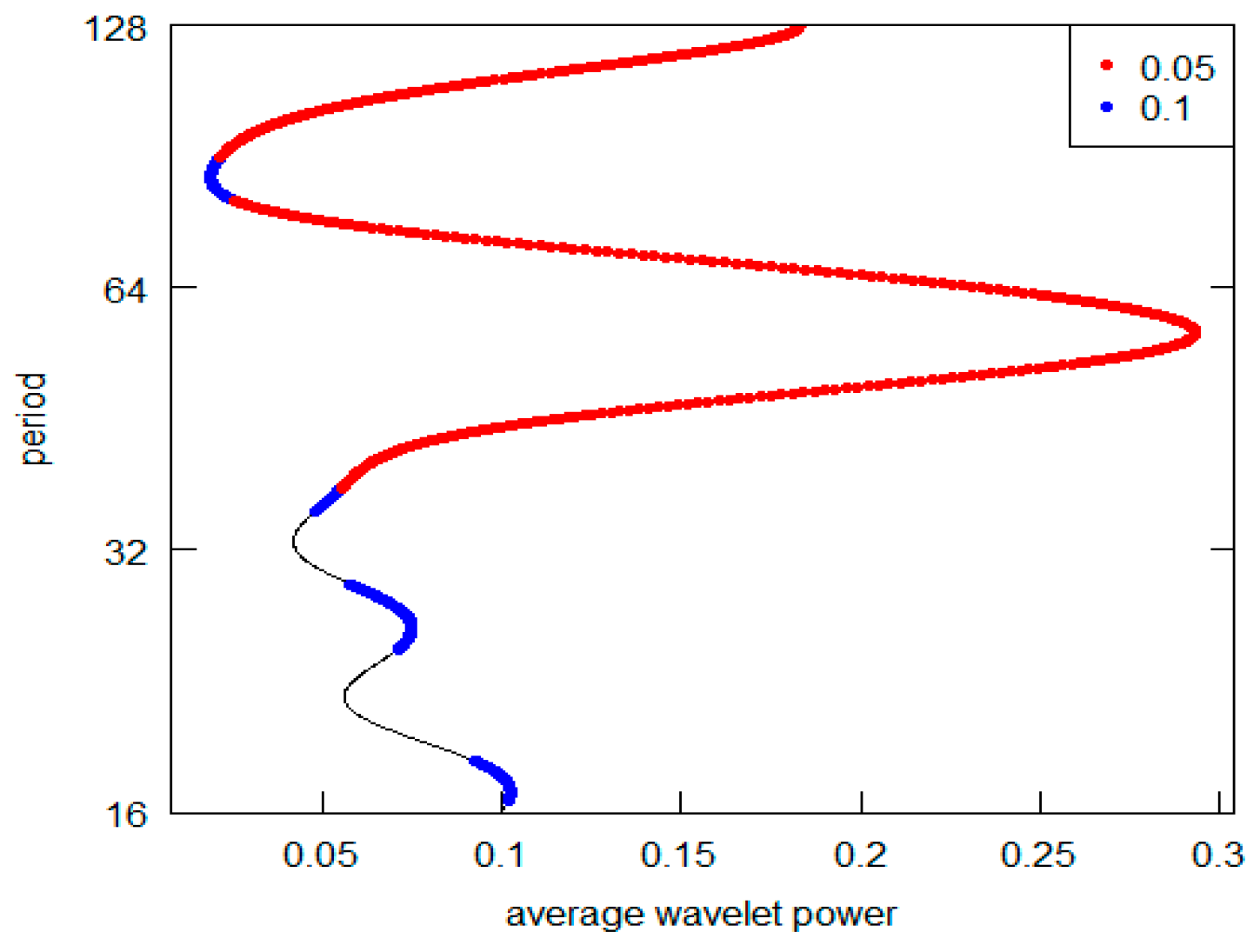


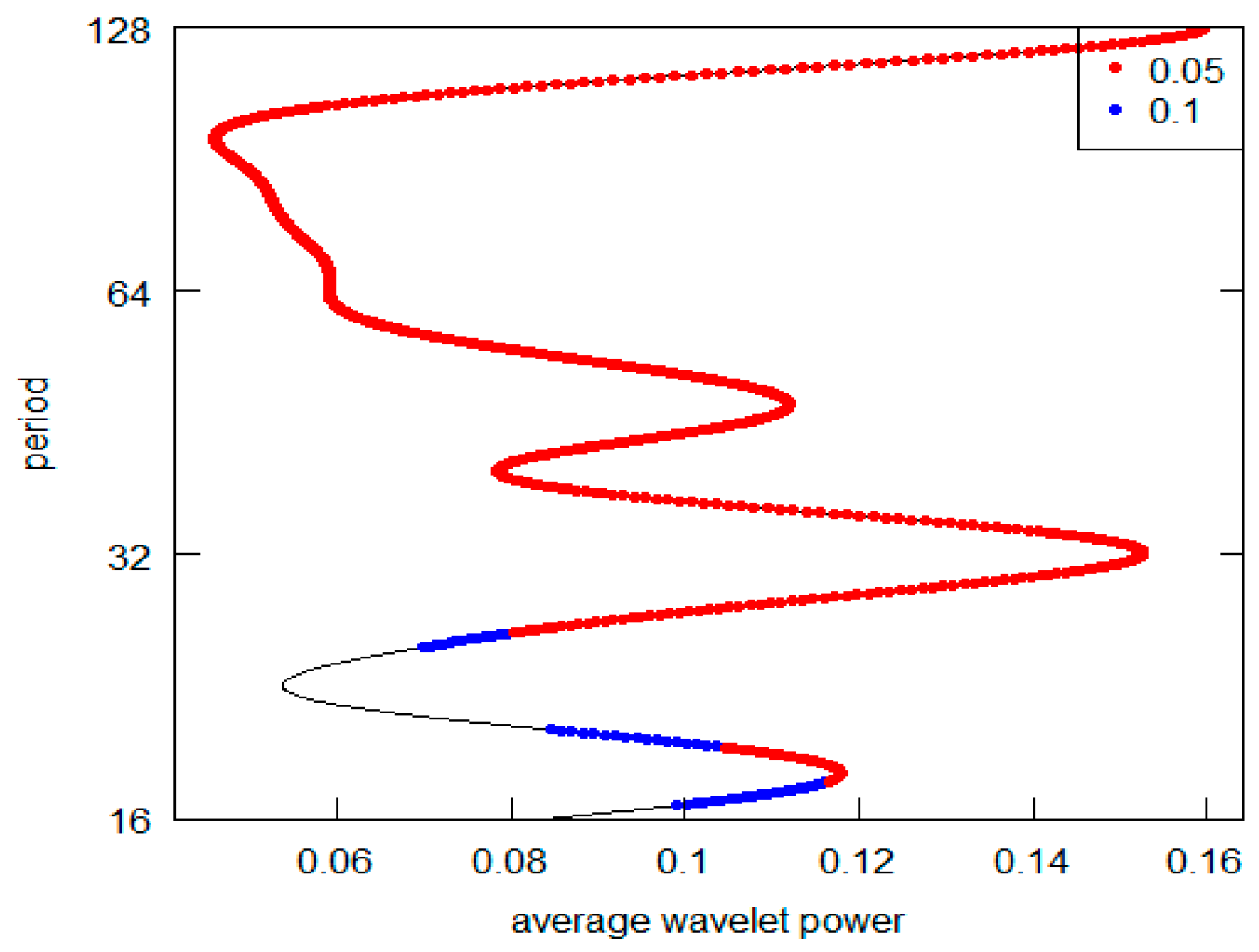
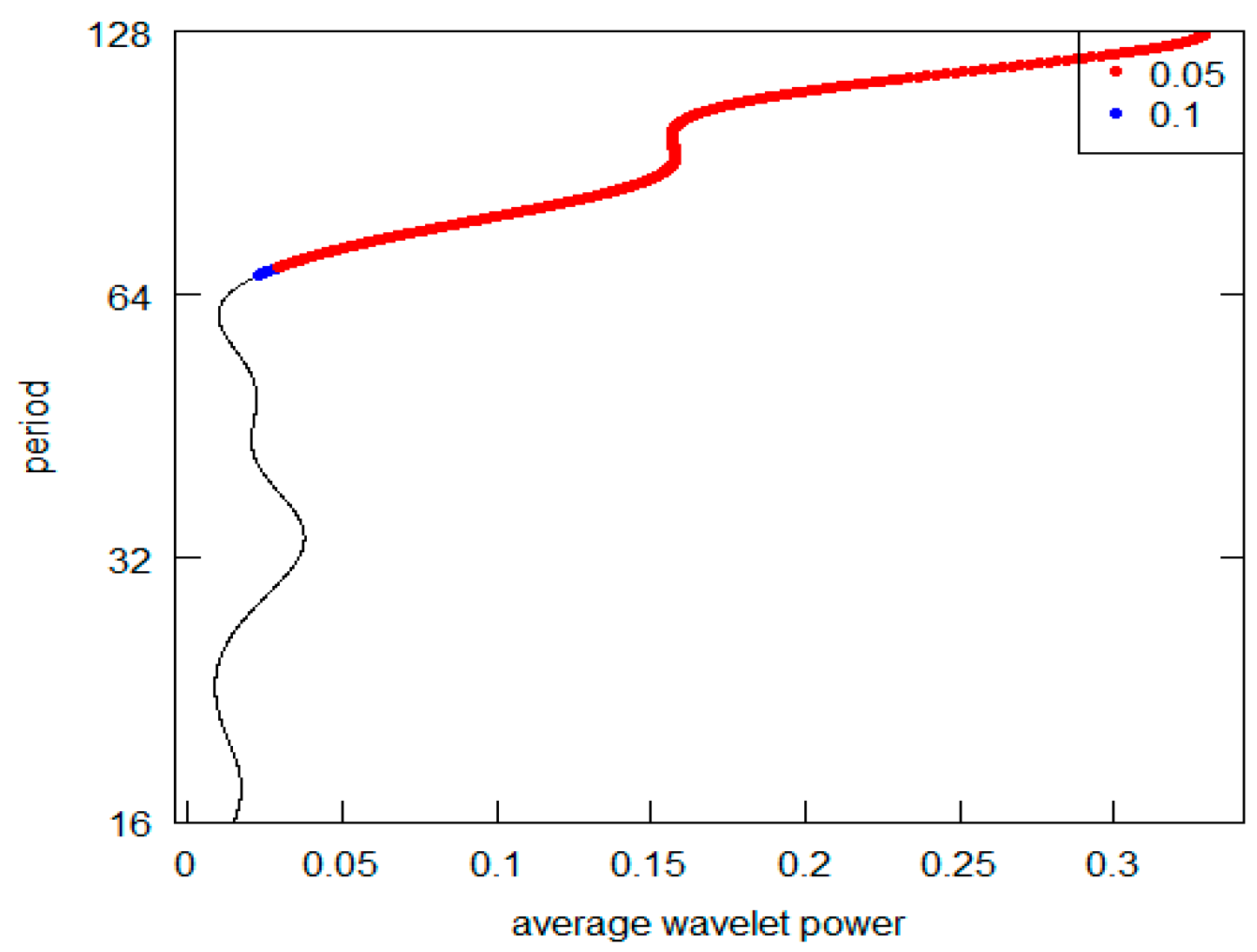


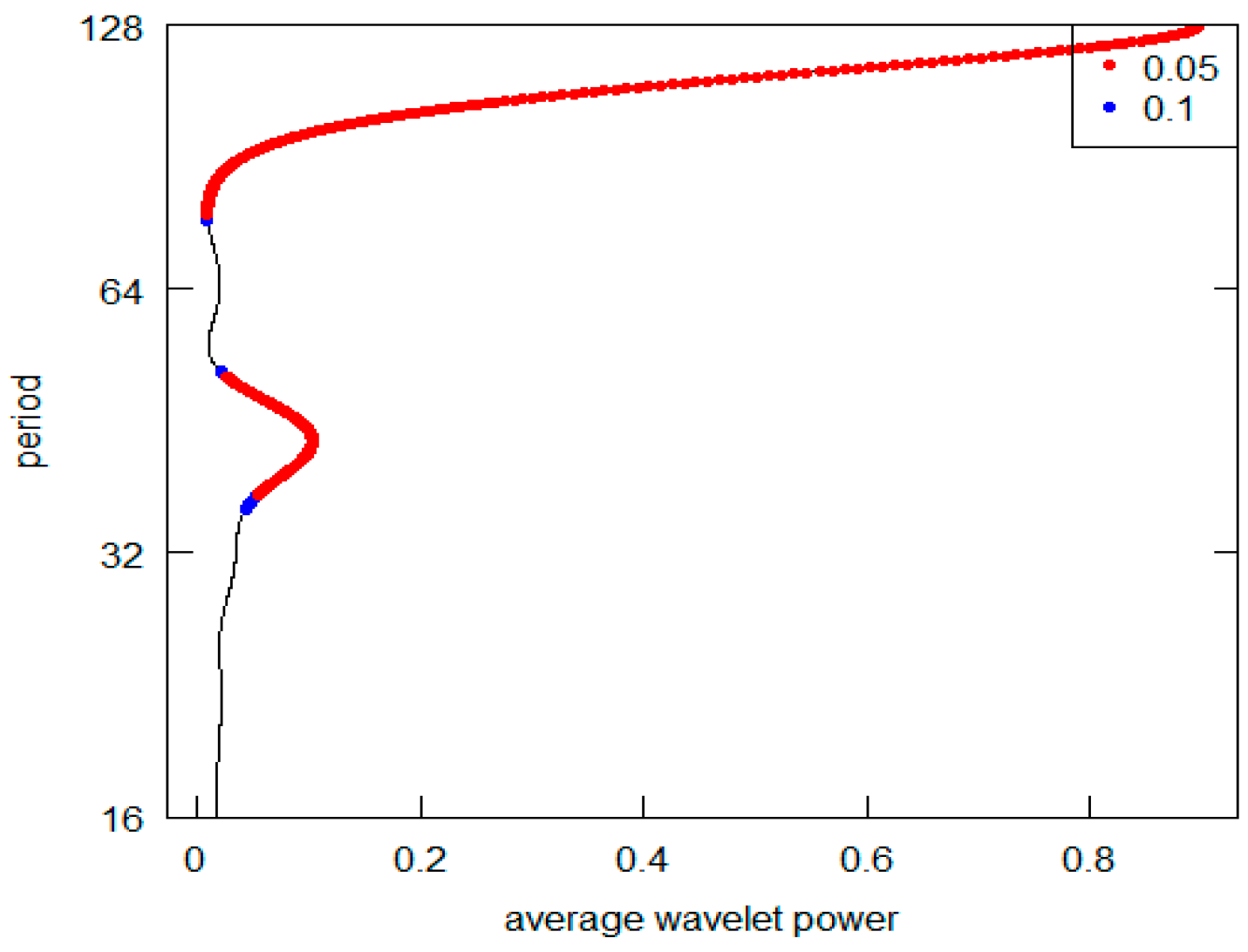
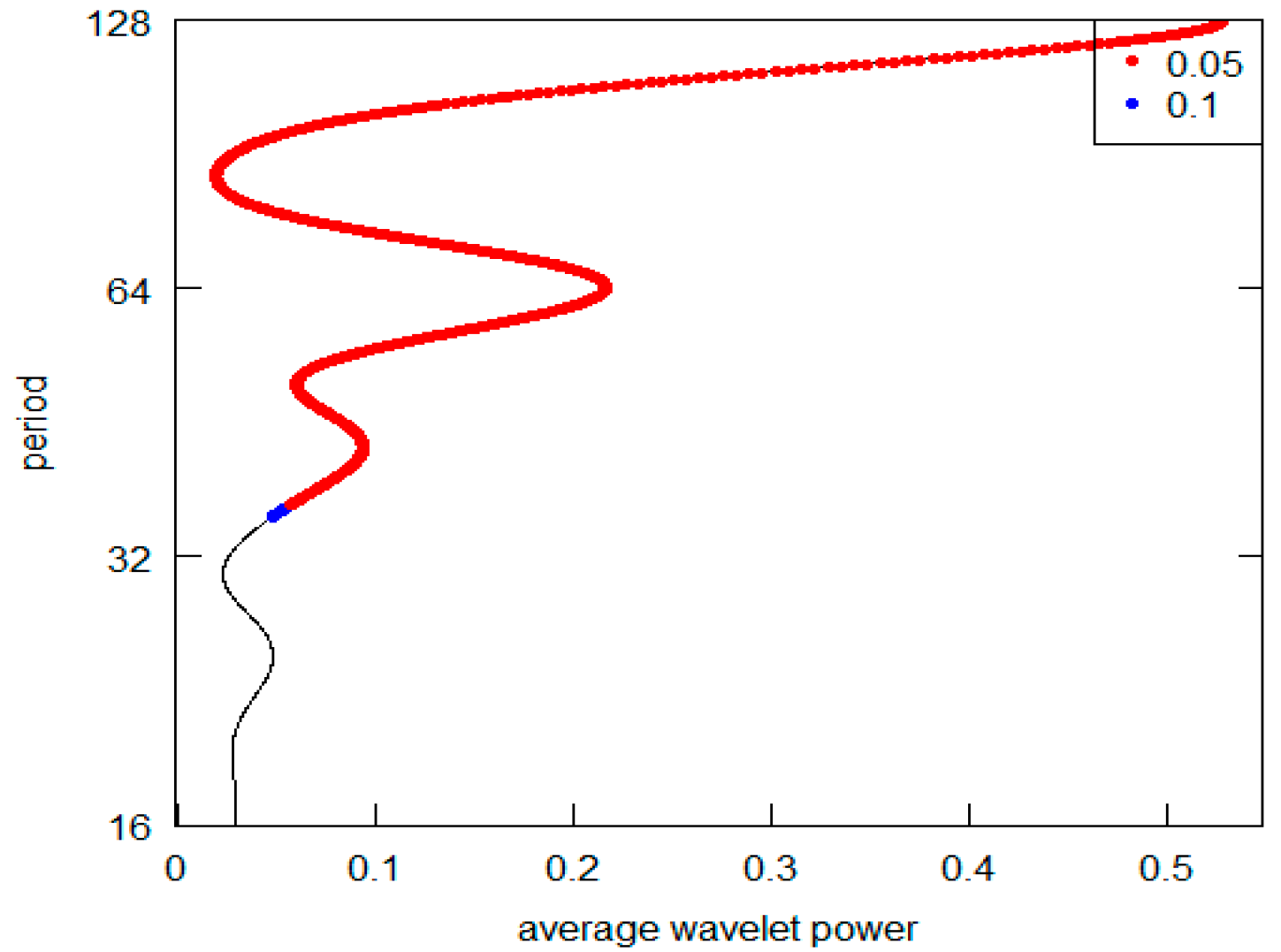
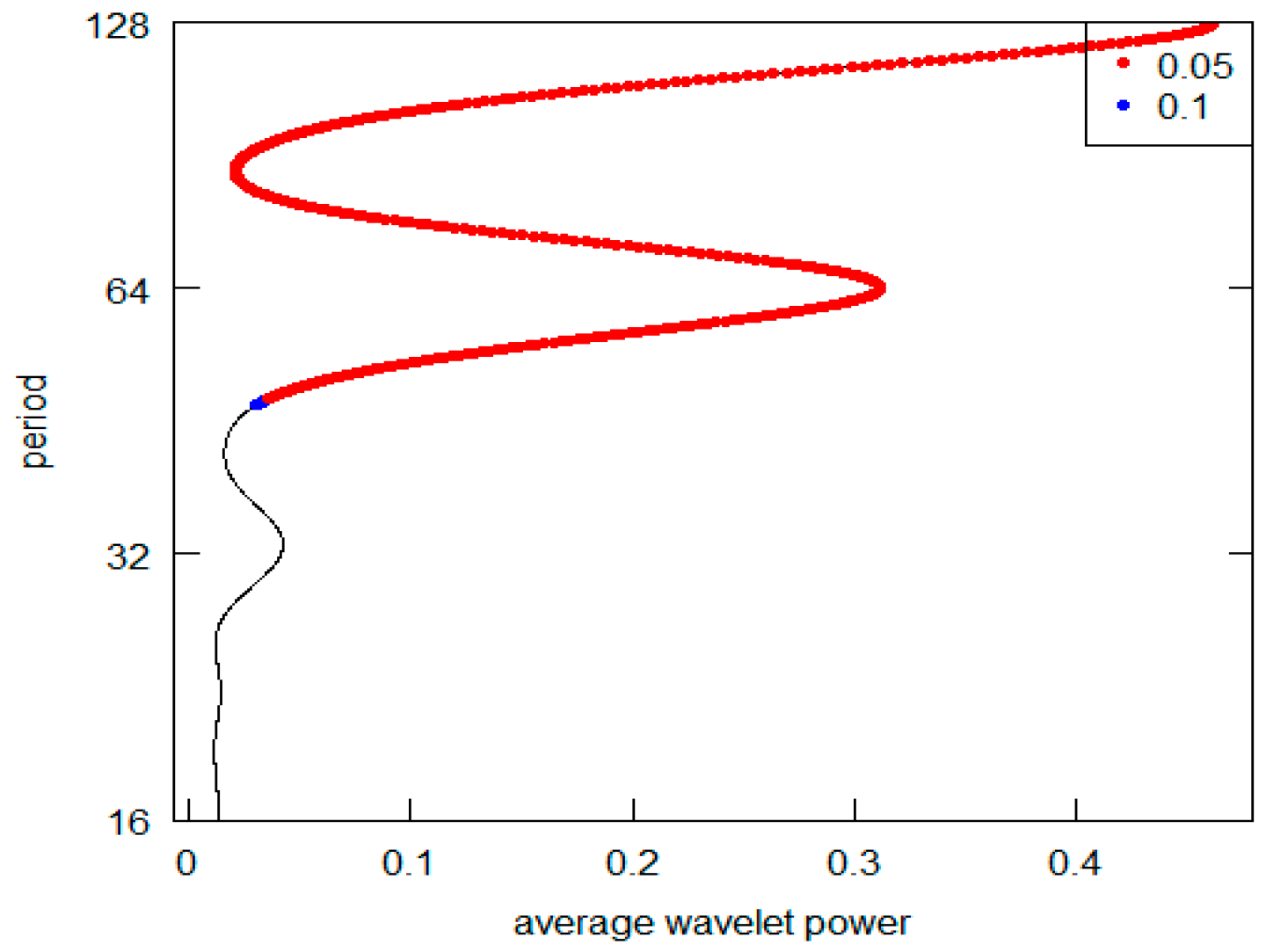
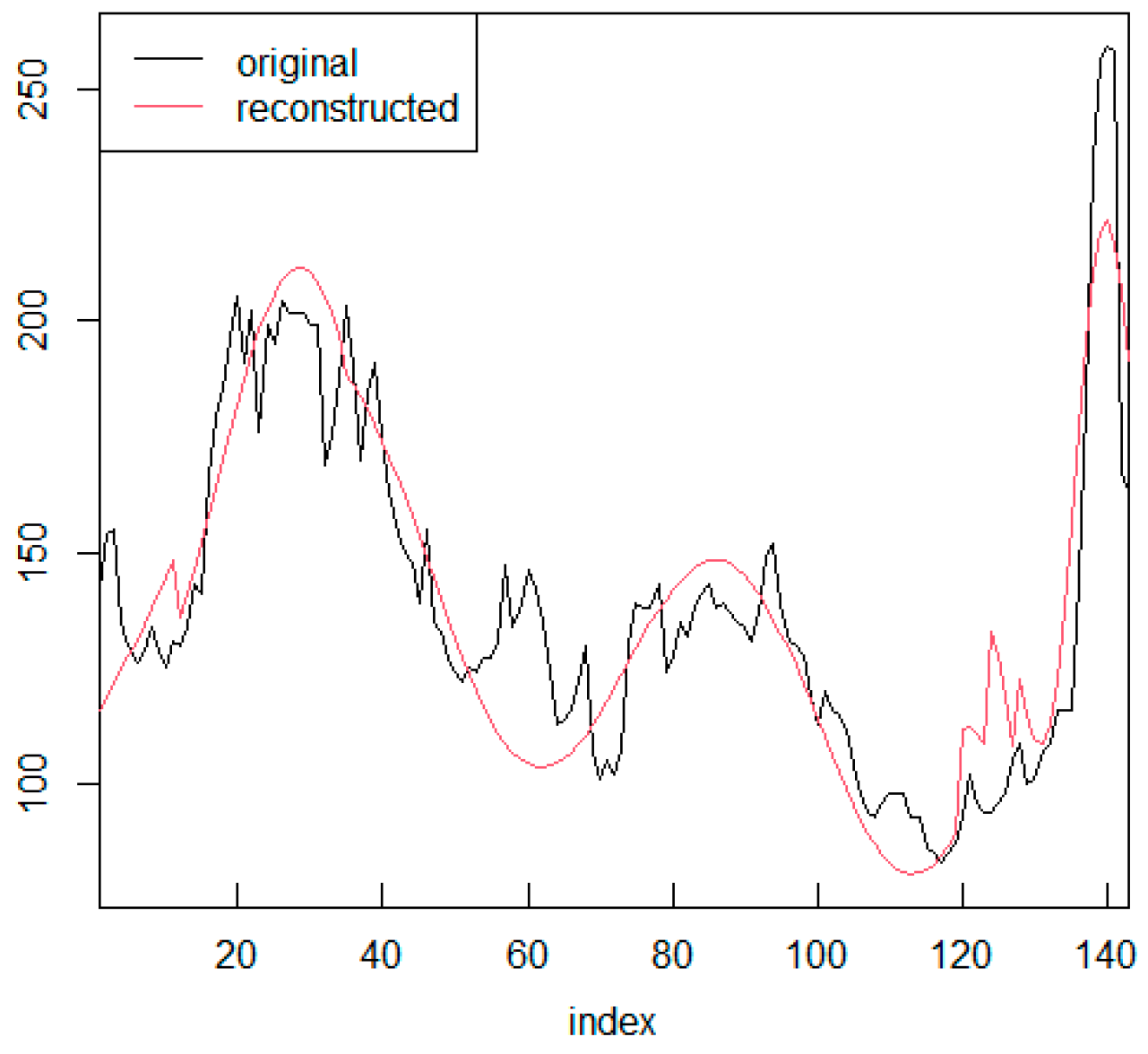
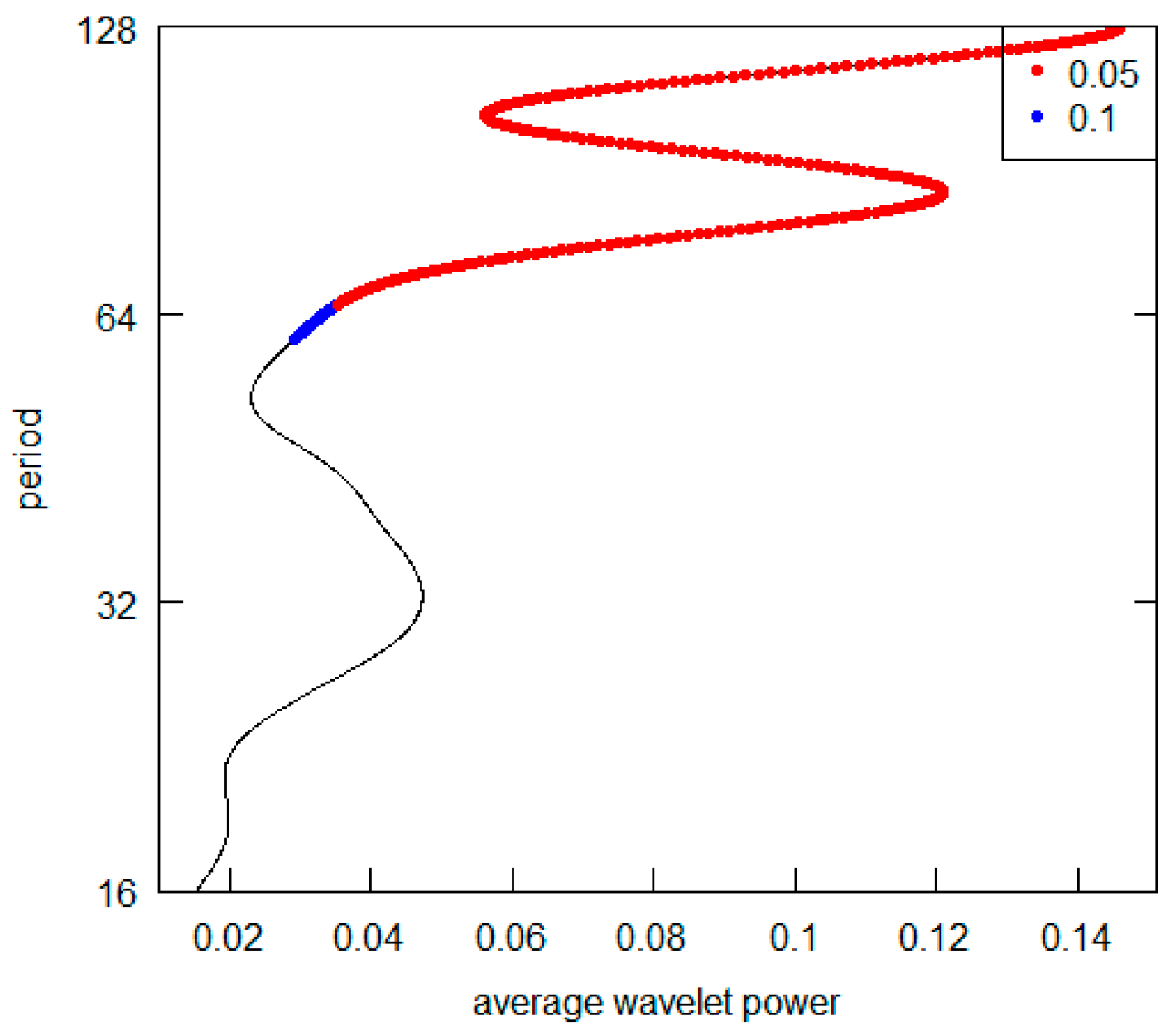

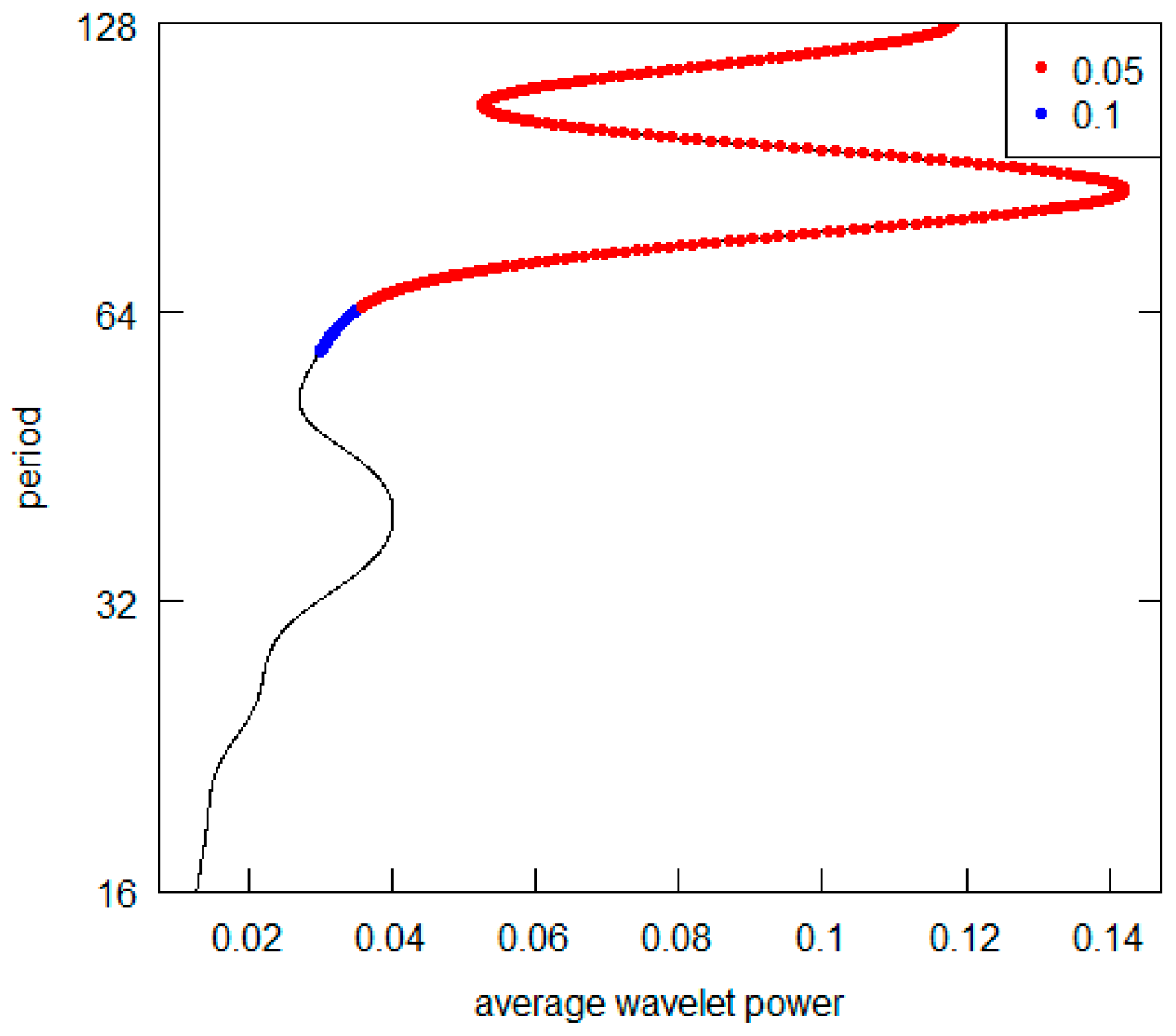

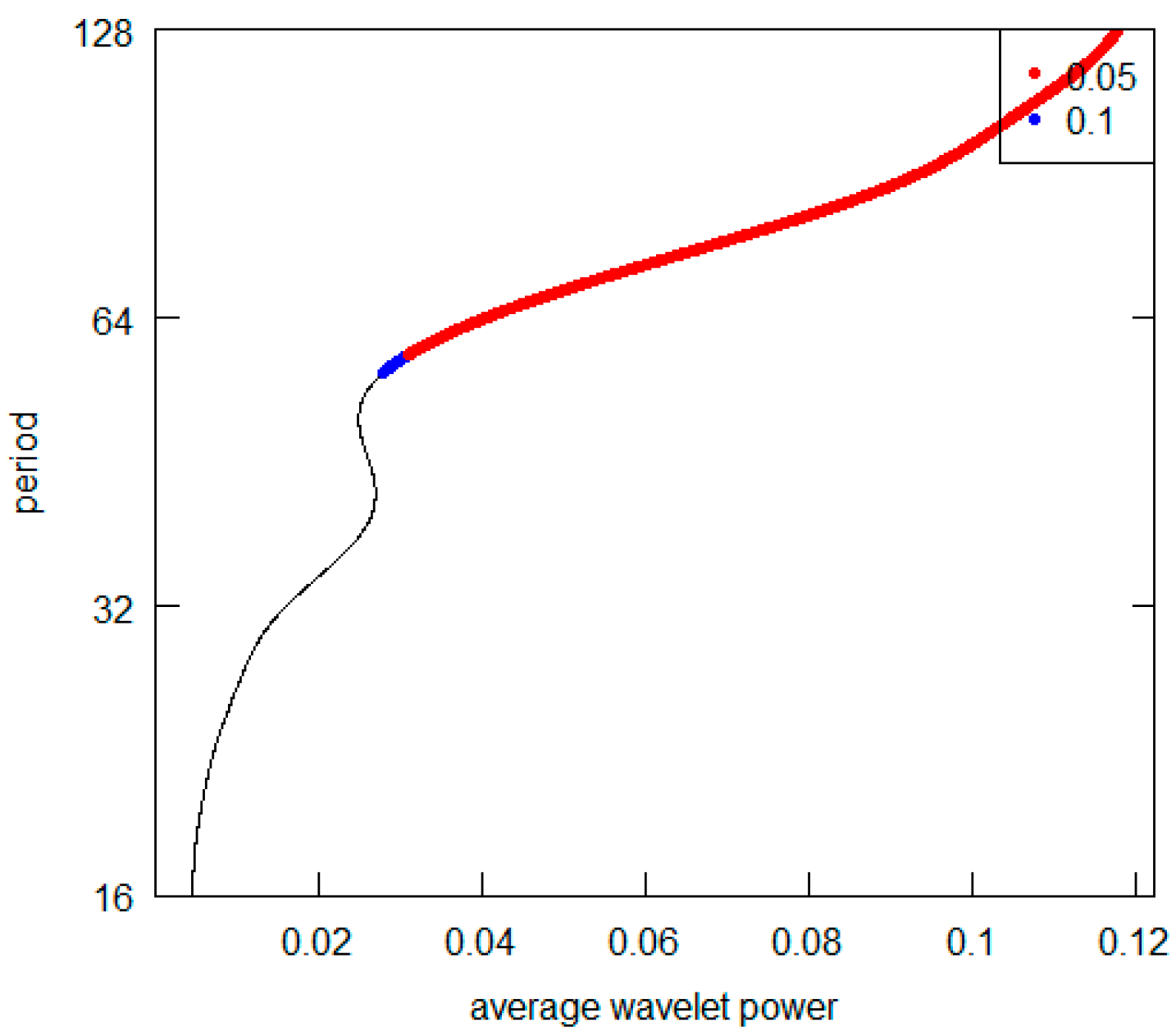
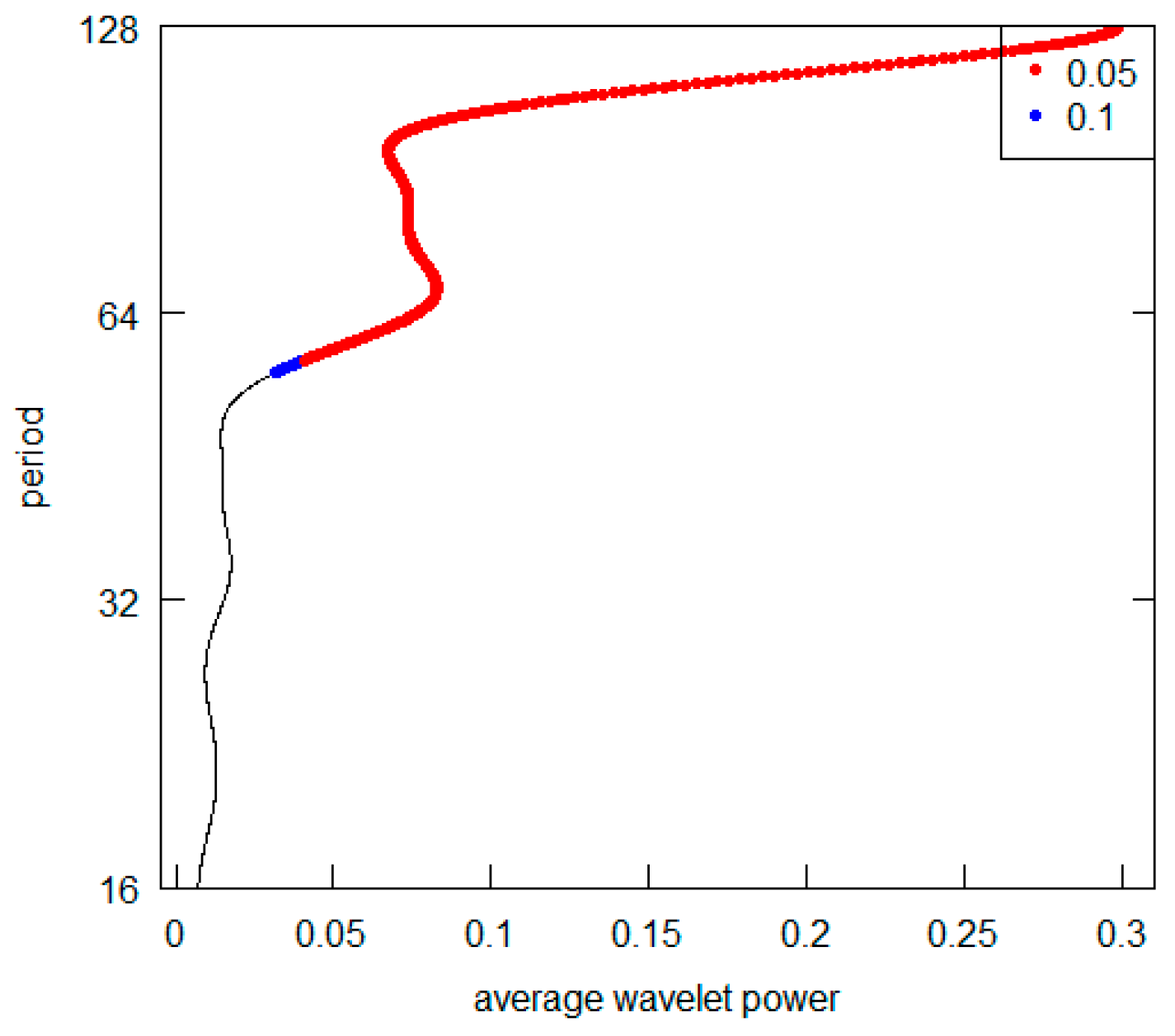

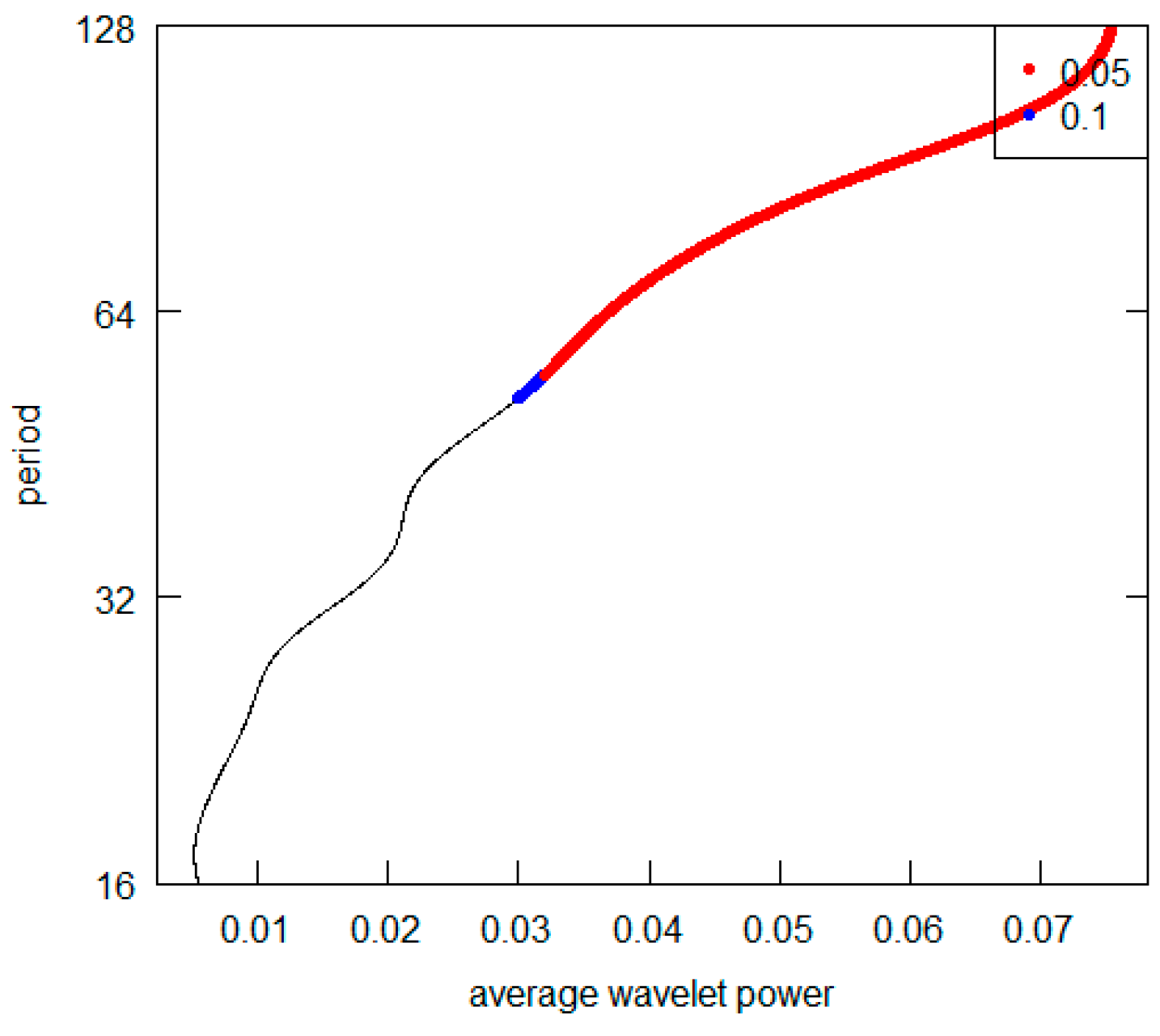
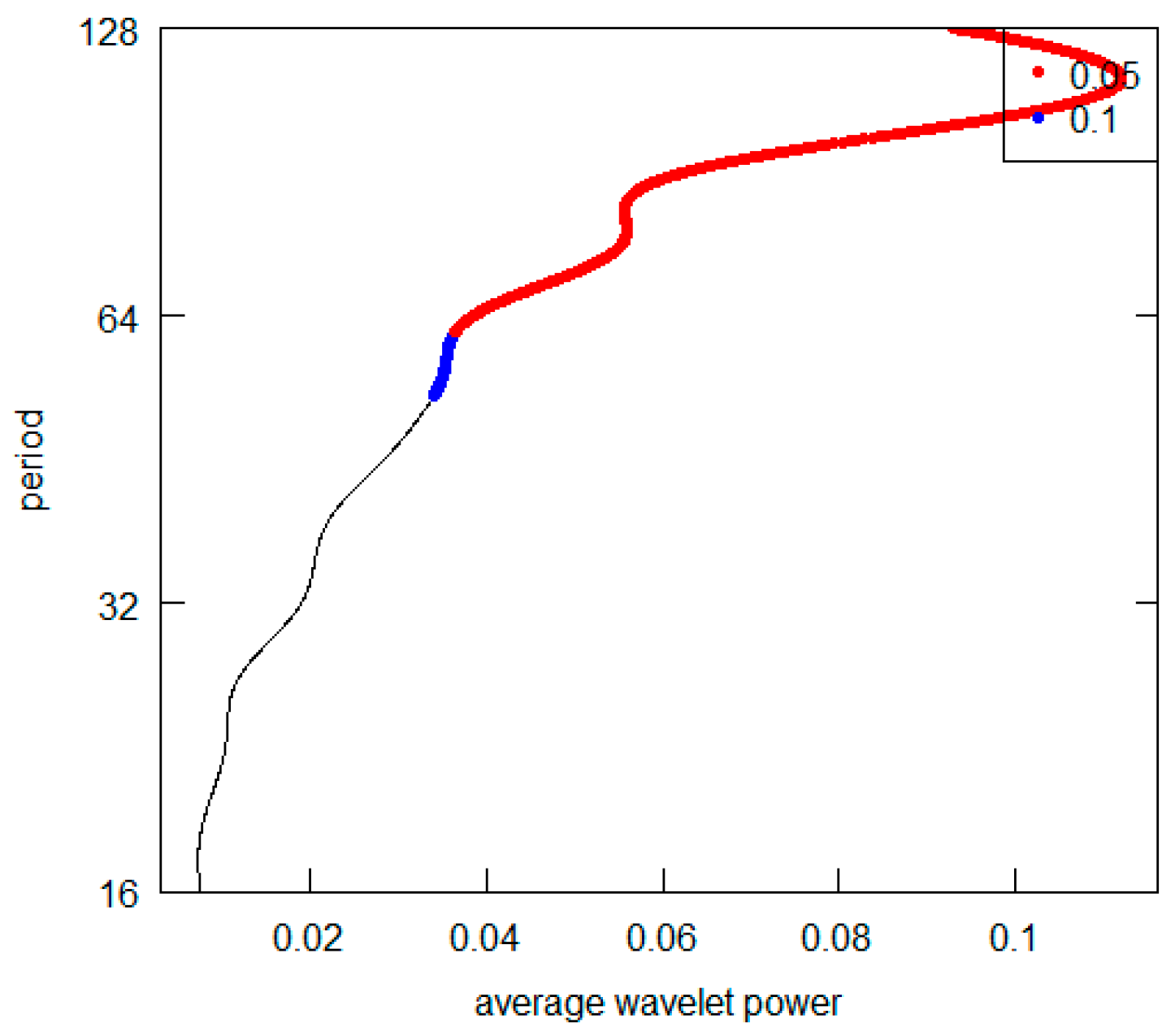
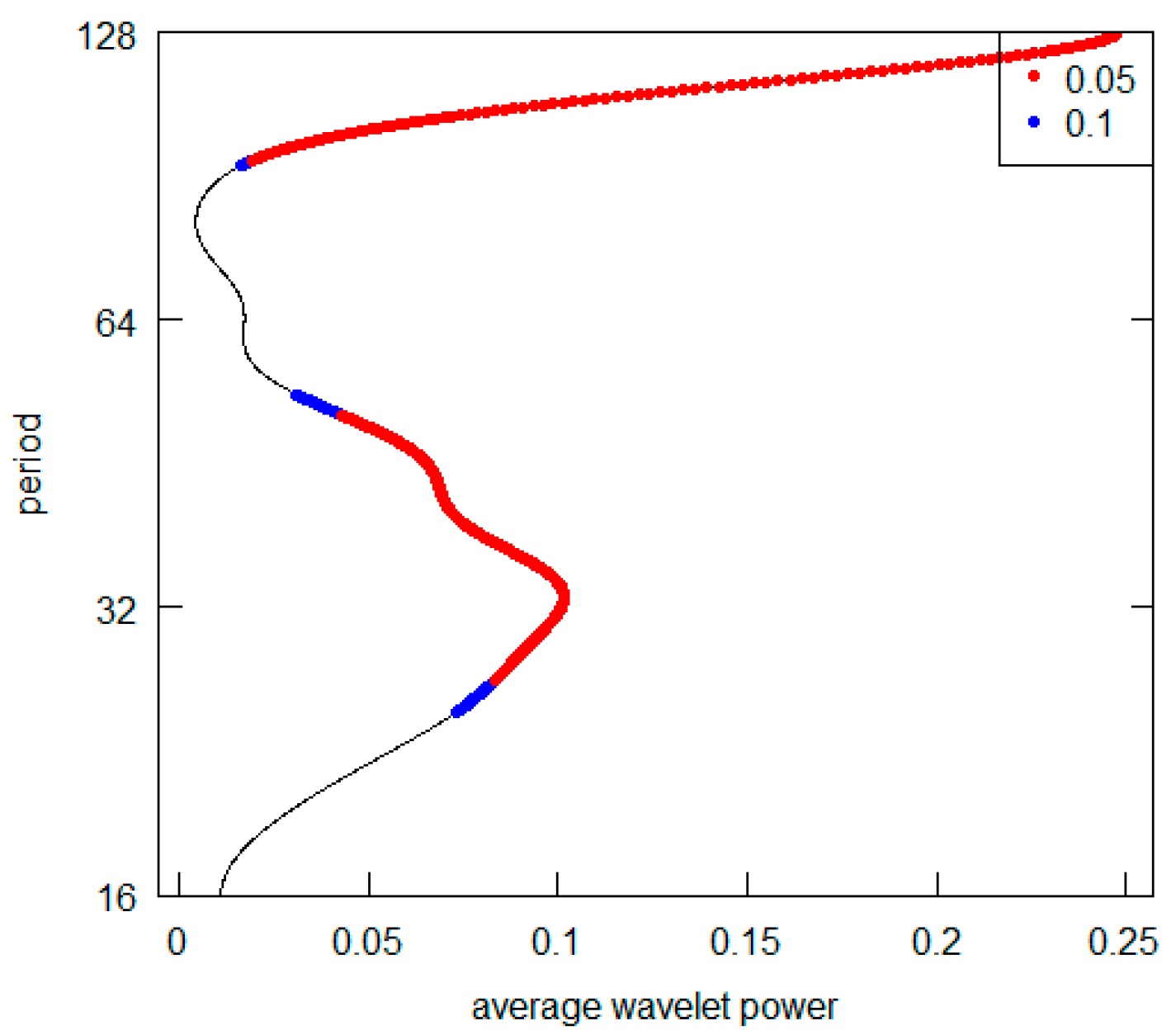
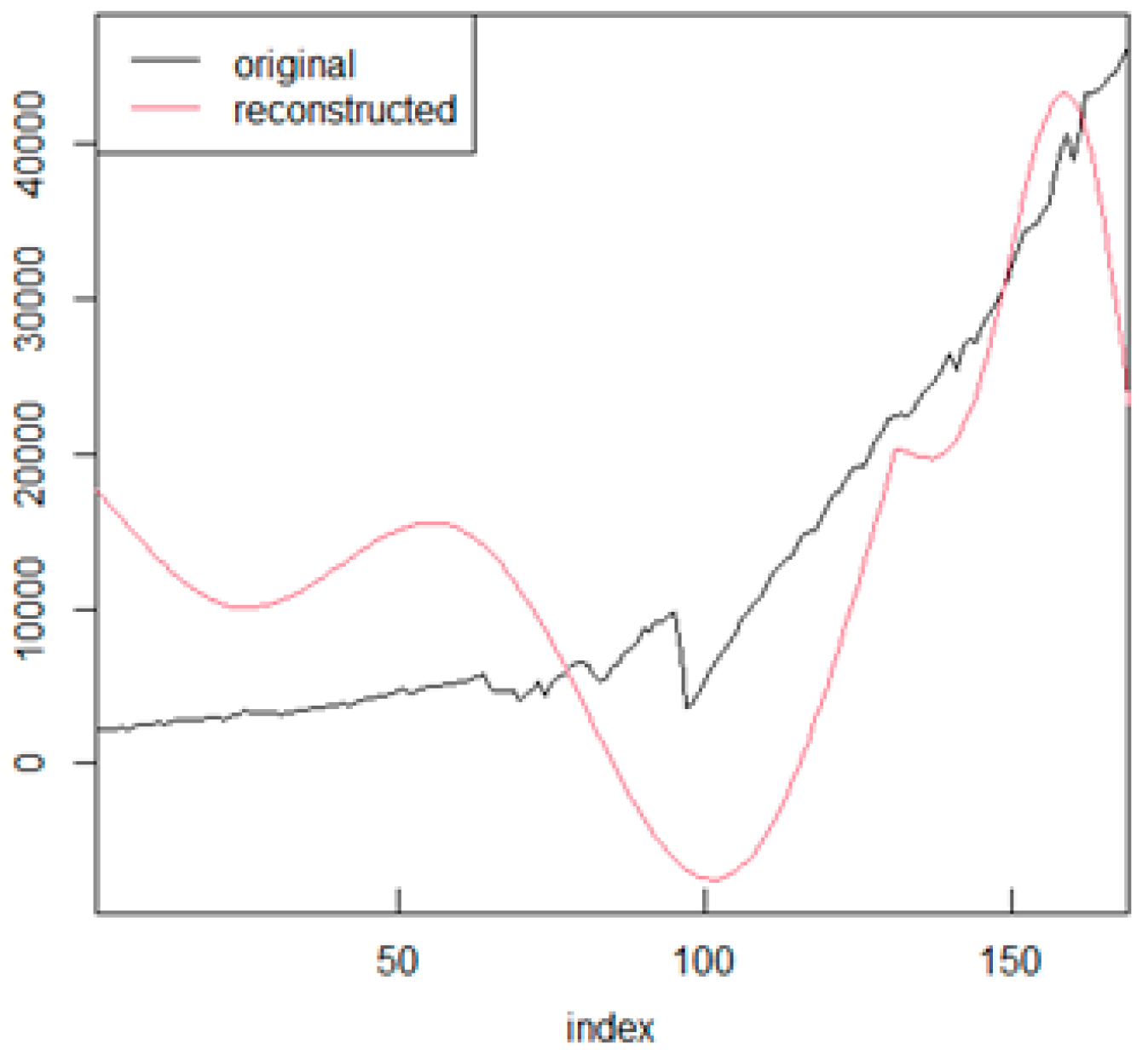
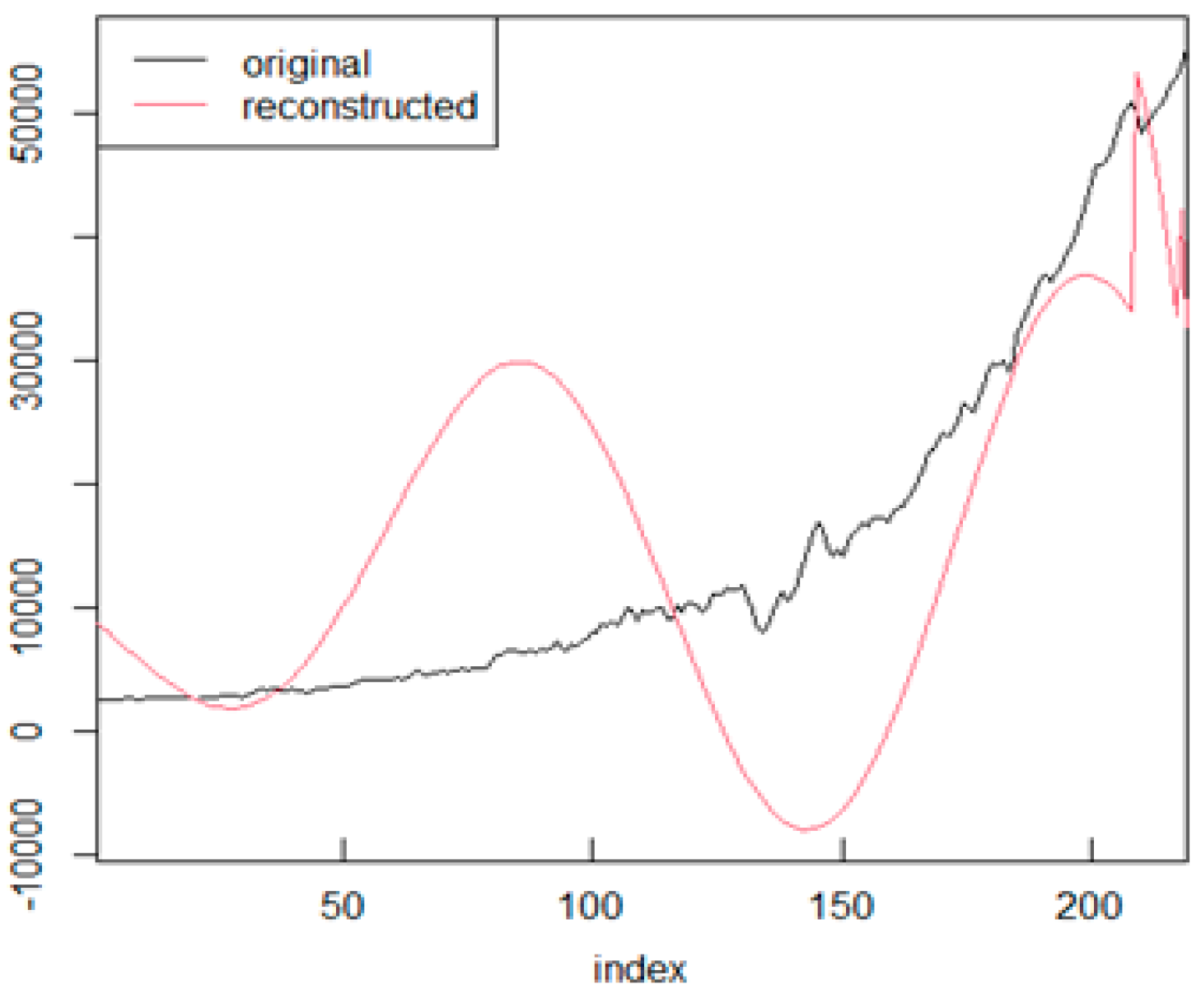
| Scale Level J | Scale Crystals (Detail Level dj) | Annual Frequency Resolution |
|---|---|---|
| 1 | d1 | 2–4 |
| 2 | d2 | 4–8 |
| 3 | d3 | 8–16 |
| 4 | d4 | 16–32 |
| 5 | d5 | 32–64 |
| 6 | d6 | 64–128 |
| 7 | d7 | 128–256 |
| Series | A | B | C | D | E | F |
| N | 143 | 65 | 132 | 107 | 109 | 125 |
| Linearity test | ||||||
| Keenan test | 14.37 | 30.68 | 10.56 | 2.23 | 3.81 | 4.80 |
| p-value | 0.00 * | 0.00 * | 0.00 * | >0.05 | 0.05 * | 0.03 * |
| BDS test p-value | 0.00 * | 0.00 * | 0.00 * | 0.00 * | 0.00 * | 0.00 * |
| Series | G | H | I | J | K | L |
| N | 107 | 87 | 63 | 87 | 75 | 66 |
| Linearity test | ||||||
| Keenan test | 0.25 | 0.29 | 5.15 | 0.22 | 9.85 | 0.91 |
| p-value | >0.05 | >0.05 | 0.03 * | >0.05 | 0.00 * | >0.05 |
| BDS test p-value | 0.00 * | 0.00 * | 0.00 * | 0.00 * | 0.00 * | 0.00 * |
| Series | Brazil | France | Germany | India | Italy | Japan |
| N | 169 | 709 | 169 | 135 | 709 | 134 |
| Linearity test | ||||||
| Keenan test | 9.06 | 21.38 | 1.75 | 64.97 | 13.84 | 37.17 |
| p-value | 0.00 * | 0.00 * | >0.05 | 0.00 * | 0.00 * | 0.00 * |
| BDS test p-value | 0.00 * | 0.00 * | 0.00 * | 0.00 * | 0.00 * | 0.00 * |
| Series | Turkey | UK | USA | USSR | ||
| N | 98 | 767 | 219 | 129 | ||
| Linearity test | ||||||
| Keenan test | 4.00 | 0.60 | 2.37 | 0.03 | ||
| p-value | 0.05 * | >0.05 | >0.05 | >0.05 | ||
| BDS test p-value | 0.00 * | 0.00 * | 0.00 * | 0.00 * |
| Series | Scale Crystals (Detail Level dj) | Annual Frequency Resolution |
|---|---|---|
| A | d5 | 32–64 |
| B | d5 | 32–64 |
| C | d5 | 32–64 |
| D | d5 | 32–64 |
| E | d5 | 32–64 |
| F | >d7 | >128 |
| G | >d7 | >128 |
| H | >d7 | >128 |
| I | >d7 | >128 |
| J | >d7 | >128 |
| K | >d7 | >128 |
| L | >d7 | >128 |
| Serie | Scale Crystals (Detail Level dj) | Annual Frequency Resolution |
|---|---|---|
| Brazil | >d7 | >128 |
| France | >d7 | >128 |
| Germany | d6 | 64–128 |
| India | >d7 | >128 |
| Italy | >d7 | >128 |
| Japan | >d7 | >128 |
| Turkey | >d7 | >128 |
| UK | >d7 | >128 |
| USA | d6 | 64–128 |
| Former USSR | >d7 | >128 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Focacci, A. A Wavelet Investigation of Periodic Long Swings in the Economy: The Original Data of Kondratieff and Some Important Series of GDP per Capita. Economies 2023, 11, 231. https://doi.org/10.3390/economies11090231
Focacci A. A Wavelet Investigation of Periodic Long Swings in the Economy: The Original Data of Kondratieff and Some Important Series of GDP per Capita. Economies. 2023; 11(9):231. https://doi.org/10.3390/economies11090231
Chicago/Turabian StyleFocacci, Antonio. 2023. "A Wavelet Investigation of Periodic Long Swings in the Economy: The Original Data of Kondratieff and Some Important Series of GDP per Capita" Economies 11, no. 9: 231. https://doi.org/10.3390/economies11090231
APA StyleFocacci, A. (2023). A Wavelet Investigation of Periodic Long Swings in the Economy: The Original Data of Kondratieff and Some Important Series of GDP per Capita. Economies, 11(9), 231. https://doi.org/10.3390/economies11090231






