A Stochastically Correlated Bivariate Square-Root Model
Abstract
1. Introduction
1.1. Motivation
1.2. Contribution
1.3. Paper Outline
2. Literature Review
3. The Model
4. Underpinning Results
5. Applications to Finance: Fixed-Income Markets with Decomposed Nominal Rates
Bond Pricing
6. Numerical Results
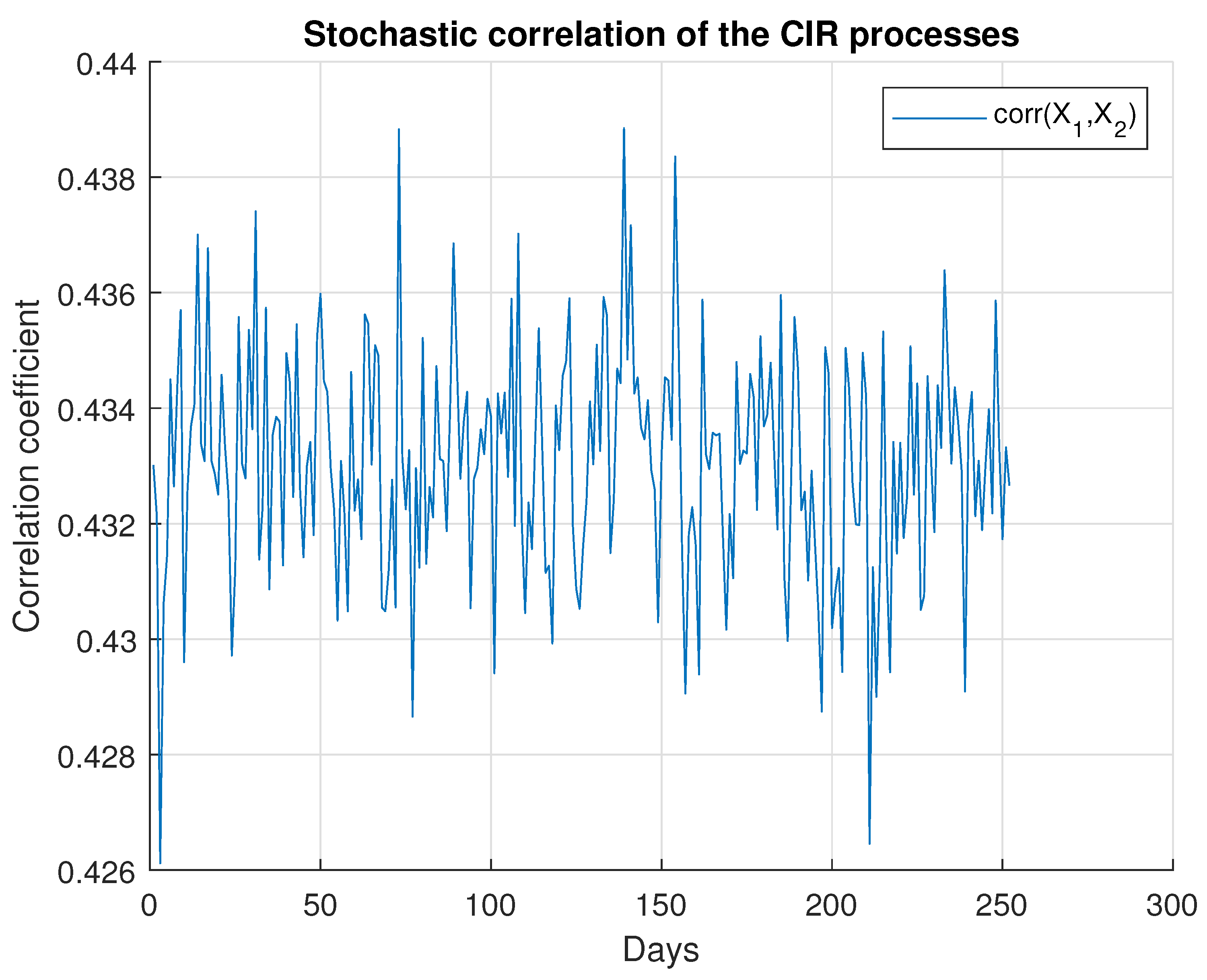
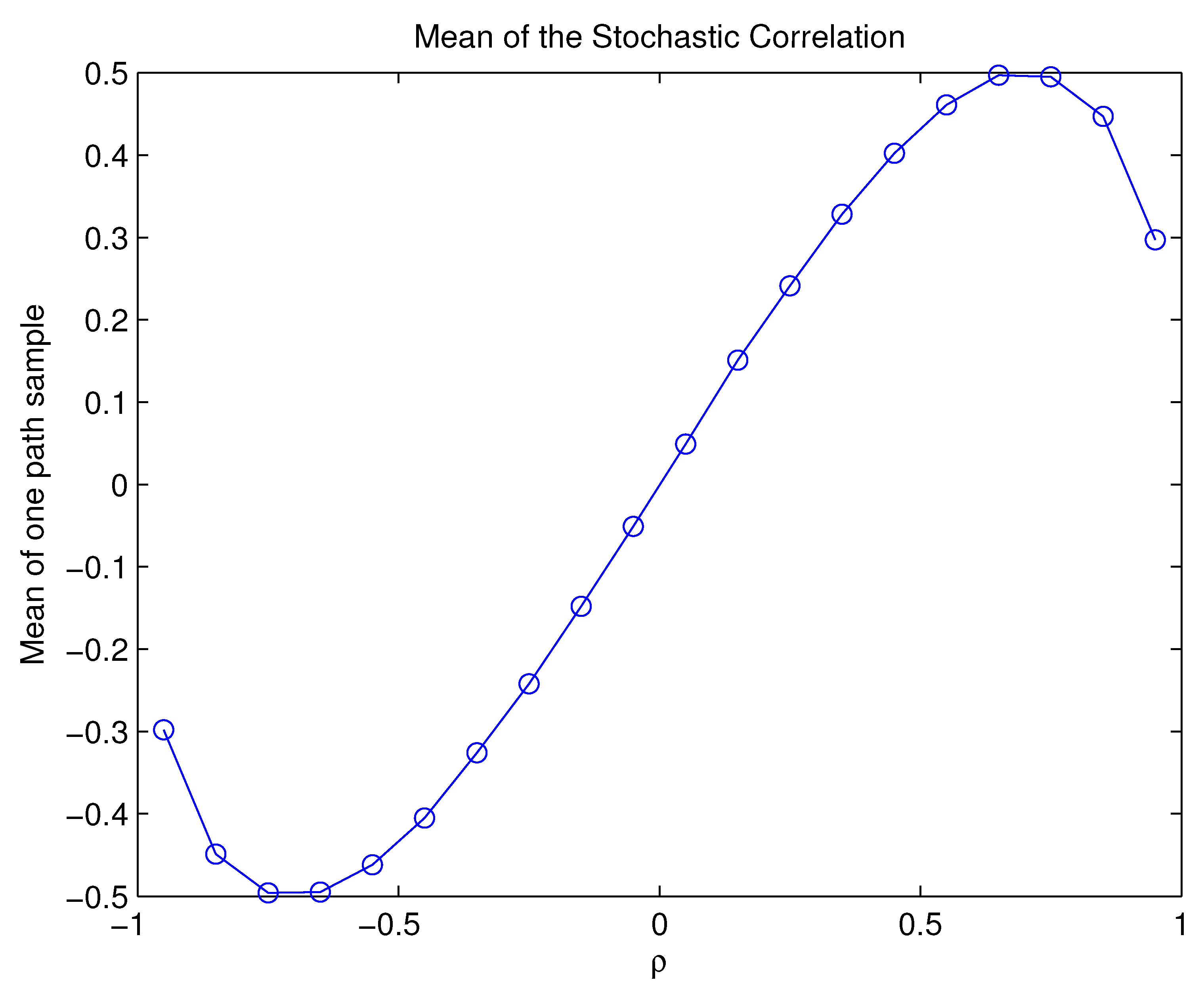
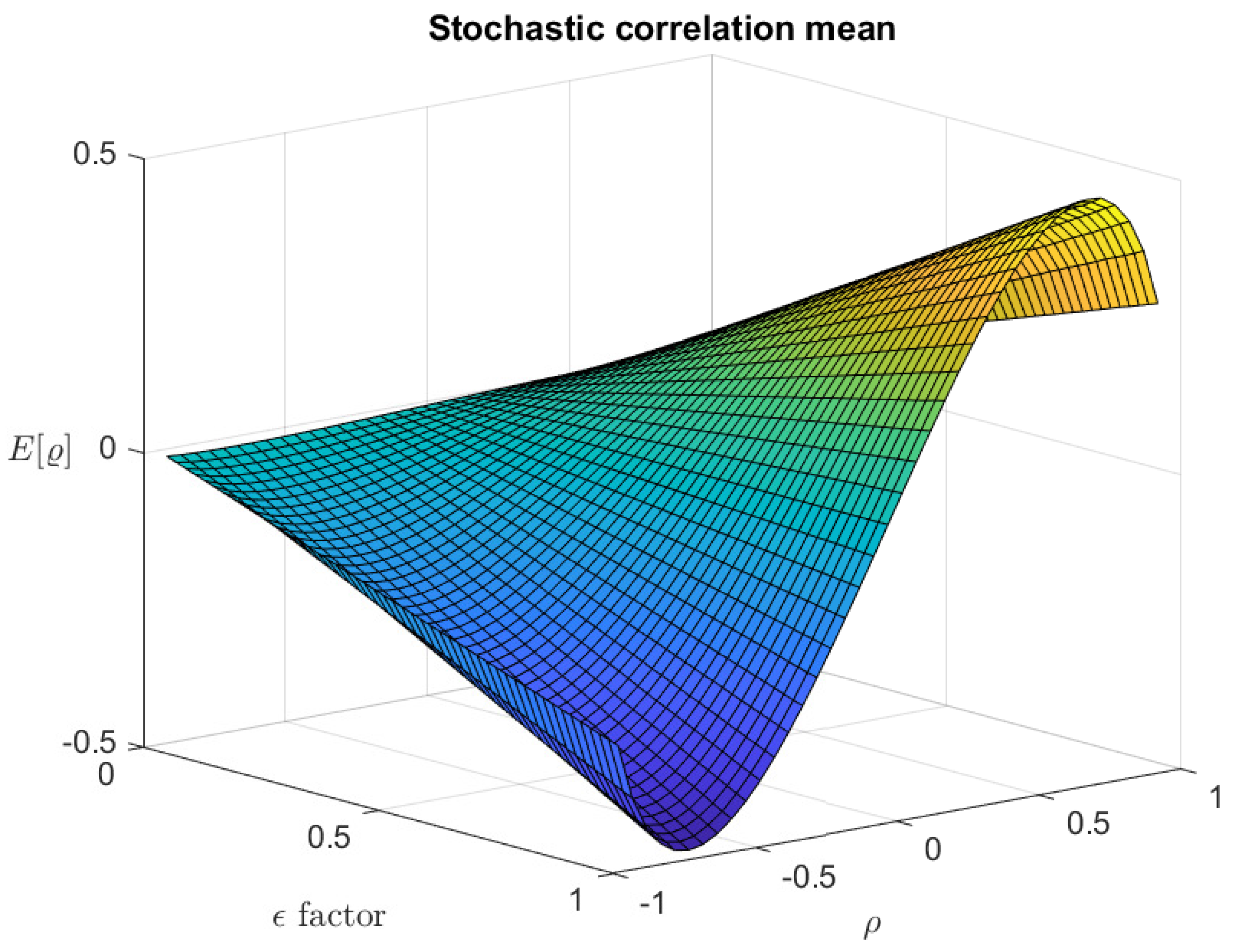
6.1. The Stochastic Correlation Condition (9) Is Mild, at Least at a Certain Niche
6.2. Hand-Conducting the Model via
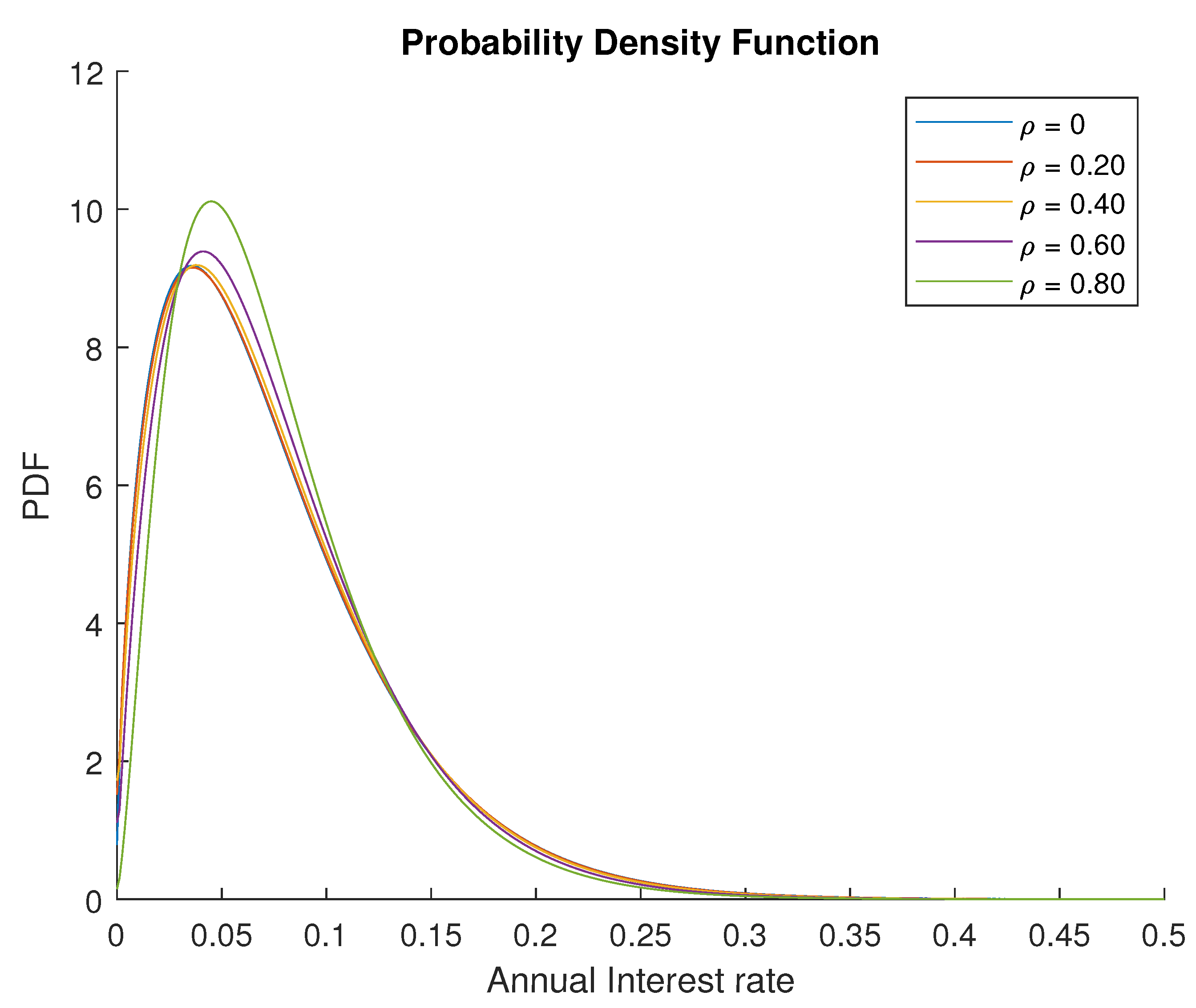
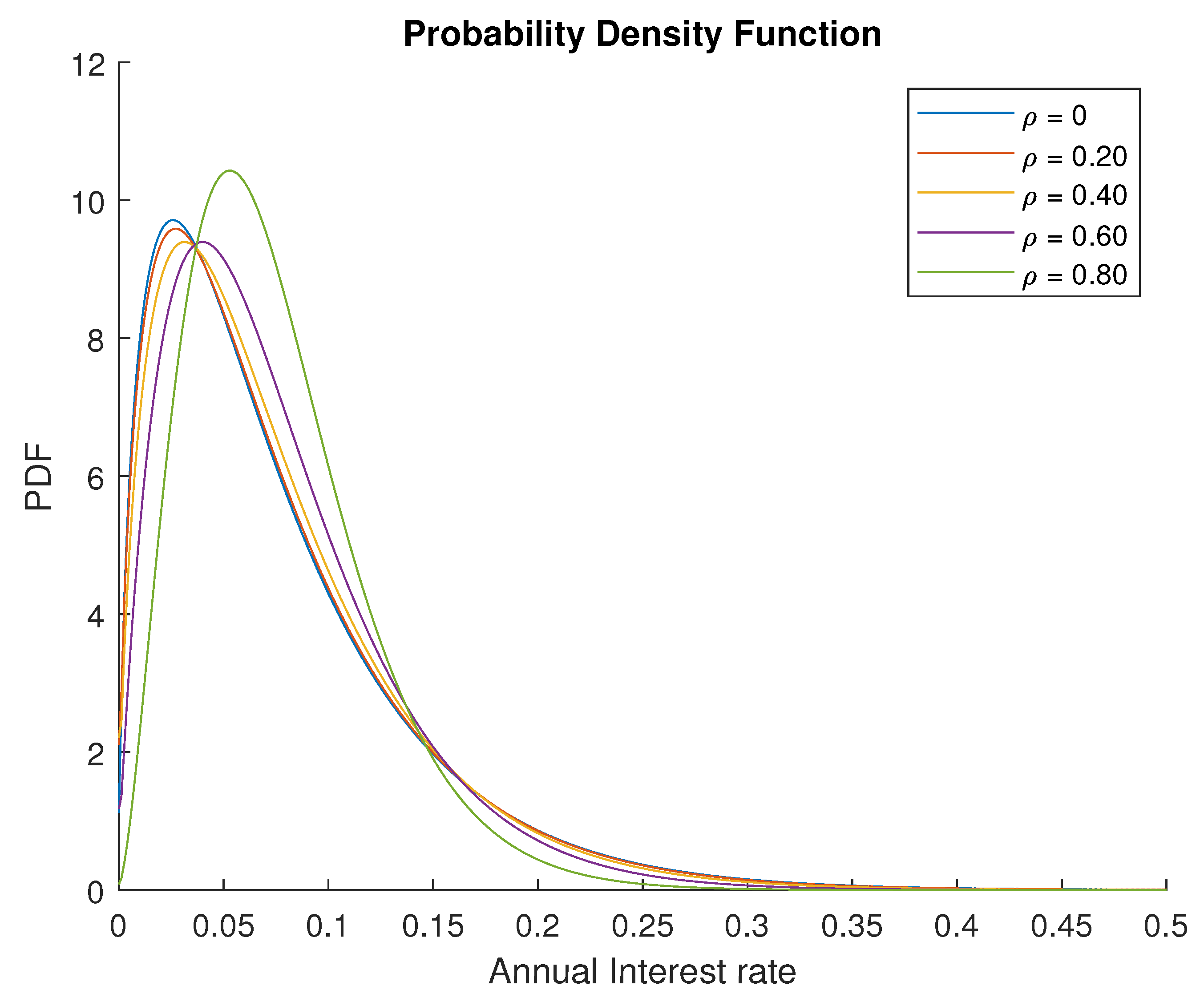
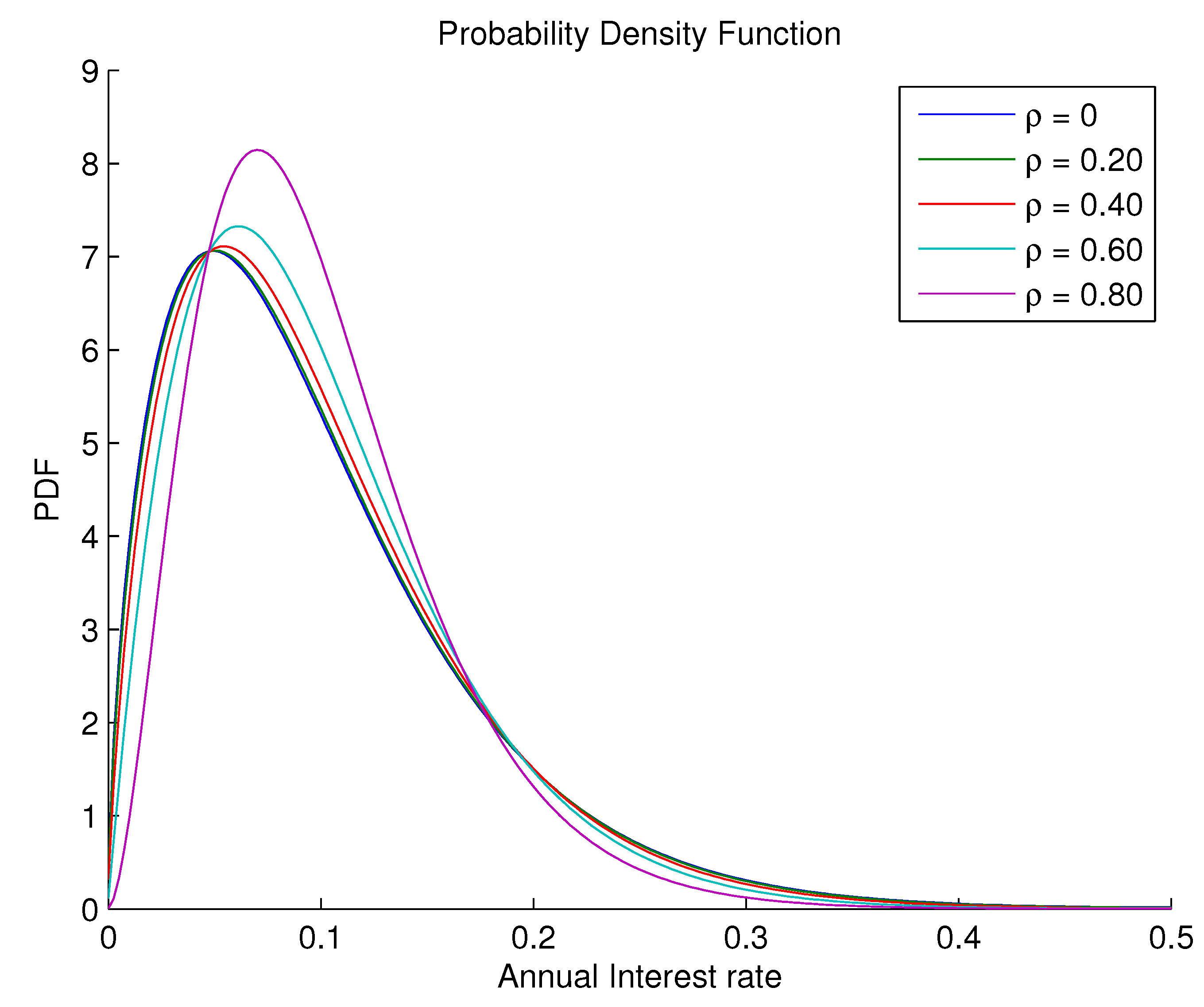
6.3. The Conditional Probability Density Functions of the Short Rate Process
6.4. Pricing the IDI Option
6.5. Term Structure Calibration
7. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Yield Curve Calibration
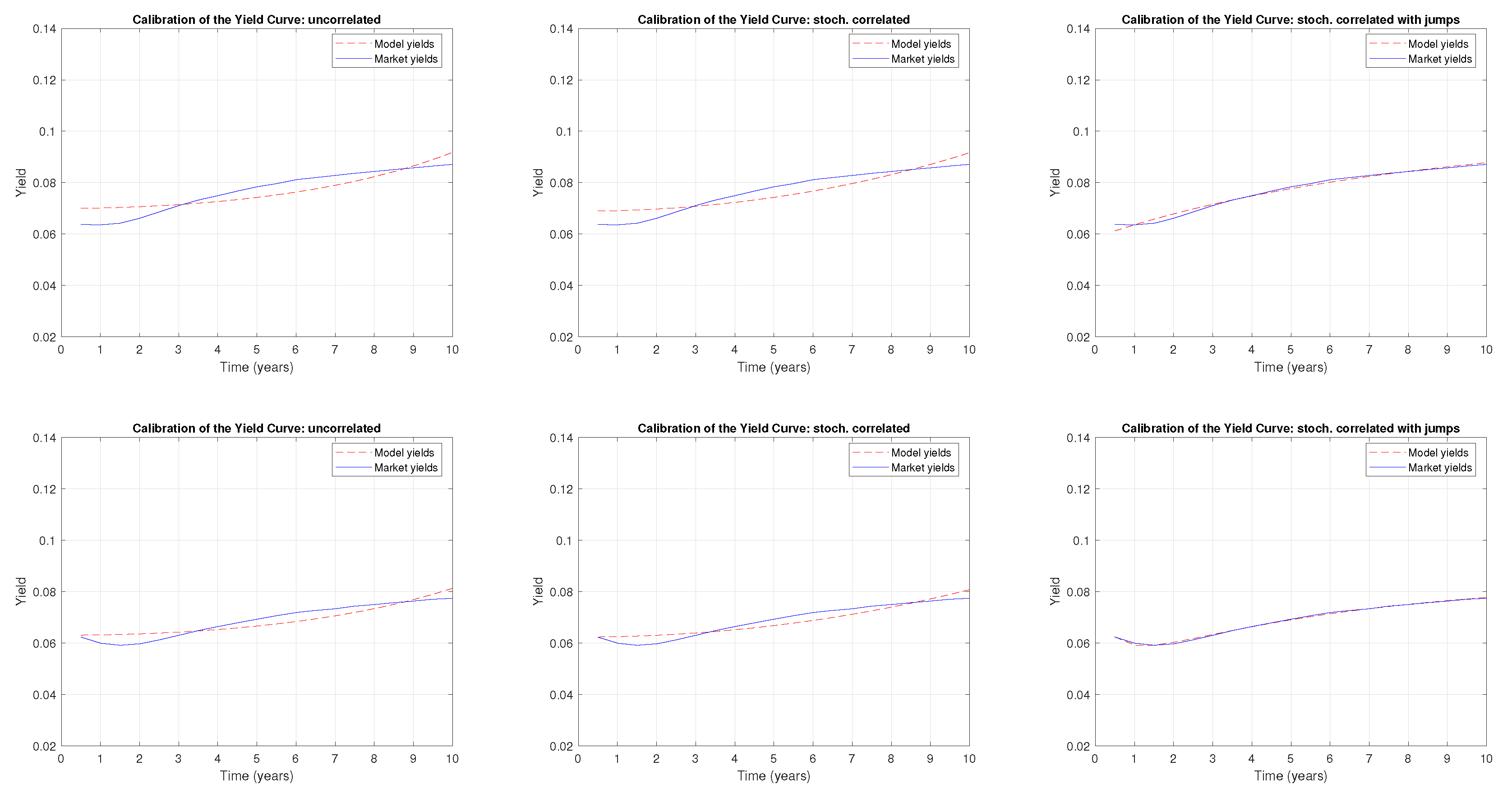
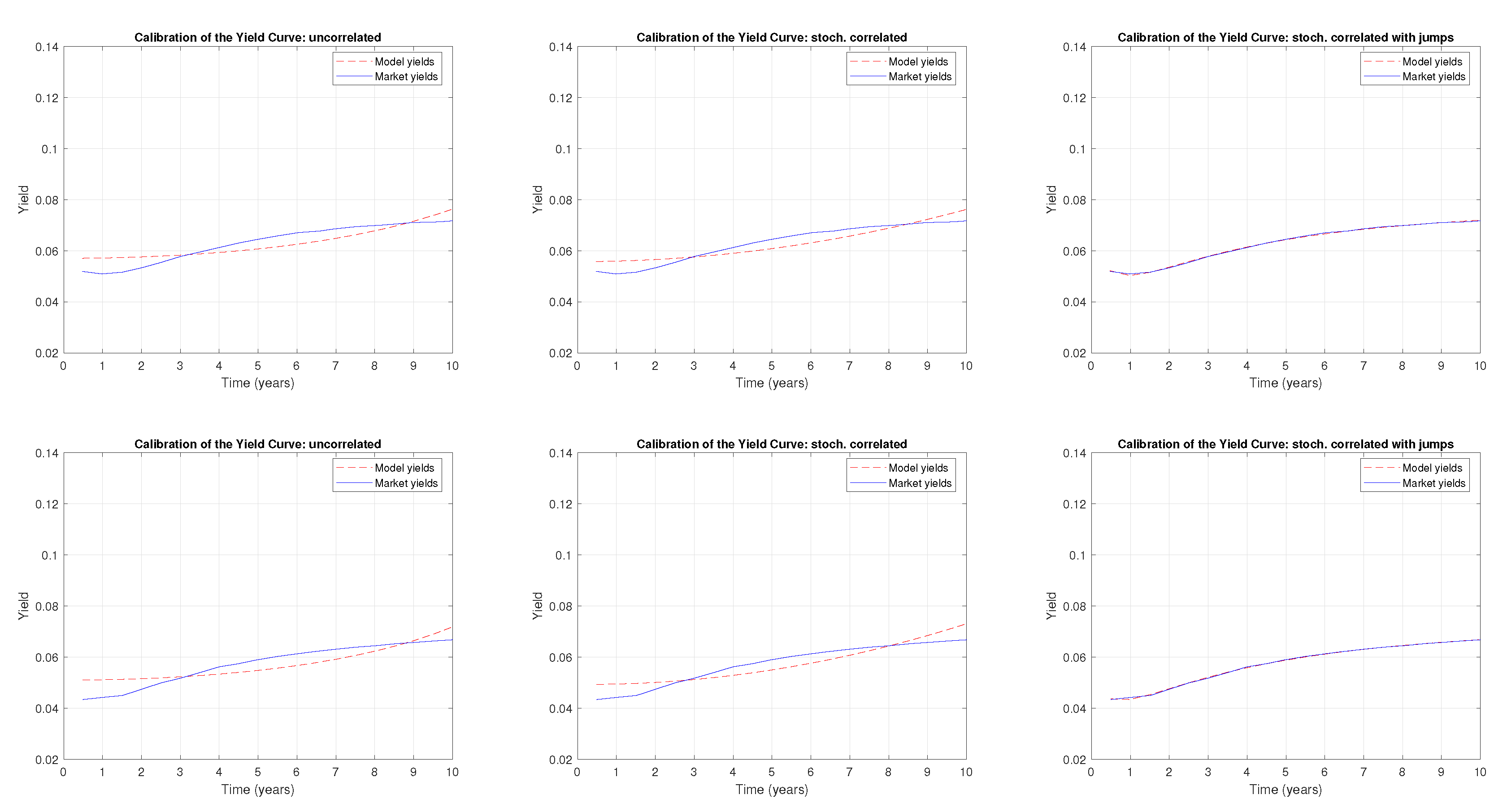
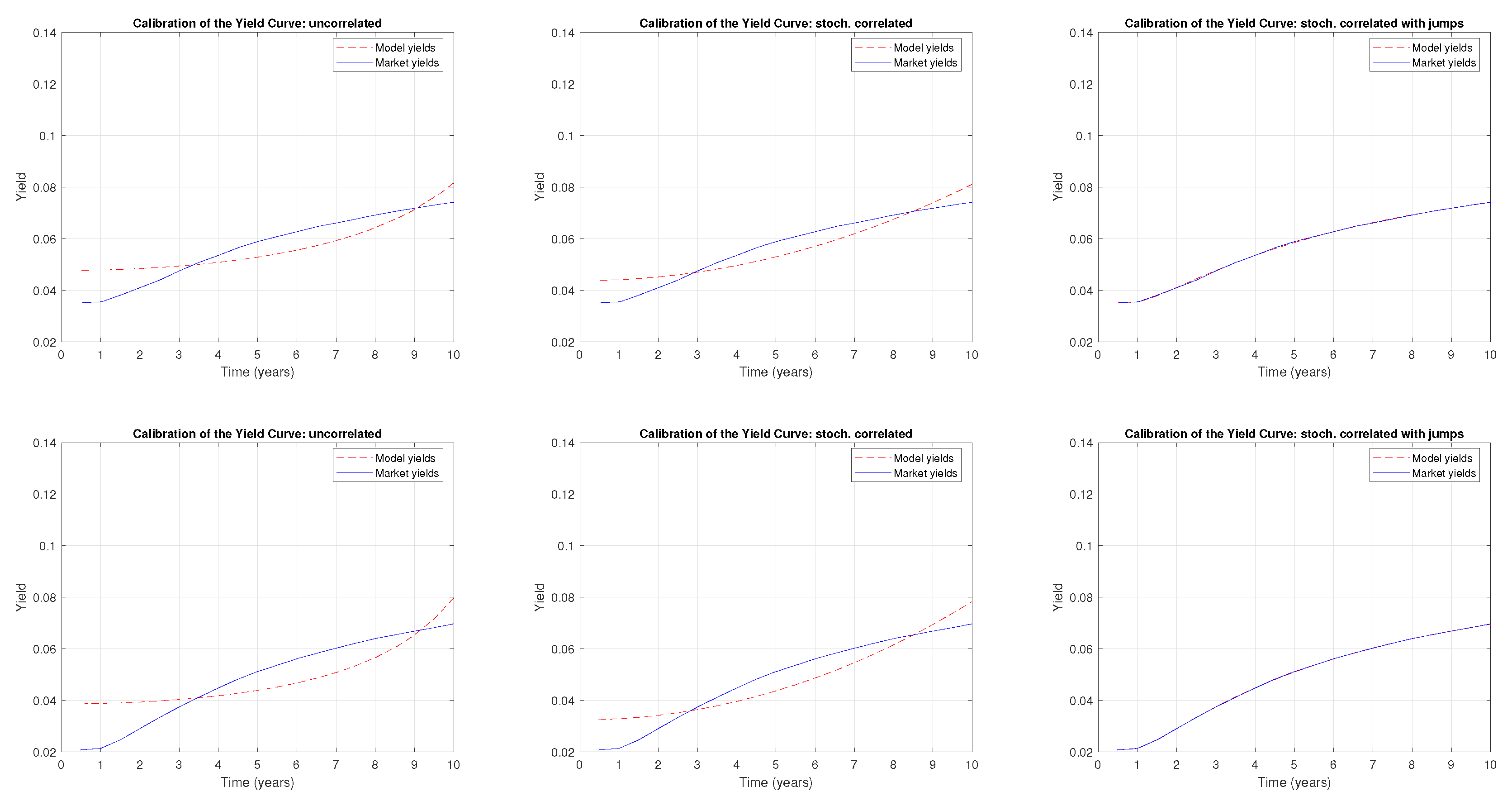
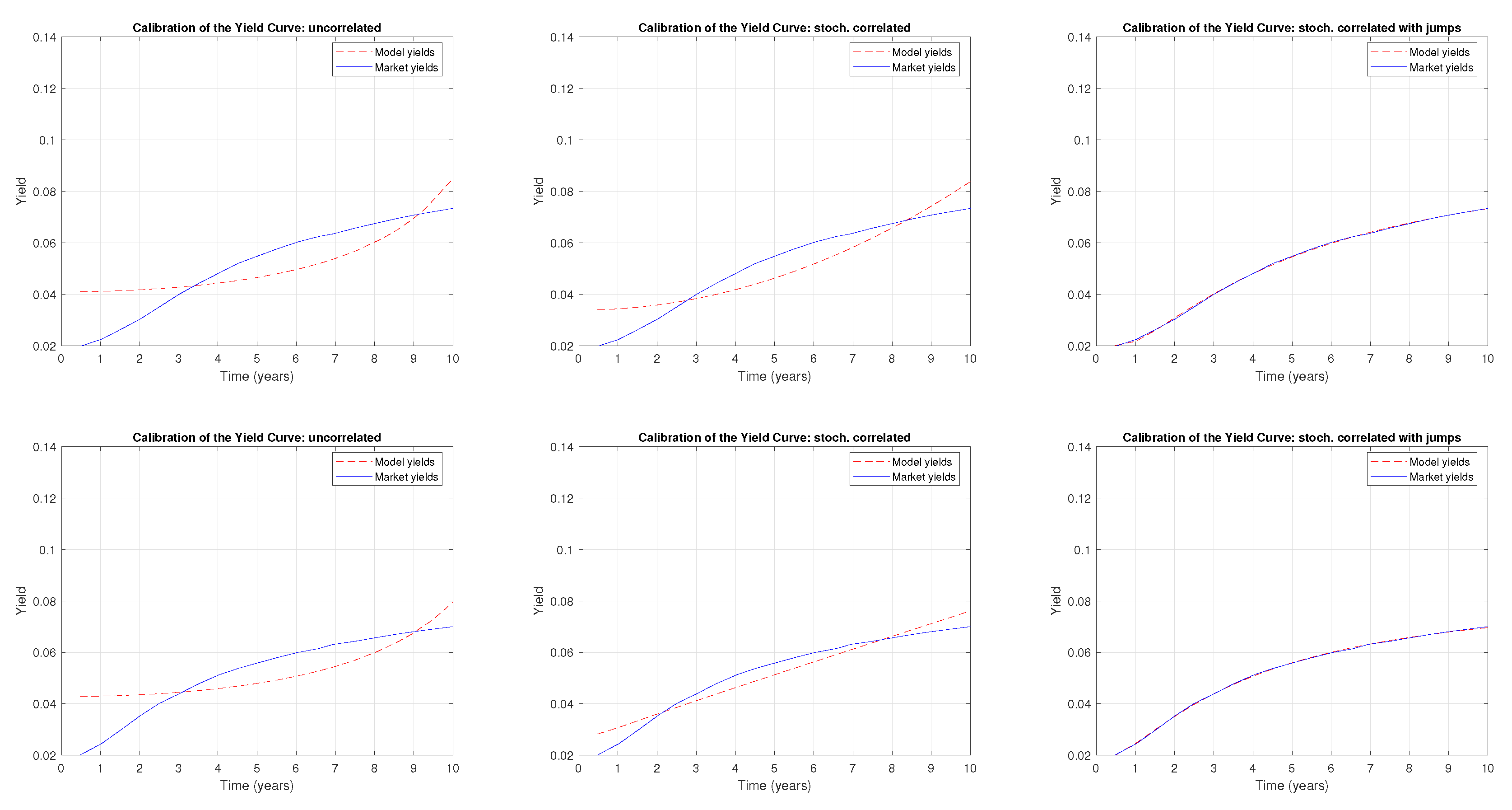

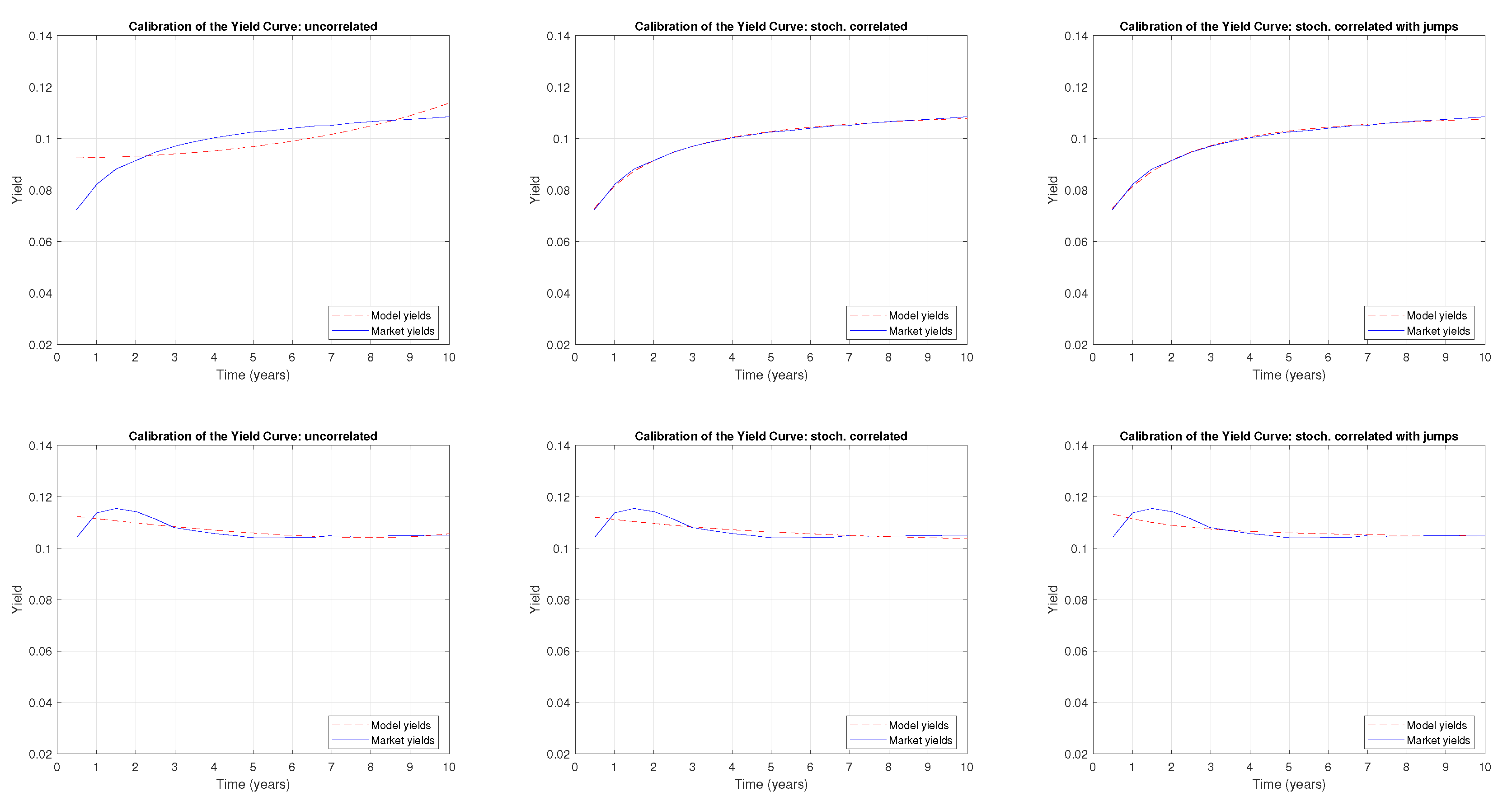
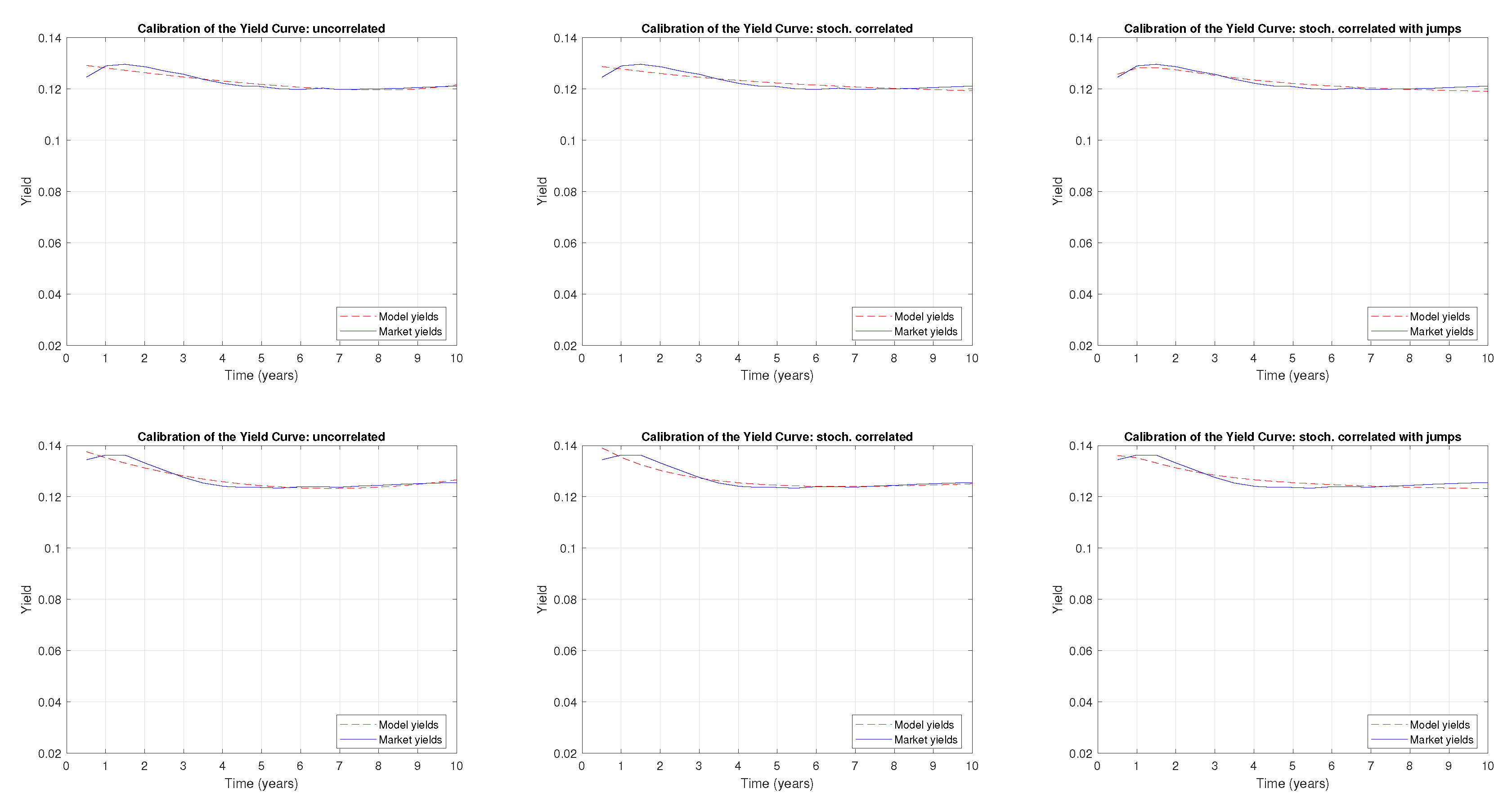
| 1 | We truncated the values to three decimal places. |
References
- Almeida, Caio, and José V. M. Vicente. 2009. Are interest rate options important for the assessment of interest rate risk? Journal of Banking and Finance 33: 1376–1387. [Google Scholar] [CrossRef]
- Bertini, Lorenzo, and Luca Passalacqua. 2008. Modelling interest rates by correlated multi-factor cir-like processes. arXiv arXiv:0807.3898. [Google Scholar] [CrossRef]
- Bouziane, Markus. 2008. Pricing Interest-Rate Derivatives: A Fourier-Transform Based Approach. Berlin: Springer. [Google Scholar]
- Brigo, Damiano, and Fabio Mercurio. 2006. Interest Rate Models—Theory and Practice. Springer Finance. Berlin: Springer. [Google Scholar]
- Cox, John C., Jonathan E. Ingersoll, Jr., and Stephen A. Ross. 1986. A theory of the term structure of interest rates. Econometrica 53: 385–407. [Google Scholar] [CrossRef]
- da Silva, Allan Jonathan, and Jack Baczynski. 2024. Discretely distributed scheduled jumps and interest rate derivatives: Pricing in the context of central bank actions. Economies 12: 73. [Google Scholar] [CrossRef]
- da Silva, Allan Jonathan, Jack Baczynski, and J. F. Silva Bragança. 2019. Path-dependent interest rate option pricing with jumps and stochastic intensities. In Computational Science—ICCS 2019. Lecture Notes in Computer Science. Cham: Springer, vol. 11540, pp. 710–16. [Google Scholar] [CrossRef]
- da Silva, Allan Jonathan, Jack Baczynski, and José Valentim Machado Vicente. 2016. A new finite method for pricing and hedging fixed income derivatives: Comparative analysis and the case of an Asian option. Journal of Computational and Applied Mathematics 297: 98–116. [Google Scholar] [CrossRef]
- da Silva, Allan Jonathan, Jack Baczynski, and José Valentim Machado Vicente. 2023. Recovering probability functions with fourier series. Pesquisa Operacional 43: e267882. [Google Scholar] [CrossRef]
- Duffie, Darrell. 2001. Dynamic Asset Pricing Theory. Princeton Series in Finance. Princeton: Princeton University Press. [Google Scholar]
- Duffie, Darrel, and Kenneth J. Singleton. 2003. Credit Risk: Pricing, Measurement, and Management. Princeton: Princeton University Press. [Google Scholar]
- Fang, Fang, and Cornelis W. Oosterlee. 2008. A novel pricing method for European options based on Fourier-cosine series expansions. SIAM Journal on Scientific Computing 31: 826–48. [Google Scholar] [CrossRef]
- Fisher, Irving. 1930. The Theory of Interest. New York: Macmillan. [Google Scholar]
- Glasserman, Paul. 2004. Monte Carlo Methods in Financial Engineering. Applications of Mathematics: Stochastic Modelling and Applied Probability. New York: Springer. [Google Scholar]
- Harrison, Michael, and Stanley Pliska. 1981. Martingales and stochastic integrals in the theory of continuous trading. Stochastic Processes and their Applications 11: 215–60. [Google Scholar] [CrossRef]
- Jamshidian, Farshid, and Yu Zhu. 1997. Scenario simulation: Theory and methodology. Finance and Stochastics I: 43–67. [Google Scholar]
- Kienitz, Joerg, and Daniel Wetterau. 2012. Financial Modelling: Theory, Implementation and Practice with MATLAB Source. The Wiley Finance Series; Hoboken: Wiley. [Google Scholar]
- Kim, Jongwook, and Junghyo Jo. 2014. Correlated stochastic processes in financial markets. Physica A 406: 230–35. [Google Scholar] [CrossRef]
- Kim, Young Shin, Hyangju Kim, Jaehyung Choi, and Frank J. Fabozzi. 2023. Multi-asset option pricing using normal tempered stable processes with stochastic correlation. The Journal of Derivatives 30: 42–64. [Google Scholar] [CrossRef]
- Leippold, Markus, and Fabio Trojani. 2010. Matrix Affine Jump Diffusions for Multivariate Risk Modeling. Available online: https://ssrn.com/abstract=1274482 (accessed on 18 March 2024).
- Luo, Cuicui, and Luis Seco. 2017. Stochastic correlation in risk analytics: A financial perspective. IEEE Systems Journal 11: 1479–85. [Google Scholar] [CrossRef]
- Márkus, László, and Ashish Kumar. 2019. Comparison of stochastic correlation models. Journal of Mathematical Sciences 237: 810–18. [Google Scholar] [CrossRef]
- Márkus, László, and Ashish Kumar. 2021. Modelling joint behaviour of asset prices using stochastic correlation. Methodology and Computing in Applied Probability 23: 341–54. [Google Scholar] [CrossRef]
- Musiela, Marek, and Marek Rutkowski. 1998. Martingale Methods in Financial Modelling. Berlin and Germany: Springer. [Google Scholar]
- Oksendal, Bernt. 2007. Stochastic Differential Equations, 6th ed. New York: Springer. [Google Scholar]
- Protter, Philip E. 2005. Stochastic Integration and Differential Equations. Berlin and Heidelberg: Springer. [Google Scholar] [CrossRef]
- Reid, William Thomas. 1972. Riccati Differential Equations. Mathematics in Science and Engineering: A Series of Monographs and Textbooks. Cambridge, MA: Academic Press. [Google Scholar]
- Ross, Sheldon M. 2007. Introduction to Probability Models. Amsterdam: Elsevier Science. [Google Scholar]
- Stehlíková, Beáta. 2020. On the bond pricing partial differential equation in a convergence model of interest rates with stochastic correlation. Mathematica Slovaca 70: 995–1002. [Google Scholar] [CrossRef]
- Teng, Long, Matthias Ehrhardt, and Michael Günther. 2017. Modelling and Calibration of Stochastic Correlation in Finance. In Novel Methods in Computational Finance. Cham: Springer. [Google Scholar] [CrossRef]
- Vasicek, Oldrich. 1977. An equilibrium characterization of the term structure. Journal of Financial Economics 5: 177–88. [Google Scholar] [CrossRef]


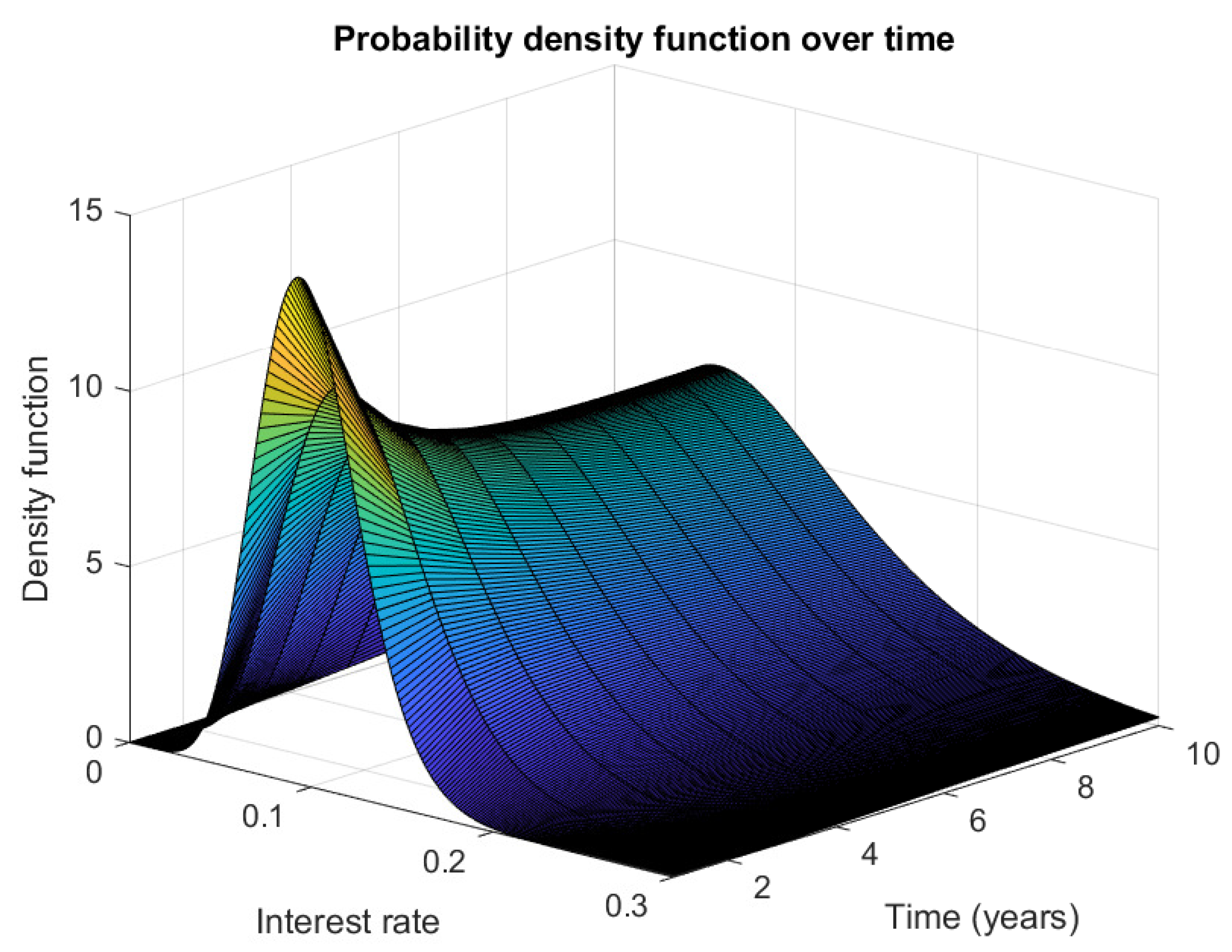
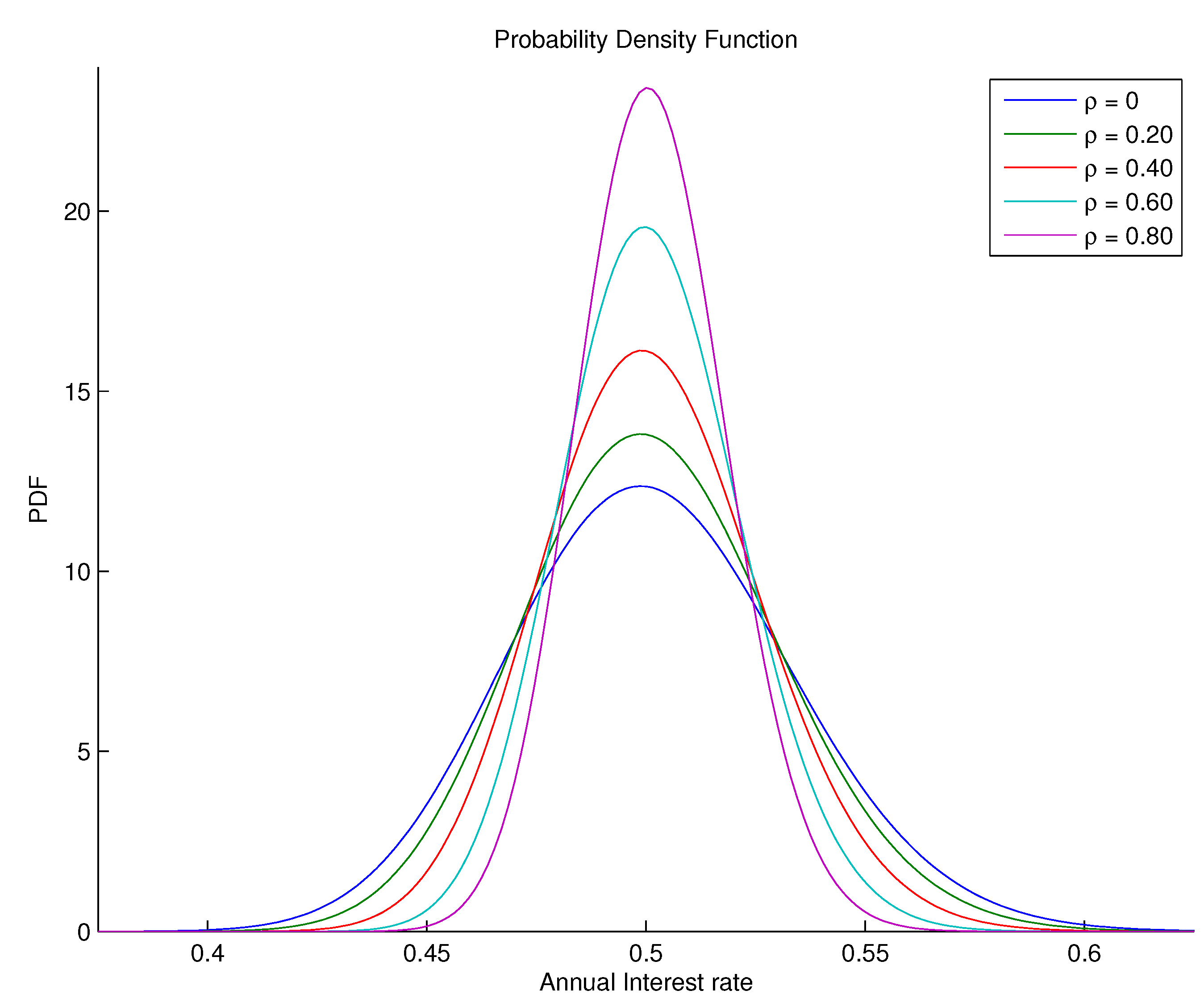

| Parameter | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Value | 11.1819 | 0.0003 | 2.0311 | 0.0291 | 4.7485 | 0.043 | 0.1507 | 0.0518 | 0.1228 |
| 0 | 0.2 | 0.4 | 0.6 | 0.8 | 0.91 | 0.93 | 0.95 | 0.97 | 0.99 | |
|---|---|---|---|---|---|---|---|---|---|---|
| I | 1.0002 | 1.0005 | 1.0005 | 1.0003 | 1.0000 | 1.0000 | 1.0000 | 1.0000 | 1.0000 | 1.0000 |
| 0.117 | 0.117 | 0.018 | 0.013 | 0.000 | 0.000 | 0.017 | 0.053 |
| 0.126 | 0.113 | 0.000 | 0.001 | 0.004 | 0.000 | 0.006 | 0.057 |
| 0.120 | 0.120 | 0.000 | 0.001 | 0.000 | 0.000 | 0.010 | 0.047 |
| 0.128 | 0.128 | 0.015 | 0.004 | 0.000 | 0.000 | 0.023 | 0.028 |
| 0.152 | 0.152 | 0.015 | 0.004 | 0.000 | 0.000 | 0.023 | 0.024 |
| 0.167 | 0.167 | 0.007 | 0.000 | 0.000 | 0.000 | 0.018 | 0.021 |
| 0.167 | 0.167 | 0.007 | 0.000 | 0.000 | 0.000 | 0.019 | 0.022 |
| 0.159 | 0.159 | 0.006 | 0.000 | 0.000 | 0.000 | 0.020 | 0.022 |
| 0.135 | 0.135 | 0.003 | 0.000 | 0.000 | 0.000 | 0.030 | 0.031 |
| 0.120 | 0.121 | 0.000 | 0.006 | 0.000 | 0.000 | 0.036 | 0.035 |
| 0.104 | 0.104 | 0.012 | 0.006 | 0.000 | 0.000 | 0.045 | 0.048 |
| 0.001 | 0.144 | 0.119 | 0.056 | 0.017 | 0.000 | 0.097 | 0.017 |
| 0.000 | 0.157 | 0.174 | 0.053 | 0.014 | 0.000 | 0.117 | 0.013 |
| 0.004 | 0.076 | 0.044 | 0.034 | 0.242 | 0.000 | 0.002 | 0.138 |
| 0.074 | 0.153 | 0.000 | 0.000 | 0.000 | 0.000 | 1.000 | 0.050 | 0.031 | 0.038 |
| 0.055 | 0.161 | 0.003 | 0.021 | 0.000 | 0.000 | 0.999 | 0.050 | 0.062 | 0.000 |
| 0.018 | 0.672 | 0.001 | 0.096 | 0.000 | 0.000 | 1.000 | 0.050 | 0.048 | 0.008 |
| 0.025 | 0.669 | 0.000 | 0.092 | 0.006 | 0.000 | 1.000 | 0.044 | 0.042 | 0.008 |
| 0.031 | 0.702 | 0.011 | 0.092 | 0.000 | 0.000 | 1.000 | 0.049 | 0.042 | 0.002 |
| 0.048 | 0.700 | 0.007 | 0.087 | 0.000 | 0.000 | 1.000 | 0.039 | 0.031 | 0.001 |
| 0.022 | 1.472 | 0.000 | 0.087 | 0.000 | 0.000 | 1.000 | 0.046 | 0.021 | 0.013 |
| 0.188 | 3.235 | 0.000 | 0.003 | 2.283 | 0.000 | 1.000 | 0.037 | 0.000 | 0.027 |
| 2.657 | 2.679 | 0.003 | 0.000 | 3.797 | 1.570 | 1.000 | 0.046 | 0.000 | 0.035 |
| 3.006 | 19.999 | 0.000 | 0.000 | 15.026 | 3.801 | 0.991 | 0.050 | 0.002 | 0.039 |
| 4.014 | 19.999 | 0.000 | 0.000 | 14.843 | 4.087 | 0.990 | 0.049 | 0.000 | 0.069 |
| 0.547 | 19.602 | 0.000 | 0.000 | 15.273 | 0.352 | 1.000 | 0.050 | 0.000 | 0.113 |
| 0.600 | 19.601 | 0.000 | 0.000 | 15.271 | 0.335 | 1.000 | 0.050 | 0.000 | 0.130 |
| 0.592 | 19.601 | 0.002 | 0.008 | 15.271 | 0.367 | 1.000 | 0.045 | 0.001 | 0.146 |
| 0.052 | 0.003 | 0.180 | 0.007 | 0.183 | 0.144 | 0.000 | 0.025 | 0.059 | 0.000 | 9.987 | 0.006 |
| 0.217 | 0.558 | 0.001 | 0.030 | 0.454 | 3.144 | 0.518 | 0.050 | 0.073 | 0.002 | 10.088 | 0.005 |
| 0.150 | 0.558 | 0.015 | 0.011 | 0.555 | 3.146 | 0.521 | 0.050 | 0.038 | 0.025 | 10.087 | 0.006 |
| 0.164 | 0.559 | 0.016 | 0.017 | 0.533 | 3.143 | 0.520 | 0.048 | 0.042 | 0.009 | 10.087 | 0.006 |
| 0.212 | 0.558 | 0.000 | 0.025 | 0.427 | 3.141 | 0.519 | 0.043 | 0.038 | 0.005 | 10.088 | 0.004 |
| 0.191 | 0.559 | 0.000 | 0.030 | 0.432 | 3.140 | 0.520 | 0.046 | 0.023 | 0.007 | 10.089 | 0.005 |
| 0.128 | 0.561 | 0.000 | 0.028 | 0.424 | 3.138 | 0.524 | 0.050 | 0.020 | 0.008 | 10.101 | 0.005 |
| 0.000 | 0.576 | 0.001 | 0.013 | 0.510 | 3.259 | 0.607 | 0.049 | 0.012 | 0.008 | 10.385 | 0.005 |
| 0.035 | 0.574 | 0.000 | 0.009 | 0.909 | 3.268 | 0.610 | 0.050 | 0.023 | 0.003 | 10.413 | 0.009 |
| 0.031 | 0.574 | 0.000 | 0.000 | 0.960 | 3.268 | 0.610 | 0.050 | 0.040 | 0.000 | 10.410 | 0.009 |
| 0.023 | 0.573 | 0.014 | 0.000 | 1.072 | 3.269 | 0.610 | 0.050 | 0.061 | 0.000 | 10.399 | 0.013 |
| 0.021 | 0.573 | 0.080 | 0.000 | 1.120 | 3.269 | 0.610 | 0.049 | 0.116 | 0.000 | 10.394 | 0.020 |
| 0.000 | 0.579 | 0.635 | 0.000 | 0.931 | 3.264 | 0.615 | 0.050 | 0.117 | 0.000 | 10.104 | 0.075 |
| 0.000 | 0.594 | 0.518 | 0.000 | 1.323 | 3.161 | 0.607 | 0.032 | 0.132 | 0.000 | 10.221 | 0.088 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
da Silva, A.J.; Baczynski, J.; Vicente, J.V.M. A Stochastically Correlated Bivariate Square-Root Model. Int. J. Financial Stud. 2024, 12, 31. https://doi.org/10.3390/ijfs12020031
da Silva AJ, Baczynski J, Vicente JVM. A Stochastically Correlated Bivariate Square-Root Model. International Journal of Financial Studies. 2024; 12(2):31. https://doi.org/10.3390/ijfs12020031
Chicago/Turabian Styleda Silva, Allan Jonathan, Jack Baczynski, and José Valentim Machado Vicente. 2024. "A Stochastically Correlated Bivariate Square-Root Model" International Journal of Financial Studies 12, no. 2: 31. https://doi.org/10.3390/ijfs12020031
APA Styleda Silva, A. J., Baczynski, J., & Vicente, J. V. M. (2024). A Stochastically Correlated Bivariate Square-Root Model. International Journal of Financial Studies, 12(2), 31. https://doi.org/10.3390/ijfs12020031






