Velocity of Money and Productivity Growth: Explaining the 2% Inflation Target in the U.S. (1959–2007) †
Abstract
1. Introduction
2. The Evolution of Money and Transaction Cost Savings
- Economizing on resource costs due to the lack of double coincidence of wants. This occurs in a barter economy. Resources are wasted due to search costs and storage/spoilage costs (Tobin 1992).
- Economizing on the resource costs to produce commodity money Friedman (1951). In a barter economy, this cost is incurred repeatedly to sustain most of the stock of “mediums of exchanges” needed for transactions, as many of these barter goods are perishable. In a commodity (metallic) standard, production costs only occur for new money and constitute a deadweight loss for society in the sense that resources are transferred to a non-productive and non-consumable goods sector of the economy. In the transition from barter to commodity money (CM), the cost savings are getting smaller over time because the marginal cost of producing CM is rising. In a fiat money (FM) economy, the cost of producing money is essentially zero.
- Economizing on the costs generated by an inefficient banking clearinghouse system (Norman et al. 2006). In a commodity money system that maintains convertibility, clearing transactions with physical money settlements is costly. The fractional reserve system of the past and our current FM system have drastically reduced these costs.
- Avoiding recessionary deflations: deflations caused by secular money supply constraints have typically been associated with an economic slowdown (Friedman 1951; Bordo and Filardo 2005). Guerrero and Parker (2006), for example, find that a higher rate of deflation reduces the subsequent economic growth rate (even if it does not always lead to recession). Thus, there is reason to believe that deflation is bad for economic growth, even if it has become a relatively rare experience for most developed economies in the postwar era.
- Advances in transaction methods, such as credit cards and online shopping and banking, all of which boost the velocity of money.
3. The Optimal Quantity and Velocity of Money
4. Data and Testing Framework
5. Test Results with M1 and Other Measures of Narrow Money
6. A Near-2% Inflation Target and a Constant Money Growth Rule
7. A Long-Term Taylor-Type Rule Compatible with a Money Growth Rule
8. A Near-2% Target to Avoid “Bad” Deflations
9. Conclusions and Extensions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. The Credit Card Market and the “Banks” General Credit Market
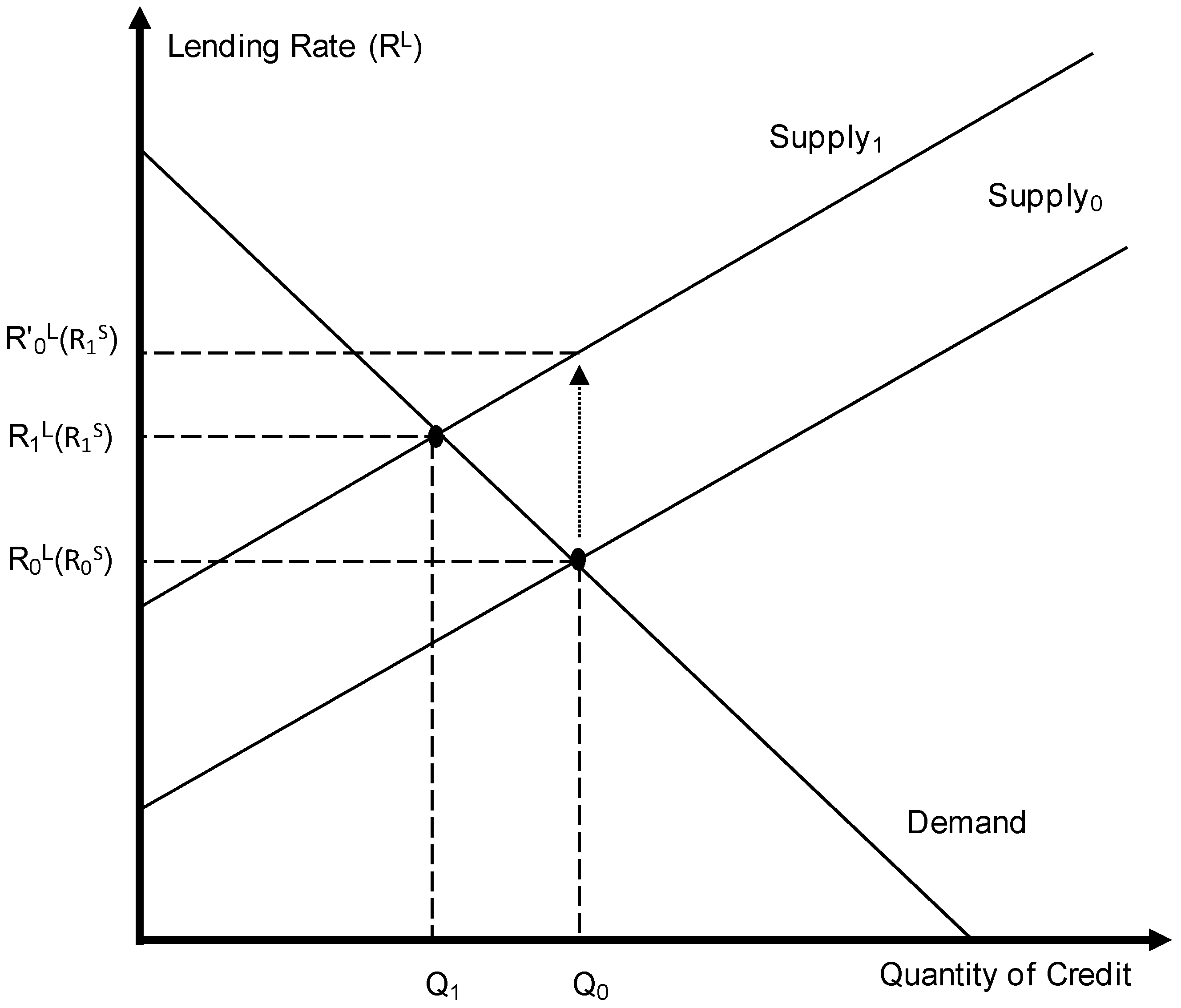

Appendix B. Proof of Proposition 1
Appendix C. The 2008–2023 Period. A Review of Unconventional Monetary Policies (UMPs) and A Robustness Test of the Long-Run Velocity Relationship
- I.
- In the aftermath of the 2008 GFC, the U.S. Federal Reserve implemented new monetary policies (UMPs).
- (a)
- Quantitative Easing (QE): Initially, the US Federal Reserve dropped the target federal funds rate (the borrowing rate between banks) to nearly zero in 2008. As policy rates approached their lower bounds, the conventional implicit guidance of the Taylor rule became and remained inefficient. Trapped at their lowest bound, policy rates lost their power to re-stimulate the economy. As a result, inflation expectations became de-anchored. Threats to price stability were asymmetric and skewed towards a deflationary recession. In that context, the U.S. Federal Reserve initiated Quantitative Easing for the first time in its history in November 2008. That is, it expanded the monetary base (central bank deposits and cash) and purchased government and other securities. QE went beyond straight open market operations as the Federal Reserve balance sheet expanded to acquire assets like high-grade mortgage-backed securities (MBSs), which was a major break with tradition. By March 2009, the Fed had USD 1.75 trillion in bank debt, MBS, and Treasury notes on its balance sheet. In June 2010, the Fed had reached a peak of USD 2.1 trillion in assets. Between 2007 and 2017, the Fed implemented three rounds of QE, and its assets increased from USD 882 billion before the crisis to USD 4.473 trillion—mostly reflecting assets that were not government securities. The Federal Reserve also successfully provided short-term liquidity and collateral to businesses when money market funds failed to do so via the Term Securities Lending Facility and Commercial Paper Finding Facility.
- (b)
- Forward Guidance (FG): The Fed undertook to provide markets with explicit signals regarding its commitment to maintain policy rates at the lower bound for a significant length of time, thus guiding medium- to long-term interest rate expectations.
- II.
- The 2020 Covid crisis and subsequent inflation acceleration.
- (a)
- Quantitative Easing during the Covid Crisis of 2020 (QE-4): Overall, the U.S. gross domestic product (GDP) decreased by roughly 2.2% in 2020 due to the Covid pandemic. In reaction to this economic collapse, the Federal Reserve implemented a new round of Quantitative Easing (QE-4). Rates were already low heading into the pandemic, as the Fed funds rate was between 1.5 and 1.75% leading into March 2020. The Fed cut interest rates twice in that month, bringing them to the effective lower bound (0–0.25%). Because rates were already so low, the stimulus to the economy from reducing rates to the lower bound was limited. At the 15 March 2020, meeting, the Fed began QE4, which included monthly purchases of USD80 billion of agency debt and USD40 billion of mortgage-backed securities. As of 15 November 2023, the Fed’s balance sheet amounted to roughly 7.1 trillion U.S. dollars.
- (b)
- Drastic interest rate policy to combat the resurgence of inflation: In June 2021, inflation in the United States started to rise, with headline PCE at 4.0 percent annually. In the June 2021 FOMC meeting, the committee decided not to raise the policy rate. Although inflation did not slow as expected and, in fact, it rose instead, the FOMC did not increase the policy rate from zero until its March 2022 meeting, at which time headline PCE inflation had risen to more than 6 percent. Subsequently, it raised the federal funds rate at its fastest rate of increase in the history of the federal reserve, going from 0.25% to 4.25% in the span of a year. While this episode falls more under the purview of conventional monetary policy (tightening interest rates in reaction to higher inflation), the wild swing in policy can be seen as unconventional and attributable to an underreaction by the Fed to the economy rebounding faster than expected, which then generated the Fed’s “overraction” with the fast pace raises of the policy rate. Figure A3 below showcases the behavior of key interest rates during these episodes.

- III.
- Robustness test of the model for the period 1959–2023
| The “endogeneized” variable is the Log of money velocity (M1 or AdjustedM1), which following Johansen’s method (1995) has a normalized coefficient of 1. The Adjusted M1 variable is adjusted for the effects of QE-1 to QE-4 (see description in Appendix C). Equations shown are those for whom the trace statistics for the rank of the cointegrating equations reject that the rank is greater than 1 at the 95% level. The Chi2 statistics shows that all parameters in the equations are together significant at least at the 99% level. All coefficients in all the cointegrating equations are significant at least at the 10% level, except the dummy d5971 which is not significant. The High and Low columns represent the 95% confidence interval around the estimate of the coefficient for Ln(realGDPc). The AIC, BIC and HQ criteria in the righmost columns indicate the goodness of fit for the VECM. | ||||||||||||||||
| Lag | Optimal Lag Criterion | Ln Velocity | Trace | RS | RL | Lnreal GDPc | d5971 | d2023 | Const. | Low | High | Ln Lklhood | Chi2 | AIC | BIC | HQ |
| 1 | None | M1 | 56.9 | 24.20 | −19.78 | 1.07 | −0.02 | 1.50 | −1.51 | 1.50 | 1.13 | 4289 | 160 | −33.1 | −33 | −32.9 |
| 2 | HQIC, SBIC | M1 | 39.9 | 62.15 | −62.37 | 0.92 | −1.00 | −1.01 | 0.24 | −0.16 | 1.99 | 4423 | 74 | −34 | −33.7 | −33.3 |
| 1 | None | M1 ♣ | 60.8 | 26.10 | −20.40 | 1.02 | −0.03 | −1.48 | −1.31 | 0.57 | 1.47 | 4293 | 136 | −33.1 | −33 | −32.8 |
| 2 | FPE, AIC, HQIC, SBIC | M1 ♣ | 45.7 | 60.23 | −58.70 | 0.87 | −0.58 | −0.98 | 0.41 | −0.14 | 1.88 | 4426 | 65 | −34 | −33.7 | −33.2 |
| 1 | SBIC | Adj. M1 | 58.7 | 31.72 | −31.55 | 1.33 | −0.24 | −1.41 | −2.03 | 0.63 | 2.03 | 3974 | 95 | −30.7 | −30.6 | −30.4 |
| 1 | SBIC | Adj. M1 ♣ | 58.7 | 14.06 | −15.57 | 1.00 | −0.18 | −1.14 | −1.12 | 0.61 | 1.39 | 3977 | 150 | −30.7 | −30.5 | −30.3 |
| 4 | LR | Adj. M1 ♣ | 50.7 | 40.72 | −41.66 | 0.94 | −0.45 | −1.23 | 0.23 | 0.17 | 1.71 | 4066 | 63 | −30.9 | −30.1 | −29 |
| 2 | SBIC | Adj. M1 ♣♣ | 45.9 | 9.25 | −8.39 | 1.00 | −0.08 | -- | −1.50 | 0.73 | 1.28 | 3585 | 218 | −27.5 | −27.2 | −26.8 |
| 1 | For instance, in a speech at the St. Louis Fed 28th Annual Policy Conference, Bernanke (2003) introduces the concept of an optimal long-run inflation rate. The speech’s main reference is an article by Coenen et al. (2003), who support a 2% target in order to keep deflation at bay. Clearly, the 2% value has great significance as a threshold point in their model. Nevertheless, no attempt is made in this or other articles in the literature to formally relate the 2% threshold to any underlying macro or microeconomic factors. 2% is indeed a focal point in the literature. Many articles either cite 2% explicitly or give a desired narrow range of around 2%. Summers (1991) asserts that the optimal long-run inflation rate is between 2 and 3%. However, his argument is brief and sketchy and does not justify why this specific range is best. Fischer (1996) lists a series of informal arguments centered on the Phillips curve and the difficulties of dealing with a zero inflation rate for stimulating the economy during slowdown periods. Goodfriend (2002) states that “Evidence from U.S. monetary history suggests that such leeway would be enough to enable a central bank to preempt deflation and stabilize the economy against most adverse shocks”. Only recently, and contrary to the mainstream literature, Blanchard et al. (2010) argue for an inflation target of around 4% in response to the 2008 financial crisis and to deal with liquidity traps. |
| 2 | This is not trivial, and the argument requires a separate and extensive analysis of the interaction between the markets for credit card loans and general “bank” loans. Putting these elements together and given that the transactional cost savings of money are reduced when more money-like substitutes (credit cards) are used, I find that there must be a positive relation between transactional cost savings and the financial sector’s net asset returns. |
| 3 | The idea has a well-established tradition. Richard Cantillon, John Locke, Knut Wicksell, Irving Fisher, and Milton Friedman all pointed to innovations as a factor speeding-up the velocity of money (Humphrey 1993). Nevertheless, measures of technical progress have not yet been empirically tied to the velocity of money, as conducted here. |
| 4 | Due to the non-traditional QE policy measures taken by the U.S. Fed, I intentionally cut my sample before the onset of the 2008 financial crisis to test this model. We also witnessed a revival of unconventional policy measures by the Federal Reserve in the wake of the 2020 covid crisis; thus, that period is not conducive to studying the long-run dynamics of the velocity of money either. Nevertheless, robustness tests are conducted in Appendix C that cover these periods. |
| 5 | The same argument is given by Benchimol and Qureshi (2020), who estimate money demand for the US over 1959–2008. |
| 6 | In Appendix C, I conduct a discussion of these extraordinary measures and a robustness test of the model for the period 1959–2023 to illustrate how these relationships are impacted. The robustness tests still validate the approach, as the discrepancies in the long-run equilibrium estimates can be explained and reduced using economically grounded arguments and adjustments to M1 that correct for the effects of UMPS. |
| 7 | |
| 8 | In this case, I consider that the relevant head count (i.e., per-capita) includes the population of individuals, businesses, and non-federal government entities holding accounts at depository institutions. I thus define the average member of society as a representative domestic economic agent who is a composite of all these categories. |
| 9 | I define transaction technology as the medium of exchange (money or barter goods) and the associated devices or methods used to facilitate transactions (for example, credit cards are associated with fiat money). |
| 10 | A limitation of this analysis is that since the late 1990s, the source of profits for the banking sector has not been based only on generating a positive net interest margin but also on banking and securitization fees and gains on securities and derivatives. |
| 11 | I recognize that, strictly speaking, real GDP/capita is not the same as labor productivity measured by output/hour. However, the two are highly correlated. Using FRED quarterly data between 1959 and 2007, the correlation between the two variables is 99.2%, and 98.5% over 1959–2023. Along a steady-state growth path for the economy, it is essentially impossible to separate out the economic effects of real GDP per-capita growth vs. that of labor-augmenting technical progress, as in the long run, these two variables are inextricably tied. This reasoning can be applied to progress in transaction cost savings. My analysis here does not focus on transitional dynamics but on steady-state growth. In that case, the sole engine of economic growth is technical progress. |
| 12 | It is possible that more primitive types of money will also receive an efficiency boost. Assuming A > 0 rules out the case where the old type of money leapfrogs the newer type. |
| 13 | Note that the cost-saving function must satisfy . Using the Quantity Theory of Money equation expressed as , the real stock of money per-capita is given by . Thus, the function can also be written as . As long as the velocity of money is not falling to zero and the stock of real money per-capita is not declining, the condition plus the proper bound on the constant A are sufficient to ensure that . In the limit case where money velocity drops to zero, this constraint can still be met as long as real money per-capita grows faster than the speed of velocity decline. |
| 14 | Along Friedman’s line, Wolman (1997) focuses on individual money holdings. He considers the marginal savings in terms of wage earnings due to less time spent transacting. Lucas (2000) also focuses part of his paper on developing a model that incorporates a shopping time constraint, again within a monetized economy. |
| 15 | Wolman (1997) assumes satiation in money holdings in order to reproduce Friedman’s (1969) result. Mulligan and Sala-i-Martin (1997) assert that the assumption of satiation can be done away with in their model as long as seignorage falls as the interest rate drops to zero. In other words, the stock of money does not grow faster than the rate of decline of the interest rate. Some cash-in-advance models appear to predict Friedman’s result without resorting to assuming satiation. However, they assume that frictionless barter is achievable as the first-best solution. Recently, a new section of the literature integrates market frictions into hybrid models combining general equilibrium and search models (Aruoba et al. 2007). These models reach conclusions at odds with Friedman’s (1969) recommendation. |
| 16 | The sample stopped in 2007 to avoid the noise created by the series of extraordinary short-term measures implemented by the Fed to fight the effects of the 2008 financial crisis. and the reprisal of such measures during the Covid crisis in 2020, followed in 2022 by a complete reversal with fast monetary tightening. In Appendix C, I conduct a robustness test for the period after 2007. |
| 17 | The site was no longer supported, and the data were updated as of 2012. The old data can be obtained upon request from the authors. |
| 18 | Cynamon, Dutkowsky, and Jones’s database tracks commercial demand deposit programs only after 1991. Thus, M1S may have been slightly biased downward prior to 1991. |
| 19 | The use of VECM for estimating money demand functions is fairly standard (see, for example, Haug and Tam (2007)). Benchimol and Qureshi (2020) estimate the US money demand on a quarterly basis, as we do here from 1959 until before the onset of the GFC in 2008 and use the VECM framework in their article. |
| 20 | I run Augmented Dickey and Fuller (1979) tests with trends for the log of velocity of money (M1, M1RS, and M1S) as well as for SM1 and the log of real GDP per-capita and with drift for the two interest rates. In all cases, the null hypothesis of non-stationarity cannot be rejected at the 1%, 5%, and 10% levels, except for the T-Bill rate, which is rejected at the 5% level but not at the 1% level. The detailed results are obtainable from the author. The other possible issue is the collinearity between the two interest rates used here. However, rather than imposing restrictions, I let the VECM structure speak for itself and determine whether there is an independent cointegrating equation governing the behavior of these two rates, with short-term adjustments away from equilibrium. The empirical results do not support that contention. Any short-term relationship between the two rates will be uncovered by the short-term dynamics of the VECM and thus separated from the cointegrating relationship (3). |
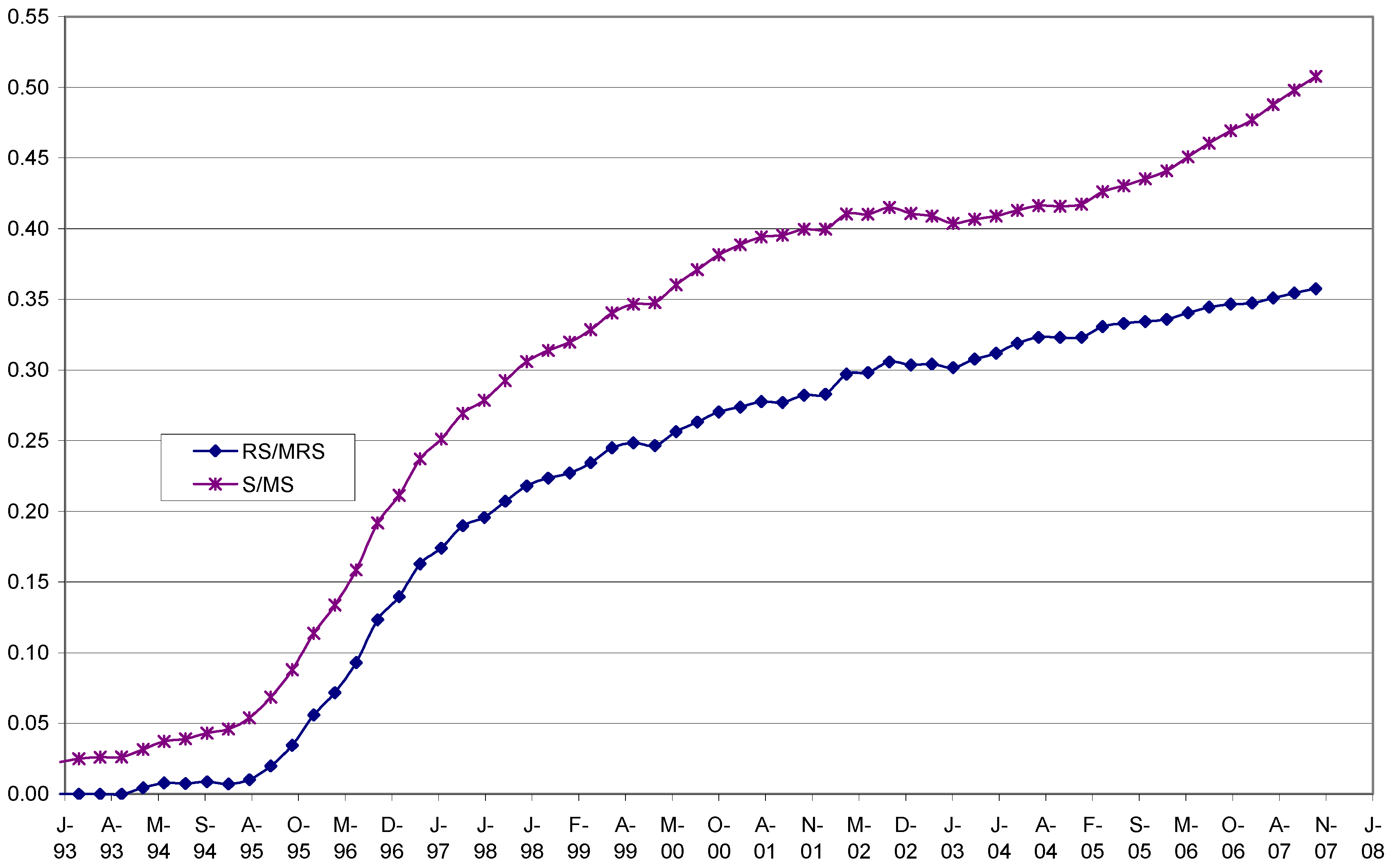
| 21 | The definition is different for M1RS and M1S. For M1RS, the variable SM1 only includes retail sweeps. Total sweeps are included in M1S. |
| 22 | I excluded instances of rank equal to 2, which occurred mostly when the number of lags equaled 4. These multiple cointegrating equations were economically meaningless. I also ran post-estimation diagnostics on the VECM. Overall, the presented equations were part of VECMs that had stable roots with normally distributed disturbances (except for the short term dynamics of the log of real GDP/capita), and exhibited some residual autocorrelation at all lags up to lag 4. The results are available from the author upon request. |
| 23 | I consider that leverage ratios with a value above 100 are outliers and, therefore, are removed from the sample. These are banks and financial institutions with the following NAICS codes: 522110 commercial banks, 522120 savings institutions, 522130 credit unions, and 522190 other depository credit intermediation institutions. After 1999, I added other institutions due to the 1999 banking deregulation. The additional codes are: 522210, 220, 291, 292, 294, 390; 523220; 524126; 127; and 210. |
| 24 | I will discuss later why M1 is a better choice to capture the actual leverage parameter. |
| 25 | I run VECM “control” experiments without dummy variables, and as can be seen from the bottom half of Table 3, none of the experiments conform to the predictions of the theory, and there is no discernible pattern for any of the money measures. |
| 26 | Interestingly, as more lenient reserve requirement rules were enacted since the 1990s, one should have expected leverage to increase in depository institutions, but in fact, one observes the opposite in Figure 3. This might be due to the counteracting effect of the Basel Accords regarding capital adequacy, especially the dip after 1990. |
| 27 | |
| 28 | There is a literature contrasting the effects of either rule in terms of their impact on economic stability (Evans and Honkapohja 2003). |
| 29 | A classic article that predicts Friedman’s result is Abel (1987). Mulligan and Sala-i-Martin (1997) provide a great survey in which they find that Friedman’s conclusion is model-dependent. Of course, theoretically, Friedman’s (1969) proposal is subject to the same limitations as mentioned before, given the assumptions of (1) satiation and (2) a pre-existing monetized economy. Also, it should be logically clear that Friedman’s concept is distinct from the issue of a liquidity trap. That is, monetary policy is becoming ineffective to kick-start the economy as the short-term interest is close to zero (Goodfriend 2000; Clouse et al. 2003; Bernanke et al. 2004). |
| 30 | While the most recognizable feature of credit cards is their ability to substitute for money, I argue that the key feature of this transmission mechanism is the grace period, which ultimately drives the demand by convenience users and possibly entices credit card users to carry a positive balance due to a lack of vigilance over meeting the card companies’ conditions (Ausubel 1991). |
| 31 | Faugere and Van Erlach (2009) find that the Fisher effect on an after-tax basis is indeed present in equity index valuations and Treasury yields. |
| 32 | These figures are available from the author upon request. |
| 33 | This is assuming the traditional commercial banking model prevails, whereas securitization has changed this situation since the mid-1990s. Currently, about 60% of all credit card loans are securitized (Getter 2008). |
| 34 | The higher lending rates (long-term) will put pressure on the long-term bond market, so the yields will go up there too. |
| 35 | The analysis is conducted so that the aggregate amount of credit is held constant once the total effects of Figure A1 and Figure A2 are combined. This is a key point because the comparative statics analysis of the transactional cost savings function requires the neat asset return to change, holding M1 constant. |
| 36 | It should also be clear to the reader that the above analysis is consistent with the bank “lending channel” approach to monetary policy as developed by Bernanke and Blinder (1988). |
| 37 | I assume that spoilage costs affect interest paid and principal in a pure barter economy, so that (1 + R)(1 − δ) − 1 ≥ 0. This is similar to comparing (in the standard macroeconomic growth model) the interest rate in an economy where capital depreciates vs. the case of zero depreciation. With zero depreciation, (1 + R) equals the marginal productivity of capital. With positive depreciation, the new rate is (1 + Rnew) = (1 + R − δ). However, it becomes (1 + Rnew) = (1 + R)(1 − δ). if the interest earned (in goods terms) is subjected to spoilage as well, for example, due to having to store principal and interest before payment. |
| 38 | I assume that the marginal benefits of holding barter goods are net of current spoilage costs and that the transaction cost savings from using money are net of the cost of producing money. |
| 39 | A case in which money and barter can co-exist for a while is that of hyperinflation. A good reference for a model of the transition from barter to fiat money is Ritter (1995). Ritter’s model is based on Kiyotaki and Wright (1993). |
| 40 | My approach implicitly necessitates the presence of a medium of account in the barter economy (a unit basket of goods), which can translate into the real money unit. For example, the medium of account could be a mixture of the reference baskets in the CPI and PPI indexes, and the unit of account is 1 unit of that mixed basket. For a discussion of the difference between medium of exchange vs. medium of account vs. unit of account, see McCallum (2003). |
| 41 | We ran the pre-diagnostics for rank and excluded all cointegrating equations with ranks above 2. |
References
- Abel, Andrew B. 1987. Optimal Monetary Growth. Journal of Monetary Economics 19: 437–50. [Google Scholar] [CrossRef]
- Adrian, Tobias, and Hyun Song Shin. 2009. Prices and Quantities in the Monetary Policy Transmission Mechanism. Staff Report No. 396. New York: Federal Reserve Bank of New York. Available online: https://www.econstor.eu/bitstream/10419/60742/1/622777742.pdf (accessed on 6 February 2023).
- Anderson, Richard G. 1997. Federal Reserve Board Data on OCD Sweep Account Programs. Available online: http://research.stlouisfed.org/aggreg/swdata.html (accessed on 30 May 2002).
- Anderson, Richard G. 2003. Retail Deposit Sweep Programs: Issues for Measurement, Modeling and Analysis. Working Paper 2003-026. St. Louis: Federal Reserve Bank of St. Louis. [Google Scholar]
- Aruoba, S. Boragan, Guillaume Rocheteau, and Chirstopher Waller. 2007. Bargaining and the value of money. Journal of Monetary Economics 54: 2636–55. [Google Scholar] [CrossRef]
- Ausubel, Lawrence M. 1991. The Failure of Competition in the Credit Card Market. The American Economic Review 81: 50–81. [Google Scholar]
- Ausubel, Lawrence M. 1997. Credit Card Defaults, Credit Card Profits, and Bankruptcy. The American Bankruptcy Law Journal 71: 249–70. [Google Scholar]
- Baba, Yoshihisa, David F. Hendry, and Ross M. Starr. 1992. The Demand for M1 in the U.S.A., 1960–1988. The Review of Economic Studies 59: 25–61. [Google Scholar] [CrossRef]
- Bailey, Martin J. 1956. The welfare cost of inflationary finance. Journal of Political Economy 64: 93–110. [Google Scholar] [CrossRef]
- Baumol, William J. 1952. The Transactions Demand for Cash: An Inventory Theoretic Approach. Quarterly Journal of Economics 66: 545–56. [Google Scholar] [CrossRef]
- Benchimol, Jonathan, and Irfan Qureshi. 2020. Time-varying money demand and real balance effects. Economic Modelling 87: 197–211. [Google Scholar] [CrossRef]
- Bernanke, Ben S. 2003. Remarks by Governor Ben S. Bernanke At the 28th Annual Policy Conference: Inflation Targeting: Prospects and Problems, Federal Reserve Bank of St. Louis, St. Louis, Missouri October 17, 2003. Available online: http://www.federalreserve.gov/Boarddocs/Speeches/2003/20031017/default.htm (accessed on 20 December 2023).
- Bernanke, Ben S., and Alan S. Blinder. 1988. Is it Money or Credit, or Both, or Neither? Credit, Money, and Aggregate Demand. American Economic Review 78: 435–39. [Google Scholar]
- Bernanke, Ben S., Thomas Laubach, Frederic S. Mishkin, and Adam S. Posen. 1999. Inflation Targeting: Lessons from the International Experience. Princeton: Princeton University Press. [Google Scholar]
- Bernanke, Ben S., Vincent R. Reinhart, and Brian Sack. 2004. Monetary Policy at the Zero Bound: An Empirical Assessment. Brookings Papers on Economic Activity 2: 1–78. [Google Scholar] [CrossRef]
- Blanchard, Olivier, Giovanni Dell’Ariccia, and Paulo Mauro. 2010. Rethinking Macroeconomic Policy. In IMF Staff Position Note. SPN/10/03. Washington: International Monetary Fund. [Google Scholar]
- Bordo, Michael D., and Andrew Filardo. 2005. Deflation in a Historical Perspective. BIS Working Paper No. 186. Basel: Monetary and Economic Department. [Google Scholar]
- Brown, Tom, and Lacey Plache. 2006. Paying with Plastic: Maybe Not So Crazy. The University of Chicago Law Review 73: 63–86. [Google Scholar]
- Brunner, Karl, and Allan H. Meltzer. 1989. Monetary Economics. Oxford: Basil Blackwell. [Google Scholar]
- Caldara, Dario, Etienne Gagnon, Enrique Martinez-Garcia, and Christopher J. Neely. 2020. Monetary Policy and Economic Performance since the Financial Crisis. In Finance and Economics Discussion Series 2020–2065. Washington: Board of Governors of the Federal Reserve System. [Google Scholar] [CrossRef]
- Calem, Paul S., and Loretta J. Mester. 1994. Consumer Behavior and the Stickiness of Credit Card Interest Rates. The American Economic Review 85: 1327–36. [Google Scholar]
- Carlson, John B., Dennis L. Hoffman, Benjamin D. Keen, and Robert H. Rasche. 2000. Results of a Study of the Stability of Cointegrating Relations Comprised of Broad Monetary Aggregates. Journal of Monetary Economics 46: 345–83. [Google Scholar] [CrossRef]
- Clemente, Jesus, Antonio Montañés, and Marcelo Reyes. 1998. Testing for a Unit Root in Variables with a Double Change in the Mean. Economics Letters 59: 175–82. [Google Scholar] [CrossRef]
- Clouse, James, Dale Henderson, Orphanides Athanasios, David Small, and Peter L. Tinsley. 2003. Monetary Policy When the Nominal Short-Term Interest rate is Zero. Topics in Macroeconomics 3. [Google Scholar] [CrossRef]
- Coenen, Gunter, Athanasios Orphanides, and Volker Wieland. 2003. Price Stability and Monetary Policy Effectiveness When Nominal Interest Rates are Bounded at Zero. Frankfurt am Main: European Central Bank. [Google Scholar]
- Dickey, David A., and Wayne A. Fuller. 1979. Distributions of the Estimators for Autoregressive Time Series with a Unit Root. Journal of American Statistical Association 74: 427–81. [Google Scholar]
- Dotsey, Michael. 1984. An Investigation of Cash Management Practices and Their Effects on the Demand for Money. FRB Richmond Economic Review 70: 3–12. [Google Scholar]
- Dueker, Michael J. 1999. Measuring monetary policy inertia in target Fed funds rate changes. Review, Federal Reserve Bank of St. Louis 81: 3–10. [Google Scholar] [CrossRef]
- Dutkowsky, Donald H., and Barry Z. Cynamon. 2003. Sweep Programs: The Fall of M1 and Rebirth of the Medium of Exchange. Journal of Money, Credit and Banking 35: 263–79. [Google Scholar] [CrossRef]
- Dutkowsky, Donald H., Barry Z. Cynamon, and Barry E. Jones. 2006. US Narrow Money for the Twenty-First Century. Economic Inquiry 44: 142–52. [Google Scholar] [CrossRef]
- Engle, Robert F., and Clive Granger. 1987. Co-integration and Error Correction: Representation, Estimation, and Testing. Econometrica 55: 251–76. [Google Scholar] [CrossRef]
- Ennis, Humberto M., and Tim Sablik. 2019. Large Excess Reserves and the Relationship between Money and Prices. Brief 19-02. Richmond: Federal Reserve Bank of Richmond Economic. [Google Scholar]
- Evans, George W., and Seppo Honkapohja. 2003. Friedman’s Money Supply Rule vs. Optimal Interest Policy. Working Paper University of Oregon. Oxford: Blackwell Publishing. [Google Scholar]
- Faugere, Christophe, and Julian Van Erlach. 2009. A Required Yield Theory of Stock Market Valuation and Treasury Yield Determination. Financial Markets, Institutions and Instruments 18: 27–88. [Google Scholar] [CrossRef]
- Fischer, Stanley. 1996. Why Are Central Banks Pursuing Long-Run Price Stability. In Achieving Price Stability. Kansas City: Federal Reserve Bank of Kansas City, pp. 7–34. [Google Scholar]
- Friedman, Milton. 1951. Commodity-Reserve Currency. The Journal of Political Economy 59: 203–32. [Google Scholar] [CrossRef]
- Friedman, Milton. 1960. A Program for Monetary Stability. New York: Fordham University Press. [Google Scholar]
- Friedman, Milton. 1969. The Optimum Quantity of Money and Other Essays. Piscataway: Transaction Publishers. [Google Scholar]
- Geanakoplos, John, and Pradeep Dubey. 2010. Credit Cards and Inflation. Games and Economic Behavior 70: 325–353. [Google Scholar] [CrossRef]
- Getter, Darryl E. 2008. The Credit Card Market: Recent Trends, Funding Cost Issues, and Repricing Practices; Congressional Research Services Report for Congress. RL34393. Washington, DC. Available online: https://digital.library.unt.edu/ark:/67531/metadc819334/m1/1/ (accessed on 6 February 2023).
- Goodfriend, Marvin. 2000. Overcoming the Zero Bound on Interest Rate Policy. Journal of Money, Credit and Banking 32: 1007–35. [Google Scholar] [CrossRef]
- Goodfriend, Marvin. 2002. Monetary Policy in the New Neoclassical Synthesis: A Primer. International Finance 90: 165–91. [Google Scholar] [CrossRef]
- Greenspan, Alan. 2007. The Age of Turbulence. New York: The Penguin Press. [Google Scholar]
- Guerrero, Federico, and Elliott Parker. 2006. Deflation and Recession: Finding the Empirical Link. Economics Letters 93: 12–17. [Google Scholar] [CrossRef]
- Haug, Alfred A., and Julie Tam. 2007. A closer look at long-run U.S. Money Demand: Linear or non-linear error-correction with M0, M1 or M2? Economic Inquiry 45: 363–76. [Google Scholar] [CrossRef]
- Hetzel, Robert L. 1989. M2 and Monetary Policy. Federal Reserve Bank of Richmond Economic Review 75: 14–29. [Google Scholar]
- Hodrick, Robert J., Narayana Kocherlakota, and Deborah Lucas. 1991. The Variability of Velocity in Cash-in-Advance Models. Journal of Political Economy 99: 358–84. [Google Scholar] [CrossRef]
- Humphrey, Thomas M. 1993. The Origins of Velocity Functions. Federal Reserve Bank of Richmond Economic Quarterly 79: 1–17. [Google Scholar]
- Ireland, Peter N. 1994. Money and Growth: An Alternative Approach. The American Economic Review 84: 47–65. [Google Scholar]
- Ireland, Peter N. 1995. Endogenous Financial Innovation and the Demand for Money. Journal of Money, Credit and Banking 27: 107–23. [Google Scholar] [CrossRef]
- Ireland, Peter N. 2008. On the Welfare Cost of Inflation and the Recent Behavior of Money Demand. American Economic Review 99: 1040–52. [Google Scholar] [CrossRef]
- Jafarey, Saqib, and Adrian Masters. 2003. Output, Prices, and the Velocity of Money in Search Equilibrium. Journal of Money, Credit and Banking 35: 871–88. [Google Scholar] [CrossRef]
- Johansen, Soren. 1988. Statistical Analysis of Cointegration Vectors. Journal of Economic Dynamics and Control 12: 231–54. [Google Scholar] [CrossRef]
- Johansen, Soren. 1991. Estimation and Hypothesis Testing of Cointegration Vectors in Gaussian Vector Autoregressive Models. Econometrica 59: 1551–80. [Google Scholar] [CrossRef]
- Johansen, Soren. 1995. Likelihood Based Inference in Cointegrated Vector Autoregressive Models. Oxford: Oxford University Press. [Google Scholar]
- King, Robert G., Charles I. Plosser, James H. Stock, and Mark W. Watson. 1991. Stochastic Trends and Economic Fluctuations. American Economic Review 81: 819–40. [Google Scholar]
- Kiyotaki, Nobuhiro, and Randall Wright. 1993. A Search-Theoretic Approach to Monetary Economics. American Economic Review 83: 63–77. [Google Scholar]
- Lucas, Robert E., Jr. 1994. On the Welfare Cost of Inflation. Center for Economic Policy Research, Stanford University. February. Available online: https://books.google.fr/books/about/On_the_welfare_cost_of_inflation.html?id=xSrYzwEACAAJ&redir_esc=y (accessed on 6 February 2023).
- Lucas, Robert E., Jr. 2000. Inflation and Welfare. Econometrica 68: 247–74. [Google Scholar] [CrossRef]
- Marty, Alvin L. 1999. The Welfare Cost of Inflation: A Critique of Bailey and Lucas. St. Louis: Federal Reserve Bank of St. Louis, pp. 41–46. [Google Scholar]
- McCallum, Bennett T. 2003. Monetary Policy in Economies with Little or No Money. Pacific Economic Review 9: 81–92. [Google Scholar] [CrossRef]
- Miyao, Ryuzo. 1996. Does a Cointegrating M2 Demand Relation Really Exist in the United States? Journal of Money, Credit, and Banking 28: 365–80. [Google Scholar] [CrossRef]
- Mulligan, Casey B., and Xavier X. Sala-i-Martin. 1996. Adoption of Financial Technologies: Implications for Money Demand and Monetary. NBER Working Paper 5504. Cambridge: National Bureau of Economic Research. [Google Scholar]
- Mulligan, Casey B., and Xavier X. Sala-i-Martin. 1997. The Optimum Quantity of Money: Theory and Evidence. Journal of Money, Credit and Banking 29: 687–715. [Google Scholar] [CrossRef]
- Nelson, Edward. 2008. Friedman and Taylor on Monetary Policy Rules: A Comparison. Federal Reserve Bank of St. Louis Review 90: 95–116. [Google Scholar] [CrossRef]
- Norman, Ben, Rachel Shaw, and George Speight. 2006. The History of Interbank Settlement Arrangements: Exploring Central Banks’ Role in the Payment System. London: Bank of England. [Google Scholar]
- Orlowski, Lucjan T. 2015. Monetary expansion and bank credit: A lack of spark. Journal of Policy Modeling 37: 510–20. [Google Scholar] [CrossRef]
- Orphanides, Athanasios. 2007. Taylor Rules. Washington: Board of Governors of the Federal Reserve System. [Google Scholar]
- Park, Albert. 2006. Risk and Household Grain Management in Developing Countries. The Economic Journal 116: 1088–15. [Google Scholar] [CrossRef]
- Poole, William. 2006. The Fed’s Monetary Policy Rule. Federal Reserve Bank of St. Louis Review 88: 1–11. [Google Scholar] [CrossRef]
- Reynard, Samuel. 2006. Money and the Great Disinflation. Swiss National Bank Working Paper 2006–2007. Bern and Zurich: Swiss National Bank. [Google Scholar]
- Ritter, Joseph A. 1995. The Transition from Barter to Fiat Money. The American Economic Review 85: 134–149. [Google Scholar]
- Sriram, Subramanian S. 1999. Survey of Literature on Demand for Money: Theoretical and Empirical Work with Special Reference to Error-Correction Models. IMF Working Papers. Washington: International Monetary Fund. [Google Scholar] [CrossRef]
- Stavins, Joanna. 1996. Can Demand Elasticities Explain Sticky Credit Card Rate? New England Economic Review (July/August). Boston: Federal Reserve Bank of Boston. [Google Scholar]
- Summers, Lawrence. 1991. Price Stability: How Should Long-Term Monetary Policy Be Determined? Journal of Money, Credit and Banking 23: 625–31. [Google Scholar] [CrossRef]
- Taylor, John B. 1993. Discretion versus Policy Rules in Practice. Carnegie-Rochester Conference Series on Public Policy 39: 195–214. [Google Scholar] [CrossRef]
- Taylor, John B. 1998. An Historical Analysis of Monetary Policy Rules. NBER Working Paper 6768. Chicago: University of Chicago Press. [Google Scholar]
- Tobin, James. 1956. The Interest Elasticity of Transactions Demand for Cash. Review of Economics and Statistics 38: 241–47. [Google Scholar] [CrossRef]
- Tobin, James. 1992. Money—For New Palgrave Money and Finance. Cowles Foundation Discussion Paper 1013. London: Palgrave Macmillan. [Google Scholar]
- Wicksell, Knut. 1936. Interest and Prices. Translation of 1898 edition by R. F. Kahn. London: Macmillan. [Google Scholar]
- Williams, John C. 2014. Monetary Policy at the Zero Lower Bound: Putting Theory into Practice. Washington: Hutchins Center on Fiscal & Monetary Policy at Brookings. [Google Scholar]
- Wolman, Alexander L. 1997. Zero Inflation and the Friedman Rule: A Welfare Comparison. Federal Reserve Bank of Richmond Economic Quarterly 83: 1–21. [Google Scholar]
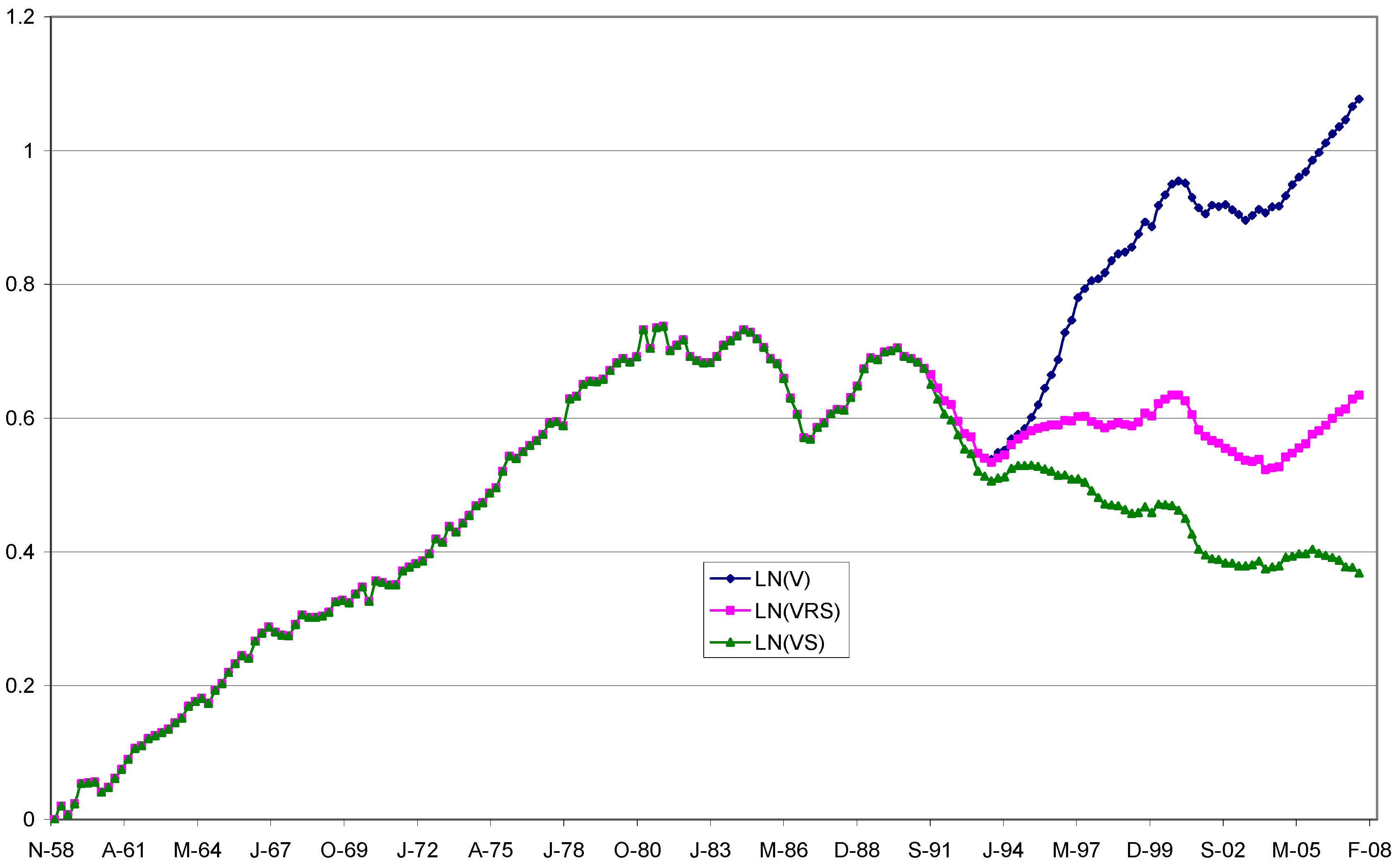
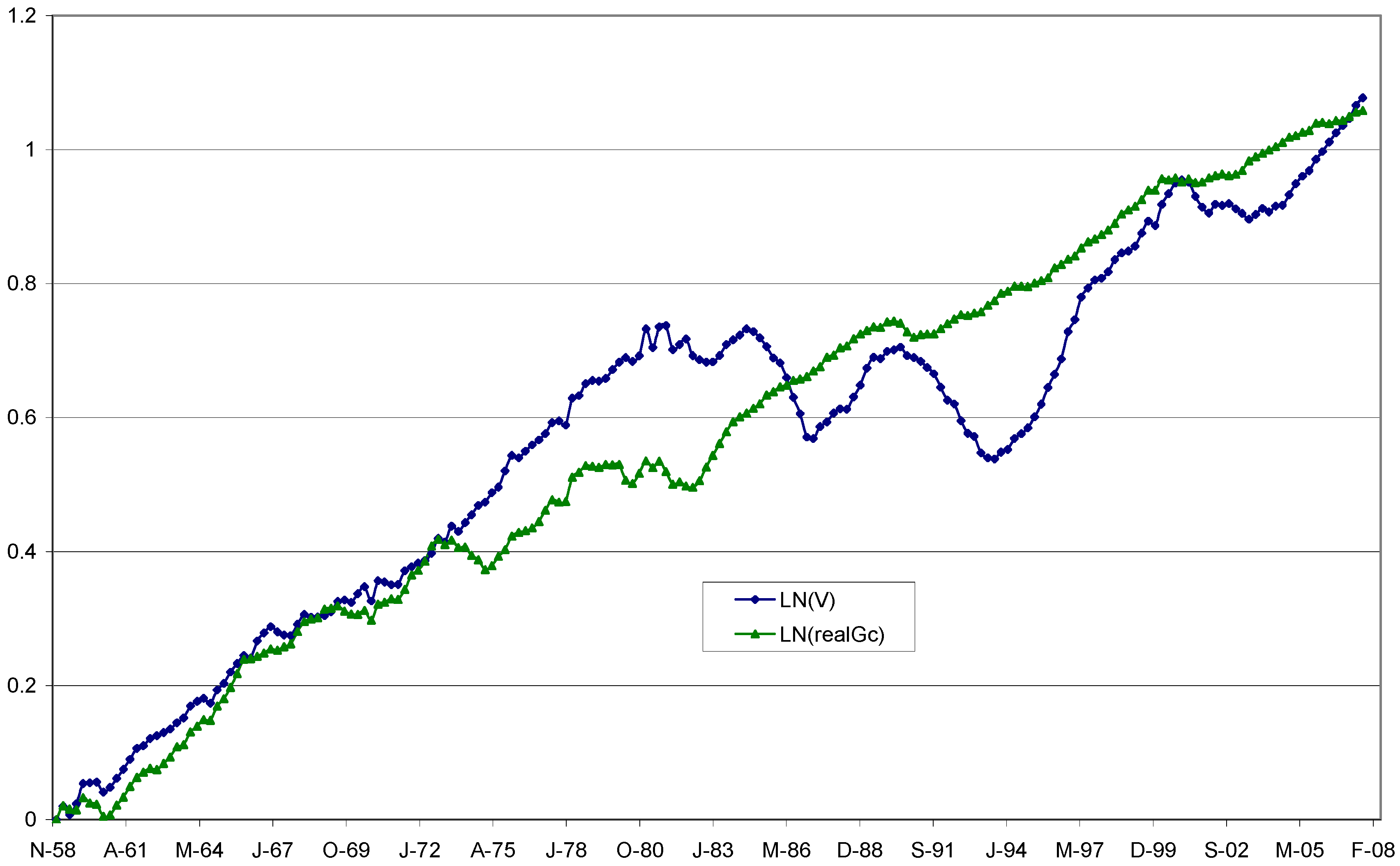
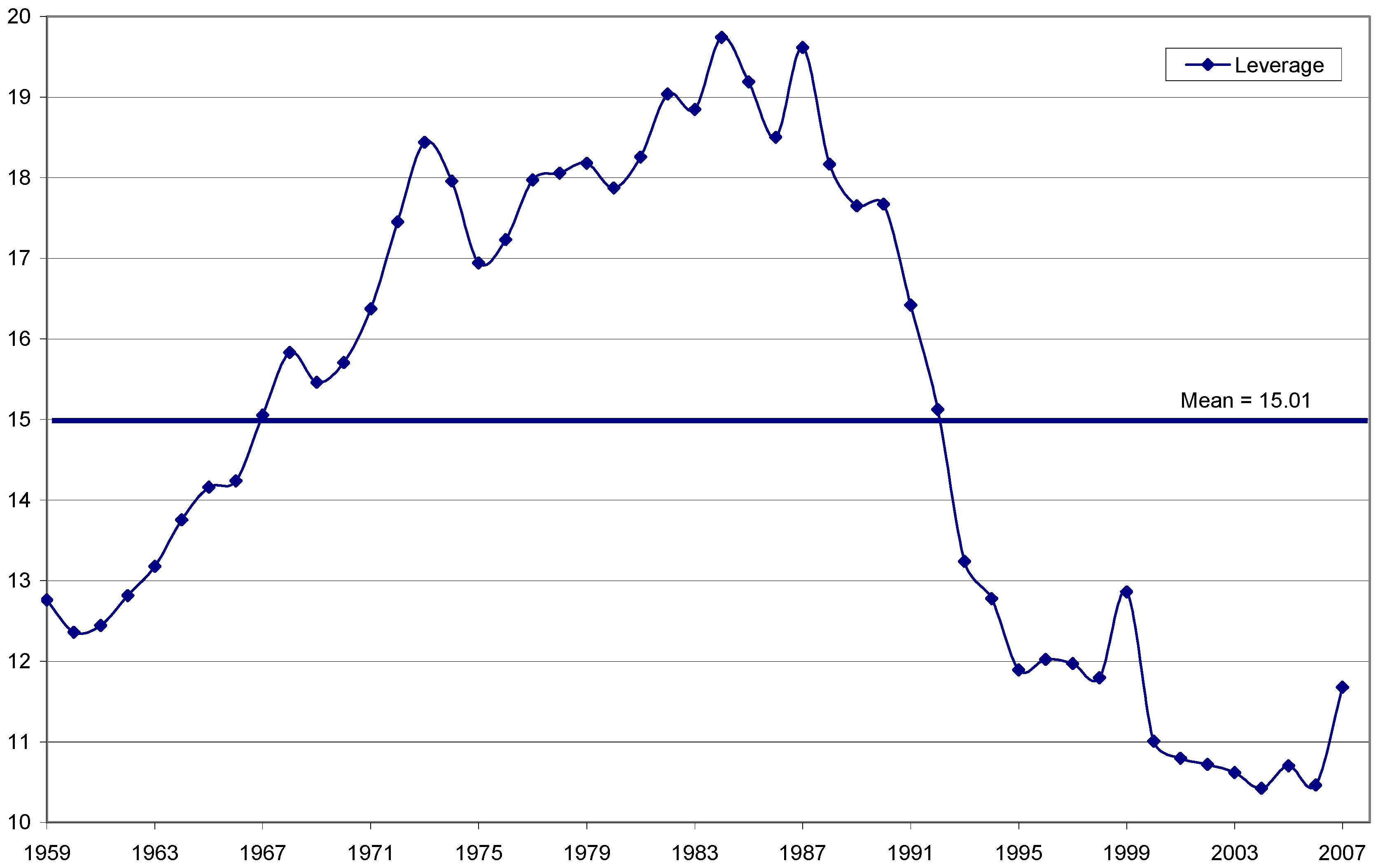
| Country | Date Adopted | Target | Target Variable |
|---|---|---|---|
| Australia | 1993 | Average of 2–3% over the medium term | Underlying PI up until October 1998 CPI thereafter |
| Canada | February 1991 | Midpoint 2% + 1% band | CPI |
| Finland | February 1993 | 2% no explicit band | CPI excluding indirect taxes, subsidies and housing-related costs |
| New Zealand | April 1988 | 0–3% | CPI excluding interest |
| Spain | November 1994 | 2% | CPI |
| Sweden | January 1993 | Midpoint 2% + 1% band | CPI |
| U.K. | October 1992 | 2.5% + 1% reporting range | Retail price index excluding mortgage interest payments |
| U.S. | January 2012 | 2% | CPI and/or PCE |
| Transaction Technology | Sample Period | Fed Chairmanship | Innovations Affecting Money Supply and Transaction Technology | Type of Progress |
|---|---|---|---|---|
| CM | 1959–71 | Mc Chesney-Martin 51–70 | Visa and American Express Cards (1958) | New Biased |
| First fully transistorized IBM 7090 mainframe computer (1959) | Neutral | |||
| GE’s ERMA Computer to Process Checks (1959) | New Biased | |||
| General purpose credit cards (BofA) (1966) | New Biased | |||
| ATMs (1967) | New Biased | |||
| Information Management System (IBM, 1968) | Neutral | |||
| Magnetic Swipe Cards (1969) | New Biased | |||
| Microprocessor (1970) | Neutral | |||
| Heap Leach Technology for Gold Mining (1970) | Old Biased | |||
| Clearing House Interbank Payment System (CHIPS, 1970) | New Biased | |||
| FM | 1972–82 | Automated Clearing House (ACH, 1972) | New Biased | |
| First PC Altair (1975) | Neutral | |||
| Burns 70–78 | Telephone Banking (1979) | New Biased | ||
| Miller 78–79 | Software Standardization of Fedwire System (1980) | Neutral | ||
| Volcker 79–87 | Activated Carbon Processes in Gold Mining (1980) | Old biased | ||
| NOW Accounts (1981), Super NOWs and MMDAs (1982) | --- | |||
| Commodore 64 (1982) | Neutral | |||
| Lotus 123 (1982) | Old Biased | |||
| 1983–93 | Volcker 79–87 Greenspan 87–06 | Graphic User Interface (1983) | Neutral | |
| Windows 1.0 (1985) | Neutral | |||
| 386 chip (1985) | Neutral | |||
| PC-Based Banking Clearinghouse Item Processing System (1986) | Neutral | |||
| World Wide Web (1989) | Neutral | |||
| Consolidation of Mainframe Computers at Fedwire (1990) | New Biased | |||
| Pentium Chip (1993) | Neutral | |||
| 1994–07 | Web Browser Mosaic and Netscape (1994) | Neutral | ||
| Greenspan 87–06 | “All Electronic” Banking Clearinghouse ACH (1994) | Neutral | ||
| Windows 95 (1995) | Neutral | |||
| Bernanke 06–14 | Online Banking (1995) | New Biased | ||
| Completion of High-Speed Network FEDNET (1996) | Neutral | |||
| TBD | Beyond 2007 | Bernanke 06–14 Yellen 14–18 Powell 18– | Digital Money | New Biased |
| E-barter | Old Biased |
| The “endogeneized” variable is the Log of money velocity (M1, M1RS or M1S), which following Johansen’s method (1995) has a normalized coefficient of 1. All samples start on January 1959 and end October 2007. All trace statistics for the rank of the cointegrating equations reject that the rank is greater than 1 at the 95% level and thus point to a unique cointegrating relation in each row. The Chi2 statistics shows that all parameters in the equations are together significant at least at the 99% level. Unless noted, each coefficient in all the cointegrating equations is significant at least at the 99% level. The High and Low columns represent the 95% confidence interval around the estimate of the coefficient for Ln(realGDPc). The AIC, BIC and HQ criteria in the righmost columns indicate the goodness of fit for the VECM. The bottom of the table shows results when dummy variables are excluded. | ||||||||||||||||
| Lag | Optimal Lag Criterion | Ln Velocity | Trace | RS | RL | Ln Real GDPc | d5971 | SM1 | Const | Low | High | Ln Lklhood | Chi2 | AIC | BIC | HQ |
| 1 | SBIC | M1 | 22.4 | 16.10 | −15.50 | 0.95 | −0.14 | --- | −1.03 | 0.76 | 1.13 | 2998 | 448 | −30.6 | −30.5 | −30.4 |
| 2 | HQIC | M1 | 21 | 14.91 | −15.09 | 0.85 | −0.21 | --- | −0.94 | 0.64 | 1.07 | 3030 | 265 | −30.8 | −30.6 | −30.2 |
| 4 | LR, FPE, AIC | M1 | 30.2 | 15.42 | −16.92 | 0.81 | −0.3 | --- | −0.54 | 0.61 | 1.00 | 3049 | 326 | −30.8 | −30.2 | −29.3 |
| 1 | HQIC, SBIC | M1 ♣ | 21.3 | 15.21 | −15.01 | 0.93 | −0.14 | --- | −0.92 | 0.75 | 1.11 | 3001 | 425 | −30.6 | −30.4 | −30.3 |
| 4 | LR, FPE, AIC | M1 ♣ | 27.8 | 14.05 | −15.8 | 0.80 | −0.29 | --- | −0.48 | 0.62 | 0.99 | 3053 | 349 | −30.8 | −30.2 | −29.2 |
| 2 | HQIC | M1RS | 57.9 | 7.37 | −5.06 | 0.58 | −0.15 | −0.29 | −0.22 | 0.42 | 0.74 | 3810 | 563 | −38.7 | −38.4 | −37.8 |
| 4 | LR, FPE, AIC | M1RS | 54.2 | 12.64 | −13.19 | 0.84 | −0.23 | −0.69 | −0.79 | 0.58 | 1.11 | 3858 | 267 | −38.9 | −38 | −36.8 |
| 2 | HQIC | M1RS ♣ | 62.9 | 7.00 | −4.89 | 0.59 | −0.15 | −0.3 | −0.23 | 0.42 | 0.75 | 3814 | 485 | −38.7 | −38.3 | −37.7 |
| 4 | LR, FPE, AIC | M1RS ♣ | 60.7 | 11.43 | −12.82 | 0.91 | −0.22 | −0.78 | −0.98 | 0.64 | 1.19 | 3864 | 244 | −38.9 | −38 | −36.7 |
| 2 | HQIC, SBIC | M1S | 56.1 | 7.93 | −5.40 | 0.64 | −0.13 | −0.35 | −0.46 | 0.47 | 0.81 | 3765 | 553 | −38.3 | −37.9 | −37.4 |
| 3 | FPE, AIC | M1S | 53.6 | 8.29 | −6.42 | 0.6 | −0.18 | −0.39 | −0.25 | 0.42 | 0.78 | 3794 | 497 | −38.4 | −37.8 | −36.9 |
| 4 | LR | M1S | 55.2 | 13.70 | −13.9 | 0.89 | −0.21 | −0.58 | −0.99 | 0.61 | 1.16 | 3808 | 275.4 | −38.4 | −37.5 | −36.2 |
| 2 | HQIC | M1S ♣ | 60.4 | 7.62 | −4.92 | 0.62 | −0.13 | −0.33 | −0.39 | 0.45 | 0.78 | 3769 | 470 | −38.2 | −37.8 | −37.2 |
| 4 | LR, FPE, AIC | M1S ♣ | 59.8 | 13.00 | −13.41 | 0.88 | −0.21 | −0.58 | −0.92 | 0.61 | 1.15 | 3812 | 239 | −38.3 | −37.4 | −36.1 |
| 1 | SBIC | M1 | 12.7 | 17.18 | −15.47 | 1.12 | --- | --- | −1.72 | 0.99 | 1.24 | 2757 | 200.7 | −28.2 | −28.1 | −28 |
| 2 | HQIC | M1 | 11.9 | 16.42 | −14.94 | 1.10 | --- | --- | −1.8 | 0.94 | 1.25 | 2783 | 201 | −28.4 | −28.2 | −28 |
| 4 | LR, FPE, AIC | M1 | 20.1 | 19.41 | −18.21 | 1.20 | --- | --- | −2.23 | 1.01 | 1.38 | 2792 | 177 | −28.5 | −28 | −27.5 |
| 1 | SBIC | M1RS | 27.6 | 11.18 | −5.73 | 0.49 | --- | --- | −0.11 | 0.41 | 0.58 | 2776 | 335.1 | −28.4 | −28.3 | −28.2 |
| 2 | HQIC | M1RS | 25.5 | 22.33 | −17.28 | 0.57 | --- | --- | −0.33 | 0.32 | 0.83 | 2800 | 59.9 | −28.6 | −28.4 | −28.1 |
| 4 | LR, FPE, AIC | M1RS | 24.7 | −10.63 | 15.94 | 0.33 | --- | --- | 0.38 | 0.18 | 0.49 | 2816 | 110 | −28.7 | −28.3 | −27.7 |
| 4 | LR, FPE, AIC | M1S | 29.2 | −9.57 | 16.13 | 0.09 | --- | --- | 1.02 | −0.05 | 0.24 | 2812 | 114 | −28.7 | −28.3 | −27.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Faugere, C. Velocity of Money and Productivity Growth: Explaining the 2% Inflation Target in the U.S. (1959–2007). Int. J. Financial Stud. 2024, 12, 15. https://doi.org/10.3390/ijfs12010015
Faugere C. Velocity of Money and Productivity Growth: Explaining the 2% Inflation Target in the U.S. (1959–2007). International Journal of Financial Studies. 2024; 12(1):15. https://doi.org/10.3390/ijfs12010015
Chicago/Turabian StyleFaugere, Christophe. 2024. "Velocity of Money and Productivity Growth: Explaining the 2% Inflation Target in the U.S. (1959–2007)" International Journal of Financial Studies 12, no. 1: 15. https://doi.org/10.3390/ijfs12010015
APA StyleFaugere, C. (2024). Velocity of Money and Productivity Growth: Explaining the 2% Inflation Target in the U.S. (1959–2007). International Journal of Financial Studies, 12(1), 15. https://doi.org/10.3390/ijfs12010015






