Durable Consumption-Based Asset Pricing Model with Foreign Factors for the Korean Stock Market
Abstract
1. Introduction
2. Model
2.1. Households
2.2. Firms
2.3. The Two-Country Linear Factor Model
- : Non-durable consumption Epstein–Zin CAPM (1C EZ-ND),
- : Power utility CCAPM (1C Power),
- and : Two-country non-durable Epstein–Zin (2C EZ-ND),
- and : Two-country power utility (2C Power).
3. Data
3.1. Portfolio Data
3.2. Macroeconomic Data for Factors
3.3. Summary Statistics
4. Cross-Sectional Test of the Two-Country Durable Consumption Model
4.1. Estimation of the Two-Country Durable Consumption Model
4.2. Estimation Results
4.2.1. Estimation with 25 Fama–French Portfolios
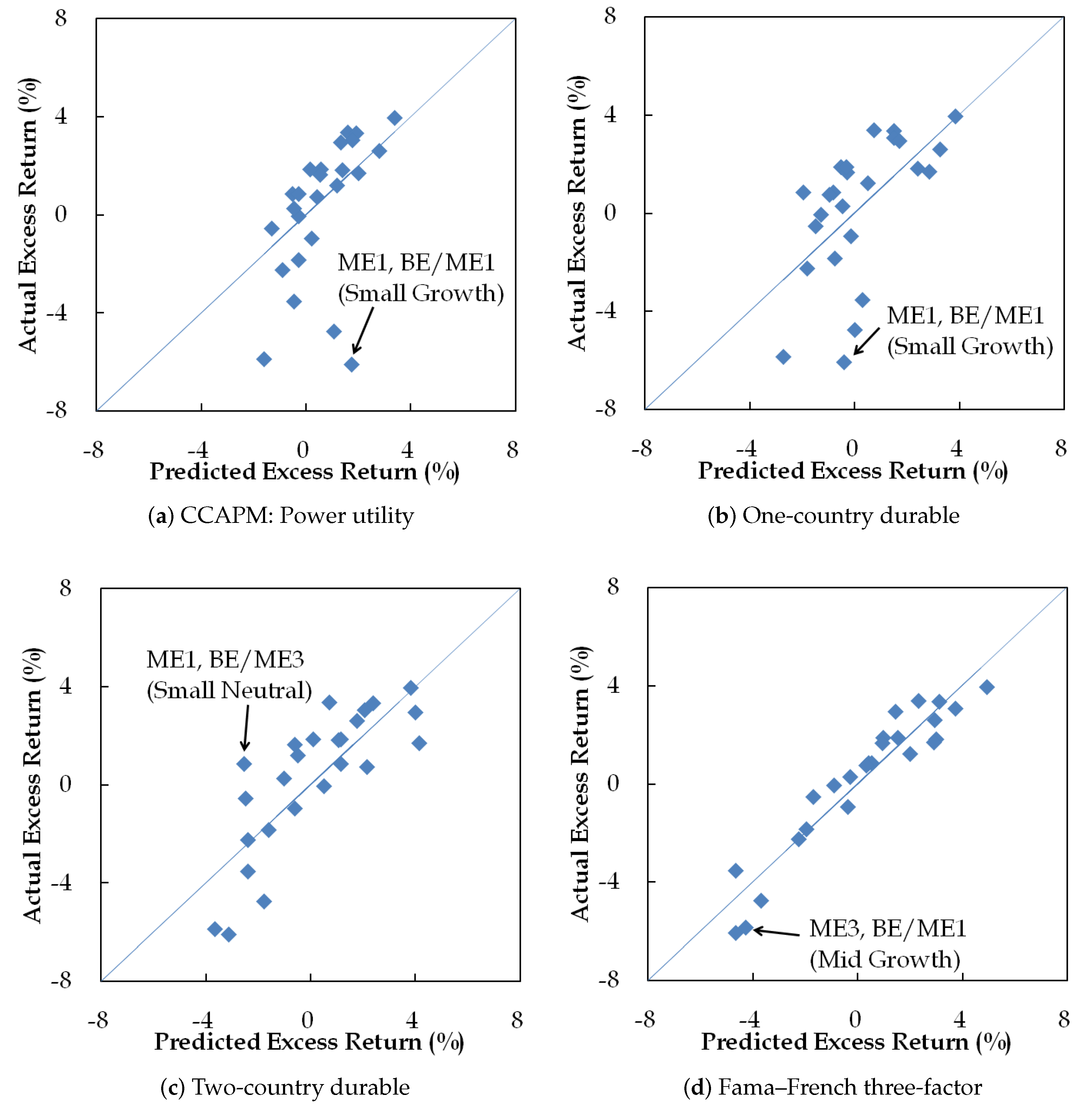
4.2.2. Estimation Results with Selected Portfolios
4.2.3. Equality Tests of Cross-Sectional s
5. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| Factors | One-Country | Two-Country | CAPM | FF | ||||
|---|---|---|---|---|---|---|---|---|
| Power | EZ-ND | EZ-D | Power | EZ-ND | EZ-D | |||
| 90.067 | 90.612 | 119.073 | 87.680 | 79.302 | 67.962 | |||
| (2.697) | (2.714) | (3.765) | (2.792) | (3.995) | (5.556) | |||
| −95.778 | −26.357 | |||||||
| (5.038) | (7.313) | |||||||
| −0.015 | 0.311 | 15.017 | 0.804 | |||||
| (0.764) | (0.850) | (0.890) | (1.461) | |||||
| 9.843 | 318.164 | −129.786 | ||||||
| (14.723) | (19.628) | (27.506) | ||||||
| 280.533 | ||||||||
| (16.725) | ||||||||
| −45.683 | −7.396 | |||||||
| (1.626) | (2.560) | |||||||
| 0.000 | 0.000 | 0.064 | ||||||
| (0.596) | (1.059) | (1.052) | ||||||
| 0.307 | 2.631 | |||||||
| (0.154) | (0.139) | |||||||
| 3.744 | ||||||||
| (0.185) | ||||||||
| 13.456 | ||||||||
| (0.230) | ||||||||
| 1.693 | 1.699 | 1.687 | 1.669 | 1.415 | 1.334 | 2.074 | 1.044 | |
| 0.176 | 0.176 | 0.291 | 0.176 | 0.452 | 0.571 | −0.018 | 0.706 | |
| J- | 2.000 | 1.997 | 1.970 | 2.000 | 1.991 | 1.958 | 2.000 | 2.002 |
| (1.000) | (1.000) | (1.000) | (1.000) | (1.000) | (1.000) | (1.000) | (1.000) | |
| (Wald Test) | : in the two-country durable consumption model | |||||||
| 1 | In China, stock markets are segmented based on investors’ nationalities. Foreigners participate only in the B-share market, whereas domestic investors trade shares mainly in the A-share market. |
| 2 | According to the Financial Investment Services and Capital Markets Act, foreigners include non-resident foreign nationals, branches of foreign corporations, and entities that were established by foreign laws. |
| 3 | Foreigners can hold unlimited stocks in all industries, except for utilities and public infrastructure. |
| 4 | We allowed for different preference parameters for the two countries. |
| 5 | , where is the nominal exchange rate, which is the price of foreign currency in terms of domestic currency. |
| 6 | Even if we use the arithmetic average, the econometric specification derived from a linear approximation does not change. |
| 7 | Let (S: set of states) be a state and be the price of an Arrow security for state s. Assuming two households and a complete market, we have for all s, where is the probability of s and is the SDF of the households in country i. Then, for all s. |
| 8 | Since , holds. |
| 9 | , where is the nominal exchange rate of the Korean won against the U.S. dollar at the end of each quarter and is the inflation rate in country j. |
| 10 | Bansal et al. (2008) showed that returns on human wealth are equivalent to the growth rate of labor income when human wealth is assumed to be proportional to labor income and we estimated human wealth based on their assumption. In addition, due to the large weight on human wealth, the returns of foreign stocks do not affect the results since investment in foreign stocks accounts for only a small fraction in both countries (about 10% and 20% of equity investment in Korea and the U.S., respectively, according to IMF CPIS). In our benchmark estimation, we did not consider stock returns from equity investment in the rest of the world. However, one can include global equity returns with a proxy such as the FT/S&P World Index, which covers 28 advanced and developing countries, excluding the U.S. and Korea. |
| 11 | Strictly speaking, Fama and French (1992) presented average returns rather than average excess returns. For average returns, our results do not change. |
| 12 | Typically, in Korea is negative, on average, whereas it is positive in the U.S. |
| 13 | The authors are grateful to the referee for this suggestion. We used eight non-financial and non-utility industries from FnGuide’s 10-industry portfolios, which were energy, materials, industrial goods and services, cyclical goods and services, essential consumer goods, healthcare, information technology, and communication services. |
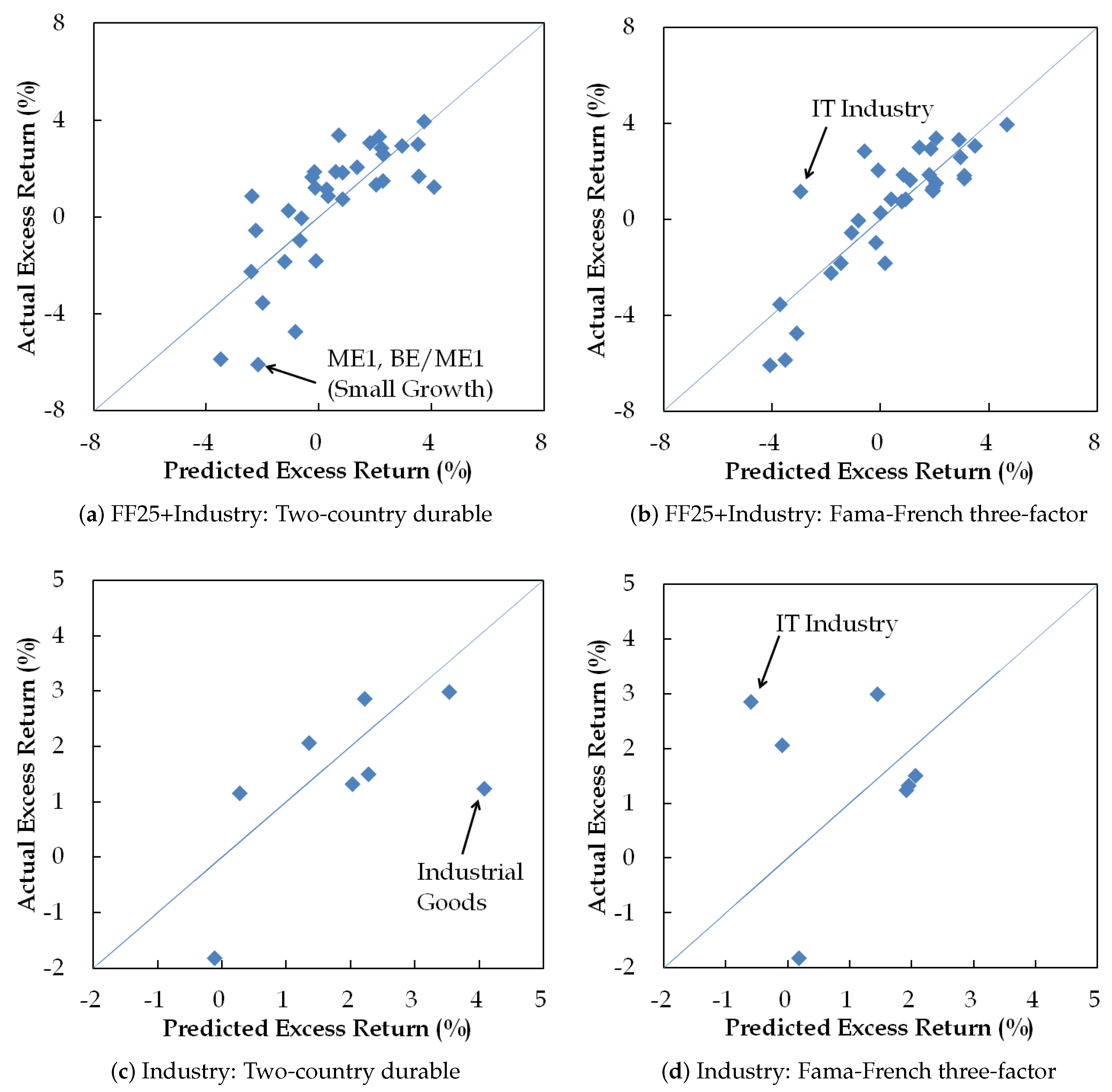
| 14 | Figure A1c,d show the fitted excess returns for only industry portfolios based on estimates with the 33 portfolios. |
| 15 | |
| 16 | Note that the two competing models are non-nested since these models have their own distinct sets of factors, meaning that the version of the KRS test for the non-nested case was sufficient for our subsequent analysis. To implement the method, we referred to the MATLAB code available at http://www-2.rotman.utoronto.ca/kan/research.htm (accessed on 20 January 2020). |
| 17 | The used in the KRS test was the standard coefficient of determination, which was computed with the total sum of squares and the explained sum of squares. |
| 18 | The KRS statistics are computed in two steps. In the first step, the factor loadings are estimated via a multivariate OLS regression. In the second step, the factor loadings are used as regressors in a cross-section GLS estimation. The KRS OLS statistic is obtained using the identity matrix as a weighting matrix. The KRS GLS is obtained when the inverse of the variance matrix of the asset returns is used as a weighting matrix. |
| 19 | See p. 2639 of Kan et al. (2013) for details. |
References
- Bae, Kee-Hong, Kalok Chan, and Angela Ng. 2004. Investibility and Return Volatility. Journal of Financial Economics 71: 239–63. [Google Scholar] [CrossRef]
- Bansal, Ravi, Thomas D. Tallarini, and Amir Yaron. 2008. The Return to Wealth, Asset Pricing and the Intertemporal Elasticity of Substitution. Society for Economic Dynamics 2008 Meeting Papers 918. Available online: https://econpapers.repec.org/paper/redsed008/918.htm (accessed on 20 January 2020).
- Bekaert, Geert, Campbell R. Harvey, and Christian Lundblad. 2005. Does financial liberalization spur growth? Journal of Finance 77: 3–55. [Google Scholar] [CrossRef]
- Brennan, Michael J., and H. Henry Cao. 1997. International Portfolio Investment Flows. Journal of Finance 52: 1851–80. [Google Scholar] [CrossRef]
- Brennan, Michael J., H. Henry Cao, Norman Strong, and Xinzhong Xu. 2005. The Dynamics of International Equity Market Expectations. Journal of Financial Economics 77: 257–88. [Google Scholar] [CrossRef]
- Campbell, John Y. 1996. Understanding Risk and Return. Journal of Political Economy 104: 298–345. [Google Scholar] [CrossRef]
- Campbell, John Y., and Tuomo Vuolteenaho. 2004. Bad Beta, Good Beta. American Economic Review 94: 1249–75. [Google Scholar] [CrossRef]
- Chan, Kalok, Albert J. Menkveld, and Zhishu Yang. 2008. Information Asymmetry and Asset Prices: Evidence from the China Foreign Share Discount. Journal of Finance 63: 159–96. [Google Scholar] [CrossRef]
- Chari, Anusha, and Peter B. Henry. 2004. Risk Sharing and Asset Prices: Evidence from a Natural Experiment. Journal of Finance 59: 1295–324. [Google Scholar] [CrossRef]
- Choe, Hyuk, Bong-Chan Kho, and Rene M. Stulz. 1999. Do Foreign Investors Destabilize Stock Markets? The Korean Experience in 1997. Journal of Financial Economics 54: 227–64. [Google Scholar] [CrossRef]
- Colacito, Riccardo, and Mariano M. Croce. 2011. Risks for the Long Run and the Real Exchange Rate. Journal of Political Economy 119: 153–81. [Google Scholar] [CrossRef]
- Constantinides, George M., and Darrell Duffie. 1996. Asset Pricing with Heterogeneous Consumers. Journal of Political Economy 104: 219–40. [Google Scholar] [CrossRef]
- Croce, Mariano M. 2014. Long-run productivity risk: A new hope for production-based asset pricing? Journal of Monetary Economics 66: 13–31. [Google Scholar] [CrossRef]
- Darrat, Ali F., Bin Li, and Jung Chul Park. 2011. Consumption-based CAPM models: International evidence. Journal of Banking & Finance 35: 579–601. [Google Scholar]
- Eyster, Erik, Matthew Rabin, and Dimitri Vayanos. 2019. Financial Markets Where Traders Neglect the Informational Content of Prices. Journal of Finance 74: 371–99. [Google Scholar] [CrossRef]
- Fama, Eugene F. 1991. Efficient Capital Markets: II. Journal of Finance 46: 1575–617. [Google Scholar] [CrossRef]
- Fama, Eugene F., and Kenneth R. French. 1992. The Cross-Section of Expected Stock Returns. Journal of Finance 47: 427–65. [Google Scholar] [CrossRef]
- Fama, Eugene F., and Kenneth R. French. 1993. Common Risk Factors in the Returns on Stocks and Bonds. Journal of Financial Economics 33: 3–56. [Google Scholar] [CrossRef]
- Fogli, Alessandra, and Fabrizio Perri. 2015. Macroeconomic Volatility and External Imbalances. Journal of Monetary Economics 69: 1–15. [Google Scholar] [CrossRef]
- Hansen, Lars Peter, and Ravi Jagannathan. 1997. Assessing specification errors in stochastic discount factor models. The Journal of Finance 52: 557–90. [Google Scholar] [CrossRef]
- Hodrick, Robert J., and Xiaoyan Zhang. 2001. Evaluating the Specification Errors of Asset Pricing Models. Journal of Financial Economics 62: 327–76. [Google Scholar] [CrossRef]
- Jeong, Daehee, Hwagyun Kim, and Joon Y. Park. 2015. Does Ambiguity Matter? Estimating Asset Pricing Models with a Multiple-Priors Recursive Utility. Journal of Financial Economics 115: 361–82. [Google Scholar] [CrossRef]
- Kaltenbrunner, Georg, and Lars Lochstoer. 2010. Long-run risk through consumption smoothing. Review of Financial Studies 23: 3141–89. [Google Scholar] [CrossRef]
- Kan, Raymond, Cesare Robotti, and Jay Shaken. 2013. Pricing model performance and the two-Pass cross-sectional regression methodology. Journal of Finance 68: 2617–49. [Google Scholar] [CrossRef]
- Li, Yuming, and Maosen Zhong. 2005. Consumption Habit and International Stock Returns. Journal of Banking & Finance 29: 579–601. [Google Scholar]
- Lustig, Hanno, and Stijn Van Nieuwerburgh. 2008. The Returns on Human Capital: Good News on Wall Street Is Bad News on Main Street. The Review of Financial Studies 21: 2097–137. [Google Scholar] [CrossRef]
- Sarkissian, Sergei. 2003. Incomplete Consumption Risk Sharing and Currency Risk Premiums. Review of Financial Studies 16: 983–1005. [Google Scholar] [CrossRef]
- Stiglitz, Joseph E. 2004. Capital Market Liberalization, Globalization, and the IMF. Oxford Review of Economic Policy 20: 57–71. [Google Scholar] [CrossRef]
- Umutlu, Mehmet, Levent Akdeniz, and Aslihan Altay-Salih. 2010. The Degree of Financial Liberalization and Aggregated Stock-Return Volatility in Emerging Markets. Journal of Banking & Finance 34: 509–21. [Google Scholar]
- Yogo, Motohiro. 2006. A Consumption-Based Explanation of Expected Stock Returns. Journal of Finance 61: 539–80. [Google Scholar] [CrossRef]
- Yun, Sang Yong, Bonil Ku, Young Ho Eom, and Jaehoon Hahn. 2009. The Cross-Section of Stock Returns in Korea: An Empirical Investigation. Asian Review of Financial Research 22: 1–44. [Google Scholar]

| ME | BE/ME | Average | ||||
|---|---|---|---|---|---|---|
| 1 Low | 2 | 3 | 4 | 5 High | ||
| 1 Small | −0.060 | 0.020 | 0.007 | 0.029 | 0.047 | 0.009 |
| 2 | −0.036 | −0.013 | −0.003 | 0.021 | 0.042 | 0.002 |
| 3 | −0.052 | −0.011 | 0.005 | 0.023 | 0.037 | 0.001 |
| 4 | −0.028 | −0.002 | 0.015 | 0.034 | 0.043 | 0.012 |
| 5 Big | 0.013 | 0.024 | 0.024 | 0.035 | 0.022 | 0.024 |
| Average | −0.033 | 0.004 | 0.010 | 0.028 | 0.038 | |
| Mean (%) | 0.175 | 1.052 | 1.119 | 0.219 | 1.042 | 0.478 | 1.186 | 6.981 | −1.825 |
| SD (%) | 1.490 | 0.865 | 0.346 | 0.517 | 3.659 | 2.910 | 11.728 | 7.906 | 11.742 |
| Correlations | |||||||||
| −0.030 | |||||||||
| 0.153 | 0.115 | ||||||||
| 0.207 | −0.045 | 0.259 | |||||||
| 0.170 | −0.009 | 0.232 | 0.660 | ||||||
| 0.207 | 0.082 | 0.621 | 0.402 | 0.077 | |||||
| 0.284 | 0.040 | 0.973 | 0.063 | 0.093 | 0.618 | ||||
| 0.153 | −0.040 | −0.133 | 0.009 | 0.209 | −0.157 | −0.164 | |||
| −0.023 | 0.156 | −0.083 | −0.021 | −0.133 | −0.085 | −0.050 | −0.694 | ||
| Factors | One-Country | Two-Country | CAPM | FF | |||||
|---|---|---|---|---|---|---|---|---|---|
| Power | EZ-ND | EZ-D | Power | EZ-ND | EZ-D | ||||
| 106.804 | 125.375 | 145.411 | 129.554 | 98.903 | 19.493 | ||||
| (5.082) | (6.816) | (8.592) | (6.567) | (8.337) | (6.243) | ||||
| −81.879 | −23.068 | ||||||||
| (8.568) | (10.909) | ||||||||
| −1.255 | −0.745 | 18.980 | 0.617 | ||||||
| (1.117) | (1.210) | (1.888) | (2.203) | ||||||
| −70.982 | 286.791 | −17.295 | |||||||
| (22.096) | (28.719) | (46.552) | |||||||
| 408.203 | |||||||||
| (53.161) | |||||||||
| −53.160 | −13.366 | ||||||||
| (3.856) | (3.803) | ||||||||
| 0.000 | 0.051 | 0.276 | |||||||
| (1.155) | (1.765) | (1.868) | |||||||
| 0.117 | 2.731 | ||||||||
| (0.163) | (0.195) | ||||||||
| 4.946 | |||||||||
| (0.267) | |||||||||
| 16.049 | |||||||||
| (0.405) | |||||||||
| 1.647 | 1.745 | 1.829 | 1.755 | 1.572 | 1.405 | 2.279 | 0.778 | ||
| 0.242 | 0.273 | 0.345 | 0.269 | 0.450 | 0.633 | −0.005 | 0.899 | ||
| J- | 1.978 | 1.967 | 1.943 | 1.945 | 1.968 | 1.875 | 1.969 | 1.981 | |
| (1.000) | (1.000) | (1.000) | (1.000) | (1.000) | (1.000) | (1.000) | (1.000) | ||
| (Wald Test) | : in the two-country durable consumption model | ||||||||
| Factors | Panel A: BE/ME2–BE/ME5 | Panel B: BE/ME3–BE/ME5 | ||||
|---|---|---|---|---|---|---|
| EZ-D | EZ-D | FF | EZ-D | EZ-D | FF | |
| One-Country | Two-Country | One-Country | Two-Country | |||
| 116.062 | 74.203 | 93.718 | 49.942 | |||
| (8.127) | (10.180) | (7.871) | (9.963) | |||
| 39.499 | 58.473 | 50.975 | 78.394 | |||
| (7.894) | (15.853) | (13.321) | (17.205) | |||
| 0.9068 | 0.825 | 1.814 | 0.920 | |||
| (1.147) | (2.198) | (1.228) | (3.872) | |||
| 9.986 | 209.734 | |||||
| (30.907) | (47.478) | |||||
| 140.539 | 113.840 | |||||
| (24.157) | (35.942) | |||||
| −4.435 | −9.256 | |||||
| (4.186) | (6.410) | |||||
| 0.314 | 0.729 | |||||
| (2.178) | (3.259) | |||||
| 2.439 | 2.335 | |||||
| (0.203) | (0.257) | |||||
| 4.513 | 4.362 | |||||
| (0.304) | (0.436) | |||||
| 13.567 | 12.431 | |||||
| (0.417) | (0.555) | |||||
| 0.819 | 0.753 | 0.556 | 0.529 | 0.473 | 0.526 | |
| 0.694 | 0.747 | 0.845 | 0.696 | 0.779 | 0.714 | |
| J- | 1.949 | 1.846 | 1.971 | 1.843 | 1.567 | 1.827 |
| (1.000) | (1.000) | (1.000) | (1.000) | (0.992) | (1.000) | |
| (Wald Test) | ||||||
| BE/ME2–BE/ME5 | BE/ME3–BE/ME5 | |||||
| 25 Fama–French | BE/ME2 | BE/ME3 | 25 Fama–French | |
|---|---|---|---|---|
| –BE/ME5 | –BE/ME5 | +8 Industries | ||
| Panel A: OLS | ||||
| 0.800 | 0.872 | 0.938 | 0.738 | |
| (0.035) | (0.062) | (0.030) | (0.096) | |
| 0.900 | 0.901 | 0.910 | 0.718 | |
| (0.044) | (0.064) | (0.070) | (0.058) | |
| −0.100 | −0.029 | 0.028 | 0.020 | |
| p-value | [0.008] | [0.522] | [0.591] | [0.829] |
| Panel B: GLS | ||||
| 0.208 | 0.372 | 0.483 | 0.269 | |
| (0.068) | (0.133) | (0.093) | (0.080) | |
| 0.571 | 0.508 | 0.448 | 0.368 | |
| (0.157) | (0.154) | (0.147) | (0.062) | |
| −0.364 | −0.136 | 0.035 | −0.098 | |
| p-value | [0.187] | [0.261] | [0.878] | [0.187] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cho, C.-K.; Jang, B. Durable Consumption-Based Asset Pricing Model with Foreign Factors for the Korean Stock Market. Int. J. Financial Stud. 2023, 11, 62. https://doi.org/10.3390/ijfs11020062
Cho C-K, Jang B. Durable Consumption-Based Asset Pricing Model with Foreign Factors for the Korean Stock Market. International Journal of Financial Studies. 2023; 11(2):62. https://doi.org/10.3390/ijfs11020062
Chicago/Turabian StyleCho, Cheol-Keun, and Bosung Jang. 2023. "Durable Consumption-Based Asset Pricing Model with Foreign Factors for the Korean Stock Market" International Journal of Financial Studies 11, no. 2: 62. https://doi.org/10.3390/ijfs11020062
APA StyleCho, C.-K., & Jang, B. (2023). Durable Consumption-Based Asset Pricing Model with Foreign Factors for the Korean Stock Market. International Journal of Financial Studies, 11(2), 62. https://doi.org/10.3390/ijfs11020062





