Midcourse Iterative Guidance Method for the Impact Time and Angle Control of Two-Pulse Interceptors
Abstract
1. Introduction
2. Iterative Guidance Method
2.1. Motion Model
2.2. Optimization Model
2.3. Optimal Solution
2.4. The IGM in the Second Pulse Section
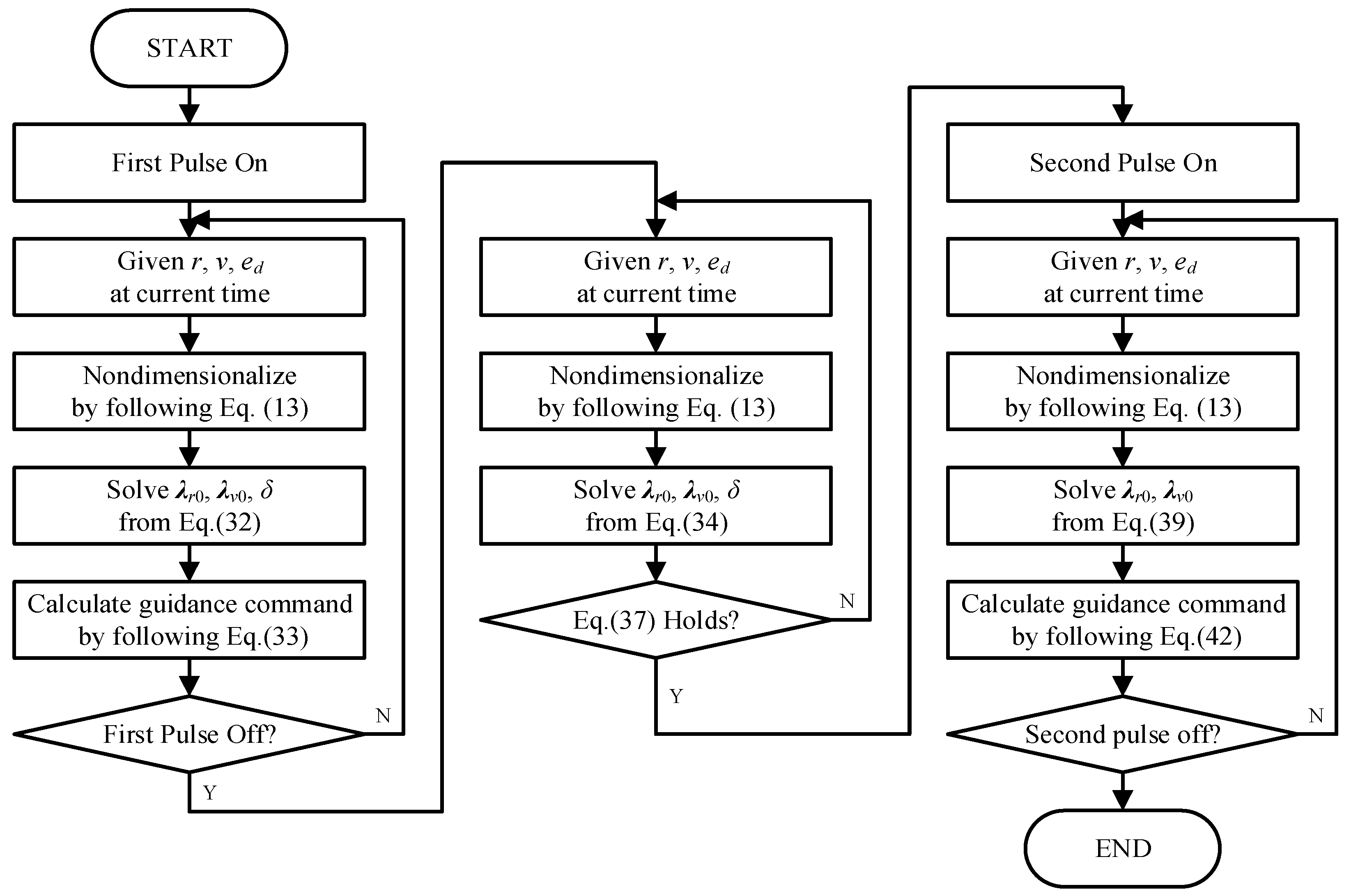
2.5. The Complete IGM Procedure
3. Simulation Results
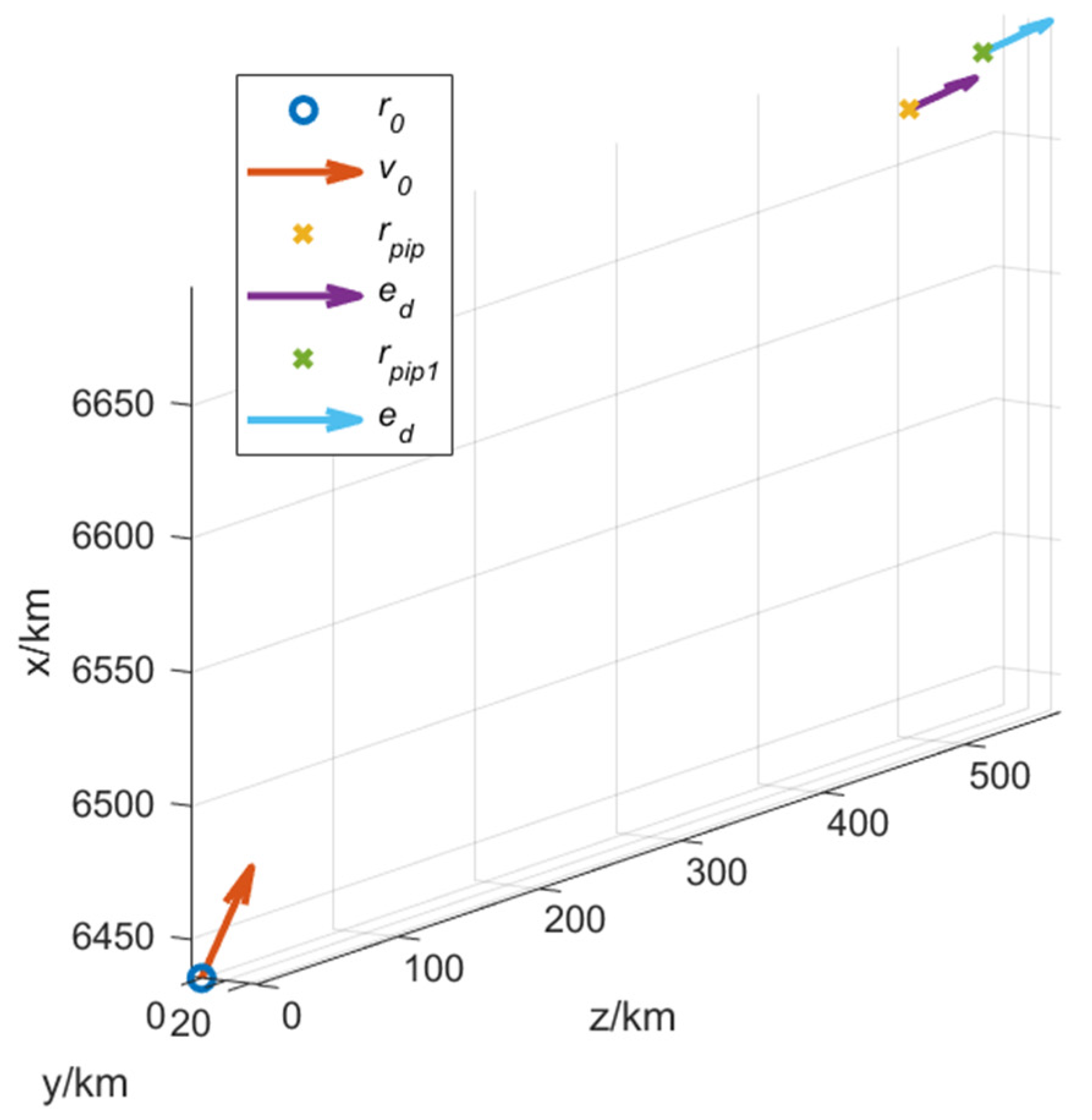
3.1. Simulation Conditions
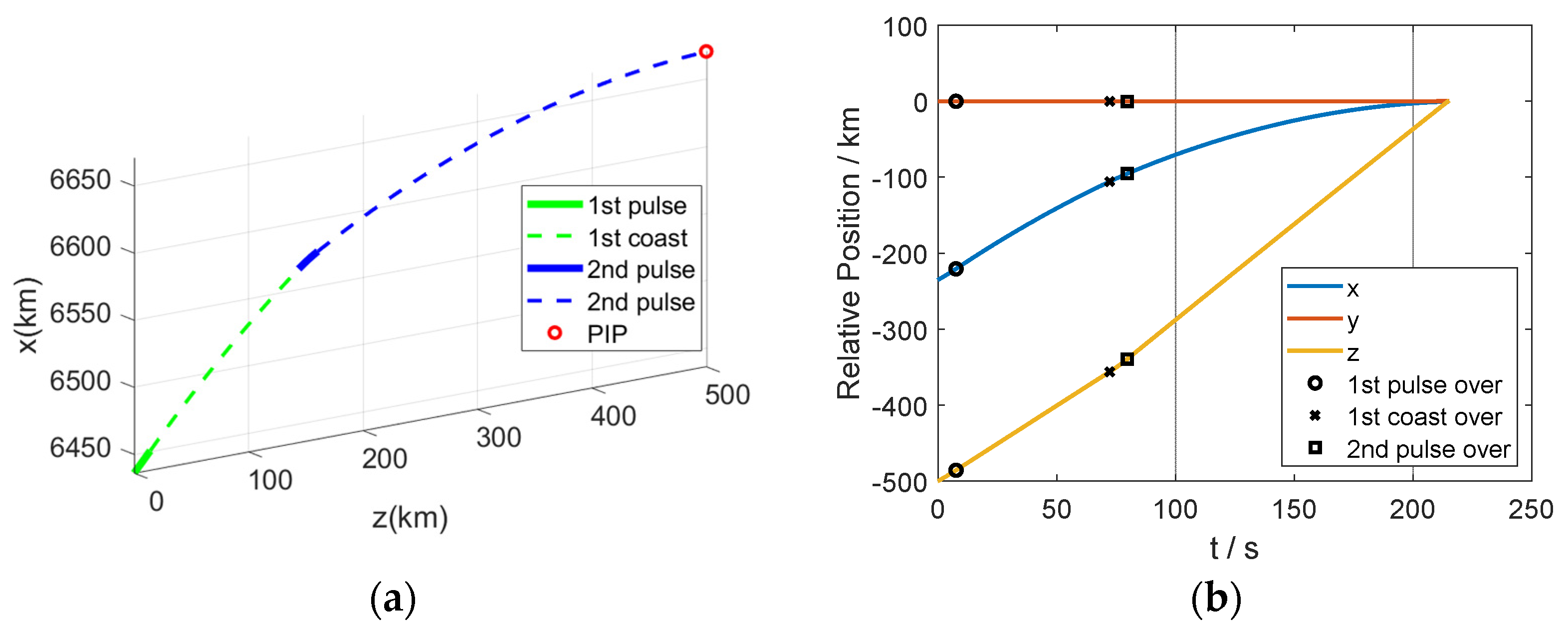
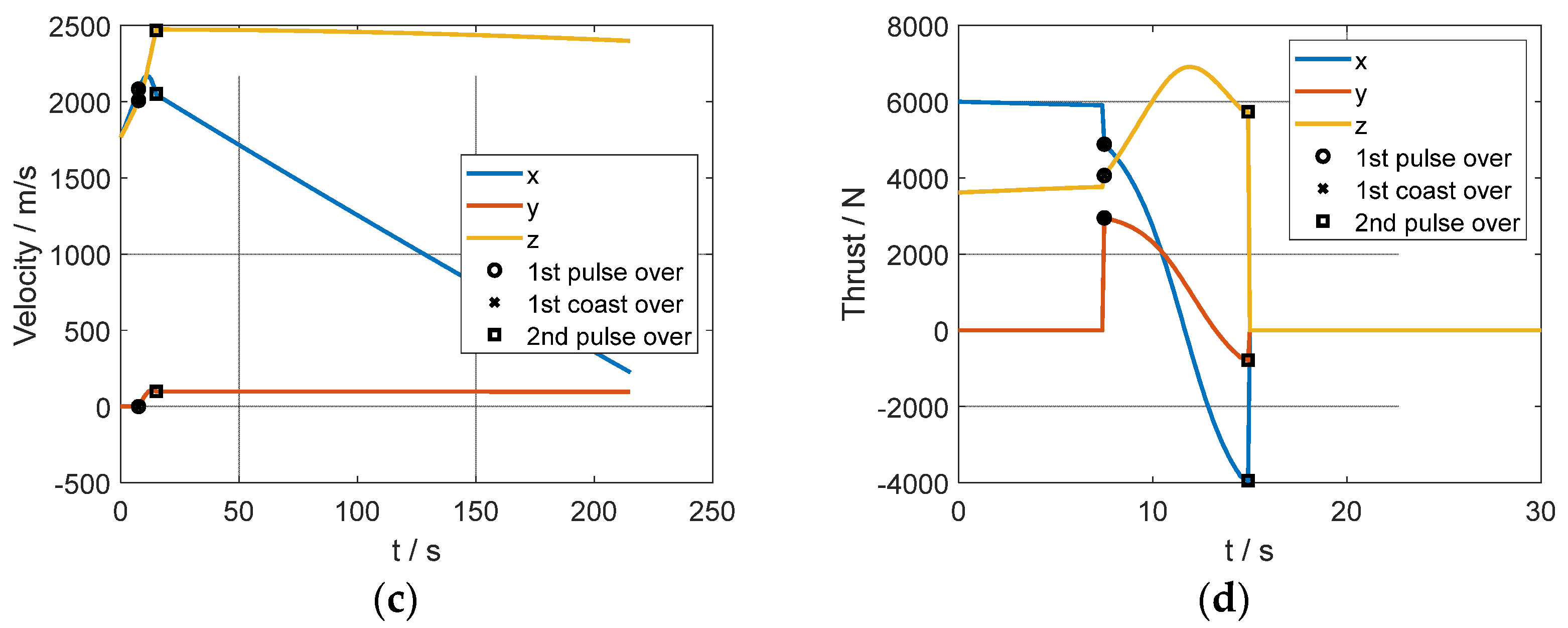
3.2. Scenario 1
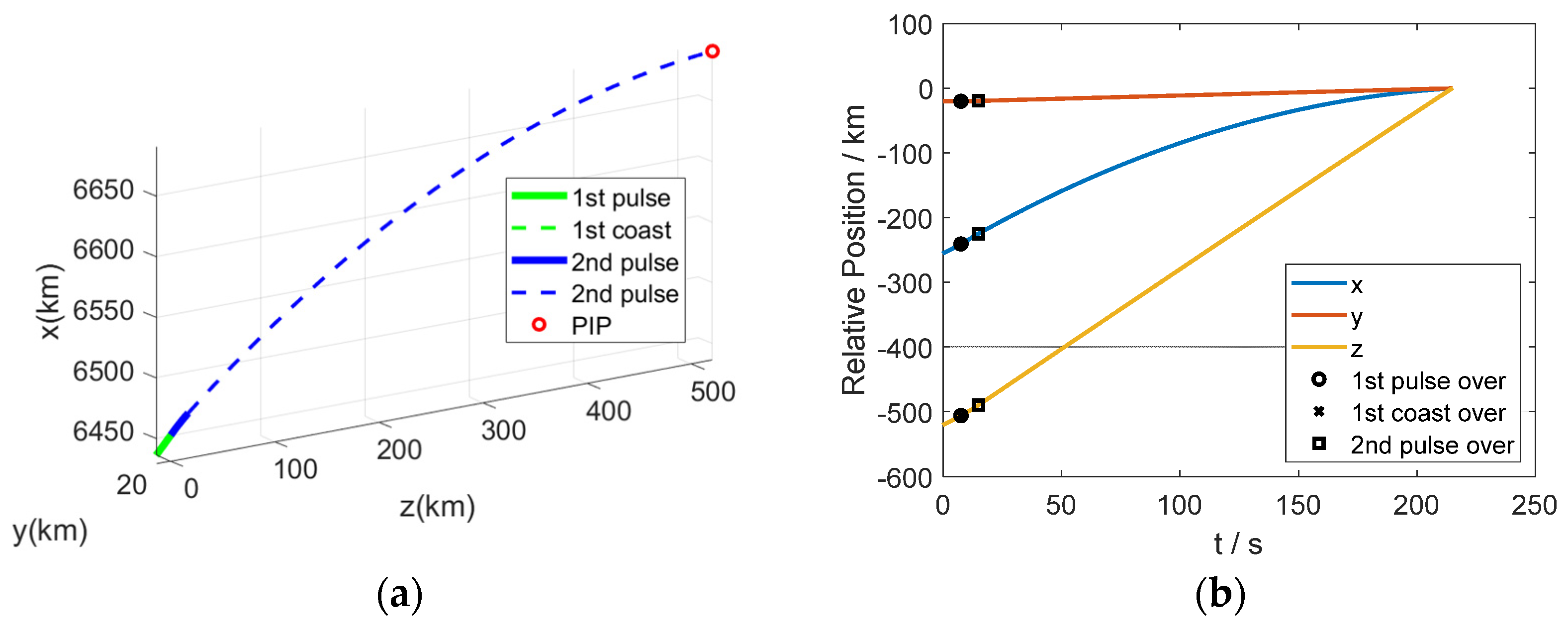
3.3. Scenario 2
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A
Appendix B
- I.
- General case (neither nor )
- II.
- Special case 1 ()
- III.
- Special case 2 ( and )
References
- Ghosh, S.; Ghose, D.; Raha, S. Composite guidance for impact angle control against higher speed targets. J. Guid. Control Dyn. 2016, 39, 98–117. [Google Scholar] [CrossRef]
- Li, B.; Tang, P.; Xu, H.; Zheng, D. Terminal Impact Angle Control Guidance Law Considering Target Observability. Aerospace 2022, 9, 193. [Google Scholar] [CrossRef]
- Shaferman, V.; Shima, T. Cooperative differential games guidance laws for imposing a relative intercept angle. J. Guid. Control Dyn. 2017, 40, 2465–2480. [Google Scholar] [CrossRef]
- Tekin, R.; Erer, K.S. Switched-gain guidance for impact angle control under physical constraints. J. Guid. Control Dyn. 2015, 38, 205–216. [Google Scholar] [CrossRef]
- Kumar, P.; Dwivedi, P.N.; Bhattacharyya, A.; Padhi, R. Terminal-lead-angle-constrained generalized explicit guidance. IEEE Trans. Aerosp. Electron. Syst. 2017, 53, 1250–1260. [Google Scholar] [CrossRef]
- Wang, X.; Wang, J. Partial integrated guidance and control for missiles with three-dimensional impact angle constraints. J. Guid. Control Dyn. 2014, 37, 644–657. [Google Scholar] [CrossRef]
- Zhao, S.; Chen, W.; Yang, L. Optimal Guidance Law with Impact-Angle Constraint and Acceleration Limit for Exo-Atmospheric Interception. Aerospace 2021, 8, 358. [Google Scholar] [CrossRef]
- Reisner, D.; Shima, T. Optimal guidance-to-collision law for an accelerating exoatmospheric interceptor missile. J. Guid. Control Dyn. 2013, 36, 1695–1708. [Google Scholar] [CrossRef]
- Ye, Q.; Liu, C.; Sun, J. A backstepping-based guidance law for an exoatmospheric missile with impact angle constraint. IEEE Trans. Aerosp. Electron. Syst. 2019, 55, 547–561. [Google Scholar] [CrossRef]
- Horn, H.J.; Chandler, D.C.; Buckele, V.L. Iterative guidance applied to generalized missions. J. Spacecr. Rockets. 1968, 6, 4–8. [Google Scholar] [CrossRef]
- Bryson, A.E.; Ho, Y.C. Applied Optimal Control Optimization Problem for Dynamic Systems with Path Constraints; Blaisdell Publishing Company: Waltham, MA, USA, 1975; Chapter 3. [Google Scholar]
- Chen, Z. Optimality Conditions Applied to Free-Time Multiburn Optimal Orbital Transfers. J. Guid. Control Dyn. 2016, 39, 2512–2521. [Google Scholar] [CrossRef][Green Version]

| Parameters | Symbol (Unit) | Value | |
|---|---|---|---|
| Initial | Position | r0/km | [Re + 65, 0, 0]T |
| Velocity | v0/m/s | 2500 × [1/√2, 1/√2, 0]T | |
| Mass | m/kg | 125 | |
| Pulse 1 | Thrust | T1/N | 7000 |
| Specific impulse | Isp1/m/s | 2800 | |
| duration | t1/s | 7.5 | |
| Pulse 2 | Thrust | T2/N | 7000 |
| Specific impulse | Isp2/m/s | 2800 | |
| duration | (t2 − t1)/s | 7.5 | |
| PIP | Time | tpip/s | 215 |
| Position | rpip/km | [Re + 300, 0, 500]T | |
| Desired direction | ed | [0, 0, 1]T | |
| PIP (correction) | Time | tpip/s | 215 |
| Position | rpip1/km | [Re + 320, 20, 520]T | |
| Desired direction | ed | [0, 0, 1]T | |
| Scenario | Duration of the First Coast/s | Position Error/m | Miss Distance/m | Impact Angle Error/deg |
|---|---|---|---|---|
| 1 | 62.4 | [0.2, 0, −0.4]T | 0.4 | 1.2 |
| 2 | 0 | [1.2, 0.8, 0.3]T | 1.5 | 5.9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, Y.; Ren, J.; Wang, X.; Cai, Y. Midcourse Iterative Guidance Method for the Impact Time and Angle Control of Two-Pulse Interceptors. Aerospace 2022, 9, 323. https://doi.org/10.3390/aerospace9060323
Deng Y, Ren J, Wang X, Cai Y. Midcourse Iterative Guidance Method for the Impact Time and Angle Control of Two-Pulse Interceptors. Aerospace. 2022; 9(6):323. https://doi.org/10.3390/aerospace9060323
Chicago/Turabian StyleDeng, Yifan, Jinlei Ren, Xu Wang, and Yuanli Cai. 2022. "Midcourse Iterative Guidance Method for the Impact Time and Angle Control of Two-Pulse Interceptors" Aerospace 9, no. 6: 323. https://doi.org/10.3390/aerospace9060323
APA StyleDeng, Y., Ren, J., Wang, X., & Cai, Y. (2022). Midcourse Iterative Guidance Method for the Impact Time and Angle Control of Two-Pulse Interceptors. Aerospace, 9(6), 323. https://doi.org/10.3390/aerospace9060323







