1. Introduction
The inlet buzz phenomenon was first observed by Oswatitsch as a pressure oscillation phenomenon that occurs when an inlet unstart occurs [
1]. The inlet buzz phenomenon reduces or blocks the flow rate into the inlet and can damage the internal structure due to severe pressure oscillation. Therefore, the prevention, prediction, and management of inlet unstart are important issues. To this end, it is essential to determine the inlet unstart flow pattern and unstart mechanism.
Ferri and Nucci conducted experimental studies on the causes and basic mechanisms of supersonic inlet buzz [
2]. As a result of the study, they found that the supersonic inlet buzz was an acoustic resonance phenomenon caused by the shear layer of the cowl lip. Likewise, Dailey conducted an experimental study to determine the occurrence mechanism of the supersonic inlet buzz phenomenon [
3]. According to Dailey’s experiment, another cause of the supersonic inlet buzz phenomenon was shock-induced flow separation on the external compression surface.
After that, a study on the supersonic inlet buzz was conducted by Fisher et al. and Trapier et al. [
4,
5,
6]. Fisher et al. conducted an experimental study on the supersonic inlet buzz phenomenon by changing the throttling ratio in Mach 2 flow [
4]. In their results, two pressure oscillation patterns with similar frequencies and different amplitudes were found, summarized as little buzz and big buzz. Furthermore, the little buzz was caused by the shear layer of the cowl lip (Ferri criterion), and the big buzz was caused by the separation bubble on the compression surface (Dailey criterion). Trapier et al. conducted experimental and numerical studies on the supersonic inlet buzz [
5,
6]. In the experimental results, pressure oscillation patterns corresponding to a little buzz and a big buzz were observed. Similar to Fisher et al., they confirmed that the cause of the little buzz was related to the Ferri criterion and that the big buzz was related to the Dailey criterion. In addition, the pressure oscillation of the little buzz was reduced by a bleeding device. In the numerical study, the experimental model was simulated using DDES (delayed detached-eddy simulation). The numerical results showed that all the features of the buzz phenomenon matched well with their experiments.
As described above, the flow patterns and mechanisms for supersonic inlet buzzes have been somewhat clarified by many studies. However, as Curran and Murthy pointed out, the unstart flow patterns of the supersonic inlet and the hypersonic inlet are different [
7]. Unlike the supersonic inlet, the hypersonic inlet has a supersonic area even after the final shock wave. Due to this difference, the causes of the unstart pattern of the hypersonic inlet vary, and the flow patterns are different for each cause [
8]. Therefore, it is difficult to directly apply the research achievements on supersonic inlet buzz to hypersonic inlet buzz.
As a result, many researchers have conducted studies to identify the unstart flow patterns and mechanisms of hypersonic inlets [
9,
10,
11,
12,
13,
14,
15]. Tan and Guo conducted an experimental study on the hypersonic inlet unstart phenomenon using a plug at the rear of the inlet model [
9]. Their experimental results showed changes in the external shock wave structure and inlet wall pressure. However, the internal flow patterns of the buzz cycle were not identified, and the conclusion was inferred based on the changes in the wall pressure distribution.
Tan et al. conducted an experimental study to identify the unstart flow pattern and buzz frequency of the hypersonic inlet [
10]. To simulate the back pressure, a plug was located at the rear of the inlet model. In the results, the pressure oscillation patterns were classified into little buzz and big buzz, but they were different from the little buzz and the big buzz of the supersonic inlet. Furthermore, they found that the oscillation mechanism of the supersonic inlet buzz based on the acoustic wave feedback loop was not suitable for the hypersonic inlet buzz. Therefore, flow spillage was considered a disturbing source, and a new oscillation mechanism for hypersonic inlet buzz was proposed based on a feedback loop suitable for the hypersonic inlet. Using this new feedback loop, the base frequency of the hypersonic inlet buzz was predicted, and it matched well with their experimental results.
However, in addition to little buzz and big buzz, there are other types of inlet unstart patterns in hypersonic inlets [
8]. Wagner conducted an experimental study on the hypersonic inlet model at Mach 5 flow [
11]. To simulate back pressure, a flap was installed at the rear of the scramjet inlet-isolator model. In the experimental results, three unstart flow patterns were observed. The three patterns were ‘high-amplitude oscillatory unstarted flow’, ‘low-amplitude oscillatory unstarted flow’, and ‘non-oscillatory unstarted flow’.
As such, there are several unstart flow patterns, and the flow characteristics of each pattern are different. With the efforts of many researchers, studies on the flow characteristics and mechanisms of hypersonic inlet unstart have progressed, but there are still components to be identified. Therefore, several follow-up studies have been conducted [
12,
13,
14].
Tan et al. conducted an experimental study on the hypersonic inlet unstart phenomenon in Mach 5 flow using a plug installed at the rear of the inlet model [
12]. In the results, the position of the pressure sensor suitable for monitoring the inlet status was presented by analyzing the Schlieren images and the pressure signals. In addition, an overall analysis of each flow pattern was presented based on the behavior of shock structures and separation bubbles, but a detailed analysis of the flow characteristics was not presented. Similarly, Li et al. conducted an experimental study on hypersonic inlet unstart using a plug-installed inlet model [
13]. In their results, the study focused on changes in pressure oscillation patterns and shock propagation speeds according to an increase in the throttling ratio rather than the mechanism of the buzz phenomenon. Koo and Raman conducted a numerical study on the inlet unstart phenomenon using LES (large-eddy simulation) by referring to Wagner’s experiment [
11,
14]. The flow characteristics of the inlet start case were in good agreement with the experimental results, but three types of pressure oscillation patterns of inlet unstart were not found in their results.
Although several follow-up studies have been conducted, explanations of the three different unstart modes found in Wagner’s experiment have rarely been reported. In the authors’ previous study, the numerical results matched well with the pressure oscillation frequency and amplitude of ‘high-amplitude oscillatory unstarted flow’ shown in Wagner’s experiment [
15]. In that paper, the flow characteristics and mechanisms of ‘high-amplitude oscillatory unstarted flow’ were explained. Furthermore, the effects of boundary layer types in ‘high-amplitude oscillatory unstarted flow’ were addressed. However, the flow characteristics and mechanisms for the other two inlet unstart modes, ‘non-oscillatory unstarted flow’ and ‘low-amplitude oscillatory unstarted flow’, are still unclear.
Therefore, in the present study, we perform numerical analysis to investigate the flow characteristics of ‘non-oscillatory unstarted flow’ as a follow-up study. The numerical method for the study and the configuration of the test model are introduced in
Section 2. In
Section 3.1, changes in pressure oscillation patterns according to various flap angles are explained. In
Section 3.2 and
Section 3.3, the detailed mechanisms of ‘non-oscillatory unstarted flow’ are explained. Finally, in
Section 3.4, we address the differences between ‘high-amplitude oscillatory unstarted flow’ and ‘non-oscillatory unstarted flow’.
2. Numerical Method
The open-source CFD (computational fluid dynamics) software OpenFOAM is used to model ‘non-oscillatory unstarted flow’ in the scramjet inlet-isolator model. In a previous study, we validated the overall numerical method through comparison with experimental results [
15]. Therefore, in this study, the same numerical method is used. The RANS (Reynolds-averaged Navier-Stokes) equations-based solver rhoCentralFoam, which is suitable for supersonic flow analysis, is employed to solve the Navier–Stokes equations [
16,
17]. The central-upwind KNP (Kurganov, Noelle, and Petrova) scheme with the van Leer limiter is applied to evaluate the flux at cell faces [
17,
18]. The boundary layer and recirculation zone are significant factors in the flow characteristics of inlet unstart. To consider these factors, Sutherland’s viscosity model is used as a viscous model, and k-ω SST (shear stress transport) is used as a turbulence model [
19,
20]. Temperature-dependent thermal properties are taken from the JANAF table [
21].
Figure 1 shows the schematic of the scramjet inlet-isolator model. As in a previous study, the configuration and inflow conditions of the scramjet inlet-isolator model are the same as the experimental conditions [
11,
15]. As shown in the figure, the inflow conditions are a Mach number of 4.9 with a total pressure of 2.5 MPa, and total temperature of 330 K. A 19.05 mm thick turbulent boundary layer is also considered in the inflow. In this case, the boundary layer thickness is 75% of the inlet height. Turbulence boundary layer theory is employed to calculate the velocity profiles of the inflow boundary layer [
22,
23].
To find the appropriate grid resolution for the problem, a grid independency test is performed.
Table 1 shows the number of grid cells used in the test. In the table, Grids 1 and 2 are used for the model with a folded flap and Grids 3 and 4 are used for the model with an opened flap. Since it is important to estimate the development of boundary layers and flow separation in unstarted flows, the average y+ on the wall is set to about 1.0 for all cases. The test results for Grids 1 and 2 are in consistent with each other, and so are those for Grids 3 and 4. Therefore, in the present study, Grid 4 is used for the model with an opened flap.
Figure 2 shows the computational domain with Grid 4 for the scramjet inlet-isolator model. The inflow boundary conditions are the same as those described in
Figure 1, and the outlet boundary conditions employ the supersonic outflow condition. The wall boundary conditions employ the no-slip adiabatic condition.
To simulate the back pressure, a flap is installed at the rear of the model. In the experimental paper, there was no information about the flap angle at the moment when ‘non-oscillatory unstarted flow’ appeared. Thus, to find the flap angle that shows ‘non-oscillatory unstarted flow’, case studies with changing flap angle are performed.
Figure 2 shows the grid system of the scramjet inlet-isolator model. As shown in the figure, dense grids are placed inside the inlet to simulate complex flow phenomena such as shock-boundary layer interactions. The total number of grid cells is approximately 42,000. For grid resolution analysis, we compare the numerical results doubling the number of grids, but there are no significant differences in the results. The inflow boundary conditions are the same as those described in
Figure 1, and the outlet boundary condition employed the supersonic outflow condition. The wall boundary conditions employ the no-slip adiabatic condition.
4. Conclusions
In this study, the characteristics and mechanisms of ‘non-oscillatory unstarted flow’ (NO mode) that appeared in Wagner’s experiment were investigated using numerical analysis. ‘High-amplitude oscillatory unstarted flow’ (HAO mode), which was another inlet unstart mode in the experiment, was compared with NO mode. Before the detailed analysis, a case study was performed to find the appropriate flap angle for NO mode appearing in the experiment. Because of the fair agreement with the experimental results, a flap angle of 18.5° was chosen for the representative case of NO mode.
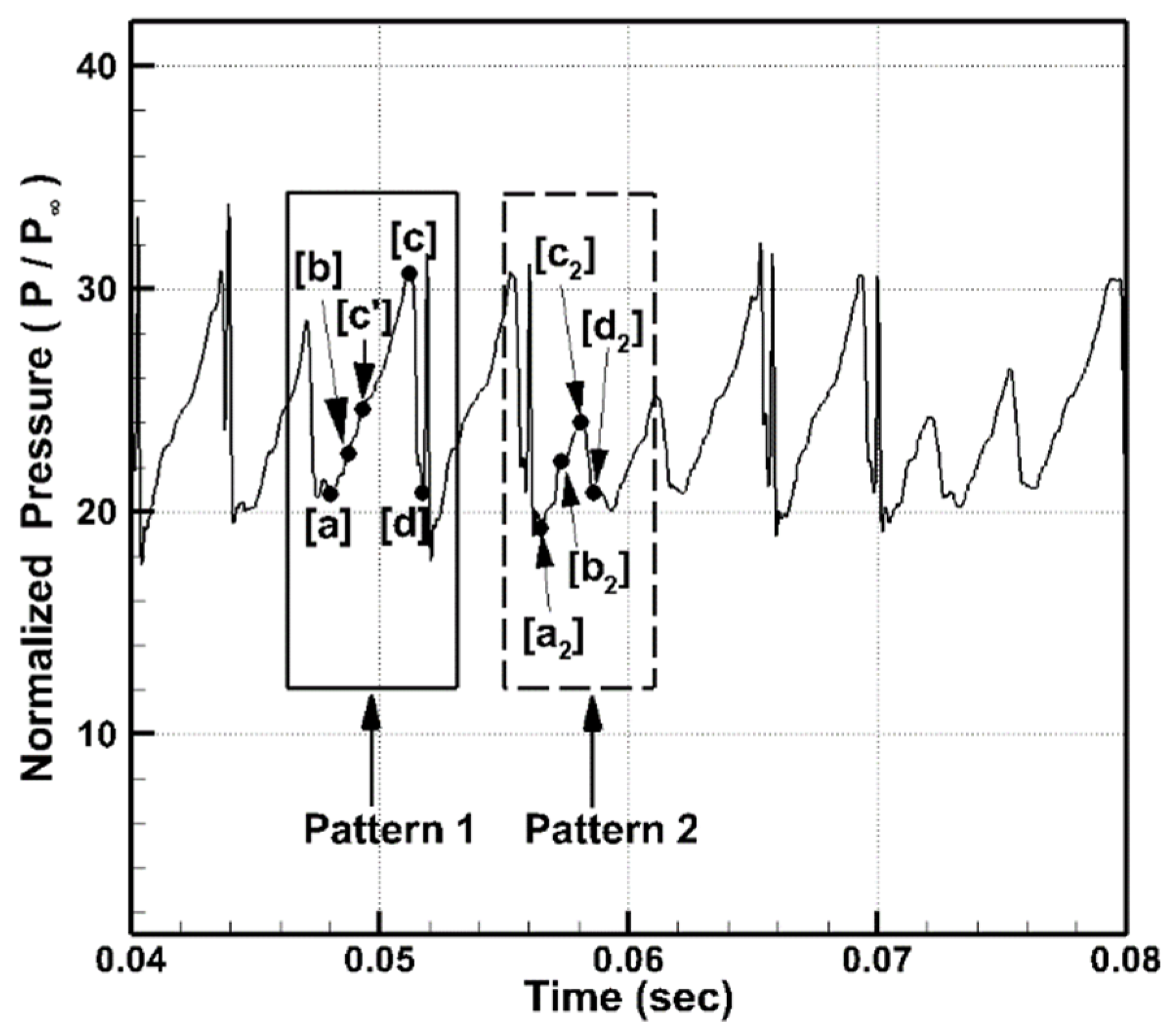
From the observation of the pressure oscillation patterns, there were two different oscillation patterns in NO mode. In Pattern 1, the normalized pressure () value oscillated between 20 and 30. However, in Pattern 2, the normalized peak pressure was less than 25. These two patterns appeared irregularly, so the oscillation was nonperiodic in NO mode.
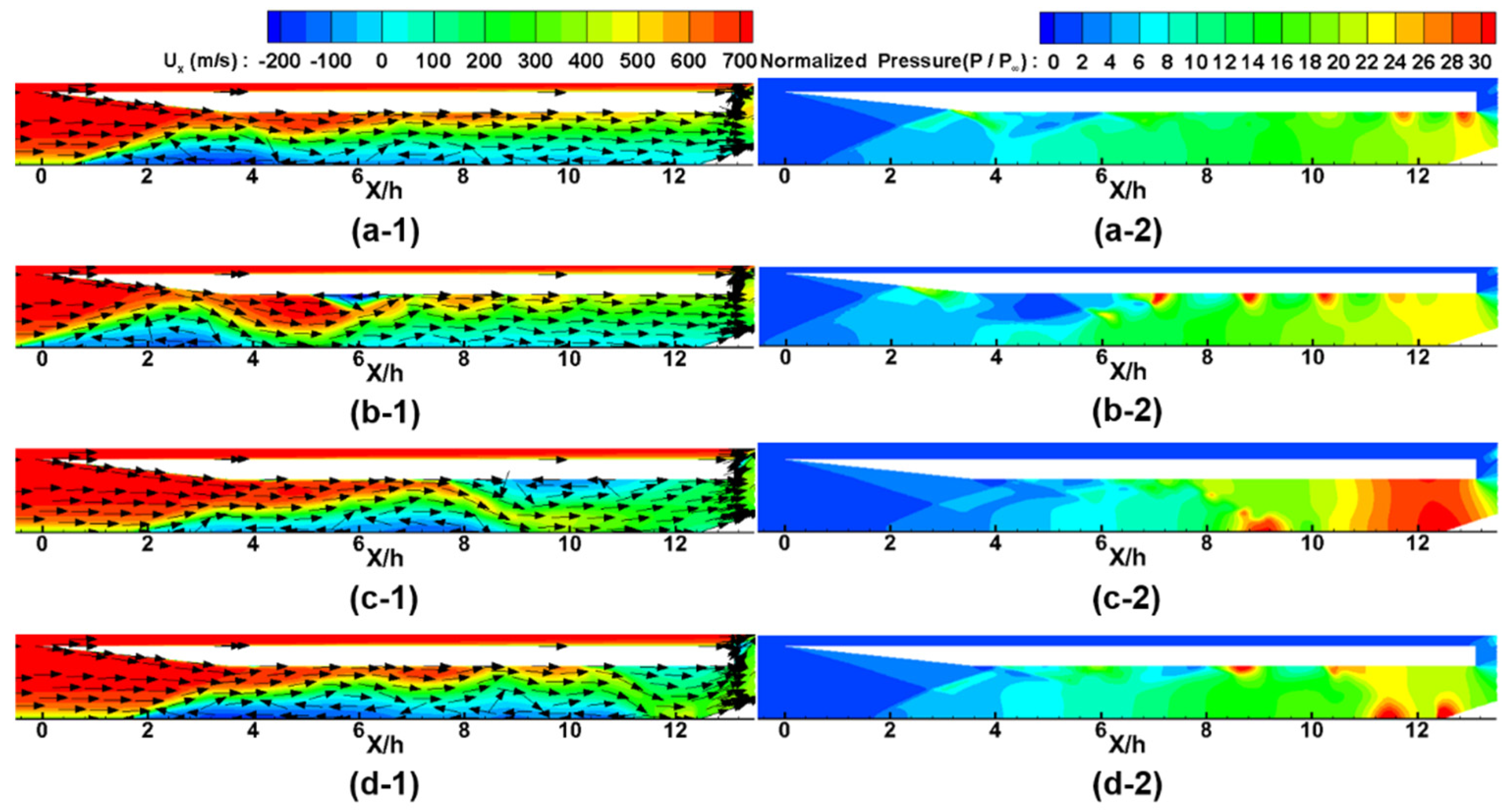
To understand the flow characteristics and mechanisms of NO mode, two pressure oscillation patterns were analyzed and compared. In the results, the basic pressure oscillation mechanisms were similar in both patterns. The back pressure increase with the inflow, the backflow due to the higher back pressure, and the back pressure decrease due to the backflow were the same in both patterns. However, the two patterns had different local maximum back pressures and different flow configurations. In the results of a more detailed analysis, a separation zone on the upper wall (UB) was generated by the third collision of the supersonic inflow into the wall in both patterns. However, slight differences in the inflow conditions from upstream may affect the state of the wall collisions, so UB could be generated or disappear irregularly. When UB was generated, it blocked the flow passage and resulted in a pressure rise. However, when UB disappeared, the back pressure was released due to flow passage expansion.
Additionally, the flow characteristics of HAO mode and NO mode were compared. In HAO mode, due to the high back pressure downstream, the backflow was very active and occupied the most flow passage. On the other hand, NO mode showed a relatively low peak pressure compared to that of HAO mode, and the incoming flow and the backflow coexisted in the flow passage. Therefore, the back pressure release was rather insufficient. The maximum back pressure differences between HAO mode and NO mode were due to the different exit areas of the model. The exit area of HAO mode was approximately half of the exit area of NO mode.