Numerical Comparison of Contact Force Models in the Discrete Element Method
Abstract
1. Introduction
2. Materials and Methods
2.1. Linear Spring–Dashpot Model
2.2. Mindlin–Deresiewicz Model (MD Model)
2.3. Hertzian Contact with the Non-Slip MD Model
2.4. Hertzian Contact with the Revised MD Model
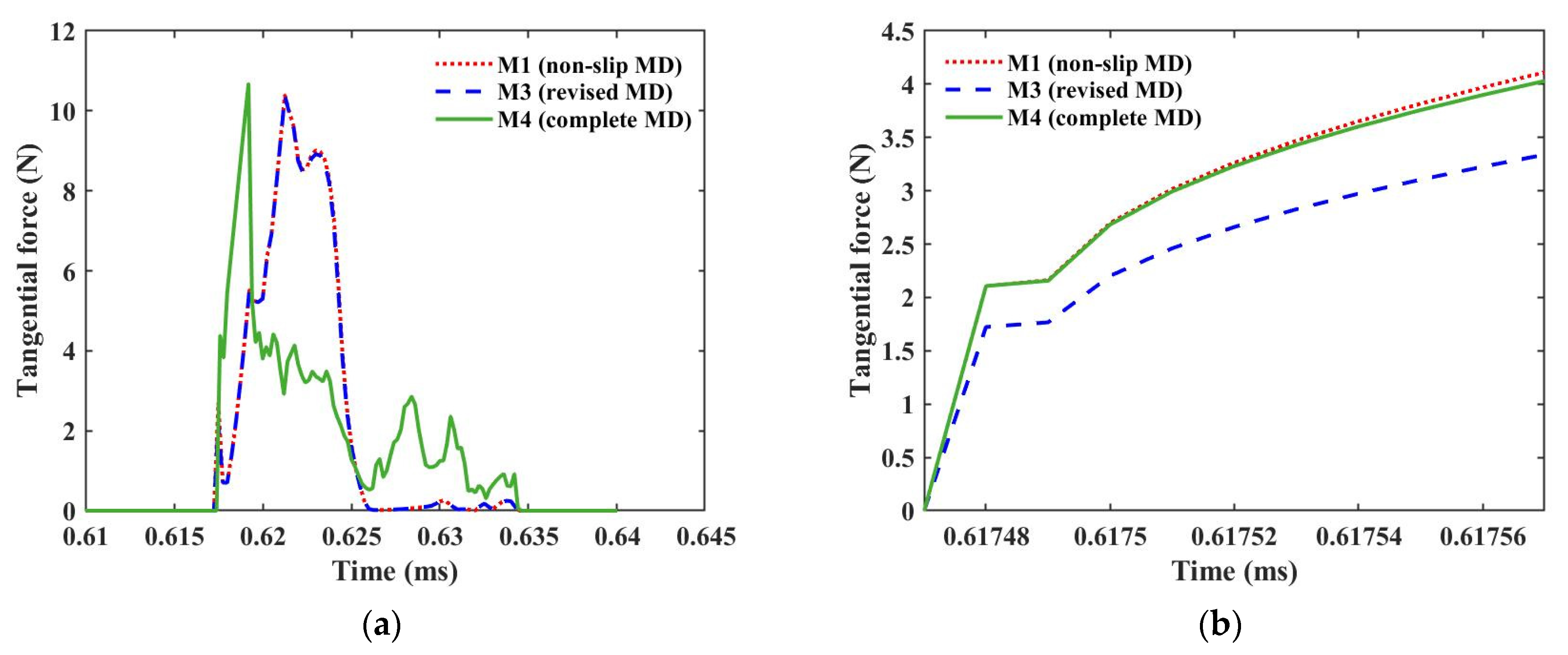
2.5. Difference of the Contact Force Models
3. Numerical Simulations
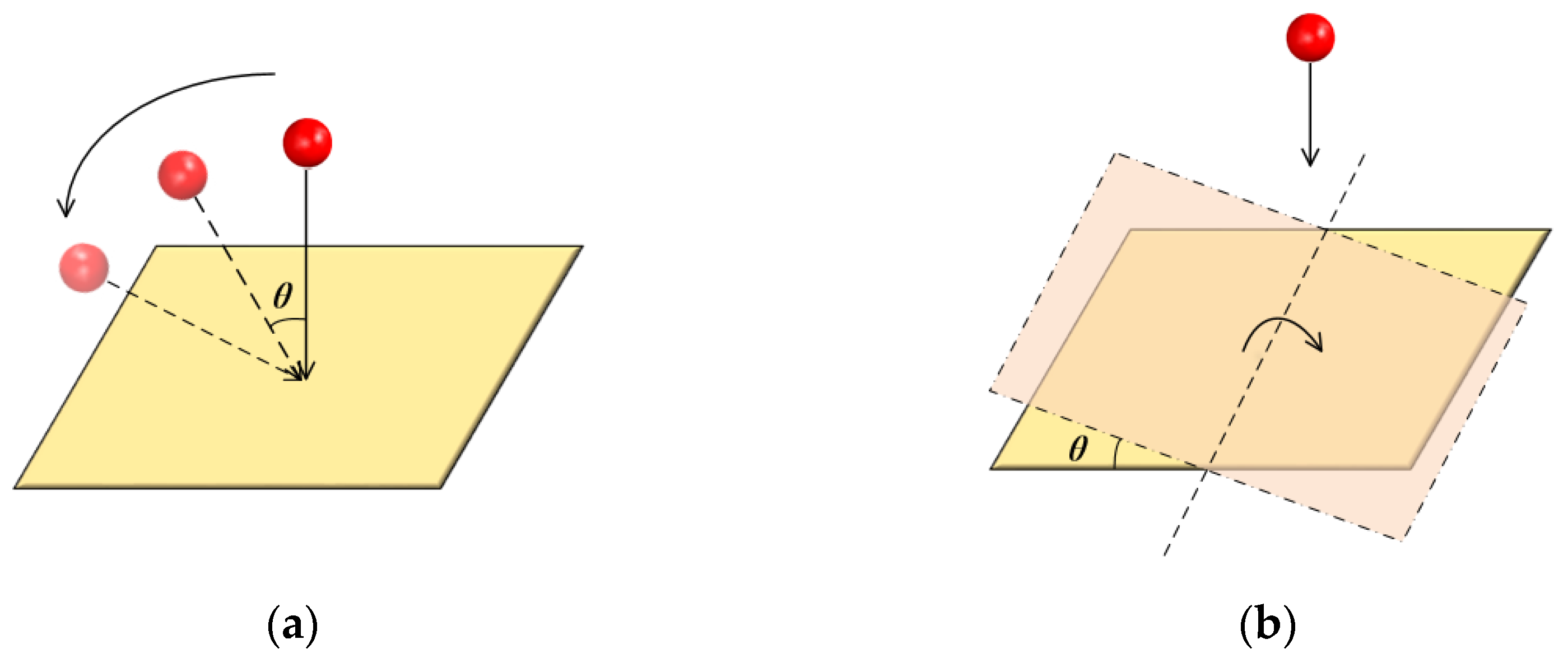
3.1. Single Collision Analyses
- (1)
- Restitution coefficients;
- (2)
- Rebound angle;
- (3)
- Angle ψ in the MBF model;
- (4)
- Energies;
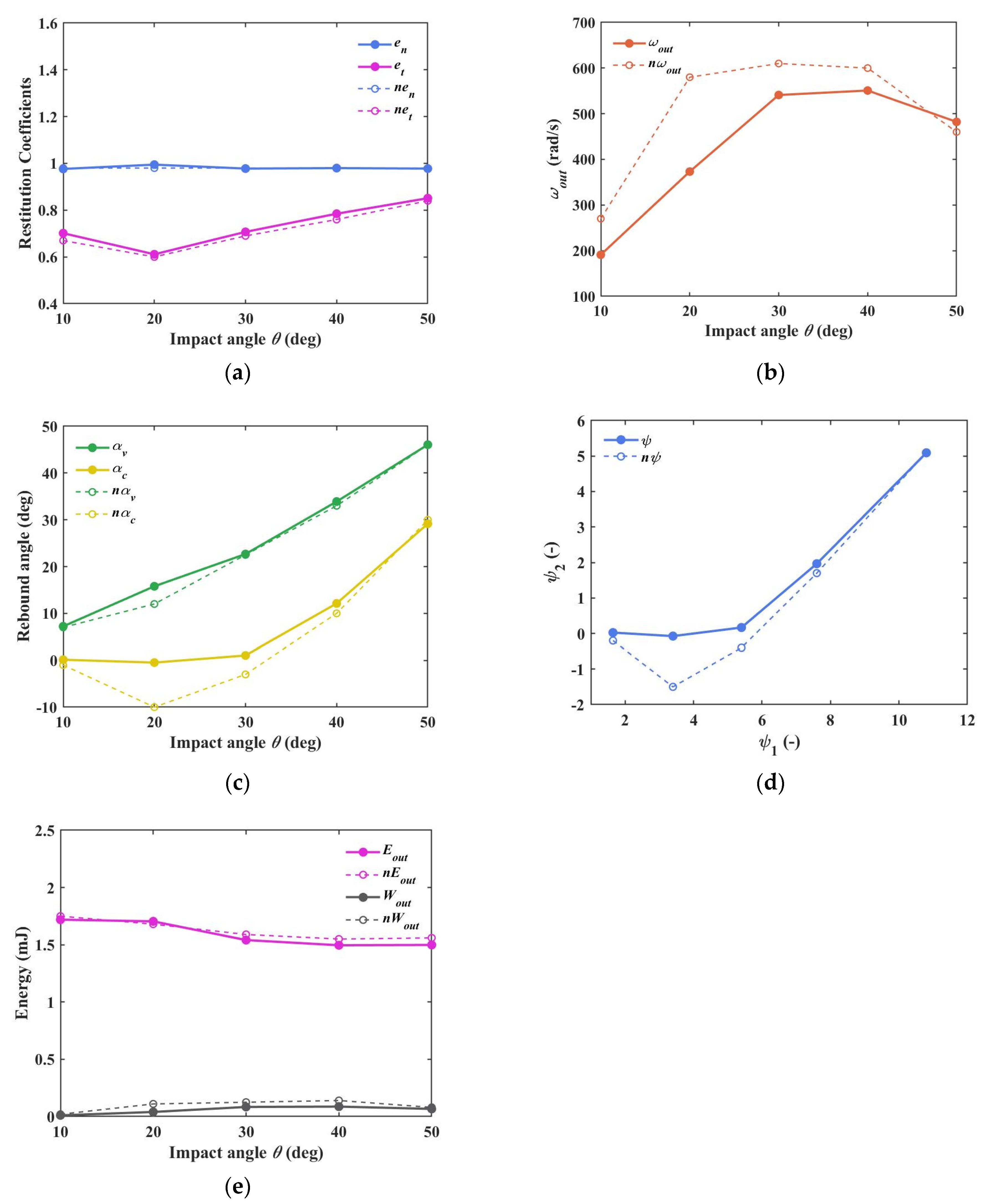
3.1.1. Performance of the Hertzian Contact with the Non-Slip MD Model (M1)
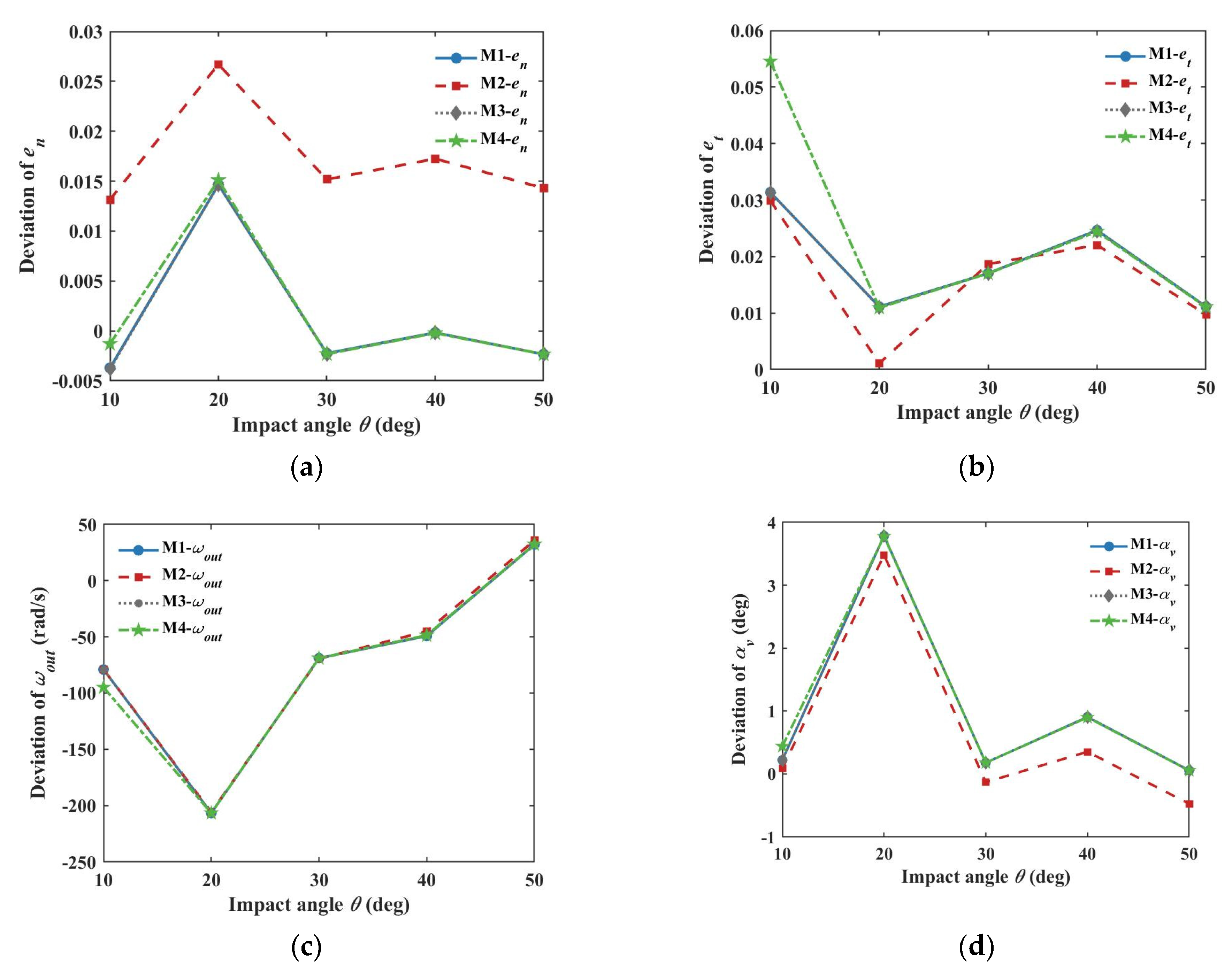
3.1.2. Comparison of the Four Contact Force Models
3.1.3. Softening of the Material
3.2. Extended Analyses Regarding the Multiple Collisions
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Cundall, P.A.; Strack, O.D.L. A discrete numerical model for granular assemblies. Geotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Zhang, Y.; Michel, P.; Barnouin, O.S.; Roberts, J.H.; Daly, M.G.; Ballouz, R.-L.; Walsh, K.J.; Richardson, D.C.; Hartzell, C.M.; Lauretta, D.S. Inferring interiors and structural history of top-shaped asteroids from external properties of asteroid (101955) Bennu. Nat. Commun. 2022, 13, 1–12. [Google Scholar] [CrossRef]
- Sánchez, P.; Renouf, M.; Azéma, E.; Mozul, R.; Dubois, F. A contact dynamics code implementation for the simulation of asteroid evolution and regolith in the asteroid environment. Icarus 2021, 363, 114441. [Google Scholar] [CrossRef]
- Schwartz, S.R.; Richardson, D.C.; Michel, P. An implementation of the soft-sphere discrete element method in a high-performance parallel gravity tree-code. Gran. Matt. 2012, 14, 363–380. [Google Scholar] [CrossRef]
- DellaGiustina, D.N.; Emery, J.P.; Golish, D.R. Properties of rubble-pile asteroid (101955) Bennu from OSIRIS-REx imaging and thermal analysis. Nat. Astron. 2019, 3, 341–351. [Google Scholar] [CrossRef]
- Zhang, X.; Luo, Y.; Xiao, Y.; Liu, D.; Guo, F.; Guo, Q. Developing prototype simulants for surface materials and morphology of near earth asteroid 2016 HO3. Space Sci. Tech. 2021, 2021, 9874929. [Google Scholar] [CrossRef]
- Huang, X.; Li, M.; Wang, X.; Hu, J.; Zhao, Y.; Guo, M.; Xu, C.; Liu, W.; Wang, Y.; Hao, C.; et al. The Tianwen-1 guidance, navigation, and control for Mars entry, descent, and landing. Space Sci. Tech. 2021, 2021, 9846185. [Google Scholar] [CrossRef]
- Hippmann, G. An algorithm for compliant contact between complexly shaped bodies. Multibody Syst. Dyn. 2004, 12, 345–362. [Google Scholar] [CrossRef]
- Richardson, D.C.; Walsh, K.J.; Murdoch, N.; Michel, P. Numerical simulations of granular dynamics: I. Hard-sphere discrete element method and tests. Icarus 2011, 212, 427–437. [Google Scholar] [CrossRef]
- Brendel, L.; Dippel, S. Lasting contacts in molecular dynamics simulations. In Physics of Dry Granular Media, 1st ed.; Herrmann, H.J., Hovi, J.P., Luding, S., Eds.; Springer: Dordrecht, The Netherlands, 1998; Volume 350, pp. 313–318. [Google Scholar] [CrossRef]
- García-Rojo, R.; McNamara, S.; Herrmann, H.J. Influence of Contact Modelling on the Macroscopic Plastic Response of Granular Soils Under Cyclic Loading. In Mathematical Models of Granular Matter, 1st ed.; Capriz, G., Mariano, P.M., Giovine, P., Eds.; Springer: Berlin, Heidelberg, 2008; Volume 1937, pp. 109–124. [Google Scholar] [CrossRef]
- Brilliantov, N.V.; Spahn, F.; Hertzsch, J.M.; Pöschel, T. Model for collisions in granular gases. Phys. Rev. E 1996, 53, 5382. [Google Scholar] [CrossRef]
- Tsuji, Y.; Tanaka, T.; Ishida, T. Lagrangian numerical simulation of plug flow of cohesionless particles in a horizontal pipe. Powder Technol. 1992, 71, 239–250. [Google Scholar] [CrossRef]
- Tsuji, Y.; Kawaguchi, T.; Tanaka, T. Discrete particle simulation of two-dimensional fluidized bed. Powder Technol. 1993, 77, 79–87. [Google Scholar] [CrossRef]
- Mindlin, R.D. Compliance of elastic bodies in contact. J. Appl. Mech. 1949, 16, 259–268. [Google Scholar] [CrossRef]
- Mindlin, R.D.; Deresiewicz, H. Elastic spheres in contact under varying oblique forces. J. Appl. Mech. 1953, 20, 327–344. [Google Scholar] [CrossRef]
- Maw, N.; Barber, J.R.; Fawcett, J.N. The oblique impact of elastic spheres. Wear 1976, 38, 101–114. [Google Scholar] [CrossRef]
- Maw, N.; Barber, J.R.; Fawcett, J.N. The role of elastic tangential compliance in oblique impact. J. Lubr. Technol. 1981, 103, 74–80. [Google Scholar] [CrossRef]
- Walton, O.R.; Braun, R.L. Viscosity, granular-temperature, and stress calculations for shearing assemblies of inelastic, frictional disks. J. Rheol. 1986, 30, 949–980. [Google Scholar] [CrossRef]
- Kruggel-Emden, H.; Wirtz, S.; Scherer, V. A study on tangential force laws applicable to the discrete element method (DEM) for materials with viscoelastic or plastic behavior. Chem. Eng. Sci. 2008, 63, 1523–1541. [Google Scholar] [CrossRef]
- Zhong, W.; Yu, A.; Liu, X.; Tong, Z.; Zhang, H. DEM/CFD-DEM modeling of non-spherical particulate systems: Theoretical developments and applications. Powder Technol. 2016, 302, 108–152. [Google Scholar] [CrossRef]
- Richardson, D.C.; Quinn, T.; Stadel, J.; Lake, G. Direct large-scale N-body simulations of planetesimal dynamics. Icarus 2000, 143, 45–59. [Google Scholar] [CrossRef]
- Cheng, B.; Yu, Y.; Baoyin, H. Collision-based understanding of the force law in granular impact dynamics. Phys. Rev. E 2018, 98, 012901. [Google Scholar] [CrossRef]
- Liu, C.; Pollard, D.D.; Shi, B. Analytical Solutions and Numerical Tests of Elastic and Failure Behaviors of Close-Packed Lattice for Brittle Rocks and Crystals. J. Geophys. Res. Solid Earth 2013, 118, 71–82. [Google Scholar] [CrossRef]
- Murdoch, N.; Drilleau, M.; Sunday, C.; Thuillet, F.; Wilhelm, A.; Nguyen, G.; Gourinat, Y. Low-velocity impacts into granular material: Application to small-body landing. Mon. Not. R. Astron. Soc. 2021, 503, 3460–3471. [Google Scholar] [CrossRef]
- Cheng, B.; Yu, Y.; Asphaug, E.; Michel, P.; Richardson, D.C.; Hirabayashi, M.; Yoshikawa, M.; Baoyin, H. Reconstructing the formation history of top-shaped asteroids from the surface boulder distribution. Nat. Astron. 2021, 5, 134–138. [Google Scholar] [CrossRef]
- Raducan, S.D.; Jutzi, M.; Zhang, Y.; Ormö, J.; Michel, P. Reshaping and ejection processes on rubble-pile asteroids from impacts. Astron. Astrophys. 2022, 665, L10. [Google Scholar] [CrossRef]
- Kruggel-Emden, H.; Wirtz, S.; Scherer, V. Applicable contact force models for the discrete element method: The single particle perspective. J. Press. Vessel Technol. 2009, 131, 024001. [Google Scholar] [CrossRef]
- Gorham, D.A.; Kharaz, A.H. The measurement of particle rebound characteristics. Powder Technol. 2000, 112, 193–202. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, J.; Zeng, Z.; Wen, T.; Li, Z. High-fidelity landing simulation of small body landers: Modeling and mass distribution effects on bouncing motion. Aerosp. Sci. Technol. 2021, 119, 107149. [Google Scholar] [CrossRef]
- Zeng, X.; Wen, T.; Li, Z.; Alfriend, K.T. Natural Landing Simulations on Generated Local Rocky Terrains for Asteroid Cubic Lander. IEEE Trans. Aerosp. Electron. Syst. 2022, 58, 3492–3508. [Google Scholar] [CrossRef]
- Wen, T.; Zeng, X.; Circi, C.; Gao, Y. Hop reachable domain on irregularly shaped asteroids. J. Guid. Control Dyn. 2020, 43, 1269–1283. [Google Scholar] [CrossRef]
- Zeng, X.; Li, Z.; Wen, T.; Zhang, Y. Influence of the lander size and shape on the ballistic landing motion. Earth Space Sci. 2022, 9, e2021EA001952. [Google Scholar] [CrossRef]
- Ebrahimi, S.; Hippmann, G.; Eberhard, P. Extension of the polygonal contact model for flexible multibody systems. Int. J. Appl. Math. Mech. 2005, 1, 33–50. [Google Scholar]
- Zeng, X.; Wen, T.; Yu, Y.; Cheng, B.; Qiao, D. New practical discrete non-spherical N-body method: Validation with the Brazil nut effect. Icarus 2022, 387, 115201. [Google Scholar] [CrossRef]
- Wen, T.; Zeng, X.; Li, Z.; Zhang, Y. Size segregation of non-spherical particles under different gravity levels. Planet. Space Sci. 2022; submitted. [Google Scholar]
- Serra-Aguila, A.; Puigoriol-Forcada, J.M.; Reyes, G.; Menacho, J. Viscoelastic models revisited: Characteristics and interconversion formulas for generalized Kelvin-Voigt and Maxwell models. Acta Mech. Sin. 2019, 35, 1191–1209. [Google Scholar] [CrossRef]
- Vu-Quoc, L.; Zhang, X. An accurate and efficient tangential force-displacement model for elastic frictional contact in particle-flow simulations. Mech. Mater. 1999, 31, 235–269. [Google Scholar] [CrossRef]
- Hunt, K.H.; Crossley, F.R.E. Coefficient of Restitution Interpreted as Damping in Vibroimpact. J. Appl. Mech. 1975, 42, 440. [Google Scholar] [CrossRef]
- Wada, K.; Senshu, H.; Matsui, T. Numerical simulation of impact cratering on granular material. Icarus 2006, 180, 528–545. [Google Scholar] [CrossRef]
- Cheng, B.; Asphaug, E.; Yu, Y.; Baoyin, H. Measuring the mechanical properties of small body regolith layers using a granular penetrometer. Astrodynamics 2023, 7, 15–29. [Google Scholar] [CrossRef]
- Antypov, D.; Elliott, J.A. On an analytical solution for the damped Hertzian spring. Europhys. Lett. 2011, 94, 50004. [Google Scholar] [CrossRef]
- Hu, G. Analysis and Simulation of Granular System by Discrete Element Method Using EDEM, 1st ed.; Wuhan University of Technology Press: Wuhan, China, 2010; pp. 12–15. [Google Scholar]
- Di Renzo, A.; Di Maio, F.P. An improved integral non-linear model for the contact of particles in distinct element simulations. Chem. Eng. Sci. 2005, 60, 1303–1312. [Google Scholar] [CrossRef]
- Di Renzo, A.; Di Maio, F.P. Comparison of contact-force models for the simulation of collisions in DEM-based granular flow codes. Chem. Eng. Sci. 2004, 59, 525–541. [Google Scholar] [CrossRef]
- Thornton, C.; Cummins, S.J.; Cleary, P.W. An investigation of the comparative behaviour of alternative contact force models during inelastic collisions. Powder Technol. 2013, 233, 30–46. [Google Scholar] [CrossRef]
- Thornton, C.; Cummins, S.J.; Cleary, P.W. An investigation of the comparative behaviour of alternative contact force models during elastic collisions. Powder Technol. 2011, 210, 189–197. [Google Scholar] [CrossRef]
- Kharaz, A.H.; Gorham, D.A.; Salman, A.D. An experimental study of the elastic rebound of spheres. Powder Technol. 2001, 120, 281–291. [Google Scholar] [CrossRef]
- Džiugys, A.; Peters, B. An approach to simulate the motion of spherical and non-spherical fuel particles in combustion chambers. Gran. Matt. 2001, 3, 231–266. [Google Scholar] [CrossRef]
- Cheng, B. Granular Dynamics for Small Body Touchdown Missions. Ph.D. Thesis, Tsinghua University, Beijing, China, 2021. [Google Scholar]
- Sun, Q.; Wang, G. Introduction to Mechanics of Granular Matter, 1st ed.; Science Press: Beijing, China, 2009; pp. 162–163. [Google Scholar]
- Hoomans, B. Granular Dynamics of Gas Solid Two Phase Flows. Ph.D. Thesis, Universiteit Twente, Enschede, The Netherlands, 2000. [Google Scholar]
- Perera, V.; Jackson, A.P.; Asphaug, E.; Ballouz, R.-L. The spherical Brazil Nut Effect and its significance to asteroids. Icarus 2016, 278, 194–203. [Google Scholar] [CrossRef]



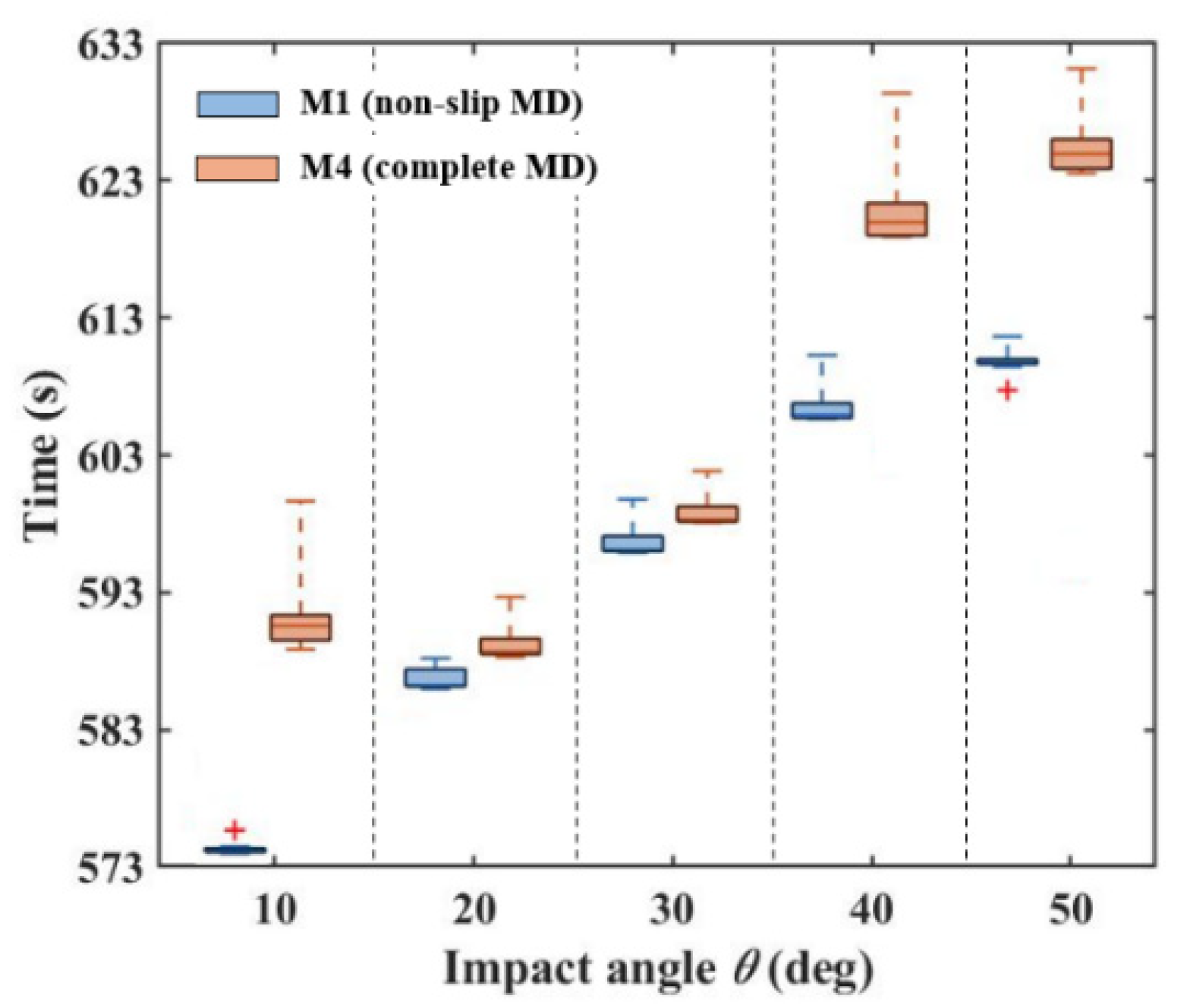
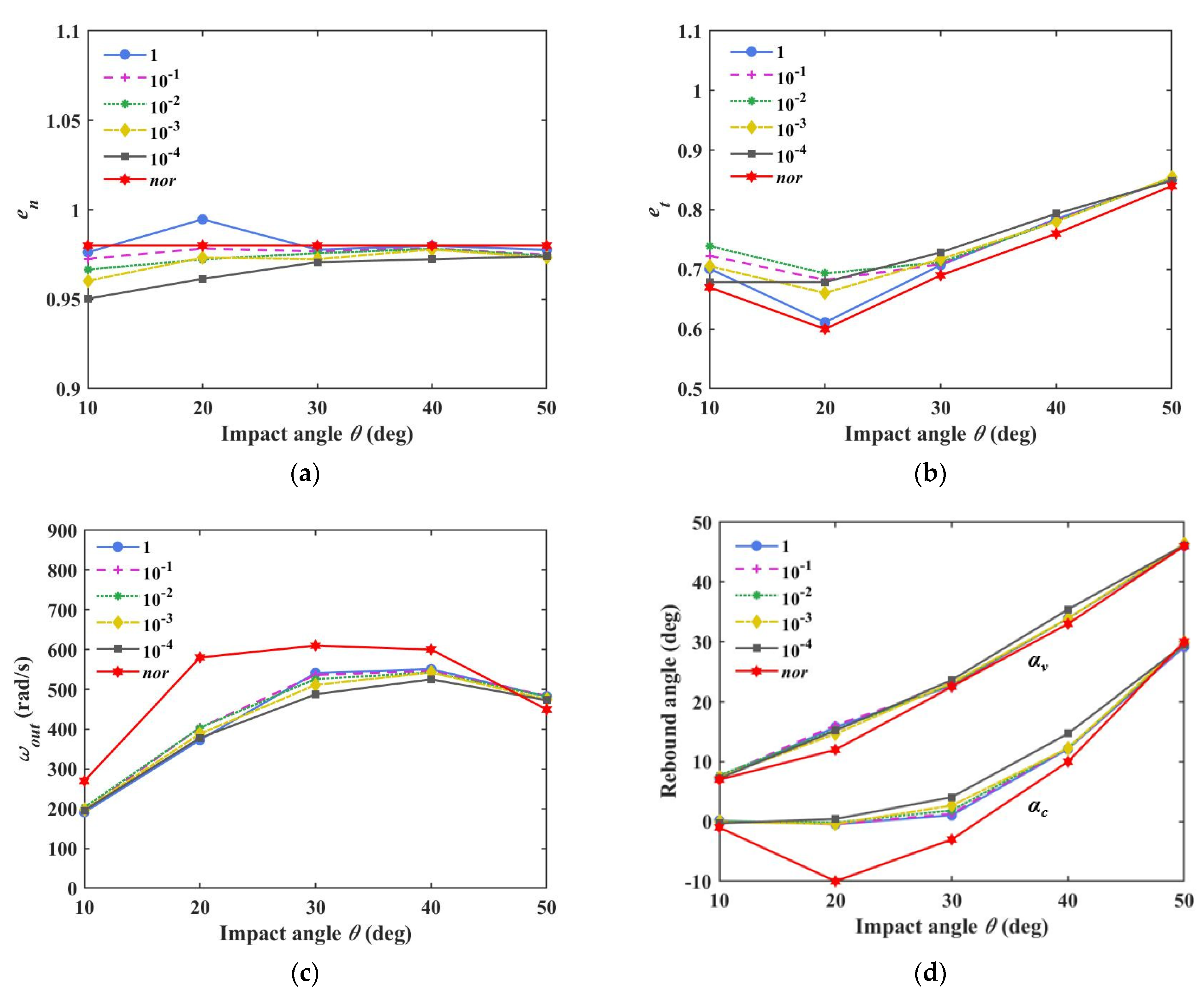

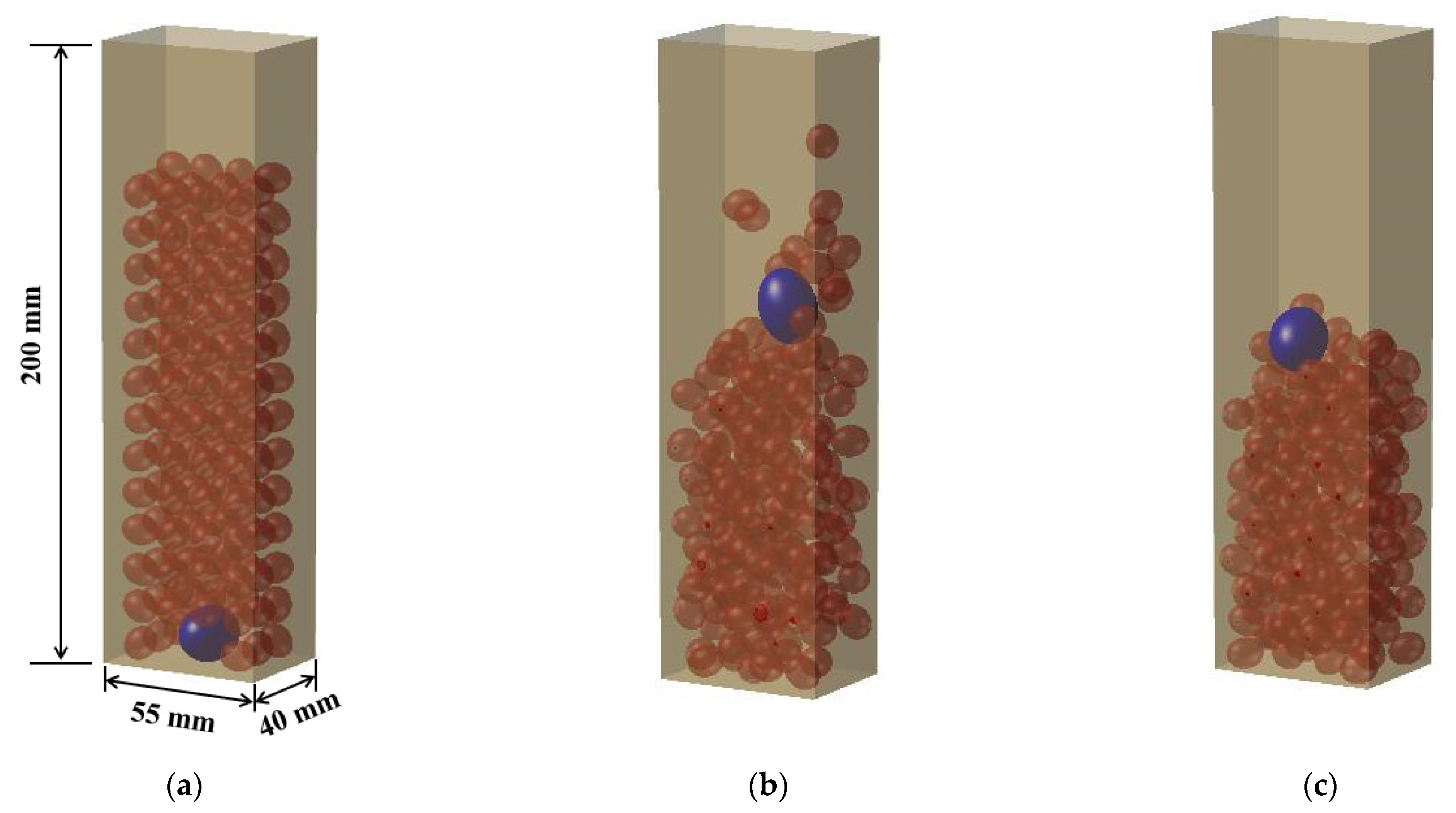
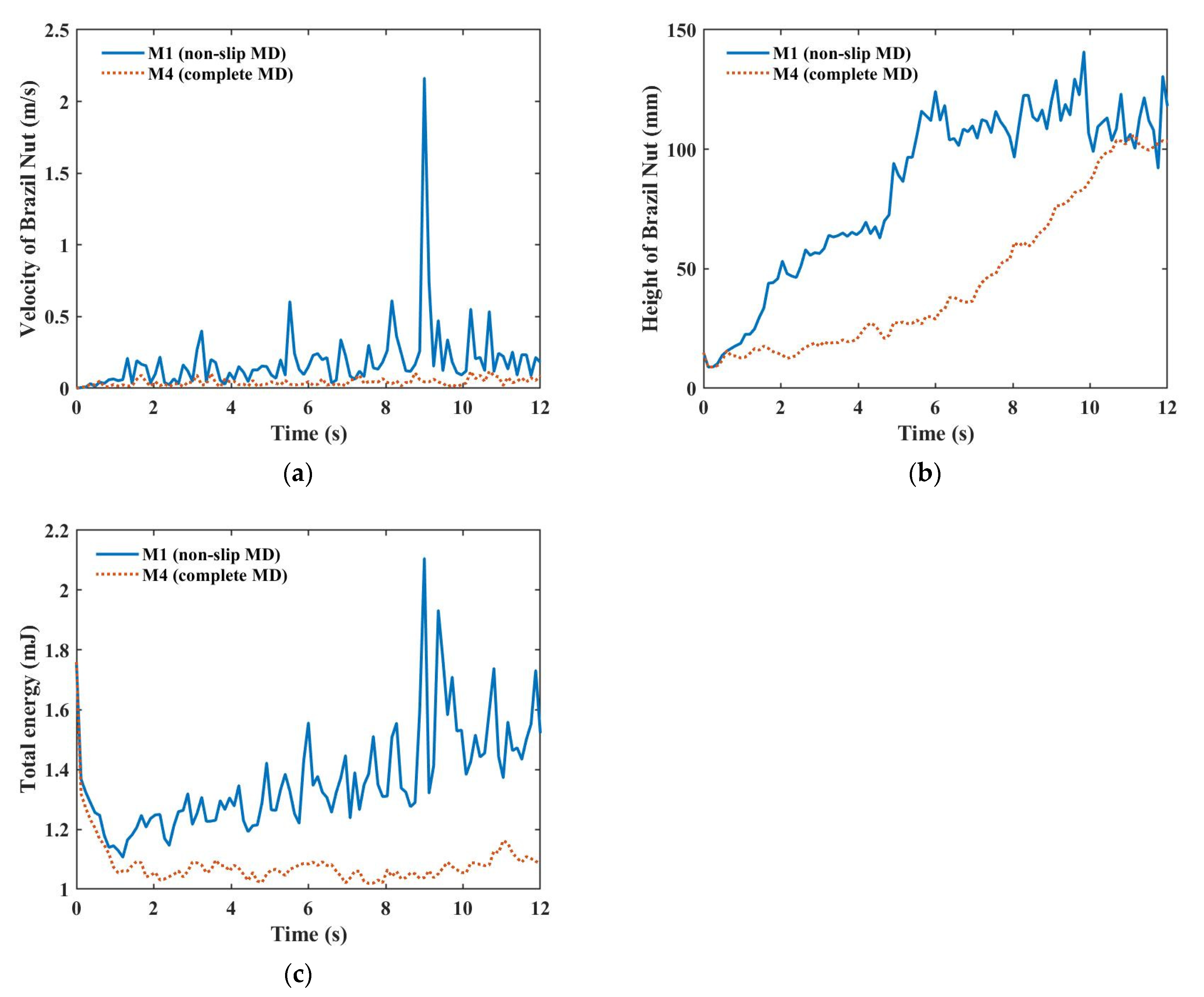
| Material | Young’s Modulus (Gpa) | Poisson Ratio | Shear Modulus (Gpa) | Vertices | Facets |
|---|---|---|---|---|---|
| Aluminum oxide sphere | 380 | 0.23 | 154 | 2562 | 5160 |
| Soda-lime glass anvil | 70 | 0.25 | 28 | 1906 | 3638 |
| Particle | Mass | Size | Vertices | Facets |
|---|---|---|---|---|
| Peanut | 0.0019 kg | 6 × 5 × 5 mm | 810 | 1616 |
| Brazil nut | 0.012 kg | 12 × 9 × 9 mm | 2864 | 5724 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.; Zeng, X.; Wen, T.; Zhang, Y. Numerical Comparison of Contact Force Models in the Discrete Element Method. Aerospace 2022, 9, 737. https://doi.org/10.3390/aerospace9110737
Li Z, Zeng X, Wen T, Zhang Y. Numerical Comparison of Contact Force Models in the Discrete Element Method. Aerospace. 2022; 9(11):737. https://doi.org/10.3390/aerospace9110737
Chicago/Turabian StyleLi, Ziwen, Xiangyuan Zeng, Tongge Wen, and Yonglong Zhang. 2022. "Numerical Comparison of Contact Force Models in the Discrete Element Method" Aerospace 9, no. 11: 737. https://doi.org/10.3390/aerospace9110737
APA StyleLi, Z., Zeng, X., Wen, T., & Zhang, Y. (2022). Numerical Comparison of Contact Force Models in the Discrete Element Method. Aerospace, 9(11), 737. https://doi.org/10.3390/aerospace9110737


_Zhu.png)




