A Planar Underactuated Compaction Mechanism with Self-Adaptability for Automated Fiber Placement Heads
Abstract
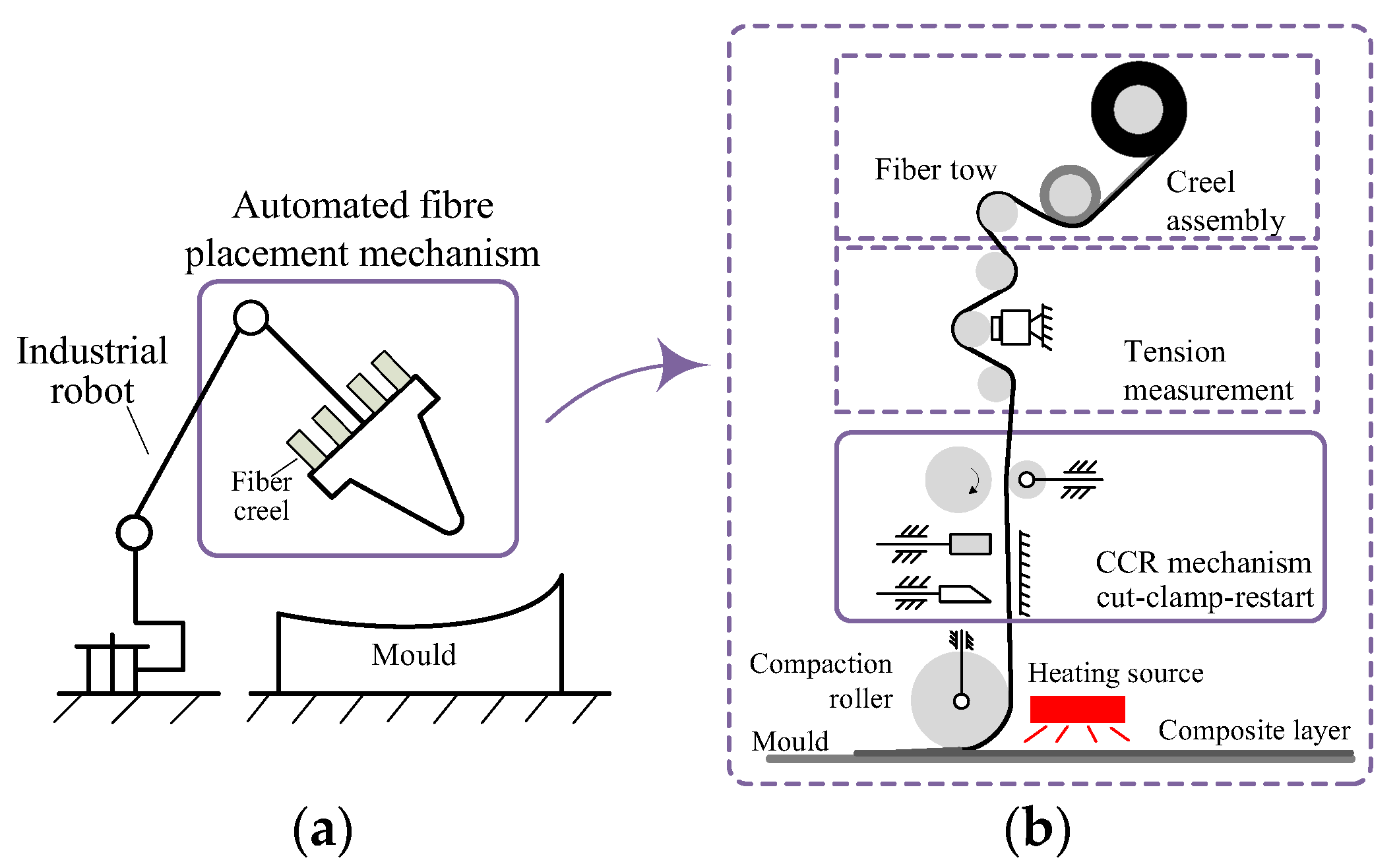
1. Introduction
2. Methods
2.1. Premise and Conceptual Design
2.1.1. Design Premise
- All masses and inertia of the links and parts are negligible;
- The flexible element only deforms in an elastic range along the link;
- The frame of the design is fixed with an AFP head, and the actuation force Fa is applied to the center of the part in the direction aligned to the mechanism frame (shown as link T in Figure 2).
2.1.2. Requirements
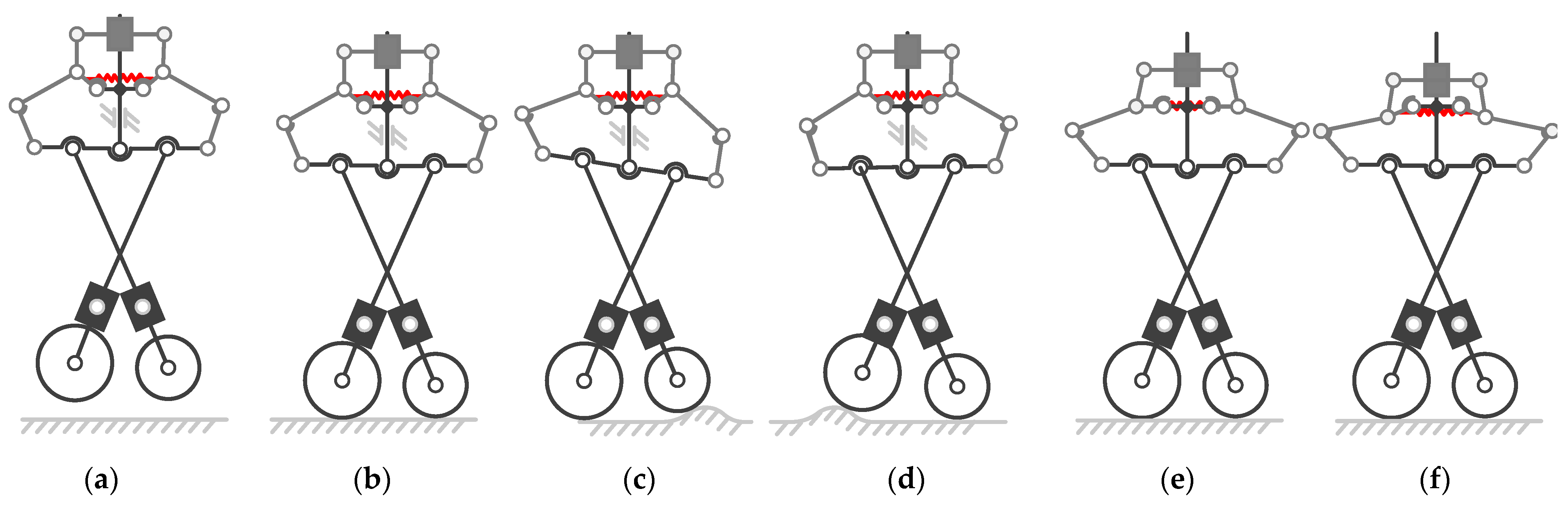
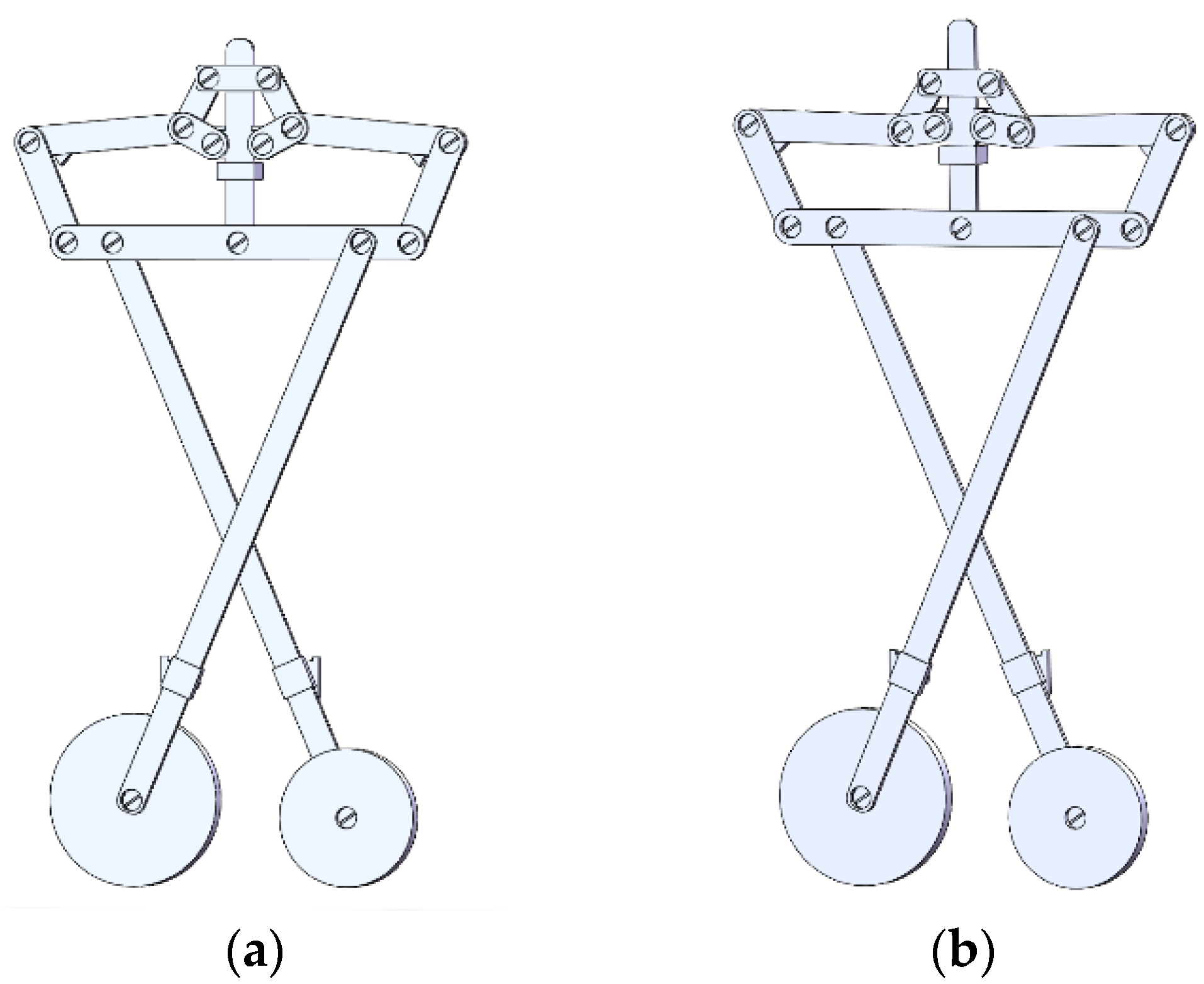
2.2. Final Concept
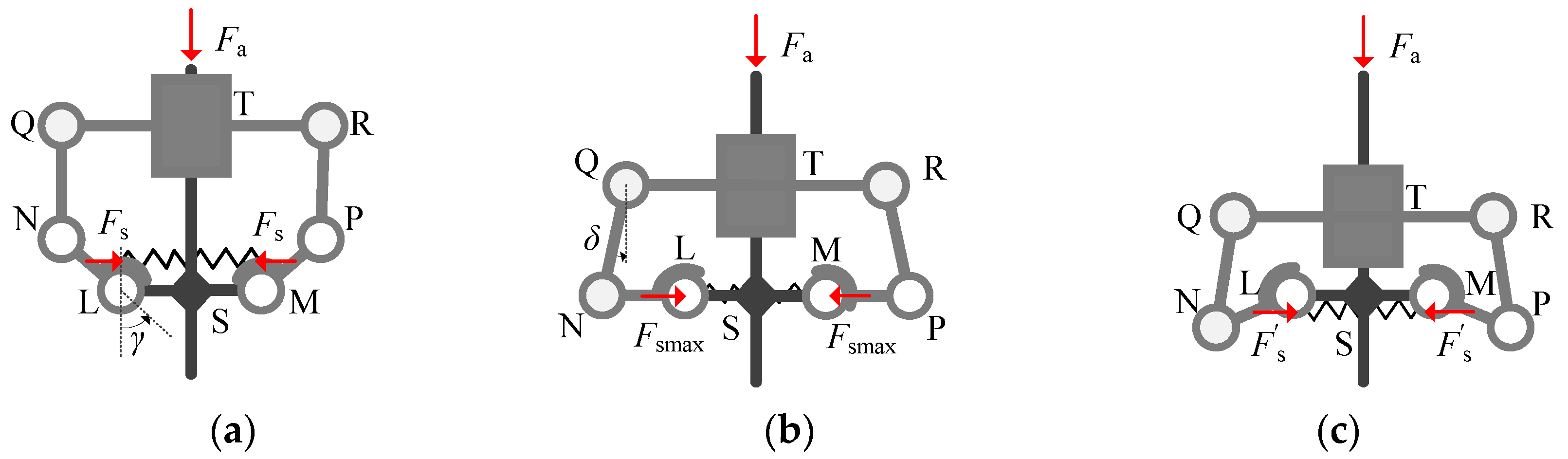
3. Analytical Model
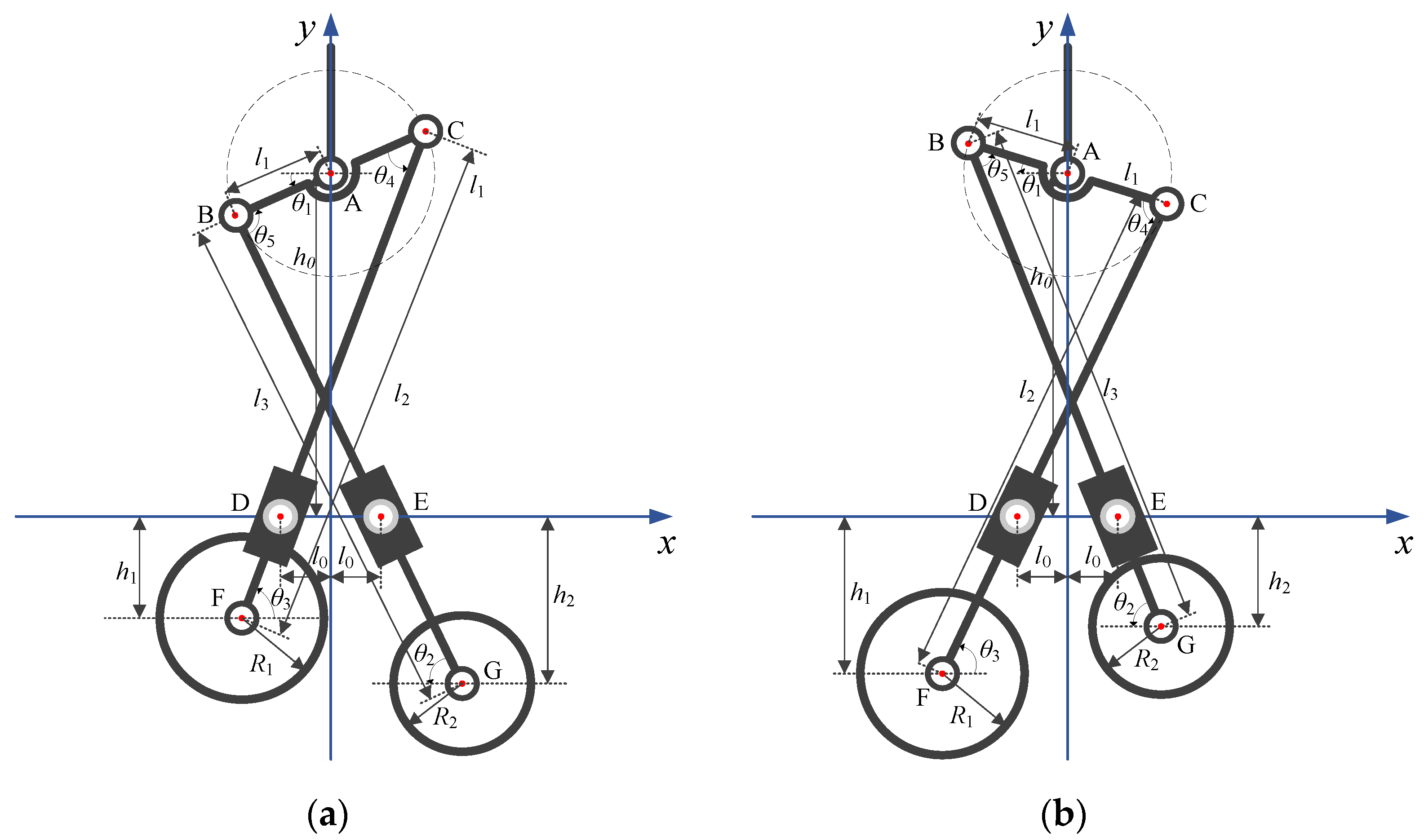
3.1. Compaction Mechanism Geometry Equation
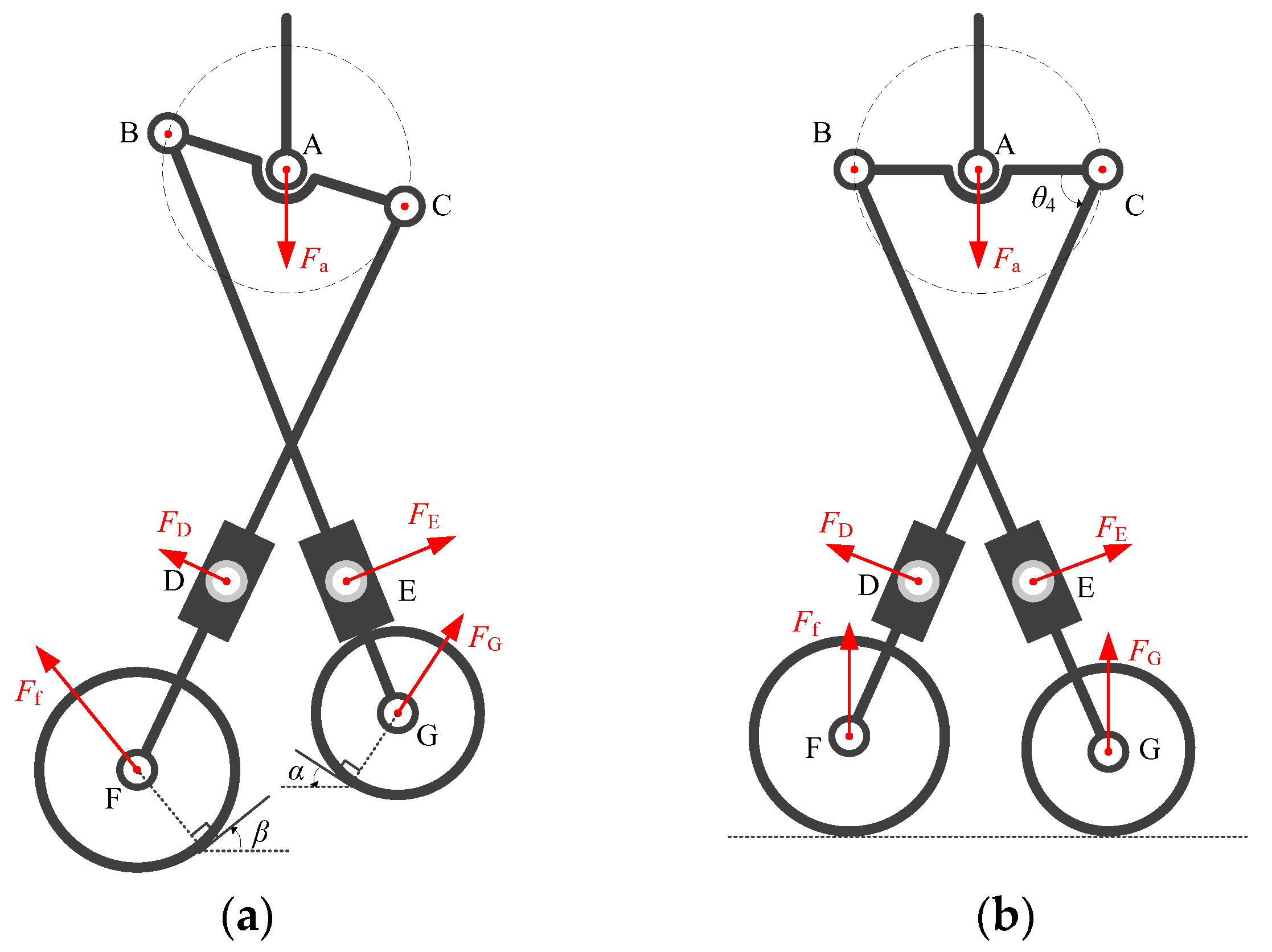
3.2. Contact Force
3.3. Dimensional Design of the Concept
- Contact forces must be required to be positive and to apply between the compaction roller and mold surface;
- Unstable compaction behavior and singular configuration are avoided by obtaining a consistent force perpendicular to the contoured surfaces;
- Clashing or overlapping among the components must be avoided.
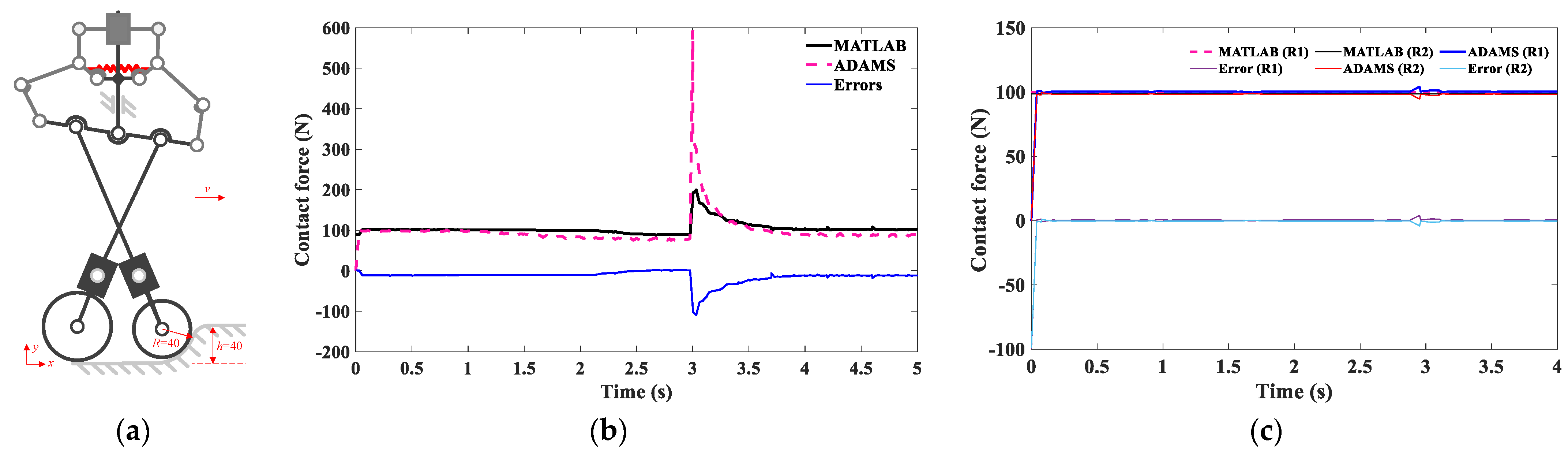
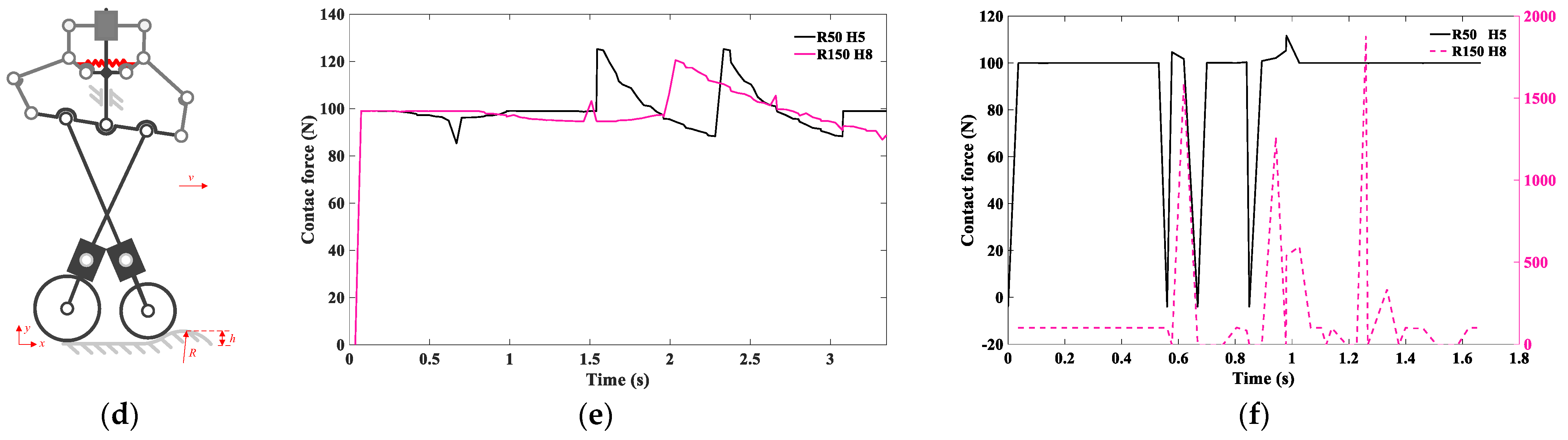
3.4. Experiments Analysis
4. Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Liu, F.; Ferraris, E.; Ivens, J. Mechanical Investigation and Microstructure Performance of a Two-Matrix Continuous Carbon Fibre Composite Fabricated by 3D Printing. J. Manuf. Process. 2022, 79, 383–393. [Google Scholar] [CrossRef]
- Croft, K.; Lessard, L.; Pasini, D.; Hojjati, M.; Chen, J.; Yousefpour, A. Experimental Study of the Effect of Automated Fiber Placement Induced Defects on Performance of Composite Laminates. Compos. Part A 2011, 42, 484–491. [Google Scholar] [CrossRef]
- Skinner, M.L. Trends, Advances and Innovations in Filament Winding. Reinf. Plast. 2006, 50, 28–33. [Google Scholar] [CrossRef]
- Grant, C. Automated Processes for Composite Aircraft Structure. Ind. Robot Int. J. 2006, 33, 117–121. [Google Scholar] [CrossRef]
- Zhang, W.; Liu, F.; Jiang, T.; Minghui, Y.I.; Chen, W.; Ding, X. Overview of Current Design and Analysis of Potential Theories for Automated Fibre Placement Mechanisms. Chin. J. Aeronaut. 2022, 35, 13. [Google Scholar] [CrossRef]
- Oromiehie, E.; Gain, A.K.; Prusty, B.G. Processing Parameter Optimisation for Automated Fibre Placement (AFP) Manufactured Thermoplastic Composites. Compos. Struct. 2021, 272, 114223. [Google Scholar] [CrossRef]
- Bendemra, H.; Vincent, M.J.; Compston, P. Optimisation of Compaction Force for Automated Fibre Placement. In Proceedings of the 8th Australasian Congress on Applied Mechanics, ACAM 2014, as Part of Engineers Australia Convention 2014, Melbourne, Australia, 25–26 November 2014; pp. 957–965. [Google Scholar]
- Vaniglia, M.M. Fiber Placement Head. U.S. Patent No. 5,110,395, 5 May 1992. [Google Scholar]
- Martin, J.P. Multiple Tape Laying Apparatus and Method. U.S. Patent No. 7,293,590, 13 November 2007. [Google Scholar]
- Yarlagadda, S.; Gillespie, J.W., Jr. Development of an Automated Materials Placement (AMP) System for Composite Processing. In Proceedings of the SAMPE 2012, Baltimore, MD, USA, 21–24 May 2012; pp. 1–14. [Google Scholar]
- Denkena, B.; Schmidt, C.; Weber, P. Automated Fiber Placement Head for Manufacturing of Innovative Aerospace Stiffening Structures. Procedia Manuf. 2016, 6, 96–104. [Google Scholar] [CrossRef]
- Mischler, P.L.; Tingley, M.C.; Hoffmann, K. Compaction Roller for a Fiber Placement Machine. U.S. Patent No. 7,810,539, 12 October 2010. [Google Scholar]
- Johnson, B.A. Conformable Compaction Apparatus for Use with a Fiber Placement Machine. U.S. Patent No. 6,390,169, 21 May 2002. [Google Scholar]
- Steeg, M.; Schledjewski, R.; Schlarb, A.K. Automation Implementation and Process Development of Thermoplastic Tape Placement for 3-Dimensional Parts. SAMPE J. 2006, 42, 18–24. [Google Scholar]
- Chapman, M.R.; Watson, R.M.; Williams, S.H. Compaction Method and Device for Automated Fiber Placement. U.S. Patent No. 9,849,635, 26 December 2017. [Google Scholar]
- Miller, L.G.; Harris, D.G.; Benson, V.M.; Shupe, K.G.; Wade, K.L. Compaction Device for Fiber Placement Using Interdependent Segment Travel. U.S. Patent No. 8,042,594, 25 October 2011. [Google Scholar]
- Hu, X. Design and Research of Fiber Placement Machine. Ph.D. Dissertation, Wuhan University of Technology, Wuhan, China, 1 June 2011. [Google Scholar]
- Van Hoa, S.; Duc Hoang, M.; Simpson, J. Manufacturing Procedure to Make Flat Thermoplastic Composite Laminates by Automated Fibre Placement and Their Mechanical Properties. J. Thermoplast. Compos. Mater. 2017, 30, 1693–1712. [Google Scholar] [CrossRef]
- Khan, M.A.; Mitschang, P.; Schledjewski, R. Identification of Some Optimal Parameters to Achieve Higher Laminate Quality through Tape Placement Process. Adv. Polym. Technol. 2010, 29, 98–111. [Google Scholar] [CrossRef]
- Oldani, L.E. Fiber Placement Head with Secondary Compaction Arrangement. U.S. Patent Application No. 15/639,944, 30 June 2018. [Google Scholar]
- August, Z.; Hauber, H. Additive Manufacturing of High-Performance Composite Structures. 2014. Available online: https://www.semanticscholar.org/paper/ADDITIVE-MANUFACTURING-OF-HIGH-PERFORMANCE-August/a2813fc138d9be4f71976f7ae56a48d12036f874 (accessed on 1 September 2022).
- Tam, A.S. Control of Thermoplastic Tow Placement. U.S. Patent No. 5,447,586, 5 September 1995. [Google Scholar]
- Lamontia, M.A.; Gruber, M.B.; Waibel, B.J.; Cope, R.D.; Hulcher, A.B. Conformable Compaction System Used in Automated Fiber Placement of Large Composite Aerospace Structures. In Proceedings of the 23rd SAMPE EUROPE Conference, Paris, France, 9–11 April 2002; pp. 9–11. [Google Scholar]
- Sorrentino, L.; Carrino, L.; Tersigni, L.; Leone, A. Innovative Tape Placement Robotic Cell: High Flexibility System to Manufacture Composite Structural Parts with Variable Thickness. J. Manuf. Sci. Eng. 2009, 131, 041002. [Google Scholar] [CrossRef]
- Firouzeh, A.; Paik, J. Grasp Mode and Compliance Control of an Underactuated Origami Gripper Using Adjustable Stiffness Joints. IEEE/ASME Trans. Mechatron. 2017, 22, 2165–2173. [Google Scholar] [CrossRef]
- Qiao, S.; Guo, H.; Liu, R.; Deng, Z. Self-Adaptive Grasp Process and Equilibrium Configuration Analysis of a 3-DOF UACT Robotic Finger. Mech. Mach. Theory 2019, 133, 250–266. [Google Scholar] [CrossRef]
- Kim, H.K.; Jeong, H.; Park, J.; Park, J.; Kim, W.-S.; Kim, N.; Park, S.; Paik, N.-J. Development of a Comprehensive Design Guideline to Evaluate the User Experiences of Meal-Assistance Robots Considering Human-Machine Social Interactions. Int. J. Hum. Comput. Interact. 2022, 38, 1687–1700. [Google Scholar] [CrossRef]
- Aukes, D.; Kim, S.; Garcia, P.; Edsinger, A.; Cutkosky, M.R. Selectively Compliant Underactuated Hand for Mobile Manipulation. In Proceedings of the IEEE International Conference on Robotics & Automation, Saint Paul, MN, USA, 14–18 May 2012; pp. 2824–2829. [Google Scholar]
- Stavenuiter, R.A.J.; Birglen, L.; Herder, J.L. A Planar Underactuated Grasper with Adjustable Compliance. Mech. Mach. Theory 2017, 112, 295–306. [Google Scholar] [CrossRef]
- Tutsoy, O.; Barkana, D.E. Model Free Adaptive Control of the Under-Actuated Robot Manipulator with the Chaotic Dynamics. ISA Trans. 2021, 118, 106–115. [Google Scholar] [CrossRef] [PubMed]
- Qin, G.; Wu, H.; Cheng, Y.; Pan, H.; Zhao, W.; Shi, S.; Song, Y.; Ji, A. Adaptive Trajectory Control of an Under-Actuated Snake Robot. Appl. Math. Model 2022, 106, 756–769. [Google Scholar] [CrossRef]
- Zhang, W.X.; Ding, X.L.; Dai, J.S. Morphological Synthesis of Metamorphic Mechanisms Based on Constraint Variation. ARCHIVE Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2011, 225, 2997–3010. [Google Scholar] [CrossRef]
- Baho, O.; Ausias, G.; Grohens, Y.; Férec, J. Simulation of Laser Heating Distribution for a Thermoplastic Composite: Effects of AFP Head Parameters. Int. J. Adv. Manuf. Technol. 2020, 110, 2105–2117. [Google Scholar] [CrossRef]


| Parameter | Value |
|---|---|
| 28 mm | |
| 60 mm | |
| 300.8 mm | |
| 292 mm | |
| 50 mm | |
| 75 mm | |
| 26 mm | |
| 16 mm | |
| 40 mm | |
| 1.396 rad | |
| 40 mm | |
| 32 mm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, F.; Zhang, W.; Shang, J.; Yi, M.; Wang, S.; Ding, X. A Planar Underactuated Compaction Mechanism with Self-Adaptability for Automated Fiber Placement Heads. Aerospace 2022, 9, 586. https://doi.org/10.3390/aerospace9100586
Liu F, Zhang W, Shang J, Yi M, Wang S, Ding X. A Planar Underactuated Compaction Mechanism with Self-Adaptability for Automated Fiber Placement Heads. Aerospace. 2022; 9(10):586. https://doi.org/10.3390/aerospace9100586
Chicago/Turabian StyleLiu, Fei, Wuxiang Zhang, Junfan Shang, Minghui Yi, Shenru Wang, and Xilun Ding. 2022. "A Planar Underactuated Compaction Mechanism with Self-Adaptability for Automated Fiber Placement Heads" Aerospace 9, no. 10: 586. https://doi.org/10.3390/aerospace9100586
APA StyleLiu, F., Zhang, W., Shang, J., Yi, M., Wang, S., & Ding, X. (2022). A Planar Underactuated Compaction Mechanism with Self-Adaptability for Automated Fiber Placement Heads. Aerospace, 9(10), 586. https://doi.org/10.3390/aerospace9100586






