Design and Analysis of the Attitude Adjustment Mechanism for a Rotor MAV Based on Compliant Mechanisms
Abstract
1. Introduction
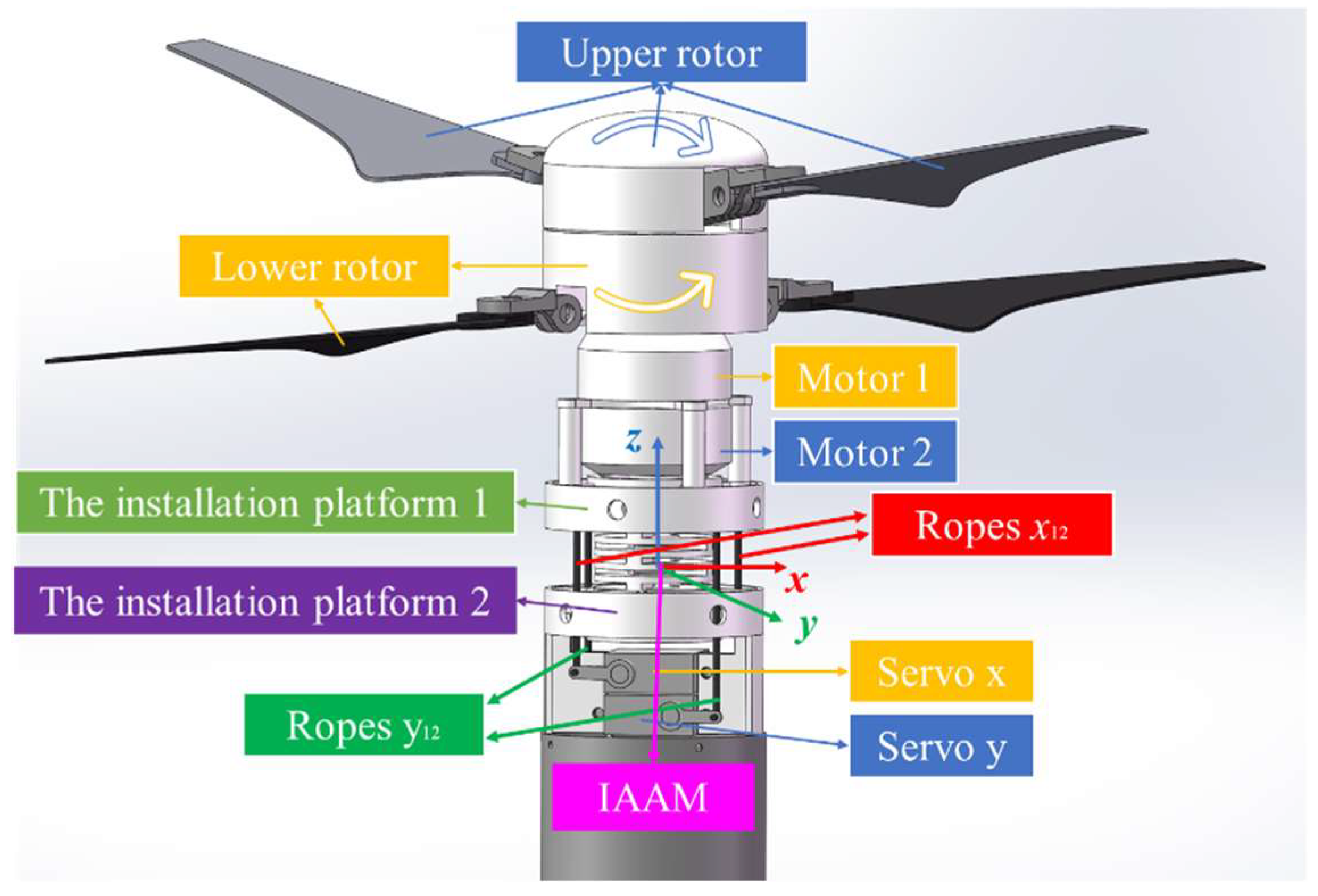
2. Mechanism Design
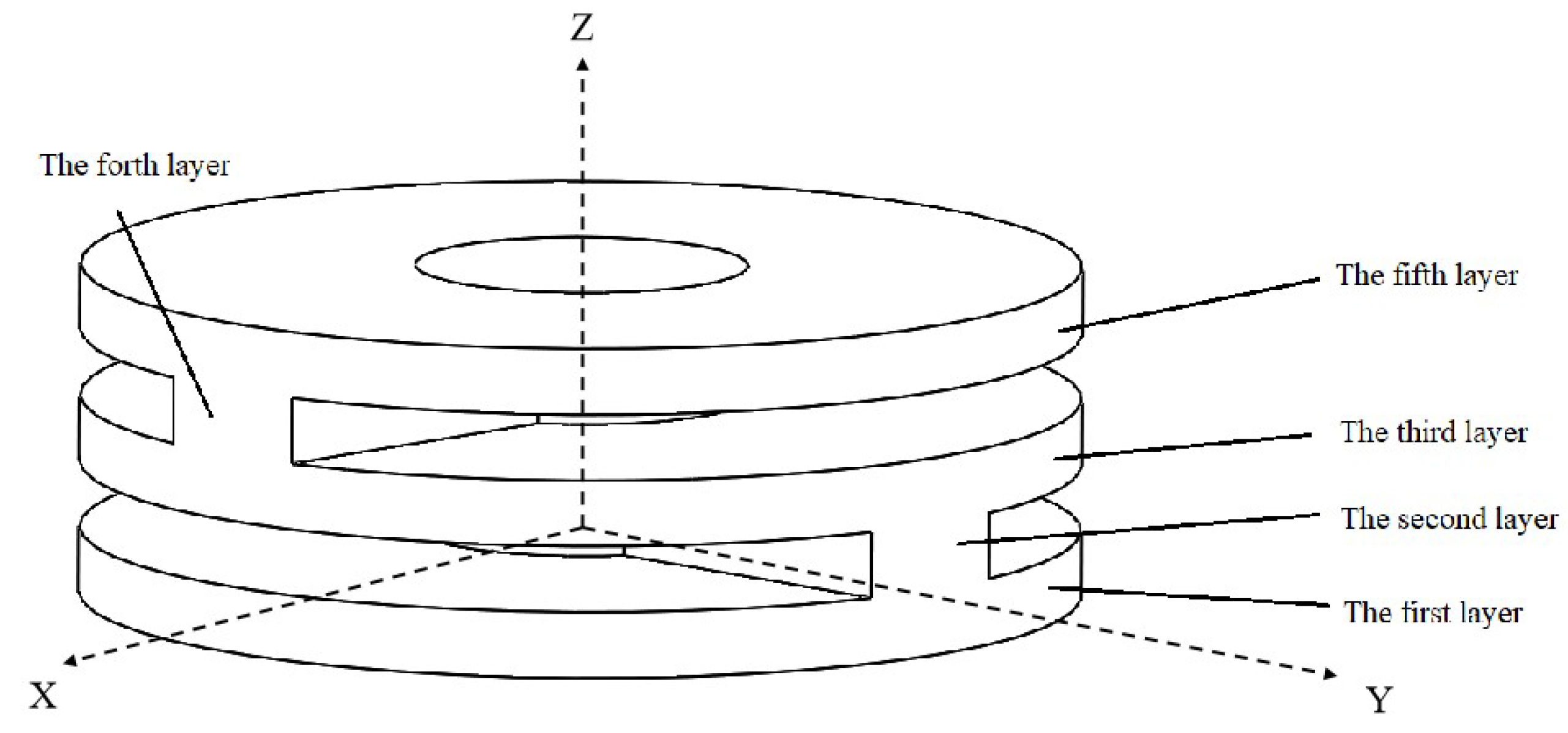
2.1. Preliminary Design of the IAAM
- For applicability to micro-rotor aircraft, the helicopter blade diameter is less than 300 mm, and the mass is less than 500 g.
- The deflection angle of the x-, y-, and z-axes (the x, y, and z coordinates are shown in Figure 2) is ± 15°;
- The stiffness in the Z-axis direction is large; thus, the vertical deformation of the IAAM is small when it is subjected to a 500 g lift during hovering. The shape variable is less than 2% of the height of the intermediate axis.
- To satisfy the requirements of a lightweight micro-helicopter, the weight of the IAAM should be less than 15 g.
- The factor of safety (FOS) of the IAAM is greater than 1.5.
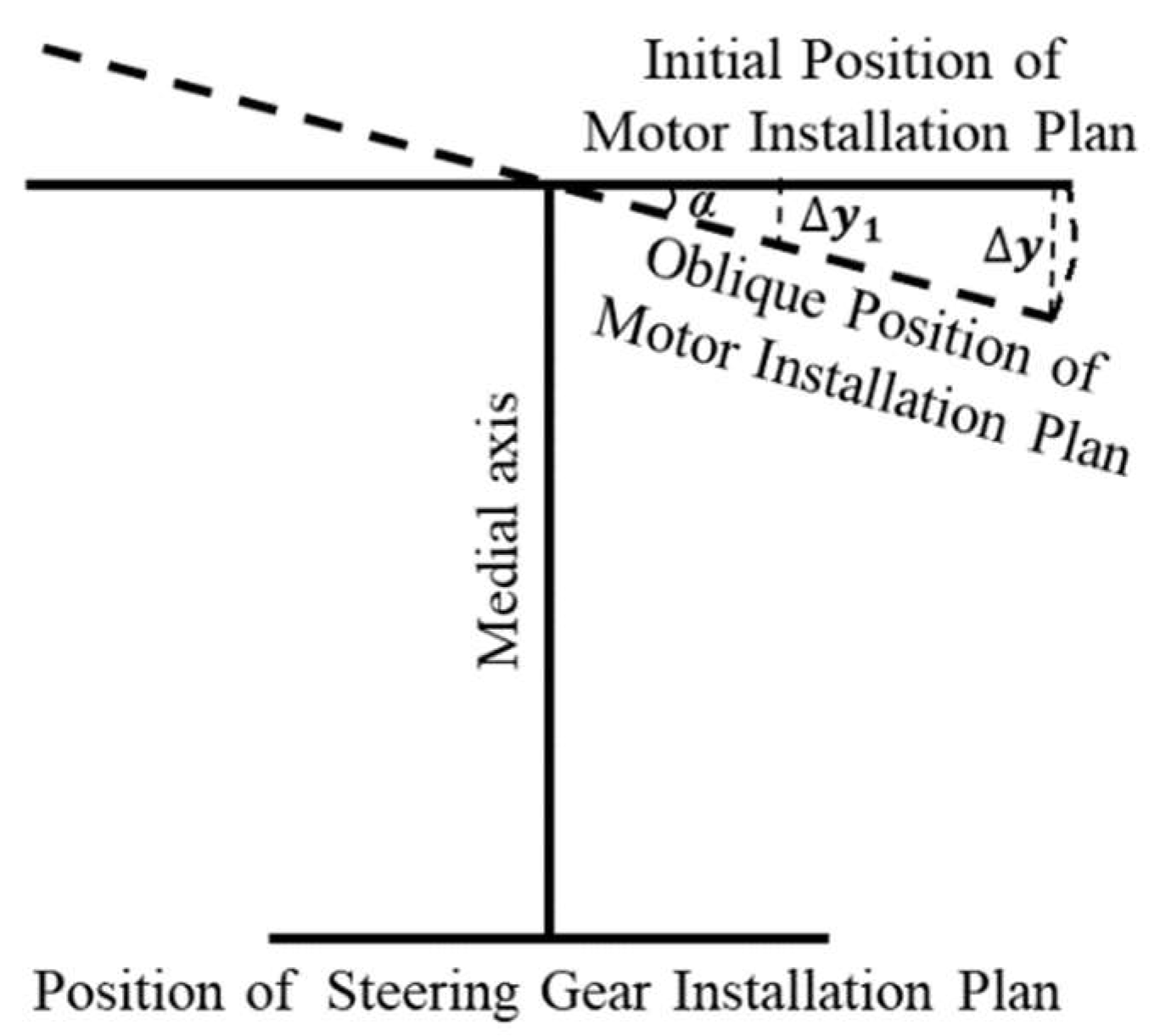
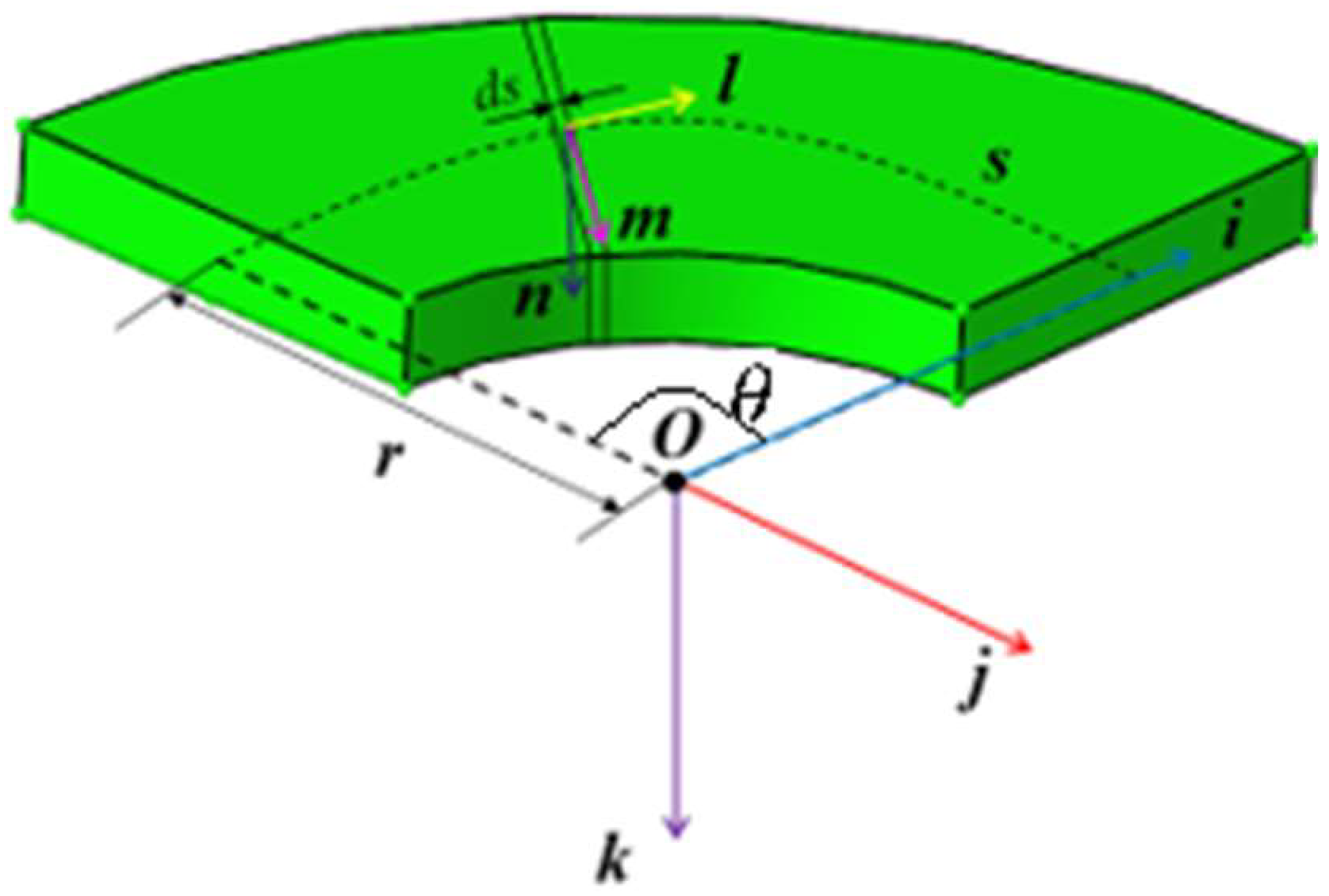
2.2. Theoretical Analysis
- Deformation occurs mostly in the flexible part of the core flexure, and deformation outside this part is ignored;
- The interferences between different gaps are neglected;
- The applied loads can be transferred 100%.
3. Numerical Analysis
3.1. Static Analysis
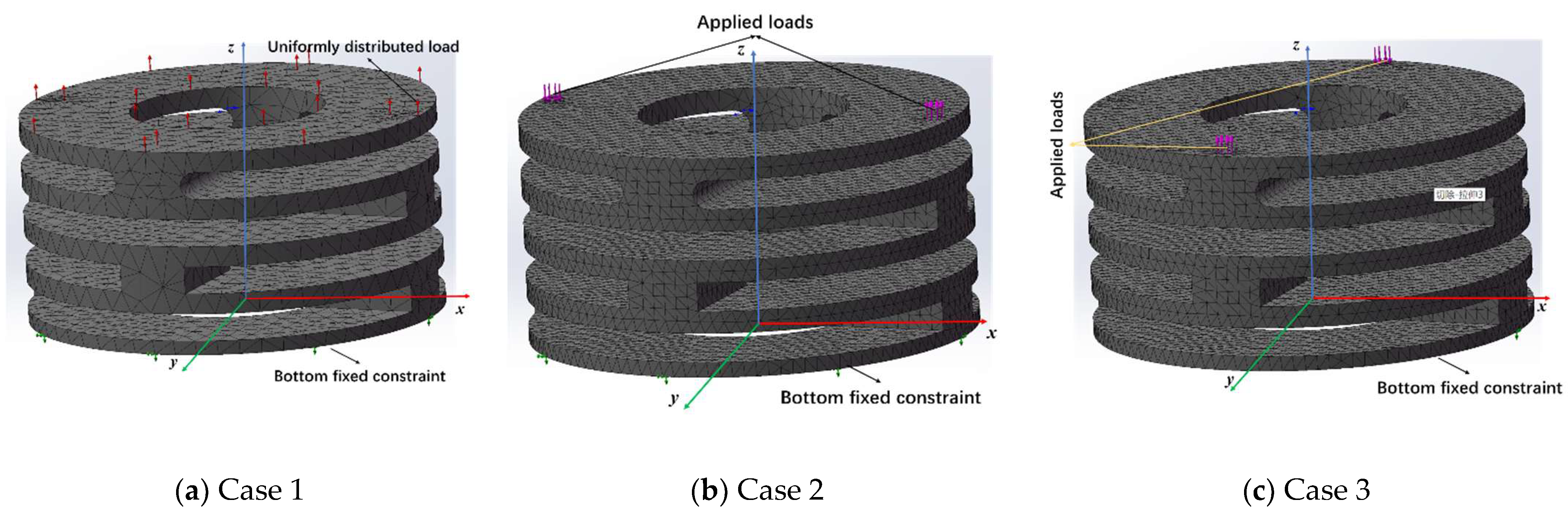
- The stress-distribution characteristics of the lift on the IAAM were analyzed by applying uniform tension on the upper surface of the finite element model. The boundary conditions and applied loads are shown in Figure 8a.
- An upward tension was applied to one end of the finite element model in the x-direction, and a downward pressure was applied to the symmetrical position to analyze the characteristics of tilting state 1 of the IAAM. The boundary conditions and applied loads are shown in Figure 8b.
- An upward tension was applied to one end of the finite element model in the y-direction, and a downward pressure was applied to the symmetrical position to analyze the characteristics of tilting state 1. The boundary conditions and applied loads are shown in Figure 8c.
3.2. Modal Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wang, J.; Zhou, Y. Micro Air Vehicle Visual Navigation Based on Principal Component Analysis. J. Acta Aeronaut. Astronaut. Sin. 2008, 5, S220–S223. [Google Scholar]
- Shen, J.; Chopra, I. A Parametric Design Study for a Swashplateless Helicopter Rotor with Trailing-Edge Flaps. J. Am. Helicopter Soc. 2004, 49, 43–53. [Google Scholar] [CrossRef]
- Paulos, J.; Yim, M. Flight Performance of A Swashplateless Micro Air Vehicle; IEEE International Conference on Robotics & Automation: Washington, DC, USA, 2015; pp. 5284–5289. [Google Scholar]
- Ganguli, R.; Jehnert, B.; Wolfram, J. Optimal location of centre of gravity for swashplateless helicopter UAV and MAV. J. Aircr. Eng. Aerosp. Technol. 2007, 79, 335–345. [Google Scholar] [CrossRef]
- Saxena, A.; Chopra, I. Design, Development, and Hover Testing of a Mach-Scale Swashplateless Rotor Using Brushless DC Motor-Actuated Trailing Edge Flaps for Rotor Primary Control. J. Am. Helicopter Soc. 2017, 62, 1–12. [Google Scholar] [CrossRef]
- Wang, J.; Wang, H.; Wu, C. Development of swashplateless helicopter blade pitch control system using the limited angle direct-drive motor (LADDM). Chin. J. Aeronaut. 2015, 28, 1416–1425. [Google Scholar] [CrossRef][Green Version]
- Howell, L.L. Compliant Mechanisms; John Wiley & Sons: New York, NY, USA, 2001. [Google Scholar]
- Howell, L.L.; Magleby, S.; Olsen, B. Handbook of Compliant Mechanisms; Wiley: New York, NY, USA, 2013. [Google Scholar]
- Seddon, J.; Newman, S. Basic Helicopter Aerodynamics; John Wiley & Sons: New York, NY, USA, 2011. [Google Scholar]
- Yu, J.; Bi, S.; Pei, X. Flexure Design: Analysis and Synthesis of Compliant Mechanism; Higher Education Press: Beijing, China, 2018. [Google Scholar]
- Mahjoob, M.J. An Exact Three-Dimensional Beam Element with Nonuniform Cross Section. J. Appl. Mech. 2010, 77, 3214–3220. [Google Scholar]
- Blake, A.; Epstein, H.I. Handbook of Mechanics, Materials, and Structures; Wiley Series in Mechanical Engineering Practice: Hoboken, NJ, USA, 1985. [Google Scholar]
- Yang, J.M.; Zeng, L.Z.; Xiong, C.H. Bending of cantilever beams of variable cross section under arbitrary loads. J. Nanchang Inst. Aeronaut. Technol. 1999, 13, 31–36. [Google Scholar]



| 1120 | 2620 | 103.65 | 0.3 1 |
| Parameters of the Cross-Section/mm | The Loads on the Free Node | Displacements on the Free Node by FEA | Displacements on the Free Node by Theory Derive | Errors/% |
|---|---|---|---|---|
| r = 6.65, t = 1.0, w = 5.3 | 0 0 8 0 0 0 | 2.1 mm | 1.9 mm | 9.52 |
| r = 9.50, t = 1.0, w = 1.0 | 0 0 1 0 0 0 | 4.98 mm | 4.6 mm | 7.63 |
| r = 19.5, t = 1.0, w = 1.0 | 0 0 1 0 0 0 | 42.7 mm | 39.3 mm | 7.96 |
| Case1 | Case2 | Case3 | |
|---|---|---|---|
| Displacement distribution | 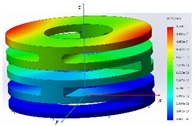 |  |  |
| Maximum displacement/mm | 0.13 | 3.36 | 3.37 |
| Tilted angle/deg | -- | 19.63 | 19.67 |
| Stress distribution |  | 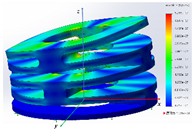 | 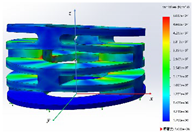 |
| Max normal stress/MPa | 3.55 | 52.9 | 50.87 |
| FOS | 29.18 | 1.96 | 2.04 |
| Orders | 1 | 2 | 3 |
|---|---|---|---|
| Vibration mode | 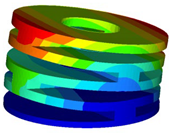 |  |  |
| Natural frequency/Hz | 901 | 963 | 1576 |
| Orders | 1 | 2 | 3 |
|---|---|---|---|
| Frequency/Hz | 901 | 963 | 1576 |
| Corresponding Motor Speed/R/min | 54,060 | 57,780 | 94,560 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Luo, J. Design and Analysis of the Attitude Adjustment Mechanism for a Rotor MAV Based on Compliant Mechanisms. Aerospace 2021, 8, 319. https://doi.org/10.3390/aerospace8110319
Zhang J, Luo J. Design and Analysis of the Attitude Adjustment Mechanism for a Rotor MAV Based on Compliant Mechanisms. Aerospace. 2021; 8(11):319. https://doi.org/10.3390/aerospace8110319
Chicago/Turabian StyleZhang, Jingjing, and Jinglin Luo. 2021. "Design and Analysis of the Attitude Adjustment Mechanism for a Rotor MAV Based on Compliant Mechanisms" Aerospace 8, no. 11: 319. https://doi.org/10.3390/aerospace8110319
APA StyleZhang, J., & Luo, J. (2021). Design and Analysis of the Attitude Adjustment Mechanism for a Rotor MAV Based on Compliant Mechanisms. Aerospace, 8(11), 319. https://doi.org/10.3390/aerospace8110319






